No CrossRef data available.
Article contents
Convergence criteria for Fourier series
Published online by Cambridge University Press: 17 April 2009
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
The following convergence criterion of Fourier series is due to M. Izumi, S. Izumi and the author:
THEOREM. Let Δ ≥ 1. If
(i) 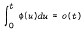 , and
, and
(ii)  as t → 0
as t → 0
for an a, 0 < a < 1 and for a δ, 0 < δ < π, then the Fourier series of φ(t) is convergent at the origin.
The object of this paper is to generalize the above theorem in the Hardy-Littlewood direction.
- Type
- Research Article
- Information
- Copyright
- Copyright © Australian Mathematical Society 1971
References
[1]Hardy, G.H. and Littlewood, J.E., “Notes on the theory of series (VII): On Young's convergence criterion for Fourier series”, Proc. London Math. Soc. (2) 28 (1928), 301–311.CrossRefGoogle Scholar
[2]Izumi, Masako, Izumi, Shin-ichi and Gopal Rao, V.V., “On the convergence criteria of Fourier series”, Proc. Japan Acad. (to appear).Google Scholar
[3]Pollard, S., “On the criteria for the convergence of a Fourier series”, J. London Math. Soc. 2 (1927), 255–262.CrossRefGoogle Scholar
[4]Sunouchi, Gen-ichirô, “Notes on Fourier analysis. XLVI. A convergence criterion for Fourier series”, Tôhoku Math. J. (2) 3 (1951), 216–219.Google Scholar


