Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Kartsatos, A.G
and
Toro, J
1978.
Comparison and oscillation theorems for equations with middle terms of order n−1.
Journal of Mathematical Analysis and Applications,
Vol. 66,
Issue. 2,
p.
297.
Sficas, Y.G.
1979.
On the behavior of nonoscillatory solutions of differential equations with deviating argument.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 3,
Issue. 3,
p.
379.
Foster, K.E
and
Grimmer, R.C
1980.
Nonoscillatory solutions of higher order delay equations.
Journal of Mathematical Analysis and Applications,
Vol. 77,
Issue. 1,
p.
150.
Kawano, Nichiro
Kusano, Takaŝi
and
Naito, Manabu
1982.
Nonoscillatory solutions of forced differential equations of the second order.
Journal of Mathematical Analysis and Applications,
Vol. 90,
Issue. 2,
p.
323.
Kosmala, Witold A
1985.
Comparison results for functional differential equations with two middle terms.
Journal of Mathematical Analysis and Applications,
Vol. 111,
Issue. 1,
p.
243.
Grace, S.R
and
Lalli, B.S
1986.
A comparison theorem for general nonlinear ordinary differential equations.
Journal of Mathematical Analysis and Applications,
Vol. 120,
Issue. 1,
p.
39.
Wong, James S. W.
1988.
Second Order Nonlinear Forced Oscillations.
SIAM Journal on Mathematical Analysis,
Vol. 19,
Issue. 3,
p.
667.
Grace, S.R
1989.
Comparison theorems for forced functional differential equations.
Journal of Mathematical Analysis and Applications,
Vol. 144,
Issue. 1,
p.
168.
Du, Michael S
and
Kwong, Man Kam
1990.
Sturm comparison theorems for second-order delay equations.
Journal of Mathematical Analysis and Applications,
Vol. 152,
Issue. 2,
p.
305.
Grace, S.R
1992.
Oscillatory and asymptotic behavior of delay differential equations with a nonlinear damping term.
Journal of Mathematical Analysis and Applications,
Vol. 168,
Issue. 2,
p.
306.
Gong Sun, Yuan
and
Wong, James S. W.
2005.
Forced oscillation of second order superlinear differential equations.
Mathematische Nachrichten,
Vol. 278,
Issue. 12-13,
p.
1621.
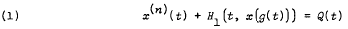
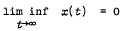 for n odd, then this is also the case for the equation
for n odd, then this is also the case for the equation