Article contents
BINDING NUMBER AND MINIMUM DEGREE FOR FRACTIONAL (k,m)-DELETED GRAPHS
Published online by Cambridge University Press: 14 October 2011
Abstract
Let G be a graph of order n, and let k≥1 be an integer. Let h:E(G)→[0,1] be a function. If ∑ e∋xh(e)=k holds for any x∈V (G), then we call G[Fh] a fractional k-factor of G with indicator function h where Fh ={e∈E(G):h(e)>0}. A graph G is called a fractional (k,m) -deleted graph if for every e∈E(H) , there exists a fractional k-factor G[Fh ] of G with indicator function h such that h(e)=0 , where H is any subgraph of G with m edges. The minimum degree of a vertex in G is denoted by δ(G) . For X⊆V (G), NG(X)=⋃ x∈XNG(x) . The binding number of G is defined by 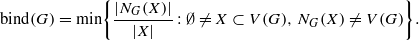 In this paper, it is proved that if
In this paper, it is proved that if  then G is a fractional (k,m) -deleted graph. Furthermore, it is shown that this result is best possible in some sense.
then G is a fractional (k,m) -deleted graph. Furthermore, it is shown that this result is best possible in some sense.
MSC classification
- Type
- Research Article
- Information
- Copyright
- Copyright © Australian Mathematical Publishing Association Inc. 2011
Footnotes
This research was supported by Natural Science Foundation of the Higher Education Institutions of Jiangsu Province (10KJB110003) and Jiangsu University of Science and Technology (2010SL101J, 2009SL154J), and was sponsored by Qing Lan Project of Jiangsu Province.
References
- 2
- Cited by


