Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Pasupathi, R.
Navascués, M. A.
and
Chand, A. K. B.
2022.
Fractal Convolution on the Rectangle.
Complex Analysis and Operator Theory,
Vol. 16,
Issue. 4,
Chandra, Subhash
and
Abbas, Syed
2022.
Fractal dimensions of mixed Katugampola fractional integral associated with vector valued functions.
Chaos, Solitons & Fractals,
Vol. 164,
Issue. ,
p.
112648.
CHANDRA, SUBHASH
and
ABBAS, SYED
2022.
ON FRACTAL DIMENSIONS OF FRACTAL FUNCTIONS USING FUNCTION SPACES.
Bulletin of the Australian Mathematical Society,
Vol. 106,
Issue. 3,
p.
470.
Attia, Najmeddine
and
Jebali, Hajer
2023.
On the construction of recurrent fractal interpolation functions using Geraghty contractions.
Electronic Research Archive,
Vol. 31,
Issue. 11,
p.
6866.
Barnsley, Michael F.
and
Viswanathan, P.
2023.
Histopolating fractal functions.
Journal of Computational and Applied Mathematics,
Vol. 425,
Issue. ,
p.
115073.
Agrawal, Vishal
Pandey, Megha
and
Som, Tanmoy
2023.
Box Dimension and Fractional Integrals of Multivariate $$\alpha $$-Fractal Functions.
Mediterranean Journal of Mathematics,
Vol. 20,
Issue. 3,
Mondal, Anarul Islam
and
Jha, Sangita
2024.
Non-stationary α-fractal functions and their dimensions in various function spaces.
Indagationes Mathematicae,
Vol. 35,
Issue. 1,
p.
159.
JIANG, LAI
2024.
BOX DIMENSION OF FRACTAL INTERPOLATION SURFACES WITH VERTICAL SCALING FUNCTION.
Fractals,
Vol. 32,
Issue. 03,
YU, BINYAN
and
LIANG, YONGSHUN
2024.
RESEARCH ON FRACTAL DIMENSIONS AND THE HÖLDER CONTINUITY OF FRACTAL FUNCTIONS UNDER OPERATIONS.
Fractals,
Vol. 32,
Issue. 03,
RUAN, HUO-JUN
and
XIAO, JIAN-CI
2024.
CORRECTION TO ‘EXISTENCE AND BOX DIMENSION OF GENERAL RECURRENT FRACTAL INTERPOLATION FUNCTIONS’.
Bulletin of the Australian Mathematical Society,
Vol. 109,
Issue. 1,
p.
174.
Attia, Najmeddine
and
Jebali, Hajer
2024.
On the box dimension of recurrent fractal interpolation functions defined with Matkowski contractions.
The Journal of Analysis,
Vol. 32,
Issue. 6,
p.
3453.
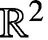 $\mathbb {R}^2$. We generalise the definition of RFIFs so that iterated functions in the recurrent system need not be contractive with respect to the first variable. We obtain the box dimensions of all self-affine RFIFs in this general setting.
$\mathbb {R}^2$. We generalise the definition of RFIFs so that iterated functions in the recurrent system need not be contractive with respect to the first variable. We obtain the box dimensions of all self-affine RFIFs in this general setting.


