Article contents
GOODSTEIN SEQUENCES BASED ON A PARAMETRIZED ACKERMANN–PÉTER FUNCTION
Published online by Cambridge University Press: 02 July 2021
Abstract
Following our [6], though with somewhat different methods here, further variants of Goodstein sequences are introduced in terms of parameterized Ackermann–Péter functions. Each of the sequences is shown to terminate, and the proof-theoretic strengths of these facts are calibrated by means of ordinal assignments, yielding independence results for a range of theories: PRA, PA, 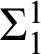 $\Sigma ^1_1$
-DC
$\Sigma ^1_1$
-DC $_0$
, ATR
$_0$
, ATR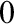 $_0$
, up to ID
$_0$
, up to ID $_1$
. The key is the so-called “Hardy hierarchy” of proof-theoretic bounding finctions, providing a uniform method for associating Goodstein-type sequences with parameterized normal form representations of positive integers.
$_1$
. The key is the so-called “Hardy hierarchy” of proof-theoretic bounding finctions, providing a uniform method for associating Goodstein-type sequences with parameterized normal form representations of positive integers.
- Type
- Articles
- Information
- Copyright
- © The Association for Symbolic Logic 2021
References
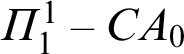 ${\varPi}_1^1-{\mathsf{\mathit{CA}}}_0$
. Annals of Pure and Applied Logic, vol. 164 (2013), pp. 1493–1506.Google Scholar
${\varPi}_1^1-{\mathsf{\mathit{CA}}}_0$
. Annals of Pure and Applied Logic, vol. 164 (2013), pp. 1493–1506.Google Scholar- 2
- Cited by



