The object of our study is

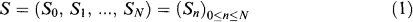
where each Sn is a m-dimensional stochastic (real valued) vector, i.e.

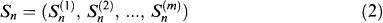
denned on a probability space (Ω, 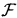, P) and adapted to a filtration (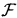n)0≤n≤N with 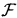0 being the σ-algebra consisting of all null sets and their complements. In this paper we interpret 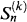as the value of some financial asset k at time n.

Remark: If the asset generates dividends or coupon payments, think of 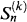as to include these payments (cum dividend process). Think of dividends as being reinvested immediately at the ex-dividend price.

Definition 1

(a) A sequence of random vectors

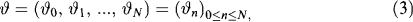
where

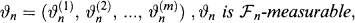
is called a trading strategy. Since our time horizon ends at time N we must always have ϑN ≡ 0.

The interpretation is obvious: 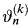stands for the number of shares of asset k you hold in the time interval [n,n + 1). You must choose ϑn at time n.

(b) The sequence of random variables

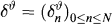
where 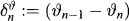Sn stands for the payment stream generated by ϑ (set ϑ−1 ≡ 0).

This (mostly) expository paper describes the importance of hedging to the pricing of modern financial products and how hedging may be achieved even when the traditional Black-Scholes assumptions are absent.

In performing Bayesian analysis of insurance losses, one usually chooses a parametric conditional loss distribution for each risk and a parametric prior distribution to describe how the conditional distributions vary across the risks. Young (1997) applies techniques from nonparametric density estimation to estimate the prior and uses the estimated model to calculate the predictive mean of future claims given past claims. A shortcoming of this method is that, in estimating the prior, one assumes the average claim amount equals the conditional claim. In this paper, we consider a class of priors obtained by perturbing the one determined nonparametrically, as in Young (1997). We thereby reflect the uncertainty in the prior that arises from the randomness in the claim data. We, then, calculate intervals for the corresponding predictive means. We illustrate our method with data from Dannenburg et al. (1996) and compare the intervals of the predictive means with nonparametric confidence intervals.

A unit-linked life insurance contract is a contract where the insurance benefits depend on the price of some specific traded stocks. We consider a model describing the uncertainty of the financial market and a portfolio of insured individuals simultaneously. Due to incompleteness the insurance claims cannot be hedged completely by trading stocks and bonds only, leaving some risk to the insurer. The theory of risk-minimization is briefly reviewed and applied after a change of measure. Risk-minimizing trading strategies and the associated intrinsic risk processes are determined for different types of unit-linked contracts. By extending the model to the situation where certain reinsurance contracts on the insured lives are traded, the direct insurer can eliminate the risk completely. The corresponding self-financing strategies are determined.

This paper provides bonus-malus systems which rest on different types of claims. Consistent estimators are given for some moments of the mixing distribution of a multi equation Poisson model with random effects. Bonus-malus coefficients are then obtained with the expected value principle, and from linear credibility predictors. Empirical results are presented for two types of claims, namely claims at fault and not at fault with respect to a third party.

In this paper we compare, from the point of view of reinsurance, the several risk adjusted premium calculation principles considered in Wang (1996b). We conclude that, with the exception of the proportional hazard (PH) premium calculation principle, all the others behave in a way similar to the expected value principle. We prove that the stop loss reinsurance premium when calculated using the PH premium principle gives a higher premium than any of the other transforms, provided that the priority is big enough. We observe a similar behaviour with respect to excess of loss reinsurance in all the examples given.

We also study the behaviour of the adjustment coefficient, both from the insurer's and the reinsurer's point of view as functions of the priority, when the PH principle is used as opposed to the expected value principle.

This article presents an explicit formula for the value of a withdrawal benefit when the times of death and withdrawal are dependent. The derivation is based on an actuarial equivalence principle. As a special case, we show that in the fully continuous case, the withdrawal benefit is the reserve when the decrements are independent. We also present a definition of antiselection and prove that the withdrawal benefit will be smaller under antiselection.

In this paper we investigate multivariate risk portfolios, where the risks are dependent. By providing some natural models for risk portfolios with the same marginal distributions we are able to compare two portfolios with different dependence structure with respect to their stop-loss premiums. In particular, some comparison results for portfolios with two-point distributions are obtained. The analysis is based on the concept of the so-called supermodular ordering. We also give some numerical results which indicate that dependencies in risk portfolios can have a severe impact on the stop-loss premium. In fact, we show that the effect of dependencies can grow beyond any given bound.

The Kolmogorov distance is used to transform arithmetic severities into equispaced arithmetic severities in order to reduce the number of calculations when using algorithms like Panjer's formulae for compound distributions. An upper bound is given for the Kolmogorov distance between the true compound distribution and the transformed one. Advantages of the Kolmogorov distance and disadvantages of the total variation distance are discussed. When the bounds are too big, a Berry-Esseen result can be used. Then almost every case can be handled by the techniques described in this paper. Numerical examples show the interest of the methods.

This paper presents a continuous time version of a stochastic investment model originally due to Wilkie. The model is constructed via stochastic differential equations. Explicit distributions are obtained in the case where the SDEs are driven by Brownian motion, which is the continuous time analogue of the time series with white noise residuals considered by Wilkie. In addition, the cases where the driving “noise” are stable processes and Gamma processes are considered.

Largest claims reinsurance covers are reconsidered. Allowing the original claims sizes to be not necessarily independent, a new, upper premium bound is derived and explored.

The Cox regression model is a standard tool in survival analysis for studying the dependence of a hazard rate on covariates (parametrically) and time (nonparametrically). This paper is a case study intended to indicate possible applications to non-life insurance, particularly occurrence of claims and rating.

We studied individuals from one Danish county holding policies in auto, property and household insurance simultaneously at some point during the four year period 1988-1991 in one company. The hazard of occurrence of claims of each type was studied as function of calendar time, time since the last claim of each type, age of policy holder, urbanization and detailed type of insurance. Particular emphasis was given to the technical advantages and disadvantages (particularly the complicated censoring patterns) of considering the nonparametrically underlying time as either calendar time or time since last claim. In the former case the theory is settled, but the results are somewhat complicated. The latter choice leads to several issues still under active methodological development. We develop a goodness-of-fit criterion which shows the lack of fit of some models, for which the practical conclusions might otherwise have been useful.

Bühlmann-Straub credibility is used to find an estimate of the mortality loss ratio for a company, relative to a standard table, for use in the statutory valuation of life insurance business. A method for calculating the margin for adverse deviation to be added to the mortality rate (in accordance with the general principle of Canadian statutory valuation) is derived. Applying credibility further to the variance of the mortality loss ratio gives a methodology for calculating the amount of the surplus (i.e. capital) required to cover annual fluctuations in mortality experience. The necessary structural parameters are calculated from industry statistics; the methodology is illustrated using Canadian life insurance data.

A number of more or less well-known, but quite complex, characterizations of stop-loss order are reviewed and proved in an elementary way. Two recent proofs of the stop-loss order preserving property for the distortion pricing principle are invalidated through a simple counterexample. A new proof is presented. It is based on the important Hardy-Littlewood transform, which is known to characterize the stop-loss order by reduction to the usual stochastic order, and the dangerousness characterization of stop-loss order under a finite crossing condition. Finally, we complete and summarize the main properties of the distortion pricing principle.

The generalized Poisson distribution with parameters θ and λ was introduced by Consul and Jain (1973) and has recently found several instances of application in the actuarial literature. The most frequently used version of the distribution assumes that θ > 0 and 0 ≤ λ < 1, in which case the mean and variance are θ/(1 − λ) and θ/(1 − λ)3, respectively. These simple moment expressions, along with nearly all of the other theoretical results available for this distribution, fail when λ < 0 or λ > 1 (e.g., Johnson, Kotz, and Kemp, 1992, page 397). In these cases, even the definition of the probability mass function usually given in the literature is not properly normalized so that its values do not sum to unity. For this reason, it is common to truncate the support of the distribution and explicitly normalize the probability mass function. In this paper we discuss the estimation of the parameters of this truncated generalized Poisson distribution using a Bayesian method.