Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-29T06:16:12.594Z Has data issue: false hasContentIssue false
  • English
  • Français

On the total length of external branches for beta-coalescents

Part of: Markov processes

Published online by Cambridge University Press:  21 March 2016

Jean-Stéphane Dhersin  and
Linglong Yuan
Jean-Stéphane Dhersin*
Affiliation:
Université Paris 13
Linglong Yuan*
Affiliation:
Université Paris 13
*
∗ Postal address: Université Paris 13, Sorbonne Paris Cité, LAGA, CNRS, UMR 7539, F-93430, Villetaneuse, France. Email address: [email protected]
∗∗ Current address: Department of Mathematics, Uppsala University, PO Box 480, SE-751 06, Uppsala, Sweden. Email address: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this paper we consider the beta(2 − α, α)-coalescents with 1 < α < 2 and study the moments of external branches, in particular, the total external branch length 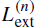of an initial sample of n individuals. For this class of coalescents, it has been proved that nα-1T(n) →DT, where T(n) is the length of an external branch chosen at random and T is a known nonnegative random variable. For beta(2 − α, α)-coalescents with 1 < α < 2, we obtain limn→+∞n3α-5 𝔼(Lext(n) − n2-α𝔼T)2 = ((α − 1)Γ(α + 1))2Γ(4 − α) / ((3 − α)Γ(4 − 2α)).

Keywords

Coalescent processbeta-coalescenttotal external branch lengthFu and Li's statistical test

MSC classification

Primary: 60J28: Applications of continuous-time Markov processes on discrete state spaces 92D25: Population dynamics (general)
Secondary: 60J25: Continuous-time Markov processes on general state spaces 60J85: Applications of branching processes
Type
General Applied Probability
Information
Advances in Applied Probability , Volume 47 , Issue 3 , September 2015 , pp. 693 - 714
Copyright
Copyright © Applied Probability Trust 2015 

References

Árnason, E. (2004). Mitochondrial cytochrome b DNA variation in the high-fecundity Atlantic cod: Trans-Atlantic clines and shallow gene genealogy. Genetics 166, 1871–1885.Google Scholar
Berestycki, J., Berestycki, N. and Limic, V. (2014). A small-time coupling between Λ-coalescents and branching processes. Ann. Appl. Prob. 24, 449–475.Google Scholar
Berestycki, J., Berestycki, N. and Limic, V. (2014). Asymptotic sampling formulae for Λ-coalescents. Ann. Inst. H. Poincaré Prob. Statist. 50, 715–731.Google Scholar
Berestycki, J., Berestycki, N. and Schweinsberg, J. (2007). Beta-coalescents and continuous stable random trees. Ann. Prob. 35, 1835–1887.CrossRefGoogle Scholar
Berestycki, N. (2009). Recent Progress in Coalescent Theory (Ensaios Matemáticos 16). Sociedade Brasileira de Mathemática, Rio de Janeiro.Google Scholar
Birkner, M. et al. (2005). Alpha-stable branching and beta-coalescents. Electron. J. Prob. 10, 303–325.Google Scholar
Blum, M. G. B. and François, O. (2005). Minimal clade size and external branch length under the neutral coalescent. Adv. Appl. Prob. 37, 647–662.Google Scholar
Bolthausen, E. and Sznitman, A.-S. (1998). On Ruelle's probability cascades and an abstract cavity method. Commun. Math. Phys. 197, 247–276.CrossRefGoogle Scholar
Boom, J. D. G., Boulding, E. G. and Beckenbach, A. T. (1994). Mitochondrial DNA variation in introduced populations of Pacific Oyster, Crassostrea gigas, in British Columbia. Canad. J. Fisheries Aquatic Sci. 51, 1608–1614.Google Scholar
Bovier, A. and Kurkova, I. (2007). Much ado about Derrida's GREM. In Spin Glasses (Lecture Notes Math. 1900), Springer, Berlin, pp. 81–115.Google Scholar
Breiman, L. (1992). Probability (Classics Appl. Math. 7). SIAM, Philadelphia, PA.Google Scholar
Caliebe, A., Neininger, R., Krawczak, M. and Rösler, U. (2007). On the length distribution of external branches in coalescence trees: genetic diversity within species. Theoret. Pop. Biol. 72, 245–252.CrossRefGoogle ScholarPubMed
Delmas, J.-F., Dhersin, J.-S. and Siri-Jégousse, A. (2008). Asymptotic results on the length of coalescent trees. Ann. Appl. Prob. 18, 997–1025.CrossRefGoogle Scholar
Dhersin, J.-S. and Möhle, M. (2013). On the external branches of coalescents with multiple collisions. Electron. J. Prob. 18, 11pp.Google Scholar
Dhersin, J.-S. and Yuan, L. (2012). Asympotic behavior of the total length of external branches for beta-coalescents. Preprint. Available at http://arxiv.org/abs/1202.5859.Google Scholar
Dhersin, J.-S., Freund, F., Siri-Jégousse, A. and Yuan, L. (2013). On the length of an external branch in the beta-coalescent. Stoch. Process. Appl. 123, 1691–1715.CrossRefGoogle Scholar
Drmota, M., Iksanov, A., Moehle, M. and Roesler, U. (2007). Asymptotic results concerning the total branch length of the Bolthausen–Sznitman coalescent. Stoch. Process. Appl. 117, 1404–1421.Google Scholar
Durrett, R. (2008). Probability Models for DNA Sequence Evolution, 2nd edn. Springer, New York.Google Scholar
Eldon, B. and Wakeley, J. (2006). Coalescent processes when the distribution of offspring number among individuals is highly skewed. Genetics 172, 2621–2633.Google Scholar
Foucart, C. and Hénard, O. (2013). Stable continuous-state branching processes with immigration and beta–Fleming–Viot processes with immigration. Electron. J. Prob. 18, 21pp.Google Scholar
Freund, F. and Möhle, M. (2009). On the time back to the most recent common ancestor and the external branch length of the Bolthausen–Sznitman coalescent. Markov Process. Relat. Fields 15, 387–416.Google Scholar
Fu, Y. X. and Li, W. H. (1993). Statistical tests of neutrality of mutations. Genetics 133, 693–709.Google Scholar
Gnedin, A. and Yakubovich, Y. (2007). On the number of collisions in Λ-coalescents. Electron. J. Prob. 12, 1547–1567.CrossRefGoogle Scholar
Gnedin, A., Iksanov, A. and Möhle, M. (2008). On asymptotics of exchangeable coalescents with multiple collisions. J. Appl. Prob. 45, 1186–1195.Google Scholar
Goldschmidt, C. and Martin, J. B. (2005). Random recursive trees and the Bolthausen–Sznitman coalescent. Electron. J. Prob. 10, 718–745.Google Scholar
Hedgecock, D. (1994). Does variance in reproductive success limit effective population sizes of marine organisms? In Genetics and Evolution of Aquatic Organisms, Chapman & Hall, London, pp. 122–134.Google Scholar
Janson, S. and Kersting, G. (2011). On the total external length of the Kingman coalescent. Electron. J. Prob. 16, 2203–2218.Google Scholar
Kallenberg, O. (2002). Foundations of Modern Probability, 2nd edn. Springer, New York.Google Scholar
Kersting, G. (2012). The asymptotic distribution of the length of beta-coalescent trees. Ann. Appl. Prob. 22, 2086–2107.CrossRefGoogle Scholar
Kersting, G., Pardo, J. C. and Siri-Jégousse, A. (2014). Total internal and external lengths of the Bolthausen–Sznitman coalescent. In Celebrating 50 years of the Applied Probability Trust (J. Appl. Prob. Spec. Vol. 51A), Applied Probability Trust, Sheffield, pp. 73–86.Google Scholar
Kersting, G., Stanciu, I. and Wakolbinger, A. (2014). The total external branch length of beta-coalescents. Combin. Prob. Comput. 23, 1010–1027 Google Scholar
Kimura, M. (1969). The number of heterozygous nucleotide sites maintained in a finite population due to steady flux of mutations. Genetics 61, 893–903.CrossRefGoogle Scholar
Kingman, J. F. C. (1982). The coalescent. Stoch. Process. Appl. 13, 235–248.Google Scholar
Kingman, J. F. C. (2000). Origins of the coalescent: 1974–1982. Genetics 156, 1461–1463.Google Scholar
Marynych, O. (2011). Stochastic recurrences and their applications to the analysis of partition-valued processes. Doctoral Thesis, Utrecht University.Google Scholar
Möhle, M. (2006). On the number of segregating sites for populations with large family sizes. Adv. Appl. Prob. 38, 750–767.CrossRefGoogle Scholar
Möhle, M. (2010). Asymptotic results for coalescent processes without proper frequencies and applications to the two-parameter Poisson–Dirichlet coalescent. Stoch. Process. Appl. 120, 2159–2173.Google Scholar
Pitman, J. (1999). Coalescents with multiple collisions. Ann. Prob. 27, 1870–1902.Google Scholar
Sagitov, S. (1999). The general coalescent with asynchronous mergers of ancestral lines. J. Appl. Prob. 36, 1116–1125.CrossRefGoogle Scholar
Schweinsberg, J. (2003). Coalescent processes obtained from supercritical Galton–Watson processes. Stoch. Process. Appl. 106, 107–139.Google Scholar
Yuan, L. (2014). On the measure division construction of Λ-coalescents. Markov Process. Relat. Fields 20, 229–264.Google Scholar