Skip to main content Accessibility help

We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.

Close cookie message
×
Hostname: page-component-78c5997874-j824f Total loading time: 0 Render date: 2024-11-13T06:23:43.009Z Has data issue: false hasContentIssue false

18 - Mixed valence, fluctuations, and topology

Published online by Cambridge University Press:  05 December 2015

Piers Coleman
Piers Coleman
Affiliation:
Rutgers University, New Jersey
Get access

Summary

A summary is not available for this content so a preview has been provided. Please use the Get access link above for information on how to access this content.
Image of the first page of this content. For PDF version, please use the ‘Save PDF’ preceeding this image.'
Type
Chapter
Information
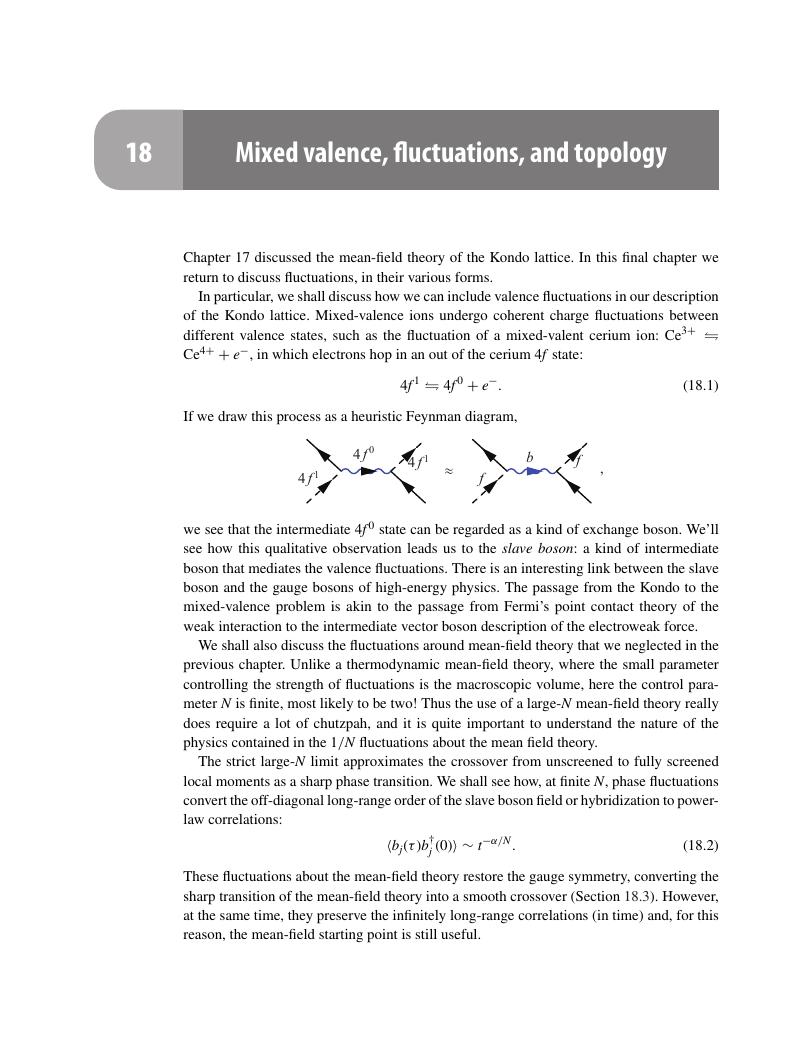
Introduction to Many-Body Physics , pp. 720 - 786
Publisher: Cambridge University Press
Print publication year: 2015

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1] P., Coleman, New approach to the mixed-valence problem, Phys. Rev. B, vol. 29, p. 3035, 1984.Google Scholar
[2] S. E., Barnes, New method for the Anderson model, J. Phys. F, vol. 6, p. 1375, 1976.Google Scholar
[3] N., Read and D. M., Newns, A new functional integral formalism for the degenerate Anderson model, J. Phys. C, vol. 29, p. L105, 1983.Google Scholar
[4] A. J., Millis and P. A., Lee, Large-orbital-degeneracy expansion for the lattice Anderson model, Phys. Rev. B, vol. 35, no. 7, p. 3394, 1987.Google Scholar
[5] M. C., Gutzwiller, Effect of correlation on the ferromagnetism of transition metals, Phys. Rev. Lett., vol. 10, no. 5, p. 159, 1963. The Hubbard model was written down independently by Gutzwiller in equation (11) of this paper.Google Scholar
[6] F. D. M., Haldane, Scaling theory of the asymmetric Anderson model, Phys. Rev. Lett., vol. 40, p. 416, 1978.Google Scholar
[7] P. W., Anderson, Infrared catastrophe in Fermi gases with local scattering potentials, Phys. Rev. Lett., vol. 18, p. 1049, 1967.Google Scholar
[8] S., Elitzur, Impossibility of spontaneously breaking local symmetries, Phys. Rev. D, vol. 12, p. 3978, 1975.Google Scholar
[9] P., Coleman, Large-N as a classical limit (1/N ˜ ħ) of mixed valence, J. Magn. Magn. Mater., vol. 47–48, p. 323, 1985.Google Scholar
[10] P., Coleman and N., Andrei, Kondo-stabilized spin liquids and heavy-fermion superconductivity, J. Phys.: Condens. Matter., vol. 1, p. 4057, 1989.Google Scholar
[11] N., Read, Role of infrared divergences in the 1/N expansion of the U =8Anderson model, J. Phys. C: Solid State Phys., vol. 18, no. 13, p. 2651, 1985.Google Scholar
[12] E., Witten, Chiral symmetry, the 1/N expansion and the SU(N) Thirring model, Nucl. Phys. B, vol. 145, p. 110, 1978.Google Scholar
[13] P., Coleman and N., Andrei, Diagonalisation of the generalised Anderson model, J. Phys. C: Solid State Phys., vol. 19, no. 17, p. 3211, 1986.Google Scholar
[14] P., Nozières and C. De, Dominicis, Singularities in the X-ray absorption and emission of metals III. one-body theory exact solution, Phys. Rev., vol. 178, no. 3, p. 1097, 1969.Google Scholar
[15] P., Coleman, J., B. Marston, and A., J. Schofield, Transport anomalies in a simplified model for a heavy electron quantum critical point, Phys. Rev. B, vol. 72, p. 245111, 2005.Google Scholar
[16] T., Senthil, M., Vojta, and S., Sachdev, Fractionalized Fermi liquids, Phys. Rev. Lett., vol. 90, p. 216403, 2003.Google Scholar
[17] P., Coleman, J. B., Marston, and A. J., Schofield, Transport anomalies in a simplified model for a heavy-electron quantum critical point, Phys. Rev., vol. 72, p. 245111, 2005.Google Scholar
[18] P. W., Anderson, The resonating valence bond state in La2CuO4 and superconductivity, Science, vol. 235, p. 1196, 1987.Google Scholar
[19] L. B., Ioffe and A. I., Larkin, Gapless fermions and gauge fields in dielectrics, Phys. Rev. B, vol. 39, p. 8988, 1989.Google Scholar
[20] J. W., Allen, B., Batlogg, and P., Wachter, Large low-temperature Hall effect and resistivity in mixed-valent SmB6, Phys. Rev. B, vol. 20, p. 4807, 1979.Google Scholar
[21] J. E., Moore, The birth of topological insulators, Nature, vol. 464, no. 7286, p. 194, 2010.Google Scholar
[22] R. B., Laughlin, Quantized Hall conductivity in two-dimensions, Phys. Rev. B, vol. 23, no. 10, p. 5632, 1981.Google Scholar
[23] D. J., Thouless, M., Kohmoto, M. P., Nightingale, and M. Den, Nijs, Quantized Hall conductance in a two-dimensional periodic potential, Phys. Rev. Lett., vol. 49, no. 6, p. 405, 1982.Google Scholar
[24] F. D. M., Haldane, Model for a quantum Hall effect without Landau levels condensed matter realization of the parity anomaly, Phys. rev. lett., vol. 61, no. 18, p. 2015, 1988.Google Scholar
[25] C. L., Kane and E. J., Mélé, Z2 topological order and the quantum spin Hall effect, Phys. Rev. Lett., vol. 95, p. 146802, 2005.Google Scholar
[26] B. A., Bernevig, T. L., Hughes, and S.-C., Zhang, Quantum spin Hall effect and topological phase transition in HgTe quantum wells, Science, vol. 314, no. 5806, p. 1757, 2006.Google Scholar
[27] R., Roy, Z2 classification of quantum spin Hall systems; an approach using timereversal invariance Phys. Rev. B, vol. 79, p. 195321, 2009.Google Scholar
[28] L., Fu, C. L., Kane, and E., J.Mélé, Topological insulators in three dimensions, Phys. Rev. Lett., vol. 98, p. 106803, 2007.Google Scholar
[29] J. E., Moore and L., Balents, Topological invariants of time-reversal-invariant band structures, Phys. Rev. B, vol. 75, p. 121306(R), 2007.Google Scholar
[30] G.E., Volovik, Fermion zero modes at the boundary of superfluid 3He-B, JETP Lett., vol. 90, no. 5, p. 398, 2009.Google Scholar
[31] G.E., Volovik, Topological invariant for superfluid 3He-B and quantum phase transitions, JETP Lett., vol. 90, no. 8, p. 587, 2009.Google Scholar
[32] M., König, S., Wiedmann, C., Brüne, A., Roth, H., Buhmann, L. W., Molenkamp, X.-L., Qi and S.-C., Zhan, Quantum spin Hall insulator state in HgTe quantum wells, Science, vol. 318, p. 766, 2007.Google Scholar
[33] D., Hsieh, D., Qian, L., Wray, Y., Xia, Y. S., Hor, R. J., Cava, and M. Z., Hasan, A topological Dirac insulator in a quantum spin Hall phase, Nature, vol. 452, no. 7190, p. 970, 2008.Google Scholar
[34] L., Fu and C. L., Kane, Topological insulators with inversion symmetry, Phys. Rev. B, vol. 76, no. 4, p. 45302, 2007.Google Scholar
[35] M., Dzero, K., Sun, V., Galitski, and P., Coleman, Topological Kondo insulators, Phys. Rev. Lett., vol. 104, p. 106408, 2010.Google Scholar
[36] J. C., Cooley, M. C., Aronson, A., Lacerda, Z., Fisk, P. C., Canfield, and R. P., Guertin, High magnetic fields and the correlation gap in SmB6, Phys. Rev. B, vol. 52, p. 7322, 1995.Google Scholar
[37] M., Dzero, K., Sun, P., Coleman, and V., Galitski, Theory of topological Kondo insulators, Phys. Rev. B, vol. 85, p. 045130, 2012.Google Scholar
[38] S., Wolgast, Ç., Kurdak, K., Sun, J.W., Allen, D.-J., Kim, and Z., Fisk, Low-temperature surface conduction in the Kondo insulator SmB6, Phys. Rev. B, vol. 88, p. 180405, 2013.Google Scholar
[39] D. J. S., Thomas, T., Grant, J., Botimer, Z., Fisk, and J., Xia, Surface Hall effect and nonlocal transport in SmB6: evidence for surface conduction, Sci. Rep., vol. 3, p. 3150, 2014.Google Scholar
[40] N., Xu, P. K., Biswas, J. H., Dil, et al. Direct observation of the spin texture in SmB6 as evidence of the topological Kondo insulator, Nat. Commun., vol. 5, p. 1, 2014.Google Scholar
[41] V., Alexandrov, P., Coleman, and O., Erten, Surface Kondo breakdown and the light surface states in topological Kondo insulators, Phys. Rev. Lett., vol. 114, p. 177202, 2015.Google Scholar
[42] W., Shockley, On the surface states associated with a periodic potential, Phys. Rev., vol. 56, p. 317, 1939.Google Scholar
[43] A. Yu., Kitaev, Unpaired Majorana fermions in quantum wires, Phys.-Usp (Supplement), vol. 44, no. 10S, p. 131, 2001.Google Scholar
[44] V., Alexandrov and P., Coleman, End states in a 1-D topological Kondo insulator, Phys. Rev. B, vol. 90, p. 115147, 2014.Google Scholar
[45] V., Alexandrov, M., Dzero, and P., Coleman, Cubic topological Kondo insulators, Phys. Rev. Lett., vol. 111, p. 226403, 2013.Google Scholar
[46] M. B., Maple and D., Wohlleben, Nonmagnetic 4f shell in the high-pressure phase of SmS, Phys. Rev. Lett., vol. 27, no. 8, p. 511, 1971.Google Scholar
[47] T., Takimoto, SmB6: a promising candidate for a topological insulator, J. Phys. Soc. Jpn., vol. 80, no. 12, p. 123710, 2011.Google Scholar
[48] M., Neupane, N., Alidoust, S. Y., Xu, and T., Kondo, Surface electronic structure of the topological Kondo-insulator candidate correlated electron system SmB6, Nat. Commun., 4:2991 DOI:0.1038/ncomms3991, 2013.Google Scholar
[49] N., Xu, X., Shi, P. K., Biswas, et al., Surface and bulk electronic structure of the strongly correlated system SmB6 and implications for a topological Kondo insulator, Phys. Rev. B, vol. 88, p. 121102, 2013.Google Scholar
[50] M., Sigrist and K., Ueda, Unconventional superconductivity, Rev. Mod. Phys., vol. 63, p. 239, 1991.Google Scholar
[51] N., Mathur, F. M., Grosche, S. R., Julian, I. R., Walker, D. M., Freye, and R. K. W., Haselwimmer, and G. G., Lonzarich, Magnetically mediated superconductivity in heavy-fermion compounds, Nature, vol. 394, p. 39, 1998.Google Scholar
[52] C., Petrovic, P. G., Pagliuso, M. F., Hundley, R., Movshovich, J. L., Sarrao, J. D., Thompson, Z., Fisk, and P., Monthoux, Heavy-fermion superconductivity in CeCoIn5 at 2.3 K, J. Phys: Condens. Matter, vol. 13, p. L337, 2001.Google Scholar
[53] N. J., Curro, T., Caldwell, E. D., Bauer et al., Unconventional superconductivity in PuCoGa5, Nature, vol. 434, p. 622, 2005.Google Scholar
[54] G., Stewart, Heavy-fermion systems, Rev. Mod. Phys., vol. 73, p. 797, 2001.Google Scholar
[55] G., Stewart, Addendum: Non-Fermi-liquid behavior in d-and f-electron metals, Rev. Mod. Phys., vol. 78, p. 743, 2006.Google Scholar
[56] P., Coleman, C., Pépin, Q., Si, and R., Ramazashvili, How do Fermi liquids get heavy and die?, J. Phys.: Condens. Matter, vol. 13, p. 273, 2001.Google Scholar
[57] C. M., Varma, Z., Nussinov, and W. van, Saarlos, Singular Fermi liquids, Phys. Rep., vol. 361, p. 267, 2002.Google Scholar
[58] H. von, Löhneysen, A., Rosch, M., Vojta, and P., Wölfle, Fermi-liquid instabilities at magnetic quantum phase transitions, Rev. Mod. Phys., vol. 79, p. 1015, Aug 2007.Google Scholar
[59] J., Custers, P., Gegenwart, H., Wilhelm, The break-up of heavy electrons at a quantum critical point, Nature, vol. 424, p. 524, 2003.Google Scholar
[60] Y., Matsumoto, S., Nakatsuji, K., Kuga, Y., Karaki, and N., Horie, Quantum criticality without tuning in the mixed valence compound ß-YbAlB4, Science, vol. 331, p. 316, 2011.Google Scholar
[61] P., Coleman and N., Andrei, Kondo-stabilised spin liquids and heavy-fermion superconductivity, J. Phys.: Condens. Matter, vol. 1, no. 26, p. 4057, 1989.Google Scholar
[62] R., Flint, M., Dzero, P., Coleman, and M., Dzero, Heavy electrons and the symplectic symmetry of spin, Nat. Phys., vol. 4, no. 8, p. 643, 2008.Google Scholar
[63] R., Flint and P., Coleman, Tandem pairing in heavy-fermion superconductors, Phys. Rev. Lett., vol. 105, p. 246404, 2010.Google Scholar
[64] R., Flint, A., Nevidomskyy, and P., Coleman, Composite pairing in a mixed-valent two-channel Anderson model, Phys. Rev. B, vol. 84, no. 6, p. 064514, 2011.Google Scholar
[65] W., Metzner and D., Vollhardt, Correlated lattice fermions in d = ∞ dimensions, Phys. Rev. Lett., vol. 62, p. 324, 1989.Google Scholar
[66] A., Georges and G., Kotliar, Hubbard model in infinite dimensions, Phys. Rev. B, vol. 45, p. 6479, 1992.Google Scholar
[67] A., Georges, G., Kotliar, W., Krauth, and M., Rozenberg, Dynamical mean-field theory of strongly correlated fermion systems and the limit of infinite dimensions, Rev. Mod. Phys., vol. 68, p. 13, 1996.Google Scholar
[68] S. R., White, Strongly correlated electron systems and the density matrix renormalization group, Phys. Rep., vol. 301, p. 187, 1998.Google Scholar
[69] K. A., Hallberg, New trends in density matrix renormalization, Adv. Phys., vol. 55, p. 477, 2006.Google Scholar
[70] T. R., Chien, Z. Z., Wang, and N. P., Ong, Effect of Zn impurities on the normal-state Hall angle in single-crystal YBa2Cu3-xZnx O7-δ, Phys. Rev. Lett., vol. 67, p. 2088, 1991.Google Scholar
[71] J., Paglione, M. A., Tanatar, D. G., Hawthorn, Nonvanishing energy scales at the quantum critical point of CeCoIn5, Phys. Rev. Lett., vol. 97, p. 106606, 2006.Google Scholar
[72] P. W., Anderson, Hall effect in the two-dimensional Luttinger liquid, Phys. Rev. Lett., vol. 67, p. 2092, 1991.Google Scholar
[73] P., Coleman, A. J., Schofield, and A. M., Tsvelik, Phenomenological transport equation for the cuprate metals, Phys. Rev. Lett., vol. 76, p. 1324, 1996.Google Scholar
[74] Y., Nakajima, K., Izawa, Y., Matsuda, Normal-state Hall angle and magnetoresistance in quasi-2D heavy fermion CeCoIn5 near a quantum critical point, J. Phys. Soc. Jpn., vol. 73, p. 5, 2004.Google Scholar
[75] J. M., Drouffe and J. B., Zuber, Strong coupling and mean-field methods in lattice gauge theories, Phys. Rep., vol. 102, p. 1, 1983.Google Scholar

Save book to Kindle

To save this book to your Kindle, first ensure [email protected] is added to your Approved Personal Document E-mail List under your Personal Document Settings on the Manage Your Content and Devices page of your Amazon account. Then enter the ‘name’ part of your Kindle email address below. Find out more about saving to your Kindle.

Note you can select to save to either the @free.kindle.com or @kindle.com variations. ‘@free.kindle.com’ emails are free but can only be saved to your device when it is connected to wi-fi. ‘@kindle.com’ emails can be delivered even when you are not connected to wi-fi, but note that service fees apply.

Find out more about the Kindle Personal Document Service.

Available formats
×

Save book to Dropbox

To save content items to your account, please confirm that you agree to abide by our usage policies. If this is the first time you use this feature, you will be asked to authorise Cambridge Core to connect with your account. Find out more about saving content to Dropbox.

Available formats
×

Save book to Google Drive

To save content items to your account, please confirm that you agree to abide by our usage policies. If this is the first time you use this feature, you will be asked to authorise Cambridge Core to connect with your account. Find out more about saving content to Google Drive.

Available formats
×