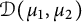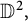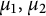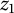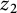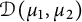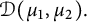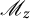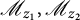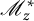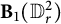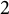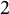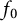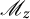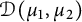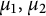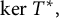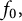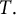We introduce and study Dirichlet-type spaces 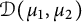 $\mathcal D(\mu _1, \mu _2)$ of the unit bidisc
$\mathcal D(\mu _1, \mu _2)$ of the unit bidisc 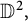 $\mathbb D^2,$ where
$\mathbb D^2,$ where 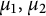 $\mu _1, \mu _2$ are finite positive Borel measures on the unit circle. We show that the coordinate functions
$\mu _1, \mu _2$ are finite positive Borel measures on the unit circle. We show that the coordinate functions 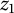 $z_1$ and
$z_1$ and 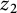 $z_2$ are multipliers for
$z_2$ are multipliers for 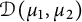 $\mathcal D(\mu _1, \mu _2)$ and the complex polynomials are dense in
$\mathcal D(\mu _1, \mu _2)$ and the complex polynomials are dense in 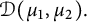 $\mathcal D(\mu _1, \mu _2).$ Further, we obtain the division property and solve Gleason’s problem for
$\mathcal D(\mu _1, \mu _2).$ Further, we obtain the division property and solve Gleason’s problem for  $\mathcal D(\mu _1, \mu _2)$ over a bidisc centered at the origin. In particular, we show that the commuting pair
$\mathcal D(\mu _1, \mu _2)$ over a bidisc centered at the origin. In particular, we show that the commuting pair 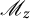 $\mathscr M_z$ of the multiplication operators
$\mathscr M_z$ of the multiplication operators 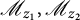 $\mathscr M_{z_1}, \mathscr M_{z_2}$ on
$\mathscr M_{z_1}, \mathscr M_{z_2}$ on  $\mathcal D(\mu _1, \mu _2)$ defines a cyclic toral
$\mathcal D(\mu _1, \mu _2)$ defines a cyclic toral  $2$-isometry and
$2$-isometry and 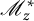 $\mathscr M^*_z$ belongs to the Cowen–Douglas class
$\mathscr M^*_z$ belongs to the Cowen–Douglas class 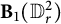 $\mathbf {B}_1(\mathbb D^2_r)$ for some
$\mathbf {B}_1(\mathbb D^2_r)$ for some  $r>0.$ Moreover, we formulate a notion of wandering subspace for commuting tuples and use it to obtain a bidisc analog of Richter’s representation theorem for cyclic analytic
$r>0.$ Moreover, we formulate a notion of wandering subspace for commuting tuples and use it to obtain a bidisc analog of Richter’s representation theorem for cyclic analytic 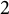 $2$-isometries. In particular, we show that a cyclic analytic toral
$2$-isometries. In particular, we show that a cyclic analytic toral 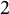 $2$-isometric pair T with cyclic vector
$2$-isometric pair T with cyclic vector 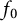 $f_0$ is unitarily equivalent to
$f_0$ is unitarily equivalent to 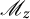 $\mathscr M_z$ on
$\mathscr M_z$ on 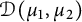 $\mathcal D(\mu _1, \mu _2)$ for some
$\mathcal D(\mu _1, \mu _2)$ for some 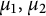 $\mu _1,\mu _2$ if and only if
$\mu _1,\mu _2$ if and only if 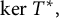 $\ker T^*,$ spanned by
$\ker T^*,$ spanned by 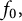 $f_0,$ is a wandering subspace for
$f_0,$ is a wandering subspace for 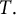 $T.$
$T.$