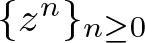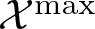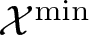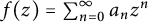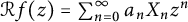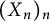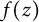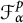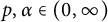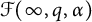Motivated by new examples of functional Banach spaces over the unit disk, arising as the symbol spaces in the study of random analytic functions, for which the monomials $\{z^n\}_{n\geq 0}$ exhibit features of an unconditional basis yet they often don’t even form a Schauder basis, we introduce a notion called solid basis for Banach spaces and p-Banach spaces and study its properties. Besides justifying the rich existence of solid bases, we study their relationship with unconditional bases, the weak-star convergence of Taylor polynomials, the problem of a solid span and the curious roles played by c0. The two features of this work are as follows: (1) during the process, we are led to revisit the axioms satisfied by a typical Banach space of analytic functions over the unit disk, leading to a notion of $\mathcal{X}^\mathrm{max}$ (and $\mathcal{X}^\mathrm{min}$), as well as a number of related functorial constructions, which are of independent interests; (2) the main interests of solid basis lie in the case of non-separable (p-)Banach spaces, such as BMOA and the Bloch space instead of VMOA and the little Bloch space.

Let $\mathcal {R}f$ be the randomization of an analytic function over the unit disk in the complex plane

$$ \begin{align*}\mathcal{R} f(z) =\sum_{n=0}^\infty a_n X_n z^n \in H({\mathbb D}), \end{align*} $$

$f(z)=\sum _{n=0}^\infty a_n z^n \in H({\mathbb D})$

$(X_n)_{n \geq 0}$

$f \in H({\mathbb D})$

${\mathcal R} f$

Let 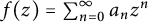$f(z)=\sum _{n=0}^\infty a_n z^n$ be an entire function on the complex plane, and let 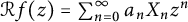${\mathcal R} f(z) = \sum _{n=0}^\infty a_n X_n z^n$ be its randomization induced by a standard sequence 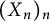$(X_n)_n$ of independent Bernoulli, Steinhaus, or Gaussian random variables. In this paper, we characterize those functions 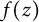$f(z)$ such that ${\mathcal R} f(z)$ is almost surely in the Fock space 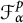${\mathcal F}_{\alpha }^p$ for any 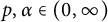$p, \alpha \in (0,\infty )$. Then such a characterization, together with embedding theorems which are of independent interests, is used to obtain a Littlewood-type theorem, also known as regularity improvement under randomization within the scale of Fock spaces. Other results obtained in this paper include: (a) a characterization of random analytic functions in the mixed-norm space 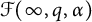${\mathcal F}(\infty , q, \alpha )$, an endpoint version of Fock spaces, via entropy integrals; (b) a complete description of random lacunary elements in Fock spaces; and (c) a complete description of random multipliers between different Fock spaces.