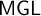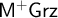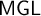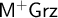1 results
MONADIC INTUITIONISTIC AND MODAL LOGICS ADMITTING PROVABILITY INTERPRETATIONS
- Part of
-
- Journal:
- The Journal of Symbolic Logic / Volume 88 / Issue 1 / March 2023
- Published online by Cambridge University Press:
- 02 December 2021, pp. 427-467
- Print publication:
- March 2023
-
- Article
- Export citation