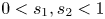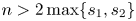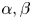1 results
Nonexistence of anti-symmetric solutions for fractional Hardy–Hénon system
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics / Volume 154 / Issue 3 / June 2024
- Published online by Cambridge University Press:
- 15 May 2023, pp. 862-886
- Print publication:
- June 2024
-
- Article
-
- You have access
- HTML
- Export citation
-
We study anti-symmetric solutions about the hyperplane $\{x_n=0\}$
for the following fractional Hardy–Hénon system:\[ \left\{\begin{array}{@{}ll} (-\Delta)^{s_1}u(x)=|x|^\alpha v^p(x), & x\in\mathbb{R}_+^n, \\ (-\Delta)^{s_2}v(x)=|x|^\beta u^q(x), & x\in\mathbb{R}_+^n, \\ u(x)\geq 0, & v(x)\geq 0,\ x\in\mathbb{R}_+^n, \end{array}\right. \]where $0< s_1,s_2<1$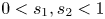
, $n>2\max \{s_1,s_2\}$
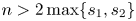. Nonexistence of anti-symmetric solutions are obtained in some appropriate domains of $(p,q)$
under some corresponding assumptions of $\alpha,\beta$
via the methods of moving spheres and moving planes. Particularly, for the case $s_1=s_2$
, one of our results shows that one domain of $(p,q)$
, where nonexistence of anti-symmetric solutions with appropriate decay conditions at infinity hold true, locates at above the fractional Sobolev's hyperbola under appropriate condition of $\alpha, \beta$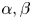
.
