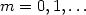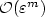In [C.W. Gear, T.J. Kaper, I.G. Kevrekidis and A. Zagaris,SIAM J. Appl. Dyn. Syst. 4 (2005) 711–732],we developeda class of iterative algorithmswithin the contextof equation-free methodsto approximatelow-dimensional,attracting,slow manifoldsin systemsof differential equationswith multiple time scales.For user-specified valuesof a finite numberof the observables,the mth memberof the classof algorithms( $m = 0, 1, \ldots$ 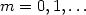 )finds iterativelyan approximationof the appropriate zeroof the (m+1)st time derivativeof the remaining variablesanduses this rootto approximate the locationof the pointon the slow manifoldcorresponding to these valuesof the observables.This articleis the firstof two articlesin whichthe accuracy and convergenceof the iterative algorithmsare analyzed.Here,we work directlywith fast-slow systems,in which there isan explicit small parameter, ε,measuring the separationof time scales.We show that,for each $m = 0, 1, \ldots$
)finds iterativelyan approximationof the appropriate zeroof the (m+1)st time derivativeof the remaining variablesanduses this rootto approximate the locationof the pointon the slow manifoldcorresponding to these valuesof the observables.This articleis the firstof two articlesin whichthe accuracy and convergenceof the iterative algorithmsare analyzed.Here,we work directlywith fast-slow systems,in which there isan explicit small parameter, ε,measuring the separationof time scales.We show that,for each $m = 0, 1, \ldots$ 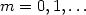 ,the fixed pointof the iterative algorithmapproximates the slow manifoldup to and includingterms of ${\mathcal O}(\varepsilon^m)$
,the fixed pointof the iterative algorithmapproximates the slow manifoldup to and includingterms of ${\mathcal O}(\varepsilon^m)$ 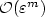 .Moreover,for each m,we identify explicitlythe conditionsunder whichthe mth iterative algorithmconverges to this fixed point.Finally,we show thatwhenthe iterationis unstable(orconverges slowly)it may be stabilized(orits convergencemay be accelerated)by applicationof the Recursive Projection Method.Alternatively,the Newton-KrylovGeneralized Minimal Residual Methodmay be used.In the subsequent article,we will considerthe accuracy and convergenceof the iterative algorithmsfor a broader classof systems – in whichthere need not bean explicitsmall parameter – to whichthe algorithms also apply.
.Moreover,for each m,we identify explicitlythe conditionsunder whichthe mth iterative algorithmconverges to this fixed point.Finally,we show thatwhenthe iterationis unstable(orconverges slowly)it may be stabilized(orits convergencemay be accelerated)by applicationof the Recursive Projection Method.Alternatively,the Newton-KrylovGeneralized Minimal Residual Methodmay be used.In the subsequent article,we will considerthe accuracy and convergenceof the iterative algorithmsfor a broader classof systems – in whichthere need not bean explicitsmall parameter – to whichthe algorithms also apply.