We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Let (Xt, t ≥ 0) be a Lévy process started at 0, with Lévymeasure ν. We consider the first passage time T x of(Xt, t ≥ 0) to level x > 0, and Kx := XTx - x theovershoot and Lx := x- XTx- the undershoot. We first provethat the Laplace transform of the random triple (Tx,Kx,Lx )satisfies some kind of integral equation. Second, assuming thatν admits exponential moments, we show that $(\widetilde{T_x},K_x,L_x)$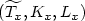 
converges in distribution asx → ∞, where $\widetilde{T_x}$ 
denotes a suitablerenormalization of T x .
