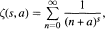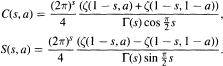1 results
Application of the Hurwitz Zeta Function to the Evaluation of Certain Integrals
-
- Journal:
- Canadian Mathematical Bulletin / Volume 36 / Issue 3 / 01 September 1993
- Published online by Cambridge University Press:
- 20 November 2018, pp. 373-384
- Print publication:
- 01 September 1993
-
- Article
-
- You have access
- Export citation