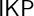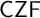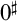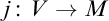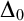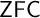Much of the theory of large cardinals beyond a measurable cardinal concerns the structure of elementary embeddings of the universe of sets into inner models. This paper seeks to answer the question of whether the inner model uniquely determines the elementary embedding.

We investigate large set axioms defined in terms of elementary embeddings over constructive set theories, focusing on 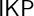$\mathsf {IKP}$ and 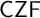$\mathsf {CZF}$. Most previously studied large set axioms, notably, the constructive analogues of large cardinals below 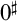$0^\sharp $, have proof-theoretic strength weaker than full Second-Order Arithmetic. On the other hand, the situation is dramatically different for those defined via elementary embeddings. We show that by adding to $\mathsf {IKP}$ the basic properties of an elementary embedding 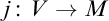$j\colon V\to M$ for 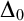$\Delta _0$-formulas, which we will denote by $\Delta _0\text {-}\mathsf {BTEE}_M$, we obtain the consistency of 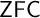$\mathsf {ZFC}$ and more. We will also see that the consistency strength of a Reinhardt set exceeds that of $\mathsf {ZF+WA}$. Furthermore, we will define super Reinhardt sets and $\mathsf {TR}$, which is a constructive analogue of V being totally Reinhardt, and prove that their proof-theoretic strength exceeds that of $\mathsf {ZF}$ with choiceless large cardinals.

I present solutions to several questions of Paul Bankston [2] by means of another version of the ultracoproduct construction, and explain the relation of ultracoproduct of compact Hausdorff spaces to other constructions combining topology, algebra and logic.