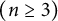1 results
Isometric dilation and Sarason’s commutant lifting theorem in several variables
- Part of
-
- Journal:
- Canadian Journal of Mathematics , First View
- Published online by Cambridge University Press:
- 25 February 2025, pp. 1-33
-
- Article
- Export citation
-
The article deals with isometric dilation and commutant lifting for a class of n-tuples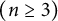
$(n\ge 3)$ of commuting contractions. We show that operator tuples in the class dilate to tuples of commuting isometries of BCL type. As a consequence of such an explicit dilation, we show that their von Neumann inequality holds on a one-dimensional variety of the closed unit polydisc. On the basis of such a dilation, we prove a commutant lifting theorem of Sarason’s type by establishing that every commutant can be lifted to the dilation space in a commuting and norm-preserving manner. This further leads us to find yet another class of n-tuples 
$(n\ge 3)$ of commuting contractions each of which possesses isometric dilation.
