We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Recursive trees have been used to model such things as the spread of epidemics, family trees of ancient manuscripts, and pyramid schemes. A tree Tn with n labeled nodes is a recursive tree if n = 1, or n > 1 and Tn can be constructed by joining node n to a node of some recursive tree Tn–1. For arbitrary nodes i < n in a random recursive tree we give the exact distribution of Xi,n, the distance between nodes i and n. We characterize this distribution as the convolution of the law of Xi,j+1 and n – i – 1 Bernoulli distributions. We further characterize the law of Xi,j+1 as a mixture of sums of Bernoullis. For i = in growing as a function of n, we show that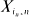 
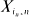is asymptotically normal in several settings.
