Khintchine's (necessary and sufficient) slowly varying function condition for the weak law of large numbers (WLLN) for the sum of n nonnegative, independent and identically distributed random variables is used as an overarching (sufficient) condition for the case that the number of summands is more generally [cn],cn→∞. Either the norming sequence {an},an→∞, or the number of summands sequence {cn}, can be chosen arbitrarily. This theorem generalizes results from a motivating branching process setting in which Khintchine's sufficient condition is automatically satisfied. A second theorem shows that Khintchine's condition is necessary for the generalized WLLN when it holds with cn→∞ and an→∞. Theorem 3, which is known, gives a necessary and sufficient condition for Khintchine's WLLN to hold with cn=n and an a specific function of n; it is extended to general cn subject to a growth restriction in Theorem 4. Section 6 returns to the branching process setting.

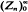Let denote the simple branching process with 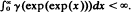Z0 = 1 and let G denote the distribution function of Z1. Suppose G satisfies x−α−γ(x)≦1 − G(x) ≦ x−α+γ(x) for large x, where (i) 0 < α < 1, (ii) γ (x) is non-negative and non-increasing, (iii) xγ(x) is non-decreasing and (iv) Then lim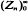n→∞α n log (Zn + 1) converges almost surely to a non-degenerate finite random variable W satisfying P(W = 0) = q = probability of extinction of the process.