1 results
Some Transformations of Hausdorff Moment Sequences and Harmonic Numbers
-
- Journal:
- Canadian Journal of Mathematics / Volume 57 / Issue 5 / 01 October 2005
- Published online by Cambridge University Press:
- 20 November 2018, pp. 941-960
- Print publication:
- 01 October 2005
-
- Article
-
- You have access
- Export citation
-
We introduce some non-linear transformations from the set of Hausdorff moment sequences into itself; among them is the one defined by the formula: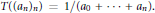
$T\left( {{\left( {{a}_{n}} \right)}_{n}} \right)\,=\,1/\left( {{a}_{0}}\,+\cdots +\,{{a}_{n}} \right).$ We give some examples of Hausdorff moment sequences arising from the transformations and provide the corresponding measures: one of these sequences is the reciprocal of the harmonic numbers 
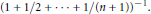${{\left( 1+1/2\,+\cdots +\,1/\left( n+1 \right) \right)}^{-1}}.$
