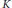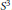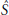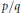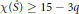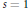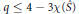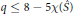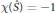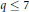For a non-trivial knot in the 3-sphere, only integral Dehn surgery can create a closed 3-manifold containing a projective plane. If we restrict ourselves to hyperbolic knots, the corresponding claim for a Klein bottle is still true. In contrast to these, we show that non-integral surgery on a hyperbolic knot can create a closed non-orientable surface of any genus greater than two.

Let $K$ be a knot in 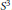${{S}^{3}}$ . This paper is devoted to Dehn surgeries which create 3-manifolds containing a closed non-orientable surface 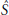$\hat{S}$ . We look at the slope 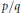$p/q$ of the surgery, the Euler characteristic $\mathcal{X}(\hat{S})$ of the surface and the intersection number $s$ between 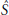$\hat{S}$ and the core of the Dehn surgery. We prove that if 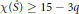$\mathcal{X}(\hat{S})\,\ge \,15\,-3q$, then 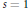$s\,=\,1$. Furthermore, if 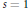$s\,=\,1$ then 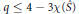$q\,\le \,4\,-\,3\,\mathcal{X}(\hat{S})$ or 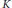$K$ is cabled and 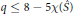$q\,\le \,8\,-5\mathcal{X}(\hat{S})$ . 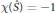As consequence, if $K$ is hyperbolic and $\mathcal{X}(\hat{S})\,=\,-1$ , then 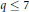$q\,\le \,7$.