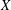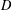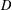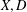In this paper, we provide an application to the random distance-t walk in finite planes and derive asymptotic formulas (as $q \to \infty $) for the probability of return to start point after $\ell $ steps based on the “vertical” equidistribution of Kloosterman sums established by N. Katz. This work relies on a “Euclidean” association scheme studied in prior work of W. M. Kwok, E. Bannai, O. Shimabukuro, and H. Tanaka. We also provide a self-contained computation of the P-matrix and intersection numbers of this scheme for convenience in our application as well as a more explicit form for the intersection numbers in the planar case.

Classical finite association schemes lead to finite-dimensional algebras which are generated by finitely many stochastic matrices. Moreover, there exist associated finite hypergroups. The notion of classical discrete association schemes can be easily extended to the possibly infinite case. Moreover, this notion can be relaxed slightly by using suitably deformed families of stochastic matrices by skipping the integrality conditions. This leads to a larger class of examples which are again associated with discrete hypergroups. In this paper we propose a topological generalization of association schemes by using a locally compact basis space 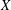$X$ and a family of Markov-kernels on $X$ indexed by some locally compact space 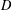$D$ where the supports of the associated probability measures satisfy some partition property. These objects, called continuous association schemes, will be related to hypergroup structures on 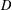$D$. We study some basic results for this notion and present several classes of examples. It turns out that, for a given commutative hypergroup, the existence of a related continuous association scheme implies that the hypergroup has many features of a double coset hypergroup. We, in particular, show that commutative hypergroups, which are associated with commutative continuous association schemes, carry dual positive product formulas for the characters. On the other hand, we prove some rigidity results in particular in the compact case which say that for given spaces 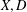$X,D$ there are only a few continuous association schemes.