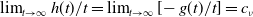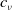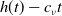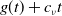Sharp asymptotic profile of the solution to a West Nile virus model with free boundary

Abstract

We consider the long-time behaviour of a West Nile virus (WNv) model consisting of a reaction–diffusion system with free boundaries. Such a model describes the spreading of WNv with the free boundary representing the expanding front of the infected region, which is a time-dependent interval $[g(t), h(t)]$ in the model (Lin and Zhu, Spatial spreading model and dynamics of West Nile virus in birds and mosquitoes with free boundary. J. Math. Biol. 75, 1381–1409, 2017). The asymptotic spreading speed of the front has been determined in Wang et al. (Spreading speed for a West Nile virus model with free boundary. J. Math. Biol. 79, 433–466, 2019) by making use of the associated semi-wave solution, namely $\lim _{t\to \infty } h(t)/t=\lim _{t\to \infty }[\!-g(t)/t]=c_\nu$, with $c_\nu$ the speed of the semi-wave solution. In this paper, by employing new techniques, we significantly improve the estimate in Wang et al. (Spreading speed for a West Nile virus model with free boundary. J. Math. Biol. 79, 433–466, 2019): we show that $h(t)-c_\nu t$ and $g(t)+c_\nu t$ converge to some constants as $t\to \infty$, and the solution of the model converges to the semi-wave solution. The results also apply to a wide class of analogous Ross–MacDonold epidemic models.

1. Introduction

The West Nile virus (WNv) is an arthropod-borne flavivirus that causes epidemics of febrile illness and sporadic encephalitis in many parts of the world. The incidence mechanism involves primarily interacting bird and mosquito populations, with birds acting as hosts and mosquitoes as vectors of the virus. For the prediction and prevention of the spreading of WNv, it is important to understand its temporal and spatial spreading dynamics.

Mathematical models may help us to understand the spreading process and to formulate suitable strategy to control the spreading of the virus. Ignoring spatial variations of the involved populations, several ODE models have been used to describe the WNv dynamics; see, for instance, Abdelrazec et al. [1], Bowman et al. [2], Kenkre et al. [22], Wonham et al. [35] and references therein. The dynamics of such an ODE model is governed by a basic reproduction number $\mathcal R_0$ . To be more specific, the virus tends to extinction if $\mathcal R_0\lt 1$ and it persists if $\mathcal R_0\gt 1$ .

To include the possible impact of spatial movement of WNv, Lewis et al. [24] first introduced suitable diffusion terms in the ODE model by considering the following reaction–diffusion system:

(1.1) \begin{eqnarray} \begin{cases} H_t=D_R H_{xx}+\alpha _R\beta _R\dfrac{N_R-H}{N_R}M-\gamma _RH,& x\in \mathbb{R}, t\gt 0, \\ M_t=D_V M_{xx}+\alpha _V\beta _R\dfrac{A_V-M}{N_R}H-d_V M,& x\in \mathbb{R}, t\gt 0, \end{cases} \end{eqnarray}

where $H(x,t)$ and $M(x,t)$ denote the densities of the infected bird and mosquito populations at spatial location $x$ and time $t$ , respectively. The parameters here are all positive constants: $D_R, D_V$ stand for the diffusion rates of birds and mosquitoes, respectively; $\alpha _V, \alpha _R$ represent the WNv transmission probability per bite to mosquitoes and to birds, respectively; $\beta _R$ is the biting rate of mosquitoes on birds; $\gamma _R$ is the recovery rate of birds from WNv; $d_V$ is the mosquito death rate; $N_R$ is the total number of susceptible and infected birds; and $A_V$ is the total number of susceptible and infected mosquitoes, which are assumed to be constants during the infection process.

To simplify the notations, we set

\begin{equation*} \begin {aligned} &a_1\,:\!=\frac {\alpha _R\beta _R}{N_R},\ a_2\,:\!=\frac {\alpha _V\beta _R}{N_R},\ b_1\,:\!=\gamma _R,\ b_2\,:\!=d_V,\\ &\ \ \ e_1\,:\!=N_R,\ e_2\,:\!=A_V,d_1\,:\!=D_R,\ d_2\,:\!=D_V, \end {aligned} \end{equation*}

and then system (1.1) is transformed to the following non-dimensional form:

(1.2) \begin{eqnarray} \begin{cases} H_t=d_1 H_{xx}+a_1(e_1-H)M-b_1H,&\quad x\in \mathbb{R},\, t\gt 0,\\ M_t=d_2 M_{xx}+a_2(e_2-M)H-b_2 M,&\quad x\in \mathbb{R},\, t\gt 0. \end{cases} \end{eqnarray}

The basic reproduction number arising from the ODE version of (1.2) is given by:

\begin{equation*} \mathcal R_0\,:\!=\sqrt {\frac {a_1a_2e_1e_2}{b_1b_2}}. \end{equation*}

Clearly, $\mathcal R_0\gt 1$ is equivalent to $a_1a_2e_1e_2\gt b_1b_2$ . Moreover, system (1.2) admits the trivial equilibrium $(0, 0)$ , and if $a_1a_2e_1e_2\gt b_1b_2$ , then it further has a unique positive constant endemic equilibrium:

\begin{equation*} (H^*, M^*)\,:\!=\left (\frac {a_1a_2e_1e_2-b_1b_2}{a_1a_2e_2+b_1a_2},\frac {a_1a_2e_1e_2-b_1b_2}{a_1a_2e_1+a_1 b_2}\right ). \end{equation*}

It is proved in [24] that if $\mathcal R_0\gt 1$ , (1.2) has a travelling wave solution $(H(x-ct), M(x-ct))$ satisfying

\begin{equation*} \lim _{(x-ct)\to -\infty }(H,M)=(H^*, M^*),\; \lim _{(x-ct)\to +\infty }(H, M)=(0,0) \end{equation*}

for every $c\geq c^*$ , where $c^*\gt 0$ is the minimal value with such a property. Moreover, Theorem 6.2 in [24] shows that if $H(x, 0)$ and $M(x,0)$ are non-negative with non-empty compact supports, then for all small $\epsilon \gt 0$ ,

\begin{equation*} \lim _{t\to \infty }\left [\sup _{|x|\leq (c^\ast -\epsilon )t}|(H(x,t), M(x,t))-(H^*, M^*)|\right ]=0 \end{equation*}

and

\begin{equation*} \lim _{t\to \infty }\left [\sup _{|x|\geq (c^\ast +\epsilon )t}|(H(x,t), M(x,t))-(0,0)|\right ]=0. \end{equation*}

Biologically, this means that the virus spreads with speed $c^*$ .

However, since the strong maximum principle implies that $H(x, t)\gt 0$ and $M(x,t)\gt 0$ for all $x\in \mathbb R$ once $t\gt 0$ , the above mathematical result for (1.2) does not provide a precise location of the spreading front of the epidemic region. When we say the virus spreads with speed $c^*$ , it is meant that for any small $\delta \gt 0$ , the level sets $\{x\,:\, H(x,t)=\delta \}$ and $\{x\,:\, M(x,t)=\delta \}$ move in space with asymptotic speed $c^*$ .

To better describe the location of the spreading front of the disease, Lin and Zhu [25] use a modified version of (1.2) to model the spreading of WNv, where the spreading front is explicitly expressed in the model as free boundaries. Under our notations here, the model of [25] has the form:

(1.3) \begin{eqnarray} \begin{cases} H_t=d_1 H_{xx}+a_1(e_1-H)M-b_1H,&g(t)\lt x\lt h(t),\quad t\gt 0,\\ M_t=d_2 M_{xx}+a_2(e_1-M)H-b_2M,&g(t)\lt x\lt h(t),\quad t\gt 0,\\ H(x,t)=M(x,t)=0,&x=g(t) \mbox{ or } x=h(t),\;\; t\gt 0,\\ g(0)=-h_0,\; \quad g^{\prime}(t)=-\nu H_{x}(g(t),t),& t\gt 0,\\ h(0)=h_0,\quad h^{\prime}(t)=-\nu H_{x}(h(t),t),& t\gt 0,\\ H(x,0)=H_{0}(x),\quad M(x,0)=M_{0}(x),&-h_0\leq x\leq h_0. \end{cases} \end{eqnarray}

The functions $x=g(t)$ and $x=h(t)$ are the moving boundaries to be determined; $\nu$ is a given constant. The initial functions satisfy, for some $h_0\gt 0$ ,

\begin{eqnarray*} \begin{cases} H_0\in C^2((\!-h_0,h_0))\cap C([\!-h_0,h_0]),\ H_0(\!-h_0)=H_0(h_0)=0, \ 0\lt H_0\leq e_1 \mbox{ in }(\!-h_0,h_0),\\ M_0\in C^2((\!-h_0,h_0))\cap C([\!-h_0,h_0]),\ M_0(\!-h_0)=M_0(h_0)=0, \ 0\lt M_0\leq e_2 \mbox{ in }(\!-h_0,h_0). \end{cases} \end{eqnarray*}

More explanations of the background and justification of the model (1.3) can be found in Section 2 of [25].

In (1.3), the population range of infected birds is represented by the changing interval $(g(t), h(t))$ , and the virus carrying mosquitoes are assumed to have the same population range. The expanding rate of the range boundaries is assumed to satisfy $g^{\prime}(t)=-\nu H_{x}(g(t),t)$ and $h^{\prime}(t)=-\nu H_x(h(t),t)$ , which coincides with the well-known Stefan free boundary condition. A detailed deduction of this free boundary condition based on suitable biological assumptions is given in [3]: if one assumes that the population range increases at a sacrifice of the species near the front, then these free boundary equations are satisfied with $\nu =d_1/k$ , where $d_1$ is the diffusion rate of $H$ and $k$ is the number of units of population loss of $H$ at the free boundary (spreading front) per unit time per unit volume/area. Similar free boundary conditions have also been used for analogous purposes in other models; see [4, 9, 11, 12, 16, 18–21, 31–34] for a small sample.

It was shown in [25] that (1.3) has a unique solution $(H,M,g,h)$ which is defined for all $t\gt 0$ , where $H, M\in C^{2,1}(\overline \Omega )$ and $g, h\in C^1([0,\infty ))$ with $\Omega \,:\!=\{(x,t)\,:\, x\in (g(t), h(t)),\ t\gt 0\}$ , and when $\mathcal R_0\leq 1$ , the virus always vanishes eventually, that is,

(1.4) \begin{equation} \mbox{$ \lim _{t\rightarrow \infty }[h(t)-g(t)]\lt \infty $ and $\lim _{t\rightarrow \infty }\left(\|M(\cdot,t)\|_{C([g(t),h(t)])}+\|H(\cdot,t)\|_{C([g(t),h(t)])}\right)=0$.} \end{equation}

If $\mathcal R_0\gt 1$ , then a spreading–vanishing dichotomy holds true:

Either (1.4) holds, or the virus spreads successfully, namely,

\begin{equation*}\left \{\begin {array}{l} \mbox { $\lim _{t\to \infty } h(t)=-\lim _{t\to \infty }g(t)=+\infty $ and } \\ \mbox { $\lim _{t\rightarrow \infty }(M(x,t),H(x,t))=(M^*, H^*)$ uniformly for $x$ in any bounded set of $\mathbb R$.} \end {array}\right. \end{equation*}

Criteria for vanishing and spreading are also established in [25]. More precisely, there is a critical length $L^*\gt 0$ so that either the range size $h(t)-g(t)$ reaches $L^*$ at a finite time and then spreading happens, or $h(t)-g(t)$ stays below this critical length $L^*$ for all time and then vanishing occurs. In particular, if $h(0)-g(0)=2h_0\geq L^*$ , then spreading always happens.

To determine the asymptotic spreading speed of the virus modelled by (1.3), we need to consider the following semi-wave problem:

(1.5) \begin{eqnarray} \left \{\begin{aligned} &d_1 u^{\prime\prime}-cu^{\prime}+a_1(e_1-u)v-b_1u=0,\quad 0\lt s\lt \infty,\\ &d_2 v^{\prime\prime}-cv^{\prime}+a_2(e_2-v)u-b_2v=0,\quad 0\lt s\lt \infty,\\ &(u(0),v(0))=(0,0),\quad (u(\infty ),v(\infty ))=(H^*, M^*). \end{aligned}\right. \end{eqnarray}

Proposition 1.1. (Theorem 3.2 of [33]) Suppose that $a_1a_2e_1e_2\gt b_1b_2$ . Then for every $ c\in [0, c^\ast )$ , system (1.5) has a unique strictly increasing solution $(u_c,v_c)\in (C^2(\mathbb{R}^+))^2$ ; for $c\geq c^\ast$ , system (1.5) has no such solution. Moreover, for any $\nu \gt 0$ there exists a unique $c_\nu \in (0,c^\ast )$ such that $u^{\prime}_{c_\nu }(0)=c_\nu/\nu$ .

The solution $(u_{c_\nu },v_{c_\nu })$ is called a semi-wave with speed $c_\nu$ , since $(\mathcal{H},\mathcal{M})(x,t)=(u_{c_\nu },v_{c_\nu })(c_\nu t-x)$ satisfies

\begin{eqnarray*} \begin{cases} \mathcal{H}_t=d_1 \mathcal{H}_{xx}+a_1(e_1-\mathcal{H})\mathcal{M}-b_1\mathcal{H},& x\lt c_\nu t,\, t\in \mathbb{R},\\ \mathcal{M}_t=d_2 \mathcal{M}_{xx}+a_2(e_2-\mathcal{M})\mathcal{H}-b_2 \mathcal{M}, & x\lt c_\nu t,\, t\in \mathbb{R},\\ (\mathcal{H},\mathcal{M})(\!-\infty,t)=(H^\ast,M^\ast ),& t\in \mathbb{R},\\ (\mathcal{H},\mathcal{M})(c_\nu t,t)=(0,0),\, (c_\nu t)^{\prime}=-\nu \mathcal{H}_x(c_\nu t,t),& t\in \mathbb{R}.\\ \end{cases} \end{eqnarray*}

When spreading happens for (1.3), by making use of such semi-waves, it is shown in [33] (see Theorem 3.15 there) that

(1.6) \begin{equation} \lim _{t\to \infty }\frac{h(t)}{t}=-\lim _{t\to \infty } \frac{g(t)}{t}=c_\nu, \end{equation}

which means that the asymptotic spreading speed determined by (1.3) is $c_\nu$ . Thus it is strictly less than that of the corresponding reaction–diffusion system (1.2) (i.e., $c_\nu \lt c^*$ ). ¹

The purpose of this paper is to provide a more precise description of the spreading profile of the solution to (1.3). We will show that, as $t\to \infty$ , $h(t)-c_\nu t$ and $g(t)+c_\nu t$ converge to some constants, and

\begin{equation*} \begin {cases} \big [H(x,t)-u_{c_\nu }(h(t)-x)\big ]\to 0, \ \big [M(x,t)-v_{c_\nu }(h(t)-x)\big ]\to 0 &\mbox { uniformly for } x\in [0, h(t)],\\ \big [H(x,t)-u_{c_\nu }(x-g(t))\big ]\to 0, \ \big [M(x,t)-v_{c_\nu }(x-g(t))\big ]\to 0 &\mbox { uniformly for } x\in [g(t), 0]. \end {cases} \end{equation*}

Thus, as time goes to infinity, the solution of the free boundary problem (1.3) behaves exactly like the semi-wave.

For a single equation with free boundaries, sharp convergence results of similar nature have been obtained in several recent works; see [11, 16, 18, 20, 21, 23] for one-dimensional problems and [12] for high-dimensional problems. To the best of our knowledge, there are no results giving such precise asymptotic profiles for systems with free boundary before this work. We believe that the techniques developed in this paper should have applications to some other free boundary systems where similar precise dynamical behaviour is expected.

The mathematical analysis of this paper is inspired by the method of Du, Matsuzawa and Zhou [11, 12], but considerable variations are needed as our model here is a system. Moreover, several new techniques are introduced here; see, for example, the proofs of Lemmas 3.4 and 4.2. Some of the new techniques here have the advantage of applicable to more general problems than those in [11, 12], where a lower estimate on the solution over a spatial domain of the form $(\!-ct, ct)$ , $0\lt c\lt c_\nu$ is required first. Even if it is applied to the same problems in [11, 12], the method here yields much simpler proofs.

Our main result is the following theorem.

Theorem 1.2. Let $(H,M,g,h)$ and $(u_{c_\nu },v_{c_\nu }, c_\nu )$ be the solutions of (1.3) and (1.5), respectively. When spreading happens to $(H,M,g,h)$ , there exist two constants $g^\ast$ and $h^\ast$ such that

(1.7) \begin{equation} \begin{cases} \lim _{t\rightarrow \infty }(g(t)+c_\nu t-g^\ast )=0,\ \lim _{t\rightarrow \infty }g^{\prime}(t)=-c_\nu,\\ \lim _{t\rightarrow \infty }(h(t)-c_\nu t-h^\ast )=0,\ \lim _{t\rightarrow \infty }h^{\prime}(t)=c_\nu. \end{cases} \end{equation}

Moreover,

(1.8) \begin{equation} \begin{cases}\lim _{t\rightarrow \infty }\|\left (H(\cdot,t),M(\cdot,t)\right )-\left (u_{c_\nu }(\!\cdot -g(t)), v_{c_\nu }(\!\cdot -g(t))\right )\|_{L^\infty ([g(t),0])}=0,\\ \lim _{t\rightarrow \infty }\|\left (H(\cdot,t),M(\cdot,t)\right )-\left (u_{c_\nu }(h(t)-\cdot ), v_{c_\nu }(h(t)-\cdot )\right )\|_{L^\infty ([0,h(t)])}=0. \end{cases} \end{equation}

We would like to further remark that the ODE version of (1.1) is widely known as the Ross–Macdonold model due to the early works of Ross [28] and Macdonald [27] on malaria, whose spreading relies on mosquito as a vector and human as a host. There is an extensive literature on the modelling of epidemic spreading by using various variations of the Ross–Macdonald model; see, for example, [26, 30] and the references therein. As a consequence, the mathematical results above are applicable to suitable free boundary versions of the Ross–Macdonold model as well.

Finally, we mention some more related works on WNv models with free boundary. Tarboush et al. [29] obtain a vanishing–spreading dichotomy for a similar model, where the equation for birds is a PDE while the equation for mosquitoes is an ODE; Cheng and Zheng [5] studied the dynamics and spreading speed of (1.3) with an advection term. However, the asymptotic profiles for these models have not been determined. To include long-distance dispersal of the virus, a WNv model with non-local diffusion and free boundaries is proposed and analysed very recently by Du and Ni [13–15]. But due to certain technical obstacles, no convergence result of the type as described in Theorem 1.2 above is available for such non-local diffusion models. Some further related recent work in this direction can be found in the review papers of Du [6, 7].

The rest of this paper is organised as follows. In Section 2, we collect some basic results including some comparison principles, rough estimate of solutions to (1.3) and the asymptotic behaviours of semi-wave solutions of (1.5). In Section 3, we show that $|g(t)+c_\nu t|$ and $|h(t)-c_\nu t|$ are both bounded for all $t\gt 0$ ; as mentioned earlier, we have to overcome several non-trivial difficulties here. In Section 4, we finish the proof of Theorem 1.2, where our arguments are based on the estimates obtained in Section 3 and on the construction of suitable upper and lower solutions.

2. Some preparations

In this section, we prepare some basic results. Firstly, we introduce some notations that will be used throughout this paper. For any vectors $\textbf{p}=(p_1,p_2,\ldots,p_m),\textbf{q}=(q_1,q_2,\ldots,q_m)\in \mathbb{R}^m$ , $\textbf{p}\preceq (\succeq ) \textbf{q}$ (resp., $\textbf{p}\prec (\succ )\textbf{q}$ ) means $p_i\leq (\geq )q_i$ (resp., $p_i\lt (\gt )q_i$ ) for $1\leq i\leq m$ . Any $B\,:\!=(b_{ij})\in \mathbb{R}^m\times \mathbb{R}^n$ is a matrix with $m$ rows and $n$ columns, whose transpose is denoted by $B^\top$ .

The following comparison principles for the free boundary problem (1.3) will be used. They are simple variations of Proposition 3.13 of [33] and can be proved by arguments similar to those used in the proof of Lemma 2.6 in [10].

Lemma 2.1. Let $(H,M,g, h)$ be the solution of (1.3). Assume that $T\in (0,\infty )$ , $\overline g, \overline{h}\in C^1([0,T])$ , $g(t)\leq \overline g(t)\lt \overline h(t)$ in $[0, T]$ , $\overline{H},\overline{M}\in C(\overline{D_T^{\ast }})\cap C^{2,1}(D_T^{\ast })$ with $D_T^{\ast }=\{(x,t)\in \mathbb{R}^2:x\in (\overline g(t),\overline{h}(t)),t\in (0,T]\}$ , and

(2.1) \begin{eqnarray} \begin{cases} \overline{H}_t-d_1\overline{H}_{xx}\geq a_1(e_1-\overline{H})\overline{M}-b_1\overline{H},\,& \overline g(t)\lt x\lt \overline{h}(t),\,0\lt t\lt T,\\ \overline{M}_t-d_2\overline{M}_{xx}\geq a_2(e_2-\overline{M})\overline{H}-b_2\overline{M},\,&\overline g(t)\lt x\lt \overline{h}(t),\,0\lt t\lt T,\\ \overline{H}(x,t)\geq H(x,t),\,\overline M(x,t)\geq M(x, t),\,& x=\overline g(t),\ 0\lt t\lt T,\\ \overline{H}(x,t)=\overline{M}(x,t)=0,\,& x=\overline{h}(t),\,0\lt t\lt T,\\ \overline{h}^{\prime}(t)\geq -\nu \overline{H}_x(\overline{h}(t),t),\,&0\lt t\lt T,\\ \overline{H}(x,0)\geq H_{0}(x),\, \overline{M}(x,0)\geq M_{0}(x),\,&\overline g(0)\leq x\leq h_0. \end{cases} \end{eqnarray}

Then

\begin{equation*}\begin {aligned} h(t)\leq \overline {h}(t),\ H(x,t)\leq \overline {H}(x,t),\ M(x,t)\leq \overline {M}(x,t)\mbox { for }\overline g(t)\leq x\leq h(t),\ 0\lt t\leq T. \end {aligned}\end{equation*}

Lemma 2.2. Let $(H,M,g, h)$ be the solution of (1.3). Assume that $T\in (0,\infty )$ , $\overline g\lt \overline{h}$ are functions in $ C^1([0,T])$ , $\overline{H},\overline{M}\in C(\overline{D_T^{\ast }})\cap C^{2,1}(D_T^{\ast })$ with $D_T^{\ast }=\{(x,t)\in \mathbb{R}^2:x\in (\overline g(t),\overline{h}(t)),t\in (0,T]\}$ , and

(2.2) \begin{eqnarray} \begin{cases} \overline{H}_t-d_1\overline{H}_{xx}\geq a_1(e_1-\overline{H})\overline{M}-b_1\overline{H},\,& \overline g(t)\lt x\lt \overline{h}(t),\,0\lt t\lt T,\\ \overline{M}_t-d_2\overline{M}_{xx}\geq a_2(e_2-\overline{M})\overline{H}-b_2\overline{M},\,&\overline g(t)\lt x\lt \overline{h}(t),\,0\lt t\lt T,\\ \overline{H}(x,t)=\overline{M}(x,t)=0,\,& x=\overline g(t) \mbox{ or } \overline{h}(t),\,0\lt t\lt T,\\ \overline{g}^{\prime}(t)\leq -\nu \overline{H}_x(\overline{g}(t),t),\ \overline{h}^{\prime}(t)\geq -\nu \overline{H}_x(\overline{h}(t),t),\,&0\lt t\lt T,\\ \overline{H}(x,0)\geq H_{0}(x),\, \overline{M}(x,0)\geq M_{0}(x),\,&-h_0\leq x\leq h_0. \end{cases} \end{eqnarray}

Then

\begin{equation*}\begin {aligned} g(t)\geq \overline g(t),\ h(t)\leq \overline {h}(t),\ H(x,t)\leq \overline {H}(x,t),\ M(x,t)\leq \overline {M}(x,t)\mbox { for } g(t)\leq x\leq h(t),\ 0\lt t\leq T. \end {aligned}\end{equation*}

Remark 2.3. (i) If the reverse inequalities in (2.1) hold, and $(\overline{H},\overline{M},\overline{g},\overline{h})$ is rewritten as $(\underline{H},\underline{M},\underline{g},\underline{h})$ , then

\begin{equation*}\begin {aligned} h(t)\geq \underline {h}(t), H(x,t)\geq \underline {H}(x,t), M(x,t)\geq \underline {M}(x,t)\mbox { for } g(t)\leq x\leq \underline {h}(t), 0\lt t\leq T. \end {aligned}\end{equation*}

(ii) Similarly, if the reverse inequalities in (2.2) hold, and $(\overline{H},\overline{M},\overline{g},\overline{h})$ is rewritten as $(\underline{H},\underline{M},\underline{g},\underline{h})$ , then

\begin{equation*}\begin {aligned} g(t)\leq \underline g(t),\ h(t)\geq \underline {h}(t), H(x,t)\geq \underline {H}(x,t), M(x,t)\geq \underline {M}(x,t)\mbox { for } \underline g(t) \leq x\leq \underline {h}(t), 0\lt t\leq T. \end {aligned}\end{equation*}

(iii) The functions $(\overline{H},\overline{M},\overline{g},\overline{h})$ and $(\underline{H},\underline{M},\underline{g},\underline{h})$ are usually called an upper solution and a lower solution of (1.3), respectively.

The global existence and uniqueness of a positive solution to (1.3) have been obtained in [25]. The following estimates on such solutions are needed later in the paper.

Lemma 2.4. (Theorem 3.1 of [25]) Suppose $(H,M,g,h)$ is the solution to (1.3). Then

\begin{equation*}(0,0)\preceq (H,M)(x,t)\preceq (e_1,e_2)\mbox { for }x\in [g(t),h(t)]\mbox { and }t\gt 0.\end{equation*}

Moreover, there exists $C_0\gt 0$ such that

\begin{equation*}-g^{\prime}(t),\ h^{\prime}(t)\in (0,C_0]\mbox { for }t\gt 0.\end{equation*}

By Lemma 3.8 and its proof in [33], we have the following result:

Lemma 2.5. Let $(u(s),v(s))$ be a monotone solution of (1.5). Then there exist constants $\hat \mu _1\lt 0$ , $p\gt 0$ and $q\gt 0$ such that, as $s\rightarrow \infty$ ,

(2.3) \begin{eqnarray} \begin{cases} (u(s),v(s))=(H^\ast,M^\ast )- e^{\hat{\mu }_1s}(p+o(1),q+o(1)),\\ (u^{\prime}(s), v^{\prime}(s))=O(e^{\hat{\mu }_1s} ). \end{cases} \end{eqnarray}

3. Bounds for $\boldsymbol{g}(\boldsymbol{t})+\boldsymbol{c}_{\boldsymbol{\nu}} \boldsymbol{t}$ and $\boldsymbol{h}(\boldsymbol{t})-\boldsymbol{c}_{\boldsymbol{\nu}} \boldsymbol{t}$

In this section, we show that when spreading happens, both $g(t)+c_\nu t$ and $h(t)-c_\nu t$ are bounded functions for $t\gt 0$ . More precisely, we will prove the following result:

Proposition 3.1. Suppose that spreading happens to the solution $(H,M,g,h)$ of (1.3). Then there exists a positive constant $C$ such that

\begin{equation*} |g(t)+c_\nu t|,|h(t)-c_\nu t|\leq C \mbox { for all }t\gt 0. \end{equation*}

We will prove this result by constructing suitable upper and lower solutions, in the spirit of Fife and McLeod [17], except that now we are dealing with a system of equations and the associated semi-waves are used.

3.1. Upper bound

In this subsection, we obtain an upper bound for $(H,M,g,h)$ by constructing an upper solution $(\overline{H},\overline{M},\bar{g},\bar{h})$ to (1.3) as follows:

(3.1) \begin{equation} \begin{aligned} \bar{g}(t)&=g(t), \ \bar{h}(t)=c_\nu (t-T^\ast )+\sigma (1-e^{-\delta (t-T^*)})+h(T^\ast )+X_0,\\ \overline{H}(x,t)&=(1+K_1e^{-\delta (t-T^*)})u_{c_\nu }(\bar{h}(t)-x),\ \overline{M}(x,t)=(1+K_1e^{-\delta (t-T^*)})v_{c_\nu }(\bar{h}(t)-x), \end{aligned} \end{equation}

where $T^\ast$ , $K_1,X_0,\delta,\sigma$ are positive constants to be determined later.

Lemma 3.2. For any given constants $T^*\gt 0$ and $X_0\gt 0$ , there exist positive constants $K_1, \delta$ and $\sigma$ , such that the solution $(H,M,h)$ to (1.3) satisfies, for $x\in [g(t),h(t)]\mbox{ and }t\gt T^\ast$ ,

(3.2) \begin{equation} (H,M)(x,t)\preceq (\overline{H},\overline{M})(x,t),\ \ h(t)\leq \bar{h}(t). \end{equation}

Proof. We claim that $(\overline{H},\overline{M},\bar{g},\bar{h})$ is an upper solution for $t\gt T^\ast$ by taking appropriate parameters $T^*,K_1,X_0,\delta$ and $\sigma$ , that is,

(3.3) \begin{eqnarray} &&\overline{H}_t\geq d_1 \overline{H}_{xx}+a_1(e_1-\overline{H})\overline{M}-b_1\overline{H},\quad \bar{g}(t)\lt x\lt \bar{h}(t),\quad t\gt T^\ast, \end{eqnarray}

(3.4) \begin{eqnarray} &&\overline{M}_t\geq d_2 \overline{M}_{xx}+a_2(e_2-\overline{M})\overline{H}-b_2 \overline{M},\quad \bar{g}(t)\lt x\lt \bar{h}(t),\quad t\gt T^\ast, \end{eqnarray}

(3.5) \begin{eqnarray} &&\overline{H}(x,t)\geq H(x,t),\quad \overline{M}(x,t)\geq M(x,t),\quad x=\bar{g}(t),\quad t\gt T^\ast, \end{eqnarray}

(3.6) \begin{eqnarray} &&\overline{H}(x,t)=0,\quad \overline{M}(x,t)=0,\quad x=\bar{h}(t),\quad t\gt T^\ast, \end{eqnarray}

(3.7) \begin{eqnarray} &&h(T^\ast )\leq \bar{h}(T^\ast ),\quad \bar{h}^{\prime}(t)\geq -\nu \overline{H}_{x}(\bar{h}(t),t),\quad t\gt T^\ast, \end{eqnarray}

(3.8) \begin{eqnarray} &&H(x,T^\ast )\leq \overline{H}(x,T^\ast ),\quad M(x,T^\ast )\leq \overline{M}(x,T^\ast ),\quad \bar{g}(T^\ast )\leq x\leq h(T^\ast ). \end{eqnarray}

If the above inequalities are verified, then we can apply Lemma 2.1 to conclude that (3.2) holds, and hence the proof is completed.

We now verify the inequalities (3.3)–(3.8). Firstly, it is clear that $(H,M)(\bar{g}(t),t)=(H,M)(g(t),t)$ $=(0,0)$ and $(\overline{H},\overline{M})(\bar{g}(t),t)\succ (0,0)$ for $t\gt T^\ast$ . Thus, (3.5) holds.

It is obvious that $(\overline{H},\overline{M})(\bar{h}(t),t)=(0,0)$ and $\bar{h}(T^\ast )=h(T^\ast )+X_0\gt h(T^\ast )$ . Moreover, direct computation gives that

\begin{equation*}\bar {h}^{\prime}(t)=c_\nu +\sigma \delta e^{-\delta (t-T^*)}\end{equation*}

and

\begin{equation*}-\nu \overline {H}_{x}(\bar {h}(t),t)=\nu (1+K_1e^{-\delta (t-T^*)})u_{c_\nu }^{\prime}(0) =c_\nu (1+K_1e^{-\delta (t-T^*)}).\end{equation*}

Hence, (3.6) and (3.7) hold provided that

(3.9) \begin{equation} \sigma \delta \geq c_\nu K_1. \end{equation}

Since $X_0\gt 0$ , for all large $K_1$ (depending on $X_0$ ), say $K_1\geq C(X_0)\gt 0$ , we have

(3.10) \begin{equation} (1+K_1)u_{c_\nu }(X_0)\geq e_1,\ (1+K_1)v_{c_\nu }(X_0)\geq e_2. \end{equation}

Hence, due to Lemma 2.4, we have, for $x\in [\overline{g}(T^\ast ),h(T^\ast )]$ ,

\begin{eqnarray*} \begin{aligned} \overline{H}(x,T^\ast )&=(1+K_1)u_{c_\nu }(\bar{h}(T^\ast )-x)\\ &=(1+K_1)u_{c_\nu }(h(T^\ast )+X_0-x)\\ &\geq (1+K_1)u_{c_\nu }(X_0)\geq e_1\\ &\geq H(x,T^\ast ). \end{aligned} \end{eqnarray*}

A similar argument gives $\overline{M}(x,T^\ast )\geq M(x,T^\ast )$ for $x\in [\overline{g}(T^\ast ),h(T^\ast )]$ . Hence, (3.8) holds true.

Finally, we show (3.3) and (3.4). Let $s=\bar{h}(t)-x$ . Then,

\begin{eqnarray*} \begin{aligned} \overline{H}_t(x,t)&=-\delta K_1e^{-\delta (t-T^*)}u_{c_\nu }(s)+(1+K_1e^{-\delta (t-T^*)})u_{c_\nu }^{\prime}(s)\bar{h}^{\prime}(t)\\ &=-\delta K_1e^{-\delta (t-T^*)}u_{c_\nu }(s)+(1+K_1e^{-\delta (t-T^*)})(c_\nu +\sigma \delta e^{-\delta (t-T^*)})u_{c_\nu }^{\prime}(s),\\ \overline{M}_t(x,t)&=-\delta K_1e^{-\delta (t-T^*)}v_{c_\nu }(s)+(1+K_1e^{-\delta (t-T^*)})v_{c_\nu }^{\prime}(s)\bar{h}^{\prime}(t)\\ &=-\delta K_1e^{-\delta (t-T^*)}v_{c_\nu }(s)+(1+K_1e^{-\delta (t-T^*)})(c_\nu +\sigma \delta e^{-\delta (t-T^*)})v_{c_\nu }^{\prime}(s) \end{aligned} \end{eqnarray*}

and

\begin{eqnarray*} \begin{aligned} \overline{H}_{xx}(x,t)=(1+K_1e^{-\delta (t-T^*)})u_{c_\nu }^{\prime\prime}(s),\ \overline{M}_{xx}(x,t)=(1+K_1e^{-\delta (t-T^*)})v_{c_\nu }^{\prime\prime}(s). \end{aligned} \end{eqnarray*}

Therefore,

\begin{eqnarray*} \begin{aligned} &\overline{H}_t- d_1 \overline{H}_{xx}-a_1(e_1-\overline{H})\overline{M}+b_1 \overline{H}\\ &\quad=-\delta K_1e^{-\delta (t-T^*)}u_{c_\nu }(s)+(1+K_1e^{-\delta (t-T^*)})(c_\nu +\sigma \delta e^{-\delta (t-T^*)})u_{c_\nu }^{\prime}(s)\\ &\qquad-d_1 (1+K_1e^{-\delta (t-T^*)})u_{c_\nu }^{\prime\prime}(s)\\ &\qquad-a_1[e_1-(1+K_1e^{-\delta (t-T^*)})u_{c_\nu }(s)](1+K_1e^{-\delta (t-T^*)})v_{c_\nu }(s)+b_1(1+K_1e^{-\delta (t-T^*)})u_{c_\nu }(s)\\ &\quad=-\delta K_1e^{-\delta (t-T^*)}u_{c_\nu }(s)+(1+K_1e^{-\delta (t-T^*)})\sigma \delta e^{-\delta (t-T^*)}u_{c_\nu }^{\prime}(s)\\ &\qquad+(1+K_1e^{-\delta (t-T^*)})\big [c_\nu u_{c_\nu }^{\prime}(s)-d_1u_{c_\nu }^{\prime\prime}(s)\big ]\\ &\qquad-a_1[e_1-(1+K_1e^{-\delta (t-T^*)})u_{c_\nu }(s)](1+K_1e^{-\delta (t-T^*)})v_{c_\nu }(s)+b_1(1+K_1e^{-\delta (t-T^*)})u_{c_\nu }(s)\\ &\quad=-\delta K_1e^{-\delta (t-T^*)}u_{c_\nu }(s)+(1+K_1e^{-\delta (t-T^*)})\sigma \delta e^{-\delta (t-T^*)}u_{c_\nu }^{\prime}(s)\\ &\qquad+(1+K_1e^{-\delta (t-T^*)})[a_1(e_1-u_{c_\nu }(s))v_{c_\nu }(s)-b_1u_{c_\nu }(s)]\\ &\qquad-a_1[e_1-(1+K_1e^{-\delta (t-T^*)})u_{c_\nu }(s)](1+K_1e^{-\delta (t-T^*)})v_{c_\nu }(s)+b_1(1+K_1e^{-\delta (t-T^*)})u_{c_\nu }(s)\\ &\quad=\ e^{-\delta (t-T^*)}\Big [\!-\delta K_1u_{c_\nu }(s)+(1+K_1e^{-\delta (t-T^*)})\sigma \delta u_{c_\nu }^{\prime}(s) +a_1K_1u_{c_\nu }(s)v_{c_\nu }(s)(1+K_1e^{-\delta (t-T^*)})\Big ]. \end{aligned} \end{eqnarray*}

Since

(3.11) \begin{eqnarray} (H^*, M^*)\succeq (u_{c_\nu },v_{c_\nu })(s)\succ (0,0) \mbox{ for } s\gt 0,\ \left(u_{c_\nu }^{\prime},v_{c_\nu }^{\prime}\right)(s)\succ (0,0) \mbox{ for } s\geq 0, \end{eqnarray}

we have

\begin{equation*} \begin {aligned} &-\delta K_1u_{c_\nu }(s)+(1+K_1e^{-\delta (t-T^*)})\sigma \delta u_{c_\nu }^{\prime}(s) +a_1K_1u_{c_\nu }(s)v_{c_\nu }(s)(1+K_1e^{-\delta (t-T^*)})\\ &\quad\geq -\delta K_1 H^*+\sigma \delta u_{c_\nu }^{\prime}(s) +a_1K_1u_{c_\nu }(s)v_{c_\nu }(s) \mbox { for all $s\geq 0$}. \end {aligned}\end{equation*}

Define

\begin{equation*} A\,:\!=\min _{s\geq 1}u_{c_\nu }(s)v_{c_\nu }(s)=u_{c_\nu }(1)v_{c_\nu }(1),\; B_1\,:\!=\min _{s\in [0, 1]} u_{c_\nu }^{\prime}(s),\ B_2\,:\!=\min _{s\in [0,1]}v_{c_\nu }^{\prime}(s). \end{equation*}

Then $A, \, B_1$ , $B_2\gt 0$ and

\begin{equation*} -\delta K_1 H^*+\sigma \delta u_{c_\nu }^{\prime}(s) +a_1K_1u_{c_\nu }(s)v_{c_\nu }(s) \geq \begin {cases} -\delta K_1 H^*+\sigma \delta B_1 & \mbox { for } s\in [0,1],\\ -\delta K_1 H^*+a_1K_1 A& \mbox { for } s\geq 1. \end {cases} \end{equation*}

Therefore, (3.3) holds provided that

(3.12) \begin{equation} \sigma B_1\geq K_1H^* \mbox{ and } a_1A\geq \delta H^*. \end{equation}

Moreover, by parallel arguments we see that (3.4) holds provided that additionally

(3.13) \begin{equation} \sigma B_2\geq K_1M^* \mbox{ and } a_2A\geq \delta M^*. \end{equation}

Now for any given $X_0\gt 0$ and $T^*\gt 0$ , we can choose $\sigma, \delta, K_1$ such that (3.9), (3.10), (3.12) and (3.13) hold simultaneously; for example, we may first choose $K_1$ large satisfying (3.10) and then choose $\delta \gt 0$ small and choose $\sigma$ large such that (3.9), (3.12) and (3.13) hold. The proof is now complete.

3.2. Lower bound

The lower bound will be obtained by constructing a lower solution $(\underline{H},\underline{M},\underline{g},\underline{h})$ to (1.3). Set

(3.14) \begin{equation} \begin{aligned} \underline{g}(t)&=-\underline h (t), \ \underline{h}(t)=c_\nu (t-T_\ast )+L-(1-e^{-\delta (t-T_*)}),\\ \underline{H}(x,t)&=(1-\tilde \epsilon e^{-\delta (t-T_*)})\big [u_{c_\nu }(\underline{h}(t)-x)+u_{c_\nu }(\underline{h}(t)+x)-u_{c_\nu }(2\underline{h}(t))\big ],\\ \underline{M}(x,t)&=(1-\tilde \epsilon e^{-\delta (t-T_*)})\big [ v_{c_\nu }(\underline{h}(t)-x)+v_{c_\nu }(\underline{h}(t)+x)-v_{c_\nu }(2\underline{h}(t))\big ], \end{aligned} \end{equation}

where $T_\ast$ , $\tilde \epsilon \in (0, 1),\delta, L$ are positive constants to be determined later.

We will need the following result from [15]:

Lemma 3.3. Suppose that $F=(f_i)\in C^2({\mathbb R}^m,{\mathbb R}^m)$ , $\mathbf{u}^*\succ 0$ and

(3.15) \begin{equation} F(\mathbf u^*)=\mathbf{0},\ \mathbf{u}^*[\nabla F(\mathbf{u}^*)]^\top \prec{\bf 0}. \end{equation}

Then there exists $\delta _0\gt 0$ small such that for $0\lt \epsilon \ll 1$ and $\mathbf u, \mathbf v\in [(1-\delta _0)\mathbf{u}^*, \mathbf{u}^*]$ satisfying

\begin{equation*} (u^*_i-u_i)(u^*_j-v_j)\leq C\delta _0\epsilon \mbox { for some $C\gt 0$ and all } i, j\in \{1,\ldots, m\}, \end{equation*}

we have

\begin{equation*} (1-\epsilon )[F(\mathbf u)+ F(\mathbf v)]- F((1-\epsilon )(\mathbf u+\mathbf v-\mathbf {u}^*))\preceq \frac \epsilon 2\mathbf {u}^*[\nabla F(\mathbf {u}^*)]^\top. \end{equation*}

We will use this lemma with $ \mathbf u^*\,:\!=(H^*, M^*)$ and

\begin{equation*} F(H,M)=(f_1(H,M), f_2(H,M))\,:\!=(a_1(e_1-H)M-b_1H, a_2(e_2-M)H-b_2M), \end{equation*}

which is easily checked to satisfy (3.15).

Lemma 3.4. For some suitable choice of $T_\ast$ , $\tilde \epsilon \in (0, 1), \delta$ and $ L$ , the solution $(H,M,g,h)$ of (1.3) satisfies, for $x\in [\underline{g}(t),\underline{h}(t)]$ and $t\gt T_\ast$ ,

(3.16) \begin{equation} (H,M)(x,t)\succeq (\underline{H},\underline{M})(x,t),\ \ [g(t), h(t)] \supset [\!-\underline{h}(t), \underline h(t)]. \end{equation}

Proof. We show that $(\underline{H},\underline{M},\underline{g},\underline{h})$ is a lower solution for $t\gt T_\ast$ by taking appropriate parameters $T_\ast$ , $\tilde \epsilon \in (0, 1),\delta$ and $L$ , namely

(3.17) \begin{align} &\underline{H}_t\leq d_1 \underline{H}_{xx}+f(\underline{H},\underline{M}),&& \underline{g}(t)\lt x\lt \underline{h}(t),\quad t\gt T_\ast, \end{align}

(3.18) \begin{align} &\underline{M}_t\leq d_2 \underline{M}_{xx}+g(\underline{H}, \underline{M}),&& \underline{g}(t)\lt x\lt \underline{h}(t),\quad t\gt T_\ast, \end{align}

(3.19) \begin{align} &\underline{H}(x,t)=0,\quad \underline{M}(x,t)=0,&& x=\underline{h}(t) \mbox{ or } \underline{g}(t),\quad t\gt T_\ast, \end{align}

(3.20) \begin{align} &-g(T_*),\ h(T_\ast )\geq \underline{h}(T_\ast ),\quad \underline{h}^{\prime}(t)\leq -\nu \underline{H}_{x}(\underline{h}(t),t),&& t\gt T_\ast, \end{align}

(3.21) \begin{align} &H(x,T_\ast )\geq \underline{H}(x,T_\ast ),\quad M(x,T_\ast )\geq \underline{M}(x,T_\ast ), &&\underline{g}(T_\ast )\leq x\leq \underline h(T_\ast ). \end{align}

If the above inequalities are verified, then we can apply Remark 2.3 (ii) to conclude that (3.16) holds, and hence the proof is completed. Note that since $\underline H(x,t)$ is even in $x$ and $\underline g(t)=-\underline h(t)$ , (3.20) implies $\underline g^{\prime}(t)\geq -\nu \underline{H}_{x}(\underline{g}(t),t)$ .

We now verify the inequalities (3.17)–(3.21). Since spreading happens, for $T_*=T_*(L, \tilde \epsilon )$ large enough, we have

\begin{equation*} [g(T_*), h(T_*)]\supset [\!-L, L]=[\underline g(T_*), \underline h(T_*)] \end{equation*}

and

\begin{equation*} (H(x, T_*), M(x,T_*))\succeq (1-\tilde \epsilon )(H^*, M^*)\succeq (\underline H(x,T_*), \underline M(x, T_*)) \mbox { for } x\in [\!-L,L]. \end{equation*}

It is obvious that $(\underline{H},\underline{M})(\pm \underline{h}(t),t)=(0,0)$ . Direct calculations yield

\begin{equation*}\underline {h}^{\prime}(t)=c_\nu - \delta e^{-\delta (t-T_*)}\end{equation*}

and

\begin{align*} -\nu \underline{H}_{x}(\underline{h}(t),t)&=\nu (1-\tilde{\epsilon }e^{-\delta (t-T_*)})\big [u_{c_\nu }^{\prime}(0)-u_{c_\nu }^{\prime}(2\underline h(t))\big ]\\ &\geq c_\nu (1-\tilde{\epsilon }e^{-\delta (t-T_*)})-C e^{2 \hat \mu _1 \underline h(t)}\\ &\geq c_\nu - c_\nu \tilde{\epsilon }e^{-\delta (t-T_*)}-C e^{2|\hat \mu _1|(1-L)}e^{2\hat \mu _1 c_\nu (t-T_*)} \end{align*}

for some $C\gt 0$ due to (2.3). We now fix

\begin{equation*} \delta \in (0, |\hat \mu _1|c_\nu )\cap (0, c_\nu ) \end{equation*}

and obtain

\begin{equation*} \underline {h}^{\prime}(t)\leq -\nu \underline {H}_{x}(\underline {h}(t),t) \mbox { for } t\geq T_* \end{equation*}

provided that

(3.22) \begin{equation} c_\nu \tilde{\epsilon }+C e^{2|\hat \mu _1|(1-L)}\leq \delta, \end{equation}

which holds when $\tilde \epsilon \gt 0$ is sufficiently small and $L$ is sufficiently large. Hence, (3.19), (3.20) and (3.21) hold.

Finally, we check (3.17) and (3.18). Clearly, writing $\epsilon =\epsilon (t)\,:\!=\tilde{\epsilon }e^{-\delta (t-T_*)}$ , we have

\begin{eqnarray*} \begin{aligned} \underline{H}_t(x,t)&=\delta \epsilon \big [u_{c_\nu }(\underline h(t)-x) +u_{c_\nu }(\underline h(t)+x)-u_{c_\nu }(2\underline h(t))\big ]\\ & \quad +(1-\epsilon )(c_\nu - \delta e^{-\delta (t-T_*)})\big [u_{c_\nu }^{\prime}(\underline h(t)-x) +u_{c_\nu }^{\prime}(\underline h(t)+x)-2u_{c_\nu }^{\prime}(2\underline h(t))\big ], \\ \underline{M}_t(x,t)&=\delta \epsilon \big [v_{c_\nu }(\underline h(t)-x) +v_{c_\nu }(\underline h(t)+x)-v_{c_\nu }(2\underline h(t))\big ]\\ & \quad +(1-\epsilon )(c_\nu - \delta e^{-\delta (t-T_*)})\big [v_{c_\nu }^{\prime}(\underline h(t)-x) +v_{c_\nu }^{\prime}(\underline h(t)+x)-2v_{c_\nu }^{\prime}(2\underline h(t))\big ] \end{aligned} \end{eqnarray*}

and

\begin{eqnarray*} \begin{aligned} \underline{H}_{xx}(x,t)&=(1-\epsilon )\big [u_{c_\nu }^{\prime\prime}(\underline h(t)-x) +u_{c_\nu }^{\prime\prime}(\underline h(t)+x)\big ],\\ \underline{M}_{xx}(x,t)&=(1-\epsilon )\big [v_{c_\nu }^{\prime\prime}(\underline h(t)-x) +v_{c_\nu }^{\prime\prime}(\underline h(t)+x)\big ]. \end{aligned} \end{eqnarray*}

Therefore,

\begin{eqnarray*} \begin{aligned} &\underline{H}_t- d_1\underline{H}_{xx}-f(\underline H, \underline M)\\ &\quad=\delta \epsilon \big [u_{c_\nu }(\underline h(t)-x) +u_{c_\nu }(\underline h(t)+x)-u_{c_\nu }(2\underline h(t))\big ]\\ &\quad \ \ \ +(1-\epsilon )(c_\nu - \delta e^{-\delta (t-T_*)})\big [u_{c_\nu }^{\prime}(\underline h(t)-x) +u_{c_\nu }^{\prime}(\underline h(t)+x)-2u_{c_\nu }^{\prime}(2\underline h(t))\big ] \\ &\quad\ \ \ -d_1(1-\epsilon )\big [u_{c_\nu }^{\prime\prime}(\underline h(t)-x) +u_{c_\nu }^{\prime\prime}(\underline h(t)+x)\big ]-f(\underline H, \underline M)\\ &\quad= \delta \epsilon \big [u_{c_\nu }(\underline h(t)-x) +u_{c_\nu }(\underline h(t)+x)-u_{c_\nu }(2\underline h(t))\big ]\\ &\quad \ \ \ +(1-\epsilon )\big [ f(u_{c_\nu }(\underline h-x),v_{c_\nu }(\underline h-x))+f(u_{c_\nu }(\underline h+x), v_{c_\nu }(\underline h+x))\big ]-f(\underline H, \underline M)\\ &\quad \ \ \ - (1-\epsilon )\delta e^{-\delta (t-T_*)}\big [u_{c_\nu }^{\prime}(\underline h(t)-x) +u_{c_\nu }^{\prime}(\underline h(t)+x)\big ] \\ &\quad \ \ \ - (1-\epsilon )(c_\nu - \delta e^{-\delta (t-T_*)})2u_{c_\nu }^{\prime}(2\underline h(t)). \end{aligned} \end{eqnarray*}

For $t\geq T_*$ and $x\in [\!-\underline h(t), \underline h(t)]$ , by the monotonicity of $u_{c_\nu }$ , we have

\begin{equation*} \delta \epsilon \big [u_{c_\nu }(\underline h(t)-x) +u_{c_\nu }(\underline h(t)+x)-u_{c_\nu }(2\underline h(t))\big ]\leq \delta H^*\epsilon (t). \end{equation*}

Since $\delta \lt c_\nu$ and $0\lt \tilde \epsilon \ll 1$ , we have

\begin{equation*} - (1-\epsilon )(c_\nu - \delta e^{-\delta (t-T_*)})2u_{c_\nu }^{\prime}(2\underline h(t))\leq 0,\end{equation*}

and by (2.3),

\begin{equation*}\begin {aligned} &-f(\underline H, \underline M)\leq -f(\underline H+(1-\epsilon )[u_{c_\nu }(2\underline h(t))-H^*], \underline M+(1-\epsilon )[v_{c_\nu }(2\underline h(t))-M^*])+Ce^{2 \hat \mu _1 \underline h(t)}\\ &\quad=-f((1-\epsilon )[u_{c_\nu }(\underline h-x)+u_{c_\nu }(\underline h+x)-H^*], (1-\epsilon )[v_{c_\nu }(\underline h-x)+v_{c_\nu }(\underline h+x)-M^*])+Ce^{2 \hat \mu _1 \underline h(t)}. \end {aligned} \end{equation*}

Hence, we have, for $t\geq T_*$ and $x\in [\!-\underline h(t), \underline h(t)]$ ,

\begin{equation*}\underline {H}_t- d_1\underline {H}_{xx}-f(\underline H, \underline M) \\ \leq \delta H^*\epsilon (t)+Ce^{2 \hat \mu _1 \underline h(t)} +A(x,t)+B(x,t), \end{equation*}

where

\begin{align*} A(x,t):&= (1-\epsilon )\big [ f(u_{c_\nu }(\underline h-x), v_{c_\nu }(\underline h-x))+f(u_{c_\nu }(\underline h+x), v_{c_\nu }(\underline h+x))\big ]\\ &\ \ \ \ -f((1-\epsilon )[u_{c_\nu }(\underline h-x)+u_{c_\nu }(\underline h+x)-H^*], (1-\epsilon )[v_{c_\nu }(\underline h-x)+v_{c_\nu }(\underline h+x)-M^*]) \end{align*}

and

\begin{equation*} B(x,t)\,:\!=-(1-\epsilon )\delta e^{-\delta (t-T_*)}\big [u_{c_\nu }^{\prime}(\underline h(t)-x) +u_{c_\nu }^{\prime}(\underline h(t)+x)\big ]. \end{equation*}

We next choose a suitable $K_0\gt 0$ and estimate

\begin{equation*} \delta H^*\epsilon (t)+Ce^{2 \hat \mu _1 \underline h(t)} +A(x,t)+B(x,t) \end{equation*}

for $x$ in the following three intervals, separately:

\begin{equation*} I_1(t)\,:\!=[\underline h(t)-K_0,\underline h(t)], \ I_2(t)\,:\!= [\!-\underline h(t),-\underline h(t)+K_0], \ I_3(t)\,:\!=[\!-\underline h(t)+K_0,\underline h(t)-K_0]. \end{equation*}

With $\delta _0\gt 0$ determined by Lemma 3.3, we fix $K_0\gt 0$ so that

\begin{equation*} H^*-u_{c_\nu }(K_0)\leq \delta _0H^*,\ \ M^*-v_{c_\nu }(K_0)\leq \delta _0M^*. \end{equation*}

Then for $x\in I_3(t)$ , $t\geq T_*$ and $0\lt \tilde \epsilon \ll 1$ , clearly

\begin{equation*} u_{c_\nu }(\underline h-x), u_{c_\nu }(\underline h+x)\in [(1-\delta _0)H^*, H^*], \ \ v_{c_\nu }(\underline h-x), v_{c_\nu }(\underline h+x)\in [(1-\delta _0)M^*, M^*]. \end{equation*}

Moreover, either $\underline h(t)-x\geq \underline h(t)$ or $\underline h(t)+x\geq \underline h(t)$ must hold, and hence

\begin{equation*} [H^*-u_{c_\nu }(\underline h-x)][M^*- v_{c_\nu }(\underline h+x)]\leq \delta _0 Ce^{ \hat \mu _1\underline h(t)}\leq \delta _0e^{ |\hat \mu _1|(1-L)}e^{ \hat \mu _1 c_\nu (t-T_*)}\leq \delta _0\epsilon (t) \end{equation*}

provided that $L$ is sufficiently large such that

(3.23) \begin{equation} e^{ |\hat \mu _1|(1-L)}\leq \tilde \epsilon. \end{equation}

Clearly, we also have

\begin{equation*} [H^*-u_{c_\nu }(\underline h+x)][M^*- v_{c_\nu }(\underline h-x)]\leq \delta _0 Ce^{ \hat \mu _1\underline h(t)}\leq \delta _0e^{ |\hat \mu _1|(1-L)}e^{ \hat \mu _1 c_\nu (t-T_*)}\leq \delta _0\epsilon (t). \end{equation*}

Thus, we can use Lemma 3.3 to obtain

\begin{equation*} A(x,t)\leq -\sigma _0\epsilon (t), \end{equation*}

where $\sigma _0\gt 0$ satisfies

\begin{equation*} \frac 12 (H^*, M^*)[\nabla F(H^*, M^*)]^\top \prec -(\sigma _0,\sigma _0). \end{equation*}

Since $B(x,t)\leq 0$ and $2\hat \mu _1 c_\nu \lt -\delta$ , we thus obtain, for $x\in I_3(t)$ and $t\geq T_*\gg 1$ ,

\begin{equation*}\begin {aligned} \delta H^*\epsilon (t)+Ce^{2 \hat \mu _1 \underline h(t)} +A(x,t)+B(x,t) &\leq C e^{2|\hat \mu _1|(1-L)}e^{2\hat \mu _1 c_\nu (t-T_*)}+(\delta H^*-\sigma _0) \epsilon (t)\\ &\leq \big [ C e^{2|\hat \mu _1|(1-L)}+(\delta H^*-\sigma _0)\tilde \epsilon \big ] e^{-\delta (t-T_*)}\lt 0 \end {aligned}\end{equation*}

provided that

(3.24) \begin{equation} C e^{2|\hat \mu _1|(1-L)}+(\delta H^*-\sigma _0) \tilde \epsilon \lt 0. \end{equation}

For $x\in I_1(t)$ and $t\geq T_*$ , with $0\lt \tilde \epsilon \ll 1$ , we have

\begin{equation*} B(x,t)\leq -\frac 12 \delta e^{-\delta (t-T_*)}\sigma _1, \end{equation*}

with

\begin{equation*} \sigma _1\,:\!=\inf _{s\in [0, K_0]}u^{\prime}_{c_\nu }(s)\gt 0, \end{equation*}

and

\begin{equation*} u_{c_\nu }(\underline h+x)=H^*+O(e^{\hat \mu _1 \underline h(t)}), \ v_{c_\nu }(\underline h+x)=M^*+O(e^{\hat \mu _1 \underline h(t)}), \end{equation*}

which imply

\begin{equation*} A(x,t)=O(\epsilon (t))+O(e^{\hat \mu _1 \underline h(t)}). \end{equation*}

Therefore, for some $\tilde C\gt 0$ , all $x\in I_1(t)$ and $t\geq T_*$ , with $0\lt \tilde \epsilon \ll 1$ , we have

\begin{equation*}\begin {aligned} &\delta H^*\epsilon (t)+Ce^{2 \hat \mu _1 \underline h(t)} +A(x,t)+B(x,t)\\ &\quad\leq \tilde C \left [e^{|\hat \mu _1|(1-L)}e^{\hat \mu _1 c_\nu (t-T_*)}+\tilde \epsilon e^{-\delta (t-T_*)t}\right ]-\frac {\sigma _1}2 \delta e^{-\delta (t-T_*)}\\ &\quad\leq \Big [\tilde Ce^{|\hat \mu _1|(1-L)}+\tilde C\tilde \epsilon -\frac {\sigma _1}2 \delta \Big ] e^{-\delta (t-T_*)}\lt 0 \end {aligned}\end{equation*}

provided that

(3.25) \begin{equation} \tilde Ce^{|\hat \mu _1|(1-L)}+\tilde C\tilde \epsilon -\frac{\sigma _1}2 \delta \lt 0. \end{equation}

By the symmetry of $A(x,t)$ and $B(x,t)$ in $x$ , we see that the above also hold for $x\in I_2(t)$ .

Let us note that, if we refine our choice of $\delta$ to

\begin{equation*} \delta \in (0, |\hat \mu _1|c_\nu )\cap (0, c_\nu )\cap (0, \sigma _0/H^*), \end{equation*}

then it is possible to take $L$ sufficiently large and $\tilde \epsilon \gt 0$ sufficiently small such that all the inequalities in (3.23), (3.22), (3.24) and (3.25) hold. Thus, for such $\tilde \epsilon$ and $L$ , (3.17) holds.

Moreover, by similar arguments we see that $\tilde \epsilon$ and $L$ can be chosen so that (3.18) holds simultaneously. Note that the value $T_*=T_*(L, \tilde \epsilon )$ is finalised only after the choice of $L$ and $\tilde \epsilon$ have been made.

Proof of Proposition 3.1. It follows from Lemmas 3.2 and 3.4 that

\begin{eqnarray*} \begin{aligned} \underline{h}(t)-c_\nu t\leq h(t)-c_\nu t\leq \overline{h}(t)-c_\nu t \end{aligned} \end{eqnarray*}

for $t\gt T=\!:\,\max \{T_\ast,T^\ast \}$ . Hence, there exists $C\gt 0$ such that

\begin{equation*}|h(t)-c_\nu t|\lt C\mbox { for all } t\gt 0.\end{equation*}

This implies, by considering the solution of (1.3) with initial function $(H_0(\!-x), M_0(\!-x))$ , that $|g(t)+c_\nu t|\lt C\mbox{ for all } t\gt 0$ . The proof of Proposition 3.1 is now complete.

4. Convergence

In this section, we prove Theorem 1.2. The crucial step is to show that $h(t)-c_\nu t\to h^\ast$ as $t\rightarrow \infty$ .

According to Proposition 3.1, there exists $C\gt 0$ such that

\begin{equation*}-C\lt h(t)-c_\nu t\lt C\mbox { for }t\gt 0.\end{equation*}

We now set

\begin{equation*}k(t)\,:\!=c_\nu t-2C,\ l(t)=h(t)-k(t)\end{equation*}

and denote

\begin{equation*}\phi (x,t)\,:\!=H(k(t)+x,t),\ \psi (x,t)\,:\!=M(k(t)+x,t).\end{equation*}

Obviously,

(4.1) \begin{equation} C\leq l(t)\leq 3C\mbox{ for }t\gt 0. \end{equation}

Moreover,

\begin{eqnarray*} \begin{aligned} &(H_x,M_x)=(\phi _x,\psi _x),\ (H_{xx},M_{xx})=(\phi _{xx},\psi _{xx}),\\ &(H_t,M_t)=(\phi _t-c_\nu \phi _x,\psi _t-c_\nu \psi _x) \end{aligned} \end{eqnarray*}

and $(\phi,\psi,l)$ satisfies

\begin{eqnarray*} \begin{cases} \phi _t=d_1 \phi _{xx}+c_\nu \phi _x+a_1(e_1-\phi )\psi -b_1\phi,&-k(t)\lt x\lt l(t),\quad t\gt 0,\\ \psi _t=d_2 \psi _{xx}+c_\nu \psi _x+a_2(e_2-\psi )\phi -b_2 \psi,&-k(t)\lt x\lt l(t),\quad t\gt 0,\\ \phi (l(t),t)=\psi (l(t),t)=0,&t\gt 0,\\ l^{\prime}(t)=-\nu \phi _{x}(l(t),t)-c_\nu,& t\gt 0. \end{cases} \end{eqnarray*}

4.1. Limit along a sequence $\boldsymbol{t}_\boldsymbol{n}\rightarrow \infty$

Let $\{t_n\}$ be a sequence satisfying $t_n\gt 0$ , $t_n\rightarrow \infty$ and $l(t_n)\to \liminf _{t\to +\infty }l(t)$ as $n\rightarrow \infty$ . Define

\begin{equation*}(k_n,l_n)(t)\,:\!=(k,l)(t+t_n),\ \ (\phi _n,\psi _n)(x,t)\,:\!=(\phi,\psi )(x,t+t_n).\end{equation*}

Lemma 4.1. Subject to a subsequence, as $n\rightarrow \infty$ ,

\begin{equation*}l_n\rightarrow L\mbox { in }C_{loc}^{1+\frac {\alpha }{2}}(\mathbb {R})\mbox { and }\|(\phi _n,\psi _n)-(\Phi,\Psi )\|_{\big [C_{loc}^{1+\alpha,\frac {1+\alpha }{2}}(\Omega )\big ]^2}\rightarrow 0,\end{equation*}

where $\alpha \in (0,1)$ , $\Omega \,:\!=\{(x,t):-\infty \lt x\lt L(t),t\in \mathbb{R}\}$ and $C_{loc}^{1+\alpha,\frac{1+\alpha }{2}}(\Omega )$ denotes the space of functions $\phi (x,t)$ which have bounded $(1+\alpha )$ -Hölder norm in $x$ and bounded $\frac{1+\alpha }{2}$ -Hölder norm in $t$ over any compact subset of $\Omega$ . Moreover, $(\Phi (x,t),\Psi (x,t),L(t))$ satisfies

(4.2) \begin{eqnarray} \begin{cases} \Phi _t=d_1 \Phi _{xx}+c_\nu \Phi _x+a_1(e_1-\Phi )\Psi -b_1\Phi,&(x,t)\in \Omega,\\ \Psi _t=d_2 \Psi _{xx}+c_\nu \Psi _x+a_2(e_2-\Psi )\Phi -b_2\Psi,&(x,t)\in \Omega,\\ \Phi (L(t),t)=\Psi (L(t),t)=0,&t\in \mathbb{R},\\ L^{\prime}(t)=-\nu \Phi _{x}(L(t),t)-c_\nu,\ L(t)\geq L(0),& t\in \mathbb{R}. \end{cases} \end{eqnarray}

Proof. It follows from Lemma 2.4 that there exists $C_0\gt 0$ such that $0\lt h^{\prime}(t)\leq C_0$ for $t\gt 0$ , which leads to

\begin{equation*}-c_\nu \lt l_n^{\prime}(t)\leq C_0-c_\nu \mbox { for } t\gt -t_n.\end{equation*}

Denote

\begin{equation*}\xi =\frac {x}{l_n(t)},\ (\tilde {\phi }_n,\tilde {\psi }_n)(\xi,t)=(\phi _n,\psi _n)(x,t).\end{equation*}

Then $(\tilde{\phi }_n(\xi,t),\tilde{\psi }_n(\xi,t),l_n(t))$ satisfies

(4.3) \begin{eqnarray} \begin{cases} \tilde{\phi }_{nt}=\dfrac{d_1}{l_n^2(t)} \tilde{\phi }_{n\xi \xi }+\dfrac{\xi l_n^{\prime}(t)+c_\nu }{l_n(t)} \tilde{\phi }_{n\xi }+a_1(e_1-\tilde{\phi }_n)\tilde{\psi }_n-b_1\tilde{\phi }_n,\\ \tilde{\psi }_{nt}=\dfrac{d_2}{l_n^2(t)} \tilde{\psi }_{n\xi \xi }+\dfrac{\xi l_n^{\prime}(t)+c_\nu }{l_n(t)} \tilde{\psi }_{n\xi }+a_2(e_2-\tilde{\psi }_n)\tilde{\phi }_n-b_2\tilde{\psi }_n \end{cases} \end{eqnarray}

for $-k_n(t)/l_n(t)\lt \xi \lt 1$ , $t\gt -t_n$ , and

(4.4) \begin{eqnarray} \begin{cases} \tilde{\phi }_n(1,t)=\tilde{\psi }_n(1,t)=0,\ t\gt -t_n,\\ l_n^{\prime}(t)=-\dfrac{\nu }{l_n(t)} \tilde{\phi }_{n\xi }(1,t)-c_\nu,\ t\gt -t_n. \end{cases} \end{eqnarray}

Owing to Lemma 2.4, $(H,M)(x,t)$ is uniformly bounded for $x\in [g(t),h(t)]$ and $t\in (0,\infty )$ , which implies that $(\phi _n,\psi _n)$ is uniformly bounded in $\{(x,t):-k_n(t)\lt x\lt l_n(t),t\geq -t_n\}$ . Hence, in view of (4.1), for any given $R\gt 0$ and $T\in \mathbb{R}$ , using the interior-boundary $L^p$ estimates to (4.3) and (4.4) over $[\!-R-2,1]\times [T-2,T+1]$ , for any $p\gt 1$ we have

\begin{equation*}\|(\tilde {\phi }_n,\tilde {\psi }_n)\|_{W_p^{2,1}([\!-R-1,1]\times [T-1,T+1])}\leq C_R \mbox { for all large }n,\end{equation*}

where $C_R$ is a constant depending on $R$ and $p$ but independent of $n$ and $T$ . Furthermore, for any $\alpha ^{\prime}\in (0,1)$ , we can take $p\gt 1$ large enough and use the Sobolev embedding theorem to obtain

(4.5) \begin{eqnarray} \|(\tilde{\phi }_n,\tilde{\psi }_n)\|_{C^{1+\alpha ^{\prime},\frac{1+\alpha ^{\prime}}{2}}([\!-R,1]\times [T,\infty ))}\leq \tilde{C}_R \mbox{ for all large }n, \end{eqnarray}

where $\tilde{C}_R$ is a constant depending on $R$ and $\alpha ^{\prime}$ but independent of $n$ and $T$ . From (4.4) and (4.5), we conclude

\begin{equation*}\|l_n\|_{C^{1+\frac {\alpha ^{\prime}}{2}}([T,\infty ))}\leq \tilde {C}_1\mbox { for all large }n,\end{equation*}

where $\tilde{C}_1$ is a constant depending on $R$ and $\alpha ^{\prime}$ but independent of $n$ and $T$ too. Hence by passing to a subsequence, still denoted by itself, we have, for some $\alpha \in (0,\alpha ^{\prime})$ ,

\begin{equation*}(\tilde {\phi }_n,\tilde {\psi }_n)\rightarrow (\tilde {\Phi },\tilde {\Psi })\mbox { in } \left (C_{loc}^{1+\alpha,\frac {1+\alpha }{2}}((\!-\infty,1]\times \mathbb {R})\right )^2,\ l_n\rightarrow L \mbox { in }C_{loc}^{1+\frac {\alpha }{2}}(\mathbb {R}).\end{equation*}

Now, applying standard regularity theory to (4.3)–(4.4), we see that $(\tilde{\Phi },\tilde{\Psi },L)$ satisfies the following equations in the classical sense:

\begin{eqnarray*} \begin{cases} \tilde{\Phi }_{t}=\dfrac{d_1}{L^2(t)} \tilde{\Phi }_{\xi \xi }+\dfrac{\xi L^{\prime}(t)+c_\nu }{L(t)} \tilde{\Phi }_{\xi }+a_1(e_1-\tilde{\Phi })\tilde{\Psi }-b_1\tilde{\Phi },&\xi \in (\!-\infty,1],\ t\in \mathbb{R},\\ \tilde{\Psi }_{t}=\dfrac{d_2}{L^2(t)} \tilde{\Psi }_{\xi \xi }+\dfrac{\xi L^{\prime}(t)+c_\nu }{L(t)} \tilde{\Psi }_{\xi }+a_2(e_2-\tilde{\Psi })\tilde{\Phi }-b_2\tilde{\Psi },&\xi \in (\!-\infty,1],\ t\in \mathbb{R},\\ \tilde{\Phi }(1,t)=\tilde{\Psi }(1,t)=0,&t\in \mathbb{R},\\ L^{\prime}(t)=-\dfrac{\nu }{L(t)} \tilde{\Phi }_{\xi }(1,t)-c_\nu,& t\in \mathbb{R}. \end{cases} \end{eqnarray*}

By setting $(\Phi,\Psi )(x,t)=(\tilde{\Phi },\tilde{\Psi })(x/L(t),t)$ , it is easy to verify that $(\Phi,\Psi,L)$ satisfies (4.2) and

\begin{equation*}\lim _{n\rightarrow \infty }\|(\Phi,\Psi )-(\phi _n,\psi _n)\|_{\big [C_{loc}^{1+\alpha,\frac {1+\alpha }{2}}(\Omega )\big ]^2}=0.\end{equation*}

Finally, since $L(0)=\lim _{n\to \infty }l(t_n)=\liminf _{t\to \infty }l(t)$ and $L(t)=\lim _{n\to \infty }l(t_n+t)$ , clearly $L(t)\geq L(0)$ for any $t\in \mathbb R$ . This completes the proof.

4.2. Determine the limit pair $(\Phi,\Psi,\boldsymbol{L})$

We show that

\begin{equation*}\mbox {$L(t)\equiv L(0)$ and $(\Phi,\Psi )(x,t)\equiv (u_{c_\nu },v_{c_\nu })(L(0)-x)$.} \end{equation*}

Due to (4.1), we have

\begin{equation*}C\leq L(t)\leq 3C\mbox { for }t\in \mathbb {R}.\end{equation*}

It follows from Lemma 3.4 that, for $x\in [\!-\underline h(t+t_n)-k(t+t_n), \underline h(t+t_n)-k(t+t_n)]$ and $t+t_n\geq T_*$ ,

(4.6) \begin{eqnarray} \begin{cases} \phi _n(x,t)\geq (1-\tilde \epsilon \,e^{-\delta (t+t_n-T_*)})\hat \phi _n(x,t),\\ \psi _n(x,t)\geq (1-\tilde \epsilon \, e^{-\delta (t+t_n-T_*)})\hat \psi _n(x,t), \end{cases} \end{eqnarray}

where

\begin{align*} \begin{cases} \hat \phi _n(x,t)\,:\!=u_{c_\nu }(\underline{h}(t+t_n)-k(t+t_n)-x)+u_{c_\nu }(\underline{h}(t+t_n)+k(t+t_n)+x)-u_{c_\nu }(2\underline{h}(t+t_n)),\\ \hat \psi _n(x,t)\,:\!=v_{c_\nu }(\underline{h}(t+t_n)-k(t+t_n)-x)+v_{c_\nu }(\underline{h}(t+t_n)+k(t+t_n)+x)-v_{c_\nu }(2\underline{h}(t+t_n)). \end{cases} \end{align*}

It is easily seen that there exists $C_0\in \mathbb R$ such that $\underline{h}(t+t_n)-k(t+t_n)\geq C_0$ for $t+t_n\geq T_*$ . Moreover,

\begin{equation*} \underline {h}(t+t_n)+k(t+t_n)\to \infty \mbox { and } \underline {h}(t+t_n)\to +\infty \mbox { as } n\to \infty. \end{equation*}

It follows that, for $x\leq C_0\leq L(t)$ and $t\in \mathbb R$ ,

\begin{equation*} \liminf _{n\to \infty }\hat \phi _n(x,t)\geq u_{c_\nu }(C_0-x),\ \liminf _{n\to \infty }\hat \psi _n(x,t)\geq v_{c_\nu }(C_0-x). \end{equation*}

Hence, letting $n\rightarrow \infty$ in (4.6) we obtain

(4.7) \begin{eqnarray} (\Phi,\Psi )(x,t)\succeq (u_{c_\nu },v_{c_\nu })(C_0-x)\mbox{ for }x\leq C_0,\ t\in \mathbb{R}. \end{eqnarray}

Now we define

\begin{equation*}R^\ast \,:\!=\sup \{R:(\Phi,\Psi )(x,t)\succeq \left ( u_{c_\nu },v_{c_\nu }\right )(R-x)\mbox { for }(x,t)\in (\!-\infty, R]\times \mathbb R\}.\end{equation*}

Thanks to (4.7) and $(\Phi,\Psi )(L(t),t)=(0, 0)$ with $L(t)\in [C, 3C]$ , we see that $R^\ast$ is finite. Moreover,

\begin{equation*}(\Phi,\Psi )(x,t)\succeq \left ( u_{c_\nu },v_{c_\nu }\right )(R^\ast -x)\mbox { for }(x,t)\in (\!-\infty, R^*]\times \mathbb R\end{equation*}

and

\begin{equation*}\inf _{t\in \mathbb {R}}L(t)=L(0) \geq R^\ast .\end{equation*}

Lemma 4.2. $R^\ast =L(0)$ .

Proof. On the contrary, suppose $R^\ast \lt L(0)=\min _{t\in \mathbb{R}}L(t)$ .

Step 1. We show that

(4.8) \begin{eqnarray} (\Phi,\Psi )(x,t)\succ \left ( u_{c_\nu },v_{c_\nu }\right )(R^\ast -x)\mbox{ for } (x,t)\in (\!-\infty, R^*]\times \mathbb R. \end{eqnarray}

Otherwise, there exists $(x_0,t_0)\in (\!-\infty, R^*)\times \mathbb R$ such that

\begin{equation*}u_{c_\nu }(R^\ast -x_0)=\Phi (x_0,t_0)\gt 0\mbox { or } v_{c_\nu }(R^\ast -x_0)=\Psi (x_0,t_0)\gt 0.\end{equation*}

Observe that $\left ( u_{c_\nu },v_{c_\nu }\right )(R^\ast -x)$ satisfies the first two equations in (4.2) for $(x,t)\in (\!-\infty, R^*)\times \mathbb R$ , and we already know $(\Phi,\Psi )(x,t)\succeq \left ( u_{c_\nu },v_{c_\nu }\right )(R^\ast -x)$ for such $(x,t)$ . Without loss of generality, we assume $\Phi (x_0,t_0)=u_{c_\nu }(R^\ast -x_0)$ . Set $\varpi (x,t)=u_{c_\nu }(R^\ast -x)-\Phi (x,t)$ and take $\overline{K}\geq a_1M^\ast$ . Then, $\varpi (x,t)\leq 0$ in $(\!-\infty, R^*)\times \mathbb R$ and

\begin{eqnarray*} \begin{aligned} &\varpi _t-d_1\varpi _{xx}-c_\nu \varpi +(b_1+\overline{K})\varpi \\ &\quad=\ a_1(e_1-u_{c_\nu }(R^\ast -x))v_{c_\nu }(R^\ast -x)-a_1(e_1-\Phi )\Psi +\overline{K}\varpi \\ &\quad\leq \ a_1(e_1-u_{c_\nu }(R^\ast -x))v_{c_\nu }(R^\ast -x)-a_1(e_1-\Phi )v_{c_\nu }(R^\ast -x)+\overline{K}\varpi \\ &\quad=-a_1\varpi v_{c_\nu }(R^\ast -x)+\overline{K}\varpi \leq 0. \end{aligned} \end{eqnarray*}

Since $\varpi (x_0,t_0)=0$ , the strong maximum principle implies that $\varpi (x,t)\equiv 0$ for $(x,t)\in (\!-\infty, R^*)\times \mathbb R$ . But this is impossible since

\begin{equation*} \varpi (R^*,t_0)=u_{c_\nu }(0)-\Phi (R^*,t_0)\lt 0 \mbox { due to } L(t_0)\gt R^*. \end{equation*}

Thus, (4.8) holds.

Step 2. We prove that, for any $x\leq R^*$ ,

(4.9) \begin{eqnarray} \begin{cases} \omega _1(x)=\sup _{y\in [x, R^*], t\in \mathbb{R}}[u_{c_\nu }(R^\ast -y)-\Phi (y,t)]\lt 0,\\ \omega _2(x)=\sup _{y\in [x, R^*], t\in \mathbb{R}}[v_{c_\nu }(R^\ast -y)-\Psi (y,t)]\lt 0. \end{cases} \end{eqnarray}

Obviously, $\omega _i(x)\leq 0$ for $i=1,2$ and $x\leq R^*$ . If (4.9) does not hold, then there exists $x_0\in (\!-\infty,R^\ast )$ such that

\begin{equation*}\omega _1(x_0)=0\mbox { or }\omega _2(x_0)=0.\end{equation*}

As a consequence of Step 1, we see that in (4.9), $\omega _i(x_0)$ is not achieved by any $(y,t)\in [x_0, R^*]\times \mathbb R$ . Therefore, there exists a sequence $\{(y_n,s_n)\}\subset [x_0, R^*]\times \mathbb{R}$ with $|s_n|\rightarrow \infty$ such that

\begin{equation*}\lim _{n\rightarrow \infty }\big [\Phi (y_n,s_n)-u_{c_\nu }(R^\ast -y_n)\big ]=0 \mbox { or } \lim _{n\rightarrow \infty }\big [\Psi (y_n,s_n)-v_{c_\nu }(R^\ast -y_n)\big ]=0.\end{equation*}

By passing to a subsequence, we may assume that $\lim _{n\to \infty }y_n=y_0\in [x_0, R^*]$ . Set

\begin{equation*}(\Phi _n(x,t),\Psi _n(x,t),L_n(t))=(\Phi (x+y_n,t+s_n),\Psi (x+y_n,t+s_n),L(t+s_n)).\end{equation*}

Then repeating the same argument used in the proof of Lemma 4.1 and passing to a subsequence if necessary, we may assume that, for $\alpha \in (0,1)$ ,

\begin{equation*}(\Phi _n,\Psi _n,L_n)\rightarrow \left(\widetilde {\Phi },\widetilde {\Psi },\widetilde {L}\right)\mbox { in }\left (C_{loc}^{1+\alpha,\frac {1+\alpha }{2}}(\widetilde {\Omega })\right )^2\times C_{loc}^{1+\frac {\alpha }{2}}(\mathbb {R})\end{equation*}

with $\widetilde{\Omega }=\{(t,x):x\lt \tilde L(t),\ t\in \mathbb R\}$ , and $\left(\widetilde{\Phi },\widetilde{\Psi },\widetilde{L}\right)$ satisfies

(4.10) \begin{eqnarray} \begin{cases} \widetilde{\Phi }_t=d_1 \widetilde{\Phi }_{xx}+c_\nu \widetilde{\Phi }_x+a_1(e_1-\widetilde{\Phi })\widetilde{\Psi }-b_1\widetilde{\Phi },&-\infty \lt x\lt \widetilde{L}(t),\ t\in \mathbb{R},\\ \widetilde{\Psi }_t=d_2 \widetilde{\Psi }_{xx}+c_\nu \widetilde{\Psi }_x+a_2(e_2-\widetilde{\Psi })\widetilde{\Phi }-b_2\widetilde{\Psi },&-\infty \lt x\lt \widetilde{L}(t),\ t\in \mathbb{R},\\ \widetilde{\Phi }(\widetilde{L}(t),t)=\widetilde{\Psi }(\widetilde{L}(t),t)=0,&t\in \mathbb{R}. \end{cases} \end{eqnarray}

Moreover, for $-\infty \lt x\lt R^*-y_0,\ t\in \mathbb{R}$ ,

\begin{eqnarray*} \left(\widetilde{\Phi },\widetilde{\Psi }\right)(x,t)\succeq \left ( u_{c_\nu },v_{c_\nu }\right )(R^\ast -y_0-x),\ \widetilde{L}(t)+y_0\geq L(0)\gt R^\ast, \end{eqnarray*}

and

\begin{eqnarray*} \widetilde{\Phi }(0,0)=u_{c_\nu }(R^\ast -y_0) \mbox{ or }\widetilde{\Psi }(0,0)=v_{c_\nu }(R^\ast -y_0). \end{eqnarray*}

Since $(u_{c_\nu },v_{c_\nu })(R^\ast -y_0-x)$ satisfies (4.10) with $\widetilde{L}$ replaced by $R^\ast -y_0$ and (4.10) is a cooperative system, repeating the same argument as in Step 1 and applying the strong maximum principle we can conclude that $\widetilde{\Phi }(x,t)\equiv u_{c_\nu }(R^\ast -y_0-x)$ or $\widetilde{\Psi }(x,t)\equiv v_{c_\nu }(R^\ast -y_0-x)$ for $x\lt R^*-y_0$ with $t\leq 0$ . It follows that $\widetilde{\Phi }(R^*-y_0,0)=0$ or $\widetilde{\Psi }(R^*-y_0,0)=0$ , which is impossible since $\tilde L(0)\gt R^*-y_0$ .

Step 3. Completion of the proof.

In view of $(u_{c_\nu },v_{c_\nu })(R^\ast -x)\rightarrow (H^\ast,M^\ast )$ as $x\rightarrow -\infty$ , for any small $\epsilon _0\gt 0$ we can find $R_0=R_0(\epsilon _0)\lt R^*$ large negative such that

\begin{equation*}\left ( u_{c_\nu },v_{c_\nu }\right )(R^\ast -x)\succeq (H^\ast -\epsilon _0,M^\ast -\epsilon _0)\mbox { for }x\leq R_0.\end{equation*}

Then choose $\epsilon \in (0,\epsilon _0)$ such that

\begin{equation*}\left ( u_{c_\nu },v_{c_\nu }\right )(R^\ast -R_0+\epsilon ) \preceq (u_{c_\nu },v_{c_\nu })(R^\ast -R_0)-(\omega _1,\omega _2)(R_0),\end{equation*}

where $\omega _i,i=1,2$ are defined in (4.9).

Consider an auxiliary problem:

(4.11) \begin{eqnarray} \begin{cases} \overline{\Phi }_t=d_1 \overline{\Phi }_{xx}+c_\nu \overline{\Phi }_x+a_1(e_1-\overline{\Phi })\overline{\Psi }-b_1\overline{\Phi },&x\lt R_0,t\gt 0,\\ \overline{\Psi }_t=d_2 \overline{\Psi }_{xx}+c_\nu \overline{\Psi }_x+a_2(e_2-\overline{\Psi })\overline{\Phi }-b_2\overline{\Psi },&x\lt R_0,t\gt 0,\\ \left(\overline{\Phi },\overline{\Psi }\right)(R_0,t)=(u_{c_\nu },v_{c_\nu })(R^\ast -R_0+\epsilon ),&t\gt 0,\\ \left(\overline{\Phi },\overline{\Psi }\right)(x,0)=\left ( u_{c_\nu },v_{c_\nu }\right )(R^\ast -x),&x\lt R_0. \end{cases} \end{eqnarray}

Obviously, $(H^\ast,M^\ast )$ and $(u_{c_\nu },v_{c_\nu })(R^\ast -x)$ are a pair of upper and lower solutions of (4.11). It follows from the comparison principle that

(4.12) \begin{eqnarray} (u_{c_\nu },v_{c_\nu })(R^\ast -x)\preceq \left(\overline{\Phi },\overline{\Psi }\right)(x,t)\preceq (H^\ast,M^\ast ) \end{eqnarray}

for all $x\lt R_0$ and $t\gt 0$ . Moreover, $\left(\overline{\Phi },\overline{\Psi }\right)(x,t)$ is non-decreasing in $t$ and

\begin{equation*}\lim _{t\rightarrow \infty }\left(\overline {\Phi },\overline {\Psi }\right)(x,t)=(\Phi ^\ast,\Psi ^\ast )(x)\mbox { for }x\lt R_0,\end{equation*}

where $(\Phi ^\ast,\Psi ^\ast )$ satisfies

(4.13) \begin{eqnarray} \begin{cases} d_1 \Phi _{xx}^\ast +c_\nu \Phi _x^\ast +a_1(e_1-\Phi ^\ast )\Psi ^\ast -b_1\Phi ^\ast =0,&-\infty \lt x\lt R_0,\\ d_2 \Psi _{xx}^\ast +c_\nu \Psi _x^\ast +a_2(e_2-\Psi ^\ast )\Phi ^\ast -b_2\Psi ^\ast =0,&-\infty \lt x\lt R_0,\\ (\Phi ^\ast,\Psi ^\ast )(\!-\infty )=(H^\ast,M^\ast ),&\\ (\Phi ^\ast,\Psi ^\ast )(R_0)=(u_{c_\nu },v_{c_\nu })(R^\ast -R_0+\epsilon ). \end{cases} \end{eqnarray}

Clearly,

\begin{equation*}(\hat {u}_{c_\nu },\hat {v}_{c_\nu })(x)\,:\!=(u_{c_\nu },v_{c_\nu })(R^\ast -x+\epsilon )\end{equation*}

also satisfies (4.13), and due to $(u_{c_\nu },v_{c_\nu })(R^\ast -x+\epsilon )\succeq (u_{c_\nu },v_{c_\nu })(R^\ast -x)$ , we can apply the comparison principle to (4.11) to deduce

\begin{equation*} (u_{c_\nu },v_{c_\nu })(R^\ast -x+\epsilon )\succeq \left(\overline {\Phi },\overline {\Psi }\right)(x,t) \mbox { for } x\lt R_0,\ t\gt 0. \end{equation*}

Letting $t\to \infty$ , we obtain

\begin{equation*}(\hat {u}_{c_\nu },\hat {v}_{c_\nu })(x)\succeq (\Phi ^\ast,\Psi ^\ast )(x)\mbox { for }-\infty \lt x\leq R_0.\end{equation*}

Let us also note that from (4.12), we have

(4.14) \begin{eqnarray} (\Phi ^*(x), \Psi ^*(x))\succeq \left ( u_{c_\nu },v_{c_\nu }\right )(R^\ast -x)\succ (H^\ast -\epsilon _0,M^\ast -\epsilon _0)\mbox{ for }x\leq R_0. \end{eqnarray}

In what follows, we prove that

(4.15) \begin{eqnarray} (\hat{u}_{c_\nu },\hat{v}_{c_\nu })(x)=(\Phi ^\ast,\Psi ^\ast )(x)\mbox{ for }-\infty \lt x\leq R_0. \end{eqnarray}

To this end, let us denote

\begin{equation*}\left(\widehat {\Phi },\widehat {\Psi }\right)(x)=(\Phi ^\ast,\Psi ^\ast )(x)-(\hat {u}_{c_\nu },\hat {v}_{c_\nu })(x).\end{equation*}

Then, $\left(\widehat{\Phi },\widehat{\Psi }\right)$ satisfies

(4.16) \begin{eqnarray} \begin{cases} d_1 \widehat{\Phi }_{xx}+c_\nu \widehat{\Phi }_x=(b_1+a_1\Psi ^\ast )\widehat{\Phi }-a_1(e_1-\hat{u}_{c_\nu })\widehat{\Psi },&-\infty \lt x\lt R_0,\\ d_2 \widehat{\Psi }_{xx}+c_\nu \widehat{\Psi }_x=(b_2+a_2\Phi ^\ast )\widehat{\Psi }-a_2(e_2-\hat{v}_{c_\nu })\widehat{\Phi },&-\infty \lt x\lt R_0 \end{cases} \end{eqnarray}

and

(4.17) \begin{eqnarray} \left(\widehat{\Phi },\widehat{\Psi }\right)(\!-\infty )=\left(\widehat{\Phi },\widehat{\Psi }\right)(R_0)=(0,0). \end{eqnarray}

Since $\left(\widehat{\Phi },\widehat{\Psi }\right)\preceq (0,0)$ for $x\lt R_0$ and (4.17) holds true, there exist $\zeta _1,\zeta _2\in \mathbb R$ such that

\begin{equation*}\widehat {\Phi }(\zeta _1)=\min _{x\in (\!-\infty,R_0]}\widehat {\Phi }(x),\ \widehat {\Psi }(\zeta _2)=\min _{x\in (\!-\infty,R_0]}\widehat {\Psi }(x).\end{equation*}

Then, (4.15) is equivalent to

\begin{equation*}\widehat {\Phi }(\zeta _1)=\widehat {\Psi }(\zeta _2)=0.\end{equation*}

Suppose $\widehat{\Phi }(\zeta _1)\lt 0$ . We can obtain a contradiction by distinguishing the following two cases:

1. (i) $[b_1+a_1(M^\ast -\epsilon _0)]\widehat{\Phi }(\zeta _1)-a_1(e_1-H^\ast +\epsilon _0)\widehat{\Psi }(\zeta _2)\lt 0$ ;

2. (ii) $[b_1+a_1(M^\ast -\epsilon _0)]\widehat{\Phi }(\zeta _1)-a_1(e_1-H^\ast +\epsilon _0)\widehat{\Psi }(\zeta _2)\geq 0$ .

When case (i) happens, in view of (4.14) and $\left(\widehat{\Phi },\widehat{\Psi }\right)(x)\preceq (0,0)$ for $x\lt R_0$ , one can use the equation for $\widehat{\Phi }$ in (4.16) to deduce

\begin{eqnarray*} \begin{aligned} 0\leq &\ d_1 \widehat{\Phi }_{xx}(\zeta _1)+c_\nu \widehat{\Phi }_x(\zeta _1)\\ \leq &\ [b_1+a_1(M^\ast -\epsilon _0)]\widehat{\Phi }(\zeta _1)-a_1(e_1-H^\ast +\epsilon _0)\widehat{\Psi }(\zeta _1)\\ \leq &\ [b_1+a_1(M^\ast -\epsilon _0)]\widehat{\Phi }(\zeta _1)-a_1(e_1-H^\ast +\epsilon _0)\widehat{\Psi }(\zeta _2)\lt 0, \end{aligned} \end{eqnarray*}

which is a contradiction, and hence case (i) is impossible.

If case (ii) happens, one can use (4.14) and the equation of $\widehat{\Psi }$ in (4.16) to deduce

\begin{eqnarray*} \begin{aligned} 0\leq &\ d_2 \widehat{\Psi }_{xx}(\zeta _2)+c_\nu \widehat{\Psi }_x(\zeta _2)\\ \leq &\ [b_2+a_2(H^\ast -\epsilon _0)]\widehat{\Psi }(\zeta _2)-a_2(e_2-M^\ast +\epsilon _0)\widehat{\Phi }(\zeta _2)\\ \leq &\ [b_2+a_2(H^\ast -\epsilon _0)]\widehat{\Psi }(\zeta _2)-a_2(e_2-M^\ast +\epsilon _0)\widehat{\Phi }(\zeta _1)\\ =&\ \frac{\widehat{\Phi }(\zeta _1)}{a_1(e_1-H^\ast +\epsilon _0)}\Big \{\frac{a_1(e_1-H^\ast +\epsilon _0)}{\widehat{\Phi }(\zeta _1)}\widehat{\Psi }(\zeta _2) [b_2+a_2(H^\ast -2\epsilon _0)]\\ &\ \def\lumina\hspace{3cm} -{a_1(e_1-H^\ast +\epsilon _0)}a_2(e_2-M^\ast +\epsilon _0)\Big \}\\ \leq &\ \frac{\widehat{\Phi }(\zeta _1)}{a_1(e_1-H^\ast +\epsilon _0)} A(\epsilon _0)\ (\mbox{due to the assumption in case (ii)}), \end{aligned} \end{eqnarray*}

where

\begin{equation*} A(\epsilon _0)\,:\!=[b_1+a_1(M^\ast -\epsilon _0)][b_2+a_2(H^\ast -\epsilon _0)] -a_1(e_1-H^\ast +\epsilon _0)a_2(e_2-M^\ast +\epsilon _0). \end{equation*}

From $\mathcal R_0\gt 1$ , we easily see by direct computation that $A(0)=a_1a_2e_1e_2-b_1b_2\gt 0$ . Therefore, by the continuity of $A(\epsilon _0)$ with respect to $\epsilon _0$ , we have $A(\epsilon _0)\gt 0$ by taking $\epsilon _0\gt 0$ small enough, which yields

\begin{equation*} \frac {\widehat {\Phi }(\zeta _1)}{a_1(e_1-H^\ast +\epsilon )} A(\epsilon _0)\lt 0\mbox { for such }\epsilon _0\gt 0. \end{equation*}

Again, we arrive at a contradiction. Therefore, $\widehat{\Phi }(\zeta _1)=0$ , or equivalently, $\widehat{\Phi }(x)=0$ for $x\lt R_0$ . Similarly, we can prove $\widehat{\Psi }(x)=0$ for $x\lt R_0$ by repeating the above arguments. Thus, (4.15) holds.

We are now ready to reach a contradiction by considering $(\Phi,\Psi )(x,t)$ , which satisfies the first two equations in (4.11). Moreover, for any $t\in \mathbb{R}$ and $x\leq R^*$ ,

\begin{align*} &(\Phi,\Psi )(x,t)\succeq \left ( u_{c_\nu },v_{c_\nu }\right )(R^\ast -x),\\ &\Phi (R_0,t)\succeq u_{c_\nu }(R^\ast -R_0)-\omega _1(R_0)\succeq u_{c_\nu }(R^\ast -R_0+\epsilon ),\\ &\Psi (R_0,t)\succeq v_{c_\nu }(R^\ast -R_0)-\omega _2(R_0)\succeq v_{c_\nu }(R^\ast -R_0+\epsilon ). \end{align*}

Therefore, we can use the comparison principle to deduce that

\begin{equation*}(\Phi,\Psi )(x,t+s)\succeq \left(\overline {\Phi },\overline {\Psi }\right)(x,t)\mbox { for all }t\gt 0,\ x\lt R_0,\ s\in \mathbb {R},\end{equation*}

which is equivalent to

\begin{equation*}(\Phi,\Psi )(x,t)\succeq \left(\overline {\Phi },\overline {\Psi }\right)(x,t-s)\mbox { for all }\ t\gt s,\ x\lt R_0,\ s\in \mathbb {R}.\end{equation*}

Letting $s\rightarrow -\infty$ , due to (4.15) we obtain

(4.18) \begin{eqnarray} (\Phi,\Psi )(x,t)\succeq (\Phi ^\ast,\Psi ^\ast )(x)=(u_{c_\nu },v_{c_\nu })(R^\ast -x+\epsilon ) \mbox{ for $x\lt R_0$ and $t\in \mathbb{R}$}. \end{eqnarray}

By Step 2,

\begin{equation*} \varepsilon \,:\!=\min \{-\omega _1(R_0), -\omega _2(R_0)\}\gt 0.\end{equation*}

Taking $\epsilon _1\in (0,\epsilon ]$ small enough, we have, for $x\in [R_0,R^\ast +\epsilon _1]$ ,

\begin{equation*}(u_{c_\nu },v_{c_\nu })(R^\ast -x+\epsilon _1)\preceq (u_{c_\nu },v_{c_\nu })(R^\ast -x)+(\varepsilon,\varepsilon ).\end{equation*}

Hence, for $x\in [R_0,R^\ast +\epsilon _1]$ and $t\in \mathbb{R}$ ,

\begin{equation*}(\Phi,\Psi )(x,t)-(u_{c_\nu },v_{c_\nu })(R^\ast -x+\epsilon _1)\succeq -(\varepsilon,\varepsilon ) -(\omega _1,\omega _2)(R_0)\succeq (0,0).\end{equation*}

Combining this with (4.18), we obtain

\begin{eqnarray*} \begin{aligned} (\Phi,\Psi )(x,t)-(u_{c_\nu },v_{c_\nu })(R^\ast -x+\epsilon _1)\succeq (0,0)\mbox{ for }x\leq R^\ast +\epsilon _1,\ t\in \mathbb{R} \end{aligned} \end{eqnarray*}

for all small $\epsilon _1\in (0,\epsilon )$ , which contradicts the definition of $R^\ast$ . This completes the proof.

Proposition 4.3. $(\Phi,\Psi )(x,t)\equiv (u_{c_\nu },v_{c_\nu })(R^\ast -x)$ and $L(t)\equiv R^*$ .

Proof. We already know that $R^*=L(0)=\min L(t)$ and

\begin{equation*}(\Phi,\Psi )(x,t)\succeq (u_{c_\nu },v_{c_\nu })(R^\ast -x)\mbox { for }x\leq R^*\mbox { and }t\in \mathbb {R}\end{equation*}

with

\begin{equation*}(\Phi,\Psi )(L(0), 0)=(u_{c_\nu },v_{c_\nu })(R^\ast -L(0))=(0,0).\end{equation*}

It follows from the strong maximum principle for cooperative systems and the Hopf boundary lemma that

\begin{equation*} (\Phi _x,\Psi _x)(L(0), 0)\prec -\left(u^{\prime}_{c_\nu },v^{\prime}_{c_\nu }\right)(0) \mbox { unless } (\Phi,\Psi )(x,t)\equiv (u_{c_\nu },v_{c_\nu })(R^\ast -x). \end{equation*}

On the other hand, $L^{\prime}(0)=0$ implies, by the last identity in (4.2),

\begin{equation*} \Phi _x(L(0), 0)=-u^{\prime}_{c_\nu }(0). \end{equation*}

Thus, we must have $(\Phi,\Psi )(x,t)\equiv (u_{c_\nu },v_{c_\nu })(R^\ast -x)$ , which implies $L(t)\equiv L(0)$ .

4.3. Proof of Theorem 1.2

We are now ready to complete the proof of Theorem 1.2. For clarity, we achieve this goal by first proving two claims.

Claim 1: Let $\{t_n\}$ be the sequence in Lemma 4.1 . Then for every $t\in \mathbb R$ , $\lim _{n\rightarrow \infty }h^{\prime}(t+t_n)=c_\nu$ . Moreover, (1.8) holds along $t=t_n$ .

It follows from Lemma 4.1 and Proposition 4.3 that $h(t+t_n)-k(t+t_n)\rightarrow L(0)=R^*$ in $C_{loc}^{1+\frac{\alpha }{2}}(\mathbb{R})$ . Hence, $h^{\prime}(t+t_n)\rightarrow c_\nu$ in $C_{loc}^{\frac{\alpha }{2}}(\mathbb{R}).$ It then follows easily from Lemma 4.1 and Proposition 4.3 that

\begin{equation*}(H,M)(x+h(t+t_n),t+t_n)\rightarrow (u_{c_\nu },v_{c_\nu })(\!-x)\mbox { in }\left (C_{loc}^{1+\alpha,\frac {1+\alpha }{2}}((\!-\infty,0]\times \mathbb {R})\right )^2 \mbox { as }n\rightarrow \infty .\end{equation*}

Hence, for any $L_0\gt 0$ ,

\begin{eqnarray*} \lim _{n\rightarrow \infty }\|(H,M)(\cdot,t_n)-(u_{c_\nu },v_{c_\nu })(h(t_n)-\cdot )\|_{L^\infty ([h(t_n)-L_0,h(t_n)])}=0. \end{eqnarray*}

On the other hand, for any given small $\epsilon \gt 0$ , by Lemmas 3.2 and 3.4, there exists $L_1\gt 0$ and some large integer $N\geq 1$ such that

\begin{equation*} (H^*-\epsilon, M^*-\epsilon )\preceq (H,M)(x,t_n)\preceq (H^*+\epsilon, M^*+\epsilon ) \mbox { for } x\in [0, h(t_n)-L_1],\, n\geq N. \end{equation*}

Clearly, for $L_2\gt 0$ large,

\begin{equation*} (H^*-\epsilon, M^*-\epsilon )\preceq (u_{c_\nu },v_{c_\nu })(h(t_n)-x)\preceq (H^*, M^*) \mbox { for } x\in (\!-\infty, h(t_n)-L_2]. \end{equation*}

Therefore, if we take $L_0=\max \{L_1, L_2\}$ , then for $n\geq N$ ,

\begin{equation*} \|(H,M)(\cdot,t_n)-(u_{c_\nu },v_{c_\nu })(h(t_n)-\cdot )\|_{L^\infty ([0, h(t_n)-L_0])}\leq 2\epsilon. \end{equation*}

It follows that

(4.19) \begin{eqnarray} \lim _{n\rightarrow \infty }\|(H,M)(\cdot,t_n)-(u_{c_\nu },v_{c_\nu })(h(t_n)-\cdot )\|_{L^\infty ([0,h(t_n)])}=0. \end{eqnarray}

Consider (1.3) with initial function $(H_0(\!-x), M_0(\!-x))$ , the above proved conclusions imply that

(4.20) \begin{eqnarray} \lim _{n\rightarrow \infty }\|(H,M)(\cdot,t_n)-(u_{c_\nu },v_{c_\nu })(\!\cdot -g(t_n))\|_{L^\infty ([g(t_n),0])}=0. \end{eqnarray}

Claim 2: $\lim _{t\rightarrow \infty }(h(t)-c_\nu t)=h^*\,:\!=R^\ast -2C=L(0)-2C$ .

By Claim 1, along a sequence $\{t_n\}$ satisfying

\begin{equation*} \lim _{n\rightarrow \infty }[h(t_n)-c_\nu t_n+2C] =\liminf _{t\to \infty }[h(t)-c_\nu t+2C]=R^*, \end{equation*}

(4.19) holds and

(4.21) \begin{equation} \lim _{n\to \infty } [h(t_n)-c_\nu t_n]=h^*,\; \lim _{n\to \infty } h^{\prime}(t_n)=c_\nu. \end{equation}

Let us note that

\begin{equation*} \lim _{n\rightarrow \infty }(h(t_n)-c_\nu t_n)=h^*=\liminf _{t\to \infty } (h(t)-c_\nu t). \end{equation*}

If the desired conclusion does not hold, then $\limsup _{t\to \infty }(h(t)-c_\nu t)=\tilde h^*\gt h^*$ . Thus, we can find a sequence $\{s_n\}$ increasing to $+\infty$ as $n\to \infty$ such that

\begin{equation*} \lim _{n\to \infty }(h(s_n)-c_\nu s_n)=\tilde h^*\gt h^*. \end{equation*}

We now examine $(\overline H,\overline M, \overline g,\overline h)$ defined in (3.1). Take $X_0=(\tilde h^*-h^*)/4\gt 0$ and $T^*=t_n$ . Then take $\sigma =(\tilde h^*-h^*)/4\gt 0$ and choose $\delta \gt 0$ , $K_1\gt 0$ such that (3.9), (3.12) and (3.13) hold. As in the proof of Lemma 3.2, by direct calculations we see that (3.3), (3.4), (3.5), (3.6) and (3.7) hold.

We show next that for all large $n$ , due to (4.19) and (4.20), the inequalities in (3.8) hold as well, and therefore the comparison principle can be applied to conclude that

(4.22) \begin{equation} h(t)\leq \overline h(t) \mbox{ for } t\gt T^*=t_n. \end{equation}

Indeed, for $x\in [\overline{g}(t_n),h(t_n)]=[g(t_n), h(t_n)]$ , by (4.19) and (4.20), we have

\begin{equation*}\begin {aligned} \overline {H}(x,t_n)&=(1+K_1)u_{c_\nu }(\bar {h}(t_n)-x)\\ &=(1+K_1)u_{c_\nu }(h(t_n)+X_0-x)\\ &\geq H(x, t_n) \mbox { for all large } n, \end {aligned} \end{equation*}

and

\begin{equation*}\begin {aligned} \overline {M}(x,t_n)&=(1+K_1)v_{c_\nu }(\bar {h}(t_n)-x)\\ &=(1+K_1)v_{c_\nu }(h(t_n)+X_0-x)\\ &\geq M(x, t_n) \mbox { for all large } n. \end {aligned} \end{equation*}

Hence, (4.22) holds for all large $n$ , say $n\geq N=N(K_1, X_0)$ . In particular, for all large integer $k$ satisfying $s_k\geq t_n$ we have

\begin{equation*} h(s_k)\leq \overline h(s_k)=c_\nu (s_k-t_n)+\sigma (1-e^{-\delta (s_k-t_n)} )+h(t_n)+X_0. \end{equation*}

It follows that

\begin{equation*} \tilde h^*=\lim _{k\to \infty } (h(s_k)-c_\nu s_k)\leq -c_\nu t_n+\sigma +h(t_n)+X_0. \end{equation*}

Letting $n\to \infty$ we then obtain

\begin{equation*} \tilde h^*\leq h^*+\sigma +X_0=h^*+(\tilde h^*-h^*)/2, \end{equation*}

which is impossible.

Thus, we have proved Claim 2 and then any positive sequence $\{t_n\}$ converging to $+\infty$ can be used in Lemma 4.1, and so it has a subsequence such that (4.21) and (4.19) hold. This clearly implies that the second part in (1.7) and (1.8) holds.

As before, consider (1.3) with initial function $(H_0(\!-x), M_0(\!-x))$ ; the above proved conclusions imply that the first part in (1.7) and (1.8) holds as well. Theorem 1.2 is now proved.