Hostname: page-component-6587cd75c8-6qszs Total loading time: 0 Render date: 2025-04-23T11:42:31.789Z Has data issue: false hasContentIssue false
  • English
  • Français

On a critical time-harmonic Maxwell equation in nonlocal media

Part of: Elliptic equations and systems Nonlinear integral equations Singular integral equations

Published online by Cambridge University Press:  29 February 2024

Minbo Yang ,
Weiwei Ye  and
Shuijin Zhang
Minbo Yang
Affiliation:
Department of Mathematics, Zhejiang Normal University, Jinhua, Zhejiang 321004, People's Republic of China ([email protected])
Weiwei Ye
Affiliation:
Department of Mathematics, Zhejiang Normal University, Jinhua, Zhejiang 321004, People's Republic of China Department of Mathematics, Fuyang Normal University, Fuyang, Anhui 236037, People's Republic of China ([email protected])
Shuijin Zhang
Affiliation:
Department of Mathematics, Zhejiang Normal University, Jinhua, Zhejiang 321004, People's Republic of China ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper, we study the existence of solutions for a critical time–harmonic Maxwell equation in nonlocal media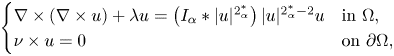

\[ \begin{cases} \nabla\times(\nabla\times u)+\lambda u=\left(I_{\alpha}\ast|u|^{2^{{\ast}}_{\alpha}}\right)|u|^{2^{{\ast}}_{\alpha}-2}u & \mathrm{in}\ \Omega,\\ \nu\times u=0 & \mathrm{on}\ \partial\Omega, \end{cases} \]
where $\Omega \subset \mathbb {R}^{3}$ is a bounded domain, either convex or with $\mathcal {C}^{1,1}$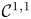 boundary, $\nu$ is the exterior normal, $\lambda <0$ is a real parameter, $2^{\ast }_{\alpha }=3+\alpha$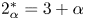 with $0<\alpha <3$ 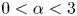is the upper critical exponent due to the Hardy–Littlewood–Sobolev inequality. By introducing some suitable Coulomb spaces involving curl operator $W^{\alpha,2^{\ast }_{\alpha }}_{0}(\mathrm {curl};\Omega )$, we are able to obtain the ground state solutions of the curl–curl equation via the method of constraining Nehari–Pankov manifold. Correspondingly, some sharp constants of the Sobolev-like inequalities with curl operator are obtained by a nonlocal version of the concentration–compactness principle.

Keywords

time–harmonic Maxwell equationBrezis–Nirenberg problemnonlocal nonlinearitycoulomb spacesharp constant

MSC classification

Primary: 35J15: Second-order elliptic equations
Secondary: 45E10: Integral equations of the convolution type (Abel, Picard, Toeplitz and Wiener-Hopf type) 45G05: Singular nonlinear integral equations
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , First View , pp. 1 - 45
Check for updates
Copyright
Copyright © The Author(s), 2024. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Amrouche, C., Bernardi, C., Dauge, M. and Girault, V.. Vector potentials in three-dimensional non-smooth domains. Math. Methods Appl. Sci. 21 (1998), 823–864.3.0.CO;2-B>CrossRefGoogle Scholar
Azzollini, A., Benci, V., D'Aprile, T. and Fortunato, D.. Existence of static solutions of the semilinear Maxwell equations. Ric. Mat. 55 (2006), 123–137.CrossRefGoogle Scholar
Bang, O., Krolikowski, W., Wyller, J. and Rasmussen, J.. Collapse arrest and soliton stabilization in nonlocal nonlinear media. Phys. Rev. E 66 (2002), 046619.CrossRefGoogle ScholarPubMed
Bartsch, T., Dohnal, T., Plum, M. and Reichel, W.. Ground states of a nonlinear curl–curl problem in cylindrically symmetric media. Nonlinear Differ. Equ. Appl. 34 (2016), 23–52.Google Scholar
Bartsch, T. and Mederski, J.. Ground and bound state solutions of semilinear time-harmonic Maxwell equations in a bounded domain. Arch. Ration. Mech. Anal. 215 (2015), 283–306.CrossRefGoogle Scholar
Bartsch, T. and Mederski, J.. Nonlinear time-harmonic Maxwell equations in an anisotropic bounded medium. J. Funct. Anal. 272 (2017), 4304–4333.CrossRefGoogle Scholar
Benci, V. and Fortunato, D.. Towards a unified field theory for classical electrodynamics. Arch. Ration. Mech. Anal. 173 (2004), 379–414.CrossRefGoogle Scholar
Benci, V. and Rabinowitz, P. H.. Critical point theorems for indefinite functionals. Invent. Math. 52 (1979), 241–273.CrossRefGoogle Scholar
Bergé, L. and Couairon, A.. Nonlinear propagation of self-guided ultra-short pulses in ionized gases. Phys. Plasmas 7 (2000), 210–230.CrossRefGoogle Scholar
Brezis, H. and Nirenberg, L.. Positive solutions of nonlinear elliptic equations involving critical Sobolev exponents. Commun. Pure Appl Math. 36 (1983), 437–477.CrossRefGoogle Scholar
Capozzi, A., Fortunato, D. and Palmieri, G.. An existence result for nonlinear elliptic problems involving critical Sobolev exponent. Ann. Inst. H. Poincaré Anal. Non Linéaire 2 (1985), 463–470.CrossRefGoogle Scholar
Cerami, G., Solimini, S. and Struwe, M.. Some existence results for superlinear elliptic boundary value problems involving critical exponents. J. Funct. Anal. 69 (1986), 289–306.CrossRefGoogle Scholar
D'Aprile, T. and Siciliano, G.. Magnetostatic solutions for a semilinear perturbation of the Maxwell equations. Adv. Differ. Equ. 16 (2011), 435–466.Google Scholar
Dörfler, W., Lechleiter, A., Plum, M., Schneider, G. and Wieners, C.. Photonic crystals: mathematical analysis and numerical approximation (Basel: Springer, 2012).Google Scholar
Dalfovo, F. et al. Theory of Bose-Einstein condensation in trapped gases. Rev. Mod. Phys. 71 (1999), 463–512.CrossRefGoogle Scholar
du Plessis, N.. An introduction to potential theory. University Mathematical Monographs vol. 7 (Edinburgh: Oliver and Boyd, 1970).Google Scholar
Du, Le. and Yang, M. B.. Uniqueness and nondegeneracy of solutions for a critical nonlocal equation. Discrete Contin. Dyn. Syst. 39 (2019), 5847–5866.CrossRefGoogle Scholar
Gao, F. S., Silva, E., Yang, M. B. and Zhou, J. Z.. Existence of solutions for critical Choquard equations via the concentration compactness method. Proc. R. Soc. Edinburgh 150 (2020), 921–954.CrossRefGoogle Scholar
Gao, F. S. and Yang, M. B.. The Brezis-Nirenberg type critical problem for the nonlinear Choquard equation. Sci. China Math. 61 (2018), 1219–1242.CrossRefGoogle Scholar
Guo, Q. Q. and Mederski, J.. Ground states of nonlinear Schrödinger equations with sum of periodic and inverse-square potentials. J. Differ. Equ. 260 (2016), 4180–4202.CrossRefGoogle Scholar
Kirsch, A. and Hettlich, F.. The mathematical theory of time-harmonic Maxwell's equations: expansion, integral, and variational methods, Applied Mathematical Science vol. 190 (Cham: Springer, 2015).CrossRefGoogle Scholar
Krolikowski, W., Bang, O., Rasmussen, J. J. and Wyller, J.. Modulational instability in nonlocal nonlinear kerr media. Phys. Rev. E 64 (2001), 016612.CrossRefGoogle ScholarPubMed
Leinfelder, H.. Gauge invariance of Schrödinger operators and related spectral properties. J. Oper. Theorey 9 (1983), 163–179.Google Scholar
Lieb, E. and Loss, M.. Analysis, graduate studies in mathematics (Providence: American Mathematical Society, 2001).Google Scholar
Lions, P. L.. The concentration-compactness principle in the calculus of variations. The limit case. Part I and II. Rev. Mat. Iberoam. 1 (1985), 145–201 [2 (1985) 45–121].CrossRefGoogle Scholar
Litvak, A. G.. Self-focusing of powerful light beams by thermal effects. JETP Lett. 4 (1966), 230–232.Google Scholar
Mandel, R.. Ground states for Maxwell's equation in nonlocal nonlinear media. Partial Differ. Equ. Appl. 3 (2022), Paper No. 22, 16pp.CrossRefGoogle Scholar
Mederski, J.. Ground states of time-harmonic semilinear Maxwell equations in $\mathbb {R}^3$ with vanishing permittivity. Arch. Ration. Mech. Anal. 218 (2015), 825–861.CrossRefGoogle Scholar
Mederski, J.. Ground states of a system of nonlinear Schrodinger equations with periodic potentials. Commun. Partial Differ. Equ. 41 (2016), 1426–1440.CrossRefGoogle Scholar
Mederski, J.. The Brezis-Nirenberg problem for the curl–curl operator. J. Funct. Anal. 274 (2018), 1345–1380.CrossRefGoogle Scholar
Mederski, J.. Nonlinear time-harmonic Maxwell equations in a bounded domain Lack of compactness. Sci. China Math. 61 (2018), 1963–1970.CrossRefGoogle Scholar
Mederski, J., Schino, J. and Szulkin, A.. Multiple solutions to a nonlinear curl–curl problem in $\mathbb {R}^3$. Arch. Ration. Mech. Anal. 236 (2019), 253–288.CrossRefGoogle Scholar
Mederski, J. and Szulkin, A.. A Sobolev-type inequality for the curl operator and ground states for the curl–curl equation with critical Sobolev exponent. Arch. Ration. Mech. Anal. 241 (2021), 1815–1842.CrossRefGoogle Scholar
Mercuri, C., Moroz, V. and Van Schaftingen, J.. Groundstates and radial solutions to nonlinear Schrödinger-Poisson-Slater equations at the critical frequency. Calc. Var. 55 (2016), Art. 146, 58pp.CrossRefGoogle Scholar
Monk, P.. Numerical Mathamatics and Scientific Computation: Finite element methods for Maxwell's equations (New York: Oxford University Press, 2003), xiv+450.CrossRefGoogle Scholar
Moroz, V. and Schaftingen, J. V.. A guide to the Choquard equation. J. Fixed Point Theory Appl. 19 (2017), 773–813.CrossRefGoogle Scholar
Nikolov, N. I., Neshev, D., Bang, O. and Królikowski, W. Z.. Quadratic solitons as nonlocal solitons. Phys. Rev. E 68 (2003), 036614.CrossRefGoogle ScholarPubMed
Padilla, W. J., Basov, D. N. and Smith, D. R.. Negative refractive index metamaterials. Mater. Today 9 (2006), 28–35.CrossRefGoogle Scholar
Picard, R., Weck, N. and Witsch, K. J.. Time-harmonic Maxwell equations in the exterior of perfectly conducting, irregular obstacles. Analysis 21 (2001), 231–263.CrossRefGoogle Scholar
Qin, D. D. and Tang, X. H.. Time-harmonic Maxwell equations with asymptotically linear polarization. Z. Angew. Math. Phys. 67 (2016), 39–67.CrossRefGoogle Scholar
Reimbert, C., Minzoni, A. and Smyth, N.. Spatial soliton evolution in nematic liquid crystals in the nonlinear local regime. J. Opt. Soc. Am. B: Opt. Phys. 23 (2006), 294–301.CrossRefGoogle Scholar
Solimini, S.. A note on compactness-type properties with respect to Lorentz norms of bounded subsets of a Sobolev space. Ann. Inst. H. Poincaré Anal. Non Linéaire 12 (1995), 319–337.CrossRefGoogle Scholar
Stuart, C. A. and Zhou, H. S.. Existence of guided cylindrical TM-models in a homogeneous self-trapping dielectric. Ann. Inst. H. Poincare Anal. Non Lineaire 18 (2001), 69–96.CrossRefGoogle Scholar
Stuart, C. A. and Zhou, H. S.. Axisymmetric TE-modes in a self-focusing dielectric. SIAM J. Math. Anal. 37 (2005), 218–237.CrossRefGoogle Scholar
Szulkin, A. and Weth, T.. Ground state solutions for some indefinite variational problems. J. Funct. Anal. 257 (2009), 3802–3822.CrossRefGoogle Scholar
Zeng, X. Y.. Cylindrically symmetric ground state solutions for curl–curl equations with critical exponent. Z. Angew. Math. Phys. 68 (2017), Paper No. 135, 12pp.CrossRefGoogle Scholar