No CrossRef data available.
Article contents
Global index of real polynomials
Part of:
Fiber spaces and bundles
Curves
Theory of singularities and catastrophe theory
Differential topology
Published online by Cambridge University Press: 18 March 2024
Abstract
We develop two methods for expressing the global index of the gradient of a 2 variable polynomial function $f$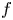 : in terms of the atypical fibres of $f$
: in terms of the atypical fibres of $f$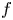 , and in terms of the clusters of Milnor arcs at infinity. These allow us to derive upper bounds for the global index, in particular refining the one that was found by Durfee in terms of the degree of $f$
, and in terms of the clusters of Milnor arcs at infinity. These allow us to derive upper bounds for the global index, in particular refining the one that was found by Durfee in terms of the degree of $f$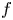 .
.
MSC classification
- Type
- Research Article
- Information
- Copyright
- Copyright © The Author(s), 2024. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh
References
Aoki, K., Fukuda, T. and Sun, W. Z.. On the number of branches of a plane curve germ. Kodai Math. J. 9 (1986), 179–187.Google Scholar
Araújo dos Santos, R. N., Chen, Y. and Tibăr, M.. Singular open book structures from real mappings. Cent. Eur. J. Math. 11 (2013), 817–828.Google Scholar
Araújo dos Santos, R. N., Chen, Y. and Tibăr, M.. Real polynomial maps and singular open books at infinity. Math. Scand. 118 (2016), 57–69.CrossRefGoogle Scholar
Araújo dos Santos, R. N. and Tibăr, M.. Real map germs and higher open book structures. Geom. Dedicata 147 (2010), 177–185.CrossRefGoogle Scholar
Arnold, V. I.. The index of a singular point of a vector field, the Petrovskii-Oleinik inequalities, and mixed Hodge structures. Funkcional. Anal. i Prilozen 12 (1978), 1–14. (Russian).CrossRefGoogle Scholar
Bekka, K.. C-régularité et trivialité topologique. Singularity Theory and Its Applications, Part I (Coventry, 1988/1989), 42–62. Lecture Notes in Math., vol. 1462 (Berlin: Springer, 1991).CrossRefGoogle Scholar
Coste, M. and de la Puente, M. J.. Atypical values at infinity of a polynomial function on the real plane: an erratum, and an algorithmic criterion. J. Pure Appl. Algebra 162 (2001), 23–35.CrossRefGoogle Scholar
Dias, L. R. G., Ruas, M. A. S. and Tibăr, M.. Regularity at infinity of real mappings and a Morse-Sard theorem. J. Topol. 5 (2012), 323–340.CrossRefGoogle Scholar
Dias, L. R. G., Joita, C. and Tibăr, M.. Atypical points at infinity and algorithmic detection of the bifurcation locus of real polynomials. Math. Z. 298 (2021), 1545–1558.CrossRefGoogle Scholar
Dias, L. R. G., Tanabé, S. and Tibăr, M.. Toward effective detection of the bifurcation locus of real polynomial maps. Found. Comput. Math. 3 (2017), 837–849.CrossRefGoogle Scholar
Dias, L. R. G. and Tibăr, M.. Detecting bifurcation values at infinity of real polynomials. Math. Z. 279 (2015), 311–319.CrossRefGoogle Scholar
Durfee, A. H.. The index of $\mathrm {{grad\ }} f(x,y)$ . Topology 37 (1998), 1339–1361.CrossRefGoogle Scholar
. Topology 37 (1998), 1339–1361.CrossRefGoogle Scholar
Durfee, A. H., Kronenfeld, N., Munson, H., Roy, J. and Westby, I.. Counting critical points of real polynomials in two variables. Am. Math. Mon. 100 (1993), 255–271.CrossRefGoogle Scholar
Gwoździewicz, J. and Płoski, A.. Formulae for the singularities at infinity of plane algebraic curves. Univ. Iagell. Acta Math. 39 (2001), 109–133.Google Scholar
Gwoździewicz, J.. The index at infinity of a polynomial in two variables. Univ. Iagel. Acta Math. 47 (2009), 243–247.Google Scholar
Gwoździewicz, J.. Ephraim's pencils. Int. Math. Res. Not. 15 (2013), 3371–3385.CrossRefGoogle Scholar
Hà, H. V. and Lê, D. T.. Sur la topologie des polynômes complexes. Acta Math. Vietnam. 9 (1985), 21–32.Google Scholar
Hà, H. V. and Nguyên, L. A.. Atypical values at infinity of polynomial and rational functions on an algebraic surface in $\mathbb {R}^n$ . Acta Math. Vietnam. 36 (2011), 537–553.Google Scholar
. Acta Math. Vietnam. 36 (2011), 537–553.Google Scholar
Hà, H. V. and Nguyen, T. T.. On the topology of polynomial mappings from ${\mathbb {C}}^n$ to ${\mathbb {C}}^{n-1}$
to ${\mathbb {C}}^{n-1}$ . Int. J. Math. 22 (2011), 435–448.Google Scholar
. Int. J. Math. 22 (2011), 435–448.Google Scholar
Jelonek, Z.. On bifurcation points of a complex polynomial. Proc. AMS 131 (2003), 1361–1367.CrossRefGoogle Scholar
Jelonek, Z. and Kurdyka, K.. On asymptotic critical values of a complex polynomial. J. Reine Angew. Math. 565 (2003), 1–11.CrossRefGoogle Scholar
Jelonek, Z. and Tibăr, M.. Bifurcation locus and branches at infinity of a polynomial $f : \mathbb {C}^2 \to \mathbb {C}$ . Math. Ann. 361 (2015), 1049–1054.CrossRefGoogle Scholar
. Math. Ann. 361 (2015), 1049–1054.CrossRefGoogle Scholar
Jelonek, Z. and Tibăr, M.. Detecting asymptotic non-regular values by polar curves. Int. Math. Res. Not. 2017 (2017), 809–829.Google Scholar
Joiţa, C. and Tibăr, M.. Bifurcation values of families of real curves. Proc. R. Soc. Edinb. Sect. A 147 (2017), 1233–1242.CrossRefGoogle Scholar
Joiţa, C. and Tibăr, M.. Bifurcation set of multi-parameter families of complex curves. J. Topol. 11 (2018), 739–751.CrossRefGoogle Scholar
Lê, V. T. and Oka, M.. Estimation of the number of the critical values at infinity of a polynomial function. Publ. RIMS Kyoto Univ. 31 (1995), 577–598.Google Scholar
Milnor, J. W.. Singular points of complex hypersurfaces. Annals of Mathematics Studies, vol. 61 (Princeton, NJ, Tokyo: Princeton University Press, University of Tokyo Press, 1968).Google Scholar
Milnor, J. W.. Topology from the differentiable viewpoint. Princeton Landmarks in Mathematics, pp. xii+64 (Princeton, NJ: Princeton University Press, 1997).Google Scholar
Monsalve, G. E.. Atypical values and the Milnor set of real polynomials in two variables. Bull. Braz. Math. Soc. (N.S.) 54 (2023), 13–19.CrossRefGoogle Scholar
Monsalve, G. E.. Bifurcation set and index at infinity of polynomials. Ph.D thesis (2023). doi: 10.11606/T.55.2023.tde-02022024-151957.CrossRefGoogle Scholar
Némethi, A. and Zaharia, A.. Milnor fibration at infinity. Indag. Math. 3 (1992), 323–335.CrossRefGoogle Scholar
Szafraniec, Z.. On the Euler characteristic of analytic and algebraic sets. Topology 25 (1986), 411–414.CrossRefGoogle Scholar
Sȩkalski, M.. On the degree of a polynomial mapping ${\mathbb {R}}^n \to {\mathbb {R}}^n$ at infinity. Univ. Iagel. Acta Math. 37 (1999), 121–125.Google Scholar
at infinity. Univ. Iagel. Acta Math. 37 (1999), 121–125.Google Scholar
Sȩkalski, M.. The degree at infinity of the gradient of a polynomial in two real variables. Ann. Polon. Math. 87 (2005), 229–235.CrossRefGoogle Scholar
Siersma, D. and Tibăr, M.. Singularities at infinity and their vanishing cycles. Duke Math. J. 80 (1995), 771–783.CrossRefGoogle Scholar
Suzuki, M.. Propriétés topologiques des polynômes de deux variables complexes, et automorphismes algébriques de l'espace ${\mathbb {C}}^2$ . J. Math. Soc. Jpn. 26 (1974), 241–257.CrossRefGoogle Scholar
. J. Math. Soc. Jpn. 26 (1974), 241–257.CrossRefGoogle Scholar
Thom, R.. Ensembles et morphismes stratifiés. Bull. Am. Math. Soc. 75 (1969), 240–284.CrossRefGoogle Scholar
Tibăr, M.. Regularity at infinity of real and complex polynomial maps, singularity theory. The C.T.C Wall Anniversary Volume, LMS Lecture Notes Series, vol. 263, pp. 249–264 (Cambridge: Cambridge University Press, 1999).CrossRefGoogle Scholar
Tibăr, M.. Polynomials and vanishing cycles. Cambridge Tracts in Mathematics, vol. 170 (Cambridge: Cambridge University Press, 2007).CrossRefGoogle Scholar
Tibăr, M. and Zaharia, A.. Asymptotic behaviour of families of real curves. Manuscr. Math. 99 (1999), 383–393.Google Scholar



