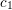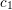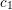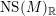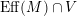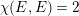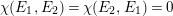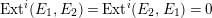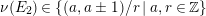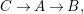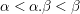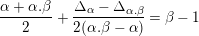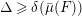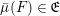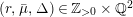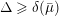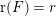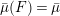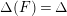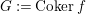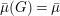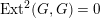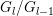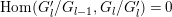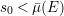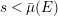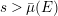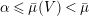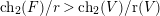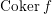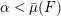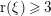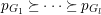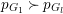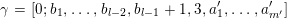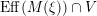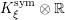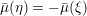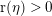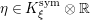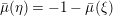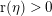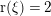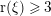1 Introduction
It is a fundamental problem to decide whether there exists a semistable sheaf with a given numerical invariant or not. In the case of
![]() $\mathbb{P}^{2}$
, Drezet and Le Potier [Reference Drezet and Le PotierDL] solved this problem completely; they determined the set of pairs
$\mathbb{P}^{2}$
, Drezet and Le Potier [Reference Drezet and Le PotierDL] solved this problem completely; they determined the set of pairs
![]() $(\unicode[STIX]{x1D707},\unicode[STIX]{x1D6E5})$
such that there exists a semistable sheaf having
$(\unicode[STIX]{x1D707},\unicode[STIX]{x1D6E5})$
such that there exists a semistable sheaf having
![]() $\unicode[STIX]{x1D707}$
as its slope and
$\unicode[STIX]{x1D707}$
as its slope and
![]() $\unicode[STIX]{x1D6E5}$
as its discriminant. In their result, exceptional bundles play an important role. In [Reference DrezetD], Drezet refined the result by introducing a function
$\unicode[STIX]{x1D6E5}$
as its discriminant. In their result, exceptional bundles play an important role. In [Reference DrezetD], Drezet refined the result by introducing a function
 $\unicode[STIX]{x1D6FF}:\mathbb{Q}\rightarrow \mathbb{Q}$
, which is also defined using exceptional bundles. He proved that the inequality
$\unicode[STIX]{x1D6FF}:\mathbb{Q}\rightarrow \mathbb{Q}$
, which is also defined using exceptional bundles. He proved that the inequality
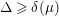 $\unicode[STIX]{x1D6E5}\geqslant \unicode[STIX]{x1D6FF}(\unicode[STIX]{x1D707})$
is a necessary and sufficient condition for
$\unicode[STIX]{x1D6E5}\geqslant \unicode[STIX]{x1D6FF}(\unicode[STIX]{x1D707})$
is a necessary and sufficient condition for
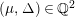 $(\unicode[STIX]{x1D707},\unicode[STIX]{x1D6E5})\in \mathbb{Q}^{2}$
to be the pair of the slope and discriminant of a nonexceptional stable sheaf. The function
$(\unicode[STIX]{x1D707},\unicode[STIX]{x1D6E5})\in \mathbb{Q}^{2}$
to be the pair of the slope and discriminant of a nonexceptional stable sheaf. The function
![]() $\unicode[STIX]{x1D6FF}$
allowed him to define an invariant height of a positive-dimensional moduli space of semistable sheaves on
$\unicode[STIX]{x1D6FF}$
allowed him to define an invariant height of a positive-dimensional moduli space of semistable sheaves on
![]() $\mathbb{P}^{2}$
, and he proved that a moduli space of height zero is isomorphic to a moduli space of representations of the Kronecker quiver [Reference DrezetD, Theorem 2].
$\mathbb{P}^{2}$
, and he proved that a moduli space of height zero is isomorphic to a moduli space of representations of the Kronecker quiver [Reference DrezetD, Theorem 2].
The Picard group of a positive-dimensional moduli space of semistable sheaves on
![]() $\mathbb{P}^{2}$
is a free abelian group of rank
$\mathbb{P}^{2}$
is a free abelian group of rank
![]() $1$
(resp.
$1$
(resp.
![]() $2$
) if its height is zero (resp. positive).
$2$
) if its height is zero (resp. positive).
When the height of a moduli space
![]() $M$
of semistable sheaves on
$M$
of semistable sheaves on
![]() $\mathbb{P}^{2}$
is positive, Coskun, Huizenga and Woolf [Reference Coskun, Huizenga and WoolfCHW] constructed a rational map of
$\mathbb{P}^{2}$
is positive, Coskun, Huizenga and Woolf [Reference Coskun, Huizenga and WoolfCHW] constructed a rational map of
![]() $M$
to a moduli space of representations of a Kronecker quiver, and using it, determined completely the effective cone of the moduli space
$M$
to a moduli space of representations of a Kronecker quiver, and using it, determined completely the effective cone of the moduli space
![]() $M$
. In [Reference AbeA15], using Coskun, Huizenga and Woolf’s rational map as one of the ingredients, the author showed the strange duality for sheaves on
$M$
. In [Reference AbeA15], using Coskun, Huizenga and Woolf’s rational map as one of the ingredients, the author showed the strange duality for sheaves on
![]() $\mathbb{P}^{2}$
when one of the moduli spaces appearing in the strange duality has height zero.
$\mathbb{P}^{2}$
when one of the moduli spaces appearing in the strange duality has height zero.
The aim of this paper is to pursue, in the case of the quadric surface
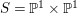 $S=\mathbb{P}^{1}\times \mathbb{P}^{1}$
under the assumption “with symmetric
$S=\mathbb{P}^{1}\times \mathbb{P}^{1}$
under the assumption “with symmetric
![]() $c_{1}$
,” analogues to the above results.
$c_{1}$
,” analogues to the above results.
1.1 Height and moduli spaces of height zero
First of all, we need to determine Chern classes of semistable sheaves with symmetric
![]() $c_{1}$
and define height for positive-dimensional moduli spaces of sheaves with symmetric
$c_{1}$
and define height for positive-dimensional moduli spaces of sheaves with symmetric
![]() $c_{1}$
on
$c_{1}$
on
![]() $S$
. In [Reference RudakovR94], Rudakov described Chern classes of semistable sheaves on
$S$
. In [Reference RudakovR94], Rudakov described Chern classes of semistable sheaves on
![]() $S$
(under the condition
$S$
(under the condition
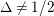 $\unicode[STIX]{x1D6E5}\neq 1/2$
). His description involves all exceptional bundles. The set of all exceptional bundles on
$\unicode[STIX]{x1D6E5}\neq 1/2$
). His description involves all exceptional bundles. The set of all exceptional bundles on
![]() $S$
is more complicated than the set of those on
$S$
is more complicated than the set of those on
![]() $\mathbb{P}^{2}$
(see [Reference RudakovR89]). So it seems difficult to define, in the quadric surface case, an analogue to the function
$\mathbb{P}^{2}$
(see [Reference RudakovR89]). So it seems difficult to define, in the quadric surface case, an analogue to the function
![]() $\unicode[STIX]{x1D6FF}$
introduced by Drezet for
$\unicode[STIX]{x1D6FF}$
introduced by Drezet for
![]() $\mathbb{P}^{2}$
, and to define height of the moduli space of semistable sheaves on
$\mathbb{P}^{2}$
, and to define height of the moduli space of semistable sheaves on
![]() $S$
.
$S$
.
The essential observation of this paper (Proposition 3.6) says that if we restrict ourselves to considering semistable sheaves on
![]() $S$
having symmetric
$S$
having symmetric
![]() $c_{1}$
, then we can describe their Chern classes using only symmetric exceptional bundles. Although the proof of this observation is easy, we note that it still is not a trivial fact because the filters of the Harder–Narasimhan filtration of a sheaf with symmetric
$c_{1}$
, then we can describe their Chern classes using only symmetric exceptional bundles. Although the proof of this observation is easy, we note that it still is not a trivial fact because the filters of the Harder–Narasimhan filtration of a sheaf with symmetric
![]() $c_{1}$
do not necessarily have symmetric
$c_{1}$
do not necessarily have symmetric
![]() $c_{1}$
. The set of symmetric exceptional bundles on
$c_{1}$
. The set of symmetric exceptional bundles on
![]() $S$
is well understood (cf. [Reference RudakovR89, Section 6]), so we can define an analogous function
$S$
is well understood (cf. [Reference RudakovR89, Section 6]), so we can define an analogous function
![]() $\unicode[STIX]{x1D6FF}$
to Drezet’s function
$\unicode[STIX]{x1D6FF}$
to Drezet’s function
![]() $\unicode[STIX]{x1D6FF}$
in the
$\unicode[STIX]{x1D6FF}$
in the
![]() $\mathbb{P}^{2}$
case, and define height of a moduli space of semistable sheaves on
$\mathbb{P}^{2}$
case, and define height of a moduli space of semistable sheaves on
![]() $S$
having symmetric
$S$
having symmetric
![]() $c_{1}$
(Definition 3.8). Then we proceed to show that a moduli space of height zero is isomorphic to a moduli space of representations of a certain quiver (see Section 5.2 for the appearing quivers). The result has some overlap with the result in [Reference KarpovKa], [Reference KuleshovKu]. In both [Reference KarpovKa] and [Reference KuleshovKu], the authors do not impose the assumption “having symmetric
$c_{1}$
(Definition 3.8). Then we proceed to show that a moduli space of height zero is isomorphic to a moduli space of representations of a certain quiver (see Section 5.2 for the appearing quivers). The result has some overlap with the result in [Reference KarpovKa], [Reference KuleshovKu]. In both [Reference KarpovKa] and [Reference KuleshovKu], the authors do not impose the assumption “having symmetric
![]() $c_{1}$
”. Karpov [Reference KarpovKa] gave a sufficient condition for a moduli space of semistable sheaves on
$c_{1}$
”. Karpov [Reference KarpovKa] gave a sufficient condition for a moduli space of semistable sheaves on
![]() $S$
to be isomorphic to a moduli space of representations of Kronecker quivers. In [Reference KarpovKa, Section 7], he considered a symmetric case, and [Reference KarpovKa, Theorem 7.3] is a special case of Theorem 5.4 in this paper. Kuleshov gave some examples of moduli spaces of semistable sheaves on
$S$
to be isomorphic to a moduli space of representations of Kronecker quivers. In [Reference KarpovKa, Section 7], he considered a symmetric case, and [Reference KarpovKa, Theorem 7.3] is a special case of Theorem 5.4 in this paper. Kuleshov gave some examples of moduli spaces of semistable sheaves on
![]() $S$
that are isomorphic to moduli spaces of representations of quivers like
$S$
that are isomorphic to moduli spaces of representations of quivers like
![]() $Q^{\unicode[STIX]{x1D6FC}}$
and
$Q^{\unicode[STIX]{x1D6FC}}$
and
![]() $Q^{\unicode[STIX]{x1D6FD}}$
(see Section 5.2 for the quivers
$Q^{\unicode[STIX]{x1D6FD}}$
(see Section 5.2 for the quivers
![]() $Q^{\unicode[STIX]{x1D6FC}}$
,
$Q^{\unicode[STIX]{x1D6FC}}$
,
![]() $Q^{\unicode[STIX]{x1D6FD}}$
).
$Q^{\unicode[STIX]{x1D6FD}}$
).
1.2 Coskun, Huizenga and Woolf’s rational map
After defining height, and describing the structure of moduli spaces of height zero, we move on to studying moduli spaces of positive height.
Let
![]() $M$
be a moduli space of positive height of semistable sheaves with symmetric
$M$
be a moduli space of positive height of semistable sheaves with symmetric
![]() $c_{1}$
on
$c_{1}$
on
![]() $S$
. What we do is the following:
$S$
. What we do is the following:
-
(i) We construct a rational map of
 $M$
to a moduli space of representations of quivers, which is an analogue of the rational map constructed by Coskun, Huizenga and Woolf in case
$M$
to a moduli space of representations of quivers, which is an analogue of the rational map constructed by Coskun, Huizenga and Woolf in case
 $\mathbb{P}^{2}$
[Reference Coskun, Huizenga and WoolfCHW].
$\mathbb{P}^{2}$
[Reference Coskun, Huizenga and WoolfCHW]. -
(ii) We determine some part of the effective cone of
 $M$
. More precisely, we define a
$M$
. More precisely, we define a
 $2$
-dimensional subspace
$2$
-dimensional subspace
 $V$
in
$V$
in
 $\text{NS}(M)_{\mathbb{R}}$
, and determine the cone
$\text{NS}(M)_{\mathbb{R}}$
, and determine the cone
 $\text{Eff}(M)\cap V$
.
$\text{Eff}(M)\cap V$
. -
(iii) We establish a strange duality for height-zero moduli spaces in the case
 $S$
.
$S$
.
Actually, once we succeeded in defining height, doing these things is a more or less straight-forward task. Having said so, there are some technical issues to cope with. The technical difficulty is caused by the following two facts:
-
∙ the Picard group of
 $S$
is not cyclic,
$S$
is not cyclic, -
∙ there are two kinds of symmetric exceptional slopes: even ones and odd ones.
For example, to carry out (i), we need to show an analogous result to [Reference Coskun, Huizenga and WoolfCHW, Theorem 4.16], which concerns continued fraction expansions of exceptional slopes. This is Theorem 7.4, and its proof is messier than [Reference Coskun, Huizenga and WoolfCHW, Theorem 4.16] due to the above second fact. Also, to prove (iii), we need to show a dimension-estimate result analogous to [Reference Le PotierLe, Lemma 18.3.1]. This is Proposition 6.1, and its proof is more involved than [Reference Le PotierLe, Lemma 18.3.1] due to the above first fact.
Comment on the method of the proof of Theorem 5.4 : in the proof of Theorem 5.4, it is essential to show that the semistability of a sheaf corresponds exactly to the semistability of the representation of a quiver. To show this, we take a different method from that in [Reference DrezetD], [Reference KarpovKa], [Reference KuleshovKu]; we employ the argument using the Bridgeland stability as in [Reference Arcara, Bertram, Coskun and HuizengaABCH], [Reference OhkawaOh]. It is just a matter of the author’s taste; the proof via the Bridgeland stability feels more conceptual.
Organization of the paper: In Section 2, we collect basic facts about symmetric exceptional bundles. In Section 3, we define the function
![]() $\unicode[STIX]{x1D6FF}$
, prove the existence theorem (Theorem 3.7), which is a counterpart of the second statement in [Reference DrezetD, Theorem 1], and define the height of a moduli space. In Section 4, we study the Bridgeland stability for symmetric exceptional bundles. The Bridgeland stability of exceptional bundles on
$\unicode[STIX]{x1D6FF}$
, prove the existence theorem (Theorem 3.7), which is a counterpart of the second statement in [Reference DrezetD, Theorem 1], and define the height of a moduli space. In Section 4, we study the Bridgeland stability for symmetric exceptional bundles. The Bridgeland stability of exceptional bundles on
![]() $\mathbb{P}^{2}$
was studied by Huizenga [Reference HuizengaH, Section 9], and we trace his argument. In Section 5, we study the relation between the usual semistability and the Bridgeland semistability. Then, after introducing notation of quivers, we show that the moduli space of height zero is isomorphic to a moduli space of representations of a quiver. Section 6 is devoted to proving Proposition 6.1, which gives a dimension estimate for the locus of nonsemistable sheaves in a complete family of torsion-free sheaves. In Section 7, we consider continued fraction expansions of symmetric exceptional slopes. This section is a counterpart of [Reference HuizengaH, Section 3] and [Reference Coskun, Huizenga and WoolfCHW, Section 4]. In Section 8, we consider resolutions of semistable sheaves by symmetric exceptional bundles. In Section 9, we define a rational map of a moduli space of sheaves to a moduli space of representations of a quiver, which is an analogue, in the quadric surface case, to Coskun, Huizenga and Woolf’s rational map. In Section 10, we define a
$\mathbb{P}^{2}$
was studied by Huizenga [Reference HuizengaH, Section 9], and we trace his argument. In Section 5, we study the relation between the usual semistability and the Bridgeland semistability. Then, after introducing notation of quivers, we show that the moduli space of height zero is isomorphic to a moduli space of representations of a quiver. Section 6 is devoted to proving Proposition 6.1, which gives a dimension estimate for the locus of nonsemistable sheaves in a complete family of torsion-free sheaves. In Section 7, we consider continued fraction expansions of symmetric exceptional slopes. This section is a counterpart of [Reference HuizengaH, Section 3] and [Reference Coskun, Huizenga and WoolfCHW, Section 4]. In Section 8, we consider resolutions of semistable sheaves by symmetric exceptional bundles. In Section 9, we define a rational map of a moduli space of sheaves to a moduli space of representations of a quiver, which is an analogue, in the quadric surface case, to Coskun, Huizenga and Woolf’s rational map. In Section 10, we define a
![]() $2$
-dimensional subspace in the real Néron–Severi group of the moduli space of sheaves, and determine the intersection of the subspace and the effective cone. In Section 11, we state a strange duality for height-zero moduli spaces in the quadric surface case. In Appendix we give a Beilinson-type spectral sequence used in the paper for readers’ convenience.
$2$
-dimensional subspace in the real Néron–Severi group of the moduli space of sheaves, and determine the intersection of the subspace and the effective cone. In Section 11, we state a strange duality for height-zero moduli spaces in the quadric surface case. In Appendix we give a Beilinson-type spectral sequence used in the paper for readers’ convenience.
Notation and Convention.
In this paper, the variety
![]() $S$
denotes
$S$
denotes
![]() $\mathbb{P}^{1}\times \mathbb{P}^{1}$
.
$\mathbb{P}^{1}\times \mathbb{P}^{1}$
.
The line bundle
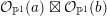 ${\mathcal{O}}_{\mathbb{P}^{1}}(a)\boxtimes {\mathcal{O}}_{\mathbb{P}^{1}}(b)$
is denoted by
${\mathcal{O}}_{\mathbb{P}^{1}}(a)\boxtimes {\mathcal{O}}_{\mathbb{P}^{1}}(b)$
is denoted by
![]() ${\mathcal{O}}(a,b)$
, and
${\mathcal{O}}(a,b)$
, and
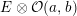 $E\otimes {\mathcal{O}}(a,b)$
is abbreviated as
$E\otimes {\mathcal{O}}(a,b)$
is abbreviated as
![]() $E(a,b)$
for a sheaf
$E(a,b)$
for a sheaf
![]() $E$
. We identify
$E$
. We identify
![]() $\text{Pic}\,S$
with
$\text{Pic}\,S$
with
![]() $\mathbb{Z}^{2}$
by the correspondence
$\mathbb{Z}^{2}$
by the correspondence
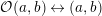 ${\mathcal{O}}(a,b)\leftrightarrow (a,b)$
.
${\mathcal{O}}(a,b)\leftrightarrow (a,b)$
.
Unless otherwise stated, a semistable sheaf is a semistable torsion-free sheaf, and semistability means Gieseker–Maruyama semistability with respect to the ample line bundle
![]() ${\mathcal{O}}(1,1)$
. The rank of a coherent sheaf
${\mathcal{O}}(1,1)$
. The rank of a coherent sheaf
![]() $E$
is denoted by
$E$
is denoted by
![]() $\text{r}(E)$
. The reduced Hilbert polynomial of a sheaf
$\text{r}(E)$
. The reduced Hilbert polynomial of a sheaf
![]() $E$
is denoted by
$E$
is denoted by
![]() $p_{E}$
, that is,
$p_{E}$
, that is,
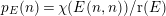 $p_{E}(n)=\unicode[STIX]{x1D712}(E(n,n))/\text{r}(E)$
for
$p_{E}(n)=\unicode[STIX]{x1D712}(E(n,n))/\text{r}(E)$
for
![]() $n\gg 0$
.
$n\gg 0$
.
For polynomials
![]() $f$
and
$f$
and
![]() $g$
,
$g$
,
![]() $f\succcurlyeq g$
(resp.
$f\succcurlyeq g$
(resp.
![]() $f\succ g$
) means that
$f\succ g$
) means that
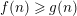 $f(n)\geqslant g(n)$
(resp.
$f(n)\geqslant g(n)$
(resp.
 $f(n)>g(n)$
) for
$f(n)>g(n)$
) for
![]() $n\gg 0$
.
$n\gg 0$
.
A class
 $\unicode[STIX]{x1D709}\in K(S)$
is said to be semistable if there exists a semistable sheaf
$\unicode[STIX]{x1D709}\in K(S)$
is said to be semistable if there exists a semistable sheaf
![]() $F$
with
$F$
with
![]() $\unicode[STIX]{x1D709}=[F]$
.
$\unicode[STIX]{x1D709}=[F]$
.
For
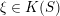 $\unicode[STIX]{x1D709}\in K(S)$
, we denote by
$\unicode[STIX]{x1D709}\in K(S)$
, we denote by
![]() $M(\unicode[STIX]{x1D709})$
the moduli space of semistable sheaves
$M(\unicode[STIX]{x1D709})$
the moduli space of semistable sheaves
![]() $F$
on
$F$
on
![]() $S$
with
$S$
with
![]() $[F]=\unicode[STIX]{x1D709}$
.
$[F]=\unicode[STIX]{x1D709}$
.
For a smooth projective variety
![]() $X$
,
$X$
,
![]() $\text{D}(X)$
denotes the bounded derived category of coherent sheaves on
$\text{D}(X)$
denotes the bounded derived category of coherent sheaves on
![]() $X$
.
$X$
.
![]() $\text{P}(x)$
denotes the polynomial
$\text{P}(x)$
denotes the polynomial
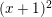 $(x+1)^{2}$
.
$(x+1)^{2}$
.
2 Preliminaries
2.1 Numerical Invariants
The rank of
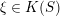 $\unicode[STIX]{x1D709}\in K(S)$
is denoted by
$\unicode[STIX]{x1D709}\in K(S)$
is denoted by
![]() $\text{r}(\unicode[STIX]{x1D709})$
. If
$\text{r}(\unicode[STIX]{x1D709})$
. If
![]() $\unicode[STIX]{x1D709}$
has rank
$\unicode[STIX]{x1D709}$
has rank
![]() $r>0$
, its discriminant is defined by
$r>0$
, its discriminant is defined by
 $$\begin{eqnarray}\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})=\frac{1}{r}\left(c_{2}(\unicode[STIX]{x1D709})-\frac{r-1}{2r}c_{1}(\unicode[STIX]{x1D709})^{2}\right)\!.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})=\frac{1}{r}\left(c_{2}(\unicode[STIX]{x1D709})-\frac{r-1}{2r}c_{1}(\unicode[STIX]{x1D709})^{2}\right)\!.\end{eqnarray}$$
If
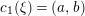 $c_{1}(\unicode[STIX]{x1D709})=(a,b)$
, then we put
$c_{1}(\unicode[STIX]{x1D709})=(a,b)$
, then we put
 $$\begin{eqnarray}\displaystyle \begin{array}{@{}rclrclrcl@{}}\unicode[STIX]{x1D708}(\unicode[STIX]{x1D709})\ & =\ & (a,b)/r, & \qquad \unicode[STIX]{x1D708}^{\prime }(\unicode[STIX]{x1D709})\ & =\ & a/r, & \qquad \unicode[STIX]{x1D708}^{\prime \prime }(\unicode[STIX]{x1D709})\ & =\ & b/r,\\ \deg \unicode[STIX]{x1D709}\ & =\ & a+b, & \qquad \unicode[STIX]{x1D707}(\unicode[STIX]{x1D709})\ & =\ & (a+b)/r, & \qquad \bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})\ & =\ & \unicode[STIX]{x1D707}(\unicode[STIX]{x1D709})/2.\end{array} & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \begin{array}{@{}rclrclrcl@{}}\unicode[STIX]{x1D708}(\unicode[STIX]{x1D709})\ & =\ & (a,b)/r, & \qquad \unicode[STIX]{x1D708}^{\prime }(\unicode[STIX]{x1D709})\ & =\ & a/r, & \qquad \unicode[STIX]{x1D708}^{\prime \prime }(\unicode[STIX]{x1D709})\ & =\ & b/r,\\ \deg \unicode[STIX]{x1D709}\ & =\ & a+b, & \qquad \unicode[STIX]{x1D707}(\unicode[STIX]{x1D709})\ & =\ & (a+b)/r, & \qquad \bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})\ & =\ & \unicode[STIX]{x1D707}(\unicode[STIX]{x1D709})/2.\end{array} & & \displaystyle \nonumber\end{eqnarray}$$
Here
![]() $\unicode[STIX]{x1D708}(\unicode[STIX]{x1D709})$
is an element of
$\unicode[STIX]{x1D708}(\unicode[STIX]{x1D709})$
is an element of
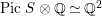 $\text{Pic}\,S\otimes \mathbb{Q}\simeq \mathbb{Q}^{2}$
. We say that
$\text{Pic}\,S\otimes \mathbb{Q}\simeq \mathbb{Q}^{2}$
. We say that
![]() $\unicode[STIX]{x1D709}$
has symmetric
$\unicode[STIX]{x1D709}$
has symmetric
![]() $c_{1}$
if
$c_{1}$
if
![]() $a=b$
. We mainly use these notations when
$a=b$
. We mainly use these notations when
![]() $\unicode[STIX]{x1D709}$
is a class of a sheaf.
$\unicode[STIX]{x1D709}$
is a class of a sheaf.
If
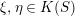 $\unicode[STIX]{x1D709},\unicode[STIX]{x1D702}\in K(S)$
have positive rank, then by the Riemann–Roch theorem we have
$\unicode[STIX]{x1D709},\unicode[STIX]{x1D702}\in K(S)$
have positive rank, then by the Riemann–Roch theorem we have
 $$\begin{eqnarray}\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709},\unicode[STIX]{x1D702})=\text{r}(\unicode[STIX]{x1D709})\text{r}(\unicode[STIX]{x1D702})\left\{\left(\unicode[STIX]{x1D708}^{\prime }(\unicode[STIX]{x1D702})-\unicode[STIX]{x1D708}^{\prime }(\unicode[STIX]{x1D709})+1\right)\left(\unicode[STIX]{x1D708}^{\prime \prime }(\unicode[STIX]{x1D702})-\unicode[STIX]{x1D708}^{\prime \prime }(\unicode[STIX]{x1D709})+1\right)-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D702})\right\}\!.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709},\unicode[STIX]{x1D702})=\text{r}(\unicode[STIX]{x1D709})\text{r}(\unicode[STIX]{x1D702})\left\{\left(\unicode[STIX]{x1D708}^{\prime }(\unicode[STIX]{x1D702})-\unicode[STIX]{x1D708}^{\prime }(\unicode[STIX]{x1D709})+1\right)\left(\unicode[STIX]{x1D708}^{\prime \prime }(\unicode[STIX]{x1D702})-\unicode[STIX]{x1D708}^{\prime \prime }(\unicode[STIX]{x1D709})+1\right)-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D702})\right\}\!.\end{eqnarray}$$
If
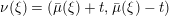 $\unicode[STIX]{x1D708}(\unicode[STIX]{x1D709})=(\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})+t,\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})-t)$
and
$\unicode[STIX]{x1D708}(\unicode[STIX]{x1D709})=(\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})+t,\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})-t)$
and
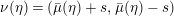 $\unicode[STIX]{x1D708}(\unicode[STIX]{x1D702})=(\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D702})+s,\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D702})-s)$
, then the above formula can also be expressed as
$\unicode[STIX]{x1D708}(\unicode[STIX]{x1D702})=(\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D702})+s,\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D702})-s)$
, then the above formula can also be expressed as
 $$\begin{eqnarray}\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709},\unicode[STIX]{x1D702})=\text{r}(\unicode[STIX]{x1D709})\text{r}(\unicode[STIX]{x1D702})\left\{\text{P}(\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D702})-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-(t-s)^{2}-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D702})\right\}\!.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709},\unicode[STIX]{x1D702})=\text{r}(\unicode[STIX]{x1D709})\text{r}(\unicode[STIX]{x1D702})\left\{\text{P}(\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D702})-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-(t-s)^{2}-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D702})\right\}\!.\end{eqnarray}$$
In particular, if
![]() $\unicode[STIX]{x1D709}$
and
$\unicode[STIX]{x1D709}$
and
![]() $\unicode[STIX]{x1D702}$
have symmetric
$\unicode[STIX]{x1D702}$
have symmetric
![]() $c_{1}$
, then
$c_{1}$
, then
 $$\begin{eqnarray}\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709},\unicode[STIX]{x1D702})=\text{r}(\unicode[STIX]{x1D709})\text{r}(\unicode[STIX]{x1D702})\left\{\text{P}(\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D702})-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D702})\right\}\!.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709},\unicode[STIX]{x1D702})=\text{r}(\unicode[STIX]{x1D709})\text{r}(\unicode[STIX]{x1D702})\left\{\text{P}(\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D702})-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D702})\right\}\!.\end{eqnarray}$$
Definition 2.1. A coherent sheaf
![]() $E$
on a smooth projective variety is said to be exceptional if
$E$
on a smooth projective variety is said to be exceptional if
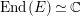 $\text{End}(E)\simeq \mathbb{C}$
and
$\text{End}(E)\simeq \mathbb{C}$
and
 $\text{Ext}^{i}(E,E)=0$
for
$\text{Ext}^{i}(E,E)=0$
for
![]() $i>0$
.
$i>0$
.
All exceptional sheaves on
![]() $S$
are locally free (cf. [Reference Kuleshov and OrlovKO, Proposition 2.9]). A general member
$S$
are locally free (cf. [Reference Kuleshov and OrlovKO, Proposition 2.9]). A general member
![]() $C$
of the anti-canonical linear system
$C$
of the anti-canonical linear system
![]() $|-K_{S}|$
is an elliptic curve, and the restriction
$|-K_{S}|$
is an elliptic curve, and the restriction
![]() $E|_{C}$
of an exceptional bundle
$E|_{C}$
of an exceptional bundle
![]() $E$
to
$E$
to
![]() $C$
is simple (cf. [Reference Kuleshov and OrlovKO, Lemma 3.6]). It follows from this that
$C$
is simple (cf. [Reference Kuleshov and OrlovKO, Lemma 3.6]). It follows from this that
![]() $E$
is
$E$
is
![]() $\unicode[STIX]{x1D707}$
-stable (with respect to the polarization
$\unicode[STIX]{x1D707}$
-stable (with respect to the polarization
![]() ${\mathcal{O}}(1,1)$
), and that if
${\mathcal{O}}(1,1)$
), and that if
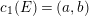 $c_{1}(E)=(a,b)$
then
$c_{1}(E)=(a,b)$
then
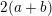 $2(a+b)$
and
$2(a+b)$
and
![]() $\text{r}(E)$
are coprime. In particular,
$\text{r}(E)$
are coprime. In particular,
![]() $\text{r}(E)$
is odd.
$\text{r}(E)$
is odd.
2.2 Symmetric Exceptional bundles
 $\unicode[STIX]{x1D704}:S\rightarrow S$
denotes the involution
$\unicode[STIX]{x1D704}:S\rightarrow S$
denotes the involution
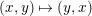 $(x,y)\mapsto (y,x)$
. A coherent sheaf
$(x,y)\mapsto (y,x)$
. A coherent sheaf
![]() $E$
on
$E$
on
![]() $S$
is said to be symmetric if
$S$
is said to be symmetric if
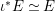 $\unicode[STIX]{x1D704}^{\ast }E\simeq E$
. We recall the following result [Reference RudakovR89, Proposition 6.1].
$\unicode[STIX]{x1D704}^{\ast }E\simeq E$
. We recall the following result [Reference RudakovR89, Proposition 6.1].
Proposition 2.2. Let
![]() $E$
be a symmetric bundle that is a direct sum
$E$
be a symmetric bundle that is a direct sum
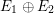 $E_{1}\oplus E_{2}$
with
$E_{1}\oplus E_{2}$
with
![]() $E_{1}$
and
$E_{1}$
and
![]() $E_{2}$
exceptional bundles. The following are equivalent.
$E_{2}$
exceptional bundles. The following are equivalent.
-
(1)
 $\unicode[STIX]{x1D712}(E,E)=2$
.
$\unicode[STIX]{x1D712}(E,E)=2$
. -
(2)
 $\unicode[STIX]{x1D712}(E_{1},E_{2})=\unicode[STIX]{x1D712}(E_{2},E_{1})=0$
.
$\unicode[STIX]{x1D712}(E_{1},E_{2})=\unicode[STIX]{x1D712}(E_{2},E_{1})=0$
. -
(3)
 $\text{Ext}^{i}(E_{1},E_{2})=\text{Ext}^{i}(E_{2},E_{1})=0$
.
$\text{Ext}^{i}(E_{1},E_{2})=\text{Ext}^{i}(E_{2},E_{1})=0$
. -
(4)
 $\unicode[STIX]{x1D708}(E_{1})$
,
$\unicode[STIX]{x1D708}(E_{1})$
,
 $\unicode[STIX]{x1D708}(E_{2})\in \left\{(a,a\pm 1)/r\mid a,r\in \mathbb{Z}\right\}\!$
.
$\unicode[STIX]{x1D708}(E_{2})\in \left\{(a,a\pm 1)/r\mid a,r\in \mathbb{Z}\right\}\!$
.
After Rudakov [Reference RudakovR89], we use the following terminology.
Definition 2.3. A symmetric exceptional bundle is either an exceptional bundle which is symmetric or a symmetric bundle satisfying the equivalent conditions in Proposition 2.2. The former is called an odd symmetric exceptional bundle, and the latter is called an even symmetric exceptional bundle. (Note that an even symmetric exceptional bundle is NOT an exceptional bundle.)
The use of the adjective “even” and “odd” is justified by the fact that the rank of an even (resp. odd) symmetric exceptional bundle is even (resp. odd).
2.3 Mutation in a general context
We recall an operation called mutation in a general context. Our references are [Reference BondalBo] and [Reference Bridgeland and SternBS].
Let
![]() ${\mathcal{D}}$
be a
${\mathcal{D}}$
be a
![]() $\mathbb{C}$
-linear triangulated category. For a full triangulated subcategory
$\mathbb{C}$
-linear triangulated category. For a full triangulated subcategory
![]() ${\mathcal{B}}$
, we put
${\mathcal{B}}$
, we put
 $$\begin{eqnarray}\displaystyle {\mathcal{B}}^{\bot } & = & \displaystyle \left\{A\in {\mathcal{D}}\mid \text{Hom}(B,A)=0\text{ for any }B\in {\mathcal{B}}\right\}\!,\nonumber\\ \displaystyle \text{}^{\bot }{\mathcal{B}} & = & \displaystyle \left\{A\in {\mathcal{D}}\mid \text{Hom}(A,B)=0\text{ for any }B\in {\mathcal{B}}\right\}\!.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle {\mathcal{B}}^{\bot } & = & \displaystyle \left\{A\in {\mathcal{D}}\mid \text{Hom}(B,A)=0\text{ for any }B\in {\mathcal{B}}\right\}\!,\nonumber\\ \displaystyle \text{}^{\bot }{\mathcal{B}} & = & \displaystyle \left\{A\in {\mathcal{D}}\mid \text{Hom}(A,B)=0\text{ for any }B\in {\mathcal{B}}\right\}\!.\nonumber\end{eqnarray}$$
Then
![]() ${\mathcal{B}}$
is defined to be left (resp. right) admissible if the inclusion functor
${\mathcal{B}}$
is defined to be left (resp. right) admissible if the inclusion functor
![]() ${\mathcal{B}}{\hookrightarrow}{\mathcal{D}}$
has a left (resp. right) adjoint.
${\mathcal{B}}{\hookrightarrow}{\mathcal{D}}$
has a left (resp. right) adjoint.
![]() ${\mathcal{B}}$
is said to be admissible if it is left and right admissible. It is known (cf. [Reference BondalBo, Lemma 3.1]) that
${\mathcal{B}}$
is said to be admissible if it is left and right admissible. It is known (cf. [Reference BondalBo, Lemma 3.1]) that
![]() ${\mathcal{B}}$
is left (resp. right) admissible if and only if the pair
${\mathcal{B}}$
is left (resp. right) admissible if and only if the pair
![]() $({\mathcal{B}},^{\bot }{\mathcal{B}})$
(resp.
$({\mathcal{B}},^{\bot }{\mathcal{B}})$
(resp.
![]() $({\mathcal{B}}^{\bot },{\mathcal{B}})$
) is a semi-orthogonal decomposition. Here, a pair
$({\mathcal{B}}^{\bot },{\mathcal{B}})$
) is a semi-orthogonal decomposition. Here, a pair
![]() $({\mathcal{B}},{\mathcal{C}})$
of full triangulated subcategories of
$({\mathcal{B}},{\mathcal{C}})$
of full triangulated subcategories of
![]() ${\mathcal{D}}$
is a semi-orthogonal decomposition if
${\mathcal{D}}$
is a semi-orthogonal decomposition if
-
∙ for
 $B\in {\mathcal{B}}$
and
$B\in {\mathcal{B}}$
and
 $C\in {\mathcal{C}}$
, we have
$C\in {\mathcal{C}}$
, we have
 $\text{Hom}(C,B)=0$
,
$\text{Hom}(C,B)=0$
, -
∙ any
 $A\in {\mathcal{D}}$
fits in a triangle (2.3)with
$A\in {\mathcal{D}}$
fits in a triangle (2.3)with $$\begin{eqnarray}C\rightarrow A\rightarrow B,\end{eqnarray}$$
$$\begin{eqnarray}C\rightarrow A\rightarrow B,\end{eqnarray}$$
 $C\in {\mathcal{C}}$
and
$C\in {\mathcal{C}}$
and
 $B\in {\mathcal{B}}$
. (One can easily see that
$B\in {\mathcal{B}}$
. (One can easily see that
 $B$
and
$B$
and
 $C$
are determined uniquely up to unique isomorphism.)
$C$
are determined uniquely up to unique isomorphism.)
Assume that
![]() ${\mathcal{B}}$
is admissible. For
${\mathcal{B}}$
is admissible. For
![]() $A\in ^{\bot }{\mathcal{B}}$
, we define
$A\in ^{\bot }{\mathcal{B}}$
, we define
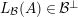 $L_{{\mathcal{B}}}(A)\in {\mathcal{B}}^{\bot }$
to be the object determined by the triangle
$L_{{\mathcal{B}}}(A)\in {\mathcal{B}}^{\bot }$
to be the object determined by the triangle
 $$\begin{eqnarray}B\rightarrow A\rightarrow L_{{\mathcal{B}}}(A)\end{eqnarray}$$
$$\begin{eqnarray}B\rightarrow A\rightarrow L_{{\mathcal{B}}}(A)\end{eqnarray}$$
with
![]() $B\in {\mathcal{B}}$
.
$B\in {\mathcal{B}}$
.
![]() $L_{{\mathcal{B}}}(A)$
is called a mutation of
$L_{{\mathcal{B}}}(A)$
is called a mutation of
![]() $A$
through
$A$
through
![]() ${\mathcal{B}}$
. Similarly, for
${\mathcal{B}}$
. Similarly, for
![]() $A\in {\mathcal{B}}^{\bot }$
, we define
$A\in {\mathcal{B}}^{\bot }$
, we define
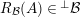 $R_{{\mathcal{B}}}(A)\in ^{\bot }{\mathcal{B}}$
to be the object determined by the triangle
$R_{{\mathcal{B}}}(A)\in ^{\bot }{\mathcal{B}}$
to be the object determined by the triangle
 $$\begin{eqnarray}R_{{\mathcal{B}}}(A)\rightarrow A\rightarrow B\end{eqnarray}$$
$$\begin{eqnarray}R_{{\mathcal{B}}}(A)\rightarrow A\rightarrow B\end{eqnarray}$$
with
![]() $B\in {\mathcal{B}}$
.
$B\in {\mathcal{B}}$
.
![]() $R_{{\mathcal{B}}}(A)$
is called a right mutation of
$R_{{\mathcal{B}}}(A)$
is called a right mutation of
![]() $A$
through
$A$
through
![]() ${\mathcal{B}}$
.
${\mathcal{B}}$
.
If
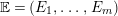 $\mathbb{E}=(E_{1},\ldots ,E_{m})$
is an exceptional collection, the full subcategory
$\mathbb{E}=(E_{1},\ldots ,E_{m})$
is an exceptional collection, the full subcategory
![]() $\langle \mathbb{E}\rangle$
generated by
$\langle \mathbb{E}\rangle$
generated by
![]() $\mathbb{E}$
is admissible (cf. [Reference BondalBo, Theorem 3.2]). We write
$\mathbb{E}$
is admissible (cf. [Reference BondalBo, Theorem 3.2]). We write
![]() $L_{\mathbb{E}}$
and
$L_{\mathbb{E}}$
and
![]() $R_{\mathbb{E}}$
for
$R_{\mathbb{E}}$
for
![]() $L_{\langle \mathbb{E}\rangle }$
and
$L_{\langle \mathbb{E}\rangle }$
and
![]() $R_{\langle \mathbb{E}\rangle }$
.
$R_{\langle \mathbb{E}\rangle }$
.
Definition 2.4. A
![]() $d$
-block exceptional collection is an exceptional collection
$d$
-block exceptional collection is an exceptional collection
![]() $\mathbb{E}$
together with a partition of
$\mathbb{E}$
together with a partition of
![]() $\mathbb{E}$
into
$\mathbb{E}$
into
![]() $d$
subcollections
$d$
subcollections
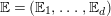 $\mathbb{E}=(\mathbb{E}_{1},\ldots ,\mathbb{E}_{d})$
such that the objects in each block
$\mathbb{E}=(\mathbb{E}_{1},\ldots ,\mathbb{E}_{d})$
such that the objects in each block
![]() $\mathbb{E}_{i}$
are mutually orthogonal, that is, for any
$\mathbb{E}_{i}$
are mutually orthogonal, that is, for any
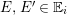 $E,E^{\prime }\in \mathbb{E}_{i}$
, we have
$E,E^{\prime }\in \mathbb{E}_{i}$
, we have
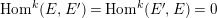 $\text{Hom}^{k}(E,E^{\prime })=\text{Hom}^{k}(E^{\prime },E)=0$
for any
$\text{Hom}^{k}(E,E^{\prime })=\text{Hom}^{k}(E^{\prime },E)=0$
for any
![]() $k$
.
$k$
.
Given a
![]() $d$
-block exceptional collection
$d$
-block exceptional collection
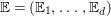 $\mathbb{E}=(\mathbb{E}_{1},\ldots ,\mathbb{E}_{d})$
, we define, for
$\mathbb{E}=(\mathbb{E}_{1},\ldots ,\mathbb{E}_{d})$
, we define, for
 $1<i\leqslant d$
, a
$1<i\leqslant d$
, a
![]() $d$
-block exceptional collection
$d$
-block exceptional collection
![]() $\unicode[STIX]{x1D70F}_{i}^{L}(\mathbb{E})$
to be
$\unicode[STIX]{x1D70F}_{i}^{L}(\mathbb{E})$
to be
 $$\begin{eqnarray}\left(\mathbb{E}_{1},\ldots ,\mathbb{E}_{i-2},L_{\mathbb{E}_{i-1}}(\mathbb{E}_{i})[-1],\mathbb{E}_{i-1},\mathbb{E}_{i+1},\ldots ,\mathbb{E}_{d}\right)\!.\end{eqnarray}$$
$$\begin{eqnarray}\left(\mathbb{E}_{1},\ldots ,\mathbb{E}_{i-2},L_{\mathbb{E}_{i-1}}(\mathbb{E}_{i})[-1],\mathbb{E}_{i-1},\mathbb{E}_{i+1},\ldots ,\mathbb{E}_{d}\right)\!.\end{eqnarray}$$
Similarly for
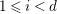 $1\leqslant i<d$
, we define
$1\leqslant i<d$
, we define
![]() $\unicode[STIX]{x1D70F}_{i}^{R}(\mathbb{E})$
to be
$\unicode[STIX]{x1D70F}_{i}^{R}(\mathbb{E})$
to be
 $$\begin{eqnarray}\left(\mathbb{E}_{1},\ldots ,\mathbb{E}_{i-1},\mathbb{E}_{i+1},R_{\mathbb{E}_{i+1}}(\mathbb{E}_{i})[1],\mathbb{E}_{i+2},\ldots ,\mathbb{E}_{d}\right)\!.\end{eqnarray}$$
$$\begin{eqnarray}\left(\mathbb{E}_{1},\ldots ,\mathbb{E}_{i-1},\mathbb{E}_{i+1},R_{\mathbb{E}_{i+1}}(\mathbb{E}_{i})[1],\mathbb{E}_{i+2},\ldots ,\mathbb{E}_{d}\right)\!.\end{eqnarray}$$
We call
![]() $\unicode[STIX]{x1D70F}_{i}^{L}$
and
$\unicode[STIX]{x1D70F}_{i}^{L}$
and
![]() $\unicode[STIX]{x1D70F}_{i}^{R}$
$\unicode[STIX]{x1D70F}_{i}^{R}$
![]() $d$
-block mutation operators.
$d$
-block mutation operators.
Now we specialize to the case
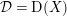 ${\mathcal{D}}=\text{D}(X)$
with
${\mathcal{D}}=\text{D}(X)$
with
![]() $X$
a smooth projective variety of dimension
$X$
a smooth projective variety of dimension
![]() $n$
. If
$n$
. If
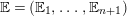 $\mathbb{E}=(\mathbb{E}_{1},\ldots ,\mathbb{E}_{n+1})$
is a full (n+1)-block exceptional collection consisting of exceptional sheaves, then
$\mathbb{E}=(\mathbb{E}_{1},\ldots ,\mathbb{E}_{n+1})$
is a full (n+1)-block exceptional collection consisting of exceptional sheaves, then
![]() $\mathbb{E}$
is strong, and
$\mathbb{E}$
is strong, and
![]() $\unicode[STIX]{x1D70F}_{i}^{L}(\mathbb{E})$
and
$\unicode[STIX]{x1D70F}_{i}^{L}(\mathbb{E})$
and
![]() $\unicode[STIX]{x1D70F}_{i}^{R}(\mathbb{E})$
also consists of exceptional sheaves (cf. [Reference Bridgeland and SternBS, Theorem 4.5]). It follows from this that if
$\unicode[STIX]{x1D70F}_{i}^{R}(\mathbb{E})$
also consists of exceptional sheaves (cf. [Reference Bridgeland and SternBS, Theorem 4.5]). It follows from this that if
 $\mathbb{E}_{i-1}=(E_{\unicode[STIX]{x1D6FC}+1},\ldots ,E_{\unicode[STIX]{x1D6FD}})$
,
$\mathbb{E}_{i-1}=(E_{\unicode[STIX]{x1D6FC}+1},\ldots ,E_{\unicode[STIX]{x1D6FD}})$
,
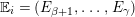 $\mathbb{E}_{i}=(E_{\unicode[STIX]{x1D6FD}+1},\ldots ,E_{\unicode[STIX]{x1D6FE}})$
and
$\mathbb{E}_{i}=(E_{\unicode[STIX]{x1D6FD}+1},\ldots ,E_{\unicode[STIX]{x1D6FE}})$
and
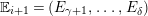 $\mathbb{E}_{i+1}=(E_{\unicode[STIX]{x1D6FE}+1},\ldots ,E_{\unicode[STIX]{x1D6FF}})$
, then for
$\mathbb{E}_{i+1}=(E_{\unicode[STIX]{x1D6FE}+1},\ldots ,E_{\unicode[STIX]{x1D6FF}})$
, then for
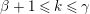 $\unicode[STIX]{x1D6FD}+1\leqslant k\leqslant \unicode[STIX]{x1D6FE}$
, the natural morphism
$\unicode[STIX]{x1D6FD}+1\leqslant k\leqslant \unicode[STIX]{x1D6FE}$
, the natural morphism
 $$\begin{eqnarray}\bigoplus _{l=\unicode[STIX]{x1D6FC}+1}^{\unicode[STIX]{x1D6FD}}\text{Hom}(E_{l},E_{k})\otimes E_{l}\rightarrow E_{k}\end{eqnarray}$$
$$\begin{eqnarray}\bigoplus _{l=\unicode[STIX]{x1D6FC}+1}^{\unicode[STIX]{x1D6FD}}\text{Hom}(E_{l},E_{k})\otimes E_{l}\rightarrow E_{k}\end{eqnarray}$$
is surjective, and
 $L_{\mathbb{E}_{i-1}}(E_{k})[-1]$
is its kernel. Similarly, the natural morphism
$L_{\mathbb{E}_{i-1}}(E_{k})[-1]$
is its kernel. Similarly, the natural morphism
 $$\begin{eqnarray}E_{k}\rightarrow \bigoplus _{l=\unicode[STIX]{x1D6FE}+1}^{\unicode[STIX]{x1D6FF}}\text{Hom}(E_{k},E_{l})^{\ast }\otimes E_{l}\end{eqnarray}$$
$$\begin{eqnarray}E_{k}\rightarrow \bigoplus _{l=\unicode[STIX]{x1D6FE}+1}^{\unicode[STIX]{x1D6FF}}\text{Hom}(E_{k},E_{l})^{\ast }\otimes E_{l}\end{eqnarray}$$
is injective, and
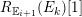 $R_{\mathbb{E}_{i+1}}(E_{k})[1]$
is its cokernel.
$R_{\mathbb{E}_{i+1}}(E_{k})[1]$
is its cokernel.
2.4 Mutation of Symmetric Exceptional bundles
A symmetric exceptional triple is a triple
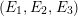 $(E_{1},E_{2},E_{3})$
of symmetric exceptional bundles on
$(E_{1},E_{2},E_{3})$
of symmetric exceptional bundles on
![]() $S$
such that one of
$S$
such that one of
![]() $E_{i}^{\prime }s$
is even, and the other two are (necessarily) odd, and that
$E_{i}^{\prime }s$
is even, and the other two are (necessarily) odd, and that
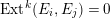 $\text{Ext}^{k}(E_{i},E_{j})=0$
for any
$\text{Ext}^{k}(E_{i},E_{j})=0$
for any
![]() $k$
if
$k$
if
![]() $i>j$
.
$i>j$
.
If
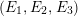 $(E_{1},E_{2},E_{3})$
is a symmetric exceptional triple, and
$(E_{1},E_{2},E_{3})$
is a symmetric exceptional triple, and
![]() $E_{i}$
is an even symmetric exceptional bundle
$E_{i}$
is an even symmetric exceptional bundle
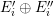 $E_{i}^{\prime }\oplus E_{i}^{\prime \prime }$
, then by substituting
$E_{i}^{\prime }\oplus E_{i}^{\prime \prime }$
, then by substituting
 $\left(E_{i}^{\prime },E_{i}^{\prime \prime }\right)$
for
$\left(E_{i}^{\prime },E_{i}^{\prime \prime }\right)$
for
![]() $E_{i}$
, we obtain a full
$E_{i}$
, we obtain a full
![]() $3$
-block exceptional collection. Conversely, if
$3$
-block exceptional collection. Conversely, if
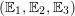 $(\mathbb{E}_{1},\mathbb{E}_{2},\mathbb{E}_{3})$
is a full
$(\mathbb{E}_{1},\mathbb{E}_{2},\mathbb{E}_{3})$
is a full
![]() $3$
-block exceptional collection, then one of the blocks
$3$
-block exceptional collection, then one of the blocks
![]() $\mathbb{E}_{i}$
’s consists of two exceptional bundles and the other two blocks consist of a single exceptional bundle. Say
$\mathbb{E}_{i}$
’s consists of two exceptional bundles and the other two blocks consist of a single exceptional bundle. Say
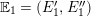 $\mathbb{E}_{1}=(E_{1}^{\prime },E_{1}^{\prime \prime })$
and
$\mathbb{E}_{1}=(E_{1}^{\prime },E_{1}^{\prime \prime })$
and
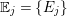 $\mathbb{E}_{j}=\{E_{j}\}$
,
$\mathbb{E}_{j}=\{E_{j}\}$
,
![]() $j=2,3$
. Then
$j=2,3$
. Then
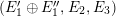 $(E_{1}^{\prime }\oplus E_{1}^{\prime \prime },E_{2},E_{3})$
is a symmetric exceptional triple if the sheaves in the triple are symmetric. By this correspondence between symmetric exceptional triples and full
$(E_{1}^{\prime }\oplus E_{1}^{\prime \prime },E_{2},E_{3})$
is a symmetric exceptional triple if the sheaves in the triple are symmetric. By this correspondence between symmetric exceptional triples and full
![]() $3$
-block exceptional collections such that the direct sum of bundles in each block is symmetric, we can apply
$3$
-block exceptional collections such that the direct sum of bundles in each block is symmetric, we can apply
![]() $3$
-block mutation operators
$3$
-block mutation operators
![]() $\unicode[STIX]{x1D70F}_{i}^{L}$
,
$\unicode[STIX]{x1D70F}_{i}^{L}$
,
![]() $\unicode[STIX]{x1D70F}_{i}^{R}$
for symmetric exceptional triples. For example, for a symmetric exceptional triple
$\unicode[STIX]{x1D70F}_{i}^{R}$
for symmetric exceptional triples. For example, for a symmetric exceptional triple
 ${\mathcal{E}}=(E_{1},E_{2},E_{3})$
,
${\mathcal{E}}=(E_{1},E_{2},E_{3})$
,
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D70F}_{3}^{L}({\mathcal{E}})=\left(E_{1},L_{E_{2}}(E_{3})[-1],E_{2}\right)\!,\qquad \unicode[STIX]{x1D70F}_{1}^{R}({\mathcal{E}})=\left(E_{2},R_{E_{2}}(E_{1})[1],E_{3}\right)\!, & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D70F}_{3}^{L}({\mathcal{E}})=\left(E_{1},L_{E_{2}}(E_{3})[-1],E_{2}\right)\!,\qquad \unicode[STIX]{x1D70F}_{1}^{R}({\mathcal{E}})=\left(E_{2},R_{E_{2}}(E_{1})[1],E_{3}\right)\!, & & \displaystyle \nonumber\end{eqnarray}$$
where if
![]() $E$
is an even symmetric exceptional bundle
$E$
is an even symmetric exceptional bundle
 $E^{\prime }\oplus E^{\prime \prime }$
, then
$E^{\prime }\oplus E^{\prime \prime }$
, then
![]() $L_{E}$
and
$L_{E}$
and
![]() $R_{E}$
are understood to be
$R_{E}$
are understood to be
 $L_{\langle E^{\prime },E^{\prime \prime }\rangle }$
and
$L_{\langle E^{\prime },E^{\prime \prime }\rangle }$
and
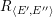 $R_{\langle E^{\prime },E^{\prime \prime }\rangle }$
, respectively.
$R_{\langle E^{\prime },E^{\prime \prime }\rangle }$
, respectively.
2.5 Construction of Symmetric Exceptional bundles
Let
![]() ${\mathcal{S}}{\mathcal{E}}$
be the set of all symmetric exceptional bundles. Put
${\mathcal{S}}{\mathcal{E}}$
be the set of all symmetric exceptional bundles. Put
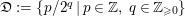 $\mathfrak{D}:=\{p/2^{q}\mid p\in \mathbb{Z},\;q\in \mathbb{Z}_{{\geqslant}0}\}$
. We define a map
$\mathfrak{D}:=\{p/2^{q}\mid p\in \mathbb{Z},\;q\in \mathbb{Z}_{{\geqslant}0}\}$
. We define a map
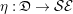 $\unicode[STIX]{x1D702}:\mathfrak{D}\rightarrow {\mathcal{S}}{\mathcal{E}}$
, by induction on
$\unicode[STIX]{x1D702}:\mathfrak{D}\rightarrow {\mathcal{S}}{\mathcal{E}}$
, by induction on
![]() $q$
, such that for odd
$q$
, such that for odd
![]() $p\in \mathbb{Z}$
and
$p\in \mathbb{Z}$
and
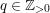 $q\in \mathbb{Z}_{{>}0}$
,
$q\in \mathbb{Z}_{{>}0}$
,
 $$\begin{eqnarray}\left(\unicode[STIX]{x1D702}\biggl(\frac{p-1}{2^{q}}\biggr),\unicode[STIX]{x1D702}\biggl(\frac{p}{2^{q}}\biggr),\unicode[STIX]{x1D702}\biggl(\frac{p+1}{2^{q}}\biggr)\right)\end{eqnarray}$$
$$\begin{eqnarray}\left(\unicode[STIX]{x1D702}\biggl(\frac{p-1}{2^{q}}\biggr),\unicode[STIX]{x1D702}\biggl(\frac{p}{2^{q}}\biggr),\unicode[STIX]{x1D702}\biggl(\frac{p+1}{2^{q}}\biggr)\right)\end{eqnarray}$$
is a symmetric exceptional triple. For
![]() $p\in \mathbb{Z}$
, we define
$p\in \mathbb{Z}$
, we define
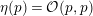 $\unicode[STIX]{x1D702}(p)={\mathcal{O}}(p,p)$
. For odd
$\unicode[STIX]{x1D702}(p)={\mathcal{O}}(p,p)$
. For odd
![]() $p\in \mathbb{Z}$
, we define
$p\in \mathbb{Z}$
, we define
 $$\begin{eqnarray}\unicode[STIX]{x1D702}(p/2)={\mathcal{O}}\left(\frac{p-1}{2},\frac{p+1}{2}\right)\oplus {\mathcal{O}}\left(\frac{p+1}{2},\frac{p-1}{2}\right)\!.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D702}(p/2)={\mathcal{O}}\left(\frac{p-1}{2},\frac{p+1}{2}\right)\oplus {\mathcal{O}}\left(\frac{p+1}{2},\frac{p-1}{2}\right)\!.\end{eqnarray}$$
Let
![]() $p$
be an odd integer and
$p$
be an odd integer and
![]() $q\geqslant 2$
. If
$q\geqslant 2$
. If
 $p\equiv 1~(\text{mod}~4)$
, then
$p\equiv 1~(\text{mod}~4)$
, then
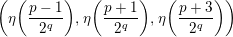 $$\begin{eqnarray}\left(\unicode[STIX]{x1D702}\biggl(\frac{p-1}{2^{q}}\biggr),\unicode[STIX]{x1D702}\biggl(\frac{p+1}{2^{q}}\biggr),\unicode[STIX]{x1D702}\biggl(\frac{p+3}{2^{q}}\biggr)\right)\end{eqnarray}$$
$$\begin{eqnarray}\left(\unicode[STIX]{x1D702}\biggl(\frac{p-1}{2^{q}}\biggr),\unicode[STIX]{x1D702}\biggl(\frac{p+1}{2^{q}}\biggr),\unicode[STIX]{x1D702}\biggl(\frac{p+3}{2^{q}}\biggr)\right)\end{eqnarray}$$
is a symmetric exceptional triple, so we define
![]() $\unicode[STIX]{x1D702}(p/2^{q})$
by
$\unicode[STIX]{x1D702}(p/2^{q})$
by
 $$\begin{eqnarray}\left(\unicode[STIX]{x1D702}\biggl(\frac{p-1}{2^{q}}\biggr),\unicode[STIX]{x1D702}\biggl(\frac{p}{2^{q}}\biggr),\unicode[STIX]{x1D702}\biggl(\frac{p+1}{2^{q}}\biggr)\right)=\unicode[STIX]{x1D70F}_{3}^{L}\left(\text{the triple (2.4)}\right)\!.\end{eqnarray}$$
$$\begin{eqnarray}\left(\unicode[STIX]{x1D702}\biggl(\frac{p-1}{2^{q}}\biggr),\unicode[STIX]{x1D702}\biggl(\frac{p}{2^{q}}\biggr),\unicode[STIX]{x1D702}\biggl(\frac{p+1}{2^{q}}\biggr)\right)=\unicode[STIX]{x1D70F}_{3}^{L}\left(\text{the triple (2.4)}\right)\!.\end{eqnarray}$$
If
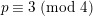 $p\equiv 3~(\text{mod}~4)$
, then
$p\equiv 3~(\text{mod}~4)$
, then
 $$\begin{eqnarray}\left(\unicode[STIX]{x1D702}\biggl(\frac{p-3}{2^{q}}\biggr),\unicode[STIX]{x1D702}\biggl(\frac{p-1}{2^{q}}\biggr),\unicode[STIX]{x1D702}\biggl(\frac{p+1}{2^{q}}\biggr)\right)\end{eqnarray}$$
$$\begin{eqnarray}\left(\unicode[STIX]{x1D702}\biggl(\frac{p-3}{2^{q}}\biggr),\unicode[STIX]{x1D702}\biggl(\frac{p-1}{2^{q}}\biggr),\unicode[STIX]{x1D702}\biggl(\frac{p+1}{2^{q}}\biggr)\right)\end{eqnarray}$$
is a symmetric exceptional triple, so we define
![]() $\unicode[STIX]{x1D702}(p/2^{q})$
by
$\unicode[STIX]{x1D702}(p/2^{q})$
by
 $$\begin{eqnarray}\left(\unicode[STIX]{x1D702}\biggl(\frac{p-1}{2^{q}}\biggr),\unicode[STIX]{x1D702}\biggl(\frac{p}{2^{q}}\biggr),\unicode[STIX]{x1D702}\biggl(\frac{p+1}{2^{q}}\biggr)\right)=\unicode[STIX]{x1D70F}_{1}^{R}\left(\text{the triple (2.5)}\right)\!.\end{eqnarray}$$
$$\begin{eqnarray}\left(\unicode[STIX]{x1D702}\biggl(\frac{p-1}{2^{q}}\biggr),\unicode[STIX]{x1D702}\biggl(\frac{p}{2^{q}}\biggr),\unicode[STIX]{x1D702}\biggl(\frac{p+1}{2^{q}}\biggr)\right)=\unicode[STIX]{x1D70F}_{1}^{R}\left(\text{the triple (2.5)}\right)\!.\end{eqnarray}$$
Rudakov proved the following theorem (cf. [Reference RudakovR89, Theorem 6.5]).
Theorem 2.5. The map
![]() $\unicode[STIX]{x1D702}$
is bijective.
$\unicode[STIX]{x1D702}$
is bijective.
2.6 Numerics of Symmetric Exceptional bundles
If
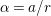 $\unicode[STIX]{x1D6FC}=a/r$
with
$\unicode[STIX]{x1D6FC}=a/r$
with
![]() $r>0$
is an irreducible fraction, we call
$r>0$
is an irreducible fraction, we call
![]() $r$
the rank of
$r$
the rank of
![]() $\unicode[STIX]{x1D6FC}$
and denote it by
$\unicode[STIX]{x1D6FC}$
and denote it by
![]() $r_{\unicode[STIX]{x1D6FC}}$
; we define the discriminant of
$r_{\unicode[STIX]{x1D6FC}}$
; we define the discriminant of
![]() $\unicode[STIX]{x1D6FC}$
by
$\unicode[STIX]{x1D6FC}$
by
 $$\begin{eqnarray}\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}=\left\{\begin{array}{@{}ll@{}}\displaystyle {\displaystyle \frac{1}{2}}\left(1-\frac{1}{r^{2}}\right)\quad & \text{if }r\text{ is odd},\\ \displaystyle {\displaystyle \frac{1}{2}}\left(1-\frac{2}{r^{2}}\right)\quad & \text{if }r\text{ is even}.\end{array}\right.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}=\left\{\begin{array}{@{}ll@{}}\displaystyle {\displaystyle \frac{1}{2}}\left(1-\frac{1}{r^{2}}\right)\quad & \text{if }r\text{ is odd},\\ \displaystyle {\displaystyle \frac{1}{2}}\left(1-\frac{2}{r^{2}}\right)\quad & \text{if }r\text{ is even}.\end{array}\right.\end{eqnarray}$$
We can see that if
![]() $E$
is a symmetric exceptional bundle, then
$E$
is a symmetric exceptional bundle, then
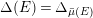 $\unicode[STIX]{x1D6E5}(E)=\unicode[STIX]{x1D6E5}_{\bar{\unicode[STIX]{x1D707}}(E)}$
. For
$\unicode[STIX]{x1D6E5}(E)=\unicode[STIX]{x1D6E5}_{\bar{\unicode[STIX]{x1D707}}(E)}$
. For
![]() $\unicode[STIX]{x1D6FC}$
,
$\unicode[STIX]{x1D6FC}$
,
![]() $\unicode[STIX]{x1D6FD}\in \mathbb{Q}$
with
$\unicode[STIX]{x1D6FD}\in \mathbb{Q}$
with
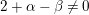 $2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}\neq 0$
, we define
$2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}\neq 0$
, we define
 $$\begin{eqnarray}\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}=\frac{\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD}}{2}+\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}=\frac{\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD}}{2}+\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}.\end{eqnarray}$$
We define a map
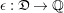 $\unicode[STIX]{x1D716}:\mathfrak{D}\rightarrow \mathbb{Q}$
inductively on
$\unicode[STIX]{x1D716}:\mathfrak{D}\rightarrow \mathbb{Q}$
inductively on
![]() $q$
as follows. For
$q$
as follows. For
![]() $p\in \mathbb{Z}$
, we define
$p\in \mathbb{Z}$
, we define
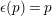 $\unicode[STIX]{x1D716}(p)=p$
. For
$\unicode[STIX]{x1D716}(p)=p$
. For
![]() $p/2^{q}$
with
$p/2^{q}$
with
![]() $p$
odd and
$p$
odd and
![]() $q>0$
, we define
$q>0$
, we define
 $$\begin{eqnarray}\unicode[STIX]{x1D716}\left(\frac{p}{2^{q}}\right)=\unicode[STIX]{x1D716}\left(\frac{p-1}{2^{q}}\right)\!.\unicode[STIX]{x1D716}\left(\frac{p+1}{2^{q}}\right)\!.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D716}\left(\frac{p}{2^{q}}\right)=\unicode[STIX]{x1D716}\left(\frac{p-1}{2^{q}}\right)\!.\unicode[STIX]{x1D716}\left(\frac{p+1}{2^{q}}\right)\!.\end{eqnarray}$$
From the lemma below, we see that
![]() $\unicode[STIX]{x1D716}$
is a strictly increasing function. For
$\unicode[STIX]{x1D716}$
is a strictly increasing function. For
![]() $\unicode[STIX]{x1D6FC}$
,
$\unicode[STIX]{x1D6FC}$
,
![]() $\unicode[STIX]{x1D6FD}\in \mathbb{Q}$
, we put
$\unicode[STIX]{x1D6FD}\in \mathbb{Q}$
, we put
 $$\begin{eqnarray}\unicode[STIX]{x1D712}(\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD}):=r_{\unicode[STIX]{x1D6FC}}r_{\unicode[STIX]{x1D6FD}}\left(\text{P}(\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}\right)\!.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D712}(\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD}):=r_{\unicode[STIX]{x1D6FC}}r_{\unicode[STIX]{x1D6FD}}\left(\text{P}(\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}\right)\!.\end{eqnarray}$$
Lemma 2.6. Let
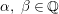 $\unicode[STIX]{x1D6FC},\,\unicode[STIX]{x1D6FD}\in \mathbb{Q}$
satisfy
$\unicode[STIX]{x1D6FC},\,\unicode[STIX]{x1D6FD}\in \mathbb{Q}$
satisfy
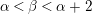 $\unicode[STIX]{x1D6FC}<\unicode[STIX]{x1D6FD}<\unicode[STIX]{x1D6FC}+2$
and
$\unicode[STIX]{x1D6FC}<\unicode[STIX]{x1D6FD}<\unicode[STIX]{x1D6FC}+2$
and
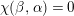 $\unicode[STIX]{x1D712}(\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FC})=0$
.
$\unicode[STIX]{x1D712}(\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FC})=0$
.
-
(1) If
 $\unicode[STIX]{x1D6FE}\in \mathbb{Q}$
satisfies
$\unicode[STIX]{x1D6FE}\in \mathbb{Q}$
satisfies
 $\unicode[STIX]{x1D712}(\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FE})=\unicode[STIX]{x1D712}(\unicode[STIX]{x1D6FE},\unicode[STIX]{x1D6FC})=0$
, then
$\unicode[STIX]{x1D712}(\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FE})=\unicode[STIX]{x1D712}(\unicode[STIX]{x1D6FE},\unicode[STIX]{x1D6FC})=0$
, then
 $\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}$
.
$\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}$
. -
(2) We have
(2.6)In particular, we have $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC}=\frac{1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})},\qquad \unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}=\frac{1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}. & & \displaystyle\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC}=\frac{1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})},\qquad \unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}=\frac{1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}. & & \displaystyle\end{eqnarray}$$
 $\unicode[STIX]{x1D6FC}<\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}<\unicode[STIX]{x1D6FD}$
.
$\unicode[STIX]{x1D6FC}<\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}<\unicode[STIX]{x1D6FD}$
.
-
(3) We have
 $$\begin{eqnarray}1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}}=\frac{(1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}})(1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}})}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}}.\end{eqnarray}$$
$$\begin{eqnarray}1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}}=\frac{(1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}})(1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}})}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}}.\end{eqnarray}$$
-
(4) We have
(2.7) $$\begin{eqnarray}\displaystyle & \displaystyle \frac{\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}}{2}+\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}}}{2(\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC})}=\unicode[STIX]{x1D6FD}-1 & \displaystyle\end{eqnarray}$$
(2.8)
$$\begin{eqnarray}\displaystyle & \displaystyle \frac{\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}}{2}+\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}}}{2(\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC})}=\unicode[STIX]{x1D6FD}-1 & \displaystyle\end{eqnarray}$$
(2.8) $$\begin{eqnarray}\displaystyle & \displaystyle \frac{\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}+\unicode[STIX]{x1D6FD}}{2}+\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}}{2(\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD})}=\unicode[STIX]{x1D6FC}+1. & \displaystyle\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle \frac{\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}+\unicode[STIX]{x1D6FD}}{2}+\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}}{2(\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD})}=\unicode[STIX]{x1D6FC}+1. & \displaystyle\end{eqnarray}$$
-
(5) We have
(2.9) $$\begin{eqnarray}\displaystyle & \displaystyle \left(\frac{\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}}{2}\right)^{2}-\frac{\text{P}(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD})}{2}+\left(\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}}}{2(\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC})}\right)^{2}=\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}} & \displaystyle\end{eqnarray}$$
(2.10)
$$\begin{eqnarray}\displaystyle & \displaystyle \left(\frac{\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}}{2}\right)^{2}-\frac{\text{P}(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD})}{2}+\left(\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}}}{2(\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC})}\right)^{2}=\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}} & \displaystyle\end{eqnarray}$$
(2.10) $$\begin{eqnarray}\displaystyle & \displaystyle \left(\frac{\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FD}}{2}\right)^{2}-\frac{\text{P}(\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FD})}{2}+\left(\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}}{2(\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD})}\right)^{2}=\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}. & \displaystyle\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle \left(\frac{\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FD}}{2}\right)^{2}-\frac{\text{P}(\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FD})}{2}+\left(\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}}{2(\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD})}\right)^{2}=\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}. & \displaystyle\end{eqnarray}$$
Proof. (1) This follows from
 $$\begin{eqnarray}\displaystyle & \displaystyle \text{P}(\unicode[STIX]{x1D6FE}-\unicode[STIX]{x1D6FD})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}=0, & \displaystyle \nonumber\\ \displaystyle & \displaystyle \text{P}(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}=0. & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle \text{P}(\unicode[STIX]{x1D6FE}-\unicode[STIX]{x1D6FD})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}=0, & \displaystyle \nonumber\\ \displaystyle & \displaystyle \text{P}(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}=0. & \displaystyle \nonumber\end{eqnarray}$$
(2) Since
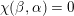 $\unicode[STIX]{x1D712}(\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FC})=0$
, we have
$\unicode[STIX]{x1D712}(\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FC})=0$
, we have
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}} & = & \displaystyle 2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-\text{P}(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})\nonumber\\ \displaystyle & = & \displaystyle 2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-1+(\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC})(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}).\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}} & = & \displaystyle 2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-\text{P}(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})\nonumber\\ \displaystyle & = & \displaystyle 2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-1+(\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC})(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}).\nonumber\end{eqnarray}$$
Thus
 $$\begin{eqnarray}\frac{1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}=\frac{\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC}}{2}+\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}=\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC}.\end{eqnarray}$$
$$\begin{eqnarray}\frac{1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}=\frac{\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC}}{2}+\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}=\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC}.\end{eqnarray}$$
The proof of the other equality is similar. Since the discriminants are less than
![]() $1/2$
, the inequalities follow.
$1/2$
, the inequalities follow.
(3) We have
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}} & = & \displaystyle \text{P}(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}\nonumber\\ \displaystyle & = & \displaystyle \left(\frac{\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}}{2}+1-\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)^{2}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{4}(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}+2)^{2}-\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}+\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}}{2}+\left(\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)^{2}\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{4}(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}+2)^{2}-\frac{1}{2}(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}+1)^{2}+\left(\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)^{2}\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{2}-\frac{(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}}{4}+\left(\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)^{2}\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{2}-\left(\frac{\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}}{2}-\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)\left(\frac{\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}}{2}+\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{2}-\left(\frac{2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-1}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)\left(\frac{2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-1}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)\!.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}} & = & \displaystyle \text{P}(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}\nonumber\\ \displaystyle & = & \displaystyle \left(\frac{\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}}{2}+1-\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)^{2}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{4}(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}+2)^{2}-\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}+\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}}{2}+\left(\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)^{2}\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{4}(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}+2)^{2}-\frac{1}{2}(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}+1)^{2}+\left(\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)^{2}\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{2}-\frac{(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})^{2}}{4}+\left(\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)^{2}\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{2}-\left(\frac{\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}}{2}-\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)\left(\frac{\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}}{2}+\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{2}-\left(\frac{2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-1}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)\left(\frac{2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-1}{2(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)\!.\nonumber\end{eqnarray}$$
Here in the first equality we used the equality
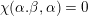 $\unicode[STIX]{x1D712}(\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FC})=0$
.
$\unicode[STIX]{x1D712}(\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FC})=0$
.
(4) We prove the first equality. From
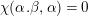 $\unicode[STIX]{x1D712}(\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FC})=0$
, we obtain
$\unicode[STIX]{x1D712}(\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FC})=0$
, we obtain
 $$\begin{eqnarray}(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD})(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}+2)+\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}}=2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-1.\end{eqnarray}$$
$$\begin{eqnarray}(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD})(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}+2)+\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}}=2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-1.\end{eqnarray}$$
Then we have
 $$\begin{eqnarray}\displaystyle \frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}}}{2(\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC})} & = & \displaystyle \frac{2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-1}{2(\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC})}+\frac{\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}+2}{2}\nonumber\\ \displaystyle & = & \displaystyle \unicode[STIX]{x1D6FD}-1-\frac{\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}}{2},\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}}}{2(\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC})} & = & \displaystyle \frac{2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-1}{2(\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC})}+\frac{\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}+2}{2}\nonumber\\ \displaystyle & = & \displaystyle \unicode[STIX]{x1D6FD}-1-\frac{\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}}{2},\nonumber\end{eqnarray}$$
where we used (2.6) in the second equality.
(5) We prove the first equality. Put
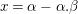 $x=\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}$
. Using (2.7), we have
$x=\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}$
. Using (2.7), we have
 $$\begin{eqnarray}\displaystyle \text{LHS. of (2.9)} & = & \displaystyle \left(\frac{x}{2}\right)^{2}-\frac{(x+1)^{2}}{2}+\left(\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC}-1+\frac{x}{2}\right)^{2}\nonumber\\ \displaystyle & = & \displaystyle (\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC}-2)x+(\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC}-1)^{2}-\frac{1}{2}\nonumber\\ \displaystyle & = & \displaystyle -\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}+\text{P}(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})=\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}},\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \text{LHS. of (2.9)} & = & \displaystyle \left(\frac{x}{2}\right)^{2}-\frac{(x+1)^{2}}{2}+\left(\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC}-1+\frac{x}{2}\right)^{2}\nonumber\\ \displaystyle & = & \displaystyle (\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC}-2)x+(\unicode[STIX]{x1D6FD}-\unicode[STIX]{x1D6FC}-1)^{2}-\frac{1}{2}\nonumber\\ \displaystyle & = & \displaystyle -\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}+\text{P}(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})=\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}},\nonumber\end{eqnarray}$$
where we used (2.6) in the third equality.◻
Proposition 2.7. We have
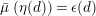 $\bar{\unicode[STIX]{x1D707}}\left(\unicode[STIX]{x1D702}(d)\right)=\unicode[STIX]{x1D716}(d)$
for
$\bar{\unicode[STIX]{x1D707}}\left(\unicode[STIX]{x1D702}(d)\right)=\unicode[STIX]{x1D716}(d)$
for
![]() $d\in \mathfrak{D}$
.
$d\in \mathfrak{D}$
.
Proof. Let
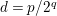 $d=p/2^{q}$
with
$d=p/2^{q}$
with
![]() $p$
odd. When
$p$
odd. When
![]() $q=0,1$
, the equality holds because
$q=0,1$
, the equality holds because
 $\bar{\unicode[STIX]{x1D707}}\left({\mathcal{O}}(p,p)\right)=p$
, and
$\bar{\unicode[STIX]{x1D707}}\left({\mathcal{O}}(p,p)\right)=p$
, and
 $$\begin{eqnarray}\bar{\unicode[STIX]{x1D707}}\left({\mathcal{O}}\left(\frac{p-1}{2},\frac{p+1}{2}\right)\oplus {\mathcal{O}}\left(\frac{p+1}{2},\frac{p-1}{2}\right)\right)=\frac{p}{2}.\end{eqnarray}$$
$$\begin{eqnarray}\bar{\unicode[STIX]{x1D707}}\left({\mathcal{O}}\left(\frac{p-1}{2},\frac{p+1}{2}\right)\oplus {\mathcal{O}}\left(\frac{p+1}{2},\frac{p-1}{2}\right)\right)=\frac{p}{2}.\end{eqnarray}$$
For
![]() $q\geqslant 2$
, the equality follows by induction on
$q\geqslant 2$
, the equality follows by induction on
![]() $q$
using Lemma 2.6(1).◻
$q$
using Lemma 2.6(1).◻
Notation 2.8. Put
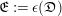 $\mathfrak{E}:=\unicode[STIX]{x1D716}(\mathfrak{D})$
. This is the set of
$\mathfrak{E}:=\unicode[STIX]{x1D716}(\mathfrak{D})$
. This is the set of
![]() $\bar{\unicode[STIX]{x1D707}}$
’s of symmetric exceptional bundles. We call elements of
$\bar{\unicode[STIX]{x1D707}}$
’s of symmetric exceptional bundles. We call elements of
![]() $\mathfrak{E}$
symmetric exceptional slopes. A symmetric exceptional bundle is determined uniquely by its value of
$\mathfrak{E}$
symmetric exceptional slopes. A symmetric exceptional bundle is determined uniquely by its value of
![]() $\bar{\unicode[STIX]{x1D707}}$
; for
$\bar{\unicode[STIX]{x1D707}}$
; for
![]() $\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
,
$\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
,
![]() $E_{\unicode[STIX]{x1D6FC}}$
denotes the symmetric exceptional bundle such that
$E_{\unicode[STIX]{x1D6FC}}$
denotes the symmetric exceptional bundle such that
 $\bar{\unicode[STIX]{x1D707}}(E_{\unicode[STIX]{x1D6FC}})=\unicode[STIX]{x1D6FC}$
. We say that a symmetric exceptional slope
$\bar{\unicode[STIX]{x1D707}}(E_{\unicode[STIX]{x1D6FC}})=\unicode[STIX]{x1D6FC}$
. We say that a symmetric exceptional slope
![]() $\unicode[STIX]{x1D6FC}$
is even (resp. odd) if
$\unicode[STIX]{x1D6FC}$
is even (resp. odd) if
![]() $r_{\unicode[STIX]{x1D6FC}}$
is even (resp. odd). (N.B. If
$r_{\unicode[STIX]{x1D6FC}}$
is even (resp. odd). (N.B. If
![]() $\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
is an integer, it is odd as a symmetric exceptional slope. This might be a little confusing because
$\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
is an integer, it is odd as a symmetric exceptional slope. This might be a little confusing because
![]() $\unicode[STIX]{x1D6FC}$
can be an even integer. But in this paper we do not mention the parity as an integer, so it does not cause confusion.)
$\unicode[STIX]{x1D6FC}$
can be an even integer. But in this paper we do not mention the parity as an integer, so it does not cause confusion.)
3 Existence of Semistable sheaves
In this section, we determine the Chern classes of semistable sheaves with symmetric
![]() $c_{1}$
on
$c_{1}$
on
![]() $S$
.
$S$
.
Lemma 3.1. Let
![]() $F$
be a semistable sheaf with symmetric
$F$
be a semistable sheaf with symmetric
![]() $c_{1}$
. Assume that
$c_{1}$
. Assume that
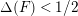 $\unicode[STIX]{x1D6E5}(F)<1/2$
. Then
$\unicode[STIX]{x1D6E5}(F)<1/2$
. Then
 $\bar{\unicode[STIX]{x1D707}}(F)\in \mathfrak{E}$
and
$\bar{\unicode[STIX]{x1D707}}(F)\in \mathfrak{E}$
and
![]() $F$
is a direct sum of
$F$
is a direct sum of
![]() $E_{\bar{\unicode[STIX]{x1D707}}(F)}$
.
$E_{\bar{\unicode[STIX]{x1D707}}(F)}$
.
Proof. We proceed by induction on the rank of
![]() $F$
. If
$F$
. If
![]() $F$
is stable, then
$F$
is stable, then
![]() $F$
is an odd symmetric exceptional bundle and we are done. If
$F$
is an odd symmetric exceptional bundle and we are done. If
![]() $F$
is not stable, then let
$F$
is not stable, then let
![]() $F_{1}$
be the first filter of a Jordan–Hölder filtration of
$F_{1}$
be the first filter of a Jordan–Hölder filtration of
![]() $F$
. Let
$F$
. Let
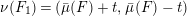 $\unicode[STIX]{x1D708}(F_{1})=(\bar{\unicode[STIX]{x1D707}}(F)+t,\bar{\unicode[STIX]{x1D707}}(F)-t)$
. Since
$\unicode[STIX]{x1D708}(F_{1})=(\bar{\unicode[STIX]{x1D707}}(F)+t,\bar{\unicode[STIX]{x1D707}}(F)-t)$
. Since
 $\unicode[STIX]{x1D712}(F_{1})/\text{r}(F_{1})=\unicode[STIX]{x1D712}(F)/\text{r}(F)$
, we have, by (2.1),
$\unicode[STIX]{x1D712}(F_{1})/\text{r}(F_{1})=\unicode[STIX]{x1D712}(F)/\text{r}(F)$
, we have, by (2.1),
 $$\begin{eqnarray}t^{2}+\unicode[STIX]{x1D6E5}(F_{1})=\unicode[STIX]{x1D6E5}(F).\end{eqnarray}$$
$$\begin{eqnarray}t^{2}+\unicode[STIX]{x1D6E5}(F_{1})=\unicode[STIX]{x1D6E5}(F).\end{eqnarray}$$
So we have
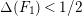 $\unicode[STIX]{x1D6E5}(F_{1})<1/2$
, and
$\unicode[STIX]{x1D6E5}(F_{1})<1/2$
, and
![]() $F_{1}$
is an exceptional bundle. If we let
$F_{1}$
is an exceptional bundle. If we let
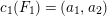 $c_{1}(F_{1})=(a_{1},a_{2})$
, then
$c_{1}(F_{1})=(a_{1},a_{2})$
, then
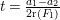 $t=\frac{a_{1}-a_{2}}{2\text{r}(F_{1})}$
. We have
$t=\frac{a_{1}-a_{2}}{2\text{r}(F_{1})}$
. We have
 $$\begin{eqnarray}\frac{1}{2}>\unicode[STIX]{x1D6E5}(F)=\unicode[STIX]{x1D6E5}(F_{1})+t^{2}=\frac{1}{2}-\frac{2-(a_{1}-a_{2})^{2}}{4\text{r}(F_{1})^{2}}.\end{eqnarray}$$
$$\begin{eqnarray}\frac{1}{2}>\unicode[STIX]{x1D6E5}(F)=\unicode[STIX]{x1D6E5}(F_{1})+t^{2}=\frac{1}{2}-\frac{2-(a_{1}-a_{2})^{2}}{4\text{r}(F_{1})^{2}}.\end{eqnarray}$$
Therefore,
 $|a_{1}-a_{2}|=0\text{ or }1$
. If
$|a_{1}-a_{2}|=0\text{ or }1$
. If
 $|a_{1}-a_{2}|=0$
, then
$|a_{1}-a_{2}|=0$
, then
![]() $F_{1}$
is an odd symmetric exceptional bundle.
$F_{1}$
is an odd symmetric exceptional bundle.
![]() $F/F_{1}$
is a semistable sheaf with symmetric
$F/F_{1}$
is a semistable sheaf with symmetric
![]() $c_{1}$
such that
$c_{1}$
such that
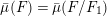 $\bar{\unicode[STIX]{x1D707}}(F)=\bar{\unicode[STIX]{x1D707}}(F/F_{1})$
and
$\bar{\unicode[STIX]{x1D707}}(F)=\bar{\unicode[STIX]{x1D707}}(F/F_{1})$
and
 $\unicode[STIX]{x1D6E5}(F)=\unicode[STIX]{x1D6E5}(F/F_{1})$
. By applying the induction hypothesis to
$\unicode[STIX]{x1D6E5}(F)=\unicode[STIX]{x1D6E5}(F/F_{1})$
. By applying the induction hypothesis to
![]() $F/F_{1}$
, we obtain the result. If
$F/F_{1}$
, we obtain the result. If
 $|a_{1}-a_{2}|=1$
, then
$|a_{1}-a_{2}|=1$
, then
![]() $F_{1}$
is a direct summand of an even symmetric exceptional bundle. Note that
$F_{1}$
is a direct summand of an even symmetric exceptional bundle. Note that
 $$\begin{eqnarray}\frac{\unicode[STIX]{x1D712}(F_{1},F)}{\text{r}(F_{1})\text{r}(F)}=1-t^{2}-\unicode[STIX]{x1D6E5}(F_{1})-\unicode[STIX]{x1D6E5}(F)=1-2\unicode[STIX]{x1D6E5}(F)>0.\end{eqnarray}$$
$$\begin{eqnarray}\frac{\unicode[STIX]{x1D712}(F_{1},F)}{\text{r}(F_{1})\text{r}(F)}=1-t^{2}-\unicode[STIX]{x1D6E5}(F_{1})-\unicode[STIX]{x1D6E5}(F)=1-2\unicode[STIX]{x1D6E5}(F)>0.\end{eqnarray}$$
By this we also have
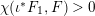 $\unicode[STIX]{x1D712}(\unicode[STIX]{x1D704}^{\ast }F_{1},F)>0$
, so
$\unicode[STIX]{x1D712}(\unicode[STIX]{x1D704}^{\ast }F_{1},F)>0$
, so
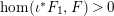 $\text{hom}(\unicode[STIX]{x1D704}^{\ast }F_{1},F)>0$
because
$\text{hom}(\unicode[STIX]{x1D704}^{\ast }F_{1},F)>0$
because
 $\text{Ext}^{2}(\unicode[STIX]{x1D704}^{\ast }F_{1},F)=0$
. Then we can find a subbundle
$\text{Ext}^{2}(\unicode[STIX]{x1D704}^{\ast }F_{1},F)=0$
. Then we can find a subbundle
![]() $G$
of
$G$
of
![]() $F$
that is S-equivalent to
$F$
that is S-equivalent to
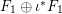 $F_{1}\oplus \unicode[STIX]{x1D704}^{\ast }F_{1}$
. Since
$F_{1}\oplus \unicode[STIX]{x1D704}^{\ast }F_{1}$
. Since
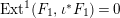 $\text{Ext}^{1}(F_{1},\unicode[STIX]{x1D704}^{\ast }F_{1})=0$
, we have
$\text{Ext}^{1}(F_{1},\unicode[STIX]{x1D704}^{\ast }F_{1})=0$
, we have
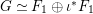 $G\simeq F_{1}\oplus \unicode[STIX]{x1D704}^{\ast }F_{1}$
. Applying the induction hypothesis to
$G\simeq F_{1}\oplus \unicode[STIX]{x1D704}^{\ast }F_{1}$
. Applying the induction hypothesis to
![]() $F/G$
, we obtain the result.◻
$F/G$
, we obtain the result.◻
Proposition 3.2. Let
![]() $F$
be a semistable sheaf on
$F$
be a semistable sheaf on
![]() $S$
with symmetric
$S$
with symmetric
![]() $c_{1}$
. Let
$c_{1}$
. Let
![]() $\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
satisfy
$\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
satisfy
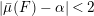 $|\bar{\unicode[STIX]{x1D707}}(F)-\unicode[STIX]{x1D6FC}|<2$
. Then the inequality
$|\bar{\unicode[STIX]{x1D707}}(F)-\unicode[STIX]{x1D6FC}|<2$
. Then the inequality
 $$\begin{eqnarray}\text{P}\left(-|\bar{\unicode[STIX]{x1D707}}(F)-\unicode[STIX]{x1D6FC}|\right)-\unicode[STIX]{x1D6E5}(F)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}\leqslant 0\end{eqnarray}$$
$$\begin{eqnarray}\text{P}\left(-|\bar{\unicode[STIX]{x1D707}}(F)-\unicode[STIX]{x1D6FC}|\right)-\unicode[STIX]{x1D6E5}(F)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}\leqslant 0\end{eqnarray}$$
holds unless
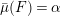 $\bar{\unicode[STIX]{x1D707}}(F)=\unicode[STIX]{x1D6FC}$
and
$\bar{\unicode[STIX]{x1D707}}(F)=\unicode[STIX]{x1D6FC}$
and
![]() $F$
is a direct sum of
$F$
is a direct sum of
![]() $E_{\unicode[STIX]{x1D6FC}}$
.
$E_{\unicode[STIX]{x1D6FC}}$
.
Proof. If
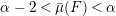 $\unicode[STIX]{x1D6FC}-2<\bar{\unicode[STIX]{x1D707}}(F)<\unicode[STIX]{x1D6FC}$
, then by semistability of
$\unicode[STIX]{x1D6FC}-2<\bar{\unicode[STIX]{x1D707}}(F)<\unicode[STIX]{x1D6FC}$
, then by semistability of
![]() $F$
and
$F$
and
![]() $E_{\unicode[STIX]{x1D6FC}}$
we have
$E_{\unicode[STIX]{x1D6FC}}$
we have
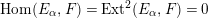 $\text{Hom}(E_{\unicode[STIX]{x1D6FC}},F)=\text{Ext}^{2}(E_{\unicode[STIX]{x1D6FC}},F)=0$
. Thus
$\text{Hom}(E_{\unicode[STIX]{x1D6FC}},F)=\text{Ext}^{2}(E_{\unicode[STIX]{x1D6FC}},F)=0$
. Thus
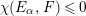 $\unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FC}},F)\leqslant 0$
, which is equivalent to (3.1). A similar argument shows (3.1) when
$\unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FC}},F)\leqslant 0$
, which is equivalent to (3.1). A similar argument shows (3.1) when
 $\unicode[STIX]{x1D6FC}<\bar{\unicode[STIX]{x1D707}}(F)<\unicode[STIX]{x1D6FC}+2$
. Suppose that
$\unicode[STIX]{x1D6FC}<\bar{\unicode[STIX]{x1D707}}(F)<\unicode[STIX]{x1D6FC}+2$
. Suppose that
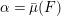 $\unicode[STIX]{x1D6FC}=\bar{\unicode[STIX]{x1D707}}(F)$
and (3.1) does not hold. We have
$\unicode[STIX]{x1D6FC}=\bar{\unicode[STIX]{x1D707}}(F)$
and (3.1) does not hold. We have
 $$\begin{eqnarray}1-\unicode[STIX]{x1D6E5}(F)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}>0.\end{eqnarray}$$
$$\begin{eqnarray}1-\unicode[STIX]{x1D6E5}(F)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}>0.\end{eqnarray}$$
We show that
![]() $F$
is a direct sum of
$F$
is a direct sum of
![]() $E_{\unicode[STIX]{x1D6FC}}$
. Since
$E_{\unicode[STIX]{x1D6FC}}$
. Since
 $\unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FC}},F)>0$
, we have a nonzero morphism
$\unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FC}},F)>0$
, we have a nonzero morphism
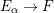 $E_{\unicode[STIX]{x1D6FC}}\rightarrow F$
. If
$E_{\unicode[STIX]{x1D6FC}}\rightarrow F$
. If
![]() $r_{\unicode[STIX]{x1D6FC}}$
is odd, then this is injective by
$r_{\unicode[STIX]{x1D6FC}}$
is odd, then this is injective by
![]() $\unicode[STIX]{x1D707}$
-stability of
$\unicode[STIX]{x1D707}$
-stability of
![]() $E_{\unicode[STIX]{x1D6FC}}$
. By semistability of
$E_{\unicode[STIX]{x1D6FC}}$
. By semistability of
![]() $F$
, we have
$F$
, we have
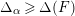 $\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}\geqslant \unicode[STIX]{x1D6E5}(F)$
. If
$\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}\geqslant \unicode[STIX]{x1D6E5}(F)$
. If
![]() $r_{\unicode[STIX]{x1D6FC}}$
is even, then
$r_{\unicode[STIX]{x1D6FC}}$
is even, then
![]() $F$
has a subsheaf isomorphic to
$F$
has a subsheaf isomorphic to
![]() $E_{\unicode[STIX]{x1D6FC}}^{\prime }$
or
$E_{\unicode[STIX]{x1D6FC}}^{\prime }$
or
![]() $E_{\unicode[STIX]{x1D6FC}}^{\prime \prime }$
. Again by semistability of
$E_{\unicode[STIX]{x1D6FC}}^{\prime \prime }$
. Again by semistability of
![]() $F$
, we have
$F$
, we have
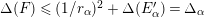 $\unicode[STIX]{x1D6E5}(F)\leqslant (1/r_{\unicode[STIX]{x1D6FC}})^{2}+\unicode[STIX]{x1D6E5}(E_{\unicode[STIX]{x1D6FC}}^{\prime })=\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}$
. In any case,
$\unicode[STIX]{x1D6E5}(F)\leqslant (1/r_{\unicode[STIX]{x1D6FC}})^{2}+\unicode[STIX]{x1D6E5}(E_{\unicode[STIX]{x1D6FC}}^{\prime })=\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}$
. In any case,
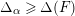 $\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}\geqslant \unicode[STIX]{x1D6E5}(F)$
. From this and (3.2), we have
$\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}\geqslant \unicode[STIX]{x1D6E5}(F)$
. From this and (3.2), we have
 $\unicode[STIX]{x1D6E5}(F)<1/2$
. Then
$\unicode[STIX]{x1D6E5}(F)<1/2$
. Then
![]() $F$
is a direct sum of
$F$
is a direct sum of
![]() $E_{\unicode[STIX]{x1D6FC}}$
by Lemma 3.1.◻
$E_{\unicode[STIX]{x1D6FC}}$
by Lemma 3.1.◻
Remark 3.3. The inequality (3.1) holds for a
![]() $\unicode[STIX]{x1D707}$
-semistable sheaf
$\unicode[STIX]{x1D707}$
-semistable sheaf
![]() $F$
with
$F$
with
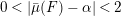 $0<|\bar{\unicode[STIX]{x1D707}}(F)-\unicode[STIX]{x1D6FC}|<2$
. (Indeed, in the above proof, for
$0<|\bar{\unicode[STIX]{x1D707}}(F)-\unicode[STIX]{x1D6FC}|<2$
. (Indeed, in the above proof, for
![]() $F$
with
$F$
with
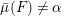 $\bar{\unicode[STIX]{x1D707}}(F)\neq \unicode[STIX]{x1D6FC}$
, only the
$\bar{\unicode[STIX]{x1D707}}(F)\neq \unicode[STIX]{x1D6FC}$
, only the
![]() $\unicode[STIX]{x1D707}$
-semistability is used.) From this, we see that if a
$\unicode[STIX]{x1D707}$
-semistability is used.) From this, we see that if a
![]() $\unicode[STIX]{x1D707}$
-semistable sheaf
$\unicode[STIX]{x1D707}$
-semistable sheaf
![]() $F$
with
$F$
with
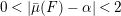 $0<|\bar{\unicode[STIX]{x1D707}}(F)-\unicode[STIX]{x1D6FC}|<2$
satisfies
$0<|\bar{\unicode[STIX]{x1D707}}(F)-\unicode[STIX]{x1D6FC}|<2$
satisfies
 $$\begin{eqnarray}\text{P}\left(-|\bar{\unicode[STIX]{x1D707}}(F)-\unicode[STIX]{x1D6FC}|\right)-\unicode[STIX]{x1D6E5}(F)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}=0,\end{eqnarray}$$
$$\begin{eqnarray}\text{P}\left(-|\bar{\unicode[STIX]{x1D707}}(F)-\unicode[STIX]{x1D6FC}|\right)-\unicode[STIX]{x1D6E5}(F)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}=0,\end{eqnarray}$$
then it is locally free.
For
![]() $\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
, put
$\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
, put
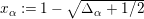 $x_{\unicode[STIX]{x1D6FC}}:=1-\sqrt{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}+1/2}$
, which is the smaller solution of the quadratic equation
$x_{\unicode[STIX]{x1D6FC}}:=1-\sqrt{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}+1/2}$
, which is the smaller solution of the quadratic equation
 $$\begin{eqnarray}\text{P}(-x)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}=\frac{1}{2}.\end{eqnarray}$$
$$\begin{eqnarray}\text{P}(-x)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}=\frac{1}{2}.\end{eqnarray}$$
Put
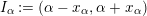 $I_{\unicode[STIX]{x1D6FC}}:=(\unicode[STIX]{x1D6FC}-x_{\unicode[STIX]{x1D6FC}},\unicode[STIX]{x1D6FC}+x_{\unicode[STIX]{x1D6FC}})$
.
$I_{\unicode[STIX]{x1D6FC}}:=(\unicode[STIX]{x1D6FC}-x_{\unicode[STIX]{x1D6FC}},\unicode[STIX]{x1D6FC}+x_{\unicode[STIX]{x1D6FC}})$
.
Proposition 3.4.
![]() $\mathbb{Q}$
is the disjoint union of
$\mathbb{Q}$
is the disjoint union of
![]() $I_{\unicode[STIX]{x1D6FC}}\cap \mathbb{Q}$
(
$I_{\unicode[STIX]{x1D6FC}}\cap \mathbb{Q}$
(
![]() $\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
).
$\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
).
Proof. The argument of the proof of [Reference DrezetD, Théorème 1(1)] applies to our quadratic surface case. Details are left to the reader. ◻
By the above proposition, the union
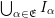 $\bigcup _{\unicode[STIX]{x1D6FC}\in \mathfrak{E}}I_{\unicode[STIX]{x1D6FC}}$
is a disjoint union, and contains the set
$\bigcup _{\unicode[STIX]{x1D6FC}\in \mathfrak{E}}I_{\unicode[STIX]{x1D6FC}}$
is a disjoint union, and contains the set
![]() $\mathbb{Q}$
of rational numbers.
$\mathbb{Q}$
of rational numbers.
We define a function
 $\unicode[STIX]{x1D6FF}:\bigcup _{\unicode[STIX]{x1D6FC}\in \mathfrak{E}}I_{\unicode[STIX]{x1D6FC}}\rightarrow \mathbb{R}$
so that
$\unicode[STIX]{x1D6FF}:\bigcup _{\unicode[STIX]{x1D6FC}\in \mathfrak{E}}I_{\unicode[STIX]{x1D6FC}}\rightarrow \mathbb{R}$
so that
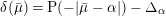 $\unicode[STIX]{x1D6FF}(\bar{\unicode[STIX]{x1D707}})=\text{P}(-|\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FC}|)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}$
for
$\unicode[STIX]{x1D6FF}(\bar{\unicode[STIX]{x1D707}})=\text{P}(-|\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FC}|)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}$
for
![]() $\bar{\unicode[STIX]{x1D707}}\in I_{\unicode[STIX]{x1D6FC}}$
(
$\bar{\unicode[STIX]{x1D707}}\in I_{\unicode[STIX]{x1D6FC}}$
(
![]() $\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
).
$\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
).
Lemma 3.5. Let
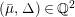 $(\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5})\in \mathbb{Q}^{2}$
satisfy
$(\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5})\in \mathbb{Q}^{2}$
satisfy
 $\unicode[STIX]{x1D6E5}\geqslant \unicode[STIX]{x1D6FF}(\bar{\unicode[STIX]{x1D707}})$
. Then for any
$\unicode[STIX]{x1D6E5}\geqslant \unicode[STIX]{x1D6FF}(\bar{\unicode[STIX]{x1D707}})$
. Then for any
![]() $\unicode[STIX]{x1D6FD}\in \mathfrak{E}$
with
$\unicode[STIX]{x1D6FD}\in \mathfrak{E}$
with
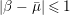 $|\unicode[STIX]{x1D6FD}-\bar{\unicode[STIX]{x1D707}}|\leqslant 1$
, we have
$|\unicode[STIX]{x1D6FD}-\bar{\unicode[STIX]{x1D707}}|\leqslant 1$
, we have
 $$\begin{eqnarray}\text{P}(-|\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FD}|)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}\leqslant \unicode[STIX]{x1D6E5}.\end{eqnarray}$$
$$\begin{eqnarray}\text{P}(-|\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FD}|)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}\leqslant \unicode[STIX]{x1D6E5}.\end{eqnarray}$$
Proof. Let
![]() $\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
be such that
$\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
be such that
![]() $\bar{\unicode[STIX]{x1D707}}\in I_{\unicode[STIX]{x1D6FC}}$
. If
$\bar{\unicode[STIX]{x1D707}}\in I_{\unicode[STIX]{x1D6FC}}$
. If
![]() $\unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D6FC}$
, the inequality (3.3) is nothing but
$\unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D6FC}$
, the inequality (3.3) is nothing but
 $\unicode[STIX]{x1D6E5}\geqslant \unicode[STIX]{x1D6FF}(\bar{\unicode[STIX]{x1D707}})$
. Assume that
$\unicode[STIX]{x1D6E5}\geqslant \unicode[STIX]{x1D6FF}(\bar{\unicode[STIX]{x1D707}})$
. Assume that
![]() $\unicode[STIX]{x1D6FD}\neq \unicode[STIX]{x1D6FC}$
. From the definition of
$\unicode[STIX]{x1D6FD}\neq \unicode[STIX]{x1D6FC}$
. From the definition of
![]() $x_{\unicode[STIX]{x1D6FD}}$
, it follows that for
$x_{\unicode[STIX]{x1D6FD}}$
, it follows that for
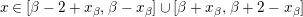 $x\in [\unicode[STIX]{x1D6FD}-2+x_{\unicode[STIX]{x1D6FD}},\unicode[STIX]{x1D6FD}-x_{\unicode[STIX]{x1D6FD}}]\cup [\unicode[STIX]{x1D6FD}+x_{\unicode[STIX]{x1D6FD}},\unicode[STIX]{x1D6FD}+2-x_{\unicode[STIX]{x1D6FD}}]$
, we have
$x\in [\unicode[STIX]{x1D6FD}-2+x_{\unicode[STIX]{x1D6FD}},\unicode[STIX]{x1D6FD}-x_{\unicode[STIX]{x1D6FD}}]\cup [\unicode[STIX]{x1D6FD}+x_{\unicode[STIX]{x1D6FD}},\unicode[STIX]{x1D6FD}+2-x_{\unicode[STIX]{x1D6FD}}]$
, we have
 $\text{P}(-|x-\unicode[STIX]{x1D6FD}|)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}\leqslant 1/2$
. Since
$\text{P}(-|x-\unicode[STIX]{x1D6FD}|)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}\leqslant 1/2$
. Since
![]() $\bar{\unicode[STIX]{x1D707}}$
lies in this union of intervals, and
$\bar{\unicode[STIX]{x1D707}}$
lies in this union of intervals, and
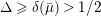 $\unicode[STIX]{x1D6E5}\geqslant \unicode[STIX]{x1D6FF}(\bar{\unicode[STIX]{x1D707}})>1/2$
, the inequality (3.3) holds.◻
$\unicode[STIX]{x1D6E5}\geqslant \unicode[STIX]{x1D6FF}(\bar{\unicode[STIX]{x1D707}})>1/2$
, the inequality (3.3) holds.◻
Proposition 3.6. Let
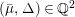 $(\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5})\in \mathbb{Q}^{2}$
satisfy
$(\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5})\in \mathbb{Q}^{2}$
satisfy
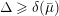 $\unicode[STIX]{x1D6E5}\geqslant \unicode[STIX]{x1D6FF}(\bar{\unicode[STIX]{x1D707}})$
. Let
$\unicode[STIX]{x1D6E5}\geqslant \unicode[STIX]{x1D6FF}(\bar{\unicode[STIX]{x1D707}})$
. Let
![]() $E$
be an exceptional bundle with
$E$
be an exceptional bundle with
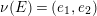 $\unicode[STIX]{x1D708}(E)=(e_{1},e_{2})$
. If
$\unicode[STIX]{x1D708}(E)=(e_{1},e_{2})$
. If
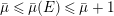 $\bar{\unicode[STIX]{x1D707}}\leqslant \bar{\unicode[STIX]{x1D707}}(E)\leqslant \bar{\unicode[STIX]{x1D707}}+1$
, then
$\bar{\unicode[STIX]{x1D707}}\leqslant \bar{\unicode[STIX]{x1D707}}(E)\leqslant \bar{\unicode[STIX]{x1D707}}+1$
, then
 $$\begin{eqnarray}(\bar{\unicode[STIX]{x1D707}}-e_{1}+1)(\bar{\unicode[STIX]{x1D707}}-e_{2}+1)-\unicode[STIX]{x1D6E5}(E)\leqslant \unicode[STIX]{x1D6E5}.\end{eqnarray}$$
$$\begin{eqnarray}(\bar{\unicode[STIX]{x1D707}}-e_{1}+1)(\bar{\unicode[STIX]{x1D707}}-e_{2}+1)-\unicode[STIX]{x1D6E5}(E)\leqslant \unicode[STIX]{x1D6E5}.\end{eqnarray}$$
If
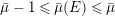 $\bar{\unicode[STIX]{x1D707}}-1\leqslant \bar{\unicode[STIX]{x1D707}}(E)\leqslant \bar{\unicode[STIX]{x1D707}}$
, then
$\bar{\unicode[STIX]{x1D707}}-1\leqslant \bar{\unicode[STIX]{x1D707}}(E)\leqslant \bar{\unicode[STIX]{x1D707}}$
, then
 $$\begin{eqnarray}(e_{1}-\bar{\unicode[STIX]{x1D707}}+1)(e_{2}-\bar{\unicode[STIX]{x1D707}}+1)-\unicode[STIX]{x1D6E5}(E)\leqslant \unicode[STIX]{x1D6E5}.\end{eqnarray}$$
$$\begin{eqnarray}(e_{1}-\bar{\unicode[STIX]{x1D707}}+1)(e_{2}-\bar{\unicode[STIX]{x1D707}}+1)-\unicode[STIX]{x1D6E5}(E)\leqslant \unicode[STIX]{x1D6E5}.\end{eqnarray}$$
Proof. Assuming
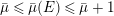 $\bar{\unicode[STIX]{x1D707}}\leqslant \bar{\unicode[STIX]{x1D707}}(E)\leqslant \bar{\unicode[STIX]{x1D707}}+1$
, we show the first inequality. The proof of the second inequality is similar. If
$\bar{\unicode[STIX]{x1D707}}\leqslant \bar{\unicode[STIX]{x1D707}}(E)\leqslant \bar{\unicode[STIX]{x1D707}}+1$
, we show the first inequality. The proof of the second inequality is similar. If
![]() $E$
is an odd symmetric exceptional bundle, the inequality follows from Lemma 3.5. If
$E$
is an odd symmetric exceptional bundle, the inequality follows from Lemma 3.5. If
![]() $E$
is a direct summand of an even symmetric exceptional bundle, then
$E$
is a direct summand of an even symmetric exceptional bundle, then
 $$\begin{eqnarray}(\bar{\unicode[STIX]{x1D707}}-e_{1}+1)(\bar{\unicode[STIX]{x1D707}}-e_{2}+1)-\unicode[STIX]{x1D6E5}(E)=\text{P}(\bar{\unicode[STIX]{x1D707}}-\bar{\unicode[STIX]{x1D707}}(E))-\unicode[STIX]{x1D6E5}(E\oplus \unicode[STIX]{x1D704}^{\ast }E)\leqslant \unicode[STIX]{x1D6E5}\end{eqnarray}$$
$$\begin{eqnarray}(\bar{\unicode[STIX]{x1D707}}-e_{1}+1)(\bar{\unicode[STIX]{x1D707}}-e_{2}+1)-\unicode[STIX]{x1D6E5}(E)=\text{P}(\bar{\unicode[STIX]{x1D707}}-\bar{\unicode[STIX]{x1D707}}(E))-\unicode[STIX]{x1D6E5}(E\oplus \unicode[STIX]{x1D704}^{\ast }E)\leqslant \unicode[STIX]{x1D6E5}\end{eqnarray}$$
again by Lemma 3.5.
Suppose that
![]() $E$
is neither an odd symmetric exceptional bundle nor a direct summand of an even symmetric exceptional bundle. We show that
$E$
is neither an odd symmetric exceptional bundle nor a direct summand of an even symmetric exceptional bundle. We show that
 $$\begin{eqnarray}(\bar{\unicode[STIX]{x1D707}}-e_{1}+1)(\bar{\unicode[STIX]{x1D707}}-e_{2}+1)-\unicode[STIX]{x1D6E5}(E)\leqslant \frac{1}{2}.\end{eqnarray}$$
$$\begin{eqnarray}(\bar{\unicode[STIX]{x1D707}}-e_{1}+1)(\bar{\unicode[STIX]{x1D707}}-e_{2}+1)-\unicode[STIX]{x1D6E5}(E)\leqslant \frac{1}{2}.\end{eqnarray}$$
The left-hand side of (3.4) attains the maximum for
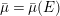 $\bar{\unicode[STIX]{x1D707}}=\bar{\unicode[STIX]{x1D707}}(E)$
. Thus
$\bar{\unicode[STIX]{x1D707}}=\bar{\unicode[STIX]{x1D707}}(E)$
. Thus
 $$\begin{eqnarray}\displaystyle \text{LHS of (3.4)} & {\leqslant} & \displaystyle (\bar{\unicode[STIX]{x1D707}}(E)-e_{1}+1)(\bar{\unicode[STIX]{x1D707}}(E)-e_{2}+1)-\unicode[STIX]{x1D6E5}(E)\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{2}+\frac{2-(a_{1}-a_{2})^{2}}{4\text{r}(E)^{2}},\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \text{LHS of (3.4)} & {\leqslant} & \displaystyle (\bar{\unicode[STIX]{x1D707}}(E)-e_{1}+1)(\bar{\unicode[STIX]{x1D707}}(E)-e_{2}+1)-\unicode[STIX]{x1D6E5}(E)\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{2}+\frac{2-(a_{1}-a_{2})^{2}}{4\text{r}(E)^{2}},\nonumber\end{eqnarray}$$
where
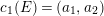 $c_{1}(E)=(a_{1},a_{2})$
. Since we are assuming that
$c_{1}(E)=(a_{1},a_{2})$
. Since we are assuming that
![]() $E$
is neither an odd symmetric exceptional bundle nor a direct summand of an even symmetric exceptional bundle, we have
$E$
is neither an odd symmetric exceptional bundle nor a direct summand of an even symmetric exceptional bundle, we have
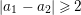 $|a_{1}-a_{2}|\geqslant 2$
, so (3.4) holds.◻
$|a_{1}-a_{2}|\geqslant 2$
, so (3.4) holds.◻
Now we come to the main theorem of this section.
Theorem 3.7.
-
(1) If
 $F$
is a semistable sheaf with symmetric
$F$
is a semistable sheaf with symmetric
 $c_{1}$
, then either
$c_{1}$
, then either
 $\unicode[STIX]{x1D6E5}\geqslant \unicode[STIX]{x1D6FF}(\bar{\unicode[STIX]{x1D707}}(F))$
, or
$\unicode[STIX]{x1D6E5}\geqslant \unicode[STIX]{x1D6FF}(\bar{\unicode[STIX]{x1D707}}(F))$
, or
 $\bar{\unicode[STIX]{x1D707}}(F)\in \mathfrak{E}$
and
$\bar{\unicode[STIX]{x1D707}}(F)\in \mathfrak{E}$
and
 $F$
is a direct sum of
$F$
is a direct sum of
 $E_{\bar{\unicode[STIX]{x1D707}}(F)}$
.
$E_{\bar{\unicode[STIX]{x1D707}}(F)}$
. -
(2) Assume that
 $(r,\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5})\in \mathbb{Z}_{{>}0}\times \mathbb{Q}^{2}$
satisfies the following conditions:
$(r,\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5})\in \mathbb{Z}_{{>}0}\times \mathbb{Q}^{2}$
satisfies the following conditions:-
(a)
 $r(\text{P}(\bar{\unicode[STIX]{x1D707}})-\unicode[STIX]{x1D6E5})\in \mathbb{Z}$
and
$r(\text{P}(\bar{\unicode[STIX]{x1D707}})-\unicode[STIX]{x1D6E5})\in \mathbb{Z}$
and
 $r\bar{\unicode[STIX]{x1D707}}\in \mathbb{Z}$
,
$r\bar{\unicode[STIX]{x1D707}}\in \mathbb{Z}$
, -
(b)
 $\unicode[STIX]{x1D6E5}\geqslant \unicode[STIX]{x1D6FF}(\bar{\unicode[STIX]{x1D707}})$
.
$\unicode[STIX]{x1D6E5}\geqslant \unicode[STIX]{x1D6FF}(\bar{\unicode[STIX]{x1D707}})$
.
Then there exists a
 $\unicode[STIX]{x1D707}$
-stable sheaf
$\unicode[STIX]{x1D707}$
-stable sheaf
 $F$
with symmetric
$F$
with symmetric
 $c_{1}$
such that
$c_{1}$
such that
 $\text{r}(F)=r$
,
$\text{r}(F)=r$
,
 $\bar{\unicode[STIX]{x1D707}}(F)=\bar{\unicode[STIX]{x1D707}}$
and
$\bar{\unicode[STIX]{x1D707}}(F)=\bar{\unicode[STIX]{x1D707}}$
and
 $\unicode[STIX]{x1D6E5}(F)=\unicode[STIX]{x1D6E5}$
.
$\unicode[STIX]{x1D6E5}(F)=\unicode[STIX]{x1D6E5}$
. -
Proof. (1) This is a consequence of Proposition 3.2.
(2) Step 1. We show the existence of stable sheaves.
By Proposition 3.6, we see that the condition (D-L) in [Reference RudakovR94, Theorem] holds. Thus by the theorem in [Reference RudakovR94], we have a desired stable sheaf
![]() $F$
. For reader’s convenience, we reproduce a sketch of proof of Rudakov’s theorem (adapted for the symmetric
$F$
. For reader’s convenience, we reproduce a sketch of proof of Rudakov’s theorem (adapted for the symmetric
![]() $c_{1}$
case).
$c_{1}$
case).
Let
![]() $t$
be the smallest integer such that
$t$
be the smallest integer such that
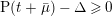 $\text{P}(t+\bar{\unicode[STIX]{x1D707}})-\unicode[STIX]{x1D6E5}\geqslant 0$
and
$\text{P}(t+\bar{\unicode[STIX]{x1D707}})-\unicode[STIX]{x1D6E5}\geqslant 0$
and
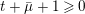 $t+\bar{\unicode[STIX]{x1D707}}+1\geqslant 0$
. Put
$t+\bar{\unicode[STIX]{x1D707}}+1\geqslant 0$
. Put
 $A:=r(\text{P}(t+\bar{\unicode[STIX]{x1D707}})-\unicode[STIX]{x1D6E5})$
,
$A:=r(\text{P}(t+\bar{\unicode[STIX]{x1D707}})-\unicode[STIX]{x1D6E5})$
,
 $B:=r((t+\bar{\unicode[STIX]{x1D707}}+1)(t+\bar{\unicode[STIX]{x1D707}})-\unicode[STIX]{x1D6E5})$
and
$B:=r((t+\bar{\unicode[STIX]{x1D707}}+1)(t+\bar{\unicode[STIX]{x1D707}})-\unicode[STIX]{x1D6E5})$
and
 $C:=r(\text{P}(t+\bar{\unicode[STIX]{x1D707}}-1)-\unicode[STIX]{x1D6E5})$
. We have
$C:=r(\text{P}(t+\bar{\unicode[STIX]{x1D707}}-1)-\unicode[STIX]{x1D6E5})$
. We have
![]() $A\geqslant 0$
and
$A\geqslant 0$
and
![]() $C<0$
. If
$C<0$
. If
![]() $B\geqslant 0$
, then we set
$B\geqslant 0$
, then we set
 $$\begin{eqnarray}\displaystyle F^{-1} & := & \displaystyle {\mathcal{O}}(-t-1,-t-1)^{-C}\oplus \left({\mathcal{O}}(-t-1,-t)\oplus {\mathcal{O}}(-t,-t-1)\right)^{B},\nonumber\\ \displaystyle F^{0} & := & \displaystyle {\mathcal{O}}(-t,-t)^{A}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle F^{-1} & := & \displaystyle {\mathcal{O}}(-t-1,-t-1)^{-C}\oplus \left({\mathcal{O}}(-t-1,-t)\oplus {\mathcal{O}}(-t,-t-1)\right)^{B},\nonumber\\ \displaystyle F^{0} & := & \displaystyle {\mathcal{O}}(-t,-t)^{A}.\nonumber\end{eqnarray}$$
If
![]() $B<0$
, then set
$B<0$
, then set
 $$\begin{eqnarray}\displaystyle F^{-1} & := & \displaystyle {\mathcal{O}}(-t-1,-t-1)^{-C},\nonumber\\ \displaystyle F^{0} & := & \displaystyle {\mathcal{O}}(-t,-t)^{A}\oplus \left({\mathcal{O}}(-t-1,-t)\oplus {\mathcal{O}}(-t,-t-1)\right)^{-B}\!.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle F^{-1} & := & \displaystyle {\mathcal{O}}(-t-1,-t-1)^{-C},\nonumber\\ \displaystyle F^{0} & := & \displaystyle {\mathcal{O}}(-t,-t)^{A}\oplus \left({\mathcal{O}}(-t-1,-t)\oplus {\mathcal{O}}(-t,-t-1)\right)^{-B}\!.\nonumber\end{eqnarray}$$
Put
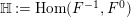 $\mathbb{H}:=\text{Hom}(F^{-1},F^{0})$
. Fix a smooth rational curve in the linear system
$\mathbb{H}:=\text{Hom}(F^{-1},F^{0})$
. Fix a smooth rational curve in the linear system
 $|{\mathcal{O}}(1,1)|$
. Then we can find a nonempty Zariski open subset
$|{\mathcal{O}}(1,1)|$
. Then we can find a nonempty Zariski open subset
![]() $U\subset \mathbb{H}$
such that for any point
$U\subset \mathbb{H}$
such that for any point
![]() $[f]\in U$
, the following hold:
$[f]\in U$
, the following hold:
-
(a)
 $f$
is injective and
$f$
is injective and
 $G:=\text{Coker}\,f$
is a torsion-free sheaf with symmetric
$G:=\text{Coker}\,f$
is a torsion-free sheaf with symmetric
 $c_{1}$
such that
$c_{1}$
such that
 $\text{r}(G)=r$
,
$\text{r}(G)=r$
,
 $\bar{\unicode[STIX]{x1D707}}(G)=\bar{\unicode[STIX]{x1D707}}$
and
$\bar{\unicode[STIX]{x1D707}}(G)=\bar{\unicode[STIX]{x1D707}}$
and
 $\unicode[STIX]{x1D6E5}(G)=\unicode[STIX]{x1D6E5}$
, and that
$\unicode[STIX]{x1D6E5}(G)=\unicode[STIX]{x1D6E5}$
, and that
 $G$
is locally free along
$G$
is locally free along
 $D$
and
$D$
and
 $G|_{D}$
is a rigid bundle,
$G|_{D}$
is a rigid bundle, -
(b) the Kodaira–Spencer map
 $T_{[f]}U\rightarrow \text{Ext}^{1}(G,G)$
is surjective,
$T_{[f]}U\rightarrow \text{Ext}^{1}(G,G)$
is surjective, -
(c)
 $\text{Ext}^{2}(G,G)=0$
.
$\text{Ext}^{2}(G,G)=0$
.
Suppose that for
![]() $[f]\in U$
,
$[f]\in U$
,
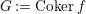 $G:=\text{Coker}\,f$
is not semistable. Let
$G:=\text{Coker}\,f$
is not semistable. Let
 $$\begin{eqnarray}0=G_{0}\subset G_{1}\subset \cdots \subset G_{l}=G\end{eqnarray}$$
$$\begin{eqnarray}0=G_{0}\subset G_{1}\subset \cdots \subset G_{l}=G\end{eqnarray}$$
be the Harder–Narasimhan filtration of
![]() $G$
. By condition (a), we have
$G$
. By condition (a), we have
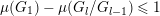 $\unicode[STIX]{x1D707}(G_{1})-\unicode[STIX]{x1D707}(G_{l}/G_{l-1})\leqslant 1$
. If
$\unicode[STIX]{x1D707}(G_{1})-\unicode[STIX]{x1D707}(G_{l}/G_{l-1})\leqslant 1$
. If
![]() $G_{1}$
is not stable, then we can find (cf. [Reference Kuleshov and OrlovKO, Proposition 4.4]) a subsheaf
$G_{1}$
is not stable, then we can find (cf. [Reference Kuleshov and OrlovKO, Proposition 4.4]) a subsheaf
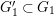 $G_{1}^{\prime }\subset G_{1}$
such that
$G_{1}^{\prime }\subset G_{1}$
such that
-
∙
 $G_{1}$
and
$G_{1}$
and
 $G_{1}^{\prime }$
have the same reduced Hilbert polynomial,
$G_{1}^{\prime }$
have the same reduced Hilbert polynomial, -
∙
 $G_{1}$
is
$G_{1}$
is
 $S$
-equivalent to
$S$
-equivalent to
 $H^{a}$
for some stable sheaf
$H^{a}$
for some stable sheaf
 $H$
,
$H$
, -
∙
 $\text{Hom}(G_{1}^{\prime },G_{1}/G_{1}^{\prime })=0$
.
$\text{Hom}(G_{1}^{\prime },G_{1}/G_{1}^{\prime })=0$
.
If
![]() $G_{1}$
is stable, let
$G_{1}$
is stable, let
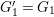 $G_{1}^{\prime }=G_{1}$
. Similarly, if
$G_{1}^{\prime }=G_{1}$
. Similarly, if
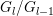 $G_{l}/G_{l-1}$
is not stable, then we can find a subsheaf
$G_{l}/G_{l-1}$
is not stable, then we can find a subsheaf
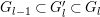 $G_{l-1}\subset G_{l}^{\prime }\subset G_{l}$
such that
$G_{l-1}\subset G_{l}^{\prime }\subset G_{l}$
such that
-
∙
 $G_{l}/G_{l-1}$
and
$G_{l}/G_{l-1}$
and
 $G_{l}/G_{l}^{\prime }$
have the same reduced Hilbert polynomial,
$G_{l}/G_{l}^{\prime }$
have the same reduced Hilbert polynomial, -
∙
 $G_{l}/G_{l}^{\prime }$
is
$G_{l}/G_{l}^{\prime }$
is
 $S$
-equivalent to
$S$
-equivalent to
 ${H^{\prime }}^{b}$
for some stable sheaf
${H^{\prime }}^{b}$
for some stable sheaf
 $H^{\prime }$
,
$H^{\prime }$
, -
∙
 $\text{Hom}(G_{l}^{\prime }/G_{l-1},G_{l}/G_{l}^{\prime })=0$
.
$\text{Hom}(G_{l}^{\prime }/G_{l-1},G_{l}/G_{l}^{\prime })=0$
.
If
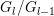 $G_{l}/G_{l-1}$
is stable, let
$G_{l}/G_{l-1}$
is stable, let
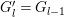 $G_{l}^{\prime }=G_{l-1}$
. We write
$G_{l}^{\prime }=G_{l-1}$
. We write
 $$\begin{eqnarray}0=\bar{G}_{0}\subset \cdots \subset \bar{G}_{m}=G\end{eqnarray}$$
$$\begin{eqnarray}0=\bar{G}_{0}\subset \cdots \subset \bar{G}_{m}=G\end{eqnarray}$$
for the filtration
 $$\begin{eqnarray}0=G_{0}\subset G_{0}^{\prime }\subset G_{1}\subset \ldots G_{l-1}\subset G_{l}^{\prime }\subset G_{l}=G.\end{eqnarray}$$
$$\begin{eqnarray}0=G_{0}\subset G_{0}^{\prime }\subset G_{1}\subset \ldots G_{l-1}\subset G_{l}^{\prime }\subset G_{l}=G.\end{eqnarray}$$
Then we have
 $\text{Ext}^{2}(\bar{G}_{i}/\bar{G}_{i-1},\bar{G}_{j}/\bar{G}_{j-1})=0$
for
$\text{Ext}^{2}(\bar{G}_{i}/\bar{G}_{i-1},\bar{G}_{j}/\bar{G}_{j-1})=0$
for
![]() $i\leqslant j$
, and
$i\leqslant j$
, and
 $\text{Hom}(\bar{G}_{i}/\bar{G}_{i-1},\bar{G}_{j}/\bar{G}_{j-1})=0$
for
$\text{Hom}(\bar{G}_{i}/\bar{G}_{i-1},\bar{G}_{j}/\bar{G}_{j-1})=0$
for
![]() $i<j$
. Moreover,
$i<j$
. Moreover,
![]() $\bar{G}_{1}$
and
$\bar{G}_{1}$
and
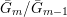 $\bar{G}_{m}/\bar{G}_{m-1}$
are S-equivalent to
$\bar{G}_{m}/\bar{G}_{m-1}$
are S-equivalent to
![]() $H^{a}$
and
$H^{a}$
and
![]() ${H^{\prime }}^{b}$
for some stable sheaves
${H^{\prime }}^{b}$
for some stable sheaves
![]() $H$
and
$H$
and
![]() $H^{\prime }$
.
$H^{\prime }$
.
Claim 3.7.1.
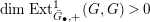 $\dim \text{Ext}_{\bar{G}_{\bullet },+}^{1}(G,G)>0$
.
$\dim \text{Ext}_{\bar{G}_{\bullet },+}^{1}(G,G)>0$
.
Proof of Claim.
If
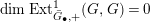 $\dim \text{Ext}_{\bar{G}_{\bullet },+}^{1}(G,G)=0$
, then
$\dim \text{Ext}_{\bar{G}_{\bullet },+}^{1}(G,G)=0$
, then
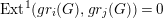 $\text{Ext}^{1}(gr_{i}(G),gr_{j}(G))=0$
for
$\text{Ext}^{1}(gr_{i}(G),gr_{j}(G))=0$
for
![]() $i<j$
, hence we have
$i<j$
, hence we have
 $$\begin{eqnarray}\unicode[STIX]{x1D712}(gr_{i}(G),gr_{j}(G))=0\text{ for }i<j.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D712}(gr_{i}(G),gr_{j}(G))=0\text{ for }i<j.\end{eqnarray}$$
If we let
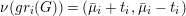 $\unicode[STIX]{x1D708}(gr_{i}(G))=(\bar{\unicode[STIX]{x1D707}}_{i}+t_{i},\bar{\unicode[STIX]{x1D707}}_{i}-t_{i})$
, then we have
$\unicode[STIX]{x1D708}(gr_{i}(G))=(\bar{\unicode[STIX]{x1D707}}_{i}+t_{i},\bar{\unicode[STIX]{x1D707}}_{i}-t_{i})$
, then we have
 $$\begin{eqnarray}0=(\bar{\unicode[STIX]{x1D707}}_{m}-\bar{\unicode[STIX]{x1D707}}_{1}+1)^{2}-(t_{1}-t_{m})^{2}-\unicode[STIX]{x1D6E5}(gr_{1}(G))-\unicode[STIX]{x1D6E5}(gr_{m}(G)).\end{eqnarray}$$
$$\begin{eqnarray}0=(\bar{\unicode[STIX]{x1D707}}_{m}-\bar{\unicode[STIX]{x1D707}}_{1}+1)^{2}-(t_{1}-t_{m})^{2}-\unicode[STIX]{x1D6E5}(gr_{1}(G))-\unicode[STIX]{x1D6E5}(gr_{m}(G)).\end{eqnarray}$$
We have
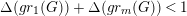 $\unicode[STIX]{x1D6E5}(gr_{1}(G))+\unicode[STIX]{x1D6E5}(gr_{m}(G))<1$
unless
$\unicode[STIX]{x1D6E5}(gr_{1}(G))+\unicode[STIX]{x1D6E5}(gr_{m}(G))<1$
unless
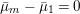 $\bar{\unicode[STIX]{x1D707}}_{m}-\bar{\unicode[STIX]{x1D707}}_{1}=0$
and
$\bar{\unicode[STIX]{x1D707}}_{m}-\bar{\unicode[STIX]{x1D707}}_{1}=0$
and
![]() $t_{1}=t_{m}$
. If
$t_{1}=t_{m}$
. If
 $\bar{\unicode[STIX]{x1D707}}_{m}-\bar{\unicode[STIX]{x1D707}}_{1}=0$
and
$\bar{\unicode[STIX]{x1D707}}_{m}-\bar{\unicode[STIX]{x1D707}}_{1}=0$
and
![]() $t_{1}=t_{m}$
, then
$t_{1}=t_{m}$
, then
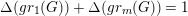 $\unicode[STIX]{x1D6E5}(gr_{1}(G))+\unicode[STIX]{x1D6E5}(gr_{m}(G))=1$
. If
$\unicode[STIX]{x1D6E5}(gr_{1}(G))+\unicode[STIX]{x1D6E5}(gr_{m}(G))=1$
. If
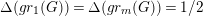 $\unicode[STIX]{x1D6E5}(gr_{1}(G))=\unicode[STIX]{x1D6E5}(gr_{m}(G))=1/2$
, then
$\unicode[STIX]{x1D6E5}(gr_{1}(G))=\unicode[STIX]{x1D6E5}(gr_{m}(G))=1/2$
, then
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D712}(gr_{m}(G))/\text{r}(gr_{m}(G)) & = & \displaystyle (\bar{\unicode[STIX]{x1D707}}_{m}+1)^{2}-t_{m}^{2}-\unicode[STIX]{x1D6E5}(gr_{m}(G))\nonumber\\ \displaystyle & = & \displaystyle (\bar{\unicode[STIX]{x1D707}}_{1}+1)^{2}-t_{1}^{2}-\unicode[STIX]{x1D6E5}(gr_{1}(G))\nonumber\\ \displaystyle & = & \displaystyle \unicode[STIX]{x1D712}(gr_{1}(G))/\text{r}(gr_{1}(G)).\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D712}(gr_{m}(G))/\text{r}(gr_{m}(G)) & = & \displaystyle (\bar{\unicode[STIX]{x1D707}}_{m}+1)^{2}-t_{m}^{2}-\unicode[STIX]{x1D6E5}(gr_{m}(G))\nonumber\\ \displaystyle & = & \displaystyle (\bar{\unicode[STIX]{x1D707}}_{1}+1)^{2}-t_{1}^{2}-\unicode[STIX]{x1D6E5}(gr_{1}(G))\nonumber\\ \displaystyle & = & \displaystyle \unicode[STIX]{x1D712}(gr_{1}(G))/\text{r}(gr_{1}(G)).\nonumber\end{eqnarray}$$
This is a contradiction. Therefore in any case, we have
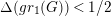 $\unicode[STIX]{x1D6E5}(gr_{1}(G))<1/2$
or
$\unicode[STIX]{x1D6E5}(gr_{1}(G))<1/2$
or
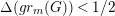 $\unicode[STIX]{x1D6E5}(gr_{m}(G))<1/2$
. Suppose
$\unicode[STIX]{x1D6E5}(gr_{m}(G))<1/2$
. Suppose
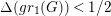 $\unicode[STIX]{x1D6E5}(gr_{1}(G))<1/2$
. Recall that
$\unicode[STIX]{x1D6E5}(gr_{1}(G))<1/2$
. Recall that
![]() $gr_{1}(G)$
is S-equivalent to
$gr_{1}(G)$
is S-equivalent to
![]() $H^{a}$
for some stable sheaf
$H^{a}$
for some stable sheaf
![]() $H$
. By
$H$
. By
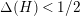 $\unicode[STIX]{x1D6E5}(H)<1/2$
,
$\unicode[STIX]{x1D6E5}(H)<1/2$
,
![]() $H$
is an exceptional bundle. Moreover,
$H$
is an exceptional bundle. Moreover,
 $$\begin{eqnarray}\unicode[STIX]{x1D712}(H,G)=\frac{1}{a}\unicode[STIX]{x1D712}(gr_{1}(G),G)=\frac{1}{a}\unicode[STIX]{x1D712}(gr_{1}(G),gr_{1}(G))=a\unicode[STIX]{x1D712}(H,H)>0,\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D712}(H,G)=\frac{1}{a}\unicode[STIX]{x1D712}(gr_{1}(G),G)=\frac{1}{a}\unicode[STIX]{x1D712}(gr_{1}(G),gr_{1}(G))=a\unicode[STIX]{x1D712}(H,H)>0,\end{eqnarray}$$
where the second equality follows from (3.6). This is a contradiction. In the case
 $\unicode[STIX]{x1D6E5}(gr_{m}(G))<1/2$
, we lead to a contradiction by a similar argument. This is the end of proof of the claim.◻
$\unicode[STIX]{x1D6E5}(gr_{m}(G))<1/2$
, we lead to a contradiction by a similar argument. This is the end of proof of the claim.◻
Using the claim, we can see (cf. the proof of [Reference Drezet and Le PotierDL, Théorème 4.7]) that there exists a nonempty Zariski open subset
![]() $U^{\prime }\subset U$
such that for any
$U^{\prime }\subset U$
such that for any
 $[f]\in U^{\prime }$
,
$[f]\in U^{\prime }$
,
 $\text{Coker}\,f$
is semistable.
$\text{Coker}\,f$
is semistable.
Suppose that for
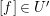 $[f]\in U^{\prime }$
,
$[f]\in U^{\prime }$
,
 $G:=\text{Coker}\,f$
is not stable. Then we can find a filtration
$G:=\text{Coker}\,f$
is not stable. Then we can find a filtration
 $$\begin{eqnarray}0=G_{0}\subset G_{1}\subset G_{2}=G\end{eqnarray}$$
$$\begin{eqnarray}0=G_{0}\subset G_{1}\subset G_{2}=G\end{eqnarray}$$
such that
![]() $gr_{1}(G)$
and
$gr_{1}(G)$
and
![]() $gr_{2}(G)$
have the same reduced Hilbert polynomial. If we let
$gr_{2}(G)$
have the same reduced Hilbert polynomial. If we let
 $\unicode[STIX]{x1D708}(gr_{i}(G))=(\bar{\unicode[STIX]{x1D707}}_{i}+t_{i},\bar{\unicode[STIX]{x1D707}}_{i}-t_{i})$
, then
$\unicode[STIX]{x1D708}(gr_{i}(G))=(\bar{\unicode[STIX]{x1D707}}_{i}+t_{i},\bar{\unicode[STIX]{x1D707}}_{i}-t_{i})$
, then
 $$\begin{eqnarray}\displaystyle \dim \text{Ext}_{G_{\bullet },+}^{1}(G,G) & = & \displaystyle \dim \text{Ext}^{1}(gr_{1}(G),gr_{2}(G))\nonumber\\ \displaystyle & {\geqslant} & \displaystyle -\unicode[STIX]{x1D712}(gr_{1}(G),gr_{2}(G))\nonumber\\ \displaystyle & {\geqslant} & \displaystyle r_{1}r_{2}\{-1+(t_{2}-t_{1})^{2}+\unicode[STIX]{x1D6E5}(gr_{1}(G))+\unicode[STIX]{x1D6E5}(gr_{2}(G))\}\nonumber\\ \displaystyle & = & \displaystyle r_{1}r_{2}\{-1-2t_{1}t_{2}+2\unicode[STIX]{x1D6E5}\}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \dim \text{Ext}_{G_{\bullet },+}^{1}(G,G) & = & \displaystyle \dim \text{Ext}^{1}(gr_{1}(G),gr_{2}(G))\nonumber\\ \displaystyle & {\geqslant} & \displaystyle -\unicode[STIX]{x1D712}(gr_{1}(G),gr_{2}(G))\nonumber\\ \displaystyle & {\geqslant} & \displaystyle r_{1}r_{2}\{-1+(t_{2}-t_{1})^{2}+\unicode[STIX]{x1D6E5}(gr_{1}(G))+\unicode[STIX]{x1D6E5}(gr_{2}(G))\}\nonumber\\ \displaystyle & = & \displaystyle r_{1}r_{2}\{-1-2t_{1}t_{2}+2\unicode[STIX]{x1D6E5}\}.\nonumber\end{eqnarray}$$
Here
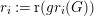 $r_{i}:=\text{r}(gr_{i}(G))$
, and we used
$r_{i}:=\text{r}(gr_{i}(G))$
, and we used
 $\unicode[STIX]{x1D6E5}(gr_{i}(G))+t_{i}^{2}=\unicode[STIX]{x1D6E5}$
. By assumption, we have
$\unicode[STIX]{x1D6E5}(gr_{i}(G))+t_{i}^{2}=\unicode[STIX]{x1D6E5}$
. By assumption, we have
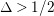 $\unicode[STIX]{x1D6E5}>1/2$
. Since
$\unicode[STIX]{x1D6E5}>1/2$
. Since
![]() $G$
has symmetric
$G$
has symmetric
![]() $c_{1}$
, we have
$c_{1}$
, we have
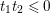 $t_{1}t_{2}\leqslant 0$
. Hence we have
$t_{1}t_{2}\leqslant 0$
. Hence we have
 $\dim \text{Ext}_{G_{\bullet },+}^{1}(G,G)>0$
. This implies (cf. the proof of [Reference Drezet and Le PotierDL, Théorème 4.10]) that there exists a nonempty Zariski open subset
$\dim \text{Ext}_{G_{\bullet },+}^{1}(G,G)>0$
. This implies (cf. the proof of [Reference Drezet and Le PotierDL, Théorème 4.10]) that there exists a nonempty Zariski open subset
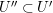 $U^{\prime \prime }\subset U^{\prime }$
such that for any
$U^{\prime \prime }\subset U^{\prime }$
such that for any
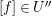 $[f]\in U^{\prime \prime }$
,
$[f]\in U^{\prime \prime }$
,
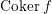 $\text{Coker}\,f$
is stable.
$\text{Coker}\,f$
is stable.
Step 2. We show the existence of
![]() $\unicode[STIX]{x1D707}$
-stable sheaves.
$\unicode[STIX]{x1D707}$
-stable sheaves.
If
![]() $r=1$
, we are done, so assume that
$r=1$
, we are done, so assume that
![]() $r\geqslant 2$
. Then there exists a nonempty Zariski open subset
$r\geqslant 2$
. Then there exists a nonempty Zariski open subset
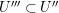 $U^{\prime \prime \prime }\subset U^{\prime \prime }$
such that for any
$U^{\prime \prime \prime }\subset U^{\prime \prime }$
such that for any
 $[f]\in U^{\prime \prime \prime }$
,
$[f]\in U^{\prime \prime \prime }$
,
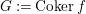 $G:=\text{Coker}\,f$
is locally free (cf. [Reference Le PotierLe, Section 17.1]). If
$G:=\text{Coker}\,f$
is locally free (cf. [Reference Le PotierLe, Section 17.1]). If
![]() $G$
is not
$G$
is not
![]() $\unicode[STIX]{x1D707}$
-stable, then the
$\unicode[STIX]{x1D707}$
-stable, then the
![]() $G^{\ast }$
is not semistable. But repeating the (first half of) argument in Step
$G^{\ast }$
is not semistable. But repeating the (first half of) argument in Step
![]() $1$
for the family
$1$
for the family
 $\{(\text{Coker}\,f)^{\ast }\}_{[f]\in U^{\prime \prime \prime }}$
of dual sheaves, we see that for general
$\{(\text{Coker}\,f)^{\ast }\}_{[f]\in U^{\prime \prime \prime }}$
of dual sheaves, we see that for general
 $[f]\in U^{\prime \prime \prime }$
,
$[f]\in U^{\prime \prime \prime }$
,
 $(\text{Coker}\,f)^{\ast }$
is semistable. So for general
$(\text{Coker}\,f)^{\ast }$
is semistable. So for general
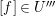 $[f]\in U^{\prime \prime \prime }$
,
$[f]\in U^{\prime \prime \prime }$
,
 $\text{Coker}\,f$
is
$\text{Coker}\,f$
is
![]() $\unicode[STIX]{x1D707}$
-stable.◻
$\unicode[STIX]{x1D707}$
-stable.◻
The theorem allows us to define an invariant height.
Definition 3.8. Let
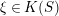 $\unicode[STIX]{x1D709}\in K(S)$
be a semistable class with symmetric
$\unicode[STIX]{x1D709}\in K(S)$
be a semistable class with symmetric
![]() $c_{1}$
. When
$c_{1}$
. When
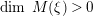 $\dim \,M(\unicode[STIX]{x1D709})>0$
, we define the height of the moduli space
$\dim \,M(\unicode[STIX]{x1D709})>0$
, we define the height of the moduli space
![]() $M(\unicode[STIX]{x1D709})$
to be the nonnegative integer
$M(\unicode[STIX]{x1D709})$
to be the nonnegative integer
 $$\begin{eqnarray}\text{r}(E_{\unicode[STIX]{x1D6FE}})\text{r}(\unicode[STIX]{x1D709})\left\{\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})-\unicode[STIX]{x1D6FF}(\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))\right\}\!,\end{eqnarray}$$
$$\begin{eqnarray}\text{r}(E_{\unicode[STIX]{x1D6FE}})\text{r}(\unicode[STIX]{x1D709})\left\{\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})-\unicode[STIX]{x1D6FF}(\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))\right\}\!,\end{eqnarray}$$
where
![]() $\unicode[STIX]{x1D6FE}$
is the unique symmetric exceptional slope such that
$\unicode[STIX]{x1D6FE}$
is the unique symmetric exceptional slope such that
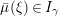 $\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})\in I_{\unicode[STIX]{x1D6FE}}$
.
$\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})\in I_{\unicode[STIX]{x1D6FE}}$
.
4 Bridgeland stability of symmetric exceptional bundles
In Section 4.1, we recall the Bridgeland semistability and walls. In Section 4.2, we consider Bridgeland semistability of symmetric exceptional bundles.
4.1 Abelian category
 ${\mathcal{A}}_{s}$
${\mathcal{A}}_{s}$
Following [Reference Arcara and BertramAB], we consider a particular kind of Bridgeland semistability. The presentation in this section follows that in [Reference Arcara, Bertram, Coskun and HuizengaABCH, Sections 5, 6].
If
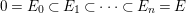 $0=E_{0}\subset E_{1}\subset \cdots \subset E_{n}=E$
is the Harder–Narasimhan filtration of a torsion-free sheaf
$0=E_{0}\subset E_{1}\subset \cdots \subset E_{n}=E$
is the Harder–Narasimhan filtration of a torsion-free sheaf
![]() $E$
for
$E$
for
![]() $\unicode[STIX]{x1D707}$
-stability, that is,
$\unicode[STIX]{x1D707}$
-stability, that is,
 $E_{i}/E_{i-1}$
,
$E_{i}/E_{i-1}$
,
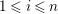 $1\leqslant i\leqslant n$
, are
$1\leqslant i\leqslant n$
, are
![]() $\unicode[STIX]{x1D707}$
-semistable and
$\unicode[STIX]{x1D707}$
-semistable and
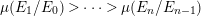 $\unicode[STIX]{x1D707}(E_{1}/E_{0})>\cdots >\unicode[STIX]{x1D707}(E_{n}/E_{n-1})$
, then we put
$\unicode[STIX]{x1D707}(E_{1}/E_{0})>\cdots >\unicode[STIX]{x1D707}(E_{n}/E_{n-1})$
, then we put
 $\bar{\unicode[STIX]{x1D707}}_{\text{min}}(E):=\bar{\unicode[STIX]{x1D707}}(E_{n}/E_{n-1})$
and
$\bar{\unicode[STIX]{x1D707}}_{\text{min}}(E):=\bar{\unicode[STIX]{x1D707}}(E_{n}/E_{n-1})$
and
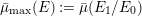 $\bar{\unicode[STIX]{x1D707}}_{\text{max}}(E):=\bar{\unicode[STIX]{x1D707}}(E_{1}/E_{0})$
.
$\bar{\unicode[STIX]{x1D707}}_{\text{max}}(E):=\bar{\unicode[STIX]{x1D707}}(E_{1}/E_{0})$
.
For
![]() $s\in \mathbb{R}$
, we let
$s\in \mathbb{R}$
, we let
![]() ${\mathcal{Q}}_{s}$
be the full subcategory of
${\mathcal{Q}}_{s}$
be the full subcategory of
![]() $\text{coh}(S)$
consisting of coherent sheaves
$\text{coh}(S)$
consisting of coherent sheaves
![]() $Q$
with
$Q$
with
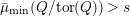 $\bar{\unicode[STIX]{x1D707}}_{\text{min}}(Q/\text{tor}(Q))>s$
, and we let
$\bar{\unicode[STIX]{x1D707}}_{\text{min}}(Q/\text{tor}(Q))>s$
, and we let
![]() ${\mathcal{F}}_{s}$
be the full subcategory of
${\mathcal{F}}_{s}$
be the full subcategory of
![]() $\text{coh}(S)$
consisting of torsion-free coherent sheaves
$\text{coh}(S)$
consisting of torsion-free coherent sheaves
![]() $F$
with
$F$
with
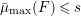 $\bar{\unicode[STIX]{x1D707}}_{\text{max}}(F)\leqslant s$
. We define a full subcategory
$\bar{\unicode[STIX]{x1D707}}_{\text{max}}(F)\leqslant s$
. We define a full subcategory
![]() ${\mathcal{A}}_{s}$
of
${\mathcal{A}}_{s}$
of
![]() $\text{D}(S)$
by
$\text{D}(S)$
by
 $$\begin{eqnarray}{\mathcal{A}}_{s}=\left\{E^{\bullet }\left|\text{H}^{0}(E^{\bullet })\in {\mathcal{Q}}_{s},\text{H}^{-1}(E^{\bullet })\in {\mathcal{F}}_{s}\text{ and }\text{H}^{i}(E^{\bullet })=0\text{ for }i\neq 0,-1\right.\!\right\}\!.\end{eqnarray}$$
$$\begin{eqnarray}{\mathcal{A}}_{s}=\left\{E^{\bullet }\left|\text{H}^{0}(E^{\bullet })\in {\mathcal{Q}}_{s},\text{H}^{-1}(E^{\bullet })\in {\mathcal{F}}_{s}\text{ and }\text{H}^{i}(E^{\bullet })=0\text{ for }i\neq 0,-1\right.\!\right\}\!.\end{eqnarray}$$
Then
![]() ${\mathcal{A}}_{s}$
is an abelian category.
${\mathcal{A}}_{s}$
is an abelian category.
For
![]() $s,t\in \mathbb{R}$
, we define the map
$s,t\in \mathbb{R}$
, we define the map
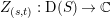 $Z_{(s,t)}:\text{D}(S)\rightarrow \mathbb{C}$
by
$Z_{(s,t)}:\text{D}(S)\rightarrow \mathbb{C}$
by
 $$\begin{eqnarray}Z_{(s,t)}(E):=-\int _{S}e^{(s+ti)c_{1}(L)}\text{ch}(E),\end{eqnarray}$$
$$\begin{eqnarray}Z_{(s,t)}(E):=-\int _{S}e^{(s+ti)c_{1}(L)}\text{ch}(E),\end{eqnarray}$$
where
 $L={\mathcal{O}}(1,1)$
. Explicitly we have
$L={\mathcal{O}}(1,1)$
. Explicitly we have
 $$\begin{eqnarray}\displaystyle Z_{(s,t)}(E) & = & \displaystyle \left(sc_{1}(E)c_{1}(L)-\text{r}(E)(s^{2}-t^{2})-\text{ch}_{2}(E)\right)\nonumber\\ \displaystyle & & \displaystyle +\,t\left(c_{1}(E)c_{1}(L)-2\text{r}(E)s\right)i.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle Z_{(s,t)}(E) & = & \displaystyle \left(sc_{1}(E)c_{1}(L)-\text{r}(E)(s^{2}-t^{2})-\text{ch}_{2}(E)\right)\nonumber\\ \displaystyle & & \displaystyle +\,t\left(c_{1}(E)c_{1}(L)-2\text{r}(E)s\right)i.\nonumber\end{eqnarray}$$
Now assume that
![]() $t>0$
, then the pair
$t>0$
, then the pair
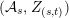 $({\mathcal{A}}_{s},Z_{(s,t)})$
is a Bridgeland stability condition (cf. [Reference Arcara and BertramAB]).
$({\mathcal{A}}_{s},Z_{(s,t)})$
is a Bridgeland stability condition (cf. [Reference Arcara and BertramAB]).
For
![]() $E\in {\mathcal{A}}_{s}$
, we put
$E\in {\mathcal{A}}_{s}$
, we put
 $$\begin{eqnarray}\unicode[STIX]{x1D707}_{s,t}(E)=-\frac{\text{Re}\,Z_{(s,t)}(E)}{\text{Im}\,Z_{(s,t)}(E)},\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D707}_{s,t}(E)=-\frac{\text{Re}\,Z_{(s,t)}(E)}{\text{Im}\,Z_{(s,t)}(E)},\end{eqnarray}$$
where it is understood as
![]() $+\infty$
if the denominator is zero. Explicitly we have
$+\infty$
if the denominator is zero. Explicitly we have
 $$\begin{eqnarray}\unicode[STIX]{x1D707}_{s,t}(E)=\frac{\text{r}(E)(s^{2}-t^{2})-sc_{1}(E)c_{1}(L)+\text{ch}_{2}(E)}{t(c_{1}(E)c_{1}(L)-2\text{r}(E)s)}.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D707}_{s,t}(E)=\frac{\text{r}(E)(s^{2}-t^{2})-sc_{1}(E)c_{1}(L)+\text{ch}_{2}(E)}{t(c_{1}(E)c_{1}(L)-2\text{r}(E)s)}.\end{eqnarray}$$
An object
![]() $E\in {\mathcal{A}}_{s}$
is said to be
$E\in {\mathcal{A}}_{s}$
is said to be
![]() $(s,t)$
-semistable if for any nonzero subobject
$(s,t)$
-semistable if for any nonzero subobject
![]() $F\subset E$
in
$F\subset E$
in
![]() ${\mathcal{A}}_{s}$
, the inequality
${\mathcal{A}}_{s}$
, the inequality
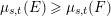 $\unicode[STIX]{x1D707}_{s,t}(E)\geqslant \unicode[STIX]{x1D707}_{s,t}(F)$
holds.
$\unicode[STIX]{x1D707}_{s,t}(E)\geqslant \unicode[STIX]{x1D707}_{s,t}(F)$
holds.
For
 $(r,c,d)\in \mathbb{R}^{3}$
, we define
$(r,c,d)\in \mathbb{R}^{3}$
, we define
 $$\begin{eqnarray}\unicode[STIX]{x1D707}_{s,t}(r,c,d)=\frac{r(s^{2}-t^{2})-sc+d}{t(c-2rs)}.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D707}_{s,t}(r,c,d)=\frac{r(s^{2}-t^{2})-sc+d}{t(c-2rs)}.\end{eqnarray}$$
For
![]() $(r,c,d)$
and
$(r,c,d)$
and
 $(r^{\prime },c^{\prime },d^{\prime })$
, we define the wall
$(r^{\prime },c^{\prime },d^{\prime })$
, we define the wall
 $W_{(r,c,d),(r^{\prime },c^{\prime },d^{\prime })}$
by
$W_{(r,c,d),(r^{\prime },c^{\prime },d^{\prime })}$
by
 $$\begin{eqnarray}W_{(r,c,d),(r^{\prime },c^{\prime },d^{\prime })}=\left\{(s,t)\in \mathbb{R}\times \mathbb{R}_{{>}0}\left|\unicode[STIX]{x1D707}_{s,t}(r,c,d)=\unicode[STIX]{x1D707}_{s^{\prime },t^{\prime }}(r^{\prime },c^{\prime },d^{\prime })\right.\!\right\}\!.\end{eqnarray}$$
$$\begin{eqnarray}W_{(r,c,d),(r^{\prime },c^{\prime },d^{\prime })}=\left\{(s,t)\in \mathbb{R}\times \mathbb{R}_{{>}0}\left|\unicode[STIX]{x1D707}_{s,t}(r,c,d)=\unicode[STIX]{x1D707}_{s^{\prime },t^{\prime }}(r^{\prime },c^{\prime },d^{\prime })\right.\!\right\}\!.\end{eqnarray}$$
The equality in the condition of the definition of the wall is equivalent to
 $$\begin{eqnarray}(r^{\prime }c-rc^{\prime })s^{2}-2(r^{\prime }d-rd^{\prime })s+(r^{\prime }c-rc^{\prime })t^{2}+c^{\prime }d-cd^{\prime }=0.\end{eqnarray}$$
$$\begin{eqnarray}(r^{\prime }c-rc^{\prime })s^{2}-2(r^{\prime }d-rd^{\prime })s+(r^{\prime }c-rc^{\prime })t^{2}+c^{\prime }d-cd^{\prime }=0.\end{eqnarray}$$
If
![]() $(r,c,d)$
and
$(r,c,d)$
and
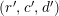 $(r^{\prime },c^{\prime },d^{\prime })$
are not proportional, then we have the following cases.
$(r^{\prime },c^{\prime },d^{\prime })$
are not proportional, then we have the following cases.
Case (1).
 $(r,r^{\prime })=(0,0)$
. In this case, the wall
$(r,r^{\prime })=(0,0)$
. In this case, the wall
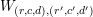 $W_{(r,c,d),(r^{\prime },c^{\prime },d^{\prime })}$
is empty.
$W_{(r,c,d),(r^{\prime },c^{\prime },d^{\prime })}$
is empty.
Case (2).
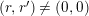 $(r,r^{\prime })\neq (0,0)$
and
$(r,r^{\prime })\neq (0,0)$
and
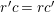 $r^{\prime }c=rc^{\prime }$
. In this case, the wall is the vertical line
$r^{\prime }c=rc^{\prime }$
. In this case, the wall is the vertical line
 $$\begin{eqnarray}s=\frac{c^{\prime }d-cd^{\prime }}{2(r^{\prime }d-rd^{\prime })}.\end{eqnarray}$$
$$\begin{eqnarray}s=\frac{c^{\prime }d-cd^{\prime }}{2(r^{\prime }d-rd^{\prime })}.\end{eqnarray}$$
So if
![]() $r\neq 0$
, then it is
$r\neq 0$
, then it is
![]() $s=\frac{c}{2r}$
.
$s=\frac{c}{2r}$
.
Case (3).
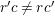 $r^{\prime }c\neq rc^{\prime }$
. In this case, the wall is a semicircle in the
$r^{\prime }c\neq rc^{\prime }$
. In this case, the wall is a semicircle in the
![]() $(s,t)$
-half plane
$(s,t)$
-half plane
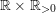 $\mathbb{R}\times \mathbb{R}_{{>}0}$
with center
$\mathbb{R}\times \mathbb{R}_{{>}0}$
with center
 $$\begin{eqnarray}\left(\frac{r^{\prime }d-rd^{\prime }}{r^{\prime }c-rc^{\prime }},0\right)\end{eqnarray}$$
$$\begin{eqnarray}\left(\frac{r^{\prime }d-rd^{\prime }}{r^{\prime }c-rc^{\prime }},0\right)\end{eqnarray}$$
and radius
 $$\begin{eqnarray}\sqrt{\left(\frac{r^{\prime }d-rd^{\prime }}{r^{\prime }c-rc^{\prime }}\right)^{2}-\frac{c^{\prime }d-cd^{\prime }}{r^{\prime }c-rc^{\prime }}}.\end{eqnarray}$$
$$\begin{eqnarray}\sqrt{\left(\frac{r^{\prime }d-rd^{\prime }}{r^{\prime }c-rc^{\prime }}\right)^{2}-\frac{c^{\prime }d-cd^{\prime }}{r^{\prime }c-rc^{\prime }}}.\end{eqnarray}$$
For
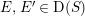 $E,E^{\prime }\in \text{D}(S)$
, we write
$E,E^{\prime }\in \text{D}(S)$
, we write
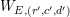 $W_{E,(r^{\prime },c^{\prime },d^{\prime })}$
for
$W_{E,(r^{\prime },c^{\prime },d^{\prime })}$
for
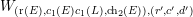 $W_{(\text{r}(E),c_{1}(E)c_{1}(L),\text{ch}_{2}(E)),(r^{\prime },c^{\prime },d^{\prime })}$
, and
$W_{(\text{r}(E),c_{1}(E)c_{1}(L),\text{ch}_{2}(E)),(r^{\prime },c^{\prime },d^{\prime })}$
, and
![]() $W_{E,E^{\prime }}$
for
$W_{E,E^{\prime }}$
for
 $W_{(\text{r}(E),c_{1}(E)c_{1}(L),\text{ch}_{2}(E)),(\text{r}(E^{\prime }),c_{1}(E^{\prime })c_{1}(L),\text{ch}_{2}(E^{\prime }))}$
. If
$W_{(\text{r}(E),c_{1}(E)c_{1}(L),\text{ch}_{2}(E)),(\text{r}(E^{\prime }),c_{1}(E^{\prime })c_{1}(L),\text{ch}_{2}(E^{\prime }))}$
. If
![]() $E$
is a semistable sheaf, then
$E$
is a semistable sheaf, then
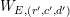 $W_{E,(r^{\prime },c^{\prime },d^{\prime })}$
is either a vertical line
$W_{E,(r^{\prime },c^{\prime },d^{\prime })}$
is either a vertical line
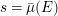 $s=\bar{\unicode[STIX]{x1D707}}(E)$
or a semicircle with the center
$s=\bar{\unicode[STIX]{x1D707}}(E)$
or a semicircle with the center
![]() $(x,0)$
, where
$(x,0)$
, where
 $$\begin{eqnarray}x=\frac{\text{r}(E)d^{\prime }-r^{\prime }\text{ch}_{2}(E)}{\text{r}(E)(c^{\prime }-2r^{\prime }\bar{\unicode[STIX]{x1D707}}(E))},\end{eqnarray}$$
$$\begin{eqnarray}x=\frac{\text{r}(E)d^{\prime }-r^{\prime }\text{ch}_{2}(E)}{\text{r}(E)(c^{\prime }-2r^{\prime }\bar{\unicode[STIX]{x1D707}}(E))},\end{eqnarray}$$
and the radius
 $$\begin{eqnarray}\sqrt{(x-\bar{\unicode[STIX]{x1D707}}(E))^{2}-\unicode[STIX]{x1D6E5}(E)+\frac{c_{1}(E)^{2}}{2\text{r}(E)^{2}}-\bar{\unicode[STIX]{x1D707}}(E)^{2}}\leqslant |x-\bar{\unicode[STIX]{x1D707}}(E)|.\end{eqnarray}$$
$$\begin{eqnarray}\sqrt{(x-\bar{\unicode[STIX]{x1D707}}(E))^{2}-\unicode[STIX]{x1D6E5}(E)+\frac{c_{1}(E)^{2}}{2\text{r}(E)^{2}}-\bar{\unicode[STIX]{x1D707}}(E)^{2}}\leqslant |x-\bar{\unicode[STIX]{x1D707}}(E)|.\end{eqnarray}$$
From this, we can see that for each point
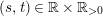 $(s,t)\in \mathbb{R}\times \mathbb{R}_{{>}0}$
, there exists a unique wall
$(s,t)\in \mathbb{R}\times \mathbb{R}_{{>}0}$
, there exists a unique wall
![]() $W_{E,\ast }$
passing through the point.
$W_{E,\ast }$
passing through the point.
Remark 4.1. It can happen that the radius (4.4) of the wall is zero. Although, strictly speaking, such a wall is empty (because we consider walls in the region
![]() $t>0$
), we call it a wall with radius zero.
$t>0$
), we call it a wall with radius zero.
4.2
 $(s,t)$
-semistability of symmetric exceptional bundles
$(s,t)$
-semistability of symmetric exceptional bundles
We consider
![]() $(s,t)$
-semistability of symmetric exceptional bundles. We follow closely the argument in [Reference HuizengaH, Section 9]. The argument goes as follows. Suppose that
$(s,t)$
-semistability of symmetric exceptional bundles. We follow closely the argument in [Reference HuizengaH, Section 9]. The argument goes as follows. Suppose that
![]() $E$
,
$E$
,
![]() $F$
and
$F$
and
![]() $G$
are symmetric exceptional bundles (or their shifts), and that there exists a triangle
$G$
are symmetric exceptional bundles (or their shifts), and that there exists a triangle
 $$\begin{eqnarray}E\rightarrow F\rightarrow G\rightarrow E[1]\end{eqnarray}$$
$$\begin{eqnarray}E\rightarrow F\rightarrow G\rightarrow E[1]\end{eqnarray}$$
in
![]() $\text{D}(S)$
. Then
$\text{D}(S)$
. Then
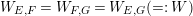 $W_{E,F}=W_{F,G}=W_{E,G}(=:W)$
. Suppose, moreover, that for a point
$W_{E,F}=W_{F,G}=W_{E,G}(=:W)$
. Suppose, moreover, that for a point
![]() $(s,t)$
on the wall
$(s,t)$
on the wall
![]() $W$
,
$W$
,
![]() $E$
,
$E$
,
![]() $F$
and
$F$
and
![]() $G$
belong to
$G$
belong to
![]() ${\mathcal{A}}_{s}$
. Then the above triangle gives rise to an exact sequence
${\mathcal{A}}_{s}$
. Then the above triangle gives rise to an exact sequence
 $$\begin{eqnarray}0\rightarrow E\rightarrow F\rightarrow G\rightarrow 0\end{eqnarray}$$
$$\begin{eqnarray}0\rightarrow E\rightarrow F\rightarrow G\rightarrow 0\end{eqnarray}$$
in the abelian category
![]() ${\mathcal{A}}_{s}$
. If two of
${\mathcal{A}}_{s}$
. If two of
![]() $E$
,
$E$
,
![]() $F$
and
$F$
and
![]() $G$
are
$G$
are
![]() $(s,t)$
-semistable, then the remaining one is also
$(s,t)$
-semistable, then the remaining one is also
![]() $(s,t)$
-semistable since their values of
$(s,t)$
-semistable since their values of
![]() $\unicode[STIX]{x1D707}_{s.t}$
are equal.
$\unicode[STIX]{x1D707}_{s.t}$
are equal.
Since all symmetric exceptional bundles are obtained from the symmetric exceptional triple
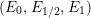 $(E_{0},E_{1/2},E_{1})$
, the following lemma is the first step of the argument.
$(E_{0},E_{1/2},E_{1})$
, the following lemma is the first step of the argument.
Lemma 4.2. Let
![]() $\unicode[STIX]{x1D6FC}$
be an integer or a half integer. Then the symmetric exceptional bundle
$\unicode[STIX]{x1D6FC}$
be an integer or a half integer. Then the symmetric exceptional bundle
![]() $E_{\unicode[STIX]{x1D6FC}}$
is
$E_{\unicode[STIX]{x1D6FC}}$
is
![]() $(s,t)$
-semistable for any
$(s,t)$
-semistable for any
![]() $s<\unicode[STIX]{x1D6FC}$
. The shift
$s<\unicode[STIX]{x1D6FC}$
. The shift
![]() $E_{\unicode[STIX]{x1D6FC}}[1]$
is
$E_{\unicode[STIX]{x1D6FC}}[1]$
is
![]() $(s,t)$
-semistable for any
$(s,t)$
-semistable for any
![]() $\unicode[STIX]{x1D6FC}\leqslant s$
.
$\unicode[STIX]{x1D6FC}\leqslant s$
.
Proof. If
![]() $\unicode[STIX]{x1D6FC}$
is an integer, then
$\unicode[STIX]{x1D6FC}$
is an integer, then
![]() $E_{\unicode[STIX]{x1D6FC}}$
is a line bundle. The
$E_{\unicode[STIX]{x1D6FC}}$
is a line bundle. The
![]() $(s,t)$
-semistability of
$(s,t)$
-semistability of
![]() $E_{\unicode[STIX]{x1D6FC}}$
and
$E_{\unicode[STIX]{x1D6FC}}$
and
![]() $E_{\unicode[STIX]{x1D6FC}}[1]$
follows from [Reference Arcara and MilesAM, Theorem 1,1] (or we can argue as in the proof of Proposition 6.2 (d) in [Reference Arcara, Bertram, Coskun and HuizengaABCH]). If
$E_{\unicode[STIX]{x1D6FC}}[1]$
follows from [Reference Arcara and MilesAM, Theorem 1,1] (or we can argue as in the proof of Proposition 6.2 (d) in [Reference Arcara, Bertram, Coskun and HuizengaABCH]). If
![]() $\unicode[STIX]{x1D6FC}$
is a half integer, then
$\unicode[STIX]{x1D6FC}$
is a half integer, then
![]() $E_{\unicode[STIX]{x1D6FC}}$
is a direct sum of two line bundles with the same
$E_{\unicode[STIX]{x1D6FC}}$
is a direct sum of two line bundles with the same
![]() $\unicode[STIX]{x1D707}_{s,t}$
. So the result follows.◻
$\unicode[STIX]{x1D707}_{s,t}$
. So the result follows.◻
Lemma 4.3. Let
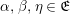 $\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D702}\in \mathfrak{E}$
satisfy
$\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D702}\in \mathfrak{E}$
satisfy
 $\unicode[STIX]{x1D6FC}<\unicode[STIX]{x1D6FD}<\unicode[STIX]{x1D702}<\unicode[STIX]{x1D6FC}+2$
and
$\unicode[STIX]{x1D6FC}<\unicode[STIX]{x1D6FD}<\unicode[STIX]{x1D702}<\unicode[STIX]{x1D6FC}+2$
and
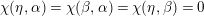 $\unicode[STIX]{x1D712}(\unicode[STIX]{x1D702},\unicode[STIX]{x1D6FC})=\unicode[STIX]{x1D712}(\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FC})=\unicode[STIX]{x1D712}(\unicode[STIX]{x1D702},\unicode[STIX]{x1D6FD})=0$
. Then the center of the semicircular wall
$\unicode[STIX]{x1D712}(\unicode[STIX]{x1D702},\unicode[STIX]{x1D6FC})=\unicode[STIX]{x1D712}(\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FC})=\unicode[STIX]{x1D712}(\unicode[STIX]{x1D702},\unicode[STIX]{x1D6FD})=0$
. Then the center of the semicircular wall
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
(resp.
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
(resp.
![]() $W_{E_{\unicode[STIX]{x1D6FD}},E_{\unicode[STIX]{x1D702}}}$
) is
$W_{E_{\unicode[STIX]{x1D6FD}},E_{\unicode[STIX]{x1D702}}}$
) is
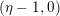 $(\unicode[STIX]{x1D702}-1,0)$
(resp.
$(\unicode[STIX]{x1D702}-1,0)$
(resp.
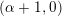 $(\unicode[STIX]{x1D6FC}+1,0)$
), and its radius is
$(\unicode[STIX]{x1D6FC}+1,0)$
), and its radius is
![]() $\sqrt{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D702}}}$
(resp.
$\sqrt{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D702}}}$
(resp.
![]() $\sqrt{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}$
).
$\sqrt{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}$
).
Proof. We prove the lemma for
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
. By (4.3), the center of
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
. By (4.3), the center of
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
is
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
is
![]() $(x,0)$
, where
$(x,0)$
, where
 $$\begin{eqnarray}\displaystyle x & = & \displaystyle \frac{\text{ch}_{2}(E_{\unicode[STIX]{x1D6FC}})/\text{r}(E_{\unicode[STIX]{x1D6FC}})-\text{ch}_{2}(E_{\unicode[STIX]{x1D6FD}})/\text{r}(E_{\unicode[STIX]{x1D6FD}})}{2(\bar{\unicode[STIX]{x1D707}}(E_{\unicode[STIX]{x1D6FC}})-\bar{\unicode[STIX]{x1D707}}(E_{\unicode[STIX]{x1D6FD}}))}\nonumber\\ \displaystyle & = & \displaystyle \frac{\unicode[STIX]{x1D6FC}^{2}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-(\unicode[STIX]{x1D6FD}^{2}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}})}{2(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}=\frac{\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD}}{2}+\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}=\unicode[STIX]{x1D702}-1,\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle x & = & \displaystyle \frac{\text{ch}_{2}(E_{\unicode[STIX]{x1D6FC}})/\text{r}(E_{\unicode[STIX]{x1D6FC}})-\text{ch}_{2}(E_{\unicode[STIX]{x1D6FD}})/\text{r}(E_{\unicode[STIX]{x1D6FD}})}{2(\bar{\unicode[STIX]{x1D707}}(E_{\unicode[STIX]{x1D6FC}})-\bar{\unicode[STIX]{x1D707}}(E_{\unicode[STIX]{x1D6FD}}))}\nonumber\\ \displaystyle & = & \displaystyle \frac{\unicode[STIX]{x1D6FC}^{2}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-(\unicode[STIX]{x1D6FD}^{2}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}})}{2(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}=\frac{\unicode[STIX]{x1D6FC}+\unicode[STIX]{x1D6FD}}{2}+\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}=\unicode[STIX]{x1D702}-1,\nonumber\end{eqnarray}$$
where we used Lemma 2.6(4) in the last equality. By (4.4), the square of the radius of
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
is
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
is
 $$\begin{eqnarray}\displaystyle \left(\frac{\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}}{2}+\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)^{2}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}} & = & \displaystyle \left(\frac{\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}}{2}\right)^{2}-\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}+\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}}{2}+\left(\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)^{2}\nonumber\\ \displaystyle & = & \displaystyle \left(\frac{\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}}{2}\right)^{2}-\frac{\text{P}(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}{2}+\left(\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)^{2}\nonumber\\ \displaystyle & = & \displaystyle \unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D702}},\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \left(\frac{\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}}{2}+\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)^{2}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}} & = & \displaystyle \left(\frac{\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}}{2}\right)^{2}-\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}+\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}}{2}+\left(\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)^{2}\nonumber\\ \displaystyle & = & \displaystyle \left(\frac{\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD}}{2}\right)^{2}-\frac{\text{P}(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}{2}+\left(\frac{\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}}{2(\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})}\right)^{2}\nonumber\\ \displaystyle & = & \displaystyle \unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D702}},\nonumber\end{eqnarray}$$
where we used
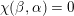 $\unicode[STIX]{x1D712}(\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FC})=0$
in the second equality, and Lemma 2.6(5) in the last equality.◻
$\unicode[STIX]{x1D712}(\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FC})=0$
in the second equality, and Lemma 2.6(5) in the last equality.◻
Now we come to the main theorem of this section.
Theorem 4.4. Consider symmetric exceptional slopes
![]() $\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD}$
and
$\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD}$
and
![]() $\unicode[STIX]{x1D702}$
given by
$\unicode[STIX]{x1D702}$
given by
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D716}\left(\frac{p}{2^{q}}\right)\!,\qquad \unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D716}\left(\frac{p+1}{2^{q}}\right)\!,\qquad \unicode[STIX]{x1D702}=\unicode[STIX]{x1D716}\left(\frac{p+2}{2^{q}}\right)\!, & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D716}\left(\frac{p}{2^{q}}\right)\!,\qquad \unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D716}\left(\frac{p+1}{2^{q}}\right)\!,\qquad \unicode[STIX]{x1D702}=\unicode[STIX]{x1D716}\left(\frac{p+2}{2^{q}}\right)\!, & & \displaystyle \nonumber\end{eqnarray}$$
where
![]() $p$
is even and
$p$
is even and
![]() $q\geqslant 1$
. Then
$q\geqslant 1$
. Then
![]() $E_{\unicode[STIX]{x1D6FD}}$
(resp.
$E_{\unicode[STIX]{x1D6FD}}$
(resp.
![]() $E_{\unicode[STIX]{x1D6FD}}[1]$
) is
$E_{\unicode[STIX]{x1D6FD}}[1]$
) is
![]() $(s,t)$
-semistable if
$(s,t)$
-semistable if
![]() $(s,t)$
with
$(s,t)$
with
![]() $s<\unicode[STIX]{x1D6FD}$
(resp.
$s<\unicode[STIX]{x1D6FD}$
(resp.
![]() $s>\unicode[STIX]{x1D6FD}$
) lies on or outside the semicircular wall
$s>\unicode[STIX]{x1D6FD}$
) lies on or outside the semicircular wall
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
(resp.
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
(resp.
![]() $W_{E_{\unicode[STIX]{x1D6FD}},E_{\unicode[STIX]{x1D702}}}$
). When the radius of the wall is zero, this sentence should be understood as “
$W_{E_{\unicode[STIX]{x1D6FD}},E_{\unicode[STIX]{x1D702}}}$
). When the radius of the wall is zero, this sentence should be understood as “
![]() $E_{\unicode[STIX]{x1D6FD}}$
(resp.
$E_{\unicode[STIX]{x1D6FD}}$
(resp.
![]() $E_{\unicode[STIX]{x1D6FD}}[1]$
) is
$E_{\unicode[STIX]{x1D6FD}}[1]$
) is
![]() $(s,t)$
-semistable for any
$(s,t)$
-semistable for any
![]() $(s,t)$
with
$(s,t)$
with
![]() $\unicode[STIX]{x1D6FD}>s$
(resp.
$\unicode[STIX]{x1D6FD}>s$
(resp.
![]() $s>\unicode[STIX]{x1D6FD}$
).”
$s>\unicode[STIX]{x1D6FD}$
).”
The proof of Theorem 4.4 goes as that of [Reference HuizengaH, Theorem 9.1], but occasionally gets more involved due to the appearance of walls with radius zero.
Lemma 4.5. Let
![]() $E$
be a semistable sheaf.
$E$
be a semistable sheaf.
-
(1) Assume that
 $E$
is
$E$
is
 $(s_{0}.t_{0})$
-semistable with
$(s_{0}.t_{0})$
-semistable with
 $s_{0}<\bar{\unicode[STIX]{x1D707}}(E)$
. If
$s_{0}<\bar{\unicode[STIX]{x1D707}}(E)$
. If
 $(s,t)$
with
$(s,t)$
with
 $s<\bar{\unicode[STIX]{x1D707}}(E)$
is outside the wall
$s<\bar{\unicode[STIX]{x1D707}}(E)$
is outside the wall
 $W_{E,\ast }$
passing through
$W_{E,\ast }$
passing through
 $(s_{0},t_{0})$
, then
$(s_{0},t_{0})$
, then
 $E$
is
$E$
is
 $(s,t)$
-semistable.
$(s,t)$
-semistable. -
(2) Assume that
 $E[1]$
is
$E[1]$
is
 $(s_{0}.t_{0})$
-semistable with
$(s_{0}.t_{0})$
-semistable with
 $s_{0}>\bar{\unicode[STIX]{x1D707}}(E)$
. If
$s_{0}>\bar{\unicode[STIX]{x1D707}}(E)$
. If
 $(s,t)$
with
$(s,t)$
with
 $s>\bar{\unicode[STIX]{x1D707}}(E)$
is outside the wall
$s>\bar{\unicode[STIX]{x1D707}}(E)$
is outside the wall
 $W_{E,\ast }$
passing through
$W_{E,\ast }$
passing through
 $(s_{0},t_{0})$
, then
$(s_{0},t_{0})$
, then
 $E[1]$
is
$E[1]$
is
 $(s,t)$
-semistable.
$(s,t)$
-semistable.
Proof. We prove (1). Let
![]() $W$
be the unique wall
$W$
be the unique wall
![]() $W_{E,\ast }$
passing through
$W_{E,\ast }$
passing through
![]() $(s,t)$
. Let
$(s,t)$
. Let
![]() $(s_{0},t^{\prime })$
be the point on
$(s_{0},t^{\prime })$
be the point on
![]() $W$
. If
$W$
. If
![]() $E$
is not
$E$
is not
![]() $(s,t)$
-semistable, then it is not
$(s,t)$
-semistable, then it is not
![]() $(s_{0},t^{\prime })$
-semistable. (In fact, if
$(s_{0},t^{\prime })$
-semistable. (In fact, if
 $M\rightarrow E$
is a
$M\rightarrow E$
is a
![]() $(s,t)$
-destabilizing subobject in
$(s,t)$
-destabilizing subobject in
![]() ${\mathcal{A}}_{s}$
with minimum rank, then the argument of the proof of [Reference Arcara, Bertram, Coskun and HuizengaABCH, Lemma 6.3] shows that
${\mathcal{A}}_{s}$
with minimum rank, then the argument of the proof of [Reference Arcara, Bertram, Coskun and HuizengaABCH, Lemma 6.3] shows that
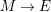 $M\rightarrow E$
is also a
$M\rightarrow E$
is also a
![]() $(s_{0},t^{\prime })$
-destabilizing subobject in
$(s_{0},t^{\prime })$
-destabilizing subobject in
![]() ${\mathcal{A}}_{s_{0}}$
.) Suppose that a subobject
${\mathcal{A}}_{s_{0}}$
.) Suppose that a subobject
![]() $M\subset E$
in
$M\subset E$
in
![]() ${\mathcal{A}}_{s_{0}}$
${\mathcal{A}}_{s_{0}}$
![]() $(s_{0},t^{\prime })$
-destabilizes
$(s_{0},t^{\prime })$
-destabilizes
![]() $E$
. The inequality
$E$
. The inequality
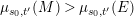 $\unicode[STIX]{x1D707}_{s_{0},t^{\prime }}(M)>\unicode[STIX]{x1D707}_{s_{0},t^{\prime }}(E)$
is equivalent to
$\unicode[STIX]{x1D707}_{s_{0},t^{\prime }}(M)>\unicode[STIX]{x1D707}_{s_{0},t^{\prime }}(E)$
is equivalent to
 $$\begin{eqnarray}\displaystyle & & \displaystyle \frac{(1/2)(s_{0}^{2}-{t^{\prime }}^{2})-s_{0}\bar{\unicode[STIX]{x1D707}}(M)+(\text{ch}_{2}(M)/2\text{r}(M))}{\bar{\unicode[STIX]{x1D707}}(M)-s_{0}}\nonumber\\ \displaystyle & & \displaystyle \quad >\frac{(1/2)(s_{0}^{2}-{t^{\prime }}^{2})-s_{0}\bar{\unicode[STIX]{x1D707}}(E)+(\text{ch}_{2}(E)/2\text{r}(E))}{\bar{\unicode[STIX]{x1D707}}(E)-s_{0}}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle \frac{(1/2)(s_{0}^{2}-{t^{\prime }}^{2})-s_{0}\bar{\unicode[STIX]{x1D707}}(M)+(\text{ch}_{2}(M)/2\text{r}(M))}{\bar{\unicode[STIX]{x1D707}}(M)-s_{0}}\nonumber\\ \displaystyle & & \displaystyle \quad >\frac{(1/2)(s_{0}^{2}-{t^{\prime }}^{2})-s_{0}\bar{\unicode[STIX]{x1D707}}(E)+(\text{ch}_{2}(E)/2\text{r}(E))}{\bar{\unicode[STIX]{x1D707}}(E)-s_{0}}.\nonumber\end{eqnarray}$$
If
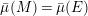 $\bar{\unicode[STIX]{x1D707}}(M)=\bar{\unicode[STIX]{x1D707}}(E)$
, then
$\bar{\unicode[STIX]{x1D707}}(M)=\bar{\unicode[STIX]{x1D707}}(E)$
, then
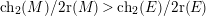 $\text{ch}_{2}(M)/2\text{r}(M)>\text{ch}_{2}(E)/2\text{r}(E)$
, which contradicts the semistability of
$\text{ch}_{2}(M)/2\text{r}(M)>\text{ch}_{2}(E)/2\text{r}(E)$
, which contradicts the semistability of
![]() $E$
. Thus
$E$
. Thus
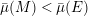 $\bar{\unicode[STIX]{x1D707}}(M)<\bar{\unicode[STIX]{x1D707}}(E)$
. Then we have
$\bar{\unicode[STIX]{x1D707}}(M)<\bar{\unicode[STIX]{x1D707}}(E)$
. Then we have
 $\unicode[STIX]{x1D707}_{s_{0},t^{\prime \prime }}(M)<\unicode[STIX]{x1D707}_{s_{0},t^{\prime \prime }}(E)$
for
$\unicode[STIX]{x1D707}_{s_{0},t^{\prime \prime }}(M)<\unicode[STIX]{x1D707}_{s_{0},t^{\prime \prime }}(E)$
for
![]() $t^{\prime \prime }\gg 0$
. This shows that the wall
$t^{\prime \prime }\gg 0$
. This shows that the wall
![]() $W_{E,M}$
lies outside
$W_{E,M}$
lies outside
![]() $W$
. On the other hand, we have
$W$
. On the other hand, we have
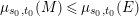 $\unicode[STIX]{x1D707}_{s_{0},t_{0}}(M)\leqslant \unicode[STIX]{x1D707}_{s_{0},t_{0}}(E)$
because
$\unicode[STIX]{x1D707}_{s_{0},t_{0}}(M)\leqslant \unicode[STIX]{x1D707}_{s_{0},t_{0}}(E)$
because
![]() $E$
is
$E$
is
![]() $(s_{0},t_{0})$
-semistable. This shows that the wall
$(s_{0},t_{0})$
-semistable. This shows that the wall
![]() $W_{E,M}$
lies inside
$W_{E,M}$
lies inside
![]() $W$
. This is absurd.◻
$W$
. This is absurd.◻
We put
 $$\begin{eqnarray}\displaystyle & \displaystyle \unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D716}\left(\frac{p}{2^{q}}\right)\!,\qquad \unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D716}\left(\frac{p+1}{2^{q}}\right)\!,\quad \unicode[STIX]{x1D702}=\unicode[STIX]{x1D716}\left(\frac{p+2}{2^{q}}\right) & \displaystyle \nonumber\\ \displaystyle & \displaystyle \unicode[STIX]{x1D701}_{2}=\unicode[STIX]{x1D716}\left(\frac{p-2}{2^{q}}\right)\!,\qquad \unicode[STIX]{x1D714}_{2}=\unicode[STIX]{x1D716}\left(\frac{p-2}{2^{q}}+2\right)\!, & \displaystyle \nonumber\\ \displaystyle & \displaystyle \unicode[STIX]{x1D714}_{0}=\unicode[STIX]{x1D716}\left(\frac{p+4}{2^{q}}\right)\!,\qquad \unicode[STIX]{x1D701}_{0}=\unicode[STIX]{x1D716}\left(\frac{p+4}{2^{q}}-2\right)\!, & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle \unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D716}\left(\frac{p}{2^{q}}\right)\!,\qquad \unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D716}\left(\frac{p+1}{2^{q}}\right)\!,\quad \unicode[STIX]{x1D702}=\unicode[STIX]{x1D716}\left(\frac{p+2}{2^{q}}\right) & \displaystyle \nonumber\\ \displaystyle & \displaystyle \unicode[STIX]{x1D701}_{2}=\unicode[STIX]{x1D716}\left(\frac{p-2}{2^{q}}\right)\!,\qquad \unicode[STIX]{x1D714}_{2}=\unicode[STIX]{x1D716}\left(\frac{p-2}{2^{q}}+2\right)\!, & \displaystyle \nonumber\\ \displaystyle & \displaystyle \unicode[STIX]{x1D714}_{0}=\unicode[STIX]{x1D716}\left(\frac{p+4}{2^{q}}\right)\!,\qquad \unicode[STIX]{x1D701}_{0}=\unicode[STIX]{x1D716}\left(\frac{p+4}{2^{q}}-2\right)\!, & \displaystyle \nonumber\end{eqnarray}$$
where
![]() $p$
is even and
$p$
is even and
![]() $q\geqslant 2$
.
$q\geqslant 2$
.
Notation 4.6. If
![]() $E$
is a symmetric exceptional bundle, we put
$E$
is a symmetric exceptional bundle, we put
 $$\begin{eqnarray}\tilde{\unicode[STIX]{x1D712}}(E,F)=\left\{\begin{array}{@{}ll@{}}\unicode[STIX]{x1D712}(E,F)/2\quad & \text{if }E\text{ is even},\\ \unicode[STIX]{x1D712}(E,F)\quad & \text{if }E\text{is odd}.\end{array}\right.\end{eqnarray}$$
$$\begin{eqnarray}\tilde{\unicode[STIX]{x1D712}}(E,F)=\left\{\begin{array}{@{}ll@{}}\unicode[STIX]{x1D712}(E,F)/2\quad & \text{if }E\text{ is even},\\ \unicode[STIX]{x1D712}(E,F)\quad & \text{if }E\text{is odd}.\end{array}\right.\end{eqnarray}$$
If
![]() $F$
is a symmetric exceptional bundle, we put
$F$
is a symmetric exceptional bundle, we put
 $$\begin{eqnarray}\tilde{\unicode[STIX]{x1D712}}^{\ast }(E,F)=\left\{\begin{array}{@{}ll@{}}\unicode[STIX]{x1D712}(E,F)/2\quad & \text{if }F\text{ is even},\\ \unicode[STIX]{x1D712}(E,F)\quad & \text{if }F\text{ is odd}.\end{array}\right.\end{eqnarray}$$
$$\begin{eqnarray}\tilde{\unicode[STIX]{x1D712}}^{\ast }(E,F)=\left\{\begin{array}{@{}ll@{}}\unicode[STIX]{x1D712}(E,F)/2\quad & \text{if }F\text{ is even},\\ \unicode[STIX]{x1D712}(E,F)\quad & \text{if }F\text{ is odd}.\end{array}\right.\end{eqnarray}$$
With this notation, we have the following lemma.
Lemma 4.7. Let
 $i\in \{0,2\}$
. For
$i\in \{0,2\}$
. For
 $p\equiv i~(\text{mod}~4)$
, there are exact sequences
$p\equiv i~(\text{mod}~4)$
, there are exact sequences
 $$\begin{eqnarray}\displaystyle & \displaystyle 0\rightarrow E_{\unicode[STIX]{x1D701}_{i}}\rightarrow E_{\unicode[STIX]{x1D6FC}}^{\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}})}\rightarrow E_{\unicode[STIX]{x1D6FD}}\rightarrow 0, & \displaystyle\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle 0\rightarrow E_{\unicode[STIX]{x1D701}_{i}}\rightarrow E_{\unicode[STIX]{x1D6FC}}^{\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}})}\rightarrow E_{\unicode[STIX]{x1D6FD}}\rightarrow 0, & \displaystyle\end{eqnarray}$$
 $$\begin{eqnarray}\displaystyle & \displaystyle 0\rightarrow E_{\unicode[STIX]{x1D6FD}}\rightarrow E_{\unicode[STIX]{x1D702}}^{\tilde{\unicode[STIX]{x1D712}}^{\ast }(E_{\unicode[STIX]{x1D6FD}},E_{\unicode[STIX]{x1D702}})}\rightarrow E_{\unicode[STIX]{x1D714}_{i}}\rightarrow 0. & \displaystyle\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle 0\rightarrow E_{\unicode[STIX]{x1D6FD}}\rightarrow E_{\unicode[STIX]{x1D702}}^{\tilde{\unicode[STIX]{x1D712}}^{\ast }(E_{\unicode[STIX]{x1D6FD}},E_{\unicode[STIX]{x1D702}})}\rightarrow E_{\unicode[STIX]{x1D714}_{i}}\rightarrow 0. & \displaystyle\end{eqnarray}$$
Proof. We prove the case
![]() $i=0$
. By the construction of symmetric exceptional bundles, we have
$i=0$
. By the construction of symmetric exceptional bundles, we have
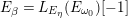 $E_{\unicode[STIX]{x1D6FD}}=L_{E_{\unicode[STIX]{x1D702}}}(E_{\unicode[STIX]{x1D714}_{0}})[-1]$
. Since right and left mutations are inverses to each other, we have
$E_{\unicode[STIX]{x1D6FD}}=L_{E_{\unicode[STIX]{x1D702}}}(E_{\unicode[STIX]{x1D714}_{0}})[-1]$
. Since right and left mutations are inverses to each other, we have
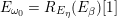 $E_{\unicode[STIX]{x1D714}_{0}}=R_{E_{\unicode[STIX]{x1D702}}}(E_{\unicode[STIX]{x1D6FD}})[1]$
, which shows (4.5). By [Reference Bridgeland and SternBS, Lemma 5.2], we have
$E_{\unicode[STIX]{x1D714}_{0}}=R_{E_{\unicode[STIX]{x1D702}}}(E_{\unicode[STIX]{x1D6FD}})[1]$
, which shows (4.5). By [Reference Bridgeland and SternBS, Lemma 5.2], we have
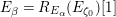 $E_{\unicode[STIX]{x1D6FD}}=R_{E_{\unicode[STIX]{x1D6FC}}}(E_{\unicode[STIX]{x1D701}_{0}})[1]$
. So we have
$E_{\unicode[STIX]{x1D6FD}}=R_{E_{\unicode[STIX]{x1D6FC}}}(E_{\unicode[STIX]{x1D701}_{0}})[1]$
. So we have
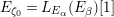 $E_{\unicode[STIX]{x1D701}_{0}}=L_{E_{\unicode[STIX]{x1D6FC}}}(E_{\unicode[STIX]{x1D6FD}})[1]$
, which show (4.6).◻
$E_{\unicode[STIX]{x1D701}_{0}}=L_{E_{\unicode[STIX]{x1D6FC}}}(E_{\unicode[STIX]{x1D6FD}})[1]$
, which show (4.6).◻
Proof of Theorem 4.4.
We prove the theorem by induction on
![]() $q$
. When
$q$
. When
![]() $q=1$
, the theorem holds because of Lemma 4.2. Suppose
$q=1$
, the theorem holds because of Lemma 4.2. Suppose
![]() $q\geqslant 2$
. We show that
$q\geqslant 2$
. We show that
![]() $E_{\unicode[STIX]{x1D6FD}}$
is
$E_{\unicode[STIX]{x1D6FD}}$
is
![]() $(s,t)$
-semistable along and outside the semicircular wall
$(s,t)$
-semistable along and outside the semicircular wall
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
. The verification for
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
. The verification for
![]() $E_{\unicode[STIX]{x1D6FD}}[1]$
is similar and left to the reader.
$E_{\unicode[STIX]{x1D6FD}}[1]$
is similar and left to the reader.
Case (i)
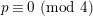 $p\equiv 0~(\text{mod}~4)$
. The center of
$p\equiv 0~(\text{mod}~4)$
. The center of
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
is
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
is
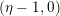 $(\unicode[STIX]{x1D702}-1,0)$
by Lemma 4.3. Since
$(\unicode[STIX]{x1D702}-1,0)$
by Lemma 4.3. Since
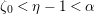 $\unicode[STIX]{x1D701}_{0}<\unicode[STIX]{x1D702}-1<\unicode[STIX]{x1D6FC}$
, the wall
$\unicode[STIX]{x1D701}_{0}<\unicode[STIX]{x1D702}-1<\unicode[STIX]{x1D6FC}$
, the wall
 $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}=W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D701}_{0}}}$
lies between the vertical lines
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}=W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D701}_{0}}}$
lies between the vertical lines
![]() $s=\unicode[STIX]{x1D701}_{0}$
and
$s=\unicode[STIX]{x1D701}_{0}$
and
![]() $s=\unicode[STIX]{x1D6FC}$
. For any point
$s=\unicode[STIX]{x1D6FC}$
. For any point
![]() $(s,t)$
on
$(s,t)$
on
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
, a shift of (4.5)
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
, a shift of (4.5)
 $$\begin{eqnarray}0\rightarrow E_{\unicode[STIX]{x1D6FC}}^{\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}})}\rightarrow E_{\unicode[STIX]{x1D6FD}}\rightarrow E_{\unicode[STIX]{x1D701}_{0}}[1]\rightarrow 0\end{eqnarray}$$
$$\begin{eqnarray}0\rightarrow E_{\unicode[STIX]{x1D6FC}}^{\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}})}\rightarrow E_{\unicode[STIX]{x1D6FD}}\rightarrow E_{\unicode[STIX]{x1D701}_{0}}[1]\rightarrow 0\end{eqnarray}$$
is an exact sequence in
![]() ${\mathcal{A}}_{s}$
. In order to show the
${\mathcal{A}}_{s}$
. In order to show the
![]() $(s,t)$
-semistability of
$(s,t)$
-semistability of
![]() $E_{\unicode[STIX]{x1D6FD}}$
for
$E_{\unicode[STIX]{x1D6FD}}$
for
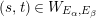 $(s,t)\in W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
, we show that
$(s,t)\in W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
, we show that
![]() $E_{\unicode[STIX]{x1D6FC}}$
and
$E_{\unicode[STIX]{x1D6FC}}$
and
![]() $E_{\unicode[STIX]{x1D701}_{0}}[1]$
are
$E_{\unicode[STIX]{x1D701}_{0}}[1]$
are
![]() $(s,t)$
-semistable. Here note that
$(s,t)$
-semistable. Here note that
![]() $E_{\unicode[STIX]{x1D6FC}}$
and
$E_{\unicode[STIX]{x1D6FC}}$
and
![]() $E_{\unicode[STIX]{x1D701}_{0}}[1]$
have the same value of
$E_{\unicode[STIX]{x1D701}_{0}}[1]$
have the same value of
![]() $\unicode[STIX]{x1D707}_{s,t}$
because
$\unicode[STIX]{x1D707}_{s,t}$
because
![]() $(s,t)$
is on
$(s,t)$
is on
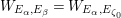 $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}=W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D701}_{0}}}$
.
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}=W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D701}_{0}}}$
.
If
![]() $\unicode[STIX]{x1D6FC}$
is an integer or a half integer, then
$\unicode[STIX]{x1D6FC}$
is an integer or a half integer, then
![]() $E_{\unicode[STIX]{x1D6FC}}$
is
$E_{\unicode[STIX]{x1D6FC}}$
is
![]() $(s,t)$
-semistable by Lemma 4.2. Otherwise, we have
$(s,t)$
-semistable by Lemma 4.2. Otherwise, we have
 $$\begin{eqnarray}\displaystyle u:=\unicode[STIX]{x1D716}\left(\frac{p^{\prime }}{2^{q^{\prime }}}\right)\!,\qquad \unicode[STIX]{x1D6FC}=\left(\frac{p^{\prime }+1}{2^{q^{\prime }}}\right)\!,\qquad v:=\unicode[STIX]{x1D716}\left(\frac{p^{\prime }+2}{2^{q^{\prime }}}\right)\!, & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle u:=\unicode[STIX]{x1D716}\left(\frac{p^{\prime }}{2^{q^{\prime }}}\right)\!,\qquad \unicode[STIX]{x1D6FC}=\left(\frac{p^{\prime }+1}{2^{q^{\prime }}}\right)\!,\qquad v:=\unicode[STIX]{x1D716}\left(\frac{p^{\prime }+2}{2^{q^{\prime }}}\right)\!, & & \displaystyle \nonumber\end{eqnarray}$$
where
![]() $p^{\prime }$
is even and
$p^{\prime }$
is even and
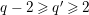 $q-2\geqslant q^{\prime }\geqslant 2$
. By the induction hypothesis,
$q-2\geqslant q^{\prime }\geqslant 2$
. By the induction hypothesis,
![]() $E_{\unicode[STIX]{x1D6FC}}$
is
$E_{\unicode[STIX]{x1D6FC}}$
is
![]() $(s,t)$
-semistable on the wall
$(s,t)$
-semistable on the wall
![]() $W_{E_{u},E_{\unicode[STIX]{x1D6FC}}}$
. To show that
$W_{E_{u},E_{\unicode[STIX]{x1D6FC}}}$
. To show that
![]() $E_{\unicode[STIX]{x1D6FC}}$
is
$E_{\unicode[STIX]{x1D6FC}}$
is
![]() $(s,t)$
-semistable on
$(s,t)$
-semistable on
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
, it suffices to show that
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
, it suffices to show that
![]() $W_{E_{u},E_{\unicode[STIX]{x1D6FC}}}$
lies inside
$W_{E_{u},E_{\unicode[STIX]{x1D6FC}}}$
lies inside
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
. The center of
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
. The center of
![]() $W_{E_{u},E_{\unicode[STIX]{x1D6FC}}}$
is
$W_{E_{u},E_{\unicode[STIX]{x1D6FC}}}$
is
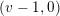 $(v-1,0)$
by Lemma 4.3. Note that
$(v-1,0)$
by Lemma 4.3. Note that
 $v-1<\unicode[STIX]{x1D6FC}$
and
$v-1<\unicode[STIX]{x1D6FC}$
and
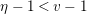 $\unicode[STIX]{x1D702}-1<v-1$
. This shows that both
$\unicode[STIX]{x1D702}-1<v-1$
. This shows that both
![]() $W_{E_{u},E_{\unicode[STIX]{x1D6FC}}}$
and
$W_{E_{u},E_{\unicode[STIX]{x1D6FC}}}$
and
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
lie to the left of the vertical line
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
lie to the left of the vertical line
![]() $s=\unicode[STIX]{x1D6FC}$
, and the center of
$s=\unicode[STIX]{x1D6FC}$
, and the center of
![]() $W_{E_{u},E_{\unicode[STIX]{x1D6FC}}}$
is right to the center of
$W_{E_{u},E_{\unicode[STIX]{x1D6FC}}}$
is right to the center of
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
. So
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
. So
![]() $W_{E_{u},E_{\unicode[STIX]{x1D6FC}}}$
lies inside
$W_{E_{u},E_{\unicode[STIX]{x1D6FC}}}$
lies inside
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
.
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
.
Next we show that
![]() $E_{\unicode[STIX]{x1D701}_{0}}[1]$
is
$E_{\unicode[STIX]{x1D701}_{0}}[1]$
is
![]() $(s,t)$
-semistable on the wall
$(s,t)$
-semistable on the wall
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
. If
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
. If
![]() $\unicode[STIX]{x1D701}_{0}$
is an integer or a half integer, this follows from Lemma 4.2. Otherwise, we have
$\unicode[STIX]{x1D701}_{0}$
is an integer or a half integer, this follows from Lemma 4.2. Otherwise, we have
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D70E}^{\prime }:=\unicode[STIX]{x1D716}\left(\frac{p^{\prime }}{2^{q^{\prime }}}\right)\!,\qquad \unicode[STIX]{x1D701}_{0}=\left(\frac{p^{\prime }+1}{2^{q^{\prime }}}\right)\!,\qquad \unicode[STIX]{x1D70F}^{\prime }:=\unicode[STIX]{x1D716}\left(\frac{p^{\prime }+2}{2^{q^{\prime }}}\right)\!, & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D70E}^{\prime }:=\unicode[STIX]{x1D716}\left(\frac{p^{\prime }}{2^{q^{\prime }}}\right)\!,\qquad \unicode[STIX]{x1D701}_{0}=\left(\frac{p^{\prime }+1}{2^{q^{\prime }}}\right)\!,\qquad \unicode[STIX]{x1D70F}^{\prime }:=\unicode[STIX]{x1D716}\left(\frac{p^{\prime }+2}{2^{q^{\prime }}}\right)\!, & & \displaystyle \nonumber\end{eqnarray}$$
where
![]() $p^{\prime }$
is even and
$p^{\prime }$
is even and
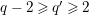 $q-2\geqslant q^{\prime }\geqslant 2$
. Note that
$q-2\geqslant q^{\prime }\geqslant 2$
. Note that
 $\unicode[STIX]{x1D70E}^{\prime }\leqslant \unicode[STIX]{x1D6FC}-2$
. By the induction hypothesis,
$\unicode[STIX]{x1D70E}^{\prime }\leqslant \unicode[STIX]{x1D6FC}-2$
. By the induction hypothesis,
![]() $E_{\unicode[STIX]{x1D701}_{0}}[1]$
is
$E_{\unicode[STIX]{x1D701}_{0}}[1]$
is
![]() $(s,t)$
-semistable on
$(s,t)$
-semistable on
 $W_{E_{\unicode[STIX]{x1D701}_{0}},E_{\unicode[STIX]{x1D70F}^{\prime }}}$
. The center of
$W_{E_{\unicode[STIX]{x1D701}_{0}},E_{\unicode[STIX]{x1D70F}^{\prime }}}$
. The center of
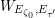 $W_{E_{\unicode[STIX]{x1D701}_{0}},E_{\unicode[STIX]{x1D70F}^{\prime }}}$
is
$W_{E_{\unicode[STIX]{x1D701}_{0}},E_{\unicode[STIX]{x1D70F}^{\prime }}}$
is
 $(\unicode[STIX]{x1D70E}^{\prime }+1,0)$
. Since
$(\unicode[STIX]{x1D70E}^{\prime }+1,0)$
. Since
 $\unicode[STIX]{x1D701}_{0}<\unicode[STIX]{x1D70E}^{\prime }+1<\unicode[STIX]{x1D702}-1$
,
$\unicode[STIX]{x1D701}_{0}<\unicode[STIX]{x1D70E}^{\prime }+1<\unicode[STIX]{x1D702}-1$
,
 $W_{E_{\unicode[STIX]{x1D701}_{0}},E_{\unicode[STIX]{x1D70F}^{\prime }}}$
lies inside
$W_{E_{\unicode[STIX]{x1D701}_{0}},E_{\unicode[STIX]{x1D70F}^{\prime }}}$
lies inside
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
.
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
.
Case (ii)
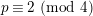 $p\equiv 2~(\text{mod}~4)$
. In this case,
$p\equiv 2~(\text{mod}~4)$
. In this case,
![]() $\unicode[STIX]{x1D702}$
can be an integer. So we consider two cases.
$\unicode[STIX]{x1D702}$
can be an integer. So we consider two cases.
Case (ii-a)
![]() $\unicode[STIX]{x1D702}$
is not an integer. In this case the semicircle
$\unicode[STIX]{x1D702}$
is not an integer. In this case the semicircle
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
has a positive radius with center
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
has a positive radius with center
 $(\unicode[STIX]{x1D702}-1,0)$
. We have
$(\unicode[STIX]{x1D702}-1,0)$
. We have
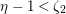 $\unicode[STIX]{x1D702}-1<\unicode[STIX]{x1D701}_{2}$
. For
$\unicode[STIX]{x1D702}-1<\unicode[STIX]{x1D701}_{2}$
. For
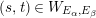 $(s,t)\in W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
, the exact sequence
$(s,t)\in W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
, the exact sequence
 $$\begin{eqnarray}0\rightarrow E_{\unicode[STIX]{x1D701}_{2}}\rightarrow E_{\unicode[STIX]{x1D6FC}}^{\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}})}\rightarrow E_{\unicode[STIX]{x1D6FD}}\rightarrow 0\end{eqnarray}$$
$$\begin{eqnarray}0\rightarrow E_{\unicode[STIX]{x1D701}_{2}}\rightarrow E_{\unicode[STIX]{x1D6FC}}^{\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}})}\rightarrow E_{\unicode[STIX]{x1D6FD}}\rightarrow 0\end{eqnarray}$$
in Lemma 4.7 is an exact sequence in
![]() ${\mathcal{A}}_{s}$
. In order to show the
${\mathcal{A}}_{s}$
. In order to show the
![]() $(s,t)$
-semistability of
$(s,t)$
-semistability of
![]() $E_{\unicode[STIX]{x1D6FD}}$
, we show
$E_{\unicode[STIX]{x1D6FD}}$
, we show
![]() $E_{\unicode[STIX]{x1D701}_{2}}$
and
$E_{\unicode[STIX]{x1D701}_{2}}$
and
![]() $E_{\unicode[STIX]{x1D6FC}}$
are
$E_{\unicode[STIX]{x1D6FC}}$
are
![]() $(s,t)$
-semistable on
$(s,t)$
-semistable on
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
.
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
.
If
![]() $\unicode[STIX]{x1D6FC}$
is a half integer, then this follows from Lemma 4.2. Otherwise,
$\unicode[STIX]{x1D6FC}$
is a half integer, then this follows from Lemma 4.2. Otherwise,
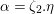 $\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D701}_{2}.\unicode[STIX]{x1D702}$
, and
$\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D701}_{2}.\unicode[STIX]{x1D702}$
, and
![]() $E_{\unicode[STIX]{x1D6FC}}$
is
$E_{\unicode[STIX]{x1D6FC}}$
is
![]() $(s,t)$
-semistable on
$(s,t)$
-semistable on
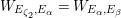 $W_{E_{\unicode[STIX]{x1D701}_{2}},E_{\unicode[STIX]{x1D6FC}}}=W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
by the induction hypothesis.
$W_{E_{\unicode[STIX]{x1D701}_{2}},E_{\unicode[STIX]{x1D6FC}}}=W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
by the induction hypothesis.
If
![]() $\unicode[STIX]{x1D701}_{2}$
is an integer or a half integer, then
$\unicode[STIX]{x1D701}_{2}$
is an integer or a half integer, then
![]() $E_{\unicode[STIX]{x1D701}_{2}}$
is
$E_{\unicode[STIX]{x1D701}_{2}}$
is
![]() $(s,t)$
-semistable on
$(s,t)$
-semistable on
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
by Lemma 4.2. Otherwise, we have
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
by Lemma 4.2. Otherwise, we have
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D70E}:=\unicode[STIX]{x1D716}\left(\frac{p^{\prime }}{2^{q^{\prime }}}\right)\!,\qquad \unicode[STIX]{x1D701}_{2}=\left(\frac{p^{\prime }+1}{2^{q^{\prime }}}\right)\!,\qquad \unicode[STIX]{x1D70F}:=\unicode[STIX]{x1D716}\left(\frac{p^{\prime }+2}{2^{q^{\prime }}}\right)\!, & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D70E}:=\unicode[STIX]{x1D716}\left(\frac{p^{\prime }}{2^{q^{\prime }}}\right)\!,\qquad \unicode[STIX]{x1D701}_{2}=\left(\frac{p^{\prime }+1}{2^{q^{\prime }}}\right)\!,\qquad \unicode[STIX]{x1D70F}:=\unicode[STIX]{x1D716}\left(\frac{p^{\prime }+2}{2^{q^{\prime }}}\right)\!, & & \displaystyle \nonumber\end{eqnarray}$$
where
![]() $p^{\prime }$
is even and
$p^{\prime }$
is even and
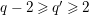 $q-2\geqslant q^{\prime }\geqslant 2$
. Then
$q-2\geqslant q^{\prime }\geqslant 2$
. Then
![]() $E_{\unicode[STIX]{x1D701}_{2}}$
is
$E_{\unicode[STIX]{x1D701}_{2}}$
is
![]() $(s,t)$
-semistable on
$(s,t)$
-semistable on
 $W_{E_{\unicode[STIX]{x1D70E}},E_{\unicode[STIX]{x1D701}_{2}}}$
by the induction hypothesis. The center of
$W_{E_{\unicode[STIX]{x1D70E}},E_{\unicode[STIX]{x1D701}_{2}}}$
by the induction hypothesis. The center of
 $W_{E_{\unicode[STIX]{x1D70E}},E_{\unicode[STIX]{x1D701}_{2}}}$
is
$W_{E_{\unicode[STIX]{x1D70E}},E_{\unicode[STIX]{x1D701}_{2}}}$
is
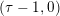 $(\unicode[STIX]{x1D70F}-1,0)$
. Since
$(\unicode[STIX]{x1D70F}-1,0)$
. Since
![]() $\unicode[STIX]{x1D702}\leqslant \unicode[STIX]{x1D70F}$
,
$\unicode[STIX]{x1D702}\leqslant \unicode[STIX]{x1D70F}$
,
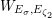 $W_{E_{\unicode[STIX]{x1D70E}},E_{\unicode[STIX]{x1D701}_{2}}}$
lies inside
$W_{E_{\unicode[STIX]{x1D70E}},E_{\unicode[STIX]{x1D701}_{2}}}$
lies inside
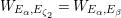 $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D701}_{2}}}=W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
.
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D701}_{2}}}=W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
.
Case (ii-b)
![]() $\unicode[STIX]{x1D702}$
is an integer. In this case
$\unicode[STIX]{x1D702}$
is an integer. In this case
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
has radius
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}$
has radius
![]() $0$
. We need to show that
$0$
. We need to show that
![]() $E_{\unicode[STIX]{x1D6FD}}$
is
$E_{\unicode[STIX]{x1D6FD}}$
is
![]() $(s,t)$
-semistable for any
$(s,t)$
-semistable for any
![]() $s<\unicode[STIX]{x1D6FD}$
. Suppose that
$s<\unicode[STIX]{x1D6FD}$
. Suppose that
![]() $E_{\unicode[STIX]{x1D6FD}}$
is not
$E_{\unicode[STIX]{x1D6FD}}$
is not
![]() $(s,t)$
-semistable for some
$(s,t)$
-semistable for some
![]() $(s,t)$
with
$(s,t)$
with
![]() $s<\unicode[STIX]{x1D6FD}$
. Then using the argument in the proof of Lemma 4.5, we can see that
$s<\unicode[STIX]{x1D6FD}$
. Then using the argument in the proof of Lemma 4.5, we can see that
![]() $E_{\unicode[STIX]{x1D6FD}}$
is not
$E_{\unicode[STIX]{x1D6FD}}$
is not
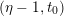 $(\unicode[STIX]{x1D702}-1,t_{0})$
-semistable for some
$(\unicode[STIX]{x1D702}-1,t_{0})$
-semistable for some
![]() $t_{0}>0$
. Consider a destabilizing subobject
$t_{0}>0$
. Consider a destabilizing subobject
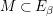 $M\subset E_{\unicode[STIX]{x1D6FD}}$
in
$M\subset E_{\unicode[STIX]{x1D6FD}}$
in
![]() ${\mathcal{A}}_{\unicode[STIX]{x1D702}-1}$
, that is,
${\mathcal{A}}_{\unicode[STIX]{x1D702}-1}$
, that is,
 $\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t_{0}}(M)>\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t_{0}}(E_{\unicode[STIX]{x1D6FD}})$
. Using the semistability of
$\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t_{0}}(M)>\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t_{0}}(E_{\unicode[STIX]{x1D6FD}})$
. Using the semistability of
![]() $E_{\unicode[STIX]{x1D6FD}}$
, we can see that
$E_{\unicode[STIX]{x1D6FD}}$
, we can see that
 $\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(M)<\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D6FD}})$
for
$\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(M)<\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D6FD}})$
for
![]() $t^{\prime }\gg 0$
. Hence the point
$t^{\prime }\gg 0$
. Hence the point
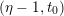 $(\unicode[STIX]{x1D702}-1,t_{0})$
lies inside the wall
$(\unicode[STIX]{x1D702}-1,t_{0})$
lies inside the wall
![]() $W_{E_{\unicode[STIX]{x1D6FD}},M}$
. It follows from this that
$W_{E_{\unicode[STIX]{x1D6FD}},M}$
. It follows from this that
 $$\begin{eqnarray}\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(M)>\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D6FD}})\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(M)>\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D6FD}})\end{eqnarray}$$
for any
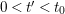 $0<t^{\prime }<t_{0}$
. Moreover, in the limit
$0<t^{\prime }<t_{0}$
. Moreover, in the limit
![]() $t^{\prime }\rightarrow 0$
, we have
$t^{\prime }\rightarrow 0$
, we have
 $$\begin{eqnarray}\lim _{t^{\prime }\rightarrow 0}t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(M)>\lim _{t^{\prime }\rightarrow 0}t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D6FD}}).\end{eqnarray}$$
$$\begin{eqnarray}\lim _{t^{\prime }\rightarrow 0}t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(M)>\lim _{t^{\prime }\rightarrow 0}t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D6FD}}).\end{eqnarray}$$
In order to obtain a contradiction, we derive the opposite inequality
 $$\begin{eqnarray}\lim _{t^{\prime }\rightarrow 0}t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(M)\leqslant \lim _{t^{\prime }\rightarrow 0}t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D6FD}}).\end{eqnarray}$$
$$\begin{eqnarray}\lim _{t^{\prime }\rightarrow 0}t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(M)\leqslant \lim _{t^{\prime }\rightarrow 0}t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D6FD}}).\end{eqnarray}$$
We first consider the case
![]() $q\geqslant 3$
. In this case, we have
$q\geqslant 3$
. In this case, we have
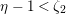 $\unicode[STIX]{x1D702}-1<\unicode[STIX]{x1D701}_{2}$
. So the sequence (4.7) is an exact sequence in
$\unicode[STIX]{x1D702}-1<\unicode[STIX]{x1D701}_{2}$
. So the sequence (4.7) is an exact sequence in
![]() ${\mathcal{A}}_{\unicode[STIX]{x1D702}-1}$
. By the induction hypothesis,
${\mathcal{A}}_{\unicode[STIX]{x1D702}-1}$
. By the induction hypothesis,
![]() $E_{\unicode[STIX]{x1D701}_{2}}$
and
$E_{\unicode[STIX]{x1D701}_{2}}$
and
![]() $E_{\unicode[STIX]{x1D6FC}}$
are
$E_{\unicode[STIX]{x1D6FC}}$
are
 $(\unicode[STIX]{x1D702}-1,t^{\prime })$
-semistable for any
$(\unicode[STIX]{x1D702}-1,t^{\prime })$
-semistable for any
![]() $t^{\prime }>0$
. Thus we have
$t^{\prime }>0$
. Thus we have
 $$\begin{eqnarray}\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(M)\leqslant \max \left\{\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D701}_{2}}),\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D6FC}})\right\}\!.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(M)\leqslant \max \left\{\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D701}_{2}}),\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D6FC}})\right\}\!.\end{eqnarray}$$
Taking the limit, we obtain
 $$\begin{eqnarray}\displaystyle \lim _{t^{\prime }\rightarrow 0}t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(M) & {\leqslant} & \displaystyle \lim _{t^{\prime }\rightarrow 0}\max \left\{t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D701}_{2}}),t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D6FC}})\right\}\nonumber\\ \displaystyle & = & \displaystyle \lim _{t^{\prime }\rightarrow 0}t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D6FD}}),\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \lim _{t^{\prime }\rightarrow 0}t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(M) & {\leqslant} & \displaystyle \lim _{t^{\prime }\rightarrow 0}\max \left\{t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D701}_{2}}),t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D6FC}})\right\}\nonumber\\ \displaystyle & = & \displaystyle \lim _{t^{\prime }\rightarrow 0}t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D6FD}}),\nonumber\end{eqnarray}$$
where the last equality holds because
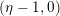 $(\unicode[STIX]{x1D702}-1,0)$
is the center of the wall
$(\unicode[STIX]{x1D702}-1,0)$
is the center of the wall
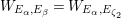 $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}=W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D701}_{2}}}$
(of radius zero).
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}}}=W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D701}_{2}}}$
(of radius zero).
Finally we consider the case
![]() $q=2$
. In this case, we have
$q=2$
. In this case, we have
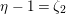 $\unicode[STIX]{x1D702}-1=\unicode[STIX]{x1D701}_{2}$
and
$\unicode[STIX]{x1D702}-1=\unicode[STIX]{x1D701}_{2}$
and
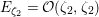 $E_{\unicode[STIX]{x1D701}_{2}}={\mathcal{O}}(\unicode[STIX]{x1D701}_{2},\unicode[STIX]{x1D701}_{2})$
. So the shift of (4.7)
$E_{\unicode[STIX]{x1D701}_{2}}={\mathcal{O}}(\unicode[STIX]{x1D701}_{2},\unicode[STIX]{x1D701}_{2})$
. So the shift of (4.7)
 $$\begin{eqnarray}0\rightarrow E_{\unicode[STIX]{x1D6FC}}^{\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}})}\rightarrow E_{\unicode[STIX]{x1D6FD}}\xrightarrow[{}]{f}E_{\unicode[STIX]{x1D701}_{2}}[1]\rightarrow 0\end{eqnarray}$$
$$\begin{eqnarray}0\rightarrow E_{\unicode[STIX]{x1D6FC}}^{\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FD}})}\rightarrow E_{\unicode[STIX]{x1D6FD}}\xrightarrow[{}]{f}E_{\unicode[STIX]{x1D701}_{2}}[1]\rightarrow 0\end{eqnarray}$$
is an exact sequence in
![]() ${\mathcal{A}}_{\unicode[STIX]{x1D702}-1}$
. By Lemma 4.8, the subobjects of
${\mathcal{A}}_{\unicode[STIX]{x1D702}-1}$
. By Lemma 4.8, the subobjects of
![]() $E_{\unicode[STIX]{x1D701}_{2}}[1]$
in
$E_{\unicode[STIX]{x1D701}_{2}}[1]$
in
![]() ${\mathcal{A}}_{\unicode[STIX]{x1D702}-1}$
are
${\mathcal{A}}_{\unicode[STIX]{x1D702}-1}$
are
![]() $0$
and
$0$
and
![]() $E_{\unicode[STIX]{x1D701}_{2}}[1]$
. If
$E_{\unicode[STIX]{x1D701}_{2}}[1]$
. If
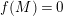 $f(M)=0$
, then
$f(M)=0$
, then
 $\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(M)\leqslant \unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D6FC}})$
. By taking the limit, we obtain (4.8). If
$\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(M)\leqslant \unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D6FC}})$
. By taking the limit, we obtain (4.8). If
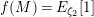 $f(M)=E_{\unicode[STIX]{x1D701}_{2}}[1]$
, then
$f(M)=E_{\unicode[STIX]{x1D701}_{2}}[1]$
, then
 $$\begin{eqnarray}Z_{(\unicode[STIX]{x1D702}-1,t^{\prime })}(M)=Z_{(\unicode[STIX]{x1D702}-1,t^{\prime })}(E_{\unicode[STIX]{x1D701}_{2}}[1])+Z_{(\unicode[STIX]{x1D702}-1,t^{\prime })}(\text{Ker}(f|_{M})).\end{eqnarray}$$
$$\begin{eqnarray}Z_{(\unicode[STIX]{x1D702}-1,t^{\prime })}(M)=Z_{(\unicode[STIX]{x1D702}-1,t^{\prime })}(E_{\unicode[STIX]{x1D701}_{2}}[1])+Z_{(\unicode[STIX]{x1D702}-1,t^{\prime })}(\text{Ker}(f|_{M})).\end{eqnarray}$$
By an easy calculation, we see that
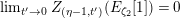 $\lim _{t^{\prime }\rightarrow 0}Z_{(\unicode[STIX]{x1D702}-1,t^{\prime })}(E_{\unicode[STIX]{x1D701}_{2}}[1])=0$
. So
$\lim _{t^{\prime }\rightarrow 0}Z_{(\unicode[STIX]{x1D702}-1,t^{\prime })}(E_{\unicode[STIX]{x1D701}_{2}}[1])=0$
. So
 $$\begin{eqnarray}\displaystyle \lim _{t^{\prime }\rightarrow 0}t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(M) & = & \displaystyle \lim _{t^{\prime }\rightarrow 0}t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(\text{Ker}(f|_{M}))\nonumber\\ \displaystyle & {\leqslant} & \displaystyle \lim _{t^{\prime }\rightarrow 0}t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D6FC}})=\lim _{t^{\prime }\rightarrow 0}t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D6FD}}).\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \lim _{t^{\prime }\rightarrow 0}t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(M) & = & \displaystyle \lim _{t^{\prime }\rightarrow 0}t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(\text{Ker}(f|_{M}))\nonumber\\ \displaystyle & {\leqslant} & \displaystyle \lim _{t^{\prime }\rightarrow 0}t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D6FC}})=\lim _{t^{\prime }\rightarrow 0}t^{\prime }\unicode[STIX]{x1D707}_{\unicode[STIX]{x1D702}-1,t^{\prime }}(E_{\unicode[STIX]{x1D6FD}}).\nonumber\end{eqnarray}$$
This is the end of the proof of Theorem 4.4. ◻
Lemma 4.8. Let
![]() $a$
be an integer and
$a$
be an integer and
![]() $V$
a finite-dimensional
$V$
a finite-dimensional
![]() $\mathbb{C}$
-vector space. The subobjects of
$\mathbb{C}$
-vector space. The subobjects of
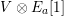 $V\otimes E_{a}[1]$
in
$V\otimes E_{a}[1]$
in
![]() ${\mathcal{A}}_{a}$
are
${\mathcal{A}}_{a}$
are
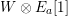 $W\otimes E_{a}[1]$
for subspaces
$W\otimes E_{a}[1]$
for subspaces
![]() $W\subset V$
.
$W\subset V$
.
To prove this lemma, we use the following lemma whose proof is left to the reader.
Lemma 4.9. Let
![]() $Y$
be a projective variety of dimension
$Y$
be a projective variety of dimension
![]() $d$
. If
$d$
. If
![]() $F$
is a
$F$
is a
![]() $\unicode[STIX]{x1D707}$
-semistable sheaf with respect to an ample line bundle
$\unicode[STIX]{x1D707}$
-semistable sheaf with respect to an ample line bundle
![]() $H$
such that
$H$
such that
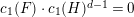 $c_{1}(F)\cdot c_{1}(H)^{d-1}=0$
, then
$c_{1}(F)\cdot c_{1}(H)^{d-1}=0$
, then
 $h^{0}(Y,F)\leqslant \text{r}(F)$
, where equality holds if and only if
$h^{0}(Y,F)\leqslant \text{r}(F)$
, where equality holds if and only if
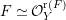 $F\simeq {\mathcal{O}}_{Y}^{\text{r}(F)}$
.
$F\simeq {\mathcal{O}}_{Y}^{\text{r}(F)}$
.
Proof of Lemma 4.8.
We may assume that
![]() $a=0$
. Then
$a=0$
. Then
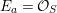 $E_{a}={\mathcal{O}}_{S}$
. Let
$E_{a}={\mathcal{O}}_{S}$
. Let
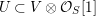 $U\subset V\otimes {\mathcal{O}}_{S}[1]$
be a subobject in
$U\subset V\otimes {\mathcal{O}}_{S}[1]$
be a subobject in
![]() ${\mathcal{A}}_{0}$
. Then we have an exact sequence
${\mathcal{A}}_{0}$
. Then we have an exact sequence
 $$\begin{eqnarray}0\rightarrow \text{H}^{-1}(U)\rightarrow V\otimes {\mathcal{O}}_{S}\xrightarrow[{}]{g}F\rightarrow \text{H}^{0}(U)\rightarrow 0\end{eqnarray}$$
$$\begin{eqnarray}0\rightarrow \text{H}^{-1}(U)\rightarrow V\otimes {\mathcal{O}}_{S}\xrightarrow[{}]{g}F\rightarrow \text{H}^{0}(U)\rightarrow 0\end{eqnarray}$$
of sheaves with
![]() $F\in {\mathcal{F}}_{0}$
. Since
$F\in {\mathcal{F}}_{0}$
. Since
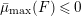 $\bar{\unicode[STIX]{x1D707}}_{\max }(F)\leqslant 0$
, we have
$\bar{\unicode[STIX]{x1D707}}_{\max }(F)\leqslant 0$
, we have
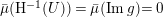 $\bar{\unicode[STIX]{x1D707}}(\text{H}^{-1}(U))=\bar{\unicode[STIX]{x1D707}}(\text{Im}\,g)=0$
, and
$\bar{\unicode[STIX]{x1D707}}(\text{H}^{-1}(U))=\bar{\unicode[STIX]{x1D707}}(\text{Im}\,g)=0$
, and
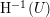 $\text{H}^{-1}(U)$
and
$\text{H}^{-1}(U)$
and
![]() $\text{Im}\,g$
are
$\text{Im}\,g$
are
![]() $\unicode[STIX]{x1D707}$
-semistable sheaves. By Lemma 4.9,
$\unicode[STIX]{x1D707}$
-semistable sheaves. By Lemma 4.9,
![]() $\text{Im}\,g$
is a trivial vector bundle. Now it is easy to show that
$\text{Im}\,g$
is a trivial vector bundle. Now it is easy to show that
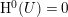 $\text{H}^{0}(U)=0$
and
$\text{H}^{0}(U)=0$
and
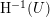 $\text{H}^{-1}(U)$
is
$\text{H}^{-1}(U)$
is
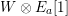 $W\otimes E_{a}[1]$
for a subspace
$W\otimes E_{a}[1]$
for a subspace
![]() $W\subset V$
.◻
$W\subset V$
.◻
5 Height-zero moduli spaces
Fix
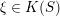 $\unicode[STIX]{x1D709}\in K(S)$
such that
$\unicode[STIX]{x1D709}\in K(S)$
such that
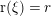 $\text{r}(\unicode[STIX]{x1D709})=r$
,
$\text{r}(\unicode[STIX]{x1D709})=r$
,
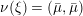 $\unicode[STIX]{x1D708}(\unicode[STIX]{x1D709})=(\bar{\unicode[STIX]{x1D707}},\bar{\unicode[STIX]{x1D707}})$
and
$\unicode[STIX]{x1D708}(\unicode[STIX]{x1D709})=(\bar{\unicode[STIX]{x1D707}},\bar{\unicode[STIX]{x1D707}})$
and
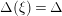 $\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})=\unicode[STIX]{x1D6E5}$
.
$\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})=\unicode[STIX]{x1D6E5}$
.
Throughout this section, we assume that the height of the moduli space
![]() $M(\unicode[STIX]{x1D709})$
is zero. We show that
$M(\unicode[STIX]{x1D709})$
is zero. We show that
![]() $M(\unicode[STIX]{x1D709})$
is isomorphic to a moduli space of representations of a quiver.
$M(\unicode[STIX]{x1D709})$
is isomorphic to a moduli space of representations of a quiver.
There is a unique
![]() $\unicode[STIX]{x1D6FE}\in \mathfrak{E}$
with
$\unicode[STIX]{x1D6FE}\in \mathfrak{E}$
with
![]() $\bar{\unicode[STIX]{x1D707}}\in I_{\unicode[STIX]{x1D6FE}}$
. We have either (i)
$\bar{\unicode[STIX]{x1D707}}\in I_{\unicode[STIX]{x1D6FE}}$
. We have either (i)
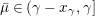 $\bar{\unicode[STIX]{x1D707}}\in (\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}},\unicode[STIX]{x1D6FE}]$
, or (ii)
$\bar{\unicode[STIX]{x1D707}}\in (\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}},\unicode[STIX]{x1D6FE}]$
, or (ii)
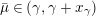 $\bar{\unicode[STIX]{x1D707}}\in (\unicode[STIX]{x1D6FE},\unicode[STIX]{x1D6FE}+x_{\unicode[STIX]{x1D6FE}})$
. We only consider the case (i). If we are in the case (ii), then by taking a dual of sheaves we will be in the case (i). (Note that in the case (ii), any
$\bar{\unicode[STIX]{x1D707}}\in (\unicode[STIX]{x1D6FE},\unicode[STIX]{x1D6FE}+x_{\unicode[STIX]{x1D6FE}})$
. We only consider the case (i). If we are in the case (ii), then by taking a dual of sheaves we will be in the case (i). (Note that in the case (ii), any
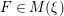 $F\in M(\unicode[STIX]{x1D709})$
is locally free by Remark 3.3.) So in the rest of this section, we assume that
$F\in M(\unicode[STIX]{x1D709})$
is locally free by Remark 3.3.) So in the rest of this section, we assume that
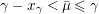 $\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}}<\bar{\unicode[STIX]{x1D707}}\leqslant \unicode[STIX]{x1D6FE}$
.
$\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}}<\bar{\unicode[STIX]{x1D707}}\leqslant \unicode[STIX]{x1D6FE}$
.
5.1 Bridgeland semistability of semistable sheaves
In this section, we study for which
![]() $(s,t)$
a sheaf
$(s,t)$
a sheaf
 $F\in M(\unicode[STIX]{x1D709})$
is
$F\in M(\unicode[STIX]{x1D709})$
is
![]() $(s,t)$
-semistable.
$(s,t)$
-semistable.
Express
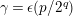 $\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D716}(p/2^{q})$
where
$\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D716}(p/2^{q})$
where
![]() $p$
is odd if
$p$
is odd if
![]() $q\geqslant 1$
. If
$q\geqslant 1$
. If
![]() $q\geqslant 1$
, then we set
$q\geqslant 1$
, then we set
 $$\begin{eqnarray}\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D716}\left(\frac{p-1}{2^{q}}\right)\qquad \text{and}\qquad \unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D716}\left(\frac{p+1}{2^{q}}\right)\!.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D716}\left(\frac{p-1}{2^{q}}\right)\qquad \text{and}\qquad \unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D716}\left(\frac{p+1}{2^{q}}\right)\!.\end{eqnarray}$$
If
![]() $q=0$
, then we set
$q=0$
, then we set
 $$\begin{eqnarray}\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D6FE}-\frac{1}{2}\qquad \text{and}\qquad \unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D6FE}+1.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D6FE}-\frac{1}{2}\qquad \text{and}\qquad \unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D6FE}+1.\end{eqnarray}$$
Then
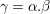 $\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}$
and
$\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}$
and
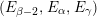 $(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FE}})$
is a symmetric exceptional triple. Put
$(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FE}})$
is a symmetric exceptional triple. Put
 $$\begin{eqnarray}\displaystyle E^{(1)} & = & \displaystyle E_{\unicode[STIX]{x1D6FD}-2},\qquad E^{(2)}=E_{\unicode[STIX]{x1D6FC}},\qquad E^{(3)}=E_{\unicode[STIX]{x1D6FE}},\nonumber\\ \displaystyle G^{(1)} & = & \displaystyle E_{\unicode[STIX]{x1D6FD}},\qquad G^{(2)}=E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},\qquad G^{(3)}=E_{\unicode[STIX]{x1D6FE}}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle E^{(1)} & = & \displaystyle E_{\unicode[STIX]{x1D6FD}-2},\qquad E^{(2)}=E_{\unicode[STIX]{x1D6FC}},\qquad E^{(3)}=E_{\unicode[STIX]{x1D6FE}},\nonumber\\ \displaystyle G^{(1)} & = & \displaystyle E_{\unicode[STIX]{x1D6FD}},\qquad G^{(2)}=E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},\qquad G^{(3)}=E_{\unicode[STIX]{x1D6FE}}.\nonumber\end{eqnarray}$$
Then for
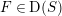 $F\in \text{D}(S)$
, there exists a spectral sequence
$F\in \text{D}(S)$
, there exists a spectral sequence
 $$\begin{eqnarray}E_{1}^{p,q}=\text{Ext}^{q}(G^{(p+3)},F)\hat{\otimes }E^{(p+3)}\end{eqnarray}$$
$$\begin{eqnarray}E_{1}^{p,q}=\text{Ext}^{q}(G^{(p+3)},F)\hat{\otimes }E^{(p+3)}\end{eqnarray}$$
converging to
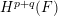 $H^{p+q}(F)$
, where
$H^{p+q}(F)$
, where
 $E_{1}^{p,q}=0$
unless
$E_{1}^{p,q}=0$
unless
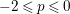 $-2\leqslant p\leqslant 0$
(see (A5) for this spectral sequence and the notation
$-2\leqslant p\leqslant 0$
(see (A5) for this spectral sequence and the notation
![]() $\hat{\otimes }$
).
$\hat{\otimes }$
).
We consider the semicircular walls
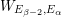 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
,
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
,
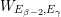 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FE}}}$
and
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FE}}}$
and
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FE}}}$
in the upper half
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FE}}}$
in the upper half
![]() $(s,t)$
-plane.
$(s,t)$
-plane.
The center of the walls
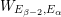 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
,
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
,
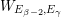 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FE}}}$
and
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FE}}}$
and
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FE}}}$
are
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FE}}}$
are
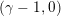 $(\unicode[STIX]{x1D6FE}-1,0)$
,
$(\unicode[STIX]{x1D6FE}-1,0)$
,
 $(\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}-1,0)$
and
$(\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}-1,0)$
and
 $(\unicode[STIX]{x1D6FD}-1,0)$
, respectively. When
$(\unicode[STIX]{x1D6FD}-1,0)$
, respectively. When
![]() $q=0$
, the walls
$q=0$
, the walls
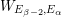 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
and
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
and
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FE}}}$
have radii
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FE}}}$
have radii
![]() $0$
, and
$0$
, and
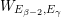 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FE}}}$
has positive radius. When
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FE}}}$
has positive radius. When
![]() $q=1$
, the wall
$q=1$
, the wall
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FE}}}$
has radius
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FE}}}$
has radius
![]() $0$
, the other two walls have positive radii; the wall
$0$
, the other two walls have positive radii; the wall
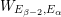 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
lies inside
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
lies inside
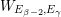 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FE}}}$
. When
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FE}}}$
. When
![]() $q\geqslant 2$
,
$q\geqslant 2$
,
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FE}}}$
lies inside
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FE}}}$
lies inside
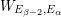 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
, and
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
, and
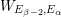 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
lies inside
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
lies inside
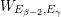 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FE}}}$
. (See Figures 1–3.)
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FE}}}$
. (See Figures 1–3.)
Figure 1. The case
![]() $q=0$
. The semicircle is
$q=0$
. The semicircle is
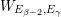 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FE}}}$
; the dot at
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FE}}}$
; the dot at
![]() $\unicode[STIX]{x1D6FD}-2$
is
$\unicode[STIX]{x1D6FD}-2$
is
 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
;the dot at
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
;the dot at
![]() $\unicode[STIX]{x1D6FE}$
is
$\unicode[STIX]{x1D6FE}$
is
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FE}}}$
.
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FE}}}$
.
Figure 2. The case
![]() $q=1$
. The outer semicircle is
$q=1$
. The outer semicircle is
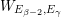 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FE}}}$
; the inner semicircle is
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FE}}}$
; the inner semicircle is
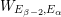 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
; the dot at
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
; the dot at
![]() $\unicode[STIX]{x1D6FC}$
is
$\unicode[STIX]{x1D6FC}$
is
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FE}}}$
.
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FE}}}$
.

Figure 3. The case
![]() $q\geqslant 2$
. The outermost semicircle is
$q\geqslant 2$
. The outermost semicircle is
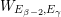 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FE}}}$
; the innermost semicircle is
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FE}}}$
; the innermost semicircle is
![]() $W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FE}}}$
; the semicircle in between is
$W_{E_{\unicode[STIX]{x1D6FC}},E_{\unicode[STIX]{x1D6FE}}}$
; the semicircle in between is
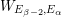 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
.
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
.
Lemma 5.1. If a point
![]() $(s_{0},t_{0})$
with
$(s_{0},t_{0})$
with
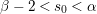 $\unicode[STIX]{x1D6FD}-2<s_{0}<\unicode[STIX]{x1D6FC}$
in the upper half
$\unicode[STIX]{x1D6FD}-2<s_{0}<\unicode[STIX]{x1D6FC}$
in the upper half
![]() $(s,t)$
-plane lies on or outside the wall
$(s,t)$
-plane lies on or outside the wall
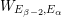 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
, then
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
, then
![]() $E_{\unicode[STIX]{x1D6FC}}$
,
$E_{\unicode[STIX]{x1D6FC}}$
,
![]() $E_{\unicode[STIX]{x1D6FE}}$
and
$E_{\unicode[STIX]{x1D6FE}}$
and
![]() $E_{\unicode[STIX]{x1D6FD}-2}[1]$
are
$E_{\unicode[STIX]{x1D6FD}-2}[1]$
are
![]() $(s_{0},t_{0})$
-semistable.
$(s_{0},t_{0})$
-semistable.
Proof. Since the proof is similar, we prove the lemma only for
![]() $E_{\unicode[STIX]{x1D6FD}-2}[1]$
. If
$E_{\unicode[STIX]{x1D6FD}-2}[1]$
. If
![]() $\unicode[STIX]{x1D6FD}$
is an integer or a half integer, this is clear by Lemma 4.2. Otherwise, we can write
$\unicode[STIX]{x1D6FD}$
is an integer or a half integer, this is clear by Lemma 4.2. Otherwise, we can write
![]() $\unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D70E}.\unicode[STIX]{x1D70F}$
, where
$\unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D70E}.\unicode[STIX]{x1D70F}$
, where
 $\unicode[STIX]{x1D70E},\,\unicode[STIX]{x1D70F}\in \mathfrak{E}$
with
$\unicode[STIX]{x1D70E},\,\unicode[STIX]{x1D70F}\in \mathfrak{E}$
with
![]() $\unicode[STIX]{x1D70E}\leqslant \unicode[STIX]{x1D6FC}$
. By Theorem 4.4,
$\unicode[STIX]{x1D70E}\leqslant \unicode[STIX]{x1D6FC}$
. By Theorem 4.4,
![]() $E_{\unicode[STIX]{x1D6FD}-2}[1]$
is
$E_{\unicode[STIX]{x1D6FD}-2}[1]$
is
![]() $(s,t)$
-semistable outside the wall
$(s,t)$
-semistable outside the wall
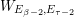 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D70F}-2}}$
whose center is
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D70F}-2}}$
whose center is
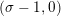 $(\unicode[STIX]{x1D70E}-1,0)$
. Since the center of
$(\unicode[STIX]{x1D70E}-1,0)$
. Since the center of
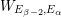 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
is
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
is
 $(\unicode[STIX]{x1D6FE}-1,0)$
, which is to the right of
$(\unicode[STIX]{x1D6FE}-1,0)$
, which is to the right of
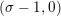 $(\unicode[STIX]{x1D70E}-1,0)$
,
$(\unicode[STIX]{x1D70E}-1,0)$
,
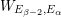 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
lies outside
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
lies outside
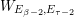 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D70F}-2}}$
.◻
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D70F}-2}}$
.◻
Proposition 5.2. A semistable (resp. stable) sheaf
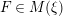 $F\in M(\unicode[STIX]{x1D709})$
is
$F\in M(\unicode[STIX]{x1D709})$
is
![]() $(s_{0},t_{0})$
-semistable (resp.
$(s_{0},t_{0})$
-semistable (resp.
![]() $(s_{0},t_{0})$
-stable) if the point
$(s_{0},t_{0})$
-stable) if the point
![]() $(s_{0},t_{0})$
with
$(s_{0},t_{0})$
with
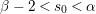 $\unicode[STIX]{x1D6FD}-2<s_{0}<\unicode[STIX]{x1D6FC}$
in the upper half
$\unicode[STIX]{x1D6FD}-2<s_{0}<\unicode[STIX]{x1D6FC}$
in the upper half
![]() $(s,t)$
-plane lies outside the wall
$(s,t)$
-plane lies outside the wall
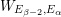 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
.
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
.
Proof. First we consider a semistable sheaf
![]() $F$
. Note that
$F$
. Note that
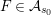 $F\in {\mathcal{A}}_{s_{0}}$
since
$F\in {\mathcal{A}}_{s_{0}}$
since
![]() $s_{0}<\bar{\unicode[STIX]{x1D707}}$
and
$s_{0}<\bar{\unicode[STIX]{x1D707}}$
and
![]() $F$
is semistable. Suppose that
$F$
is semistable. Suppose that
![]() $F$
is not
$F$
is not
![]() $(s_{0},t_{0})$
-semistable. Then there exists a subobject
$(s_{0},t_{0})$
-semistable. Then there exists a subobject
![]() $S\subset F$
in
$S\subset F$
in
![]() ${\mathcal{A}}_{s_{0}}$
such that
${\mathcal{A}}_{s_{0}}$
such that
 $$\begin{eqnarray}\unicode[STIX]{x1D707}_{s_{0},t_{0}}(S)>\unicode[STIX]{x1D707}_{s_{0},t_{0}}(F).\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D707}_{s_{0},t_{0}}(S)>\unicode[STIX]{x1D707}_{s_{0},t_{0}}(F).\end{eqnarray}$$
Then
![]() $S$
is a sheaf and there exists an exact sequence
$S$
is a sheaf and there exists an exact sequence
 $$\begin{eqnarray}0\rightarrow K\rightarrow S\rightarrow F\rightarrow Q\rightarrow 0\end{eqnarray}$$
$$\begin{eqnarray}0\rightarrow K\rightarrow S\rightarrow F\rightarrow Q\rightarrow 0\end{eqnarray}$$
of sheaves. We choose such an
![]() $S$
with minimal rank. Then for any
$S$
with minimal rank. Then for any
![]() $(s,t)$
on the wall
$(s,t)$
on the wall
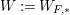 $W:=W_{F,\ast }$
passing through
$W:=W_{F,\ast }$
passing through
![]() $(s_{0},t_{0})$
,
$(s_{0},t_{0})$
,
![]() $S$
is a subobject of
$S$
is a subobject of
![]() $F$
and
$F$
and
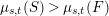 $\unicode[STIX]{x1D707}_{s,t}(S)>\unicode[STIX]{x1D707}_{s,t}(F)$
(cf. the argument of the proof of [Reference Arcara, Bertram, Coskun and HuizengaABCH, Lemma 6.3]).
$\unicode[STIX]{x1D707}_{s,t}(S)>\unicode[STIX]{x1D707}_{s,t}(F)$
(cf. the argument of the proof of [Reference Arcara, Bertram, Coskun and HuizengaABCH, Lemma 6.3]).
Claim.
The wall
![]() $W$
lies outside
$W$
lies outside
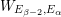 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
.
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
.
Proof of Claim.
Using the spectral sequence (5.1), we see that there exists a short exact sequence
 $$\begin{eqnarray}0\rightarrow E_{\unicode[STIX]{x1D6FD}-2}^{m}\rightarrow E_{\unicode[STIX]{x1D6FC}}^{n}\rightarrow F\rightarrow 0,\end{eqnarray}$$
$$\begin{eqnarray}0\rightarrow E_{\unicode[STIX]{x1D6FD}-2}^{m}\rightarrow E_{\unicode[STIX]{x1D6FC}}^{n}\rightarrow F\rightarrow 0,\end{eqnarray}$$
where
 $$\begin{eqnarray}m=-\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FD}},F)\qquad \text{and}\qquad n=-\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},F).\end{eqnarray}$$
$$\begin{eqnarray}m=-\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FD}},F)\qquad \text{and}\qquad n=-\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},F).\end{eqnarray}$$
So
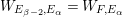 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}=W_{F,E_{\unicode[STIX]{x1D6FC}}}$
. Both
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}=W_{F,E_{\unicode[STIX]{x1D6FC}}}$
. Both
![]() $W$
and
$W$
and
![]() $W_{F,E_{\unicode[STIX]{x1D6FC}}}$
are walls of the form
$W_{F,E_{\unicode[STIX]{x1D6FC}}}$
are walls of the form
![]() $W_{F,\ast }$
, so they are disjoint. Since the point
$W_{F,\ast }$
, so they are disjoint. Since the point
![]() $(s_{0},t_{0})$
is outside
$(s_{0},t_{0})$
is outside
![]() $W_{F,E_{\unicode[STIX]{x1D6FC}}}$
,
$W_{F,E_{\unicode[STIX]{x1D6FC}}}$
,
![]() $W$
is outside
$W$
is outside
![]() $W_{F,E_{\unicode[STIX]{x1D6FC}}}$
. This is the end of the proof of the claim.◻
$W_{F,E_{\unicode[STIX]{x1D6FC}}}$
. This is the end of the proof of the claim.◻
Put
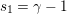 $s_{1}=\unicode[STIX]{x1D6FE}-1$
. Then
$s_{1}=\unicode[STIX]{x1D6FE}-1$
. Then
![]() $(s_{1},0)$
be the center of
$(s_{1},0)$
be the center of
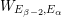 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
. Let
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
. Let
![]() $(s_{1},t_{1})$
and
$(s_{1},t_{1})$
and
![]() $(s_{1},t_{2})$
be the point on
$(s_{1},t_{2})$
be the point on
![]() $W$
and
$W$
and
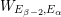 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
, respectively. (When
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
, respectively. (When
![]() $\unicode[STIX]{x1D6FE}$
is an integer, we understand that
$\unicode[STIX]{x1D6FE}$
is an integer, we understand that
![]() $t_{2}=0$
.) The exact sequence (5.3) and the semistability of
$t_{2}=0$
.) The exact sequence (5.3) and the semistability of
![]() $F$
imply either
$F$
imply either
 $$\begin{eqnarray}\bar{\unicode[STIX]{x1D707}}(S)<\bar{\unicode[STIX]{x1D707}}(F)\end{eqnarray}$$
$$\begin{eqnarray}\bar{\unicode[STIX]{x1D707}}(S)<\bar{\unicode[STIX]{x1D707}}(F)\end{eqnarray}$$
or
 $$\begin{eqnarray}K=0\quad \text{and}\qquad \bar{\unicode[STIX]{x1D707}}(S)=\bar{\unicode[STIX]{x1D707}}(F)\qquad \text{and}\qquad \frac{\text{ch}_{2}(S)}{\text{r}(S)}\leqslant \frac{\text{ch}_{2}(F)}{r}.\end{eqnarray}$$
$$\begin{eqnarray}K=0\quad \text{and}\qquad \bar{\unicode[STIX]{x1D707}}(S)=\bar{\unicode[STIX]{x1D707}}(F)\qquad \text{and}\qquad \frac{\text{ch}_{2}(S)}{\text{r}(S)}\leqslant \frac{\text{ch}_{2}(F)}{r}.\end{eqnarray}$$
If (5.7) holds, then
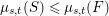 $\unicode[STIX]{x1D707}_{s,t}(S)\leqslant \unicode[STIX]{x1D707}_{s,t}(F)$
for any
$\unicode[STIX]{x1D707}_{s,t}(S)\leqslant \unicode[STIX]{x1D707}_{s,t}(F)$
for any
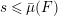 $s\leqslant \bar{\unicode[STIX]{x1D707}}(F)$
, this contradicts the inequality (5.2). If (5.6) holds, then
$s\leqslant \bar{\unicode[STIX]{x1D707}}(F)$
, this contradicts the inequality (5.2). If (5.6) holds, then
 $\unicode[STIX]{x1D707}_{s_{1},t}(S)<\unicode[STIX]{x1D707}_{s_{1},t}(F)$
for
$\unicode[STIX]{x1D707}_{s_{1},t}(S)<\unicode[STIX]{x1D707}_{s_{1},t}(F)$
for
![]() $t\gg 0$
, so there exists
$t\gg 0$
, so there exists
![]() $t_{3}>t_{1}$
such that
$t_{3}>t_{1}$
such that
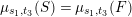 $\unicode[STIX]{x1D707}_{s_{1},t_{3}}(S)=\unicode[STIX]{x1D707}_{s_{1},t_{3}}(F)$
. This shows that
$\unicode[STIX]{x1D707}_{s_{1},t_{3}}(S)=\unicode[STIX]{x1D707}_{s_{1},t_{3}}(F)$
. This shows that
 $$\begin{eqnarray}\unicode[STIX]{x1D707}_{s_{1},t}(S)>\unicode[STIX]{x1D707}_{s_{1},t}(F)\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D707}_{s_{1},t}(S)>\unicode[STIX]{x1D707}_{s_{1},t}(F)\end{eqnarray}$$
for any
 $0<t<t_{3}$
. Moreover, we have
$0<t<t_{3}$
. Moreover, we have
 $$\begin{eqnarray}\lim _{t\rightarrow +0}t\unicode[STIX]{x1D707}_{s_{1},t}(S)>\lim _{t\rightarrow +0}t\unicode[STIX]{x1D707}_{s_{1},t}(F).\end{eqnarray}$$
$$\begin{eqnarray}\lim _{t\rightarrow +0}t\unicode[STIX]{x1D707}_{s_{1},t}(S)>\lim _{t\rightarrow +0}t\unicode[STIX]{x1D707}_{s_{1},t}(F).\end{eqnarray}$$
In order to obtain a contradiction, we show the opposite inequality.
Consider first the case when
![]() $\unicode[STIX]{x1D6FE}$
is not an integer. Then
$\unicode[STIX]{x1D6FE}$
is not an integer. Then
 $\unicode[STIX]{x1D6FD}-2<s_{1}<\unicode[STIX]{x1D6FC}$
and
$\unicode[STIX]{x1D6FD}-2<s_{1}<\unicode[STIX]{x1D6FC}$
and
![]() $t_{2}>0$
. By Lemma 5.1,
$t_{2}>0$
. By Lemma 5.1,
![]() $E_{\unicode[STIX]{x1D6FD}-2}[1]$
and
$E_{\unicode[STIX]{x1D6FD}-2}[1]$
and
![]() $E_{\unicode[STIX]{x1D6FC}}$
are
$E_{\unicode[STIX]{x1D6FC}}$
are
![]() $(s_{1},t_{2})$
-semistable and from (5.4) we obtain a short exact sequence
$(s_{1},t_{2})$
-semistable and from (5.4) we obtain a short exact sequence
 $$\begin{eqnarray}0\rightarrow E_{\unicode[STIX]{x1D6FC}}^{n}\rightarrow F\xrightarrow[{}]{f}E_{\unicode[STIX]{x1D6FD}-2}[1]^{m}\rightarrow 0\end{eqnarray}$$
$$\begin{eqnarray}0\rightarrow E_{\unicode[STIX]{x1D6FC}}^{n}\rightarrow F\xrightarrow[{}]{f}E_{\unicode[STIX]{x1D6FD}-2}[1]^{m}\rightarrow 0\end{eqnarray}$$
in
![]() ${\mathcal{A}}_{s_{1}}$
. So we have
${\mathcal{A}}_{s_{1}}$
. So we have
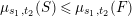 $\unicode[STIX]{x1D707}_{s_{1},t_{2}}(S)\leqslant \unicode[STIX]{x1D707}_{s_{1},t_{2}}(F)$
. This contradicts the inequality (5.8).
$\unicode[STIX]{x1D707}_{s_{1},t_{2}}(S)\leqslant \unicode[STIX]{x1D707}_{s_{1},t_{2}}(F)$
. This contradicts the inequality (5.8).
Finally consider the case when
![]() $\unicode[STIX]{x1D6FE}$
is an integer. In this case, we have
$\unicode[STIX]{x1D6FE}$
is an integer. In this case, we have
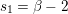 $s_{1}=\unicode[STIX]{x1D6FD}-2$
and
$s_{1}=\unicode[STIX]{x1D6FD}-2$
and
![]() $t_{2}=0$
. By Lemma 4.2,
$t_{2}=0$
. By Lemma 4.2,
![]() $E_{\unicode[STIX]{x1D6FD}-2}[1]$
and
$E_{\unicode[STIX]{x1D6FD}-2}[1]$
and
![]() $E_{\unicode[STIX]{x1D6FC}}$
are
$E_{\unicode[STIX]{x1D6FC}}$
are
![]() $(s_{1},t)$
-semistable for any
$(s_{1},t)$
-semistable for any
![]() $t>0$
, and (5.10) is a short exact sequence in
$t>0$
, and (5.10) is a short exact sequence in
![]() ${\mathcal{A}}_{s_{1}}$
. By Lemma 4.8, the subobject
${\mathcal{A}}_{s_{1}}$
. By Lemma 4.8, the subobject
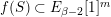 $f(S)\subset E_{\unicode[STIX]{x1D6FD}-2}[1]^{m}$
is isomorphic to
$f(S)\subset E_{\unicode[STIX]{x1D6FD}-2}[1]^{m}$
is isomorphic to
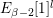 $E_{\unicode[STIX]{x1D6FD}-2}[1]^{l}$
for some
$E_{\unicode[STIX]{x1D6FD}-2}[1]^{l}$
for some
![]() $l\geqslant 0$
. We have
$l\geqslant 0$
. We have
 $$\begin{eqnarray}Z_{s_{1},t}(S)=lZ_{s_{1},t}(E_{\unicode[STIX]{x1D6FD}-2}[1])+Z(\text{Ker}\,(f|_{S}))\end{eqnarray}$$
$$\begin{eqnarray}Z_{s_{1},t}(S)=lZ_{s_{1},t}(E_{\unicode[STIX]{x1D6FD}-2}[1])+Z(\text{Ker}\,(f|_{S}))\end{eqnarray}$$
and
 $$\begin{eqnarray}Z_{s_{1},t}(E_{\unicode[STIX]{x1D6FD}-2}[1])=-t^{2}.\end{eqnarray}$$
$$\begin{eqnarray}Z_{s_{1},t}(E_{\unicode[STIX]{x1D6FD}-2}[1])=-t^{2}.\end{eqnarray}$$
This implies that
 $$\begin{eqnarray}\displaystyle \lim _{t\rightarrow +0}t\unicode[STIX]{x1D707}_{s_{1},t}(S) & = & \displaystyle \displaystyle \lim _{t\rightarrow +0}t\unicode[STIX]{x1D707}_{s_{1},t}(\text{Ker}\,(f|_{S}))\nonumber\\ \displaystyle & {\leqslant} & \displaystyle \displaystyle \lim _{t\rightarrow +0}t\unicode[STIX]{x1D707}_{s_{1},t}(E_{\unicode[STIX]{x1D6FC}})=\lim _{t\rightarrow +0}t\unicode[STIX]{x1D707}_{s_{1},t}(F).\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \lim _{t\rightarrow +0}t\unicode[STIX]{x1D707}_{s_{1},t}(S) & = & \displaystyle \displaystyle \lim _{t\rightarrow +0}t\unicode[STIX]{x1D707}_{s_{1},t}(\text{Ker}\,(f|_{S}))\nonumber\\ \displaystyle & {\leqslant} & \displaystyle \displaystyle \lim _{t\rightarrow +0}t\unicode[STIX]{x1D707}_{s_{1},t}(E_{\unicode[STIX]{x1D6FC}})=\lim _{t\rightarrow +0}t\unicode[STIX]{x1D707}_{s_{1},t}(F).\nonumber\end{eqnarray}$$
This contradicts (5.9).
Finally we have to show that if
![]() $F$
is stable, then it is
$F$
is stable, then it is
![]() $(s_{0},t_{0})$
-stable. But this can be proved by a similar argument. In fact, we already know that
$(s_{0},t_{0})$
-stable. But this can be proved by a similar argument. In fact, we already know that
![]() $F$
is
$F$
is
![]() $(s_{0},t_{0})$
-semistable. If
$(s_{0},t_{0})$
-semistable. If
![]() $F$
is not
$F$
is not
![]() $(s_{0},t_{0})$
-stable, then there exists a subobject
$(s_{0},t_{0})$
-stable, then there exists a subobject
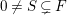 $0\neq S\subsetneq F$
in
$0\neq S\subsetneq F$
in
![]() ${\mathcal{A}}_{s_{0}}$
with
${\mathcal{A}}_{s_{0}}$
with
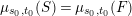 $\unicode[STIX]{x1D707}_{s_{0},t_{0}}(S)=\unicode[STIX]{x1D707}_{s_{0},t_{0}}(F)$
. Arguing in the semistable case, we obtain a contradiction.◻
$\unicode[STIX]{x1D707}_{s_{0},t_{0}}(S)=\unicode[STIX]{x1D707}_{s_{0},t_{0}}(F)$
. Arguing in the semistable case, we obtain a contradiction.◻
Conversely
![]() $(s,t)$
-semistability implies usual semistability:
$(s,t)$
-semistability implies usual semistability:
Proposition 5.3. Assume that the point
![]() $(s_{0},t_{0})$
with
$(s_{0},t_{0})$
with
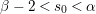 $\unicode[STIX]{x1D6FD}-2<s_{0}<\unicode[STIX]{x1D6FC}$
in the upper half
$\unicode[STIX]{x1D6FD}-2<s_{0}<\unicode[STIX]{x1D6FC}$
in the upper half
![]() $(s,t)$
-plane lies outside the wall
$(s,t)$
-plane lies outside the wall
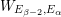 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
. Let
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
. Let
![]() $F^{\bullet }$
be an object in
$F^{\bullet }$
be an object in
![]() ${\mathcal{A}}_{s_{0}}$
with
${\mathcal{A}}_{s_{0}}$
with
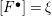 $[F^{\bullet }]=\unicode[STIX]{x1D709}$
in
$[F^{\bullet }]=\unicode[STIX]{x1D709}$
in
![]() $K(S)$
. Assume that
$K(S)$
. Assume that
![]() $F^{\bullet }$
is
$F^{\bullet }$
is
![]() $(s_{0},t_{0})$
-semistable (resp.
$(s_{0},t_{0})$
-semistable (resp.
![]() $(s_{0},t_{0})$
-stable). Then
$(s_{0},t_{0})$
-stable). Then
![]() $F^{\bullet }$
is a semistable (resp. stable) sheaf.
$F^{\bullet }$
is a semistable (resp. stable) sheaf.
Proof. We show the semistable case first.
Step 1. We show that
![]() $F^{\bullet }$
is quasi-isomorphic to a
$F^{\bullet }$
is quasi-isomorphic to a
![]() $2$
-term complex
$2$
-term complex
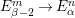 $E_{\unicode[STIX]{x1D6FD}-2}^{m}\rightarrow E_{\unicode[STIX]{x1D6FC}}^{n}$
, where
$E_{\unicode[STIX]{x1D6FD}-2}^{m}\rightarrow E_{\unicode[STIX]{x1D6FC}}^{n}$
, where
![]() $E_{\unicode[STIX]{x1D6FC}}^{n}$
and
$E_{\unicode[STIX]{x1D6FC}}^{n}$
and
![]() $E_{\unicode[STIX]{x1D6FD}-2}^{m}$
are in degree
$E_{\unicode[STIX]{x1D6FD}-2}^{m}$
are in degree
![]() $0$
and
$0$
and
![]() $-1$
, respectively, and
$-1$
, respectively, and
![]() $m$
,
$m$
,
![]() $n$
are those in (5.5). We use the spectral sequence (5.1). Since
$n$
are those in (5.5). We use the spectral sequence (5.1). Since
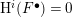 $\text{H}^{i}(F^{\bullet })=0$
except
$\text{H}^{i}(F^{\bullet })=0$
except
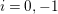 $i=0,-1$
, the term
$i=0,-1$
, the term
![]() $E_{1}^{p,q}$
in the spectral sequence is zero unless
$E_{1}^{p,q}$
in the spectral sequence is zero unless
 $-1\leqslant q\leqslant 2$
. If
$-1\leqslant q\leqslant 2$
. If
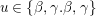 $u\in \{\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FE}\}$
, then
$u\in \{\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FE}\}$
, then
 $\text{Ext}^{2}(E_{u},F^{\bullet })=0$
because
$\text{Ext}^{2}(E_{u},F^{\bullet })=0$
because
 $$\begin{eqnarray}\text{Ext}^{2}(E_{u},\text{H}^{0}(F^{\bullet }))\simeq \text{Hom}(\text{H}^{0}(F^{\bullet }),E_{u-2})=0\end{eqnarray}$$
$$\begin{eqnarray}\text{Ext}^{2}(E_{u},\text{H}^{0}(F^{\bullet }))\simeq \text{Hom}(\text{H}^{0}(F^{\bullet }),E_{u-2})=0\end{eqnarray}$$
(note that
 $\bar{\unicode[STIX]{x1D707}}_{\min }(\text{H}^{0}(F^{\bullet })/tor)>s_{0}>u-2$
). We can also show that
$\bar{\unicode[STIX]{x1D707}}_{\min }(\text{H}^{0}(F^{\bullet })/tor)>s_{0}>u-2$
). We can also show that
 $\text{Ext}^{-1}(E_{u},F^{\bullet })=0$
by a similar argument.
$\text{Ext}^{-1}(E_{u},F^{\bullet })=0$
by a similar argument.
Claim.
We have
 $E_{1}^{0,0}=0$
.
$E_{1}^{0,0}=0$
.
Proof of Claim.
We show that
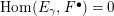 $\text{Hom}(E_{\unicode[STIX]{x1D6FE}},F^{\bullet })=0$
. By the
$\text{Hom}(E_{\unicode[STIX]{x1D6FE}},F^{\bullet })=0$
. By the
![]() $(s_{0},t_{0})$
-semistability of
$(s_{0},t_{0})$
-semistability of
![]() $F^{\bullet }$
, it suffices to show that
$F^{\bullet }$
, it suffices to show that
 $\unicode[STIX]{x1D707}_{s_{0},t_{0}}(E_{\unicode[STIX]{x1D6FE}})>\unicode[STIX]{x1D707}_{s_{0},t_{0}}(F^{\bullet })$
. Since
$\unicode[STIX]{x1D707}_{s_{0},t_{0}}(E_{\unicode[STIX]{x1D6FE}})>\unicode[STIX]{x1D707}_{s_{0},t_{0}}(F^{\bullet })$
. Since
 $$\begin{eqnarray}Z_{s_{0},t_{0}}(F^{\bullet })=nZ_{s_{0},t_{0}}(E_{\unicode[STIX]{x1D6FC}})+mZ_{s_{0},t_{0}}(E_{\unicode[STIX]{x1D6FD}-2}^{m}[1])\end{eqnarray}$$
$$\begin{eqnarray}Z_{s_{0},t_{0}}(F^{\bullet })=nZ_{s_{0},t_{0}}(E_{\unicode[STIX]{x1D6FC}})+mZ_{s_{0},t_{0}}(E_{\unicode[STIX]{x1D6FD}-2}^{m}[1])\end{eqnarray}$$
by the condition on the K-class of
![]() $F^{\bullet }$
, and
$F^{\bullet }$
, and
 $\unicode[STIX]{x1D707}_{s_{0},t_{0}}(E_{\unicode[STIX]{x1D6FD}-2}[1])>\unicode[STIX]{x1D707}_{s_{0},t_{0}}(E_{\unicode[STIX]{x1D6FC}})$
, we have
$\unicode[STIX]{x1D707}_{s_{0},t_{0}}(E_{\unicode[STIX]{x1D6FD}-2}[1])>\unicode[STIX]{x1D707}_{s_{0},t_{0}}(E_{\unicode[STIX]{x1D6FC}})$
, we have
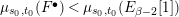 $\unicode[STIX]{x1D707}_{s_{0},t_{0}}(F^{\bullet })<\unicode[STIX]{x1D707}_{s_{0},t_{0}}(E_{\unicode[STIX]{x1D6FD}-2}[1])$
. If
$\unicode[STIX]{x1D707}_{s_{0},t_{0}}(F^{\bullet })<\unicode[STIX]{x1D707}_{s_{0},t_{0}}(E_{\unicode[STIX]{x1D6FD}-2}[1])$
. If
![]() $(s_{0},t_{0})$
lies inside the wall
$(s_{0},t_{0})$
lies inside the wall
 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FE}}}$
, then
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FE}}}$
, then
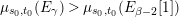 $\unicode[STIX]{x1D707}_{s_{0},t_{0}}(E_{\unicode[STIX]{x1D6FE}})>\unicode[STIX]{x1D707}_{s_{0},t_{0}}(E_{\unicode[STIX]{x1D6FD}-2}[1])$
, thus
$\unicode[STIX]{x1D707}_{s_{0},t_{0}}(E_{\unicode[STIX]{x1D6FE}})>\unicode[STIX]{x1D707}_{s_{0},t_{0}}(E_{\unicode[STIX]{x1D6FD}-2}[1])$
, thus
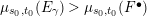 $\unicode[STIX]{x1D707}_{s_{0},t_{0}}(E_{\unicode[STIX]{x1D6FE}})>\unicode[STIX]{x1D707}_{s_{0},t_{0}}(F^{\bullet })$
. For
$\unicode[STIX]{x1D707}_{s_{0},t_{0}}(E_{\unicode[STIX]{x1D6FE}})>\unicode[STIX]{x1D707}_{s_{0},t_{0}}(F^{\bullet })$
. For
![]() $t\gg 0$
, we have
$t\gg 0$
, we have
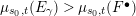 $\unicode[STIX]{x1D707}_{s_{0},t}(E_{\unicode[STIX]{x1D6FE}})>\unicode[STIX]{x1D707}_{s_{0},t}(F^{\bullet })$
because
$\unicode[STIX]{x1D707}_{s_{0},t}(E_{\unicode[STIX]{x1D6FE}})>\unicode[STIX]{x1D707}_{s_{0},t}(F^{\bullet })$
because
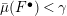 $\bar{\unicode[STIX]{x1D707}}(F^{\bullet })<\unicode[STIX]{x1D6FE}$
, or
$\bar{\unicode[STIX]{x1D707}}(F^{\bullet })<\unicode[STIX]{x1D6FE}$
, or
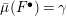 $\bar{\unicode[STIX]{x1D707}}(F^{\bullet })=\unicode[STIX]{x1D6FE}$
and
$\bar{\unicode[STIX]{x1D707}}(F^{\bullet })=\unicode[STIX]{x1D6FE}$
and
 $\text{ch}_{2}(F^{\bullet })/r<\text{ch}_{2}(E_{\unicode[STIX]{x1D6FE}})/\text{r}(E_{\unicode[STIX]{x1D6FE}})$
. These show that
$\text{ch}_{2}(F^{\bullet })/r<\text{ch}_{2}(E_{\unicode[STIX]{x1D6FE}})/\text{r}(E_{\unicode[STIX]{x1D6FE}})$
. These show that
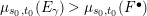 $\unicode[STIX]{x1D707}_{s_{0},t_{0}}(E_{\unicode[STIX]{x1D6FE}})>\unicode[STIX]{x1D707}_{s_{0},t_{0}}(F^{\bullet })$
for any
$\unicode[STIX]{x1D707}_{s_{0},t_{0}}(E_{\unicode[STIX]{x1D6FE}})>\unicode[STIX]{x1D707}_{s_{0},t_{0}}(F^{\bullet })$
for any
![]() $(s_{0},t_{0})$
in the proposition. This is the end of the proof of the claim.◻
$(s_{0},t_{0})$
in the proposition. This is the end of the proof of the claim.◻
Since
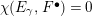 $\unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FE}},F^{\bullet })=0$
, we have
$\unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FE}},F^{\bullet })=0$
, we have
 $E_{1}^{0,1}=0$
. Now we have shown that
$E_{1}^{0,1}=0$
. Now we have shown that
![]() $E_{1}^{p,q}$
is zero except
$E_{1}^{p,q}$
is zero except
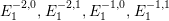 $E_{1}^{-2,0},E_{1}^{-2,1},E_{1}^{-1,0},E_{1}^{-1,1}$
. Since
$E_{1}^{-2,0},E_{1}^{-2,1},E_{1}^{-1,0},E_{1}^{-1,1}$
. Since
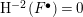 $\text{H}^{-2}(F^{\bullet })=0$
, the kernel of the map
$\text{H}^{-2}(F^{\bullet })=0$
, the kernel of the map
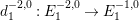 $d_{1}^{-2,0}:E_{1}^{-2,0}\rightarrow E_{1}^{-1,0}$
is zero. The cokernel of
$d_{1}^{-2,0}:E_{1}^{-2,0}\rightarrow E_{1}^{-1,0}$
is zero. The cokernel of
![]() $d_{1}^{-2,0}$
is a subsheaf of
$d_{1}^{-2,0}$
is a subsheaf of
 $H^{-1}(F^{\bullet })$
. Since
$H^{-1}(F^{\bullet })$
. Since
 $\text{Hom}(E_{\unicode[STIX]{x1D6FC}},\text{H}^{-1}(F^{\bullet }))=0$
by the inequality
$\text{Hom}(E_{\unicode[STIX]{x1D6FC}},\text{H}^{-1}(F^{\bullet }))=0$
by the inequality
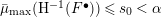 $\bar{\unicode[STIX]{x1D707}}_{\max }(\text{H}^{-1}(F^{\bullet }))\leqslant s_{0}<\unicode[STIX]{x1D6FC}$
, we have
$\bar{\unicode[STIX]{x1D707}}_{\max }(\text{H}^{-1}(F^{\bullet }))\leqslant s_{0}<\unicode[STIX]{x1D6FC}$
, we have
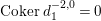 $\text{Coker}\,d_{1}^{-2,0}=0$
. Hence
$\text{Coker}\,d_{1}^{-2,0}=0$
. Hence
![]() $d_{1}^{-2,0}$
is an isomorphism. This occurs only when
$d_{1}^{-2,0}$
is an isomorphism. This occurs only when
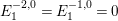 $E_{1}^{-2,0}=E_{1}^{-1,0}=0$
.
$E_{1}^{-2,0}=E_{1}^{-1,0}=0$
.
Step 2. We let
 $$\begin{eqnarray}F^{\bullet }=[E_{\unicode[STIX]{x1D6FD}-2}^{m}\xrightarrow[{}]{f}E_{\unicode[STIX]{x1D6FC}}^{n}].\end{eqnarray}$$
$$\begin{eqnarray}F^{\bullet }=[E_{\unicode[STIX]{x1D6FD}-2}^{m}\xrightarrow[{}]{f}E_{\unicode[STIX]{x1D6FC}}^{n}].\end{eqnarray}$$
We show that if
![]() $f$
is not injective, or if
$f$
is not injective, or if
![]() $f$
is injective and
$f$
is injective and
![]() $\text{Coker}$
is not semistable, then there exists a surjection
$\text{Coker}$
is not semistable, then there exists a surjection
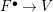 $F^{\bullet }\rightarrow V$
in
$F^{\bullet }\rightarrow V$
in
![]() ${\mathcal{A}}_{s_{0}}$
, where
${\mathcal{A}}_{s_{0}}$
, where
![]() $V$
is a semistable sheaf such that the following condition (a) or (b) holds:
$V$
is a semistable sheaf such that the following condition (a) or (b) holds:
-
(a)
 $\unicode[STIX]{x1D6FC}\leqslant \bar{\unicode[STIX]{x1D707}}(V)<\bar{\unicode[STIX]{x1D707}}$
,
$\unicode[STIX]{x1D6FC}\leqslant \bar{\unicode[STIX]{x1D707}}(V)<\bar{\unicode[STIX]{x1D707}}$
, -
(b)
 $\bar{\unicode[STIX]{x1D707}}(V)=\bar{\unicode[STIX]{x1D707}}$
and
$\bar{\unicode[STIX]{x1D707}}(V)=\bar{\unicode[STIX]{x1D707}}$
and
 $\text{ch}_{2}(F)/r>\text{ch}_{2}(V)/\text{r}(V)$
.
$\text{ch}_{2}(F)/r>\text{ch}_{2}(V)/\text{r}(V)$
.
To prove this, we consider
![]() $3$
cases:
$3$
cases:
-
(i)
 $f$
is not injective,
$f$
is not injective, -
(ii)
 $f$
is injective and
$f$
is injective and
 $\text{Coker}\,f$
is not torsion-free,
$\text{Coker}\,f$
is not torsion-free, -
(iii)
 $f$
is injective,
$f$
is injective,
 $\text{Coker}\,f$
is torsion-free, and
$\text{Coker}\,f$
is torsion-free, and
 $\text{Coker}\,f$
is not semistable.
$\text{Coker}\,f$
is not semistable.
Case (i): We have
 $$\begin{eqnarray}\displaystyle \bar{\unicode[STIX]{x1D707}}(\text{Coker}\,f) & = & \displaystyle \frac{\deg E_{\unicode[STIX]{x1D6FC}}^{n}-\deg \text{Im}\,f}{2(\text{r}(E_{\unicode[STIX]{x1D6FC}}^{n})-\text{r}(\text{Im}\,f))}\nonumber\\ \displaystyle & {\leqslant} & \displaystyle \frac{\deg E_{\unicode[STIX]{x1D6FC}}^{n}-\unicode[STIX]{x1D707}(E_{\unicode[STIX]{x1D6FD}-2})\text{r}(\text{Im}\,f)}{2(\text{r}(E_{\unicode[STIX]{x1D6FC}}^{n})-\text{r}(\text{Im}\,f))}<\frac{\deg E_{\unicode[STIX]{x1D6FC}}^{n}-\deg E_{\unicode[STIX]{x1D6FD}-2}^{m}}{2(\text{r}(E_{\unicode[STIX]{x1D6FC}}^{n})-\text{r}(E_{\unicode[STIX]{x1D6FD}-2}^{m}))}\nonumber\\ \displaystyle & = & \displaystyle \bar{\unicode[STIX]{x1D707}}(F^{\bullet })=\bar{\unicode[STIX]{x1D707}}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \bar{\unicode[STIX]{x1D707}}(\text{Coker}\,f) & = & \displaystyle \frac{\deg E_{\unicode[STIX]{x1D6FC}}^{n}-\deg \text{Im}\,f}{2(\text{r}(E_{\unicode[STIX]{x1D6FC}}^{n})-\text{r}(\text{Im}\,f))}\nonumber\\ \displaystyle & {\leqslant} & \displaystyle \frac{\deg E_{\unicode[STIX]{x1D6FC}}^{n}-\unicode[STIX]{x1D707}(E_{\unicode[STIX]{x1D6FD}-2})\text{r}(\text{Im}\,f)}{2(\text{r}(E_{\unicode[STIX]{x1D6FC}}^{n})-\text{r}(\text{Im}\,f))}<\frac{\deg E_{\unicode[STIX]{x1D6FC}}^{n}-\deg E_{\unicode[STIX]{x1D6FD}-2}^{m}}{2(\text{r}(E_{\unicode[STIX]{x1D6FC}}^{n})-\text{r}(E_{\unicode[STIX]{x1D6FD}-2}^{m}))}\nonumber\\ \displaystyle & = & \displaystyle \bar{\unicode[STIX]{x1D707}}(F^{\bullet })=\bar{\unicode[STIX]{x1D707}}.\nonumber\end{eqnarray}$$
Let
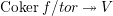 $\text{Coker}\,f/tor{\twoheadrightarrow}V$
be the quotient with minimum value of
$\text{Coker}\,f/tor{\twoheadrightarrow}V$
be the quotient with minimum value of
![]() $\bar{\unicode[STIX]{x1D707}}$
. Then the composite of morphisms
$\bar{\unicode[STIX]{x1D707}}$
. Then the composite of morphisms
 $$\begin{eqnarray}F^{\bullet }\rightarrow \text{H}^{0}(F^{\bullet })=\text{Coker}\,f\rightarrow \text{Coker}\,f/tor{\twoheadrightarrow}V\end{eqnarray}$$
$$\begin{eqnarray}F^{\bullet }\rightarrow \text{H}^{0}(F^{\bullet })=\text{Coker}\,f\rightarrow \text{Coker}\,f/tor{\twoheadrightarrow}V\end{eqnarray}$$
is a surjection in
![]() ${\mathcal{A}}_{s_{0}}$
and
${\mathcal{A}}_{s_{0}}$
and
![]() $V$
is a semistable sheaf satisfying (a).
$V$
is a semistable sheaf satisfying (a).
Case (ii): We have
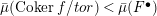 $\bar{\unicode[STIX]{x1D707}}(\text{Coker}\,f/tor)<\bar{\unicode[STIX]{x1D707}}(F^{\bullet })$
. We define
$\bar{\unicode[STIX]{x1D707}}(\text{Coker}\,f/tor)<\bar{\unicode[STIX]{x1D707}}(F^{\bullet })$
. We define
![]() $V$
and
$V$
and
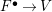 $F^{\bullet }\rightarrow V$
as in Case (i). Then
$F^{\bullet }\rightarrow V$
as in Case (i). Then
![]() $V$
satisfies (a).
$V$
satisfies (a).
Case (iii): There exists a surjective morphism
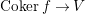 $\text{Coker}\,f\rightarrow V$
of sheaves such that
$\text{Coker}\,f\rightarrow V$
of sheaves such that
![]() $V$
is a semistable sheaf satisfying (a) or (b). The composite (5.11) (with tor
$V$
is a semistable sheaf satisfying (a) or (b). The composite (5.11) (with tor
![]() $=$
0) is what we want.
$=$
0) is what we want.
Step
![]() $3$
. Let
$3$
. Let
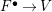 $F^{\bullet }\rightarrow V$
be the surjection in
$F^{\bullet }\rightarrow V$
be the surjection in
![]() ${\mathcal{A}}_{s_{0}}$
which we obtained in Step 2. By the
${\mathcal{A}}_{s_{0}}$
which we obtained in Step 2. By the
![]() $(s_{0},t_{0})$
-semistability of
$(s_{0},t_{0})$
-semistability of
![]() $F^{\bullet }$
, we have
$F^{\bullet }$
, we have
 $$\begin{eqnarray}\unicode[STIX]{x1D707}_{s_{0},t_{0}}(F^{\bullet })\leqslant \unicode[STIX]{x1D707}_{s_{0},t_{0}}(V).\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D707}_{s_{0},t_{0}}(F^{\bullet })\leqslant \unicode[STIX]{x1D707}_{s_{0},t_{0}}(V).\end{eqnarray}$$
We have
 $$\begin{eqnarray}\unicode[STIX]{x1D707}_{s_{0},t}(F^{\bullet })>\unicode[STIX]{x1D707}_{s_{0},t}(V)\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D707}_{s_{0},t}(F^{\bullet })>\unicode[STIX]{x1D707}_{s_{0},t}(V)\end{eqnarray}$$
for
![]() $t\gg 0$
because of the conditions (a) and (b). So we have
$t\gg 0$
because of the conditions (a) and (b). So we have
 $$\begin{eqnarray}\unicode[STIX]{x1D707}_{s_{0},t_{1}}(F^{\bullet })=\unicode[STIX]{x1D707}_{s_{0},t_{1}}(V)\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D707}_{s_{0},t_{1}}(F^{\bullet })=\unicode[STIX]{x1D707}_{s_{0},t_{1}}(V)\end{eqnarray}$$
for some
![]() $t_{1}\geqslant t_{0}$
.
$t_{1}\geqslant t_{0}$
.
Suppose that the condition (b) in Step 2 holds. Then the wall
![]() $W_{F^{\bullet },V}$
is a vertical wall and we see that
$W_{F^{\bullet },V}$
is a vertical wall and we see that
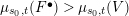 $\unicode[STIX]{x1D707}_{s_{0},t}(F^{\bullet })>\unicode[STIX]{x1D707}_{s_{0},t}(V)$
for any
$\unicode[STIX]{x1D707}_{s_{0},t}(F^{\bullet })>\unicode[STIX]{x1D707}_{s_{0},t}(V)$
for any
![]() $t>0$
. This is a contradiction.
$t>0$
. This is a contradiction.
Suppose that the condition (a) in Step 2 holds. The center of the semicircular wall
![]() $W_{F^{\bullet },V}$
is
$W_{F^{\bullet },V}$
is
![]() $(x,0)$
, where
$(x,0)$
, where
 $$\begin{eqnarray}x=\frac{\text{ch}_{2}(F^{\bullet })/r-\text{ch}_{2}(V)/\text{r}(V)}{\unicode[STIX]{x1D707}(F^{\bullet })-\unicode[STIX]{x1D707}(V)}.\end{eqnarray}$$
$$\begin{eqnarray}x=\frac{\text{ch}_{2}(F^{\bullet })/r-\text{ch}_{2}(V)/\text{r}(V)}{\unicode[STIX]{x1D707}(F^{\bullet })-\unicode[STIX]{x1D707}(V)}.\end{eqnarray}$$
Since the moduli space
![]() $M(\unicode[STIX]{x1D709})$
has height zero, we have
$M(\unicode[STIX]{x1D709})$
has height zero, we have
 $$\begin{eqnarray}\displaystyle 0 & = & \displaystyle \frac{\unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FE}},F^{\bullet })}{\text{r}(E_{\unicode[STIX]{x1D6FE}})r}=\unicode[STIX]{x1D712}({\mathcal{O}}_{S})+\frac{\text{ch}_{2}(F^{\bullet })}{r}\nonumber\\ \displaystyle & & \displaystyle -\,\frac{c_{1}(E_{\unicode[STIX]{x1D6FE}})c_{1}(F)}{\text{r}(E_{\unicode[STIX]{x1D6FE}})r}+\frac{\text{ch}_{2}(E_{\unicode[STIX]{x1D6FE}})}{\text{r}(E_{\unicode[STIX]{x1D6FE}})}+\unicode[STIX]{x1D707}(F^{\bullet })-\unicode[STIX]{x1D707}(E_{\unicode[STIX]{x1D6FE}}).\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle 0 & = & \displaystyle \frac{\unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FE}},F^{\bullet })}{\text{r}(E_{\unicode[STIX]{x1D6FE}})r}=\unicode[STIX]{x1D712}({\mathcal{O}}_{S})+\frac{\text{ch}_{2}(F^{\bullet })}{r}\nonumber\\ \displaystyle & & \displaystyle -\,\frac{c_{1}(E_{\unicode[STIX]{x1D6FE}})c_{1}(F)}{\text{r}(E_{\unicode[STIX]{x1D6FE}})r}+\frac{\text{ch}_{2}(E_{\unicode[STIX]{x1D6FE}})}{\text{r}(E_{\unicode[STIX]{x1D6FE}})}+\unicode[STIX]{x1D707}(F^{\bullet })-\unicode[STIX]{x1D707}(E_{\unicode[STIX]{x1D6FE}}).\end{eqnarray}$$
Since the semistable sheaf
![]() $V$
satisfies (a), we have
$V$
satisfies (a), we have
 $$\begin{eqnarray}\displaystyle 0 & {\geqslant} & \displaystyle \frac{\unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FE}},V)}{\text{r}(E_{\unicode[STIX]{x1D6FE}})\text{r}(V)}=\unicode[STIX]{x1D712}({\mathcal{O}}_{S})+\frac{\text{ch}_{2}(V)}{\text{r}(V)}\nonumber\\ \displaystyle & & \displaystyle -\,\frac{c_{1}(E_{\unicode[STIX]{x1D6FE}})c_{1}(V)}{\text{r}(E_{\unicode[STIX]{x1D6FE}})\text{r}(V)}+\frac{\text{ch}_{2}(E_{\unicode[STIX]{x1D6FE}})}{\text{r}(E_{\unicode[STIX]{x1D6FE}})}+\unicode[STIX]{x1D707}(V)-\unicode[STIX]{x1D707}(E_{\unicode[STIX]{x1D6FE}}).\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle 0 & {\geqslant} & \displaystyle \frac{\unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FE}},V)}{\text{r}(E_{\unicode[STIX]{x1D6FE}})\text{r}(V)}=\unicode[STIX]{x1D712}({\mathcal{O}}_{S})+\frac{\text{ch}_{2}(V)}{\text{r}(V)}\nonumber\\ \displaystyle & & \displaystyle -\,\frac{c_{1}(E_{\unicode[STIX]{x1D6FE}})c_{1}(V)}{\text{r}(E_{\unicode[STIX]{x1D6FE}})\text{r}(V)}+\frac{\text{ch}_{2}(E_{\unicode[STIX]{x1D6FE}})}{\text{r}(E_{\unicode[STIX]{x1D6FE}})}+\unicode[STIX]{x1D707}(V)-\unicode[STIX]{x1D707}(E_{\unicode[STIX]{x1D6FE}}).\end{eqnarray}$$
From (5.14) and (5.15), we obtain
 $$\begin{eqnarray}x=-\frac{\unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FE}},V)}{\text{r}(E_{\unicode[STIX]{x1D6FE}})\text{r}(V)(\unicode[STIX]{x1D707}(F^{\bullet })-\unicode[STIX]{x1D707}(V))}+\unicode[STIX]{x1D6FE}-1\geqslant \unicode[STIX]{x1D6FE}-1.\end{eqnarray}$$
$$\begin{eqnarray}x=-\frac{\unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FE}},V)}{\text{r}(E_{\unicode[STIX]{x1D6FE}})\text{r}(V)(\unicode[STIX]{x1D707}(F^{\bullet })-\unicode[STIX]{x1D707}(V))}+\unicode[STIX]{x1D6FE}-1\geqslant \unicode[STIX]{x1D6FE}-1.\end{eqnarray}$$
Since the center of the wall
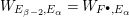 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}=W_{F^{\bullet },E_{\unicode[STIX]{x1D6FC}}}$
is
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}=W_{F^{\bullet },E_{\unicode[STIX]{x1D6FC}}}$
is
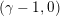 $(\unicode[STIX]{x1D6FE}-1,0)$
, the wall
$(\unicode[STIX]{x1D6FE}-1,0)$
, the wall
![]() $W_{F^{\bullet },V}$
is equal to or lies inside
$W_{F^{\bullet },V}$
is equal to or lies inside
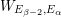 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
. This contradicts (5.13).
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
. This contradicts (5.13).
Finally we consider the stable case. If
![]() $F^{\bullet }$
is
$F^{\bullet }$
is
![]() $(s_{0},t_{0})$
-stable, we already know it is a semistable sheaf
$(s_{0},t_{0})$
-stable, we already know it is a semistable sheaf
![]() $F$
. If
$F$
. If
![]() $F$
is not stable, then there is a subsheaf
$F$
is not stable, then there is a subsheaf
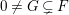 $0\neq G\subsetneq F$
with
$0\neq G\subsetneq F$
with
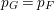 $p_{G}=p_{F}$
. Then
$p_{G}=p_{F}$
. Then
![]() $G$
is a subobject of
$G$
is a subobject of
![]() $F$
in
$F$
in
![]() ${\mathcal{A}}_{s_{0}}$
with
${\mathcal{A}}_{s_{0}}$
with
 $\unicode[STIX]{x1D707}_{s_{0},t_{0}}(G)=\unicode[STIX]{x1D707}_{s_{0},t_{0}}(F)$
. This contradicts the
$\unicode[STIX]{x1D707}_{s_{0},t_{0}}(G)=\unicode[STIX]{x1D707}_{s_{0},t_{0}}(F)$
. This contradicts the
![]() $(s_{0},t_{0})$
-stability of
$(s_{0},t_{0})$
-stability of
![]() $F$
.◻
$F$
.◻
5.2 Isomorphism to moduli of quiver representations
In this section, we see that
![]() $M(\unicode[STIX]{x1D709})$
is isomorphic to a moduli space of quiver representations.
$M(\unicode[STIX]{x1D709})$
is isomorphic to a moduli space of quiver representations.
A quiver is a quadruple
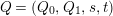 $Q=(Q_{0},Q_{1},s,t)$
, where
$Q=(Q_{0},Q_{1},s,t)$
, where
![]() $Q_{0}$
is the set of vertexes,
$Q_{0}$
is the set of vertexes,
![]() $Q_{1}$
is the set of arrows, and the maps
$Q_{1}$
is the set of arrows, and the maps
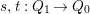 $s,t:Q_{1}\rightarrow Q_{0}$
send an arrow to its source and target respectively. A path of the quiver
$s,t:Q_{1}\rightarrow Q_{0}$
send an arrow to its source and target respectively. A path of the quiver
![]() $Q$
is a sequence of arrows
$Q$
is a sequence of arrows
 $(\unicode[STIX]{x1D6FC}_{n},\ldots ,\unicode[STIX]{x1D6FC}_{1})$
such that
$(\unicode[STIX]{x1D6FC}_{n},\ldots ,\unicode[STIX]{x1D6FC}_{1})$
such that
 $t(\unicode[STIX]{x1D6FC}_{i})=s(\unicode[STIX]{x1D6FC}_{i+1})$
. For a path
$t(\unicode[STIX]{x1D6FC}_{i})=s(\unicode[STIX]{x1D6FC}_{i+1})$
. For a path
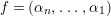 $f=(\unicode[STIX]{x1D6FC}_{n},\ldots ,\unicode[STIX]{x1D6FC}_{1})$
, we define
$f=(\unicode[STIX]{x1D6FC}_{n},\ldots ,\unicode[STIX]{x1D6FC}_{1})$
, we define
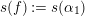 $s(f):=s(\unicode[STIX]{x1D6FC}_{1})$
and
$s(f):=s(\unicode[STIX]{x1D6FC}_{1})$
and
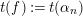 $t(f):=t(\unicode[STIX]{x1D6FC}_{n})$
. The path algebra
$t(f):=t(\unicode[STIX]{x1D6FC}_{n})$
. The path algebra
![]() $A(Q)$
of a quiver is the algebra whose elements are
$A(Q)$
of a quiver is the algebra whose elements are
![]() $\mathbb{C}$
-linear combinations of paths of
$\mathbb{C}$
-linear combinations of paths of
![]() $Q$
. If
$Q$
. If
![]() $f$
and
$f$
and
![]() $g$
are paths of
$g$
are paths of
![]() $Q$
such that
$Q$
such that
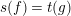 $s(f)=t(g)$
, then
$s(f)=t(g)$
, then
![]() $fg$
is the path obtained by connecting
$fg$
is the path obtained by connecting
![]() $g$
and
$g$
and
![]() $f$
.
$f$
.
Giving a left
![]() $A(Q)$
-module is equivalent to giving data
$A(Q)$
-module is equivalent to giving data
 $$\begin{eqnarray}\left(\left\{V_{v}\right\}_{v\in Q_{0}},\left\{\unicode[STIX]{x1D70C}_{\unicode[STIX]{x1D6FC}}\right\}_{\unicode[STIX]{x1D6FC}\in Q_{1}}\right)\!,\end{eqnarray}$$
$$\begin{eqnarray}\left(\left\{V_{v}\right\}_{v\in Q_{0}},\left\{\unicode[STIX]{x1D70C}_{\unicode[STIX]{x1D6FC}}\right\}_{\unicode[STIX]{x1D6FC}\in Q_{1}}\right)\!,\end{eqnarray}$$
where
![]() $V_{v}$
is a
$V_{v}$
is a
![]() $\mathbb{C}$
-vector space, and
$\mathbb{C}$
-vector space, and
 $\unicode[STIX]{x1D70C}_{\unicode[STIX]{x1D6FC}}:V_{s(\unicode[STIX]{x1D6FC})}\rightarrow V_{t(\unicode[STIX]{x1D6FC})}$
is a
$\unicode[STIX]{x1D70C}_{\unicode[STIX]{x1D6FC}}:V_{s(\unicode[STIX]{x1D6FC})}\rightarrow V_{t(\unicode[STIX]{x1D6FC})}$
is a
![]() $\mathbb{C}$
-linear map.
$\mathbb{C}$
-linear map.
We call the data (5.16) a representation of the quiver
![]() $Q$
. The map
$Q$
. The map
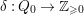 $\unicode[STIX]{x1D6FF}:Q_{0}\rightarrow \mathbb{Z}_{{\geqslant}0}$
given by
$\unicode[STIX]{x1D6FF}:Q_{0}\rightarrow \mathbb{Z}_{{\geqslant}0}$
given by
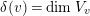 $\unicode[STIX]{x1D6FF}(v)=\dim V_{v}$
is called the dimension vector of the representation. Fix a map
$\unicode[STIX]{x1D6FF}(v)=\dim V_{v}$
is called the dimension vector of the representation. Fix a map
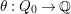 $\unicode[STIX]{x1D703}:Q_{0}\rightarrow \mathbb{Q}$
, called a weight vector, such that
$\unicode[STIX]{x1D703}:Q_{0}\rightarrow \mathbb{Q}$
, called a weight vector, such that
 $\sum _{v\in Q_{0}}\unicode[STIX]{x1D6FF}(v)\unicode[STIX]{x1D703}(v)=0$
. The representation (5.16) of the quiver is said to be
$\sum _{v\in Q_{0}}\unicode[STIX]{x1D6FF}(v)\unicode[STIX]{x1D703}(v)=0$
. The representation (5.16) of the quiver is said to be
![]() $\unicode[STIX]{x1D703}$
-semistable if for all subrepresentations
$\unicode[STIX]{x1D703}$
-semistable if for all subrepresentations
 $$\begin{eqnarray}\left(\left\{V_{v}^{\prime }\right\}_{v\in Q_{0}},\left\{\unicode[STIX]{x1D70C}_{\unicode[STIX]{x1D6FC}}|_{V_{s(\unicode[STIX]{x1D6FC})}^{\prime }}\right\}_{\unicode[STIX]{x1D6FC}\in Q_{1}}\right)\!,\end{eqnarray}$$
$$\begin{eqnarray}\left(\left\{V_{v}^{\prime }\right\}_{v\in Q_{0}},\left\{\unicode[STIX]{x1D70C}_{\unicode[STIX]{x1D6FC}}|_{V_{s(\unicode[STIX]{x1D6FC})}^{\prime }}\right\}_{\unicode[STIX]{x1D6FC}\in Q_{1}}\right)\!,\end{eqnarray}$$
of (5.16), the inequality
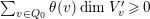 $\sum _{v\in Q_{0}}\unicode[STIX]{x1D703}(v)\dim V_{v}^{\prime }\geqslant 0$
holds.
$\sum _{v\in Q_{0}}\unicode[STIX]{x1D703}(v)\dim V_{v}^{\prime }\geqslant 0$
holds.
In the following, in order to describe a quiver, we use the notation
 $$\begin{eqnarray}Q=(Q_{0},\{N_{v,v^{\prime }}\}_{(v,v^{\prime })\in Q_{0}\times Q_{0}}),\end{eqnarray}$$
$$\begin{eqnarray}Q=(Q_{0},\{N_{v,v^{\prime }}\}_{(v,v^{\prime })\in Q_{0}\times Q_{0}}),\end{eqnarray}$$
where
![]() $N_{v,v^{\prime }}$
means the number of arrows
$N_{v,v^{\prime }}$
means the number of arrows
![]() $\unicode[STIX]{x1D6FC}$
with
$\unicode[STIX]{x1D6FC}$
with
 $s(\unicode[STIX]{x1D6FC})=v$
and
$s(\unicode[STIX]{x1D6FC})=v$
and
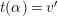 $t(\unicode[STIX]{x1D6FC})=v^{\prime }$
.
$t(\unicode[STIX]{x1D6FC})=v^{\prime }$
.
Recall
![]() $\unicode[STIX]{x1D6FC}$
,
$\unicode[STIX]{x1D6FC}$
,
![]() $\unicode[STIX]{x1D6FD}$
and
$\unicode[STIX]{x1D6FD}$
and
![]() $\unicode[STIX]{x1D6FE}$
defined at the beginning of Section 5.1. When
$\unicode[STIX]{x1D6FE}$
defined at the beginning of Section 5.1. When
![]() $\unicode[STIX]{x1D6FC}$
,
$\unicode[STIX]{x1D6FC}$
,
![]() $\unicode[STIX]{x1D6FD}$
or
$\unicode[STIX]{x1D6FD}$
or
![]() $\unicode[STIX]{x1D6FE}$
is even respectively, we define quivers
$\unicode[STIX]{x1D6FE}$
is even respectively, we define quivers
![]() $Q^{\unicode[STIX]{x1D6FC}}$
,
$Q^{\unicode[STIX]{x1D6FC}}$
,
![]() $Q^{\unicode[STIX]{x1D6FD}}$
and
$Q^{\unicode[STIX]{x1D6FD}}$
and
![]() $Q^{\unicode[STIX]{x1D6FE}}$
as follows.
$Q^{\unicode[STIX]{x1D6FE}}$
as follows.
The quiver
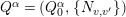 $Q^{\unicode[STIX]{x1D6FC}}=(Q_{0}^{\unicode[STIX]{x1D6FC}},\{N_{v,v^{\prime }}\})$
is defined by
$Q^{\unicode[STIX]{x1D6FC}}=(Q_{0}^{\unicode[STIX]{x1D6FC}},\{N_{v,v^{\prime }}\})$
is defined by
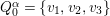 $Q_{0}^{\unicode[STIX]{x1D6FC}}=\{v_{1},v_{2},v_{3}\}$
and
$Q_{0}^{\unicode[STIX]{x1D6FC}}=\{v_{1},v_{2},v_{3}\}$
and
 $N_{v_{1},v_{2}}=N_{v_{1},v_{3}}=\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},E_{\unicode[STIX]{x1D6FD}})$
, and
$N_{v_{1},v_{2}}=N_{v_{1},v_{3}}=\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},E_{\unicode[STIX]{x1D6FD}})$
, and
 $N_{v_{i},v_{j}}=0$
for other pairs
$N_{v_{i},v_{j}}=0$
for other pairs
![]() $(v_{i},v_{j})$
(see Notation 4.6 for the notation
$(v_{i},v_{j})$
(see Notation 4.6 for the notation
![]() $\tilde{\unicode[STIX]{x1D712}}$
).
$\tilde{\unicode[STIX]{x1D712}}$
).
The quiver
 $Q^{\unicode[STIX]{x1D6FD}}=(Q_{0}^{\unicode[STIX]{x1D6FD}},\{N_{v,v^{\prime }}\})$
is defined by
$Q^{\unicode[STIX]{x1D6FD}}=(Q_{0}^{\unicode[STIX]{x1D6FD}},\{N_{v,v^{\prime }}\})$
is defined by
 $Q_{0}^{\unicode[STIX]{x1D6FD}}=\{v_{1},v_{2},v_{3}\}$
and
$Q_{0}^{\unicode[STIX]{x1D6FD}}=\{v_{1},v_{2},v_{3}\}$
and
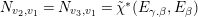 $N_{v_{2},v_{1}}=N_{v_{3},v_{1}}=\tilde{\unicode[STIX]{x1D712}}^{\ast }(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},E_{\unicode[STIX]{x1D6FD}})$
, and
$N_{v_{2},v_{1}}=N_{v_{3},v_{1}}=\tilde{\unicode[STIX]{x1D712}}^{\ast }(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},E_{\unicode[STIX]{x1D6FD}})$
, and
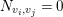 $N_{v_{i},v_{j}}=0$
for other pairs
$N_{v_{i},v_{j}}=0$
for other pairs
![]() $(v_{i},v_{j})$
.
$(v_{i},v_{j})$
.
The quiver
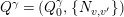 $Q^{\unicode[STIX]{x1D6FE}}=(Q_{0}^{\unicode[STIX]{x1D6FE}},\{N_{v,v^{\prime }}\})$
is defined by
$Q^{\unicode[STIX]{x1D6FE}}=(Q_{0}^{\unicode[STIX]{x1D6FE}},\{N_{v,v^{\prime }}\})$
is defined by
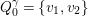 $Q_{0}^{\unicode[STIX]{x1D6FE}}=\{v_{1},v_{2}\}$
and
$Q_{0}^{\unicode[STIX]{x1D6FE}}=\{v_{1},v_{2}\}$
and
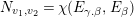 $N_{v_{1},v_{2}}=\unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},E_{\unicode[STIX]{x1D6FD}})$
, and
$N_{v_{1},v_{2}}=\unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},E_{\unicode[STIX]{x1D6FD}})$
, and
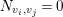 $N_{v_{i},v_{j}}=0$
for other pairs
$N_{v_{i},v_{j}}=0$
for other pairs
![]() $(v_{i},v_{j})$
. (See Figures 4–6.)
$(v_{i},v_{j})$
. (See Figures 4–6.)

Figure 4.
![]() $Q^{\unicode[STIX]{x1D6FC}}$
.
$Q^{\unicode[STIX]{x1D6FC}}$
.

Figure 5.
![]() $Q^{\unicode[STIX]{x1D6FD}}$
.
$Q^{\unicode[STIX]{x1D6FD}}$
.

Figure 6.
![]() $Q^{\unicode[STIX]{x1D6FE}}$
.
$Q^{\unicode[STIX]{x1D6FE}}$
.
We define moduli spaces
![]() $N^{\unicode[STIX]{x1D6FC}}$
,
$N^{\unicode[STIX]{x1D6FC}}$
,
![]() $N^{\unicode[STIX]{x1D6FD}}$
and
$N^{\unicode[STIX]{x1D6FD}}$
and
![]() $N^{\unicode[STIX]{x1D6FE}}$
of representations of quivers as follows.
$N^{\unicode[STIX]{x1D6FE}}$
of representations of quivers as follows.
![]() $N^{\unicode[STIX]{x1D6FC}}$
(resp.
$N^{\unicode[STIX]{x1D6FC}}$
(resp.
![]() $N^{\unicode[STIX]{x1D6FD}}$
) is the coarse moduli space of
$N^{\unicode[STIX]{x1D6FD}}$
) is the coarse moduli space of
![]() $\unicode[STIX]{x1D703}$
-semistable representations of the quiver
$\unicode[STIX]{x1D703}$
-semistable representations of the quiver
![]() $Q^{\unicode[STIX]{x1D6FC}}$
(resp.
$Q^{\unicode[STIX]{x1D6FC}}$
(resp.
![]() $Q^{\unicode[STIX]{x1D6FD}}$
) with dimension vector
$Q^{\unicode[STIX]{x1D6FD}}$
) with dimension vector
 $(\unicode[STIX]{x1D6FF}(v_{1}),\unicode[STIX]{x1D6FF}(v_{2}),\unicode[STIX]{x1D6FF}(v_{3}))$
equal to
$(\unicode[STIX]{x1D6FF}(v_{1}),\unicode[STIX]{x1D6FF}(v_{2}),\unicode[STIX]{x1D6FF}(v_{3}))$
equal to
 $(m,n,n)$
(resp.
$(m,n,n)$
(resp.
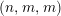 $(n,m,m)$
), where the weight vector
$(n,m,m)$
), where the weight vector
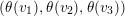 $(\unicode[STIX]{x1D703}(v_{1}),\unicode[STIX]{x1D703}(v_{2}),\unicode[STIX]{x1D703}(v_{3}))$
is
$(\unicode[STIX]{x1D703}(v_{1}),\unicode[STIX]{x1D703}(v_{2}),\unicode[STIX]{x1D703}(v_{3}))$
is
 $(-2n,m,m)$
(resp.
$(-2n,m,m)$
(resp.
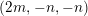 $(2m,-n,-n)$
).
$(2m,-n,-n)$
).
![]() $N^{\unicode[STIX]{x1D6FE}}$
is the coarse moduli space of
$N^{\unicode[STIX]{x1D6FE}}$
is the coarse moduli space of
![]() $\unicode[STIX]{x1D703}$
-semistable representations of the quiver
$\unicode[STIX]{x1D703}$
-semistable representations of the quiver
![]() $Q^{\unicode[STIX]{x1D6FE}}$
with dimension vector
$Q^{\unicode[STIX]{x1D6FE}}$
with dimension vector
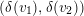 $(\unicode[STIX]{x1D6FF}(v_{1}),\unicode[STIX]{x1D6FF}(v_{2}))$
equal to
$(\unicode[STIX]{x1D6FF}(v_{1}),\unicode[STIX]{x1D6FF}(v_{2}))$
equal to
![]() $(m,n)$
, where the weight vector
$(m,n)$
, where the weight vector
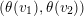 $(\unicode[STIX]{x1D703}(v_{1}),\unicode[STIX]{x1D703}(v_{2}))$
is
$(\unicode[STIX]{x1D703}(v_{1}),\unicode[STIX]{x1D703}(v_{2}))$
is
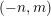 $(-n,m)$
.
$(-n,m)$
.
Theorem 5.4. If the symmetric exceptional bundle
![]() $E_{\unicode[STIX]{x1D6FC}}$
(resp.
$E_{\unicode[STIX]{x1D6FC}}$
(resp.
![]() $E_{\unicode[STIX]{x1D6FD}}$
or
$E_{\unicode[STIX]{x1D6FD}}$
or
![]() $E_{\unicode[STIX]{x1D6FE}}$
) is even, then the moduli space
$E_{\unicode[STIX]{x1D6FE}}$
) is even, then the moduli space
![]() $M(\unicode[STIX]{x1D709})$
is isomorphic to
$M(\unicode[STIX]{x1D709})$
is isomorphic to
![]() $N^{\unicode[STIX]{x1D6FC}}$
(resp.
$N^{\unicode[STIX]{x1D6FC}}$
(resp.
![]() $N^{\unicode[STIX]{x1D6FD}}$
or
$N^{\unicode[STIX]{x1D6FD}}$
or
![]() $N^{\unicode[STIX]{x1D6FE}}$
).
$N^{\unicode[STIX]{x1D6FE}}$
).
For the proof of the theorem, we imitate the argument in [Reference Arcara, Bertram, Coskun and HuizengaABCH, Sections 7, 8], [Reference OhkawaOh].
Fix a point with
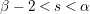 $\unicode[STIX]{x1D6FD}-2<s<\unicode[STIX]{x1D6FC}$
in the upper half
$\unicode[STIX]{x1D6FD}-2<s<\unicode[STIX]{x1D6FC}$
in the upper half
![]() $(s,t)$
-plane such that
$(s,t)$
-plane such that
![]() $(s,t)$
lies outside the wall
$(s,t)$
lies outside the wall
 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
and inside
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
and inside
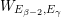 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FE}}}$
. Then we have
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FE}}}$
. Then we have
 $$\begin{eqnarray}\unicode[STIX]{x1D707}_{s,t}(E_{\unicode[STIX]{x1D6FE}})>\unicode[STIX]{x1D707}_{s,t}(E_{\unicode[STIX]{x1D6FD}-2}[1])>\unicode[STIX]{x1D707}(E_{\unicode[STIX]{x1D6FC}}).\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D707}_{s,t}(E_{\unicode[STIX]{x1D6FE}})>\unicode[STIX]{x1D707}_{s,t}(E_{\unicode[STIX]{x1D6FD}-2}[1])>\unicode[STIX]{x1D707}(E_{\unicode[STIX]{x1D6FC}}).\end{eqnarray}$$
Fix
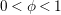 $0<\unicode[STIX]{x1D719}<1$
such that
$0<\unicode[STIX]{x1D719}<1$
such that
 $$\begin{eqnarray}\arg Z_{s,t}(E_{\unicode[STIX]{x1D6FE}})>\unicode[STIX]{x1D719}>\arg Z_{s,t}(E_{\unicode[STIX]{x1D6FD}-2}[1]).\end{eqnarray}$$
$$\begin{eqnarray}\arg Z_{s,t}(E_{\unicode[STIX]{x1D6FE}})>\unicode[STIX]{x1D719}>\arg Z_{s,t}(E_{\unicode[STIX]{x1D6FD}-2}[1]).\end{eqnarray}$$
Put
 $$\begin{eqnarray}\displaystyle {\mathcal{Q}}_{\unicode[STIX]{x1D719}} & := & \displaystyle \langle Q\in {\mathcal{A}}_{s}\mid Q\text{ is }(s,t)\text{-semistable,}\arg Z_{s,t}(Q)>\unicode[STIX]{x1D719}\unicode[STIX]{x1D70B}\rangle \nonumber\\ \displaystyle {\mathcal{F}}_{\unicode[STIX]{x1D719}} & := & \displaystyle \langle F\in {\mathcal{A}}_{s}\mid F\text{ is }(s,t)\text{-semistable,}\arg Z_{s,t}(Q)\leqslant \unicode[STIX]{x1D719}\unicode[STIX]{x1D70B}\rangle ,\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle {\mathcal{Q}}_{\unicode[STIX]{x1D719}} & := & \displaystyle \langle Q\in {\mathcal{A}}_{s}\mid Q\text{ is }(s,t)\text{-semistable,}\arg Z_{s,t}(Q)>\unicode[STIX]{x1D719}\unicode[STIX]{x1D70B}\rangle \nonumber\\ \displaystyle {\mathcal{F}}_{\unicode[STIX]{x1D719}} & := & \displaystyle \langle F\in {\mathcal{A}}_{s}\mid F\text{ is }(s,t)\text{-semistable,}\arg Z_{s,t}(Q)\leqslant \unicode[STIX]{x1D719}\unicode[STIX]{x1D70B}\rangle ,\nonumber\end{eqnarray}$$
and define
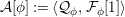 ${\mathcal{A}}[\unicode[STIX]{x1D719}]:=\langle {\mathcal{Q}}_{\unicode[STIX]{x1D719}},{\mathcal{F}}_{\unicode[STIX]{x1D719}}[1]\rangle$
and
${\mathcal{A}}[\unicode[STIX]{x1D719}]:=\langle {\mathcal{Q}}_{\unicode[STIX]{x1D719}},{\mathcal{F}}_{\unicode[STIX]{x1D719}}[1]\rangle$
and
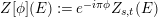 $Z[\unicode[STIX]{x1D719}](E):=e^{-i\unicode[STIX]{x1D70B}\unicode[STIX]{x1D719}}Z_{s,t}(E)$
. Then the pair
$Z[\unicode[STIX]{x1D719}](E):=e^{-i\unicode[STIX]{x1D70B}\unicode[STIX]{x1D719}}Z_{s,t}(E)$
. Then the pair
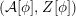 $({\mathcal{A}}[\unicode[STIX]{x1D719}],Z[\unicode[STIX]{x1D719}])$
is also a Bridgeland stability condition of
$({\mathcal{A}}[\unicode[STIX]{x1D719}],Z[\unicode[STIX]{x1D719}])$
is also a Bridgeland stability condition of
![]() $\text{D}(S)$
. Since the operation “
$\text{D}(S)$
. Since the operation “
![]() $[\unicode[STIX]{x1D719}]$
” does not change the Bridgeland semistable objects, the moduli space of
$[\unicode[STIX]{x1D719}]$
” does not change the Bridgeland semistable objects, the moduli space of
![]() $(s,t)$
-semistable objects with K-class equal to
$(s,t)$
-semistable objects with K-class equal to
![]() $\unicode[STIX]{x1D709}$
is isomorphic to the moduli space of
$\unicode[STIX]{x1D709}$
is isomorphic to the moduli space of
![]() $Z[\unicode[STIX]{x1D719}]$
-semistable objects in
$Z[\unicode[STIX]{x1D719}]$
-semistable objects in
![]() ${\mathcal{A}}[\unicode[STIX]{x1D719}]$
with K-class
${\mathcal{A}}[\unicode[STIX]{x1D719}]$
with K-class
![]() $-\unicode[STIX]{x1D709}$
by the correspondence
$-\unicode[STIX]{x1D709}$
by the correspondence
![]() $F^{\bullet }$
to
$F^{\bullet }$
to
![]() $F^{\bullet }[1]$
.
$F^{\bullet }[1]$
.
Put
 $$\begin{eqnarray}E:=E_{\unicode[STIX]{x1D6FE}}\oplus E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}}\oplus E_{\unicode[STIX]{x1D6FD}}\quad \text{and}\quad A:=\text{End}(E).\end{eqnarray}$$
$$\begin{eqnarray}E:=E_{\unicode[STIX]{x1D6FE}}\oplus E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}}\oplus E_{\unicode[STIX]{x1D6FD}}\quad \text{and}\quad A:=\text{End}(E).\end{eqnarray}$$
We define a functor
 $$\begin{eqnarray}\unicode[STIX]{x1D6F7}:D(S)\rightarrow D(A^{op}-\text{mod})\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6F7}:D(S)\rightarrow D(A^{op}-\text{mod})\end{eqnarray}$$
by
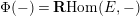 $\unicode[STIX]{x1D6F7}(-)=\mathbf{R}\text{Hom}(E,-)$
, where
$\unicode[STIX]{x1D6F7}(-)=\mathbf{R}\text{Hom}(E,-)$
, where
 $D(A^{op}-\text{mod})$
is the bounded derived category of right
$D(A^{op}-\text{mod})$
is the bounded derived category of right
![]() $A$
-modules with finite-dimensional cohomology. This gives an equivalence of triangulated categories (cf. [Reference BondalBo, Theorem 6.2]).
$A$
-modules with finite-dimensional cohomology. This gives an equivalence of triangulated categories (cf. [Reference BondalBo, Theorem 6.2]).
The algebra
![]() $A^{op}$
is isomorphic to the path algebra of a quiver modulo a certain ideal. We define quivers
$A^{op}$
is isomorphic to the path algebra of a quiver modulo a certain ideal. We define quivers
![]() $\tilde{Q}^{\unicode[STIX]{x1D6FC}}$
,
$\tilde{Q}^{\unicode[STIX]{x1D6FC}}$
,
![]() $\tilde{Q}^{\unicode[STIX]{x1D6FD}}$
and
$\tilde{Q}^{\unicode[STIX]{x1D6FD}}$
and
![]() $\tilde{Q}^{\unicode[STIX]{x1D6FE}}$
as follows.
$\tilde{Q}^{\unicode[STIX]{x1D6FE}}$
as follows.
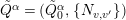 $\tilde{Q}^{\unicode[STIX]{x1D6FC}}=(\tilde{Q}_{0}^{\unicode[STIX]{x1D6FC}},\{N_{v,v^{\prime }}\})$
is defined by
$\tilde{Q}^{\unicode[STIX]{x1D6FC}}=(\tilde{Q}_{0}^{\unicode[STIX]{x1D6FC}},\{N_{v,v^{\prime }}\})$
is defined by
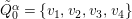 $\tilde{Q}_{0}^{\unicode[STIX]{x1D6FC}}=\{v_{1},v_{2},v_{3},v_{4}\}$
and
$\tilde{Q}_{0}^{\unicode[STIX]{x1D6FC}}=\{v_{1},v_{2},v_{3},v_{4}\}$
and
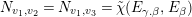 $N_{v_{1},v_{2}}=N_{v_{1},v_{3}}=\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},E_{\unicode[STIX]{x1D6FD}})$
,
$N_{v_{1},v_{2}}=N_{v_{1},v_{3}}=\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},E_{\unicode[STIX]{x1D6FD}})$
,
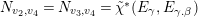 $N_{v_{2},v_{4}}=N_{v_{3},v_{4}}=\tilde{\unicode[STIX]{x1D712}}^{\ast }(E_{\unicode[STIX]{x1D6FE}},E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}})$
and
$N_{v_{2},v_{4}}=N_{v_{3},v_{4}}=\tilde{\unicode[STIX]{x1D712}}^{\ast }(E_{\unicode[STIX]{x1D6FE}},E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}})$
and
 $N_{v_{i},v_{j}}=0$
for other pairs
$N_{v_{i},v_{j}}=0$
for other pairs
![]() $(v_{i},v_{j})$
.
$(v_{i},v_{j})$
.
 $\tilde{Q}^{\unicode[STIX]{x1D6FD}}=(\tilde{Q}_{0}^{\unicode[STIX]{x1D6FC}},\{N_{v,v^{\prime }}\})$
is defined by
$\tilde{Q}^{\unicode[STIX]{x1D6FD}}=(\tilde{Q}_{0}^{\unicode[STIX]{x1D6FC}},\{N_{v,v^{\prime }}\})$
is defined by
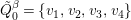 $\tilde{Q}_{0}^{\unicode[STIX]{x1D6FD}}=\{v_{1},v_{2},v_{3},v_{4}\}$
and
$\tilde{Q}_{0}^{\unicode[STIX]{x1D6FD}}=\{v_{1},v_{2},v_{3},v_{4}\}$
and
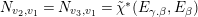 $N_{v_{2},v_{1}}=N_{v_{3},v_{1}}=\tilde{\unicode[STIX]{x1D712}}^{\ast }(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},E_{\unicode[STIX]{x1D6FD}})$
,
$N_{v_{2},v_{1}}=N_{v_{3},v_{1}}=\tilde{\unicode[STIX]{x1D712}}^{\ast }(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},E_{\unicode[STIX]{x1D6FD}})$
,
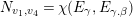 $N_{v_{1},v_{4}}=\unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FE}},E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}})$
and
$N_{v_{1},v_{4}}=\unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FE}},E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}})$
and
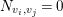 $N_{v_{i},v_{j}}=0$
for other pairs
$N_{v_{i},v_{j}}=0$
for other pairs
![]() $(v_{i},v_{j})$
.
$(v_{i},v_{j})$
.
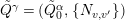 $\tilde{Q}^{\unicode[STIX]{x1D6FE}}=(\tilde{Q}_{0}^{\unicode[STIX]{x1D6FC}},\{N_{v,v^{\prime }}\})$
is defined by
$\tilde{Q}^{\unicode[STIX]{x1D6FE}}=(\tilde{Q}_{0}^{\unicode[STIX]{x1D6FC}},\{N_{v,v^{\prime }}\})$
is defined by
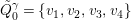 $\tilde{Q}_{0}^{\unicode[STIX]{x1D6FE}}=\{v_{1},v_{2},v_{3},v_{4}\}$
and
$\tilde{Q}_{0}^{\unicode[STIX]{x1D6FE}}=\{v_{1},v_{2},v_{3},v_{4}\}$
and
 $N_{v_{1},v_{2}}=\unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},E_{\unicode[STIX]{x1D6FD}})$
,
$N_{v_{1},v_{2}}=\unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},E_{\unicode[STIX]{x1D6FD}})$
,
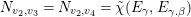 $N_{v_{2},v_{3}}=N_{v_{2},v_{4}}=\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FE}},E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}})$
and
$N_{v_{2},v_{3}}=N_{v_{2},v_{4}}=\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FE}},E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}})$
and
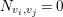 $N_{v_{i},v_{j}}=0$
for other pairs
$N_{v_{i},v_{j}}=0$
for other pairs
![]() $(v_{i},v_{j})$
. (See Figures 7–9.)
$(v_{i},v_{j})$
. (See Figures 7–9.)

Figure 7.
![]() $\tilde{Q}^{\unicode[STIX]{x1D6FC}}$
.
$\tilde{Q}^{\unicode[STIX]{x1D6FC}}$
.

Figure 8.
![]() $\tilde{Q}^{\unicode[STIX]{x1D6FD}}$
.
$\tilde{Q}^{\unicode[STIX]{x1D6FD}}$
.

Figure 9.
![]() $\tilde{Q}^{\unicode[STIX]{x1D6FE}}$
.
$\tilde{Q}^{\unicode[STIX]{x1D6FE}}$
.
When
![]() $E_{\unicode[STIX]{x1D6FC}}$
(resp.
$E_{\unicode[STIX]{x1D6FC}}$
(resp.
![]() $E_{\unicode[STIX]{x1D6FD}}$
) is even, the algebra
$E_{\unicode[STIX]{x1D6FD}}$
) is even, the algebra
![]() $A^{op}$
is isomorphic to the path algebra
$A^{op}$
is isomorphic to the path algebra
![]() $A(\tilde{Q}^{\unicode[STIX]{x1D6FC}})$
(resp.
$A(\tilde{Q}^{\unicode[STIX]{x1D6FC}})$
(resp.
![]() $A(\tilde{Q}^{\unicode[STIX]{x1D6FD}})$
) modulo an ideal generated by linear combinations of paths from
$A(\tilde{Q}^{\unicode[STIX]{x1D6FD}})$
) modulo an ideal generated by linear combinations of paths from
![]() $v_{1}$
to
$v_{1}$
to
![]() $v_{4}$
. When
$v_{4}$
. When
![]() $E_{\unicode[STIX]{x1D6FE}}$
is even, the algebra
$E_{\unicode[STIX]{x1D6FE}}$
is even, the algebra
![]() $A^{op}$
is isomorphic to the path algebra
$A^{op}$
is isomorphic to the path algebra
![]() $A(\tilde{Q}^{\unicode[STIX]{x1D6FE}})$
modulo an ideal generated by linear combinations of paths from
$A(\tilde{Q}^{\unicode[STIX]{x1D6FE}})$
modulo an ideal generated by linear combinations of paths from
![]() $v_{1}$
to
$v_{1}$
to
![]() $v_{4}$
, and from
$v_{4}$
, and from
![]() $v_{1}$
to
$v_{1}$
to
![]() $v_{3}$
.
$v_{3}$
.
Proof of Theorem 5.4.
We give the proof of Theorem 5.4 in case
![]() $E_{\unicode[STIX]{x1D6FC}}$
is even. The other cases can be handled similarly.
$E_{\unicode[STIX]{x1D6FC}}$
is even. The other cases can be handled similarly.
The objects
![]() $E_{\unicode[STIX]{x1D6FD}-2}[2]$
,
$E_{\unicode[STIX]{x1D6FD}-2}[2]$
,
![]() $E_{\unicode[STIX]{x1D6FC}}^{\prime }[1]$
,
$E_{\unicode[STIX]{x1D6FC}}^{\prime }[1]$
,
![]() $E_{\unicode[STIX]{x1D6FC}}^{\prime \prime }[1]$
and
$E_{\unicode[STIX]{x1D6FC}}^{\prime \prime }[1]$
and
![]() $E_{\unicode[STIX]{x1D6FE}}$
in
$E_{\unicode[STIX]{x1D6FE}}$
in
![]() ${\mathcal{A}}[\unicode[STIX]{x1D719}]$
are mapped, via
${\mathcal{A}}[\unicode[STIX]{x1D719}]$
are mapped, via
![]() $\unicode[STIX]{x1D6F7}$
, to simple
$\unicode[STIX]{x1D6F7}$
, to simple
![]() $A(\tilde{Q}^{\unicode[STIX]{x1D6FC}})$
-modules with dimension vector
$A(\tilde{Q}^{\unicode[STIX]{x1D6FC}})$
-modules with dimension vector
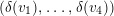 $(\unicode[STIX]{x1D6FF}(v_{1}),\ldots ,\unicode[STIX]{x1D6FF}(v_{4}))$
equal to
$(\unicode[STIX]{x1D6FF}(v_{1}),\ldots ,\unicode[STIX]{x1D6FF}(v_{4}))$
equal to
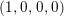 $(1,0,0,0)$
,
$(1,0,0,0)$
,
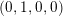 $(0,1,0,0)$
,
$(0,1,0,0)$
,
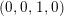 $(0,0,1,0)$
and
$(0,0,1,0)$
and
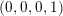 $(0,0,0,1)$
, respectively. These simple modules generate the abelian category
$(0,0,0,1)$
, respectively. These simple modules generate the abelian category
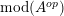 $\text{mod}(A^{op})$
of finite- dimensional
$\text{mod}(A^{op})$
of finite- dimensional
![]() $A^{op}$
-modules. We have
$A^{op}$
-modules. We have
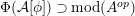 $\unicode[STIX]{x1D6F7}({\mathcal{A}}[\unicode[STIX]{x1D719}])\supset \text{mod}(A^{op})$
. Since both abelian categories
$\unicode[STIX]{x1D6F7}({\mathcal{A}}[\unicode[STIX]{x1D719}])\supset \text{mod}(A^{op})$
. Since both abelian categories
![]() ${\mathcal{A}}[\unicode[STIX]{x1D719}]$
and
${\mathcal{A}}[\unicode[STIX]{x1D719}]$
and
 $\text{mod}(A^{op})$
are hearts of a
$\text{mod}(A^{op})$
are hearts of a
![]() $t$
-structure, we have
$t$
-structure, we have
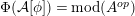 $\unicode[STIX]{x1D6F7}({\mathcal{A}}[\unicode[STIX]{x1D719}])=\text{mod}(A^{op})$
. So the
$\unicode[STIX]{x1D6F7}({\mathcal{A}}[\unicode[STIX]{x1D719}])=\text{mod}(A^{op})$
. So the
![]() $Z[\unicode[STIX]{x1D719}]$
-semistable objects in
$Z[\unicode[STIX]{x1D719}]$
-semistable objects in
![]() ${\mathcal{A}}[\unicode[STIX]{x1D719}]$
correspond to
${\mathcal{A}}[\unicode[STIX]{x1D719}]$
correspond to
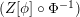 $(Z[\unicode[STIX]{x1D719}]\circ \unicode[STIX]{x1D6F7}^{-1})$
-semistable objects in
$(Z[\unicode[STIX]{x1D719}]\circ \unicode[STIX]{x1D6F7}^{-1})$
-semistable objects in
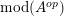 $\text{mod}(A^{op})$
.
$\text{mod}(A^{op})$
.
Consider a complex
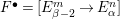 $F^{\bullet }=[E_{\unicode[STIX]{x1D6FD}-2}^{m}\rightarrow E_{\unicode[STIX]{x1D6FC}}^{n}]$
, where
$F^{\bullet }=[E_{\unicode[STIX]{x1D6FD}-2}^{m}\rightarrow E_{\unicode[STIX]{x1D6FC}}^{n}]$
, where
![]() $m,n$
are those in (5.5), and
$m,n$
are those in (5.5), and
![]() $E_{\unicode[STIX]{x1D6FC}}^{n}$
is in degree
$E_{\unicode[STIX]{x1D6FC}}^{n}$
is in degree
![]() $0$
. Then
$0$
. Then
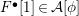 $F^{\bullet }[1]\in {\mathcal{A}}[\unicode[STIX]{x1D719}]$
and
$F^{\bullet }[1]\in {\mathcal{A}}[\unicode[STIX]{x1D719}]$
and
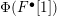 $\unicode[STIX]{x1D6F7}(F^{\bullet }[1])$
is a representation of the quiver
$\unicode[STIX]{x1D6F7}(F^{\bullet }[1])$
is a representation of the quiver
![]() $\tilde{Q}^{\unicode[STIX]{x1D6FC}}$
with dimension vector
$\tilde{Q}^{\unicode[STIX]{x1D6FC}}$
with dimension vector
 $(\unicode[STIX]{x1D6FF}(v_{1}),\ldots ,\unicode[STIX]{x1D6FF}(v_{4}))$
equal to
$(\unicode[STIX]{x1D6FF}(v_{1}),\ldots ,\unicode[STIX]{x1D6FF}(v_{4}))$
equal to
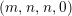 $(m,n,n,0)$
, which can be regarded as a representation of the quiver
$(m,n,n,0)$
, which can be regarded as a representation of the quiver
![]() $Q^{\unicode[STIX]{x1D6FC}}$
with dimension vector
$Q^{\unicode[STIX]{x1D6FC}}$
with dimension vector
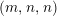 $(m,n,n)$
.
$(m,n,n)$
.
Put
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FC}}:=Z_{(s,t)}(E_{\unicode[STIX]{x1D6FC}}),\qquad \unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FD}}:=Z_{(s,t)}(E_{\unicode[STIX]{x1D6FD}-2}[1]),\qquad \unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FE}}:=Z_{(s,t)}(E_{\unicode[STIX]{x1D6FE}}). & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FC}}:=Z_{(s,t)}(E_{\unicode[STIX]{x1D6FC}}),\qquad \unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FD}}:=Z_{(s,t)}(E_{\unicode[STIX]{x1D6FD}-2}[1]),\qquad \unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FE}}:=Z_{(s,t)}(E_{\unicode[STIX]{x1D6FE}}). & & \displaystyle \nonumber\end{eqnarray}$$
Then we have
 $$\begin{eqnarray}\displaystyle & \displaystyle Z[\unicode[STIX]{x1D719}](E_{\unicode[STIX]{x1D6FC}}^{\prime }[1])=Z[\unicode[STIX]{x1D719}](E_{\unicode[STIX]{x1D6FC}}^{\prime \prime }[1])=-{\textstyle \frac{1}{2}}e^{-i\unicode[STIX]{x1D70B}\unicode[STIX]{x1D719}}\unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FC}} & \displaystyle \nonumber\\ \displaystyle & \displaystyle Z[\unicode[STIX]{x1D719}](E_{\unicode[STIX]{x1D6FD}-2}[2])=-e^{i\unicode[STIX]{x1D70B}\unicode[STIX]{x1D719}}\unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FD}},\qquad Z[\unicode[STIX]{x1D719}](E_{\unicode[STIX]{x1D6FE}})=e^{-i\unicode[STIX]{x1D70B}\unicode[STIX]{x1D719}}\unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FE}}. & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle Z[\unicode[STIX]{x1D719}](E_{\unicode[STIX]{x1D6FC}}^{\prime }[1])=Z[\unicode[STIX]{x1D719}](E_{\unicode[STIX]{x1D6FC}}^{\prime \prime }[1])=-{\textstyle \frac{1}{2}}e^{-i\unicode[STIX]{x1D70B}\unicode[STIX]{x1D719}}\unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FC}} & \displaystyle \nonumber\\ \displaystyle & \displaystyle Z[\unicode[STIX]{x1D719}](E_{\unicode[STIX]{x1D6FD}-2}[2])=-e^{i\unicode[STIX]{x1D70B}\unicode[STIX]{x1D719}}\unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FD}},\qquad Z[\unicode[STIX]{x1D719}](E_{\unicode[STIX]{x1D6FE}})=e^{-i\unicode[STIX]{x1D70B}\unicode[STIX]{x1D719}}\unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FE}}. & \displaystyle \nonumber\end{eqnarray}$$
The representation
![]() $\unicode[STIX]{x1D6F7}(F^{\bullet })$
of the quiver
$\unicode[STIX]{x1D6F7}(F^{\bullet })$
of the quiver
![]() $Q^{\unicode[STIX]{x1D6FC}}$
is
$Q^{\unicode[STIX]{x1D6FC}}$
is
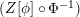 $(Z[\unicode[STIX]{x1D719}]\circ \unicode[STIX]{x1D6F7}^{-1})$
-semistable if and only if for any subrepresentation
$(Z[\unicode[STIX]{x1D719}]\circ \unicode[STIX]{x1D6F7}^{-1})$
-semistable if and only if for any subrepresentation
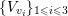 $\{V_{v_{i}}\}_{1\leqslant i\leqslant 3}$
of
$\{V_{v_{i}}\}_{1\leqslant i\leqslant 3}$
of
![]() $\unicode[STIX]{x1D6F7}(F^{\bullet })$
, the following inequality holds
$\unicode[STIX]{x1D6F7}(F^{\bullet })$
, the following inequality holds
 $$\begin{eqnarray}\text{Im}\frac{-me^{-i\unicode[STIX]{x1D70B}\unicode[STIX]{x1D719}}\unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FD}}-ne^{-i\unicode[STIX]{x1D70B}\unicode[STIX]{x1D719}}\unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FC}}}{-(\dim V_{v_{1}})e^{-i\unicode[STIX]{x1D70B}\unicode[STIX]{x1D719}}\unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FD}}-\frac{1}{2}(\dim V_{v_{2}}+\dim V_{v_{3}})e^{-i\unicode[STIX]{x1D70B}\unicode[STIX]{x1D719}}\unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FC}}}\geqslant 0.\end{eqnarray}$$
$$\begin{eqnarray}\text{Im}\frac{-me^{-i\unicode[STIX]{x1D70B}\unicode[STIX]{x1D719}}\unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FD}}-ne^{-i\unicode[STIX]{x1D70B}\unicode[STIX]{x1D719}}\unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FC}}}{-(\dim V_{v_{1}})e^{-i\unicode[STIX]{x1D70B}\unicode[STIX]{x1D719}}\unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FD}}-\frac{1}{2}(\dim V_{v_{2}}+\dim V_{v_{3}})e^{-i\unicode[STIX]{x1D70B}\unicode[STIX]{x1D719}}\unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FC}}}\geqslant 0.\end{eqnarray}$$
Using
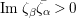 $\text{Im}\,\unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FD}}\bar{\unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FC}}}>0$
, one can see that the inequality (5.17) is equivalent to
$\text{Im}\,\unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FD}}\bar{\unicode[STIX]{x1D701}_{\unicode[STIX]{x1D6FC}}}>0$
, one can see that the inequality (5.17) is equivalent to
 $$\begin{eqnarray}m(\dim V_{v_{2}}+\dim V_{v_{3}})-2n\dim V_{v_{1}}\geqslant 0.\end{eqnarray}$$
$$\begin{eqnarray}m(\dim V_{v_{2}}+\dim V_{v_{3}})-2n\dim V_{v_{1}}\geqslant 0.\end{eqnarray}$$
This shows that
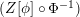 $(Z[\unicode[STIX]{x1D719}]\circ \unicode[STIX]{x1D6F7}^{-1})$
-semistability is equivalent to
$(Z[\unicode[STIX]{x1D719}]\circ \unicode[STIX]{x1D6F7}^{-1})$
-semistability is equivalent to
 $(-2n,m,m)$
-semistability of a representation of the quiver
$(-2n,m,m)$
-semistability of a representation of the quiver
![]() $Q^{\unicode[STIX]{x1D6FC}}$
.
$Q^{\unicode[STIX]{x1D6FC}}$
.
All in all, by associating
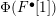 $\unicode[STIX]{x1D6F7}(F^{\bullet }[1])$
to
$\unicode[STIX]{x1D6F7}(F^{\bullet }[1])$
to
![]() $F^{\bullet }$
, we have an isomorphism
$F^{\bullet }$
, we have an isomorphism
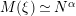 $M(\unicode[STIX]{x1D709})\simeq N^{\unicode[STIX]{x1D6FC}}$
. This completes the proof of Theorem 5.4.◻
$M(\unicode[STIX]{x1D709})\simeq N^{\unicode[STIX]{x1D6FC}}$
. This completes the proof of Theorem 5.4.◻
Remark 5.5. To
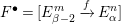 $F^{\bullet }=[E_{\unicode[STIX]{x1D6FD}-2}^{m}\xrightarrow[{}]{f}E_{\unicode[STIX]{x1D6FC}}^{n}]$
, we associated
$F^{\bullet }=[E_{\unicode[STIX]{x1D6FD}-2}^{m}\xrightarrow[{}]{f}E_{\unicode[STIX]{x1D6FC}}^{n}]$
, we associated
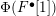 $\unicode[STIX]{x1D6F7}(F^{\bullet }[1])$
, a representation of a quiver. Here is another way to associate the representation of the quiver. Assume, for example, that
$\unicode[STIX]{x1D6F7}(F^{\bullet }[1])$
, a representation of a quiver. Here is another way to associate the representation of the quiver. Assume, for example, that
![]() $\unicode[STIX]{x1D6FC}$
is even, and
$\unicode[STIX]{x1D6FC}$
is even, and
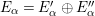 $E_{\unicode[STIX]{x1D6FC}}=E_{\unicode[STIX]{x1D6FC}}^{\prime }\oplus E_{\unicode[STIX]{x1D6FC}}^{\prime \prime }$
. We can regard
$E_{\unicode[STIX]{x1D6FC}}=E_{\unicode[STIX]{x1D6FC}}^{\prime }\oplus E_{\unicode[STIX]{x1D6FC}}^{\prime \prime }$
. We can regard
![]() $f$
as an element of
$f$
as an element of
 $$\begin{eqnarray}\text{Hom}(\mathbb{C}^{m},\mathbb{C}^{n})\otimes \left(\text{Hom}(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}^{\prime })\oplus \text{Hom}(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}^{\prime \prime })\right)\!.\end{eqnarray}$$
$$\begin{eqnarray}\text{Hom}(\mathbb{C}^{m},\mathbb{C}^{n})\otimes \left(\text{Hom}(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}^{\prime })\oplus \text{Hom}(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}^{\prime \prime })\right)\!.\end{eqnarray}$$
We can see that there is a canonical isomorphism
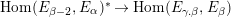 $\text{Hom}(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}})^{\ast }\rightarrow \text{Hom}(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},E_{\unicode[STIX]{x1D6FD}})$
compatible with the direct sum decompositions
$\text{Hom}(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}})^{\ast }\rightarrow \text{Hom}(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},E_{\unicode[STIX]{x1D6FD}})$
compatible with the direct sum decompositions
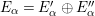 $E_{\unicode[STIX]{x1D6FC}}=E_{\unicode[STIX]{x1D6FC}}^{\prime }\oplus E_{\unicode[STIX]{x1D6FC}}^{\prime \prime }$
and
$E_{\unicode[STIX]{x1D6FC}}=E_{\unicode[STIX]{x1D6FC}}^{\prime }\oplus E_{\unicode[STIX]{x1D6FC}}^{\prime \prime }$
and
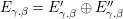 $E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}}=E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}}^{\prime }\oplus E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}}^{\prime \prime }$
. Therefore,
$E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}}=E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}}^{\prime }\oplus E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}}^{\prime \prime }$
. Therefore,
![]() $f$
gives a representation of the quiver
$f$
gives a representation of the quiver
![]() $Q^{\unicode[STIX]{x1D6FC}}$
with dimension vector
$Q^{\unicode[STIX]{x1D6FC}}$
with dimension vector
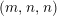 $(m,n,n)$
. We can verify that this representation is isomorphic to
$(m,n,n)$
. We can verify that this representation is isomorphic to
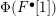 $\unicode[STIX]{x1D6F7}(F^{\bullet }[1])$
.
$\unicode[STIX]{x1D6F7}(F^{\bullet }[1])$
.
5.3 Complements
Some arguments in this section remain valid even for moduli spaces of positive height. Fix
![]() $\unicode[STIX]{x1D6FE}\in \mathfrak{E}$
, and define
$\unicode[STIX]{x1D6FE}\in \mathfrak{E}$
, and define
![]() $\unicode[STIX]{x1D6FC}$
and
$\unicode[STIX]{x1D6FC}$
and
![]() $\unicode[STIX]{x1D6FD}$
as at the beginning of Section 5.1 so that
$\unicode[STIX]{x1D6FD}$
as at the beginning of Section 5.1 so that
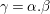 $\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}$
. Let
$\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}$
. Let
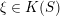 $\unicode[STIX]{x1D709}\in K(S)$
with symmetric
$\unicode[STIX]{x1D709}\in K(S)$
with symmetric
![]() $c_{1}$
and
$c_{1}$
and
 $\text{r}(\unicode[STIX]{x1D709})>0$
. Assume that
$\text{r}(\unicode[STIX]{x1D709})>0$
. Assume that
 $$\begin{eqnarray}\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}}<\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})\qquad \text{and}\qquad \unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FE}},\unicode[STIX]{x1D709})=0.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}}<\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})\qquad \text{and}\qquad \unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FE}},\unicode[STIX]{x1D709})=0.\end{eqnarray}$$
We can easily check the condition in Theorem 3.7(2), so
![]() $\unicode[STIX]{x1D709}$
is a semistable class. If moreover
$\unicode[STIX]{x1D709}$
is a semistable class. If moreover
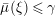 $\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})\leqslant \unicode[STIX]{x1D6FE}$
, then the moduli space
$\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})\leqslant \unicode[STIX]{x1D6FE}$
, then the moduli space
![]() $M(\unicode[STIX]{x1D709})$
is of height zero and isomorphic to one of
$M(\unicode[STIX]{x1D709})$
is of height zero and isomorphic to one of
![]() $N^{\unicode[STIX]{x1D6FC}}$
,
$N^{\unicode[STIX]{x1D6FC}}$
,
![]() $N^{\unicode[STIX]{x1D6FD}}$
,
$N^{\unicode[STIX]{x1D6FD}}$
,
![]() $N^{\unicode[STIX]{x1D6FE}}$
. But even if
$N^{\unicode[STIX]{x1D6FE}}$
. But even if
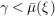 $\unicode[STIX]{x1D6FE}<\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})$
, we can see that
$\unicode[STIX]{x1D6FE}<\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})$
, we can see that
![]() $M(\unicode[STIX]{x1D709})$
is birational to one of
$M(\unicode[STIX]{x1D709})$
is birational to one of
![]() $N^{\unicode[STIX]{x1D6FC}}$
,
$N^{\unicode[STIX]{x1D6FC}}$
,
![]() $N^{\unicode[STIX]{x1D6FD}}$
,
$N^{\unicode[STIX]{x1D6FD}}$
,
![]() $N^{\unicode[STIX]{x1D6FE}}$
as follows.
$N^{\unicode[STIX]{x1D6FE}}$
as follows.
Under the condition (5.18), we have
 $$\begin{eqnarray}m:=-\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FD}},\unicode[STIX]{x1D709})>0\qquad \text{and}\qquad n:=-\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},\unicode[STIX]{x1D709})>0.\end{eqnarray}$$
$$\begin{eqnarray}m:=-\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FD}},\unicode[STIX]{x1D709})>0\qquad \text{and}\qquad n:=-\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},\unicode[STIX]{x1D709})>0.\end{eqnarray}$$
(This follows by verifying that the
![]() $\bar{\unicode[STIX]{x1D707}}$
-coordinate of the intersection of the parabolas
$\bar{\unicode[STIX]{x1D707}}$
-coordinate of the intersection of the parabolas
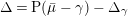 $\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}$
and
$\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}$
and
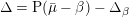 $\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FD})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}$
(resp.
$\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FD})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FD}}$
(resp.
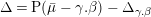 $\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}}$
) is smaller than
$\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}}$
) is smaller than
![]() $\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}}$
.) Put
$\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}}$
.) Put
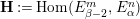 $\mathbf{H}:=\text{Hom}(E_{\unicode[STIX]{x1D6FD}-2}^{m},E_{\unicode[STIX]{x1D6FC}}^{n})$
, and define
$\mathbf{H}:=\text{Hom}(E_{\unicode[STIX]{x1D6FD}-2}^{m},E_{\unicode[STIX]{x1D6FC}}^{n})$
, and define
![]() $U\subset \mathbf{H}$
to be the open subset consisting of such
$U\subset \mathbf{H}$
to be the open subset consisting of such
 $\unicode[STIX]{x1D711}:E_{\unicode[STIX]{x1D6FD}-2}^{m}\rightarrow E_{\unicode[STIX]{x1D6FC}}^{n}$
that
$\unicode[STIX]{x1D711}:E_{\unicode[STIX]{x1D6FD}-2}^{m}\rightarrow E_{\unicode[STIX]{x1D6FC}}^{n}$
that
![]() $\unicode[STIX]{x1D711}$
is injective and
$\unicode[STIX]{x1D711}$
is injective and
 $\text{Coker}\,\unicode[STIX]{x1D711}$
is torsion-free. By a standard dimension estimate, we can see that
$\text{Coker}\,\unicode[STIX]{x1D711}$
is torsion-free. By a standard dimension estimate, we can see that
![]() $U\neq \emptyset$
. We can check that the family
$U\neq \emptyset$
. We can check that the family
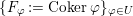 $\{F_{\unicode[STIX]{x1D711}}:=\text{Coker}\,\unicode[STIX]{x1D711}\}_{\unicode[STIX]{x1D711}\in U}$
is a complete family such that
$\{F_{\unicode[STIX]{x1D711}}:=\text{Coker}\,\unicode[STIX]{x1D711}\}_{\unicode[STIX]{x1D711}\in U}$
is a complete family such that
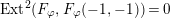 $\text{Ext}^{2}(F_{\unicode[STIX]{x1D711}},F_{\unicode[STIX]{x1D711}}(-1,-1))=0$
. If we put
$\text{Ext}^{2}(F_{\unicode[STIX]{x1D711}},F_{\unicode[STIX]{x1D711}}(-1,-1))=0$
. If we put
 $$\begin{eqnarray}U\supset U^{\prime }:=\left\{\unicode[STIX]{x1D711}\in U\mid F_{\unicode[STIX]{x1D711}}\text{ is stable}\right\}\!,\end{eqnarray}$$
$$\begin{eqnarray}U\supset U^{\prime }:=\left\{\unicode[STIX]{x1D711}\in U\mid F_{\unicode[STIX]{x1D711}}\text{ is stable}\right\}\!,\end{eqnarray}$$
then we can see that
![]() $U^{\prime }\neq \emptyset$
(repeat the argument of the proof of Theorem 3.7). In the proof of Proposition 5.2, we only used the fact
$U^{\prime }\neq \emptyset$
(repeat the argument of the proof of Theorem 3.7). In the proof of Proposition 5.2, we only used the fact
-
∙
 $\unicode[STIX]{x1D6FC}<\bar{\unicode[STIX]{x1D707}}(F)$
,
$\unicode[STIX]{x1D6FC}<\bar{\unicode[STIX]{x1D707}}(F)$
, -
∙
 $F$
is a (semi)stable sheaf fitting in a short exact sequence (5.4).
$F$
is a (semi)stable sheaf fitting in a short exact sequence (5.4).
So by the same proof, we obtain the following.
Proposition 5.6. If a point
![]() $(s_{0},t_{0})$
with
$(s_{0},t_{0})$
with
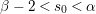 $\unicode[STIX]{x1D6FD}-2<s_{0}<\unicode[STIX]{x1D6FC}$
in the upper half
$\unicode[STIX]{x1D6FD}-2<s_{0}<\unicode[STIX]{x1D6FC}$
in the upper half
![]() $(s,t)$
-plane lies outside the wall
$(s,t)$
-plane lies outside the wall
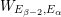 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
, then
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
, then
![]() $F_{\unicode[STIX]{x1D711}}$
is
$F_{\unicode[STIX]{x1D711}}$
is
![]() $(s_{0},t_{0})$
-stable for
$(s_{0},t_{0})$
-stable for
![]() $\unicode[STIX]{x1D711}\in U^{\prime }$
.
$\unicode[STIX]{x1D711}\in U^{\prime }$
.
Denote by
![]() $M(\unicode[STIX]{x1D709})^{\diamond }$
the open subscheme of
$M(\unicode[STIX]{x1D709})^{\diamond }$
the open subscheme of
![]() $M(\unicode[STIX]{x1D709})$
parametrizing stable sheaves fitting in a short exact sequence (5.4). By the same proof of Theorem 5.4, we have the following.
$M(\unicode[STIX]{x1D709})$
parametrizing stable sheaves fitting in a short exact sequence (5.4). By the same proof of Theorem 5.4, we have the following.
Proposition 5.7. Let
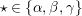 $\star \in \{\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FE}\}$
. If the symmetric exceptional bundle
$\star \in \{\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FE}\}$
. If the symmetric exceptional bundle
![]() $E_{\star }$
is even, then
$E_{\star }$
is even, then
![]() $M(\unicode[STIX]{x1D709})^{\diamond }$
is isomorphic to an open subscheme of
$M(\unicode[STIX]{x1D709})^{\diamond }$
is isomorphic to an open subscheme of
![]() $N^{\star s}$
, where
$N^{\star s}$
, where
![]() $N^{\star s}$
is the subscheme of
$N^{\star s}$
is the subscheme of
![]() $N^{\star }$
consisting of
$N^{\star }$
consisting of
![]() $\unicode[STIX]{x1D703}$
-stable representations. In particular,
$\unicode[STIX]{x1D703}$
-stable representations. In particular,
![]() $N^{\star s}$
is nonempty.
$N^{\star s}$
is nonempty.
5.4 Torsion-sheaves
We can also apply the same arguments in the preceding section to torsion-sheaves. Fix a K-class
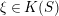 $\unicode[STIX]{x1D709}\in K(S)$
with symmetric
$\unicode[STIX]{x1D709}\in K(S)$
with symmetric
![]() $c_{1}$
such that
$c_{1}$
such that
 $$\begin{eqnarray}\text{r}(\unicode[STIX]{x1D709})=0,\qquad \deg \unicode[STIX]{x1D709}:=c_{1}(\unicode[STIX]{x1D709})\cdot c_{1}(L)>0\qquad \text{and}\qquad \unicode[STIX]{x1D6FE}:=\frac{\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709})}{\deg \unicode[STIX]{x1D709}}\in \mathfrak{E}.\end{eqnarray}$$
$$\begin{eqnarray}\text{r}(\unicode[STIX]{x1D709})=0,\qquad \deg \unicode[STIX]{x1D709}:=c_{1}(\unicode[STIX]{x1D709})\cdot c_{1}(L)>0\qquad \text{and}\qquad \unicode[STIX]{x1D6FE}:=\frac{\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709})}{\deg \unicode[STIX]{x1D709}}\in \mathfrak{E}.\end{eqnarray}$$
Define
![]() $\unicode[STIX]{x1D6FC}$
and
$\unicode[STIX]{x1D6FC}$
and
![]() $\unicode[STIX]{x1D6FD}$
as at the beginning of Section 5.1. We have
$\unicode[STIX]{x1D6FD}$
as at the beginning of Section 5.1. We have
 $$\begin{eqnarray}m:=-\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FD}},\unicode[STIX]{x1D709})>0\qquad \text{and}\qquad n:=-\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},\unicode[STIX]{x1D709})>0.\end{eqnarray}$$
$$\begin{eqnarray}m:=-\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FD}},\unicode[STIX]{x1D709})>0\qquad \text{and}\qquad n:=-\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},\unicode[STIX]{x1D709})>0.\end{eqnarray}$$
As before we put
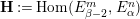 $\mathbf{H}:=\text{Hom}(E_{\unicode[STIX]{x1D6FD}-2}^{m},E_{\unicode[STIX]{x1D6FC}}^{n})$
, and define
$\mathbf{H}:=\text{Hom}(E_{\unicode[STIX]{x1D6FD}-2}^{m},E_{\unicode[STIX]{x1D6FC}}^{n})$
, and define
![]() $U\subset \mathbf{H}$
to be the open subset consisting of such
$U\subset \mathbf{H}$
to be the open subset consisting of such
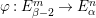 $\unicode[STIX]{x1D711}:E_{\unicode[STIX]{x1D6FD}-2}^{m}\rightarrow E_{\unicode[STIX]{x1D6FC}}^{n}$
that
$\unicode[STIX]{x1D711}:E_{\unicode[STIX]{x1D6FD}-2}^{m}\rightarrow E_{\unicode[STIX]{x1D6FC}}^{n}$
that
![]() $\unicode[STIX]{x1D711}$
is injective. Then for any
$\unicode[STIX]{x1D711}$
is injective. Then for any
![]() $\unicode[STIX]{x1D711}\in U$
,
$\unicode[STIX]{x1D711}\in U$
,
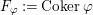 $F_{\unicode[STIX]{x1D711}}:=\text{Coker}\,\unicode[STIX]{x1D711}$
is a semistable pure
$F_{\unicode[STIX]{x1D711}}:=\text{Coker}\,\unicode[STIX]{x1D711}$
is a semistable pure
![]() $1$
-dimensional sheaf. Indeed, since
$1$
-dimensional sheaf. Indeed, since
 $F:=F_{\unicode[STIX]{x1D711}}$
fits in a short exact sequence (5.4), we have
$F:=F_{\unicode[STIX]{x1D711}}$
fits in a short exact sequence (5.4), we have
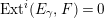 $\text{Ext}^{i}(E_{\unicode[STIX]{x1D6FE}},F)=0$
for any
$\text{Ext}^{i}(E_{\unicode[STIX]{x1D6FE}},F)=0$
for any
![]() $i$
. If
$i$
. If
![]() $F$
has a subsheaf
$F$
has a subsheaf
![]() $F^{\prime }$
with
$F^{\prime }$
with
 $\unicode[STIX]{x1D712}(F^{\prime })/\deg \,F^{\prime }>\unicode[STIX]{x1D6FE}$
, then
$\unicode[STIX]{x1D712}(F^{\prime })/\deg \,F^{\prime }>\unicode[STIX]{x1D6FE}$
, then
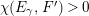 $\unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FE}},F^{\prime })>0$
, thus
$\unicode[STIX]{x1D712}(E_{\unicode[STIX]{x1D6FE}},F^{\prime })>0$
, thus
 $\text{Hom}(E_{\unicode[STIX]{x1D6FE}},F^{\prime })\neq 0$
. This contradicts
$\text{Hom}(E_{\unicode[STIX]{x1D6FE}},F^{\prime })\neq 0$
. This contradicts
 $\text{Hom}(E_{\unicode[STIX]{x1D6FE}},F)=0$
. Put
$\text{Hom}(E_{\unicode[STIX]{x1D6FE}},F)=0$
. Put
 $$\begin{eqnarray}U\supset U^{\prime }:=\left\{\unicode[STIX]{x1D711}\in U\mid F_{\unicode[STIX]{x1D711}}\text{ is stable}\right\}\!.\end{eqnarray}$$
$$\begin{eqnarray}U\supset U^{\prime }:=\left\{\unicode[STIX]{x1D711}\in U\mid F_{\unicode[STIX]{x1D711}}\text{ is stable}\right\}\!.\end{eqnarray}$$
We claim that
![]() $U^{\prime }\neq \emptyset$
. If
$U^{\prime }\neq \emptyset$
. If
 $F:=F_{\unicode[STIX]{x1D711}}$
,
$F:=F_{\unicode[STIX]{x1D711}}$
,
![]() $\unicode[STIX]{x1D711}\in U$
, is not stable, then
$\unicode[STIX]{x1D711}\in U$
, is not stable, then
![]() $F$
has a filtration
$F$
has a filtration
 $$\begin{eqnarray}0=F_{0}\subset \cdots \subset F_{l}=F,\quad l\geqslant 2\end{eqnarray}$$
$$\begin{eqnarray}0=F_{0}\subset \cdots \subset F_{l}=F,\quad l\geqslant 2\end{eqnarray}$$
such that
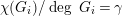 $\unicode[STIX]{x1D712}(G_{i})/\deg \,G_{i}=\unicode[STIX]{x1D6FE}$
for any
$\unicode[STIX]{x1D712}(G_{i})/\deg \,G_{i}=\unicode[STIX]{x1D6FE}$
for any
![]() $i$
, where
$i$
, where
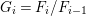 $G_{i}=F_{i}/F_{i-1}$
. We can see, by calculation, that
$G_{i}=F_{i}/F_{i-1}$
. We can see, by calculation, that
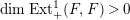 $\dim \text{Ext}_{+}^{1}(F,F)>0$
for this filtration. This implies that
$\dim \text{Ext}_{+}^{1}(F,F)>0$
for this filtration. This implies that
![]() $U^{\prime }\neq \emptyset$
(see the argument in the proof of [Reference Drezet and Le PotierDL, Theorem 4.10]). Adapting the proof of Proposition 5.2, we can obtain the following.
$U^{\prime }\neq \emptyset$
(see the argument in the proof of [Reference Drezet and Le PotierDL, Theorem 4.10]). Adapting the proof of Proposition 5.2, we can obtain the following.
Proposition 5.8. If a point
![]() $(s_{0},t_{0})$
with
$(s_{0},t_{0})$
with
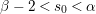 $\unicode[STIX]{x1D6FD}-2<s_{0}<\unicode[STIX]{x1D6FC}$
in the upper half
$\unicode[STIX]{x1D6FD}-2<s_{0}<\unicode[STIX]{x1D6FC}$
in the upper half
![]() $(s,t)$
-plane lies outside the wall
$(s,t)$
-plane lies outside the wall
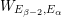 $W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
, then
$W_{E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}}}$
, then
![]() $F_{\unicode[STIX]{x1D711}}$
is
$F_{\unicode[STIX]{x1D711}}$
is
![]() $(s_{0},t_{0})$
-stable for
$(s_{0},t_{0})$
-stable for
![]() $\unicode[STIX]{x1D711}\in U^{\prime }$
.
$\unicode[STIX]{x1D711}\in U^{\prime }$
.
Denote by
![]() $M(\unicode[STIX]{x1D709})^{\diamond }$
the moduli space of stable pure
$M(\unicode[STIX]{x1D709})^{\diamond }$
the moduli space of stable pure
![]() $1$
-dimensional sheaves with K-class
$1$
-dimensional sheaves with K-class
![]() $\unicode[STIX]{x1D709}$
fitting in a short exact sequence (5.4). By arguing as in the proof of Theorem 5.4, we have the following.
$\unicode[STIX]{x1D709}$
fitting in a short exact sequence (5.4). By arguing as in the proof of Theorem 5.4, we have the following.
Proposition 5.9. Let
 $\star \in \{\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FE}\}$
. If the symmetric exceptional bundle
$\star \in \{\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FE}\}$
. If the symmetric exceptional bundle
![]() $E_{\star }$
is even, then
$E_{\star }$
is even, then
![]() $M(\unicode[STIX]{x1D709})^{\diamond }$
is isomorphic to an open subscheme of
$M(\unicode[STIX]{x1D709})^{\diamond }$
is isomorphic to an open subscheme of
![]() $N^{\star s}$
, where
$N^{\star s}$
, where
![]() $N^{\star s}$
is the subscheme of
$N^{\star s}$
is the subscheme of
![]() $N^{\star }$
consisting of
$N^{\star }$
consisting of
![]() $\unicode[STIX]{x1D703}$
-stable representations. In particular,
$\unicode[STIX]{x1D703}$
-stable representations. In particular,
![]() $N^{\star s}$
is nonempty.
$N^{\star s}$
is nonempty.
6 Dimension Estimate
The following proposition is the quadric surface counterpart of [Reference Le PotierLe, Lemma 18.3.1].
Proposition 6.1. Let
 $\unicode[STIX]{x1D709}\in K(S)$
be a semistable class with symmetric
$\unicode[STIX]{x1D709}\in K(S)$
be a semistable class with symmetric
![]() $c_{1}$
such that the height of the moduli space
$c_{1}$
such that the height of the moduli space
![]() $M(\unicode[STIX]{x1D709})$
is positive. Consider a complete family
$M(\unicode[STIX]{x1D709})$
is positive. Consider a complete family
![]() $\{F_{t}\}$
of torsion-free coherent sheaves on
$\{F_{t}\}$
of torsion-free coherent sheaves on
![]() $S$
with K-class
$S$
with K-class
![]() $\unicode[STIX]{x1D709}$
parametrized by a smooth variety
$\unicode[STIX]{x1D709}$
parametrized by a smooth variety
![]() $T$
. Assume that
$T$
. Assume that
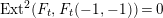 $\text{Ext}^{2}(F_{t},F_{t}(-1,-1))=0$
for any
$\text{Ext}^{2}(F_{t},F_{t}(-1,-1))=0$
for any
![]() $t\in T$
.
$t\in T$
.
-
(1) The set of points
 $t\in T$
such that
$t\in T$
such that
 $F_{t}$
is not stable forms a closed subset of at least codimension
$F_{t}$
is not stable forms a closed subset of at least codimension
 $2$
.
$2$
. -
(2) If
 $\text{r}(\unicode[STIX]{x1D709})\geqslant 3$
, then the set of points
$\text{r}(\unicode[STIX]{x1D709})\geqslant 3$
, then the set of points
 $t\in T$
such that
$t\in T$
such that
 $F_{t}$
is not a
$F_{t}$
is not a
 $\unicode[STIX]{x1D707}$
-stable bundle forms a closed subset of at least codimension
$\unicode[STIX]{x1D707}$
-stable bundle forms a closed subset of at least codimension
 $2$
.
$2$
.
Only in the proof of the proposition, we use the terminology “multistable”: a torsion-free sheaf
![]() $F$
is said to be multistable if
$F$
is said to be multistable if
![]() $F$
is semistable and
$F$
is semistable and
![]() $S$
-equivalent to
$S$
-equivalent to
![]() $G^{a}$
with
$G^{a}$
with
![]() $G$
a stable sheaf.
$G$
a stable sheaf.
Proof. (1) By a similar argument as in the proof of [Reference Le PotierLe, Corollary 15.4.4], we can see that the set of points
![]() $t\in T$
such that
$t\in T$
such that
 $\unicode[STIX]{x1D707}_{\max }(F_{t})-\unicode[STIX]{x1D707}_{\min }(F_{t})>2$
forms a closed subset of codimension at least
$\unicode[STIX]{x1D707}_{\max }(F_{t})-\unicode[STIX]{x1D707}_{\min }(F_{t})>2$
forms a closed subset of codimension at least
![]() $2$
. So we may assume that
$2$
. So we may assume that
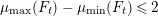 $\unicode[STIX]{x1D707}_{\max }(F_{t})-\unicode[STIX]{x1D707}_{\min }(F_{t})\leqslant 2$
for any
$\unicode[STIX]{x1D707}_{\max }(F_{t})-\unicode[STIX]{x1D707}_{\min }(F_{t})\leqslant 2$
for any
![]() $t\in T$
.
$t\in T$
.
We first show that the set of points
![]() $t\in T$
such that
$t\in T$
such that
![]() $F_{t}$
is not semistable is of codimension at least
$F_{t}$
is not semistable is of codimension at least
![]() $2$
. To this end, it suffices to show that if
$2$
. To this end, it suffices to show that if
![]() $F=F_{t}$
is not semistable, then there exists a filtration
$F=F_{t}$
is not semistable, then there exists a filtration
 $$\begin{eqnarray}0=F_{0}\subset F_{1}\subset \cdots \subset F_{l}=F\end{eqnarray}$$
$$\begin{eqnarray}0=F_{0}\subset F_{1}\subset \cdots \subset F_{l}=F\end{eqnarray}$$
satisfying the following conditions (i), (ii) and (iii):
-
(i)
 $G_{i}:=F_{i}/F_{i-1}$
is semistable,
$G_{i}:=F_{i}/F_{i-1}$
is semistable,
 $p_{G_{1}}\succcurlyeq \cdots \succcurlyeq p_{G_{l}}$
,
$p_{G_{1}}\succcurlyeq \cdots \succcurlyeq p_{G_{l}}$
,
 $p_{G_{1}}\succ p_{G_{l}}$
, and
$p_{G_{1}}\succ p_{G_{l}}$
, and
 $\bar{\unicode[STIX]{x1D707}}(G_{1})-\bar{\unicode[STIX]{x1D707}}(G_{l})\leqslant 1$
;
$\bar{\unicode[STIX]{x1D707}}(G_{1})-\bar{\unicode[STIX]{x1D707}}(G_{l})\leqslant 1$
; -
(ii)
 $\text{Hom}(G_{i},G_{j})=0$
for
$\text{Hom}(G_{i},G_{j})=0$
for
 $i<j$
;
$i<j$
; -
(iii)
 $\sum _{i<j}\unicode[STIX]{x1D712}(G_{i},G_{j})<-1$
.
$\sum _{i<j}\unicode[STIX]{x1D712}(G_{i},G_{j})<-1$
.
In fact, if the filtration (6.1) satisfies (i), (ii) and (iii), then
 $$\begin{eqnarray}\dim \text{Ext}_{+}^{1}(F,F)=-\mathop{\sum }_{i<j}\unicode[STIX]{x1D712}(G_{i},G_{j})\geqslant 2.\end{eqnarray}$$
$$\begin{eqnarray}\dim \text{Ext}_{+}^{1}(F,F)=-\mathop{\sum }_{i<j}\unicode[STIX]{x1D712}(G_{i},G_{j})\geqslant 2.\end{eqnarray}$$
Using [Reference Drezet and Le PotierDL, Propositions 1.5 and 1.7], we see that the subset of sheaves having a filtration satisfying (i), (ii) and (iii) is of codimension at least
![]() $2$
.
$2$
.
In the following, we shall use repeatedly the fact that for the filtration (6.1) satisfying (i) and (ii), we have
 $\unicode[STIX]{x1D712}(G_{i},G_{j})\leqslant 0$
for
$\unicode[STIX]{x1D712}(G_{i},G_{j})\leqslant 0$
for
![]() $i<j$
.
$i<j$
.
Step 1. We show that if
![]() $F=F_{t}$
has a filtration (6.1) satisfying the conditions (i), (ii) and the following condition (iv), then (iii) or (v) below holds.
$F=F_{t}$
has a filtration (6.1) satisfying the conditions (i), (ii) and the following condition (iv), then (iii) or (v) below holds.
-
(iv)
 $G_{1}$
and
$G_{1}$
and
 $G_{l}$
are multistable.
$G_{l}$
are multistable.
-
(v)
 $\unicode[STIX]{x1D712}(G_{1},G_{l})=-1$
, and
$\unicode[STIX]{x1D712}(G_{1},G_{l})=-1$
, and
 $\unicode[STIX]{x1D712}(G_{i},G_{j})=0$
for
$\unicode[STIX]{x1D712}(G_{i},G_{j})=0$
for
 $i<j$
with
$i<j$
with
 $(i,j)\neq (1,l)$
. Moreover,
$(i,j)\neq (1,l)$
. Moreover,
 $G_{i}$
is not multistable for
$G_{i}$
is not multistable for
 $i\neq 1,l$
.
$i\neq 1,l$
.
Suppose that the filtration (6.1) satisfies (i), (ii) and (iv), and the inequality
 $$\begin{eqnarray}\mathop{\sum }_{i<j}\unicode[STIX]{x1D712}(G_{i},G_{j})\geqslant -1\end{eqnarray}$$
$$\begin{eqnarray}\mathop{\sum }_{i<j}\unicode[STIX]{x1D712}(G_{i},G_{j})\geqslant -1\end{eqnarray}$$
holds. Let us show that (v) holds.
Claim 6.1.1. If
![]() $G_{i}$
is multistable, then
$G_{i}$
is multistable, then
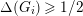 $\unicode[STIX]{x1D6E5}(G_{i})\geqslant 1/2$
. In particular,
$\unicode[STIX]{x1D6E5}(G_{i})\geqslant 1/2$
. In particular,
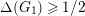 $\unicode[STIX]{x1D6E5}(G_{1})\geqslant 1/2$
and
$\unicode[STIX]{x1D6E5}(G_{1})\geqslant 1/2$
and
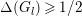 $\unicode[STIX]{x1D6E5}(G_{l})\geqslant 1/2$
.
$\unicode[STIX]{x1D6E5}(G_{l})\geqslant 1/2$
.
Proof of Claim 6.1.1.
Suppose that
![]() $G_{i}$
is multistable and
$G_{i}$
is multistable and
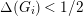 $\unicode[STIX]{x1D6E5}(G_{i})<1/2$
. Then
$\unicode[STIX]{x1D6E5}(G_{i})<1/2$
. Then
![]() $G_{i}$
is S-equivalent to
$G_{i}$
is S-equivalent to
![]() $E^{a}$
with
$E^{a}$
with
![]() $E$
an exceptional bundle. If
$E$
an exceptional bundle. If
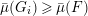 $\bar{\unicode[STIX]{x1D707}}(G_{i})\geqslant \bar{\unicode[STIX]{x1D707}}(F)$
, then we have
$\bar{\unicode[STIX]{x1D707}}(G_{i})\geqslant \bar{\unicode[STIX]{x1D707}}(F)$
, then we have
 $$\begin{eqnarray}\displaystyle 0>\unicode[STIX]{x1D712}(G_{i},F) & = & \displaystyle \mathop{\sum }_{j<i}\unicode[STIX]{x1D712}(G_{i},G_{j})+\unicode[STIX]{x1D712}(G_{i},G_{i})+\mathop{\sum }_{i<j}\unicode[STIX]{x1D712}(G_{i},G_{j})\nonumber\\ \displaystyle & {\geqslant} & \displaystyle \mathop{\sum }_{j<i}\unicode[STIX]{x1D712}(G_{j},G_{i})+\unicode[STIX]{x1D712}(G_{i},G_{i})+\mathop{\sum }_{i<j}\unicode[STIX]{x1D712}(G_{i},G_{j})\nonumber\\ \displaystyle & {\geqslant} & \displaystyle -1+\unicode[STIX]{x1D712}(G_{i},G_{i})=-1+a^{2}\geqslant 0,\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle 0>\unicode[STIX]{x1D712}(G_{i},F) & = & \displaystyle \mathop{\sum }_{j<i}\unicode[STIX]{x1D712}(G_{i},G_{j})+\unicode[STIX]{x1D712}(G_{i},G_{i})+\mathop{\sum }_{i<j}\unicode[STIX]{x1D712}(G_{i},G_{j})\nonumber\\ \displaystyle & {\geqslant} & \displaystyle \mathop{\sum }_{j<i}\unicode[STIX]{x1D712}(G_{j},G_{i})+\unicode[STIX]{x1D712}(G_{i},G_{i})+\mathop{\sum }_{i<j}\unicode[STIX]{x1D712}(G_{i},G_{j})\nonumber\\ \displaystyle & {\geqslant} & \displaystyle -1+\unicode[STIX]{x1D712}(G_{i},G_{i})=-1+a^{2}\geqslant 0,\nonumber\end{eqnarray}$$
which is a contradiction. Here the first inequality follows from the assumption that the height of
![]() $M(\unicode[STIX]{x1D709})$
is positive, and Proposition 3.6. If
$M(\unicode[STIX]{x1D709})$
is positive, and Proposition 3.6. If
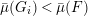 $\bar{\unicode[STIX]{x1D707}}(G_{i})<\bar{\unicode[STIX]{x1D707}}(F)$
, then by considering
$\bar{\unicode[STIX]{x1D707}}(G_{i})<\bar{\unicode[STIX]{x1D707}}(F)$
, then by considering
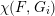 $\unicode[STIX]{x1D712}(F,G_{i})$
, we obtain a contradiction as well.◻
$\unicode[STIX]{x1D712}(F,G_{i})$
, we obtain a contradiction as well.◻
Claim 6.1.2. If
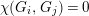 $\unicode[STIX]{x1D712}(G_{i},G_{j})=0$
for some
$\unicode[STIX]{x1D712}(G_{i},G_{j})=0$
for some
![]() $i<j$
, then
$i<j$
, then
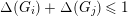 $\unicode[STIX]{x1D6E5}(G_{i})+\unicode[STIX]{x1D6E5}(G_{j})\leqslant 1$
, where the equality holds if and only if
$\unicode[STIX]{x1D6E5}(G_{i})+\unicode[STIX]{x1D6E5}(G_{j})\leqslant 1$
, where the equality holds if and only if
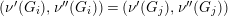 $(\unicode[STIX]{x1D708}^{\prime }(G_{i}),\unicode[STIX]{x1D708}^{\prime \prime }(G_{i}))=(\unicode[STIX]{x1D708}^{\prime }(G_{j}),\unicode[STIX]{x1D708}^{\prime \prime }(G_{j}))$
.
$(\unicode[STIX]{x1D708}^{\prime }(G_{i}),\unicode[STIX]{x1D708}^{\prime \prime }(G_{i}))=(\unicode[STIX]{x1D708}^{\prime }(G_{j}),\unicode[STIX]{x1D708}^{\prime \prime }(G_{j}))$
.
Proof of Claim 6.1.2.
By
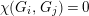 $\unicode[STIX]{x1D712}(G_{i},G_{j})=0$
, we have
$\unicode[STIX]{x1D712}(G_{i},G_{j})=0$
, we have
 $$\begin{eqnarray}\left(\unicode[STIX]{x1D708}^{\prime }(G_{j})-\unicode[STIX]{x1D708}^{\prime }(G_{i})+1\right)\left(\unicode[STIX]{x1D708}^{\prime \prime }(G_{j})-\unicode[STIX]{x1D708}^{\prime \prime }(G_{i})+1\right)=\unicode[STIX]{x1D6E5}(G_{i})+\unicode[STIX]{x1D6E5}(G_{j}).\end{eqnarray}$$
$$\begin{eqnarray}\left(\unicode[STIX]{x1D708}^{\prime }(G_{j})-\unicode[STIX]{x1D708}^{\prime }(G_{i})+1\right)\left(\unicode[STIX]{x1D708}^{\prime \prime }(G_{j})-\unicode[STIX]{x1D708}^{\prime \prime }(G_{i})+1\right)=\unicode[STIX]{x1D6E5}(G_{i})+\unicode[STIX]{x1D6E5}(G_{j}).\end{eqnarray}$$
Since
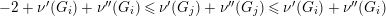 $-2+\unicode[STIX]{x1D708}^{\prime }(G_{i})+\unicode[STIX]{x1D708}^{\prime \prime }(G_{i})\leqslant \unicode[STIX]{x1D708}^{\prime }(G_{j})+\unicode[STIX]{x1D708}^{\prime \prime }(G_{j})\leqslant \unicode[STIX]{x1D708}^{\prime }(G_{i})+\unicode[STIX]{x1D708}^{\prime \prime }(G_{i})$
, we see, by calculation, that the maximum of left-hand side of (6.3) is
$-2+\unicode[STIX]{x1D708}^{\prime }(G_{i})+\unicode[STIX]{x1D708}^{\prime \prime }(G_{i})\leqslant \unicode[STIX]{x1D708}^{\prime }(G_{j})+\unicode[STIX]{x1D708}^{\prime \prime }(G_{j})\leqslant \unicode[STIX]{x1D708}^{\prime }(G_{i})+\unicode[STIX]{x1D708}^{\prime \prime }(G_{i})$
, we see, by calculation, that the maximum of left-hand side of (6.3) is
![]() $1$
, and the maximum is attained only when
$1$
, and the maximum is attained only when
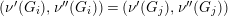 $(\unicode[STIX]{x1D708}^{\prime }(G_{i}),\unicode[STIX]{x1D708}^{\prime \prime }(G_{i}))=(\unicode[STIX]{x1D708}^{\prime }(G_{j}),\unicode[STIX]{x1D708}^{\prime \prime }(G_{j}))$
.◻
$(\unicode[STIX]{x1D708}^{\prime }(G_{i}),\unicode[STIX]{x1D708}^{\prime \prime }(G_{i}))=(\unicode[STIX]{x1D708}^{\prime }(G_{j}),\unicode[STIX]{x1D708}^{\prime \prime }(G_{j}))$
.◻
We have
 $\unicode[STIX]{x1D712}(G_{1},G_{l})<0$
. Indeed, if
$\unicode[STIX]{x1D712}(G_{1},G_{l})<0$
. Indeed, if
 $\unicode[STIX]{x1D712}(G_{1},G_{l})=0$
, then Claims 6.1.1 and 6.1.2 imply that
$\unicode[STIX]{x1D712}(G_{1},G_{l})=0$
, then Claims 6.1.1 and 6.1.2 imply that
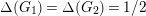 $\unicode[STIX]{x1D6E5}(G_{1})=\unicode[STIX]{x1D6E5}(G_{2})=1/2$
and
$\unicode[STIX]{x1D6E5}(G_{1})=\unicode[STIX]{x1D6E5}(G_{2})=1/2$
and
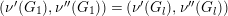 $(\unicode[STIX]{x1D708}^{\prime }(G_{1}),\unicode[STIX]{x1D708}^{\prime \prime }(G_{1}))=(\unicode[STIX]{x1D708}^{\prime }(G_{l}),\unicode[STIX]{x1D708}^{\prime \prime }(G_{l}))$
. This shows that
$(\unicode[STIX]{x1D708}^{\prime }(G_{1}),\unicode[STIX]{x1D708}^{\prime \prime }(G_{1}))=(\unicode[STIX]{x1D708}^{\prime }(G_{l}),\unicode[STIX]{x1D708}^{\prime \prime }(G_{l}))$
. This shows that
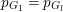 $p_{G_{1}}=p_{G_{l}}$
, which contradicts (i).
$p_{G_{1}}=p_{G_{l}}$
, which contradicts (i).
By the inequality (6.2), we have
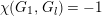 $\unicode[STIX]{x1D712}(G_{1},G_{l})=-1$
and
$\unicode[STIX]{x1D712}(G_{1},G_{l})=-1$
and
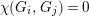 $\unicode[STIX]{x1D712}(G_{i},G_{j})=0$
for
$\unicode[STIX]{x1D712}(G_{i},G_{j})=0$
for
![]() $i<j$
with
$i<j$
with
 $(i,j)\neq (1,l)$
. If
$(i,j)\neq (1,l)$
. If
![]() $G_{i}$
is multistable for
$G_{i}$
is multistable for
![]() $i\neq 1,l$
, then by
$i\neq 1,l$
, then by
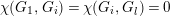 $\unicode[STIX]{x1D712}(G_{1},G_{i})=\unicode[STIX]{x1D712}(G_{i},G_{l})=0$
and
$\unicode[STIX]{x1D712}(G_{1},G_{i})=\unicode[STIX]{x1D712}(G_{i},G_{l})=0$
and
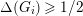 $\unicode[STIX]{x1D6E5}(G_{i})\geqslant 1/2$
, as in the preceding paragraph, we obtain
$\unicode[STIX]{x1D6E5}(G_{i})\geqslant 1/2$
, as in the preceding paragraph, we obtain
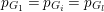 $p_{G_{1}}=p_{G_{i}}=p_{G_{l}}$
, which contradicts (i).
$p_{G_{1}}=p_{G_{i}}=p_{G_{l}}$
, which contradicts (i).
Step 2. We show that if
![]() $F=F_{t}$
is not semistable, then either
$F=F_{t}$
is not semistable, then either
![]() $F$
has a filtration (6.1) satisfying (i), (ii) and (iii), or
$F$
has a filtration (6.1) satisfying (i), (ii) and (iii), or
![]() $F$
has a filtration
$F$
has a filtration
 $$\begin{eqnarray}0=F_{0}\subset F_{1}\subset F_{2}=F\end{eqnarray}$$
$$\begin{eqnarray}0=F_{0}\subset F_{1}\subset F_{2}=F\end{eqnarray}$$
such that
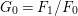 $G_{0}=F_{1}/F_{0}$
and
$G_{0}=F_{1}/F_{0}$
and
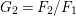 $G_{2}=F_{2}/F_{1}$
are stable,
$G_{2}=F_{2}/F_{1}$
are stable,
 $p_{G_{1}}\succ p_{G_{2}}$
and
$p_{G_{1}}\succ p_{G_{2}}$
and
 $\unicode[STIX]{x1D712}(G_{1},G_{2})=-1$
.
$\unicode[STIX]{x1D712}(G_{1},G_{2})=-1$
.
Let
 $$\begin{eqnarray}0=F^{(0)}\subset F^{(1)}\subset \cdots \subset F^{(k)}=F\end{eqnarray}$$
$$\begin{eqnarray}0=F^{(0)}\subset F^{(1)}\subset \cdots \subset F^{(k)}=F\end{eqnarray}$$
be the Harder–Narasimhan filtration of
![]() $F$
. We have
$F$
. We have
![]() $k\geqslant 2$
because
$k\geqslant 2$
because
![]() $F$
is not semistable. If
$F$
is not semistable. If
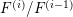 $F^{(i)}/F^{(i-1)}$
,
$F^{(i)}/F^{(i-1)}$
,
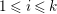 $1\leqslant i\leqslant k$
, is not multistable, then insert a filter
$1\leqslant i\leqslant k$
, is not multistable, then insert a filter
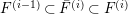 $F^{(i-1)}\subset \bar{F}^{(i)}\subset F^{(i)}$
such that
$F^{(i-1)}\subset \bar{F}^{(i)}\subset F^{(i)}$
such that
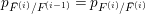 $p_{\bar{F}^{(i)}/F^{(i-1)}}=p_{F^{(i)}/\bar{F}^{(i)}}$
,
$p_{\bar{F}^{(i)}/F^{(i-1)}}=p_{F^{(i)}/\bar{F}^{(i)}}$
,
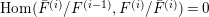 $\text{Hom}(\bar{F}^{(i)}/F^{(i-1)},F^{(i)}/\bar{F}^{(i)})=0$
, and moreover for
$\text{Hom}(\bar{F}^{(i)}/F^{(i-1)},F^{(i)}/\bar{F}^{(i)})=0$
, and moreover for
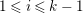 $1\leqslant i\leqslant k-1$
(resp. for
$1\leqslant i\leqslant k-1$
(resp. for
![]() $i=k$
)
$i=k$
)
 $\bar{F}^{(i)}/F^{(i-1)}$
(resp.
$\bar{F}^{(i)}/F^{(i-1)}$
(resp.
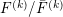 $F^{(k)}/\bar{F}^{(k)}$
) is multistable (cf. [Reference Kuleshov and OrlovKO, Proposition 4.4]). The resulting filtration
$F^{(k)}/\bar{F}^{(k)}$
) is multistable (cf. [Reference Kuleshov and OrlovKO, Proposition 4.4]). The resulting filtration
 $$\begin{eqnarray}0=F_{0}\subset F_{1}\subset \cdots \subset F_{l}=F\end{eqnarray}$$
$$\begin{eqnarray}0=F_{0}\subset F_{1}\subset \cdots \subset F_{l}=F\end{eqnarray}$$
satisfies (i), (ii) and (iv).
If
![]() $k\geqslant 3$
, then at least
$k\geqslant 3$
, then at least
![]() $3$
of the graded sheaves of the resulting filtration are multistable. So the filtration satisfies (iii) by Step 1.
$3$
of the graded sheaves of the resulting filtration are multistable. So the filtration satisfies (iii) by Step 1.
Next we consider the case
![]() $k=2$
, and at least one of
$k=2$
, and at least one of
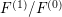 $F^{(1)}/F^{(0)}$
,
$F^{(1)}/F^{(0)}$
,
 $F^{(2)}/F^{(1)}$
is not multistable. We treat the case where
$F^{(2)}/F^{(1)}$
is not multistable. We treat the case where
 $F^{(1)}/F^{(0)}$
is not multistable. (The case where
$F^{(1)}/F^{(0)}$
is not multistable. (The case where
 $F^{(2)}/F^{(1)}$
is not multistable can be handled similarly.) The filtration (6.6) in this case is either
$F^{(2)}/F^{(1)}$
is not multistable can be handled similarly.) The filtration (6.6) in this case is either
 $$\begin{eqnarray}\begin{array}{@{}rl@{}} & 0=F^{(0)}\subset \bar{F}^{(1)}\subset F^{(1)}\subset F^{(2)}=F\\ \text{or } & 0=F^{(0)}\subset \bar{F}^{(1)}\subset F^{(1)}\subset \bar{F}^{(2)}\subset F^{(2)}=F.\end{array}\end{eqnarray}$$
$$\begin{eqnarray}\begin{array}{@{}rl@{}} & 0=F^{(0)}\subset \bar{F}^{(1)}\subset F^{(1)}\subset F^{(2)}=F\\ \text{or } & 0=F^{(0)}\subset \bar{F}^{(1)}\subset F^{(1)}\subset \bar{F}^{(2)}\subset F^{(2)}=F.\end{array}\end{eqnarray}$$
If the filtration (6.7) satisfies (iii), then we are done. If not, then the filtration satisfies (v) by Step 1. Then
![]() $\bar{F}^{(1)}$
must be stable because
$\bar{F}^{(1)}$
must be stable because
 $\unicode[STIX]{x1D712}(G_{1},G_{l})=-1$
. Since
$\unicode[STIX]{x1D712}(G_{1},G_{l})=-1$
. Since
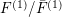 $F^{(1)}/\bar{F}^{(1)}$
is not multistable, we can find a subsheaf
$F^{(1)}/\bar{F}^{(1)}$
is not multistable, we can find a subsheaf
 $\bar{F}^{(1)}\subset D\subset F^{(1)}$
such that
$\bar{F}^{(1)}\subset D\subset F^{(1)}$
such that
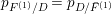 $p_{F^{(1)}/D}=p_{D/\bar{F}^{(1)}}$
,
$p_{F^{(1)}/D}=p_{D/\bar{F}^{(1)}}$
,
 $\text{Hom}(D/\bar{F}^{(1)},F^{(1)}/D)=0$
, and
$\text{Hom}(D/\bar{F}^{(1)},F^{(1)}/D)=0$
, and
![]() $F^{(1)}/D$
is multistable. If
$F^{(1)}/D$
is multistable. If
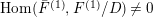 $\text{Hom}(\bar{F}^{(1)},F^{(1)}/D)\neq 0$
, then
$\text{Hom}(\bar{F}^{(1)},F^{(1)}/D)\neq 0$
, then
![]() $F^{(1)}/D$
is S-equivalent to a direct sum of some
$F^{(1)}/D$
is S-equivalent to a direct sum of some
![]() $\bar{F}^{(1)}$
’s. Then we have
$\bar{F}^{(1)}$
’s. Then we have
 $\unicode[STIX]{x1D712}(F^{(1)}/D,G_{l})<0$
since
$\unicode[STIX]{x1D712}(F^{(1)}/D,G_{l})<0$
since
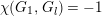 $\unicode[STIX]{x1D712}(G_{1},G_{l})=-1$
. On the other hand, we have
$\unicode[STIX]{x1D712}(G_{1},G_{l})=-1$
. On the other hand, we have
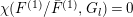 $\unicode[STIX]{x1D712}(F^{(1)}/\bar{F}^{(1)},G_{l})=0$
, so we have
$\unicode[STIX]{x1D712}(F^{(1)}/\bar{F}^{(1)},G_{l})=0$
, so we have
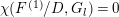 $\unicode[STIX]{x1D712}(F^{(1)}/D,G_{l})=0$
. This is a contradiction. Therefore, we have
$\unicode[STIX]{x1D712}(F^{(1)}/D,G_{l})=0$
. This is a contradiction. Therefore, we have
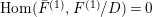 $\text{Hom}(\bar{F}^{(1)},F^{(1)}/D)=0$
. Then the filtration obtained from (6.7) by inserting
$\text{Hom}(\bar{F}^{(1)},F^{(1)}/D)=0$
. Then the filtration obtained from (6.7) by inserting
![]() $D$
as a filter satisfies (i), (ii) and (iv), and at least
$D$
as a filter satisfies (i), (ii) and (iv), and at least
![]() $3$
of the graded sheaves are multistable. So it satisfies (iii) by Step 1.
$3$
of the graded sheaves are multistable. So it satisfies (iii) by Step 1.
Finally we consider the case where
![]() $k=2$
, and both
$k=2$
, and both
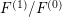 $F^{(1)}/F^{(0)}$
and
$F^{(1)}/F^{(0)}$
and
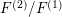 $F^{(2)}/F^{(1)}$
are multistable. The filtration (6.5) satisfies (i), (ii) and (iv). If (6.5) satisfies (iii), then we are done. If not, it satisfies (v) by Step 1. Since
$F^{(2)}/F^{(1)}$
are multistable. The filtration (6.5) satisfies (i), (ii) and (iv). If (6.5) satisfies (iii), then we are done. If not, it satisfies (v) by Step 1. Since
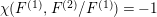 $\unicode[STIX]{x1D712}(F^{(1)},F^{(2)}/F^{(1)})=-1$
, both
$\unicode[STIX]{x1D712}(F^{(1)},F^{(2)}/F^{(1)})=-1$
, both
![]() $F^{(1)}$
and
$F^{(1)}$
and
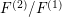 $F^{(2)}/F^{(1)}$
must be stable. So we obtain a desired filtration.
$F^{(2)}/F^{(1)}$
must be stable. So we obtain a desired filtration.
Step 3. To conclude that
![]() $F=F_{t}$
that is not semistable has a filtration (6.1) satisfying (i), (ii) and (iii), we have only to show that
$F=F_{t}$
that is not semistable has a filtration (6.1) satisfying (i), (ii) and (iii), we have only to show that
![]() $F$
does not have a filtration (6.4) such that
$F$
does not have a filtration (6.4) such that
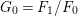 $G_{0}=F_{1}/F_{0}$
and
$G_{0}=F_{1}/F_{0}$
and
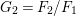 $G_{2}=F_{2}/F_{1}$
are stable,
$G_{2}=F_{2}/F_{1}$
are stable,
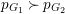 $p_{G_{1}}\succ p_{G_{2}}$
and
$p_{G_{1}}\succ p_{G_{2}}$
and
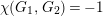 $\unicode[STIX]{x1D712}(G_{1},G_{2})=-1$
.
$\unicode[STIX]{x1D712}(G_{1},G_{2})=-1$
.
Suppose that such a filtration exists. For
![]() $i=1,2$
, put
$i=1,2$
, put
 $$\begin{eqnarray}\displaystyle r_{i}=\text{r}(G_{i}),\qquad \unicode[STIX]{x1D6E5}_{i}=\unicode[STIX]{x1D6E5}(G_{i}),\qquad \bar{\unicode[STIX]{x1D707}}_{i} & =\bar{\unicode[STIX]{x1D707}}(G_{i}), & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle r_{i}=\text{r}(G_{i}),\qquad \unicode[STIX]{x1D6E5}_{i}=\unicode[STIX]{x1D6E5}(G_{i}),\qquad \bar{\unicode[STIX]{x1D707}}_{i} & =\bar{\unicode[STIX]{x1D707}}(G_{i}), & \displaystyle \nonumber\end{eqnarray}$$
and define
![]() $\unicode[STIX]{x1D6FF}_{i}$
by
$\unicode[STIX]{x1D6FF}_{i}$
by
 $$\begin{eqnarray}\left(\unicode[STIX]{x1D708}^{\prime }(G_{i}),\unicode[STIX]{x1D708}^{\prime \prime }(G_{i})\right)=(\bar{\unicode[STIX]{x1D707}}_{i}+\unicode[STIX]{x1D6FF}_{i},\bar{\unicode[STIX]{x1D707}}_{i}-\unicode[STIX]{x1D6FF}_{i}).\end{eqnarray}$$
$$\begin{eqnarray}\left(\unicode[STIX]{x1D708}^{\prime }(G_{i}),\unicode[STIX]{x1D708}^{\prime \prime }(G_{i})\right)=(\bar{\unicode[STIX]{x1D707}}_{i}+\unicode[STIX]{x1D6FF}_{i},\bar{\unicode[STIX]{x1D707}}_{i}-\unicode[STIX]{x1D6FF}_{i}).\end{eqnarray}$$
From
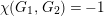 $\unicode[STIX]{x1D712}(G_{1},G_{2})=-1$
, we have
$\unicode[STIX]{x1D712}(G_{1},G_{2})=-1$
, we have
 $$\begin{eqnarray}(1+\bar{\unicode[STIX]{x1D707}}_{2}-\bar{\unicode[STIX]{x1D707}}_{1})^{2}-(\unicode[STIX]{x1D6FF}_{2}-\unicode[STIX]{x1D6FF}_{1})^{2}=\unicode[STIX]{x1D6E5}_{1}+\unicode[STIX]{x1D6E5}_{2}-\frac{1}{r_{1}r_{2}}.\end{eqnarray}$$
$$\begin{eqnarray}(1+\bar{\unicode[STIX]{x1D707}}_{2}-\bar{\unicode[STIX]{x1D707}}_{1})^{2}-(\unicode[STIX]{x1D6FF}_{2}-\unicode[STIX]{x1D6FF}_{1})^{2}=\unicode[STIX]{x1D6E5}_{1}+\unicode[STIX]{x1D6E5}_{2}-\frac{1}{r_{1}r_{2}}.\end{eqnarray}$$
Define a nonnegative integer
![]() $k$
by
$k$
by
 $$\begin{eqnarray}2\bar{\unicode[STIX]{x1D707}}_{1}=2\bar{\unicode[STIX]{x1D707}}_{2}+\frac{k}{r_{1}r_{2}}.\end{eqnarray}$$
$$\begin{eqnarray}2\bar{\unicode[STIX]{x1D707}}_{1}=2\bar{\unicode[STIX]{x1D707}}_{2}+\frac{k}{r_{1}r_{2}}.\end{eqnarray}$$
Since
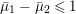 $\bar{\unicode[STIX]{x1D707}}_{1}-\bar{\unicode[STIX]{x1D707}}_{2}\leqslant 1$
, we have
$\bar{\unicode[STIX]{x1D707}}_{1}-\bar{\unicode[STIX]{x1D707}}_{2}\leqslant 1$
, we have
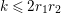 $k\leqslant 2r_{1}r_{2}$
. By (6.8), we have
$k\leqslant 2r_{1}r_{2}$
. By (6.8), we have
 $$\begin{eqnarray}\left(1-\frac{k}{2r_{1}r_{2}}\right)^{2}\geqslant \unicode[STIX]{x1D6E5}_{1}+\unicode[STIX]{x1D6E5}_{2}-\frac{1}{r_{1}r_{2}}\geqslant 1-\frac{1}{r_{1}r_{2}},\end{eqnarray}$$
$$\begin{eqnarray}\left(1-\frac{k}{2r_{1}r_{2}}\right)^{2}\geqslant \unicode[STIX]{x1D6E5}_{1}+\unicode[STIX]{x1D6E5}_{2}-\frac{1}{r_{1}r_{2}}\geqslant 1-\frac{1}{r_{1}r_{2}},\end{eqnarray}$$
where the last inequality follows from Claim 6.1.1. From this, we have
 $$\begin{eqnarray}1\geqslant k\left(1-\frac{k}{4r_{1}r_{2}}\right)\geqslant \frac{k}{2}.\end{eqnarray}$$
$$\begin{eqnarray}1\geqslant k\left(1-\frac{k}{4r_{1}r_{2}}\right)\geqslant \frac{k}{2}.\end{eqnarray}$$
Thus
![]() $k\leqslant 2$
. If
$k\leqslant 2$
. If
![]() $k=2$
, then the inequalities in (6.9) are all equality. Thus
$k=2$
, then the inequalities in (6.9) are all equality. Thus
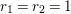 $r_{1}=r_{2}=1$
and
$r_{1}=r_{2}=1$
and
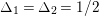 $\unicode[STIX]{x1D6E5}_{1}=\unicode[STIX]{x1D6E5}_{2}=1/2$
. This is absurd because the discriminant of a rank one sheaf is an integer.
$\unicode[STIX]{x1D6E5}_{1}=\unicode[STIX]{x1D6E5}_{2}=1/2$
. This is absurd because the discriminant of a rank one sheaf is an integer.
Before we consider the case
![]() $k=0,1$
, we note that we have
$k=0,1$
, we note that we have
 $$\begin{eqnarray}r_{1}\unicode[STIX]{x1D6FF}_{1}+r_{2}\unicode[STIX]{x1D6FF}_{2}=0\end{eqnarray}$$
$$\begin{eqnarray}r_{1}\unicode[STIX]{x1D6FF}_{1}+r_{2}\unicode[STIX]{x1D6FF}_{2}=0\end{eqnarray}$$
because
![]() $c_{1}(\unicode[STIX]{x1D709})$
is symmetric.
$c_{1}(\unicode[STIX]{x1D709})$
is symmetric.
Consider the case
![]() $k=0$
. We have
$k=0$
. We have
![]() $\bar{\unicode[STIX]{x1D707}}_{1}=\bar{\unicode[STIX]{x1D707}}_{2}$
. From (6.8), we have
$\bar{\unicode[STIX]{x1D707}}_{1}=\bar{\unicode[STIX]{x1D707}}_{2}$
. From (6.8), we have
 $$\begin{eqnarray}\displaystyle \frac{1}{r_{1}r_{2}} & = & \displaystyle \unicode[STIX]{x1D6E5}_{1}+\unicode[STIX]{x1D6E5}_{2}-1+(\unicode[STIX]{x1D6FF}_{1}-\unicode[STIX]{x1D6FF}_{2})^{2}\nonumber\\ \displaystyle & {\geqslant} & \displaystyle (\unicode[STIX]{x1D6FF}_{1}-\unicode[STIX]{x1D6FF}_{2})^{2}=\left(\frac{r_{1}+r_{2}}{r_{2}}\right)^{2}\unicode[STIX]{x1D6FF}_{1}^{2}.\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \frac{1}{r_{1}r_{2}} & = & \displaystyle \unicode[STIX]{x1D6E5}_{1}+\unicode[STIX]{x1D6E5}_{2}-1+(\unicode[STIX]{x1D6FF}_{1}-\unicode[STIX]{x1D6FF}_{2})^{2}\nonumber\\ \displaystyle & {\geqslant} & \displaystyle (\unicode[STIX]{x1D6FF}_{1}-\unicode[STIX]{x1D6FF}_{2})^{2}=\left(\frac{r_{1}+r_{2}}{r_{2}}\right)^{2}\unicode[STIX]{x1D6FF}_{1}^{2}.\end{eqnarray}$$
If
![]() $\unicode[STIX]{x1D6FF}_{1}\neq 0$
, then
$\unicode[STIX]{x1D6FF}_{1}\neq 0$
, then
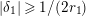 $|\unicode[STIX]{x1D6FF}_{1}|\geqslant 1/(2r_{1})$
because
$|\unicode[STIX]{x1D6FF}_{1}|\geqslant 1/(2r_{1})$
because
![]() $2r_{1}\unicode[STIX]{x1D6FF}_{1}$
is an integer. We have
$2r_{1}\unicode[STIX]{x1D6FF}_{1}$
is an integer. We have
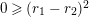 $0\geqslant (r_{1}-r_{2})^{2}$
from (6.11). Then
$0\geqslant (r_{1}-r_{2})^{2}$
from (6.11). Then
![]() $r_{1}=r_{2}$
, and the inequality in (6.11) is an equality, thus
$r_{1}=r_{2}$
, and the inequality in (6.11) is an equality, thus
 $\unicode[STIX]{x1D6E5}_{1}=\unicode[STIX]{x1D6E5}_{2}=1/2$
. Then we have
$\unicode[STIX]{x1D6E5}_{1}=\unicode[STIX]{x1D6E5}_{2}=1/2$
. Then we have
 $\unicode[STIX]{x1D6E5}_{1}+\unicode[STIX]{x1D6FF}_{1}^{2}=\unicode[STIX]{x1D6E5}_{2}+\unicode[STIX]{x1D6FF}_{2}^{2}$
, which implies
$\unicode[STIX]{x1D6E5}_{1}+\unicode[STIX]{x1D6FF}_{1}^{2}=\unicode[STIX]{x1D6E5}_{2}+\unicode[STIX]{x1D6FF}_{2}^{2}$
, which implies
 $p_{G_{1}}=p_{G_{2}}$
, a contradiction.
$p_{G_{1}}=p_{G_{2}}$
, a contradiction.
If
![]() $\unicode[STIX]{x1D6FF}_{1}=0$
, then both
$\unicode[STIX]{x1D6FF}_{1}=0$
, then both
![]() $c_{1}(G_{1})$
and
$c_{1}(G_{1})$
and
![]() $c_{1}(G_{2})$
are symmetric. Thus
$c_{1}(G_{2})$
are symmetric. Thus
 $\unicode[STIX]{x1D6E5}_{i}>1/2$
. More precisely, we have
$\unicode[STIX]{x1D6E5}_{i}>1/2$
. More precisely, we have
 $$\begin{eqnarray}\unicode[STIX]{x1D6E5}_{i}\geqslant \frac{1}{2}+\frac{1}{2r_{i}^{2}}.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6E5}_{i}\geqslant \frac{1}{2}+\frac{1}{2r_{i}^{2}}.\end{eqnarray}$$
From (6.8), we have
 $$\begin{eqnarray}\frac{1}{r_{1}r_{2}}\geqslant \frac{1}{2r_{1}^{2}}+\frac{1}{2r_{2}^{2}},\end{eqnarray}$$
$$\begin{eqnarray}\frac{1}{r_{1}r_{2}}\geqslant \frac{1}{2r_{1}^{2}}+\frac{1}{2r_{2}^{2}},\end{eqnarray}$$
thus
 $0\geqslant (r_{1}-r_{2})^{2}$
. Then we have
$0\geqslant (r_{1}-r_{2})^{2}$
. Then we have
![]() $r_{1}=r_{2}$
and
$r_{1}=r_{2}$
and
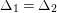 $\unicode[STIX]{x1D6E5}_{1}=\unicode[STIX]{x1D6E5}_{2}$
, which implies a contradiction as in the preceding paragraph.
$\unicode[STIX]{x1D6E5}_{1}=\unicode[STIX]{x1D6E5}_{2}$
, which implies a contradiction as in the preceding paragraph.
Consider the case
![]() $k=1$
. From (6.8), we have
$k=1$
. From (6.8), we have
 $$\begin{eqnarray}\left(1-\frac{1}{2r_{1}r_{2}}\right)^{2}-(\unicode[STIX]{x1D6FF}_{2}-\unicode[STIX]{x1D6FF}_{1})^{2}=\unicode[STIX]{x1D6E5}_{1}+\unicode[STIX]{x1D6E5}_{2}-\frac{1}{r_{1}r_{2}}.\end{eqnarray}$$
$$\begin{eqnarray}\left(1-\frac{1}{2r_{1}r_{2}}\right)^{2}-(\unicode[STIX]{x1D6FF}_{2}-\unicode[STIX]{x1D6FF}_{1})^{2}=\unicode[STIX]{x1D6E5}_{1}+\unicode[STIX]{x1D6E5}_{2}-\frac{1}{r_{1}r_{2}}.\end{eqnarray}$$
From this, we have
 $$\begin{eqnarray}\displaystyle 1-(\unicode[STIX]{x1D6FF}_{1}-\unicode[STIX]{x1D6FF}_{2})^{2} & = & \displaystyle \unicode[STIX]{x1D6E5}_{1}+\unicode[STIX]{x1D6E5}_{2}-\frac{1}{4r_{1}^{2}r_{2}^{2}}\nonumber\\ \displaystyle & {\geqslant} & \displaystyle 1-\frac{1}{4r_{1}^{2}r_{2}^{2}}.\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle 1-(\unicode[STIX]{x1D6FF}_{1}-\unicode[STIX]{x1D6FF}_{2})^{2} & = & \displaystyle \unicode[STIX]{x1D6E5}_{1}+\unicode[STIX]{x1D6E5}_{2}-\frac{1}{4r_{1}^{2}r_{2}^{2}}\nonumber\\ \displaystyle & {\geqslant} & \displaystyle 1-\frac{1}{4r_{1}^{2}r_{2}^{2}}.\end{eqnarray}$$
Therefore,
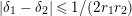 $|\unicode[STIX]{x1D6FF}_{1}-\unicode[STIX]{x1D6FF}_{2}|\leqslant 1/(2r_{1}r_{2})$
.
$|\unicode[STIX]{x1D6FF}_{1}-\unicode[STIX]{x1D6FF}_{2}|\leqslant 1/(2r_{1}r_{2})$
.
If
![]() $\unicode[STIX]{x1D6FF}_{1}=\unicode[STIX]{x1D6FF}_{2}$
, then
$\unicode[STIX]{x1D6FF}_{1}=\unicode[STIX]{x1D6FF}_{2}$
, then
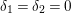 $\unicode[STIX]{x1D6FF}_{1}=\unicode[STIX]{x1D6FF}_{2}=0$
by (6.10). Since both
$\unicode[STIX]{x1D6FF}_{1}=\unicode[STIX]{x1D6FF}_{2}=0$
by (6.10). Since both
![]() $c_{1}(G_{1})$
and
$c_{1}(G_{1})$
and
![]() $c_{1}(G_{2})$
are symmetric, we have
$c_{1}(G_{2})$
are symmetric, we have
 $$\begin{eqnarray}\unicode[STIX]{x1D6E5}_{i}\geqslant \frac{1}{2}+\frac{1}{2r_{i}^{2}}.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6E5}_{i}\geqslant \frac{1}{2}+\frac{1}{2r_{i}^{2}}.\end{eqnarray}$$
From (6.12) and (6.13), we have
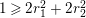 $1\geqslant 2r_{1}^{2}+2r_{2}^{2}$
, which is absurd.
$1\geqslant 2r_{1}^{2}+2r_{2}^{2}$
, which is absurd.
If
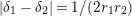 $|\unicode[STIX]{x1D6FF}_{1}-\unicode[STIX]{x1D6FF}_{2}|=1/(2r_{1}r_{2})$
, then we have
$|\unicode[STIX]{x1D6FF}_{1}-\unicode[STIX]{x1D6FF}_{2}|=1/(2r_{1}r_{2})$
, then we have
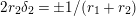 $2r_{2}\unicode[STIX]{x1D6FF}_{2}=\pm 1/(r_{1}+r_{2})$
by (6.10). This is a contradiction because
$2r_{2}\unicode[STIX]{x1D6FF}_{2}=\pm 1/(r_{1}+r_{2})$
by (6.10). This is a contradiction because
![]() $2r_{2}\unicode[STIX]{x1D6FF}_{2}$
is an integer.
$2r_{2}\unicode[STIX]{x1D6FF}_{2}$
is an integer.
From Steps 1 to 3, we can conclude that the set of points
![]() $t\in T$
such that
$t\in T$
such that
![]() $F_{t}$
is not semistable is of codimension at least
$F_{t}$
is not semistable is of codimension at least
![]() $2$
.
$2$
.
Step 4. We claim that the set of points
![]() $t\in T$
such that
$t\in T$
such that
![]() $F_{t}$
is not stable is of codimension at least
$F_{t}$
is not stable is of codimension at least
![]() $2$
.
$2$
.
If
![]() $F=F_{t}$
is semistable, but not stable, then there is a filtration
$F=F_{t}$
is semistable, but not stable, then there is a filtration
 $$\begin{eqnarray}0=F_{0}\subset F_{1}\subset \cdots \subset F_{l}=F,\quad l\geqslant 2\end{eqnarray}$$
$$\begin{eqnarray}0=F_{0}\subset F_{1}\subset \cdots \subset F_{l}=F,\quad l\geqslant 2\end{eqnarray}$$
such that each
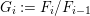 $G_{i}:=F_{i}/F_{i-1}$
is stable, and its reduced Hilbert polynomial is equal to
$G_{i}:=F_{i}/F_{i-1}$
is stable, and its reduced Hilbert polynomial is equal to
![]() $p_{F}$
. Put
$p_{F}$
. Put
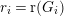 $r_{i}=\text{r}(G_{i})$
,
$r_{i}=\text{r}(G_{i})$
,
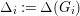 $\unicode[STIX]{x1D6E5}_{i}:=\unicode[STIX]{x1D6E5}(G_{i})$
and define
$\unicode[STIX]{x1D6E5}_{i}:=\unicode[STIX]{x1D6E5}(G_{i})$
and define
![]() $\unicode[STIX]{x1D6FF}_{i}$
by
$\unicode[STIX]{x1D6FF}_{i}$
by
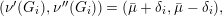 $\left(\unicode[STIX]{x1D708}^{\prime }(G_{i}),\unicode[STIX]{x1D708}^{\prime \prime }(G_{i})\right)=(\bar{\unicode[STIX]{x1D707}}+\unicode[STIX]{x1D6FF}_{i},\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FF}_{i}),$
where
$\left(\unicode[STIX]{x1D708}^{\prime }(G_{i}),\unicode[STIX]{x1D708}^{\prime \prime }(G_{i})\right)=(\bar{\unicode[STIX]{x1D707}}+\unicode[STIX]{x1D6FF}_{i},\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FF}_{i}),$
where
 $\bar{\unicode[STIX]{x1D707}}:=\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})$
. Put
$\bar{\unicode[STIX]{x1D707}}:=\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})$
. Put
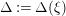 $\unicode[STIX]{x1D6E5}:=\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})$
. To prove the claim in Step 4, we shall show that
$\unicode[STIX]{x1D6E5}:=\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})$
. To prove the claim in Step 4, we shall show that
 $\dim \text{Ext}_{+}^{1}(F,F)\geqslant 2$
for this filtration.
$\dim \text{Ext}_{+}^{1}(F,F)\geqslant 2$
for this filtration.
We have
 $$\begin{eqnarray}\mathop{\sum }_{i}(-1)^{i}\dim \text{Ext}_{+}^{i}(F,F)=\mathop{\sum }_{i<j}\unicode[STIX]{x1D712}(G_{i},G_{j}).\end{eqnarray}$$
$$\begin{eqnarray}\mathop{\sum }_{i}(-1)^{i}\dim \text{Ext}_{+}^{i}(F,F)=\mathop{\sum }_{i<j}\unicode[STIX]{x1D712}(G_{i},G_{j}).\end{eqnarray}$$
By
 $\text{Ext}_{+}^{2}(F,F)=0$
, we have
$\text{Ext}_{+}^{2}(F,F)=0$
, we have
 $$\begin{eqnarray}\displaystyle \dim \text{Ext}_{+}^{1}(F,F)\geqslant -\mathop{\sum }_{i<j}\unicode[STIX]{x1D712}(G_{i},G_{j}) & = & \displaystyle \mathop{\sum }_{i<j}r_{i}r_{j}(-1-2\unicode[STIX]{x1D6FF}_{i}\unicode[STIX]{x1D6FF}_{j}+2\unicode[STIX]{x1D6E5})\nonumber\\ \displaystyle & = & \displaystyle \mathop{\sum }_{i<j}r_{i}r_{j}(2\unicode[STIX]{x1D6E5}-1)+\mathop{\sum }_{k}r_{k}^{2}\unicode[STIX]{x1D6FF}_{k}^{2},\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \dim \text{Ext}_{+}^{1}(F,F)\geqslant -\mathop{\sum }_{i<j}\unicode[STIX]{x1D712}(G_{i},G_{j}) & = & \displaystyle \mathop{\sum }_{i<j}r_{i}r_{j}(-1-2\unicode[STIX]{x1D6FF}_{i}\unicode[STIX]{x1D6FF}_{j}+2\unicode[STIX]{x1D6E5})\nonumber\\ \displaystyle & = & \displaystyle \mathop{\sum }_{i<j}r_{i}r_{j}(2\unicode[STIX]{x1D6E5}-1)+\mathop{\sum }_{k}r_{k}^{2}\unicode[STIX]{x1D6FF}_{k}^{2},\end{eqnarray}$$
where we used
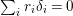 $\sum _{i}r_{i}\unicode[STIX]{x1D6FF}_{i}=0$
. This implies
$\sum _{i}r_{i}\unicode[STIX]{x1D6FF}_{i}=0$
. This implies
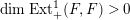 $\dim \text{Ext}_{+}^{1}(F,F)>0$
. Assuming the value (6.15) is equal to
$\dim \text{Ext}_{+}^{1}(F,F)>0$
. Assuming the value (6.15) is equal to
![]() $1$
, we shall obtain a contradiction.
$1$
, we shall obtain a contradiction.
Since each
![]() $2r_{i}\unicode[STIX]{x1D6FF}_{i}$
is an integer, we have
$2r_{i}\unicode[STIX]{x1D6FF}_{i}$
is an integer, we have
 $r_{i}r_{j}(2\unicode[STIX]{x1D6E5}-1)\in \frac{1}{2}\mathbb{Z}$
. So we must have
$r_{i}r_{j}(2\unicode[STIX]{x1D6E5}-1)\in \frac{1}{2}\mathbb{Z}$
. So we must have
![]() $l=2$
. There are two cases:
$l=2$
. There are two cases:
 $$\begin{eqnarray}(r_{1}r_{2}(2\unicode[STIX]{x1D6E5}-1),r_{1}\unicode[STIX]{x1D6FF}_{1},r_{2}\unicode[STIX]{x1D6FF}_{2})=(1,0,0)\qquad \text{or}\qquad \left(\frac{1}{2},\pm \frac{1}{2},\mp \frac{1}{2}\right)\!.\end{eqnarray}$$
$$\begin{eqnarray}(r_{1}r_{2}(2\unicode[STIX]{x1D6E5}-1),r_{1}\unicode[STIX]{x1D6FF}_{1},r_{2}\unicode[STIX]{x1D6FF}_{2})=(1,0,0)\qquad \text{or}\qquad \left(\frac{1}{2},\pm \frac{1}{2},\mp \frac{1}{2}\right)\!.\end{eqnarray}$$
Case
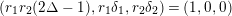 $(r_{1}r_{2}(2\unicode[STIX]{x1D6E5}-1),r_{1}\unicode[STIX]{x1D6FF}_{1},r_{2}\unicode[STIX]{x1D6FF}_{2})=(1,0,0)$
. In this case, both
$(r_{1}r_{2}(2\unicode[STIX]{x1D6E5}-1),r_{1}\unicode[STIX]{x1D6FF}_{1},r_{2}\unicode[STIX]{x1D6FF}_{2})=(1,0,0)$
. In this case, both
![]() $G_{1}$
and
$G_{1}$
and
![]() $G_{2}$
have symmetric
$G_{2}$
have symmetric
![]() $c_{1}$
and
$c_{1}$
and
 $$\begin{eqnarray}\unicode[STIX]{x1D6E5}=\unicode[STIX]{x1D6E5}_{1}=\unicode[STIX]{x1D6E5}_{2}=\frac{1}{2}\left(1+\frac{1}{r_{1}r_{2}}\right)\!.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6E5}=\unicode[STIX]{x1D6E5}_{1}=\unicode[STIX]{x1D6E5}_{2}=\frac{1}{2}\left(1+\frac{1}{r_{1}r_{2}}\right)\!.\end{eqnarray}$$
Since
 $\unicode[STIX]{x1D712}(G_{1},G_{2})=-1$
, we have
$\unicode[STIX]{x1D712}(G_{1},G_{2})=-1$
, we have
![]() $r_{1}=r_{2}$
, so we have
$r_{1}=r_{2}$
, so we have
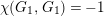 $\unicode[STIX]{x1D712}(G_{1},G_{1})=-1$
. Then
$\unicode[STIX]{x1D712}(G_{1},G_{1})=-1$
. Then
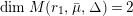 $\dim M(r_{1},\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5})=2$
. This does not occur by Lemma 6.2.
$\dim M(r_{1},\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5})=2$
. This does not occur by Lemma 6.2.
Case
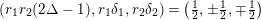 $(r_{1}r_{2}(2\unicode[STIX]{x1D6E5}-1),r_{1}\unicode[STIX]{x1D6FF}_{1},r_{2}\unicode[STIX]{x1D6FF}_{2})=\left(\frac{1}{2},\pm \frac{1}{2},\mp \frac{1}{2}\right)$
. We have
$(r_{1}r_{2}(2\unicode[STIX]{x1D6E5}-1),r_{1}\unicode[STIX]{x1D6FF}_{1},r_{2}\unicode[STIX]{x1D6FF}_{2})=\left(\frac{1}{2},\pm \frac{1}{2},\mp \frac{1}{2}\right)$
. We have
 $$\begin{eqnarray}\unicode[STIX]{x1D6E5}=\frac{1}{2}\left(1+\frac{1}{2r_{1}r_{2}}\right)=\unicode[STIX]{x1D6E5}_{i}+\frac{1}{4r_{i}^{2}}.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6E5}=\frac{1}{2}\left(1+\frac{1}{2r_{1}r_{2}}\right)=\unicode[STIX]{x1D6E5}_{i}+\frac{1}{4r_{i}^{2}}.\end{eqnarray}$$
We first consider the case
![]() $r_{1}\neq r_{2}$
, say
$r_{1}\neq r_{2}$
, say
![]() $r_{1}<r_{2}$
. Then
$r_{1}<r_{2}$
. Then
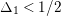 $\unicode[STIX]{x1D6E5}_{1}<1/2$
, so
$\unicode[STIX]{x1D6E5}_{1}<1/2$
, so
![]() $G_{1}$
is rigid and we have
$G_{1}$
is rigid and we have
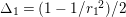 $\unicode[STIX]{x1D6E5}_{1}=(1-1/{r_{1}}^{2})/2$
. Combining this and (6.16), we have
$\unicode[STIX]{x1D6E5}_{1}=(1-1/{r_{1}}^{2})/2$
. Combining this and (6.16), we have
 $r_{1}+r_{2}=0$
, which is absurd. Finally consider the case
$r_{1}+r_{2}=0$
, which is absurd. Finally consider the case
 $r_{1}=r_{2}=r/2$
. Then
$r_{1}=r_{2}=r/2$
. Then
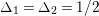 $\unicode[STIX]{x1D6E5}_{1}=\unicode[STIX]{x1D6E5}_{2}=1/2$
. We have
$\unicode[STIX]{x1D6E5}_{1}=\unicode[STIX]{x1D6E5}_{2}=1/2$
. We have
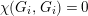 $\unicode[STIX]{x1D712}(G_{i},G_{i})=0$
. Then
$\unicode[STIX]{x1D712}(G_{i},G_{i})=0$
. Then
 $$\begin{eqnarray}\unicode[STIX]{x1D712}(F,F)=\unicode[STIX]{x1D712}(G_{1},G_{2})+\unicode[STIX]{x1D712}(G_{2},G_{1})=-2.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D712}(F,F)=\unicode[STIX]{x1D712}(G_{1},G_{2})+\unicode[STIX]{x1D712}(G_{2},G_{1})=-2.\end{eqnarray}$$
We have
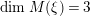 $\dim M(\unicode[STIX]{x1D709})=3$
. By Lemma 6.2,
$\dim M(\unicode[STIX]{x1D709})=3$
. By Lemma 6.2,
![]() $\bar{\unicode[STIX]{x1D707}}\in \mathfrak{E}$
. Since the height of
$\bar{\unicode[STIX]{x1D707}}\in \mathfrak{E}$
. Since the height of
![]() $M(\unicode[STIX]{x1D709})$
is positive, we have
$M(\unicode[STIX]{x1D709})$
is positive, we have
 $$\begin{eqnarray}\unicode[STIX]{x1D6E5}>\unicode[STIX]{x1D6FF}(\bar{\unicode[STIX]{x1D707}})=\frac{1}{2}\left(1+\frac{e}{r_{\bar{\unicode[STIX]{x1D707}}}^{2}}\right)\!,\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6E5}>\unicode[STIX]{x1D6FF}(\bar{\unicode[STIX]{x1D707}})=\frac{1}{2}\left(1+\frac{e}{r_{\bar{\unicode[STIX]{x1D707}}}^{2}}\right)\!,\end{eqnarray}$$
where
![]() $e$
is
$e$
is
![]() $1$
or
$1$
or
![]() $2$
, depending on whether
$2$
, depending on whether
![]() $r_{\bar{\unicode[STIX]{x1D707}}}$
is odd or even. From (6.16) and (6.17), we have
$r_{\bar{\unicode[STIX]{x1D707}}}$
is odd or even. From (6.16) and (6.17), we have
 $$\begin{eqnarray}r_{\bar{\unicode[STIX]{x1D707}}}>r\sqrt{e/2}.\end{eqnarray}$$
$$\begin{eqnarray}r_{\bar{\unicode[STIX]{x1D707}}}>r\sqrt{e/2}.\end{eqnarray}$$
Since
![]() $r\bar{\unicode[STIX]{x1D707}}$
is an integer, and since
$r\bar{\unicode[STIX]{x1D707}}$
is an integer, and since
![]() $r_{\bar{\unicode[STIX]{x1D707}}}$
is the denominator of the irreducible fraction expression of
$r_{\bar{\unicode[STIX]{x1D707}}}$
is the denominator of the irreducible fraction expression of
![]() $\bar{\unicode[STIX]{x1D707}}$
, we have
$\bar{\unicode[STIX]{x1D707}}$
, we have
![]() $r\geqslant r_{\bar{\unicode[STIX]{x1D707}}}$
. Moreover, when
$r\geqslant r_{\bar{\unicode[STIX]{x1D707}}}$
. Moreover, when
![]() $r_{\bar{\unicode[STIX]{x1D707}}}$
is odd,
$r_{\bar{\unicode[STIX]{x1D707}}}$
is odd,
![]() $r\geqslant 2r_{\bar{\unicode[STIX]{x1D707}}}$
since
$r\geqslant 2r_{\bar{\unicode[STIX]{x1D707}}}$
since
![]() $r$
is even. This contradicts (6.18).
$r$
is even. This contradicts (6.18).
(2) By (1), we may assume that the complete family
![]() $\{F_{t}\}$
consists of stable sheaves. Since
$\{F_{t}\}$
consists of stable sheaves. Since
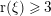 $\text{r}(\unicode[STIX]{x1D709})\geqslant 3$
, the set of points
$\text{r}(\unicode[STIX]{x1D709})\geqslant 3$
, the set of points
![]() $t\in T$
with
$t\in T$
with
![]() $F_{t}$
not locally free has codimension at least
$F_{t}$
not locally free has codimension at least
![]() $2$
(see [Reference Le PotierLe, Section 17.1]). Thus we may assume that
$2$
(see [Reference Le PotierLe, Section 17.1]). Thus we may assume that
![]() $F_{t}$
is locally free for all
$F_{t}$
is locally free for all
![]() $t\in T$
.
$t\in T$
.
If
![]() $F=F_{t}$
is not
$F=F_{t}$
is not
![]() $\unicode[STIX]{x1D707}$
-stable, then there exists a surjection
$\unicode[STIX]{x1D707}$
-stable, then there exists a surjection
![]() $F{\twoheadrightarrow}G$
such that
$F{\twoheadrightarrow}G$
such that
![]() $G$
is a torsion-free sheaf such that
$G$
is a torsion-free sheaf such that
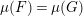 $\unicode[STIX]{x1D707}(F)=\unicode[STIX]{x1D707}(G)$
and
$\unicode[STIX]{x1D707}(F)=\unicode[STIX]{x1D707}(G)$
and
 $\unicode[STIX]{x1D712}(F)/\text{r}(F)<\unicode[STIX]{x1D712}(G)/\text{r}(G)$
. Consider the dual map
$\unicode[STIX]{x1D712}(F)/\text{r}(F)<\unicode[STIX]{x1D712}(G)/\text{r}(G)$
. Consider the dual map
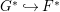 $G^{\ast }{\hookrightarrow}F^{\ast }$
. We have
$G^{\ast }{\hookrightarrow}F^{\ast }$
. We have
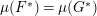 $\unicode[STIX]{x1D707}(F^{\ast })=\unicode[STIX]{x1D707}(G^{\ast })$
and
$\unicode[STIX]{x1D707}(F^{\ast })=\unicode[STIX]{x1D707}(G^{\ast })$
and
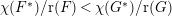 $\unicode[STIX]{x1D712}(F^{\ast })/\text{r}(F)<\unicode[STIX]{x1D712}(G^{\ast })/\text{r}(G)$
. So
$\unicode[STIX]{x1D712}(F^{\ast })/\text{r}(F)<\unicode[STIX]{x1D712}(G^{\ast })/\text{r}(G)$
. So
![]() $F^{\ast }$
is not stable. But applying (1) to the family
$F^{\ast }$
is not stable. But applying (1) to the family
![]() $\{F_{t}^{\ast }\}$
of dual bundles, we see that the subset of
$\{F_{t}^{\ast }\}$
of dual bundles, we see that the subset of
![]() $t\in T$
such that
$t\in T$
such that
![]() $F_{t}^{\ast }$
is not stable has codimension at least
$F_{t}^{\ast }$
is not stable has codimension at least
![]() $2$
. This proves (2).◻
$2$
. This proves (2).◻
Lemma 6.2. Let
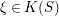 $\unicode[STIX]{x1D709}\in K(S)$
be a semistable class with symmetric
$\unicode[STIX]{x1D709}\in K(S)$
be a semistable class with symmetric
![]() $c_{1}$
such that the height of the moduli space
$c_{1}$
such that the height of the moduli space
![]() $M(\unicode[STIX]{x1D709})$
is positive. Then
$M(\unicode[STIX]{x1D709})$
is positive. Then
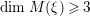 $\dim M(\unicode[STIX]{x1D709})\geqslant 3$
. If moreover
$\dim M(\unicode[STIX]{x1D709})\geqslant 3$
. If moreover
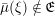 $\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})\notin \mathfrak{E}$
, then
$\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})\notin \mathfrak{E}$
, then
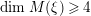 $\dim M(\unicode[STIX]{x1D709})\geqslant 4$
.
$\dim M(\unicode[STIX]{x1D709})\geqslant 4$
.
Proof. We imitate the proof of [Reference DrezetD, Proposition 33].
Put
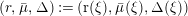 $(r,\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5}):=(\text{r}(\unicode[STIX]{x1D709}),\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}),\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709}))$
and
$(r,\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5}):=(\text{r}(\unicode[STIX]{x1D709}),\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}),\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709}))$
and
 $d:=\dim M(\unicode[STIX]{x1D709})>0$
. We have
$d:=\dim M(\unicode[STIX]{x1D709})>0$
. We have
 $$\begin{eqnarray}1-d=\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709},\unicode[STIX]{x1D709})=r^{2}(1-2\unicode[STIX]{x1D6E5}),\end{eqnarray}$$
$$\begin{eqnarray}1-d=\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709},\unicode[STIX]{x1D709})=r^{2}(1-2\unicode[STIX]{x1D6E5}),\end{eqnarray}$$
thus
 $$\begin{eqnarray}\unicode[STIX]{x1D6E5}=\frac{1}{2}\left(1+\frac{d-1}{r^{2}}\right)\!.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6E5}=\frac{1}{2}\left(1+\frac{d-1}{r^{2}}\right)\!.\end{eqnarray}$$
Take
![]() $\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
such that
$\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
such that
![]() $\bar{\unicode[STIX]{x1D707}}\in I_{\unicode[STIX]{x1D6FC}}$
. We may assume that
$\bar{\unicode[STIX]{x1D707}}\in I_{\unicode[STIX]{x1D6FC}}$
. We may assume that
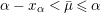 $\unicode[STIX]{x1D6FC}-x_{\unicode[STIX]{x1D6FC}}<\bar{\unicode[STIX]{x1D707}}\leqslant \unicode[STIX]{x1D6FC}$
. (If
$\unicode[STIX]{x1D6FC}-x_{\unicode[STIX]{x1D6FC}}<\bar{\unicode[STIX]{x1D707}}\leqslant \unicode[STIX]{x1D6FC}$
. (If
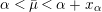 $\unicode[STIX]{x1D6FC}<\bar{\unicode[STIX]{x1D707}}<\unicode[STIX]{x1D6FC}+x_{\unicode[STIX]{x1D6FC}}$
, then consider the dual of sheaves.)
$\unicode[STIX]{x1D6FC}<\bar{\unicode[STIX]{x1D707}}<\unicode[STIX]{x1D6FC}+x_{\unicode[STIX]{x1D6FC}}$
, then consider the dual of sheaves.)
Claim.
If
![]() $d\leqslant 2$
, then we have
$d\leqslant 2$
, then we have
![]() $\bar{\unicode[STIX]{x1D707}}\neq \unicode[STIX]{x1D6FC}$
.
$\bar{\unicode[STIX]{x1D707}}\neq \unicode[STIX]{x1D6FC}$
.
Proof of the Claim.
If
![]() $\bar{\unicode[STIX]{x1D707}}=\unicode[STIX]{x1D6FC}$
, then
$\bar{\unicode[STIX]{x1D707}}=\unicode[STIX]{x1D6FC}$
, then
 $$\begin{eqnarray}\unicode[STIX]{x1D6E5}>1-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}=\frac{1}{2}\left(1+\frac{e}{r_{\unicode[STIX]{x1D6FC}}^{2}}\right)\!,\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6E5}>1-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}=\frac{1}{2}\left(1+\frac{e}{r_{\unicode[STIX]{x1D6FC}}^{2}}\right)\!,\end{eqnarray}$$
where
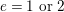 $e=1\text{ or }2$
depending on whether
$e=1\text{ or }2$
depending on whether
![]() $r_{\unicode[STIX]{x1D6FC}}$
is odd or even. By (6.19) and (6.20), we have
$r_{\unicode[STIX]{x1D6FC}}$
is odd or even. By (6.19) and (6.20), we have
 $\sqrt{d-1}r_{\unicode[STIX]{x1D6FC}}>r$
, thus
$\sqrt{d-1}r_{\unicode[STIX]{x1D6FC}}>r$
, thus
![]() $r_{\unicode[STIX]{x1D6FC}}>r$
. This is absurd because
$r_{\unicode[STIX]{x1D6FC}}>r$
. This is absurd because
![]() $r_{\unicode[STIX]{x1D6FC}}$
is the denominator of the irreducible fraction expression of
$r_{\unicode[STIX]{x1D6FC}}$
is the denominator of the irreducible fraction expression of
![]() $\unicode[STIX]{x1D6FC}$
. This is the end of the proof of the claim.◻
$\unicode[STIX]{x1D6FC}$
. This is the end of the proof of the claim.◻
To prove the lemma, we claim that if
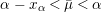 $\unicode[STIX]{x1D6FC}-x_{\unicode[STIX]{x1D6FC}}<\bar{\unicode[STIX]{x1D707}}<\unicode[STIX]{x1D6FC}$
, then
$\unicode[STIX]{x1D6FC}-x_{\unicode[STIX]{x1D6FC}}<\bar{\unicode[STIX]{x1D707}}<\unicode[STIX]{x1D6FC}$
, then
![]() $d\geqslant 4$
. Indeed, when
$d\geqslant 4$
. Indeed, when
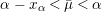 $\unicode[STIX]{x1D6FC}-x_{\unicode[STIX]{x1D6FC}}<\bar{\unicode[STIX]{x1D707}}<\unicode[STIX]{x1D6FC}$
, we have
$\unicode[STIX]{x1D6FC}-x_{\unicode[STIX]{x1D6FC}}<\bar{\unicode[STIX]{x1D707}}<\unicode[STIX]{x1D6FC}$
, we have
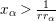 $x_{\unicode[STIX]{x1D6FC}}>\frac{1}{rr_{\unicode[STIX]{x1D6FC}}}$
. Denoting by
$x_{\unicode[STIX]{x1D6FC}}>\frac{1}{rr_{\unicode[STIX]{x1D6FC}}}$
. Denoting by
![]() $h$
the height of the moduli space, we have
$h$
the height of the moduli space, we have
 $$\begin{eqnarray}\displaystyle 0<h & = & \displaystyle rr_{\unicode[STIX]{x1D6FC}}\left(\unicode[STIX]{x1D6E5}+\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-\text{P}(\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FC})\right)<rr_{\unicode[STIX]{x1D6FC}}\left(\unicode[STIX]{x1D6E5}-\frac{1}{2}\right)\nonumber\\ \displaystyle & = & \displaystyle \frac{(d-1)r_{\unicode[STIX]{x1D6FC}}}{2r}\leqslant \frac{(d-1)r_{\unicode[STIX]{x1D6FC}}^{2}x_{\unicode[STIX]{x1D6FC}}}{2}\nonumber\\ \displaystyle & = & \displaystyle \frac{(d-1)e}{4\left(1+\sqrt{1-\frac{e}{2r_{\unicode[STIX]{x1D6FC}}^{2}}}\right)}\leqslant \frac{(d-1)e}{4},\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle 0<h & = & \displaystyle rr_{\unicode[STIX]{x1D6FC}}\left(\unicode[STIX]{x1D6E5}+\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}-\text{P}(\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FC})\right)<rr_{\unicode[STIX]{x1D6FC}}\left(\unicode[STIX]{x1D6E5}-\frac{1}{2}\right)\nonumber\\ \displaystyle & = & \displaystyle \frac{(d-1)r_{\unicode[STIX]{x1D6FC}}}{2r}\leqslant \frac{(d-1)r_{\unicode[STIX]{x1D6FC}}^{2}x_{\unicode[STIX]{x1D6FC}}}{2}\nonumber\\ \displaystyle & = & \displaystyle \frac{(d-1)e}{4\left(1+\sqrt{1-\frac{e}{2r_{\unicode[STIX]{x1D6FC}}^{2}}}\right)}\leqslant \frac{(d-1)e}{4},\nonumber\end{eqnarray}$$
where the inequality in the second line follows from
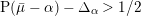 $\text{P}(\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FC})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}>1/2$
. We have
$\text{P}(\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FC})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FC}}>1/2$
. We have
 $$\begin{eqnarray}\hspace{132.00017pt}d>\frac{4h}{e}+1\geqslant 3.\hspace{102.00012pt}\square\end{eqnarray}$$
$$\begin{eqnarray}\hspace{132.00017pt}d>\frac{4h}{e}+1\geqslant 3.\hspace{102.00012pt}\square\end{eqnarray}$$
7 Properties of the set
 $\mathfrak{E}$
$\mathfrak{E}$
The goal of this section is Theorem 7.4, a counterpart of [Reference Coskun, Huizenga and WoolfCHW, Theorem 4.16].
7.1 Notation of continued fraction expansion
We use the same notation as in [Reference HuizengaH, Section 3], and follow the presentation there.
For real numbers
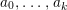 $a_{0},\ldots ,a_{k}$
, we define the number
$a_{0},\ldots ,a_{k}$
, we define the number
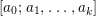 $[a_{0};a_{1},\ldots ,a_{k}]$
by
$[a_{0};a_{1},\ldots ,a_{k}]$
by
 $$\begin{eqnarray}[a_{0};a_{1},\ldots ,a_{k}]:=a_{0}+\displaystyle \frac{1}{a_{1}+\displaystyle \frac{1}{\ddots +\displaystyle \frac{1}{a_{k}}}},\end{eqnarray}$$
$$\begin{eqnarray}[a_{0};a_{1},\ldots ,a_{k}]:=a_{0}+\displaystyle \frac{1}{a_{1}+\displaystyle \frac{1}{\ddots +\displaystyle \frac{1}{a_{k}}}},\end{eqnarray}$$
when it makes sense.
Any rational number
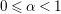 $0\leqslant \unicode[STIX]{x1D6FC}<1$
has a unique continued fraction expansion
$0\leqslant \unicode[STIX]{x1D6FC}<1$
has a unique continued fraction expansion
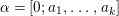 $\unicode[STIX]{x1D6FC}=[0;a_{1},\ldots ,a_{k}]$
where
$\unicode[STIX]{x1D6FC}=[0;a_{1},\ldots ,a_{k}]$
where
![]() $a_{i}$
are positive integers and
$a_{i}$
are positive integers and
![]() $k$
is even. This is called the even length continued fraction expansion of
$k$
is even. This is called the even length continued fraction expansion of
![]() $\unicode[STIX]{x1D6FC}$
. If
$\unicode[STIX]{x1D6FC}$
. If
![]() $p_{n}$
and
$p_{n}$
and
![]() $q_{n}$
are the numerator and denominator of
$q_{n}$
are the numerator and denominator of
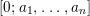 $[0;a_{1},\ldots ,a_{n}]$
, the
$[0;a_{1},\ldots ,a_{n}]$
, the
![]() $n$
th convergent of
$n$
th convergent of
![]() $\unicode[STIX]{x1D6FC}$
, then we have the relation
$\unicode[STIX]{x1D6FC}$
, then we have the relation
 $$\begin{eqnarray}\left(\begin{array}{@{}cc@{}}q_{n} & q_{n-1}\\ p_{n} & p_{n-1}\end{array}\right)=\left(\begin{array}{@{}cc@{}}a_{1} & 1\\ 1 & 0\end{array}\right)\left(\begin{array}{@{}cc@{}}a_{2} & 1\\ 1 & 0\end{array}\right)\ldots \left(\begin{array}{@{}cc@{}}a_{n} & 1\\ 1 & 0\end{array}\right).\end{eqnarray}$$
$$\begin{eqnarray}\left(\begin{array}{@{}cc@{}}q_{n} & q_{n-1}\\ p_{n} & p_{n-1}\end{array}\right)=\left(\begin{array}{@{}cc@{}}a_{1} & 1\\ 1 & 0\end{array}\right)\left(\begin{array}{@{}cc@{}}a_{2} & 1\\ 1 & 0\end{array}\right)\ldots \left(\begin{array}{@{}cc@{}}a_{n} & 1\\ 1 & 0\end{array}\right).\end{eqnarray}$$
From this, it follows that
 $q_{n}p_{n-1}-q_{n-1}p_{n}=(-1)^{n}$
. Moreover, taking the transpose of the above equation, we obtain the following fact [Reference HuizengaH, Lemma 3.1].
$q_{n}p_{n-1}-q_{n-1}p_{n}=(-1)^{n}$
. Moreover, taking the transpose of the above equation, we obtain the following fact [Reference HuizengaH, Lemma 3.1].
Lemma 7.1. A continued fraction expansion
 $[0;a_{1},\ldots ,a_{k}]$
is palindromic, that is,
$[0;a_{1},\ldots ,a_{k}]$
is palindromic, that is,
 $a_{i}=a_{k+1-i}$
, if and only if
$a_{i}=a_{k+1-i}$
, if and only if
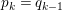 $p_{k}=q_{k-1}$
.
$p_{k}=q_{k-1}$
.
By the same reasoning, we have the following.
Lemma 7.2. Assume that for continued fraction expansions
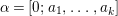 $\unicode[STIX]{x1D6FC}=[0;a_{1},\ldots ,a_{k}]$
and
$\unicode[STIX]{x1D6FC}=[0;a_{1},\ldots ,a_{k}]$
and
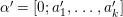 $\unicode[STIX]{x1D6FC}^{\prime }=[0;a_{1}^{\prime },\ldots ,a_{k}^{\prime }]$
, the inequalities
$\unicode[STIX]{x1D6FC}^{\prime }=[0;a_{1}^{\prime },\ldots ,a_{k}^{\prime }]$
, the inequalities
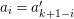 $a_{i}=a_{k+1-i}^{\prime }$
hold. Let
$a_{i}=a_{k+1-i}^{\prime }$
hold. Let
![]() $p_{n}/q_{n}$
and
$p_{n}/q_{n}$
and
![]() $p_{n}^{\prime }/q_{n}^{\prime }$
be the
$p_{n}^{\prime }/q_{n}^{\prime }$
be the
![]() $n$
th convergents of
$n$
th convergents of
![]() $\unicode[STIX]{x1D6FC}$
and
$\unicode[STIX]{x1D6FC}$
and
![]() $\unicode[STIX]{x1D6FC}^{\prime }$
, respectively. Then we have
$\unicode[STIX]{x1D6FC}^{\prime }$
, respectively. Then we have
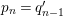 $p_{n}=q_{n-1}^{\prime }$
.
$p_{n}=q_{n-1}^{\prime }$
.
7.2 Continued fraction expansion of symmetric exceptional slopes
In the case of the projective plane, the even length continued fraction expansion of an exceptional slope
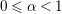 $0\leqslant \unicode[STIX]{x1D6FC}<1$
is palindromic (cf. [Reference HuizengaH, Theorem 3.2]). In our quadric surface case, the behavior of the even length continued fraction expansion of a symmetric exceptional slope
$0\leqslant \unicode[STIX]{x1D6FC}<1$
is palindromic (cf. [Reference HuizengaH, Theorem 3.2]). In our quadric surface case, the behavior of the even length continued fraction expansion of a symmetric exceptional slope
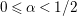 $0\leqslant \unicode[STIX]{x1D6FC}<1/2$
depends on the parity of
$0\leqslant \unicode[STIX]{x1D6FC}<1/2$
depends on the parity of
![]() $\unicode[STIX]{x1D6FC}$
.
$\unicode[STIX]{x1D6FC}$
.
Theorem 7.3. Take
![]() $\unicode[STIX]{x1D6FE}\in \mathfrak{E}$
with
$\unicode[STIX]{x1D6FE}\in \mathfrak{E}$
with
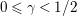 $0\leqslant \unicode[STIX]{x1D6FE}<1/2$
. Let
$0\leqslant \unicode[STIX]{x1D6FE}<1/2$
. Let
 $[0;c_{1},\ldots ,c_{n}]$
and
$[0;c_{1},\ldots ,c_{n}]$
and
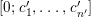 $[0;c_{1}^{\prime },\ldots ,c_{n^{\prime }}^{\prime }]$
be the even length continued fraction expansions of
$[0;c_{1}^{\prime },\ldots ,c_{n^{\prime }}^{\prime }]$
be the even length continued fraction expansions of
![]() $\unicode[STIX]{x1D6FE}$
and
$\unicode[STIX]{x1D6FE}$
and
![]() $2\unicode[STIX]{x1D6FE}$
, respectively. (If
$2\unicode[STIX]{x1D6FE}$
, respectively. (If
![]() $\unicode[STIX]{x1D6FE}=0$
, we understand
$\unicode[STIX]{x1D6FE}=0$
, we understand
 $n=n^{\prime }=0$
.)
$n=n^{\prime }=0$
.)
-
(1) We have
 $c_{1}=2\text{ or}$
3, and
$c_{1}=2\text{ or}$
3, and
 $c_{1}^{\prime }=1$
.
$c_{1}^{\prime }=1$
. -
(2) If the symmetric exceptional slope
 $\unicode[STIX]{x1D6FE}$
is even, then
$\unicode[STIX]{x1D6FE}$
is even, then
 $n^{\prime }=n+2$
, and the continued fraction expansions are palindromic.
$n^{\prime }=n+2$
, and the continued fraction expansions are palindromic. -
(3) If the symmetric exceptional slope
 $\unicode[STIX]{x1D6FE}$
is odd, then
$\unicode[STIX]{x1D6FE}$
is odd, then
 $n=n^{\prime }$
and
$n=n^{\prime }$
and
 $c_{i}=c_{n+1-i}^{\prime }$
.
$c_{i}=c_{n+1-i}^{\prime }$
.
Proof. We imitate the proof of [Reference HuizengaH, Theorem 3.2].
If
![]() $\unicode[STIX]{x1D6FE}=0$
, then the theorem holds obviously.
$\unicode[STIX]{x1D6FE}=0$
, then the theorem holds obviously.
Consider
![]() $\unicode[STIX]{x1D6FE}\in \mathfrak{E}$
with
$\unicode[STIX]{x1D6FE}\in \mathfrak{E}$
with
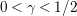 $0<\unicode[STIX]{x1D6FE}<1/2$
. It is expressed as
$0<\unicode[STIX]{x1D6FE}<1/2$
. It is expressed as
 $$\begin{eqnarray}\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D716}\left(p/2^{q}\right)\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D716}\left(p/2^{q}\right)\end{eqnarray}$$
with
![]() $p$
odd and
$p$
odd and
![]() $q\geqslant 2$
. We proceed by induction on
$q\geqslant 2$
. We proceed by induction on
![]() $q$
. But we first treat the case
$q$
. But we first treat the case
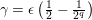 $\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D716}\left(\frac{1}{2}-\frac{1}{2^{q}}\right)$
separately. This
$\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D716}\left(\frac{1}{2}-\frac{1}{2^{q}}\right)$
separately. This
![]() $\unicode[STIX]{x1D6FE}$
is an odd symmetric exceptional slope. Since
$\unicode[STIX]{x1D6FE}$
is an odd symmetric exceptional slope. Since
 $$\begin{eqnarray}\unicode[STIX]{x1D716}\left(\frac{1}{2}-\frac{1}{2^{q+1}}\right)=\unicode[STIX]{x1D6FE}\,.\,\frac{1}{2},\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D716}\left(\frac{1}{2}-\frac{1}{2^{q+1}}\right)=\unicode[STIX]{x1D6FE}\,.\,\frac{1}{2},\end{eqnarray}$$
we have, by Lemma 2.6(2),
 $$\begin{eqnarray}\unicode[STIX]{x1D716}\left(\frac{1}{2}-\frac{1}{2^{q+1}}\right)=\frac{1}{2}-\frac{1}{6+4\unicode[STIX]{x1D6FE}}.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D716}\left(\frac{1}{2}-\frac{1}{2^{q+1}}\right)=\frac{1}{2}-\frac{1}{6+4\unicode[STIX]{x1D6FE}}.\end{eqnarray}$$
From this, by an easy calculation, we find
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D716}\left(\frac{1}{2}-\frac{1}{2^{q+1}}\right)=[0;2,1+\unicode[STIX]{x1D6FE}],\qquad 2\unicode[STIX]{x1D716}\left(\frac{1}{2}-\frac{1}{2^{q+1}}\right)=[0;1,2+2\unicode[STIX]{x1D6FE}]. & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D716}\left(\frac{1}{2}-\frac{1}{2^{q+1}}\right)=[0;2,1+\unicode[STIX]{x1D6FE}],\qquad 2\unicode[STIX]{x1D716}\left(\frac{1}{2}-\frac{1}{2^{q+1}}\right)=[0;1,2+2\unicode[STIX]{x1D6FE}]. & & \displaystyle \nonumber\end{eqnarray}$$
Since
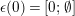 $\unicode[STIX]{x1D716}(0)=[0;\emptyset ]$
, we have
$\unicode[STIX]{x1D716}(0)=[0;\emptyset ]$
, we have
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D716}\left(\frac{1}{2}-\frac{1}{2^{q}}\right)=[0;(2,1)^{q-1}],\qquad 2\unicode[STIX]{x1D716}\left(\frac{1}{2}-\frac{1}{2^{q}}\right)=[0;(1,2)^{q-1}] & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D716}\left(\frac{1}{2}-\frac{1}{2^{q}}\right)=[0;(2,1)^{q-1}],\qquad 2\unicode[STIX]{x1D716}\left(\frac{1}{2}-\frac{1}{2^{q}}\right)=[0;(1,2)^{q-1}] & & \displaystyle \nonumber\end{eqnarray}$$
for
![]() $q\geqslant 2$
. Thus the theorem holds in this case.
$q\geqslant 2$
. Thus the theorem holds in this case.
Now let us consider
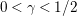 $0<\unicode[STIX]{x1D6FE}<1/2$
expressed as in (7.2). The case
$0<\unicode[STIX]{x1D6FE}<1/2$
expressed as in (7.2). The case
![]() $q=2$
is covered by the above consideration, so we let
$q=2$
is covered by the above consideration, so we let
![]() $q\geqslant 3$
. Let
$q\geqslant 3$
. Let
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D716}\left(\frac{p-1}{2^{q}}\right)\!,\qquad \unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D716}\left(\frac{p+1}{2^{q}}\right) & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D716}\left(\frac{p-1}{2^{q}}\right)\!,\qquad \unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D716}\left(\frac{p+1}{2^{q}}\right) & & \displaystyle \nonumber\end{eqnarray}$$
so that
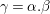 $\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}$
. We put the even length continued fraction expansions as follows:
$\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}$
. We put the even length continued fraction expansions as follows:
 $$\begin{eqnarray}\displaystyle \begin{array}{@{}rclcrc@{}}\unicode[STIX]{x1D6FC}\ & =\ & [0;a_{1},\ldots ,a_{m}], & \qquad 2\unicode[STIX]{x1D6FC} & =\ & [0;a_{1}^{\prime },\ldots ,a_{m^{\prime }}^{\prime }],~\\ \unicode[STIX]{x1D6FD}\ & =\ & [0;b_{1},\ldots ,b_{l}], & \qquad 2\unicode[STIX]{x1D6FD} & =\ & [0;b_{1}^{\prime },\ldots ,b_{l^{\prime }}^{\prime }].~\end{array} & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \begin{array}{@{}rclcrc@{}}\unicode[STIX]{x1D6FC}\ & =\ & [0;a_{1},\ldots ,a_{m}], & \qquad 2\unicode[STIX]{x1D6FC} & =\ & [0;a_{1}^{\prime },\ldots ,a_{m^{\prime }}^{\prime }],~\\ \unicode[STIX]{x1D6FD}\ & =\ & [0;b_{1},\ldots ,b_{l}], & \qquad 2\unicode[STIX]{x1D6FD} & =\ & [0;b_{1}^{\prime },\ldots ,b_{l^{\prime }}^{\prime }].~\end{array} & & \displaystyle \nonumber\end{eqnarray}$$
Claim 7.3.1. The even length continued fraction expansions of
![]() $\unicode[STIX]{x1D6FE}$
and
$\unicode[STIX]{x1D6FE}$
and
![]() $2\unicode[STIX]{x1D6FE}$
are given as follows.
$2\unicode[STIX]{x1D6FE}$
are given as follows.
-
(1) If
 $\unicode[STIX]{x1D6FC}$
is even, then (7.3)
$\unicode[STIX]{x1D6FC}$
is even, then (7.3) $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FE} & = & \displaystyle [0;a_{1},\ldots ,a_{m},1,1,b_{1}-1,b_{2},\ldots ,b_{l}]\end{eqnarray}$$
(7.4)
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FE} & = & \displaystyle [0;a_{1},\ldots ,a_{m},1,1,b_{1}-1,b_{2},\ldots ,b_{l}]\end{eqnarray}$$
(7.4) $$\begin{eqnarray}\displaystyle 2\unicode[STIX]{x1D6FE} & = & \displaystyle [0;a_{1}^{\prime },\ldots ,a_{m^{\prime }}^{\prime },3,b_{2}^{\prime }+1,b_{3}^{\prime },\ldots ,b_{l^{\prime }}^{\prime }].\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle 2\unicode[STIX]{x1D6FE} & = & \displaystyle [0;a_{1}^{\prime },\ldots ,a_{m^{\prime }}^{\prime },3,b_{2}^{\prime }+1,b_{3}^{\prime },\ldots ,b_{l^{\prime }}^{\prime }].\end{eqnarray}$$
-
(2) If
 $\unicode[STIX]{x1D6FC}$
is odd, then (7.5)
$\unicode[STIX]{x1D6FC}$
is odd, then (7.5) $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FE} & = & \displaystyle [0;a_{1},\ldots ,a_{m},3,b_{2}^{\prime }+1,b_{3}^{\prime },\ldots ,b_{l^{\prime }}^{\prime }]\end{eqnarray}$$
(7.6)
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FE} & = & \displaystyle [0;a_{1},\ldots ,a_{m},3,b_{2}^{\prime }+1,b_{3}^{\prime },\ldots ,b_{l^{\prime }}^{\prime }]\end{eqnarray}$$
(7.6) $$\begin{eqnarray}\displaystyle 2\unicode[STIX]{x1D6FE} & = & \displaystyle [0;a_{1}^{\prime },\ldots ,a_{m^{\prime }}^{\prime },1,1,,b_{1}-1,b_{2},\ldots ,b_{l}].\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle 2\unicode[STIX]{x1D6FE} & = & \displaystyle [0;a_{1}^{\prime },\ldots ,a_{m^{\prime }}^{\prime },1,1,,b_{1}-1,b_{2},\ldots ,b_{l}].\end{eqnarray}$$
-
(3) If
 $\unicode[STIX]{x1D6FD}$
is even, then (7.7)
$\unicode[STIX]{x1D6FD}$
is even, then (7.7) $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FE} & = & \displaystyle [0;b_{1},\ldots ,b_{l-1},b_{l}-1,1,1,a_{1},\ldots ,a_{m}]\end{eqnarray}$$
(7.8)
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FE} & = & \displaystyle [0;b_{1},\ldots ,b_{l-1},b_{l}-1,1,1,a_{1},\ldots ,a_{m}]\end{eqnarray}$$
(7.8) $$\begin{eqnarray}\displaystyle 2\unicode[STIX]{x1D6FE} & = & \displaystyle [0;b_{1}^{\prime },\ldots ,b_{l^{\prime }-2}^{\prime },b_{l^{\prime }-1}^{\prime }+1,3,a_{1}^{\prime },\ldots ,a_{m^{\prime }}^{\prime }].\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle 2\unicode[STIX]{x1D6FE} & = & \displaystyle [0;b_{1}^{\prime },\ldots ,b_{l^{\prime }-2}^{\prime },b_{l^{\prime }-1}^{\prime }+1,3,a_{1}^{\prime },\ldots ,a_{m^{\prime }}^{\prime }].\end{eqnarray}$$
-
(4) If
 $\unicode[STIX]{x1D6FD}$
is odd, then (7.9)
$\unicode[STIX]{x1D6FD}$
is odd, then (7.9) $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FE} & = & \displaystyle [0;b_{1},\ldots ,b_{l-2},b_{l-1}+1,3,a_{1}^{\prime },\ldots ,a_{m^{\prime }}^{\prime }]\end{eqnarray}$$
(7.10)
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FE} & = & \displaystyle [0;b_{1},\ldots ,b_{l-2},b_{l-1}+1,3,a_{1}^{\prime },\ldots ,a_{m^{\prime }}^{\prime }]\end{eqnarray}$$
(7.10) $$\begin{eqnarray}\displaystyle 2\unicode[STIX]{x1D6FE} & = & \displaystyle [0;b_{1}^{\prime },\ldots ,b_{l^{\prime }-1}^{\prime },b_{l^{\prime }}^{\prime }-1,1,1,a_{1},\ldots ,a_{m}].\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle 2\unicode[STIX]{x1D6FE} & = & \displaystyle [0;b_{1}^{\prime },\ldots ,b_{l^{\prime }-1}^{\prime },b_{l^{\prime }}^{\prime }-1,1,1,a_{1},\ldots ,a_{m}].\end{eqnarray}$$
We first see that the claim implies the theorem. If
![]() $\unicode[STIX]{x1D6FE}$
is even, then both
$\unicode[STIX]{x1D6FE}$
is even, then both
![]() $\unicode[STIX]{x1D6FC}$
and
$\unicode[STIX]{x1D6FC}$
and
![]() $\unicode[STIX]{x1D6FD}$
are odd. Comparing the two expressions (7.5) and (7.9) of the continued fraction expansion of
$\unicode[STIX]{x1D6FD}$
are odd. Comparing the two expressions (7.5) and (7.9) of the continued fraction expansion of
![]() $\unicode[STIX]{x1D6FE}$
, we infer that the expansion is palindromic, using the induction hypothesis. For the expansion of
$\unicode[STIX]{x1D6FE}$
, we infer that the expansion is palindromic, using the induction hypothesis. For the expansion of
![]() $2\unicode[STIX]{x1D6FE}$
, we can argue similarly. If
$2\unicode[STIX]{x1D6FE}$
, we can argue similarly. If
![]() $\unicode[STIX]{x1D6FE}$
is odd, then one of
$\unicode[STIX]{x1D6FE}$
is odd, then one of
![]() $\unicode[STIX]{x1D6FC}$
,
$\unicode[STIX]{x1D6FC}$
,
![]() $\unicode[STIX]{x1D6FD}$
is odd, and the other is even. Comparing (7.3), (7.4) and (7.9), (7.10), or (7.5), (7.6) and (7.7), (7.8), we can see that the theorem holds in this case as well.
$\unicode[STIX]{x1D6FD}$
is odd, and the other is even. Comparing (7.3), (7.4) and (7.9), (7.10), or (7.5), (7.6) and (7.7), (7.8), we can see that the theorem holds in this case as well.
It remains to prove the claim. We give a proof for the equalities (7.4) and (7.9); the other equalities can be proved similarly.
Assume that
![]() $\unicode[STIX]{x1D6FC}$
is even. Let
$\unicode[STIX]{x1D6FC}$
is even. Let
![]() $p_{k}^{\prime }/q_{k}^{\prime }$
be the
$p_{k}^{\prime }/q_{k}^{\prime }$
be the
![]() $k$
th convergent of
$k$
th convergent of
![]() $2\unicode[STIX]{x1D6FC}$
, that is,
$2\unicode[STIX]{x1D6FC}$
, that is,
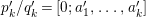 $p_{k}^{\prime }/q_{k}^{\prime }=[0;a_{1}^{\prime },\ldots ,a_{k}^{\prime }]$
. We have
$p_{k}^{\prime }/q_{k}^{\prime }=[0;a_{1}^{\prime },\ldots ,a_{k}^{\prime }]$
. We have
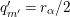 $q_{m^{\prime }}^{\prime }=r_{\unicode[STIX]{x1D6FC}}/2$
and
$q_{m^{\prime }}^{\prime }=r_{\unicode[STIX]{x1D6FC}}/2$
and
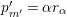 $p_{m^{\prime }}^{\prime }=\unicode[STIX]{x1D6FC}r_{\unicode[STIX]{x1D6FC}}$
. Since the continued fraction expansion of
$p_{m^{\prime }}^{\prime }=\unicode[STIX]{x1D6FC}r_{\unicode[STIX]{x1D6FC}}$
. Since the continued fraction expansion of
![]() $2\unicode[STIX]{x1D6FC}$
is palindromic, we have
$2\unicode[STIX]{x1D6FC}$
is palindromic, we have
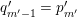 $q_{m^{\prime }-1}^{\prime }=p_{m^{\prime }}^{\prime }$
by Lemma 7.1. By
$q_{m^{\prime }-1}^{\prime }=p_{m^{\prime }}^{\prime }$
by Lemma 7.1. By
 $q_{m^{\prime }}^{\prime }p_{m^{\prime }-1}^{\prime }-p_{m^{\prime }}^{\prime }q_{m^{\prime }-1}^{\prime }=1$
, we have
$q_{m^{\prime }}^{\prime }p_{m^{\prime }-1}^{\prime }-p_{m^{\prime }}^{\prime }q_{m^{\prime }-1}^{\prime }=1$
, we have
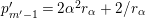 $p_{m^{\prime }-1}^{\prime }=2\unicode[STIX]{x1D6FC}^{2}r_{\unicode[STIX]{x1D6FC}}+2/r_{\unicode[STIX]{x1D6FC}}$
. Using by Lemma 2.6(2), we have
$p_{m^{\prime }-1}^{\prime }=2\unicode[STIX]{x1D6FC}^{2}r_{\unicode[STIX]{x1D6FC}}+2/r_{\unicode[STIX]{x1D6FC}}$
. Using by Lemma 2.6(2), we have
 $$\begin{eqnarray}\displaystyle 2\unicode[STIX]{x1D6FE}=2\unicode[STIX]{x1D6FC}+\frac{2}{r_{\unicode[STIX]{x1D6FC}}^{2}(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})} & = & \displaystyle \frac{(4-2\unicode[STIX]{x1D6FD})\unicode[STIX]{x1D6FC}r_{\unicode[STIX]{x1D6FC}}+2\unicode[STIX]{x1D6FC}^{2}r_{\unicode[STIX]{x1D6FC}}+2/r_{\unicode[STIX]{x1D6FC}}}{(4-2\unicode[STIX]{x1D6FD})r_{\unicode[STIX]{x1D6FC}}/2+\unicode[STIX]{x1D6FC}r_{\unicode[STIX]{x1D6FC}}}\nonumber\\ \displaystyle & = & \displaystyle \frac{(4-2\unicode[STIX]{x1D6FD})p_{m^{\prime }}^{\prime }+p_{m^{\prime }-1}^{\prime }}{(4-2\unicode[STIX]{x1D6FD})q_{m^{\prime }}^{\prime }+q_{m^{\prime }-1}^{\prime }}\nonumber\\ \displaystyle & = & \displaystyle [0;a_{1}^{\prime },\ldots ,a_{m^{\prime }}^{\prime },4-2\unicode[STIX]{x1D6FD}].\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle 2\unicode[STIX]{x1D6FE}=2\unicode[STIX]{x1D6FC}+\frac{2}{r_{\unicode[STIX]{x1D6FC}}^{2}(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})} & = & \displaystyle \frac{(4-2\unicode[STIX]{x1D6FD})\unicode[STIX]{x1D6FC}r_{\unicode[STIX]{x1D6FC}}+2\unicode[STIX]{x1D6FC}^{2}r_{\unicode[STIX]{x1D6FC}}+2/r_{\unicode[STIX]{x1D6FC}}}{(4-2\unicode[STIX]{x1D6FD})r_{\unicode[STIX]{x1D6FC}}/2+\unicode[STIX]{x1D6FC}r_{\unicode[STIX]{x1D6FC}}}\nonumber\\ \displaystyle & = & \displaystyle \frac{(4-2\unicode[STIX]{x1D6FD})p_{m^{\prime }}^{\prime }+p_{m^{\prime }-1}^{\prime }}{(4-2\unicode[STIX]{x1D6FD})q_{m^{\prime }}^{\prime }+q_{m^{\prime }-1}^{\prime }}\nonumber\\ \displaystyle & = & \displaystyle [0;a_{1}^{\prime },\ldots ,a_{m^{\prime }}^{\prime },4-2\unicode[STIX]{x1D6FD}].\end{eqnarray}$$
Using
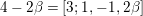 $4-2\unicode[STIX]{x1D6FD}=[3;1,-1,2\unicode[STIX]{x1D6FD}]$
, the continued fraction expansion (7.11) can be rewritten as (7.4), using
$4-2\unicode[STIX]{x1D6FD}=[3;1,-1,2\unicode[STIX]{x1D6FD}]$
, the continued fraction expansion (7.11) can be rewritten as (7.4), using
![]() $b_{1}^{\prime }=1$
.
$b_{1}^{\prime }=1$
.
Finally assume that
![]() $\unicode[STIX]{x1D6FD}$
is odd. Let
$\unicode[STIX]{x1D6FD}$
is odd. Let
![]() $p_{k}/q_{k}$
and
$p_{k}/q_{k}$
and
![]() $p_{k}^{\prime }/q_{k}^{\prime }$
be the
$p_{k}^{\prime }/q_{k}^{\prime }$
be the
![]() $k$
th convergents of
$k$
th convergents of
![]() $\unicode[STIX]{x1D6FD}$
and
$\unicode[STIX]{x1D6FD}$
and
![]() $2\unicode[STIX]{x1D6FD}$
, respectively. We have
$2\unicode[STIX]{x1D6FD}$
, respectively. We have
![]() $q_{l}=r_{\unicode[STIX]{x1D6FD}}$
and
$q_{l}=r_{\unicode[STIX]{x1D6FD}}$
and
 $p_{l}=\unicode[STIX]{x1D6FD}r_{\unicode[STIX]{x1D6FD}}$
. Since
$p_{l}=\unicode[STIX]{x1D6FD}r_{\unicode[STIX]{x1D6FD}}$
. Since
![]() $l=l^{\prime }$
and
$l=l^{\prime }$
and
 $b_{i}=b_{l+1-i}^{\prime }$
, we have
$b_{i}=b_{l+1-i}^{\prime }$
, we have
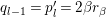 $q_{l-1}=p_{l}^{\prime }=2\unicode[STIX]{x1D6FD}r_{\unicode[STIX]{x1D6FD}}$
by Lemma 7.2. So we have
$q_{l-1}=p_{l}^{\prime }=2\unicode[STIX]{x1D6FD}r_{\unicode[STIX]{x1D6FD}}$
by Lemma 7.2. So we have
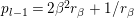 $p_{l-1}=2\unicode[STIX]{x1D6FD}^{2}r_{\unicode[STIX]{x1D6FD}}+1/r_{\unicode[STIX]{x1D6FD}}$
.
$p_{l-1}=2\unicode[STIX]{x1D6FD}^{2}r_{\unicode[STIX]{x1D6FD}}+1/r_{\unicode[STIX]{x1D6FD}}$
.
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D6FD}-\frac{1}{2r_{\unicode[STIX]{x1D6FD}}^{2}(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})} & = & \displaystyle \frac{-(4+2\unicode[STIX]{x1D6FC})\unicode[STIX]{x1D6FD}r_{\unicode[STIX]{x1D6FD}}+2\unicode[STIX]{x1D6FD}^{2}r_{\unicode[STIX]{x1D6FD}}+1/r_{\unicode[STIX]{x1D6FD}}}{-(4+2\unicode[STIX]{x1D6FC})r_{\unicode[STIX]{x1D6FD}}+2\unicode[STIX]{x1D6FD}r_{\unicode[STIX]{x1D6FD}}}\nonumber\\ \displaystyle & = & \displaystyle \frac{-(4+2\unicode[STIX]{x1D6FC})p_{l}+p_{l-1}}{-(4+2\unicode[STIX]{x1D6FC})q_{l}+q_{l-1}}\nonumber\\ \displaystyle & = & \displaystyle [0;b_{1},\ldots ,b_{l},-(4+2\unicode[STIX]{x1D6FC})]\nonumber\\ \displaystyle & = & \displaystyle [0;b_{1},\ldots ,b_{l-1},1,-(4+2\unicode[STIX]{x1D6FC})].\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D6FD}-\frac{1}{2r_{\unicode[STIX]{x1D6FD}}^{2}(2+\unicode[STIX]{x1D6FC}-\unicode[STIX]{x1D6FD})} & = & \displaystyle \frac{-(4+2\unicode[STIX]{x1D6FC})\unicode[STIX]{x1D6FD}r_{\unicode[STIX]{x1D6FD}}+2\unicode[STIX]{x1D6FD}^{2}r_{\unicode[STIX]{x1D6FD}}+1/r_{\unicode[STIX]{x1D6FD}}}{-(4+2\unicode[STIX]{x1D6FC})r_{\unicode[STIX]{x1D6FD}}+2\unicode[STIX]{x1D6FD}r_{\unicode[STIX]{x1D6FD}}}\nonumber\\ \displaystyle & = & \displaystyle \frac{-(4+2\unicode[STIX]{x1D6FC})p_{l}+p_{l-1}}{-(4+2\unicode[STIX]{x1D6FC})q_{l}+q_{l-1}}\nonumber\\ \displaystyle & = & \displaystyle [0;b_{1},\ldots ,b_{l},-(4+2\unicode[STIX]{x1D6FC})]\nonumber\\ \displaystyle & = & \displaystyle [0;b_{1},\ldots ,b_{l-1},1,-(4+2\unicode[STIX]{x1D6FC})].\end{eqnarray}$$
Using
 $[0;1;-(4+2\unicode[STIX]{x1D6FC})]=[1;3+2\unicode[STIX]{x1D6FC}]$
, we can rewrite (7.12) as (7.9).◻
$[0;1;-(4+2\unicode[STIX]{x1D6FC})]=[1;3+2\unicode[STIX]{x1D6FC}]$
, we can rewrite (7.12) as (7.9).◻
Put
 $C:=\mathbb{R}\setminus \bigcup _{\unicode[STIX]{x1D6FC}\in \mathfrak{E}}I_{\unicode[STIX]{x1D6FC}}$
. Any
$C:=\mathbb{R}\setminus \bigcup _{\unicode[STIX]{x1D6FC}\in \mathfrak{E}}I_{\unicode[STIX]{x1D6FC}}$
. Any
![]() $x\in C$
is irrational.
$x\in C$
is irrational.
Theorem 7.4. If
![]() $x\in C$
is not an endpoint of an interval
$x\in C$
is not an endpoint of an interval
![]() $I_{\unicode[STIX]{x1D6FC}}$
, then it is not a quadratic irrational number.
$I_{\unicode[STIX]{x1D6FC}}$
, then it is not a quadratic irrational number.
To prove the theorem, we follow the argument in [Reference Coskun, Huizenga and WoolfCHW, Section 4]. In our case, the argument becomes messier due to the parity of symmetric exceptional slopes.
To show that
![]() $x\in C$
in the theorem is not a quadratic irrational number, we use the following fact : if
$x\in C$
in the theorem is not a quadratic irrational number, we use the following fact : if
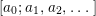 $[a_{0};a_{1},a_{2},\ldots \,]$
is a continued fraction expansion of an irrational number, then it is a quadratic irrational number if and only if the continued fraction expansion is eventually periodic, that is, there exist positive integers
$[a_{0};a_{1},a_{2},\ldots \,]$
is a continued fraction expansion of an irrational number, then it is a quadratic irrational number if and only if the continued fraction expansion is eventually periodic, that is, there exist positive integers
![]() $p$
and
$p$
and
![]() $c$
such that
$c$
such that
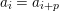 $a_{i}=a_{i+p}$
for all
$a_{i}=a_{i+p}$
for all
![]() $i>c$
. So, we will study the continued fraction expansion of
$i>c$
. So, we will study the continued fraction expansion of
![]() $x$
.
$x$
.
We need some preparation.
Put
 $C^{\prime }=C\cap (0,1/2)$
and
$C^{\prime }=C\cap (0,1/2)$
and
 $$\begin{eqnarray}C_{n}=(0,1/2)\Big\backslash\mathop{\bigcup }_{\unicode[STIX]{x1D6FC}\in \mathfrak{E},\;\text{ord}\,\unicode[STIX]{x1D6FC}\leqslant n}I_{\unicode[STIX]{x1D6FC}}.\end{eqnarray}$$
$$\begin{eqnarray}C_{n}=(0,1/2)\Big\backslash\mathop{\bigcup }_{\unicode[STIX]{x1D6FC}\in \mathfrak{E},\;\text{ord}\,\unicode[STIX]{x1D6FC}\leqslant n}I_{\unicode[STIX]{x1D6FC}}.\end{eqnarray}$$
Here the order of a symmetric exceptional slope
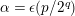 $\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D716}(p/2^{q})$
, where
$\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D716}(p/2^{q})$
, where
![]() $p$
is odd if
$p$
is odd if
![]() $q>0$
, is defined by
$q>0$
, is defined by
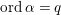 $\text{ord}\,\unicode[STIX]{x1D6FC}=q$
.
$\text{ord}\,\unicode[STIX]{x1D6FC}=q$
.
To each
![]() $x\in C^{\prime }$
, we associate an infinite sequence
$x\in C^{\prime }$
, we associate an infinite sequence
![]() $\unicode[STIX]{x1D70E}_{x}$
of letters
$\unicode[STIX]{x1D70E}_{x}$
of letters
![]() $L$
and
$L$
and
![]() $R$
as follows. We have
$R$
as follows. We have
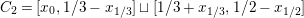 $C_{2}=[x_{0},1/3-x_{1/3}]\sqcup [1/3+x_{1/3},1/2-x_{1/2}]$
, and
$C_{2}=[x_{0},1/3-x_{1/3}]\sqcup [1/3+x_{1/3},1/2-x_{1/2}]$
, and
![]() $x$
lies in one of the two intervals. If it lies in the left (resp. right) interval, then the first term of
$x$
lies in one of the two intervals. If it lies in the left (resp. right) interval, then the first term of
![]() $\unicode[STIX]{x1D70E}_{x}$
is
$\unicode[STIX]{x1D70E}_{x}$
is
![]() $L$
(resp.
$L$
(resp.
![]() $R$
). If we denote by
$R$
). If we denote by
![]() $I_{2,x}$
the interval of
$I_{2,x}$
the interval of
![]() $C_{2}$
containing
$C_{2}$
containing
![]() $x$
, then
$x$
, then
 $I_{2,x}\cap C_{3}$
consists of two disjoint intervals, and
$I_{2,x}\cap C_{3}$
consists of two disjoint intervals, and
![]() $x$
lies in one of them. If it lies in the left (resp. right) interval, then the second term of
$x$
lies in one of them. If it lies in the left (resp. right) interval, then the second term of
![]() $\unicode[STIX]{x1D70E}_{x}$
is
$\unicode[STIX]{x1D70E}_{x}$
is
![]() $L$
(resp.
$L$
(resp.
![]() $R$
). We can proceed inductively. More precisely, if we denote by
$R$
). We can proceed inductively. More precisely, if we denote by
![]() $I_{n,x}$
the interval of
$I_{n,x}$
the interval of
![]() $C_{n}$
containing
$C_{n}$
containing
![]() $x$
, then
$x$
, then
 $I_{n,x}\cap C_{n+1}$
consists of two disjoint intervals, and
$I_{n,x}\cap C_{n+1}$
consists of two disjoint intervals, and
![]() $x$
lies in one of them. If it lies in the left (resp. right) interval, then the
$x$
lies in one of them. If it lies in the left (resp. right) interval, then the
![]() $n$
th term of
$n$
th term of
![]() $\unicode[STIX]{x1D70E}_{x}$
is
$\unicode[STIX]{x1D70E}_{x}$
is
![]() $L$
(resp.
$L$
(resp.
![]() $R$
).
$R$
).
The correspondence
![]() $x\rightarrow \unicode[STIX]{x1D70E}_{x}$
gives a bijection between the set
$x\rightarrow \unicode[STIX]{x1D70E}_{x}$
gives a bijection between the set
![]() $C^{\prime }$
and the set
$C^{\prime }$
and the set
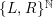 $\{L,R\}^{\mathbb{N}}$
of infinite sequences of letters
$\{L,R\}^{\mathbb{N}}$
of infinite sequences of letters
![]() $L$
and
$L$
and
![]() $R$
. The following lemma is clear from the construction of
$R$
. The following lemma is clear from the construction of
![]() $\unicode[STIX]{x1D70E}_{x}$
.
$\unicode[STIX]{x1D70E}_{x}$
.
Lemma 7.5. For
![]() $x\in C^{\prime }$
,
$x\in C^{\prime }$
,
![]() $x$
is an endpoint of an interval
$x$
is an endpoint of an interval
![]() $I_{\unicode[STIX]{x1D6FC}}$
,
$I_{\unicode[STIX]{x1D6FC}}$
,
![]() $\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
, if and only if the infinite sequence
$\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
, if and only if the infinite sequence
![]() $\unicode[STIX]{x1D70E}_{x}$
is eventually constant, that is, there exists an
$\unicode[STIX]{x1D70E}_{x}$
is eventually constant, that is, there exists an
![]() $N>0$
such that all letters in
$N>0$
such that all letters in
![]() $\unicode[STIX]{x1D70E}_{x}$
are the same after the
$\unicode[STIX]{x1D70E}_{x}$
are the same after the
![]() $N$
th term.
$N$
th term.
Consider a symmetric exceptional slope
![]() $\unicode[STIX]{x1D6FE}\in \mathfrak{E}$
expressed as
$\unicode[STIX]{x1D6FE}\in \mathfrak{E}$
expressed as
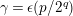 $\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D716}(p/2^{q})$
where
$\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D716}(p/2^{q})$
where
![]() $q>0$
and
$q>0$
and
![]() $p$
is odd. Let
$p$
is odd. Let
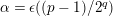 $\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D716}((p-1)/2^{q})$
and
$\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D716}((p-1)/2^{q})$
and
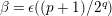 $\unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D716}((p+1)/2^{q})$
so that
$\unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D716}((p+1)/2^{q})$
so that
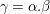 $\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}$
. We define the symmetric exceptional slopes
$\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}$
. We define the symmetric exceptional slopes
![]() $\unicode[STIX]{x1D6FE}\cdot L$
and
$\unicode[STIX]{x1D6FE}\cdot L$
and
![]() $\unicode[STIX]{x1D6FE}\cdot R$
by
$\unicode[STIX]{x1D6FE}\cdot R$
by
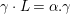 $\unicode[STIX]{x1D6FE}\cdot L=\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FE}$
and
$\unicode[STIX]{x1D6FE}\cdot L=\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FE}$
and
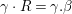 $\unicode[STIX]{x1D6FE}\cdot R=\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}$
. If
$\unicode[STIX]{x1D6FE}\cdot R=\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}$
. If
 $\unicode[STIX]{x1D70E}=(S_{1},\ldots ,S_{n})$
is a finite sequence of letters
$\unicode[STIX]{x1D70E}=(S_{1},\ldots ,S_{n})$
is a finite sequence of letters
![]() $L$
and
$L$
and
![]() $R$
, then we define the symmetric exceptional slope
$R$
, then we define the symmetric exceptional slope
![]() $\unicode[STIX]{x1D6FE}\cdot \unicode[STIX]{x1D70E}$
by
$\unicode[STIX]{x1D6FE}\cdot \unicode[STIX]{x1D70E}$
by
 $(\ldots ((\unicode[STIX]{x1D6FE}\cdot S_{1})\cdot S_{2})\ldots \,)\cdot S_{n}$
.
$(\ldots ((\unicode[STIX]{x1D6FE}\cdot S_{1})\cdot S_{2})\ldots \,)\cdot S_{n}$
.
Let
![]() $\mathfrak{E}^{\prime }$
be the set of symmetric exceptional slopes
$\mathfrak{E}^{\prime }$
be the set of symmetric exceptional slopes
![]() $\unicode[STIX]{x1D6FC}$
with
$\unicode[STIX]{x1D6FC}$
with
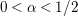 $0<\unicode[STIX]{x1D6FC}<1/2$
. If we note
$0<\unicode[STIX]{x1D6FC}<1/2$
. If we note
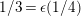 $1/3=\unicode[STIX]{x1D716}(1/4)$
, the following is clear.
$1/3=\unicode[STIX]{x1D716}(1/4)$
, the following is clear.
Lemma 7.6. Denote by
 $\{L,R\}^{\ast }$
the set of finite sequences of letters
$\{L,R\}^{\ast }$
the set of finite sequences of letters
![]() $L$
and
$L$
and
![]() $R$
(including the empty sequence). The map
$R$
(including the empty sequence). The map
 $\{L,R\}^{\ast }\rightarrow \mathfrak{E}^{\prime }$
given by
$\{L,R\}^{\ast }\rightarrow \mathfrak{E}^{\prime }$
given by
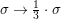 $\unicode[STIX]{x1D70E}\rightarrow \frac{1}{3}\cdot \unicode[STIX]{x1D70E}$
is a bijection.
$\unicode[STIX]{x1D70E}\rightarrow \frac{1}{3}\cdot \unicode[STIX]{x1D70E}$
is a bijection.
Continued fractions Take
![]() $\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
, and let
$\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
, and let
![]() $\unicode[STIX]{x1D6FD}$
be
$\unicode[STIX]{x1D6FD}$
be
![]() $\unicode[STIX]{x1D6FC}\cdot L$
or
$\unicode[STIX]{x1D6FC}\cdot L$
or
![]() $\unicode[STIX]{x1D6FC}\cdot R$
. Let
$\unicode[STIX]{x1D6FC}\cdot R$
. Let
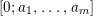 $[0;a_{1},\ldots ,a_{m}]$
and
$[0;a_{1},\ldots ,a_{m}]$
and
 $[0;b_{1},\ldots ,b_{n}]$
be the even length continued fraction expressions of
$[0;b_{1},\ldots ,b_{n}]$
be the even length continued fraction expressions of
![]() $\unicode[STIX]{x1D6FC}$
and
$\unicode[STIX]{x1D6FC}$
and
![]() $\unicode[STIX]{x1D6FD}$
respectively. Then from Claim 7.3.1, we see that
$\unicode[STIX]{x1D6FD}$
respectively. Then from Claim 7.3.1, we see that
![]() $a_{i}=b_{i}$
for
$a_{i}=b_{i}$
for
 $1\leqslant i\leqslant m-2$
.
$1\leqslant i\leqslant m-2$
.
Take
![]() $x\in C^{\prime }$
and consider the corresponding infinite sequence
$x\in C^{\prime }$
and consider the corresponding infinite sequence
![]() $\unicode[STIX]{x1D70E}_{x}$
. Let
$\unicode[STIX]{x1D70E}_{x}$
. Let
![]() $\unicode[STIX]{x1D70E}_{x}^{{\leqslant}n}$
be the finite sequence of initial
$\unicode[STIX]{x1D70E}_{x}^{{\leqslant}n}$
be the finite sequence of initial
![]() $n$
terms. Put
$n$
terms. Put
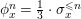 $\unicode[STIX]{x1D719}_{x}^{n}=\frac{1}{3}\cdot \unicode[STIX]{x1D70E}_{x}^{{\leqslant}n}$
. We have
$\unicode[STIX]{x1D719}_{x}^{n}=\frac{1}{3}\cdot \unicode[STIX]{x1D70E}_{x}^{{\leqslant}n}$
. We have
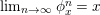 $\lim _{n\rightarrow \infty }\unicode[STIX]{x1D719}_{x}^{n}=x$
. From the observation above, the following lemma is clear.
$\lim _{n\rightarrow \infty }\unicode[STIX]{x1D719}_{x}^{n}=x$
. From the observation above, the following lemma is clear.
Lemma 7.7. If
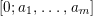 $[0;a_{1},\ldots ,a_{m}]$
is the even length continued fraction expansion of
$[0;a_{1},\ldots ,a_{m}]$
is the even length continued fraction expansion of
![]() $\unicode[STIX]{x1D719}_{x}^{n}$
, and
$\unicode[STIX]{x1D719}_{x}^{n}$
, and
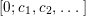 $[0;c_{1},c_{2},\ldots \,]$
is the continued fraction expansion of
$[0;c_{1},c_{2},\ldots \,]$
is the continued fraction expansion of
![]() $x$
, then
$x$
, then
![]() $a_{i}=c_{i}$
for
$a_{i}=c_{i}$
for
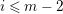 $i\leqslant m-2$
.
$i\leqslant m-2$
.
Now assume that
![]() $x\in C^{\prime }$
is not an endpoint of
$x\in C^{\prime }$
is not an endpoint of
![]() $I_{\unicode[STIX]{x1D6FC}}$
,
$I_{\unicode[STIX]{x1D6FC}}$
,
![]() $\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
.
$\unicode[STIX]{x1D6FC}\in \mathfrak{E}$
.
By Lemma 7.5,
![]() $\unicode[STIX]{x1D70E}_{x}$
is not eventually constant. Then we can easily see that there are infinitely many even symmetric exceptional slopes in the sequence
$\unicode[STIX]{x1D70E}_{x}$
is not eventually constant. Then we can easily see that there are infinitely many even symmetric exceptional slopes in the sequence
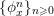 $\{\unicode[STIX]{x1D719}_{x}^{n}\}_{n\geqslant 0}$
. We say that an even symmetric exceptional slope
$\{\unicode[STIX]{x1D719}_{x}^{n}\}_{n\geqslant 0}$
. We say that an even symmetric exceptional slope
![]() $\unicode[STIX]{x1D719}_{x}^{n}$
is of Type A if
$\unicode[STIX]{x1D719}_{x}^{n}$
is of Type A if
![]() $\unicode[STIX]{x1D70E}_{x}^{n<}$
starts as
$\unicode[STIX]{x1D70E}_{x}^{n<}$
starts as
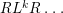 $RL^{k}R\ldots \,$
for some
$RL^{k}R\ldots \,$
for some
![]() $k>0$
; of type B if
$k>0$
; of type B if
![]() $\unicode[STIX]{x1D70E}_{x}^{n<}$
starts as
$\unicode[STIX]{x1D70E}_{x}^{n<}$
starts as
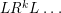 $LR^{k}L\ldots \,$
for some
$LR^{k}L\ldots \,$
for some
![]() $k>0$
; and of Type C if
$k>0$
; and of Type C if
![]() $\unicode[STIX]{x1D70E}_{x}^{n<}$
starts as
$\unicode[STIX]{x1D70E}_{x}^{n<}$
starts as
 $RRLL\ldots \,$
, where
$RRLL\ldots \,$
, where
![]() $\unicode[STIX]{x1D70E}_{x}^{n<}$
is the infinite sequence obtained from
$\unicode[STIX]{x1D70E}_{x}^{n<}$
is the infinite sequence obtained from
![]() $\unicode[STIX]{x1D70E}_{x}$
by deleting the initial
$\unicode[STIX]{x1D70E}_{x}$
by deleting the initial
![]() $n$
terms. We can see that for at least one type of A, B or C, an infinite number of even
$n$
terms. We can see that for at least one type of A, B or C, an infinite number of even
![]() $\unicode[STIX]{x1D719}_{x}^{n}$
of such type appear.
$\unicode[STIX]{x1D719}_{x}^{n}$
of such type appear.
In the next lemma, we use the following notation. For a sequence
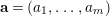 $\mathbf{a}=(a_{1},\ldots ,a_{m})$
of integers, we let
$\mathbf{a}=(a_{1},\ldots ,a_{m})$
of integers, we let
 $$\begin{eqnarray}\displaystyle & \displaystyle \text{}^{+}\mathbf{a}=(3,a_{2}+1,a_{3},\ldots ,a_{m}),\qquad \text{}^{-}\mathbf{a}=(a_{1}-1,a_{2},\ldots ,a_{m}), & \displaystyle \nonumber\\ \displaystyle & \displaystyle \mathbf{a}^{+}=(a_{1},\ldots ,a_{m-2},a_{m-1}+1,3),\qquad \mathbf{a}^{-}=(a_{1},\ldots ,a_{m-1},a_{m}-1). & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & \displaystyle \text{}^{+}\mathbf{a}=(3,a_{2}+1,a_{3},\ldots ,a_{m}),\qquad \text{}^{-}\mathbf{a}=(a_{1}-1,a_{2},\ldots ,a_{m}), & \displaystyle \nonumber\\ \displaystyle & \displaystyle \mathbf{a}^{+}=(a_{1},\ldots ,a_{m-2},a_{m-1}+1,3),\qquad \mathbf{a}^{-}=(a_{1},\ldots ,a_{m-1},a_{m}-1). & \displaystyle \nonumber\end{eqnarray}$$
We define
![]() $^{+}\mathbf{a}^{+}$
,
$^{+}\mathbf{a}^{+}$
,
![]() $^{+}\mathbf{a}^{-}$
, and so forth obviously.
$^{+}\mathbf{a}^{-}$
, and so forth obviously.
Lemma 7.8. Assume that
![]() $\unicode[STIX]{x1D719}_{x}^{n}$
is an even symmetric exceptional slope. Put
$\unicode[STIX]{x1D719}_{x}^{n}$
is an even symmetric exceptional slope. Put
![]() $\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D719}_{x}^{n}$
. Express
$\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D719}_{x}^{n}$
. Express
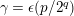 $\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D716}(p/2^{q})$
with
$\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D716}(p/2^{q})$
with
![]() $p$
odd, and let
$p$
odd, and let
 $\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D716}((p-1)/2^{q})$
and
$\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D716}((p-1)/2^{q})$
and
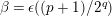 $\unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D716}((p+1)/2^{q})$
. Let
$\unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D716}((p+1)/2^{q})$
. Let
![]() $[0;\mathbf{c}]$
,
$[0;\mathbf{c}]$
,
![]() $[0;\mathbf{a}]$
and
$[0;\mathbf{a}]$
and
![]() $[0;\mathbf{b}]$
be the even length continued fraction expansions of
$[0;\mathbf{b}]$
be the even length continued fraction expansions of
![]() $\unicode[STIX]{x1D6FE}$
,
$\unicode[STIX]{x1D6FE}$
,
![]() $\unicode[STIX]{x1D6FC}$
and
$\unicode[STIX]{x1D6FC}$
and
![]() $\unicode[STIX]{x1D6FD}$
, respectively, and let
$\unicode[STIX]{x1D6FD}$
, respectively, and let
![]() $[0;\mathbf{c}^{\prime }]$
,
$[0;\mathbf{c}^{\prime }]$
,
![]() $[0;\mathbf{a}^{\prime }]$
and
$[0;\mathbf{a}^{\prime }]$
and
![]() $[0;\mathbf{b}^{\prime }]$
be the even length continued fraction expansions of
$[0;\mathbf{b}^{\prime }]$
be the even length continued fraction expansions of
![]() $2\unicode[STIX]{x1D6FE}$
,
$2\unicode[STIX]{x1D6FE}$
,
![]() $2\unicode[STIX]{x1D6FC}$
and
$2\unicode[STIX]{x1D6FC}$
and
![]() $2\unicode[STIX]{x1D6FD}$
, respectively.
$2\unicode[STIX]{x1D6FD}$
, respectively.
![]() $(1)$
If
$(1)$
If
![]() $\unicode[STIX]{x1D6FE}$
is of Type A, and
$\unicode[STIX]{x1D6FE}$
is of Type A, and
![]() $\unicode[STIX]{x1D70E}^{n<}$
starts as
$\unicode[STIX]{x1D70E}^{n<}$
starts as
 $RL^{k}R\ldots \,$
, then the even length continued fraction expansion of
$RL^{k}R\ldots \,$
, then the even length continued fraction expansion of
 $\unicode[STIX]{x1D6FE}\cdot RL^{k}R(=\unicode[STIX]{x1D719}_{x}^{n+k+2})$
is
$\unicode[STIX]{x1D6FE}\cdot RL^{k}R(=\unicode[STIX]{x1D719}_{x}^{n+k+2})$
is
 $$\begin{eqnarray}[0;\mathbf{c},1,1,^{-}\mathbf{b}^{+},(\mathbf{c}^{\prime +})^{k-1},\mathbf{c}^{\prime },^{+}\mathbf{c}^{\prime },^{+}\mathbf{b}^{\prime -},(1,1,\mathbf{c}^{-})^{k-2},1,1,\mathbf{c}],\end{eqnarray}$$
$$\begin{eqnarray}[0;\mathbf{c},1,1,^{-}\mathbf{b}^{+},(\mathbf{c}^{\prime +})^{k-1},\mathbf{c}^{\prime },^{+}\mathbf{c}^{\prime },^{+}\mathbf{b}^{\prime -},(1,1,\mathbf{c}^{-})^{k-2},1,1,\mathbf{c}],\end{eqnarray}$$
where when
![]() $k=1$
, the last part
$k=1$
, the last part
 $^{+}\mathbf{b}^{\prime -},(1,1,\mathbf{c}^{-})^{k-2},1,1,\mathbf{c}$
reads
$^{+}\mathbf{b}^{\prime -},(1,1,\mathbf{c}^{-})^{k-2},1,1,\mathbf{c}$
reads
![]() $^{+}\mathbf{b}^{\prime }$
.
$^{+}\mathbf{b}^{\prime }$
.
![]() $(2)$
If
$(2)$
If
![]() $\unicode[STIX]{x1D6FE}$
is of Type B, and
$\unicode[STIX]{x1D6FE}$
is of Type B, and
![]() $\unicode[STIX]{x1D70E}^{n<}$
starts as
$\unicode[STIX]{x1D70E}^{n<}$
starts as
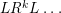 $LR^{k}L\ldots \,$
, then the even length continued fraction expansion of
$LR^{k}L\ldots \,$
, then the even length continued fraction expansion of
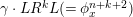 $\unicode[STIX]{x1D6FE}\cdot LR^{k}L(=\unicode[STIX]{x1D719}_{x}^{n+k+2})$
is
$\unicode[STIX]{x1D6FE}\cdot LR^{k}L(=\unicode[STIX]{x1D719}_{x}^{n+k+2})$
is
 $$\begin{eqnarray}[0;\mathbf{c}^{-},1,1,\mathbf{a},(\text{}^{+}\mathbf{c}^{\prime })^{k-1},\text{}^{+}\mathbf{c}^{\prime +},\mathbf{c}^{\prime +},\mathbf{a}^{\prime },(1,1,^{-}\mathbf{c})^{k-1}].\end{eqnarray}$$
$$\begin{eqnarray}[0;\mathbf{c}^{-},1,1,\mathbf{a},(\text{}^{+}\mathbf{c}^{\prime })^{k-1},\text{}^{+}\mathbf{c}^{\prime +},\mathbf{c}^{\prime +},\mathbf{a}^{\prime },(1,1,^{-}\mathbf{c})^{k-1}].\end{eqnarray}$$
![]() $(3)$
If
$(3)$
If
![]() $\unicode[STIX]{x1D6FE}$
is of Type C, then the even length continued fraction expansion of
$\unicode[STIX]{x1D6FE}$
is of Type C, then the even length continued fraction expansion of
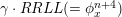 $\unicode[STIX]{x1D6FE}\cdot RRLL(=\unicode[STIX]{x1D719}_{x}^{n+4})$
is
$\unicode[STIX]{x1D6FE}\cdot RRLL(=\unicode[STIX]{x1D719}_{x}^{n+4})$
is
 $$\begin{eqnarray}[0;\mathbf{c},1,1,^{-}\mathbf{b},^{+}\mathbf{c}^{\prime },^{+}\mathbf{b}^{\prime },1,1,^{-}\mathbf{b}^{+},\mathbf{c}^{\prime },^{+}\mathbf{b}^{\prime }].\end{eqnarray}$$
$$\begin{eqnarray}[0;\mathbf{c},1,1,^{-}\mathbf{b},^{+}\mathbf{c}^{\prime },^{+}\mathbf{b}^{\prime },1,1,^{-}\mathbf{b}^{+},\mathbf{c}^{\prime },^{+}\mathbf{b}^{\prime }].\end{eqnarray}$$
Proof. (1) By (7.3) and (7.4), we have
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FE}\cdot R=[0;\mathbf{c},1,1,^{-}\mathbf{b}],\qquad 2(\unicode[STIX]{x1D6FE}\cdot R)=[0;\mathbf{c}^{\prime },^{+}\mathbf{b}^{\prime }]. & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FE}\cdot R=[0;\mathbf{c},1,1,^{-}\mathbf{b}],\qquad 2(\unicode[STIX]{x1D6FE}\cdot R)=[0;\mathbf{c}^{\prime },^{+}\mathbf{b}^{\prime }]. & & \displaystyle \nonumber\end{eqnarray}$$
Using (7.9) and (7.10), we have
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FE}\cdot RL^{k} & = & \displaystyle [0;\mathbf{c},1,1,^{-}\mathbf{b}^{+},(\mathbf{c}^{\prime +})^{k-1},\mathbf{c}^{\prime }],\nonumber\\ \displaystyle 2(\unicode[STIX]{x1D6FE}\cdot RL^{k}) & = & \displaystyle [0;\mathbf{c}^{\prime },^{+}\mathbf{b}^{\prime -},(1,1,\mathbf{c}^{-})^{k-1},1,1,\mathbf{c}].\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FE}\cdot RL^{k} & = & \displaystyle [0;\mathbf{c},1,1,^{-}\mathbf{b}^{+},(\mathbf{c}^{\prime +})^{k-1},\mathbf{c}^{\prime }],\nonumber\\ \displaystyle 2(\unicode[STIX]{x1D6FE}\cdot RL^{k}) & = & \displaystyle [0;\mathbf{c}^{\prime },^{+}\mathbf{b}^{\prime -},(1,1,\mathbf{c}^{-})^{k-1},1,1,\mathbf{c}].\nonumber\end{eqnarray}$$
Using (7.5), we obtain the result.
(2) The proof is similar as in (1).
(3) Using (7.3) and (7.4), we have
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FE}\cdot R=[0;\mathbf{c},1,1,^{-}\mathbf{b}],\qquad 2(\unicode[STIX]{x1D6FE}\cdot R)=[0;\mathbf{c}^{\prime },^{+}\mathbf{b}^{\prime }]. & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FE}\cdot R=[0;\mathbf{c},1,1,^{-}\mathbf{b}],\qquad 2(\unicode[STIX]{x1D6FE}\cdot R)=[0;\mathbf{c}^{\prime },^{+}\mathbf{b}^{\prime }]. & & \displaystyle \nonumber\end{eqnarray}$$
Using (7.5) and (7.6), we have
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FE}\cdot RR=[0;\mathbf{c},1,1,^{-}\mathbf{b},^{+}\mathbf{b}^{\prime }],\qquad 2(\unicode[STIX]{x1D6FE}\cdot RR)=[0;\mathbf{c}^{\prime },^{+}\mathbf{b}^{\prime },1,1,^{-}\mathbf{b}]. & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FE}\cdot RR=[0;\mathbf{c},1,1,^{-}\mathbf{b},^{+}\mathbf{b}^{\prime }],\qquad 2(\unicode[STIX]{x1D6FE}\cdot RR)=[0;\mathbf{c}^{\prime },^{+}\mathbf{b}^{\prime },1,1,^{-}\mathbf{b}]. & & \displaystyle \nonumber\end{eqnarray}$$
Using (7.7) and (7.8), we have
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FE}\cdot RRL & = & \displaystyle [0;\mathbf{c},1,1,^{-}\mathbf{b},^{+}\mathbf{b}^{\prime -},1,1,\mathbf{c},1,1,^{-}\mathbf{b}]\nonumber\\ \displaystyle 2(\unicode[STIX]{x1D6FE}\cdot RRL) & = & \displaystyle [0;\mathbf{c}^{\prime },^{+}\mathbf{b}^{\prime },1,1,^{-}\mathbf{b}^{+},\mathbf{c}^{\prime },^{+}\mathbf{b}^{\prime }].\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FE}\cdot RRL & = & \displaystyle [0;\mathbf{c},1,1,^{-}\mathbf{b},^{+}\mathbf{b}^{\prime -},1,1,\mathbf{c},1,1,^{-}\mathbf{b}]\nonumber\\ \displaystyle 2(\unicode[STIX]{x1D6FE}\cdot RRL) & = & \displaystyle [0;\mathbf{c}^{\prime },^{+}\mathbf{b}^{\prime },1,1,^{-}\mathbf{b}^{+},\mathbf{c}^{\prime },^{+}\mathbf{b}^{\prime }].\nonumber\end{eqnarray}$$
Using (7.5), we have the result.◻
We say that a sequence
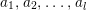 $a_{1},a_{2},\ldots ,a_{l}$
is periodic with period
$a_{1},a_{2},\ldots ,a_{l}$
is periodic with period
![]() $p$
if
$p$
if
 $a_{i}=a_{i+p}$
for
$a_{i}=a_{i+p}$
for
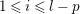 $1\leqslant i\leqslant l-p$
.
$1\leqslant i\leqslant l-p$
.
Lemma 7.9. Let
![]() $A$
,
$A$
,
![]() $B$
and
$B$
and
![]() $M$
be sequences. Assume that the concatenated sequence
$M$
be sequences. Assume that the concatenated sequence
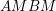 $AMBM$
is periodic, and let
$AMBM$
is periodic, and let
![]() $d$
be the minimum period. Assume moreover that
$d$
be the minimum period. Assume moreover that
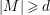 $|M|\geqslant d$
. (Here
$|M|\geqslant d$
. (Here
![]() $|M|$
denotes the length of the sequence
$|M|$
denotes the length of the sequence
![]() $M$
.) Then
$M$
.) Then
![]() $d$
divides
$d$
divides
![]() $|MB|$
. In particular, the last term of
$|MB|$
. In particular, the last term of
![]() $A$
and
$A$
and
![]() $B$
are the same.
$B$
are the same.
Proof. Let
 $M=(z_{1},\ldots ,z_{m})$
. Suppose that
$M=(z_{1},\ldots ,z_{m})$
. Suppose that
![]() $d$
does not divide
$d$
does not divide
![]() $|MB|$
. Then considering the initial term of the latter
$|MB|$
. Then considering the initial term of the latter
![]() $M$
in
$M$
in
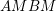 $AMBM$
, we have
$AMBM$
, we have
![]() $z_{1}=z_{l}$
with
$z_{1}=z_{l}$
with
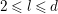 $2\leqslant l\leqslant d$
. Then
$2\leqslant l\leqslant d$
. Then
 $AMBM$
has period
$AMBM$
has period
![]() $l-1$
, which contradicts the minimality of
$l-1$
, which contradicts the minimality of
![]() $d$
.◻
$d$
.◻
Proof of Theorem 7.4.
Take
![]() $x\in C^{\prime }$
that is not an endpoint of an interval
$x\in C^{\prime }$
that is not an endpoint of an interval
![]() $I_{\unicode[STIX]{x1D6FC}}$
, and suppose that the continued fraction expansion
$I_{\unicode[STIX]{x1D6FC}}$
, and suppose that the continued fraction expansion
 $[0;x_{1},x_{2},\ldots \,]$
of
$[0;x_{1},x_{2},\ldots \,]$
of
![]() $x$
is eventually periodic with period
$x$
is eventually periodic with period
![]() $p$
, that is, there exists
$p$
, that is, there exists
![]() $c>0$
such that
$c>0$
such that
 $x_{i}=x_{i+p}$
for all
$x_{i}=x_{i+p}$
for all
![]() $fi>c$
.
$fi>c$
.
Consider the case that infinitely many
![]() $\unicode[STIX]{x1D719}_{x}^{n}$
of Type A appear in
$\unicode[STIX]{x1D719}_{x}^{n}$
of Type A appear in
 $\{\unicode[STIX]{x1D719}_{x}^{n}\}_{n\geqslant 0}$
. Take a
$\{\unicode[STIX]{x1D719}_{x}^{n}\}_{n\geqslant 0}$
. Take a
![]() $\unicode[STIX]{x1D719}_{x}^{n}$
of Type A for
$\unicode[STIX]{x1D719}_{x}^{n}$
of Type A for
![]() $n\gg 0$
. We use the same notation as in Lemma 7.8 (for example
$n\gg 0$
. We use the same notation as in Lemma 7.8 (for example
 $\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D719}_{x}^{n}=[0;\mathbf{c}]$
). Since
$\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D719}_{x}^{n}=[0;\mathbf{c}]$
). Since
![]() $n$
is sufficiently large, we may assume
$n$
is sufficiently large, we may assume
 $m^{\prime }:=|\mathbf{c}^{\prime }|\gg \max \{c,p\}$
. By Lemma 7.8, the even length continued fraction expansion of
$m^{\prime }:=|\mathbf{c}^{\prime }|\gg \max \{c,p\}$
. By Lemma 7.8, the even length continued fraction expansion of
![]() $\unicode[STIX]{x1D719}_{x}^{n+k+2}$
is given by (7.13). If we delete the initial
$\unicode[STIX]{x1D719}_{x}^{n+k+2}$
is given by (7.13). If we delete the initial
![]() $c$
terms and the last two terms from (7.13), then the remaining sequence is periodic with period
$c$
terms and the last two terms from (7.13), then the remaining sequence is periodic with period
![]() $p$
(see Lemma 7.7). In particular, the subsequence
$p$
(see Lemma 7.7). In particular, the subsequence
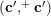 $(\mathbf{c}^{\prime },^{+}\mathbf{c}^{\prime })$
is periodic with period
$(\mathbf{c}^{\prime },^{+}\mathbf{c}^{\prime })$
is periodic with period
![]() $p$
. Put
$p$
. Put
 $A=(c_{1}^{\prime },c_{2}^{\prime })$
,
$A=(c_{1}^{\prime },c_{2}^{\prime })$
,
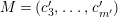 $M=(c_{3}^{\prime },\ldots ,c_{m^{\prime }}^{\prime })$
and
$M=(c_{3}^{\prime },\ldots ,c_{m^{\prime }}^{\prime })$
and
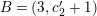 $B=(3,c_{2}^{\prime }+1)$
, where
$B=(3,c_{2}^{\prime }+1)$
, where
 $\mathbf{c}^{\prime }=(c_{1}^{\prime },\ldots ,c_{m^{\prime }}^{\prime })$
. Applying Lemma 7.9, we see
$\mathbf{c}^{\prime }=(c_{1}^{\prime },\ldots ,c_{m^{\prime }}^{\prime })$
. Applying Lemma 7.9, we see
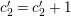 $c_{2}^{\prime }=c_{2}^{\prime }+1$
, which is a contradiction.
$c_{2}^{\prime }=c_{2}^{\prime }+1$
, which is a contradiction.
Other cases can be handled similarly.
When infinitely many
![]() $\unicode[STIX]{x1D719}_{x}^{n}$
of Type B appear, by arguing as in Type A case, the subsequence
$\unicode[STIX]{x1D719}_{x}^{n}$
of Type B appear, by arguing as in Type A case, the subsequence
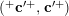 $(\text{}^{+}\mathbf{c}^{\prime +},\mathbf{c}^{\prime +})$
in (7.14) is periodic. We put
$(\text{}^{+}\mathbf{c}^{\prime +},\mathbf{c}^{\prime +})$
in (7.14) is periodic. We put
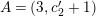 $A=(3,c_{2}^{\prime }+1)$
,
$A=(3,c_{2}^{\prime }+1)$
,
 $M=(c_{3}^{\prime },\ldots ,c_{m^{\prime }-2}^{\prime })$
and
$M=(c_{3}^{\prime },\ldots ,c_{m^{\prime }-2}^{\prime })$
and
 $B=(c_{m^{\prime }-1}+1,3,c_{1}^{\prime },c_{2}^{\prime })$
.
$B=(c_{m^{\prime }-1}+1,3,c_{1}^{\prime },c_{2}^{\prime })$
.
When infinitely many
![]() $\unicode[STIX]{x1D719}_{x}^{n}$
of Type C appear, we see that the subsequence
$\unicode[STIX]{x1D719}_{x}^{n}$
of Type C appear, we see that the subsequence
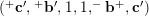 $(\text{}^{+}\mathbf{c}^{\prime },\text{}^{+}\mathbf{b}^{\prime },1,1,^{-}\mathbf{b}^{+},\mathbf{c}^{\prime })$
is periodic. We put
$(\text{}^{+}\mathbf{c}^{\prime },\text{}^{+}\mathbf{b}^{\prime },1,1,^{-}\mathbf{b}^{+},\mathbf{c}^{\prime })$
is periodic. We put
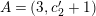 $A=(3,c_{2}^{\prime }+1)$
,
$A=(3,c_{2}^{\prime }+1)$
,
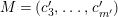 $M=(c_{3}^{\prime },\ldots ,c_{m^{\prime }}^{\prime })$
and
$M=(c_{3}^{\prime },\ldots ,c_{m^{\prime }}^{\prime })$
and
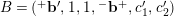 $B=(\text{}^{+}\mathbf{b}^{\prime },1,1,\text{}^{-}\mathbf{b}^{+},c_{1}^{\prime },c_{2}^{\prime })$
.◻
$B=(\text{}^{+}\mathbf{b}^{\prime },1,1,\text{}^{-}\mathbf{b}^{+},c_{1}^{\prime },c_{2}^{\prime })$
.◻
8 Resolution of sheaves
In this section, imitating the argument in [Reference Coskun, Huizenga and WoolfCHW, Section 5], we construct a resolution of a semistable sheaf with symmetric
![]() $c_{1}$
on
$c_{1}$
on
![]() $S$
.
$S$
.
Fix a semistable element
 $\unicode[STIX]{x1D709}\in K(S)$
such that the height of the moduli space
$\unicode[STIX]{x1D709}\in K(S)$
such that the height of the moduli space
![]() $M(\unicode[STIX]{x1D709})$
is positive. In the
$M(\unicode[STIX]{x1D709})$
is positive. In the
![]() $(\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5})$
-plane, the parabola
$(\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5})$
-plane, the parabola
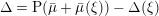 $\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})$
intersects the line
$\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})$
intersects the line
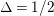 $\unicode[STIX]{x1D6E5}=1/2$
at two points. Let
$\unicode[STIX]{x1D6E5}=1/2$
at two points. Let
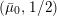 $(\bar{\unicode[STIX]{x1D707}}_{0},1/2)$
be the intersection point with the larger
$(\bar{\unicode[STIX]{x1D707}}_{0},1/2)$
be the intersection point with the larger
![]() $\bar{\unicode[STIX]{x1D707}}$
coordinate.
$\bar{\unicode[STIX]{x1D707}}$
coordinate.
Lemma 8.1. There exists a unique
![]() $\unicode[STIX]{x1D6FE}\in \mathfrak{E}$
such that
$\unicode[STIX]{x1D6FE}\in \mathfrak{E}$
such that
![]() $\bar{\unicode[STIX]{x1D707}}_{0}\in I_{\unicode[STIX]{x1D6FE}}$
.
$\bar{\unicode[STIX]{x1D707}}_{0}\in I_{\unicode[STIX]{x1D6FE}}$
.
Proof. Once we have Theorem 7.4, the same proof of [Reference Coskun, Huizenga and WoolfCHW, Theorem 3.1] applies. ◻
There are
![]() $3$
cases:
$3$
cases:
Case (1)
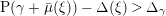 $\text{P}(\unicode[STIX]{x1D6FE}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})>\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}$
.
$\text{P}(\unicode[STIX]{x1D6FE}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})>\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}$
.
Case (2)
 $\text{P}(\unicode[STIX]{x1D6FE}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})=\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}$
.
$\text{P}(\unicode[STIX]{x1D6FE}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})=\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}$
.
Case (3)
 $\text{P}(\unicode[STIX]{x1D6FE}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})<\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}$
.
$\text{P}(\unicode[STIX]{x1D6FE}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})<\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}$
.
We express
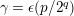 $\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D716}(p/2^{q})$
, where
$\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D716}(p/2^{q})$
, where
![]() $q\geqslant 0$
, and
$q\geqslant 0$
, and
![]() $p$
is odd if
$p$
is odd if
![]() $q\geqslant 1$
. If
$q\geqslant 1$
. If
![]() $q=0$
, then put
$q=0$
, then put
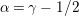 $\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D6FE}-1/2$
and
$\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D6FE}-1/2$
and
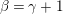 $\unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D6FE}+1$
. If
$\unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D6FE}+1$
. If
![]() $q>0$
, then put
$q>0$
, then put
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D716}\left(\frac{p-1}{2^{q}}\right)\!,\qquad \unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D716}\left(\frac{p+1}{2^{q}}\right)\!. & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D716}\left(\frac{p-1}{2^{q}}\right)\!,\qquad \unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D716}\left(\frac{p+1}{2^{q}}\right)\!. & & \displaystyle \nonumber\end{eqnarray}$$
Then we have
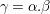 $\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}$
.
$\unicode[STIX]{x1D6FE}=\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FD}$
.
In Case (1) and Case (2), we put
 $$\begin{eqnarray}\displaystyle m_{1}:=\tilde{\unicode[STIX]{x1D712}}(E_{-\unicode[STIX]{x1D6FE}},\unicode[STIX]{x1D709}),\qquad m_{2}:=-\tilde{\unicode[STIX]{x1D712}}(E_{-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FE}},\unicode[STIX]{x1D709}),\qquad m_{3}:=-\tilde{\unicode[STIX]{x1D712}}(E_{-\unicode[STIX]{x1D6FC}},\unicode[STIX]{x1D709}), & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle m_{1}:=\tilde{\unicode[STIX]{x1D712}}(E_{-\unicode[STIX]{x1D6FE}},\unicode[STIX]{x1D709}),\qquad m_{2}:=-\tilde{\unicode[STIX]{x1D712}}(E_{-\unicode[STIX]{x1D6FC}.\unicode[STIX]{x1D6FE}},\unicode[STIX]{x1D709}),\qquad m_{3}:=-\tilde{\unicode[STIX]{x1D712}}(E_{-\unicode[STIX]{x1D6FC}},\unicode[STIX]{x1D709}), & & \displaystyle \nonumber\end{eqnarray}$$
and
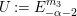 $U:=E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}$
and
$U:=E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}$
and
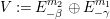 $V:=E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}\oplus E_{-\unicode[STIX]{x1D6FE}}^{m_{1}}$
. Here we have
$V:=E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}\oplus E_{-\unicode[STIX]{x1D6FE}}^{m_{1}}$
. Here we have
![]() $m_{2}>0$
,
$m_{2}>0$
,
![]() $m_{3}>0$
; and
$m_{3}>0$
; and
![]() $m_{1}>0$
in Case (1) and
$m_{1}>0$
in Case (1) and
![]() $m_{1}=0$
in Case (2). (See Notation 4.6 for the notation
$m_{1}=0$
in Case (2). (See Notation 4.6 for the notation
![]() $\tilde{\unicode[STIX]{x1D712}}$
.)
$\tilde{\unicode[STIX]{x1D712}}$
.)
In Case (3), we put
 $$\begin{eqnarray}\displaystyle m_{1}:=-\tilde{\unicode[STIX]{x1D712}}(E_{-\unicode[STIX]{x1D6FE}},\unicode[STIX]{x1D709})>0,\qquad m_{2}:=\tilde{\unicode[STIX]{x1D712}}(E_{-\unicode[STIX]{x1D6FD}},\unicode[STIX]{x1D709})>0,\qquad m_{3}:=\tilde{\unicode[STIX]{x1D712}}(E_{-\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},\unicode[STIX]{x1D709})>0, & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle m_{1}:=-\tilde{\unicode[STIX]{x1D712}}(E_{-\unicode[STIX]{x1D6FE}},\unicode[STIX]{x1D709})>0,\qquad m_{2}:=\tilde{\unicode[STIX]{x1D712}}(E_{-\unicode[STIX]{x1D6FD}},\unicode[STIX]{x1D709})>0,\qquad m_{3}:=\tilde{\unicode[STIX]{x1D712}}(E_{-\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},\unicode[STIX]{x1D709})>0, & & \displaystyle \nonumber\end{eqnarray}$$
and
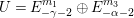 $U=E_{-\unicode[STIX]{x1D6FE}-2}^{m_{1}}\oplus E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}$
and
$U=E_{-\unicode[STIX]{x1D6FE}-2}^{m_{1}}\oplus E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}$
and
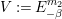 $V:=E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}$
. Using Lemma 8.3 below, we can see that
$V:=E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}$
. Using Lemma 8.3 below, we can see that
 ${\mathcal{H}}om(U,V)$
is globally generated. By a standard dimension estimate, we can see that there is a closed subset
${\mathcal{H}}om(U,V)$
is globally generated. By a standard dimension estimate, we can see that there is a closed subset
![]() $Z$
of codimension at least
$Z$
of codimension at least
![]() $2$
in the affine space
$2$
in the affine space
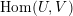 $\text{Hom}(U,V)$
such that for any point
$\text{Hom}(U,V)$
such that for any point
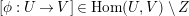 $[\unicode[STIX]{x1D719}:U\rightarrow V]\in \text{Hom}(U,V)\setminus Z$
,
$[\unicode[STIX]{x1D719}:U\rightarrow V]\in \text{Hom}(U,V)\setminus Z$
,
![]() $\unicode[STIX]{x1D719}$
is injective and
$\unicode[STIX]{x1D719}$
is injective and
 $\text{Coker}\,\unicode[STIX]{x1D719}$
is torsion-free. Put
$\text{Coker}\,\unicode[STIX]{x1D719}$
is torsion-free. Put
 $Y:=\text{Hom}(U,V)\setminus Z$
, and
$Y:=\text{Hom}(U,V)\setminus Z$
, and
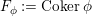 $F_{\unicode[STIX]{x1D719}}:=\text{Coker}\,\unicode[STIX]{x1D719}$
for
$F_{\unicode[STIX]{x1D719}}:=\text{Coker}\,\unicode[STIX]{x1D719}$
for
![]() $\unicode[STIX]{x1D719}\in Y$
.
$\unicode[STIX]{x1D719}\in Y$
.
One can see that the family
![]() $\{F_{\unicode[STIX]{x1D719}}\}$
parametrized by
$\{F_{\unicode[STIX]{x1D719}}\}$
parametrized by
![]() $Y$
is a complete family of sheaves with K-class
$Y$
is a complete family of sheaves with K-class
![]() $\unicode[STIX]{x1D709}$
(cf. the proof of [Reference Coskun, Huizenga and WoolfCHW, Proposition 5.3]). Moreover, using Lemma 8.3(2) below, we can see that
$\unicode[STIX]{x1D709}$
(cf. the proof of [Reference Coskun, Huizenga and WoolfCHW, Proposition 5.3]). Moreover, using Lemma 8.3(2) below, we can see that
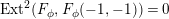 $\text{Ext}^{2}(F_{\unicode[STIX]{x1D719}},F_{\unicode[STIX]{x1D719}}(-1,-1))=0$
for any
$\text{Ext}^{2}(F_{\unicode[STIX]{x1D719}},F_{\unicode[STIX]{x1D719}}(-1,-1))=0$
for any
![]() $\unicode[STIX]{x1D719}\in Y$
. Let
$\unicode[STIX]{x1D719}\in Y$
. Let
![]() $Y^{\prime }$
be the open subset of
$Y^{\prime }$
be the open subset of
![]() $Y$
consisting of
$Y$
consisting of
![]() $\unicode[STIX]{x1D719}$
with
$\unicode[STIX]{x1D719}$
with
![]() $F_{\unicode[STIX]{x1D719}}$
stable. Then by Proposition 6.1 (1), the codimension of
$F_{\unicode[STIX]{x1D719}}$
stable. Then by Proposition 6.1 (1), the codimension of
![]() $Y\setminus Y^{\prime }$
in
$Y\setminus Y^{\prime }$
in
![]() $Y$
is at least
$Y$
is at least
![]() $2$
.
$2$
.
Remark 8.2. When
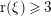 $\text{r}(\unicode[STIX]{x1D709})\geqslant 3$
, if we let
$\text{r}(\unicode[STIX]{x1D709})\geqslant 3$
, if we let
![]() $Y^{\prime \prime }$
be the open subset of
$Y^{\prime \prime }$
be the open subset of
![]() $Y$
consisting of
$Y$
consisting of
![]() $\unicode[STIX]{x1D719}$
such that
$\unicode[STIX]{x1D719}$
such that
![]() $F_{\unicode[STIX]{x1D719}}$
is a
$F_{\unicode[STIX]{x1D719}}$
is a
![]() $\unicode[STIX]{x1D707}$
-stable bundle, then by Proposition 6.1(2), the codimension
$\unicode[STIX]{x1D707}$
-stable bundle, then by Proposition 6.1(2), the codimension
![]() $Y\setminus Y^{\prime \prime }$
in
$Y\setminus Y^{\prime \prime }$
in
![]() $Y$
is at least
$Y$
is at least
![]() $2$
.
$2$
.
Lemma 8.3. Let
 $$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D716}\left(\frac{p}{2^{q}}\right)\!,\qquad \unicode[STIX]{x1D6FD}=\left(\frac{p+1}{2^{q}}\right) & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D716}\left(\frac{p}{2^{q}}\right)\!,\qquad \unicode[STIX]{x1D6FD}=\left(\frac{p+1}{2^{q}}\right) & & \displaystyle \nonumber\end{eqnarray}$$
with
![]() $q\geqslant 0$
.
$q\geqslant 0$
.
-
(1) The sheaf
 ${\mathcal{H}}om(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}})$
is globally generated.
${\mathcal{H}}om(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}})$
is globally generated. -
(2) We have
 $\text{Ext}^{1}(E_{\unicode[STIX]{x1D6FD}-1},E_{\unicode[STIX]{x1D6FC}})=0$
.
$\text{Ext}^{1}(E_{\unicode[STIX]{x1D6FD}-1},E_{\unicode[STIX]{x1D6FC}})=0$
.
Proof. (1) The proof is identical to that of [Reference HuizengaH, Lemma 5.4]. For
![]() $q=0$
,
$q=0$
,
 $E_{\unicode[STIX]{x1D6FC}}={\mathcal{O}}(p,p)$
and
$E_{\unicode[STIX]{x1D6FC}}={\mathcal{O}}(p,p)$
and
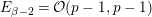 $E_{\unicode[STIX]{x1D6FD}-2}={\mathcal{O}}(p-1,p-1)$
, thus
$E_{\unicode[STIX]{x1D6FD}-2}={\mathcal{O}}(p-1,p-1)$
, thus
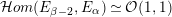 ${\mathcal{H}}om(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}})\simeq {\mathcal{O}}(1,1)$
. The lemma holds.
${\mathcal{H}}om(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}})\simeq {\mathcal{O}}(1,1)$
. The lemma holds.
Assume
![]() $q\geqslant 1$
. When
$q\geqslant 1$
. When
![]() $p$
is odd, put
$p$
is odd, put
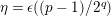 $\unicode[STIX]{x1D702}=\unicode[STIX]{x1D716}((p-1)/2^{q})$
, and
$\unicode[STIX]{x1D702}=\unicode[STIX]{x1D716}((p-1)/2^{q})$
, and
 $$\begin{eqnarray}\unicode[STIX]{x1D6FF}=\left\{\begin{array}{@{}ll@{}}\displaystyle \unicode[STIX]{x1D716}\left(\frac{p+3}{2^{q}}-2\right)\quad & \text{if }p\equiv 1~(\text{mod}~4),\\ \displaystyle \unicode[STIX]{x1D716}\left(\frac{p-3}{2^{q}}\right)\quad & \text{if }p\equiv -1~(\text{mod}~4).\end{array}\right.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6FF}=\left\{\begin{array}{@{}ll@{}}\displaystyle \unicode[STIX]{x1D716}\left(\frac{p+3}{2^{q}}-2\right)\quad & \text{if }p\equiv 1~(\text{mod}~4),\\ \displaystyle \unicode[STIX]{x1D716}\left(\frac{p-3}{2^{q}}\right)\quad & \text{if }p\equiv -1~(\text{mod}~4).\end{array}\right.\end{eqnarray}$$
Then we have an exact sequence (cf. Lemma 4.7)
 $$\begin{eqnarray}0\rightarrow E_{\unicode[STIX]{x1D6FF}}\rightarrow E_{\unicode[STIX]{x1D702}}^{\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D702}},E_{\unicode[STIX]{x1D6FC}})}\rightarrow E_{\unicode[STIX]{x1D6FC}}\rightarrow 0.\end{eqnarray}$$
$$\begin{eqnarray}0\rightarrow E_{\unicode[STIX]{x1D6FF}}\rightarrow E_{\unicode[STIX]{x1D702}}^{\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D702}},E_{\unicode[STIX]{x1D6FC}})}\rightarrow E_{\unicode[STIX]{x1D6FC}}\rightarrow 0.\end{eqnarray}$$
So we have a surjective map
 ${\mathcal{H}}om(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D702}})^{\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D702}},E_{\unicode[STIX]{x1D6FC}})}\rightarrow {\mathcal{H}}om(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}})$
and
${\mathcal{H}}om(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D702}})^{\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D702}},E_{\unicode[STIX]{x1D6FC}})}\rightarrow {\mathcal{H}}om(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}})$
and
 ${\mathcal{H}}om(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D702}})$
is globally generated by the induction hypothesis, so
${\mathcal{H}}om(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D702}})$
is globally generated by the induction hypothesis, so
 ${\mathcal{H}}om(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}})$
is globally generated. When
${\mathcal{H}}om(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}})$
is globally generated. When
![]() $p$
is even, considering the isomorphism
$p$
is even, considering the isomorphism
 ${\mathcal{H}}om(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}})\simeq {\mathcal{H}}om(E_{-\unicode[STIX]{x1D6FC}-2},E_{-\unicode[STIX]{x1D6FD}})$
, the proof is reduced to the case
${\mathcal{H}}om(E_{\unicode[STIX]{x1D6FD}-2},E_{\unicode[STIX]{x1D6FC}})\simeq {\mathcal{H}}om(E_{-\unicode[STIX]{x1D6FC}-2},E_{-\unicode[STIX]{x1D6FD}})$
, the proof is reduced to the case
![]() $p$
odd.
$p$
odd.
(2) For
![]() $q=0,1$
, the vanishing of
$q=0,1$
, the vanishing of
![]() $\text{Ext}^{1}$
can be checked directly. Assume
$\text{Ext}^{1}$
can be checked directly. Assume
![]() $q\geqslant 2$
. As in (1), we may consider only the case where
$q\geqslant 2$
. As in (1), we may consider only the case where
![]() $p$
is odd. We have a short exact sequence (8.1). From this, we obtain an exact sequence
$p$
is odd. We have a short exact sequence (8.1). From this, we obtain an exact sequence
 $$\begin{eqnarray}\text{Ext}^{1}(E_{\unicode[STIX]{x1D6FD}-1},E_{\unicode[STIX]{x1D702}})^{\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D702}},E_{\unicode[STIX]{x1D6FC}})}\rightarrow \text{Ext}^{1}(E_{\unicode[STIX]{x1D6FD}-1},E_{\unicode[STIX]{x1D6FC}})\rightarrow \text{Ext}^{2}(E_{\unicode[STIX]{x1D6FD}-1},E_{\unicode[STIX]{x1D6FF}}).\end{eqnarray}$$
$$\begin{eqnarray}\text{Ext}^{1}(E_{\unicode[STIX]{x1D6FD}-1},E_{\unicode[STIX]{x1D702}})^{\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D702}},E_{\unicode[STIX]{x1D6FC}})}\rightarrow \text{Ext}^{1}(E_{\unicode[STIX]{x1D6FD}-1},E_{\unicode[STIX]{x1D6FC}})\rightarrow \text{Ext}^{2}(E_{\unicode[STIX]{x1D6FD}-1},E_{\unicode[STIX]{x1D6FF}}).\end{eqnarray}$$
By the induction hypothesis, we have
 $\text{Ext}^{1}(E_{\unicode[STIX]{x1D6FD}-1},E_{\unicode[STIX]{x1D702}})=0$
. We have
$\text{Ext}^{1}(E_{\unicode[STIX]{x1D6FD}-1},E_{\unicode[STIX]{x1D702}})=0$
. We have
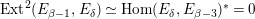 $\text{Ext}^{2}(E_{\unicode[STIX]{x1D6FD}-1},E_{\unicode[STIX]{x1D6FF}})\simeq \text{Hom}(E_{\unicode[STIX]{x1D6FF}},E_{\unicode[STIX]{x1D6FD}-3})^{\ast }=0$
since
$\text{Ext}^{2}(E_{\unicode[STIX]{x1D6FD}-1},E_{\unicode[STIX]{x1D6FF}})\simeq \text{Hom}(E_{\unicode[STIX]{x1D6FF}},E_{\unicode[STIX]{x1D6FD}-3})^{\ast }=0$
since
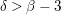 $\unicode[STIX]{x1D6FF}>\unicode[STIX]{x1D6FD}-3$
. The result follows from the above exact sequence.◻
$\unicode[STIX]{x1D6FF}>\unicode[STIX]{x1D6FD}-3$
. The result follows from the above exact sequence.◻
9 Rational map to moduli of quiver representations
Retain the notation
![]() $\unicode[STIX]{x1D709}$
,
$\unicode[STIX]{x1D709}$
,
![]() $\unicode[STIX]{x1D6FC}$
,
$\unicode[STIX]{x1D6FC}$
,
![]() $\unicode[STIX]{x1D6FD}$
and
$\unicode[STIX]{x1D6FD}$
and
![]() $\unicode[STIX]{x1D6FE}$
in the previous section. In this section, we define three kinds of quivers, and construct a rational map of
$\unicode[STIX]{x1D6FE}$
in the previous section. In this section, we define three kinds of quivers, and construct a rational map of
![]() $M(\unicode[STIX]{x1D709})$
to the moduli space of representations of a quiver.
$M(\unicode[STIX]{x1D709})$
to the moduli space of representations of a quiver.
9.1 Quivers
To describe a quiver
![]() $Q$
, we use the notation
$Q$
, we use the notation
 $$\begin{eqnarray}Q=(Q_{0},\{N_{v,v^{\prime }}\}_{(v,v^{\prime })\in Q_{0}\times Q_{0}}),\end{eqnarray}$$
$$\begin{eqnarray}Q=(Q_{0},\{N_{v,v^{\prime }}\}_{(v,v^{\prime })\in Q_{0}\times Q_{0}}),\end{eqnarray}$$
where
![]() $Q_{0}$
is the set of vertexes of the quiver, and
$Q_{0}$
is the set of vertexes of the quiver, and
![]() $N_{v,v^{\prime }}$
is the number of arrows having
$N_{v,v^{\prime }}$
is the number of arrows having
![]() $v$
as the source and
$v$
as the source and
![]() $v^{\prime }$
as the target.
$v^{\prime }$
as the target.
We define quivers
![]() $R^{\unicode[STIX]{x1D6FC}}$
,
$R^{\unicode[STIX]{x1D6FC}}$
,
![]() $R^{\unicode[STIX]{x1D6FD}}$
and
$R^{\unicode[STIX]{x1D6FD}}$
and
![]() $R^{\unicode[STIX]{x1D6FE}}$
as follows.
$R^{\unicode[STIX]{x1D6FE}}$
as follows.
When
![]() $\unicode[STIX]{x1D6FC}$
is even, the quiver
$\unicode[STIX]{x1D6FC}$
is even, the quiver
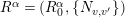 $R^{\unicode[STIX]{x1D6FC}}=(R_{0}^{\unicode[STIX]{x1D6FC}},\{N_{v,v^{\prime }}\})$
is defined by
$R^{\unicode[STIX]{x1D6FC}}=(R_{0}^{\unicode[STIX]{x1D6FC}},\{N_{v,v^{\prime }}\})$
is defined by
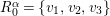 $R_{0}^{\unicode[STIX]{x1D6FC}}=\{v_{1},v_{2},v_{3}\}$
and
$R_{0}^{\unicode[STIX]{x1D6FC}}=\{v_{1},v_{2},v_{3}\}$
and
 $N_{v_{2},v_{1}}=N_{v_{3},v_{1}}=\tilde{\unicode[STIX]{x1D712}}(E_{-\unicode[STIX]{x1D6FC}-2},E_{-\unicode[STIX]{x1D6FD}})$
and
$N_{v_{2},v_{1}}=N_{v_{3},v_{1}}=\tilde{\unicode[STIX]{x1D712}}(E_{-\unicode[STIX]{x1D6FC}-2},E_{-\unicode[STIX]{x1D6FD}})$
and
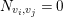 $N_{v_{i},v_{j}}=0$
for other pairs
$N_{v_{i},v_{j}}=0$
for other pairs
![]() $(v_{i},v_{j})$
.
$(v_{i},v_{j})$
.
When
![]() $\unicode[STIX]{x1D6FD}$
is even, the quiver
$\unicode[STIX]{x1D6FD}$
is even, the quiver
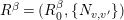 $R^{\unicode[STIX]{x1D6FD}}=(R_{0}^{\unicode[STIX]{x1D6FD}},\{N_{v,v^{\prime }}\})$
is defined by
$R^{\unicode[STIX]{x1D6FD}}=(R_{0}^{\unicode[STIX]{x1D6FD}},\{N_{v,v^{\prime }}\})$
is defined by
 $R_{0}^{\unicode[STIX]{x1D6FD}}=\{v_{1},v_{2},v_{3}\}$
and
$R_{0}^{\unicode[STIX]{x1D6FD}}=\{v_{1},v_{2},v_{3}\}$
and
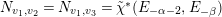 $N_{v_{1},v_{2}}=N_{v_{1},v_{3}}=\tilde{\unicode[STIX]{x1D712}}^{\ast }(E_{-\unicode[STIX]{x1D6FC}-2},E_{-\unicode[STIX]{x1D6FD}})$
and
$N_{v_{1},v_{2}}=N_{v_{1},v_{3}}=\tilde{\unicode[STIX]{x1D712}}^{\ast }(E_{-\unicode[STIX]{x1D6FC}-2},E_{-\unicode[STIX]{x1D6FD}})$
and
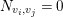 $N_{v_{i},v_{j}}=0$
for other pairs
$N_{v_{i},v_{j}}=0$
for other pairs
![]() $(v_{i},v_{j})$
.
$(v_{i},v_{j})$
.
When
![]() $\unicode[STIX]{x1D6FE}$
is even, the quiver
$\unicode[STIX]{x1D6FE}$
is even, the quiver
 $R^{\unicode[STIX]{x1D6FE}}=(R_{0}^{\unicode[STIX]{x1D6FE}},\{N_{v,v^{\prime }}\})$
is defined by
$R^{\unicode[STIX]{x1D6FE}}=(R_{0}^{\unicode[STIX]{x1D6FE}},\{N_{v,v^{\prime }}\})$
is defined by
 $R_{0}^{\unicode[STIX]{x1D6FE}}=\{v_{1},v_{2}\}$
and
$R_{0}^{\unicode[STIX]{x1D6FE}}=\{v_{1},v_{2}\}$
and
 $N_{v_{2},v_{1}}=\unicode[STIX]{x1D712}(E_{-\unicode[STIX]{x1D6FC}-2},E_{-\unicode[STIX]{x1D6FD}})$
and
$N_{v_{2},v_{1}}=\unicode[STIX]{x1D712}(E_{-\unicode[STIX]{x1D6FC}-2},E_{-\unicode[STIX]{x1D6FD}})$
and
 $N_{v_{i},v_{j}}=0$
for other pairs
$N_{v_{i},v_{j}}=0$
for other pairs
![]() $(v_{i},v_{j})$
.
$(v_{i},v_{j})$
.
In summary, the quivers
![]() $R^{\unicode[STIX]{x1D6FC}}$
,
$R^{\unicode[STIX]{x1D6FC}}$
,
![]() $R^{\unicode[STIX]{x1D6FD}}$
and
$R^{\unicode[STIX]{x1D6FD}}$
and
![]() $R^{\unicode[STIX]{x1D6FE}}$
are the quivers obtained from the quivers
$R^{\unicode[STIX]{x1D6FE}}$
are the quivers obtained from the quivers
![]() $Q^{\unicode[STIX]{x1D6FC}}$
,
$Q^{\unicode[STIX]{x1D6FC}}$
,
![]() $Q^{\unicode[STIX]{x1D6FD}}$
and
$Q^{\unicode[STIX]{x1D6FD}}$
and
![]() $Q^{\unicode[STIX]{x1D6FE}}$
in Section 5.2 by reversing the arrows. (See Figures 10–12.
$Q^{\unicode[STIX]{x1D6FE}}$
in Section 5.2 by reversing the arrows. (See Figures 10–12.

Figure 10.
![]() $R^{\unicode[STIX]{x1D6FC}}$
.
$R^{\unicode[STIX]{x1D6FC}}$
.
Figure 11.
![]() $R^{\unicode[STIX]{x1D6FD}}$
.
$R^{\unicode[STIX]{x1D6FD}}$
.

Figure 12.
![]() $R^{\unicode[STIX]{x1D6FE}}$
.
$R^{\unicode[STIX]{x1D6FE}}$
.
We define moduli spaces
![]() $M^{\unicode[STIX]{x1D6FC}}$
,
$M^{\unicode[STIX]{x1D6FC}}$
,
![]() $M^{\unicode[STIX]{x1D6FD}}$
and
$M^{\unicode[STIX]{x1D6FD}}$
and
![]() $M^{\unicode[STIX]{x1D6FE}}$
of representations of quivers as follows.
$M^{\unicode[STIX]{x1D6FE}}$
of representations of quivers as follows.
![]() $M^{\unicode[STIX]{x1D6FC}}$
(resp.
$M^{\unicode[STIX]{x1D6FC}}$
(resp.
![]() $M^{\unicode[STIX]{x1D6FD}}$
) is the coarse moduli space of
$M^{\unicode[STIX]{x1D6FD}}$
) is the coarse moduli space of
![]() $\unicode[STIX]{x1D703}$
-semistable representations of the quiver
$\unicode[STIX]{x1D703}$
-semistable representations of the quiver
![]() $R^{\unicode[STIX]{x1D6FC}}$
(resp.
$R^{\unicode[STIX]{x1D6FC}}$
(resp.
![]() $R^{\unicode[STIX]{x1D6FD}}$
) with dimension vector
$R^{\unicode[STIX]{x1D6FD}}$
) with dimension vector
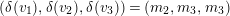 $(\unicode[STIX]{x1D6FF}(v_{1}),\unicode[STIX]{x1D6FF}(v_{2}),\unicode[STIX]{x1D6FF}(v_{3}))=(m_{2},m_{3},m_{3})$
(resp.
$(\unicode[STIX]{x1D6FF}(v_{1}),\unicode[STIX]{x1D6FF}(v_{2}),\unicode[STIX]{x1D6FF}(v_{3}))=(m_{2},m_{3},m_{3})$
(resp.
![]() $=$
$=$
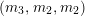 $(m_{3},m_{2},m_{2})$
), where the weight vector
$(m_{3},m_{2},m_{2})$
), where the weight vector
 $$\begin{eqnarray}(\unicode[STIX]{x1D703}(v_{1}),\unicode[STIX]{x1D703}(v_{2}),\unicode[STIX]{x1D703}(v_{3}))=(2m_{3},-m_{2},-m_{2})\end{eqnarray}$$
$$\begin{eqnarray}(\unicode[STIX]{x1D703}(v_{1}),\unicode[STIX]{x1D703}(v_{2}),\unicode[STIX]{x1D703}(v_{3}))=(2m_{3},-m_{2},-m_{2})\end{eqnarray}$$
(resp.
 $=(-2m_{2},m_{3},m_{3})$
).
$=(-2m_{2},m_{3},m_{3})$
).
![]() $M^{\unicode[STIX]{x1D6FE}}$
is the coarse moduli space of
$M^{\unicode[STIX]{x1D6FE}}$
is the coarse moduli space of
![]() $\unicode[STIX]{x1D703}$
-semistable representations of the quiver
$\unicode[STIX]{x1D703}$
-semistable representations of the quiver
![]() $R^{\unicode[STIX]{x1D6FE}}$
with dimension vector
$R^{\unicode[STIX]{x1D6FE}}$
with dimension vector
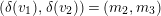 $(\unicode[STIX]{x1D6FF}(v_{1}),\unicode[STIX]{x1D6FF}(v_{2}))=(m_{2},m_{3})$
, where the weight vector
$(\unicode[STIX]{x1D6FF}(v_{1}),\unicode[STIX]{x1D6FF}(v_{2}))=(m_{2},m_{3})$
, where the weight vector
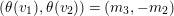 $(\unicode[STIX]{x1D703}(v_{1}),\unicode[STIX]{x1D703}(v_{2}))=(m_{3},-m_{2})$
. The nonemptyness of these moduli spaces will be verified later.
$(\unicode[STIX]{x1D703}(v_{1}),\unicode[STIX]{x1D703}(v_{2}))=(m_{3},-m_{2})$
. The nonemptyness of these moduli spaces will be verified later.
9.2 Rational map
We define a rational map from
![]() $M(\unicode[STIX]{x1D709})$
to
$M(\unicode[STIX]{x1D709})$
to
![]() $M^{\unicode[STIX]{x1D6FC}}$
,
$M^{\unicode[STIX]{x1D6FC}}$
,
![]() $M^{\unicode[STIX]{x1D6FD}}$
or
$M^{\unicode[STIX]{x1D6FD}}$
or
![]() $M^{\unicode[STIX]{x1D6FE}}$
depending on which one of
$M^{\unicode[STIX]{x1D6FE}}$
depending on which one of
![]() $\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FE}$
is even.
$\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FE}$
is even.
Case (1) or (2) A general sheaf
 $F\in M(\unicode[STIX]{x1D709})$
has a resolution of the form
$F\in M(\unicode[STIX]{x1D709})$
has a resolution of the form
 $$\begin{eqnarray}E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}\xrightarrow[{}]{(f,g)}E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}\oplus E_{-\unicode[STIX]{x1D6FE}}^{m_{1}}.\end{eqnarray}$$
$$\begin{eqnarray}E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}\xrightarrow[{}]{(f,g)}E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}\oplus E_{-\unicode[STIX]{x1D6FE}}^{m_{1}}.\end{eqnarray}$$
Let
![]() $M(\unicode[STIX]{x1D709})^{\circ }$
be the open subscheme of
$M(\unicode[STIX]{x1D709})^{\circ }$
be the open subscheme of
![]() $M(\unicode[STIX]{x1D709})$
consisting of stable sheaves having the resolution of this form.
$M(\unicode[STIX]{x1D709})$
consisting of stable sheaves having the resolution of this form.
Subcase (1 or 2,
![]() $\unicode[STIX]{x1D6FC}$
). If
$\unicode[STIX]{x1D6FC}$
). If
![]() $\unicode[STIX]{x1D6FC}$
is even, then
$\unicode[STIX]{x1D6FC}$
is even, then
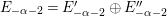 $E_{-\unicode[STIX]{x1D6FC}-2}=E_{-\unicode[STIX]{x1D6FC}-2}^{\prime }\oplus E_{-\unicode[STIX]{x1D6FC}-2}^{\prime \prime }$
. Giving the map
$E_{-\unicode[STIX]{x1D6FC}-2}=E_{-\unicode[STIX]{x1D6FC}-2}^{\prime }\oplus E_{-\unicode[STIX]{x1D6FC}-2}^{\prime \prime }$
. Giving the map
 $$\begin{eqnarray}f=f^{\prime }+f^{\prime \prime }:E_{-\unicode[STIX]{x1D6FC}-2}^{\prime \;m_{3}}\oplus E_{-\unicode[STIX]{x1D6FC}-2}^{\prime \prime \;m_{3}}\rightarrow E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}\end{eqnarray}$$
$$\begin{eqnarray}f=f^{\prime }+f^{\prime \prime }:E_{-\unicode[STIX]{x1D6FC}-2}^{\prime \;m_{3}}\oplus E_{-\unicode[STIX]{x1D6FC}-2}^{\prime \prime \;m_{3}}\rightarrow E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}\end{eqnarray}$$
is equivalent to giving linear maps
 $$\begin{eqnarray}\mathbb{C}^{m_{3}}\rightarrow \text{Hom}(E_{-\unicode[STIX]{x1D6FC}-2}^{\prime },E_{-\unicode[STIX]{x1D6FD}})^{m_{2}}\qquad \text{and}\qquad \mathbb{C}^{m_{3}}\rightarrow \text{Hom}(E_{-\unicode[STIX]{x1D6FC}-2}^{\prime \prime },E_{-\unicode[STIX]{x1D6FD}})^{m_{2}},\end{eqnarray}$$
$$\begin{eqnarray}\mathbb{C}^{m_{3}}\rightarrow \text{Hom}(E_{-\unicode[STIX]{x1D6FC}-2}^{\prime },E_{-\unicode[STIX]{x1D6FD}})^{m_{2}}\qquad \text{and}\qquad \mathbb{C}^{m_{3}}\rightarrow \text{Hom}(E_{-\unicode[STIX]{x1D6FC}-2}^{\prime \prime },E_{-\unicode[STIX]{x1D6FD}})^{m_{2}},\end{eqnarray}$$
which are obtained from
![]() $f^{\prime }$
and
$f^{\prime }$
and
![]() $f^{\prime \prime }$
by applying
$f^{\prime \prime }$
by applying
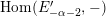 $\text{Hom}(E_{-\unicode[STIX]{x1D6FC}-2}^{\prime },-)$
and
$\text{Hom}(E_{-\unicode[STIX]{x1D6FC}-2}^{\prime },-)$
and
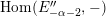 $\text{Hom}(E_{-\unicode[STIX]{x1D6FC}-2}^{\prime \prime },-)$
, respectively. The linear maps (9.1) determine a representation of the quiver
$\text{Hom}(E_{-\unicode[STIX]{x1D6FC}-2}^{\prime \prime },-)$
, respectively. The linear maps (9.1) determine a representation of the quiver
![]() $R^{\unicode[STIX]{x1D6FC}}$
, denoted by
$R^{\unicode[STIX]{x1D6FC}}$
, denoted by
![]() $\unicode[STIX]{x1D70B}(F)$
, with dimension vector
$\unicode[STIX]{x1D70B}(F)$
, with dimension vector
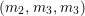 $(m_{2},m_{3},m_{3})$
.
$(m_{2},m_{3},m_{3})$
.
Subcase (1 or 2,
![]() $\unicode[STIX]{x1D6FD}$
). If
$\unicode[STIX]{x1D6FD}$
). If
![]() $\unicode[STIX]{x1D6FD}$
is even, then
$\unicode[STIX]{x1D6FD}$
is even, then
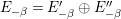 $E_{-\unicode[STIX]{x1D6FD}}=E_{-\unicode[STIX]{x1D6FD}}^{\prime }\oplus E_{-\unicode[STIX]{x1D6FD}}^{\prime \prime }$
. As in the previous case, the map
$E_{-\unicode[STIX]{x1D6FD}}=E_{-\unicode[STIX]{x1D6FD}}^{\prime }\oplus E_{-\unicode[STIX]{x1D6FD}}^{\prime \prime }$
. As in the previous case, the map
![]() $f$
determines a representation of the quiver
$f$
determines a representation of the quiver
![]() $R^{\unicode[STIX]{x1D6FD}}$
, denoted by
$R^{\unicode[STIX]{x1D6FD}}$
, denoted by
![]() $\unicode[STIX]{x1D70B}(F)$
, with dimension vector
$\unicode[STIX]{x1D70B}(F)$
, with dimension vector
 $(m_{3},m_{2},m_{2})$
.
$(m_{3},m_{2},m_{2})$
.
Subcase (1 or 2,
![]() $\unicode[STIX]{x1D6FE}$
). If
$\unicode[STIX]{x1D6FE}$
). If
![]() $\unicode[STIX]{x1D6FE}$
is even, then both
$\unicode[STIX]{x1D6FE}$
is even, then both
![]() $E_{-\unicode[STIX]{x1D6FC}-2}$
and
$E_{-\unicode[STIX]{x1D6FC}-2}$
and
![]() $E_{-\unicode[STIX]{x1D6FD}}$
are simple bundles. Giving map
$E_{-\unicode[STIX]{x1D6FD}}$
are simple bundles. Giving map
![]() $f$
is equivalent to giving a linear map
$f$
is equivalent to giving a linear map
 $$\begin{eqnarray}\mathbb{C}^{m_{3}}\rightarrow \text{Hom}(E_{-\unicode[STIX]{x1D6FC}-2},E_{-\unicode[STIX]{x1D6FD}})^{m_{2}},\end{eqnarray}$$
$$\begin{eqnarray}\mathbb{C}^{m_{3}}\rightarrow \text{Hom}(E_{-\unicode[STIX]{x1D6FC}-2},E_{-\unicode[STIX]{x1D6FD}})^{m_{2}},\end{eqnarray}$$
which is obtained from
![]() $f$
by applying
$f$
by applying
 $\text{Hom}(E_{-\unicode[STIX]{x1D6FC}-2},-)$
. The map (9.2) determines a representation of the quiver
$\text{Hom}(E_{-\unicode[STIX]{x1D6FC}-2},-)$
. The map (9.2) determines a representation of the quiver
![]() $R^{\unicode[STIX]{x1D6FE}}$
, denoted by
$R^{\unicode[STIX]{x1D6FE}}$
, denoted by
![]() $\unicode[STIX]{x1D70B}(F)$
, with dimension vector
$\unicode[STIX]{x1D70B}(F)$
, with dimension vector
 $(m_{2},m_{3})$
.
$(m_{2},m_{3})$
.
Case (3). A general sheaf
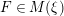 $F\in M(\unicode[STIX]{x1D709})$
has a resolution of the form
$F\in M(\unicode[STIX]{x1D709})$
has a resolution of the form
 $$\begin{eqnarray}E_{-\unicode[STIX]{x1D6FE}-2}^{m_{1}}\oplus E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}\xrightarrow[{}]{g+f}E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}.\end{eqnarray}$$
$$\begin{eqnarray}E_{-\unicode[STIX]{x1D6FE}-2}^{m_{1}}\oplus E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}\xrightarrow[{}]{g+f}E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}.\end{eqnarray}$$
Let
![]() $M(\unicode[STIX]{x1D709})^{\circ }$
be the open subscheme of
$M(\unicode[STIX]{x1D709})^{\circ }$
be the open subscheme of
![]() $M(\unicode[STIX]{x1D709})$
consisting of stable sheaves having the resolution of this form. We consider the map
$M(\unicode[STIX]{x1D709})$
consisting of stable sheaves having the resolution of this form. We consider the map
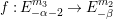 $f:E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}\rightarrow E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}$
. As in the preceding cases, we see that
$f:E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}\rightarrow E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}$
. As in the preceding cases, we see that
![]() $f$
determines a representation of the quiver
$f$
determines a representation of the quiver
![]() $R^{\unicode[STIX]{x1D6FC}}$
,
$R^{\unicode[STIX]{x1D6FC}}$
,
![]() $R^{\unicode[STIX]{x1D6FD}}$
or
$R^{\unicode[STIX]{x1D6FD}}$
or
![]() $R^{\unicode[STIX]{x1D6FE}}$
, denoted by
$R^{\unicode[STIX]{x1D6FE}}$
, denoted by
![]() $\unicode[STIX]{x1D70B}(F)$
, depending on whether
$\unicode[STIX]{x1D70B}(F)$
, depending on whether
![]() $\unicode[STIX]{x1D6FC}$
,
$\unicode[STIX]{x1D6FC}$
,
![]() $\unicode[STIX]{x1D6FD}$
or
$\unicode[STIX]{x1D6FD}$
or
![]() $\unicode[STIX]{x1D6FE}$
is even.
$\unicode[STIX]{x1D6FE}$
is even.
For
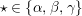 $\star \in \{\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FE}\}$
, let
$\star \in \{\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FE}\}$
, let
![]() $M^{\star s}$
be the open subscheme of
$M^{\star s}$
be the open subscheme of
![]() $M^{\star }$
consisting of
$M^{\star }$
consisting of
![]() $\unicode[STIX]{x1D703}$
-stable representations, where the weight
$\unicode[STIX]{x1D703}$
-stable representations, where the weight
![]() $\unicode[STIX]{x1D703}$
is the one defined before. Let
$\unicode[STIX]{x1D703}$
is the one defined before. Let
![]() $M(\unicode[STIX]{x1D709})^{\circ \unicode[STIX]{x1D70E}}$
be the open subscheme of
$M(\unicode[STIX]{x1D709})^{\circ \unicode[STIX]{x1D70E}}$
be the open subscheme of
![]() $M(\unicode[STIX]{x1D709})^{\circ }$
consisting of such
$M(\unicode[STIX]{x1D709})^{\circ }$
consisting of such
![]() $F$
that
$F$
that
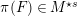 $\unicode[STIX]{x1D70B}(F)\in M^{\star s}$
. The rational map
$\unicode[STIX]{x1D70B}(F)\in M^{\star s}$
. The rational map
![]() $\unicode[STIX]{x1D70B}$
from
$\unicode[STIX]{x1D70B}$
from
![]() $M(\unicode[STIX]{x1D709})$
to
$M(\unicode[STIX]{x1D709})$
to
![]() $M^{\unicode[STIX]{x1D6FC}}$
,
$M^{\unicode[STIX]{x1D6FC}}$
,
![]() $M^{\unicode[STIX]{x1D6FD}}$
or
$M^{\unicode[STIX]{x1D6FD}}$
or
![]() $M^{\unicode[STIX]{x1D6FE}}$
is defined to map
$M^{\unicode[STIX]{x1D6FE}}$
is defined to map
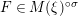 $F\in M(\unicode[STIX]{x1D709})^{\circ \unicode[STIX]{x1D70E}}$
to
$F\in M(\unicode[STIX]{x1D709})^{\circ \unicode[STIX]{x1D70E}}$
to
![]() $\unicode[STIX]{x1D70B}(F)$
. The following proposition guarantees that the domain of definition of the rational map
$\unicode[STIX]{x1D70B}(F)$
. The following proposition guarantees that the domain of definition of the rational map
![]() $\unicode[STIX]{x1D70B}$
is nonempty.
$\unicode[STIX]{x1D70B}$
is nonempty.
Proposition 9.1.
![]() $M(\unicode[STIX]{x1D709})^{\circ \unicode[STIX]{x1D70E}}$
is nonempty. Moreover, in Case (2), we have
$M(\unicode[STIX]{x1D709})^{\circ \unicode[STIX]{x1D70E}}$
is nonempty. Moreover, in Case (2), we have
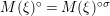 $M(\unicode[STIX]{x1D709})^{\circ }=M(\unicode[STIX]{x1D709})^{\circ \unicode[STIX]{x1D70E}}$
.
$M(\unicode[STIX]{x1D709})^{\circ }=M(\unicode[STIX]{x1D709})^{\circ \unicode[STIX]{x1D70E}}$
.
Proof. In Case (2),
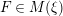 $F\in M(\unicode[STIX]{x1D709})$
fits in a short exact sequence
$F\in M(\unicode[STIX]{x1D709})$
fits in a short exact sequence
 $$\begin{eqnarray}0\rightarrow E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}\xrightarrow[{}]{f}E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}\rightarrow F\rightarrow 0.\end{eqnarray}$$
$$\begin{eqnarray}0\rightarrow E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}\xrightarrow[{}]{f}E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}\rightarrow F\rightarrow 0.\end{eqnarray}$$
So the result follows from Proposition 5.7.
Next we consider Case (3). We have only to show that
![]() $M^{\star s}$
is nonempty (
$M^{\star s}$
is nonempty (
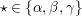 $\star \in \{\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FE}\}$
). Consider
$\star \in \{\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D6FE}\}$
). Consider
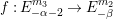 $f:E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}\rightarrow E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}$
. For general
$f:E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}\rightarrow E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}$
. For general
![]() $f$
,
$f$
,
![]() $f$
is injective and the rank of
$f$
is injective and the rank of
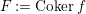 $F:=\text{Coker}\,f$
is positive.
$F:=\text{Coker}\,f$
is positive.
Claim 9.1.1. We have
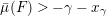 $\bar{\unicode[STIX]{x1D707}}(F)>-\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}}$
.
$\bar{\unicode[STIX]{x1D707}}(F)>-\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}}$
.
Proof of Claim.
Let
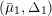 $(\bar{\unicode[STIX]{x1D707}}_{1},\unicode[STIX]{x1D6E5}_{1})$
be the intersection of the parabolas
$(\bar{\unicode[STIX]{x1D707}}_{1},\unicode[STIX]{x1D6E5}_{1})$
be the intersection of the parabolas
 $$\begin{eqnarray}\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})\qquad \text{and}\qquad \unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FE}-2)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})\qquad \text{and}\qquad \unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FE}-2)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}\end{eqnarray}$$
in the
![]() $(\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5})$
-plane. The point
$(\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5})$
-plane. The point
 $(\bar{\unicode[STIX]{x1D707}}(F),\unicode[STIX]{x1D6E5}(F))$
lies on the parabolas
$(\bar{\unicode[STIX]{x1D707}}(F),\unicode[STIX]{x1D6E5}(F))$
lies on the parabolas
 $$\begin{eqnarray}\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}\qquad \text{and}\qquad \unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\bar{\unicode[STIX]{x1D707}}_{1})-\unicode[STIX]{x1D6E5}_{1}.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}\qquad \text{and}\qquad \unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\bar{\unicode[STIX]{x1D707}}_{1})-\unicode[STIX]{x1D6E5}_{1}.\end{eqnarray}$$
By calculation, we have
 $$\begin{eqnarray}\displaystyle \bar{\unicode[STIX]{x1D707}}(F) & = & \displaystyle \frac{-\unicode[STIX]{x1D6FE}-\bar{\unicode[STIX]{x1D707}}_{1}-2}{2}+\frac{\unicode[STIX]{x1D6E5}_{1}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}}{2(\bar{\unicode[STIX]{x1D707}}_{1}-\unicode[STIX]{x1D6FE})}\nonumber\\ \displaystyle & & \displaystyle >\frac{-\unicode[STIX]{x1D6FE}-(\unicode[STIX]{x1D6FE}+x_{\unicode[STIX]{x1D6FE}})-2}{2}+\frac{(1/2)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}}{2x_{\unicode[STIX]{x1D6FE}}}=-\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}}.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \bar{\unicode[STIX]{x1D707}}(F) & = & \displaystyle \frac{-\unicode[STIX]{x1D6FE}-\bar{\unicode[STIX]{x1D707}}_{1}-2}{2}+\frac{\unicode[STIX]{x1D6E5}_{1}-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}}{2(\bar{\unicode[STIX]{x1D707}}_{1}-\unicode[STIX]{x1D6FE})}\nonumber\\ \displaystyle & & \displaystyle >\frac{-\unicode[STIX]{x1D6FE}-(\unicode[STIX]{x1D6FE}+x_{\unicode[STIX]{x1D6FE}})-2}{2}+\frac{(1/2)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}}{2x_{\unicode[STIX]{x1D6FE}}}=-\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}}.\nonumber\end{eqnarray}$$
This is the end of proof of the claim. ◻
So for the K-class
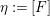 $\unicode[STIX]{x1D702}:=[F]$
, we can apply Proposition 5.7 and conclude that
$\unicode[STIX]{x1D702}:=[F]$
, we can apply Proposition 5.7 and conclude that
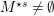 $M^{\star s}\neq \emptyset$
.
$M^{\star s}\neq \emptyset$
.
Finally we consider Case (1).
Claim 9.1.2. We have
 $-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})<\unicode[STIX]{x1D6FE}$
.
$-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})<\unicode[STIX]{x1D6FE}$
.
Proof. Recall that
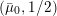 $(\bar{\unicode[STIX]{x1D707}}_{0},1/2)$
is the intersection point with larger
$(\bar{\unicode[STIX]{x1D707}}_{0},1/2)$
is the intersection point with larger
![]() $\bar{\unicode[STIX]{x1D707}}$
-coordinate of the parabola
$\bar{\unicode[STIX]{x1D707}}$
-coordinate of the parabola
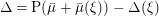 $\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})$
and the line
$\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})$
and the line
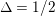 $\unicode[STIX]{x1D6E5}=1/2$
in the
$\unicode[STIX]{x1D6E5}=1/2$
in the
![]() $(\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5})$
-plane. Since
$(\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5})$
-plane. Since
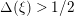 $\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})>1/2$
, we have
$\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})>1/2$
, we have
 $-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})<\bar{\unicode[STIX]{x1D707}}_{0}$
. Let
$-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})<\bar{\unicode[STIX]{x1D707}}_{0}$
. Let
![]() $\unicode[STIX]{x1D708}\in \mathfrak{E}$
with
$\unicode[STIX]{x1D708}\in \mathfrak{E}$
with
 $-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})\in I_{\unicode[STIX]{x1D708}}$
. We have
$-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})\in I_{\unicode[STIX]{x1D708}}$
. We have
![]() $\unicode[STIX]{x1D708}\leqslant \unicode[STIX]{x1D6FE}$
. If
$\unicode[STIX]{x1D708}\leqslant \unicode[STIX]{x1D6FE}$
. If
![]() $\unicode[STIX]{x1D708}<\unicode[STIX]{x1D6FE}$
, then clearly
$\unicode[STIX]{x1D708}<\unicode[STIX]{x1D6FE}$
, then clearly
 $-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})<\unicode[STIX]{x1D6FE}$
and we are done.
$-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})<\unicode[STIX]{x1D6FE}$
and we are done.
Consider the case
![]() $\unicode[STIX]{x1D708}=\unicode[STIX]{x1D6FE}$
. We claim that
$\unicode[STIX]{x1D708}=\unicode[STIX]{x1D6FE}$
. We claim that
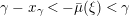 $\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}}<-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})<\unicode[STIX]{x1D6FE}$
. Indeed, if
$\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}}<-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})<\unicode[STIX]{x1D6FE}$
. Indeed, if
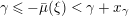 $\unicode[STIX]{x1D6FE}\leqslant -\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})<\unicode[STIX]{x1D6FE}+x_{\unicode[STIX]{x1D6FE}}$
, then
$\unicode[STIX]{x1D6FE}\leqslant -\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})<\unicode[STIX]{x1D6FE}+x_{\unicode[STIX]{x1D6FE}}$
, then
 $-\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}}<\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})\leqslant -\unicode[STIX]{x1D6FE}$
, and since the point
$-\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}}<\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})\leqslant -\unicode[STIX]{x1D6FE}$
, and since the point
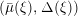 $(\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}),\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709}))$
in the
$(\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}),\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709}))$
in the
![]() $(\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5})$
-plane is above the graph of the function
$(\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5})$
-plane is above the graph of the function
![]() $\unicode[STIX]{x1D6FF}(\bar{\unicode[STIX]{x1D707}})$
, we have
$\unicode[STIX]{x1D6FF}(\bar{\unicode[STIX]{x1D707}})$
, we have
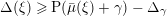 $\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})\geqslant \text{P}(\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})+\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}$
. On the other hand, in Case (1), we have
$\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})\geqslant \text{P}(\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})+\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}$
. On the other hand, in Case (1), we have
 $\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}<\text{P}(\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})+\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})$
. This is a contradiction. This is the end of proof of the claim.◻
$\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}<\text{P}(\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})+\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})$
. This is a contradiction. This is the end of proof of the claim.◻
Let
 $(\bar{\unicode[STIX]{x1D707}}_{1},\unicode[STIX]{x1D6E5}_{1})$
be the intersection of the parabolas
$(\bar{\unicode[STIX]{x1D707}}_{1},\unicode[STIX]{x1D6E5}_{1})$
be the intersection of the parabolas
 $$\begin{eqnarray}\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}\qquad \text{and}\qquad \unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709}).\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}\qquad \text{and}\qquad \unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709}).\end{eqnarray}$$
Using Claim 9.1.2, we can easily check
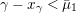 $\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}}<\bar{\unicode[STIX]{x1D707}}_{1}$
. We consider three cases:
$\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}}<\bar{\unicode[STIX]{x1D707}}_{1}$
. We consider three cases:
Case (1-i)
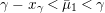 $\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}}<\bar{\unicode[STIX]{x1D707}}_{1}<\unicode[STIX]{x1D6FE}$
,
$\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}}<\bar{\unicode[STIX]{x1D707}}_{1}<\unicode[STIX]{x1D6FE}$
,
Case (1-ii).
![]() $\bar{\unicode[STIX]{x1D707}}_{1}=\unicode[STIX]{x1D6FE}$
,
$\bar{\unicode[STIX]{x1D707}}_{1}=\unicode[STIX]{x1D6FE}$
,
Case (1-iii).
![]() $\unicode[STIX]{x1D6FE}<\bar{\unicode[STIX]{x1D707}}_{1}$
.
$\unicode[STIX]{x1D6FE}<\bar{\unicode[STIX]{x1D707}}_{1}$
.
By calculation we can obtain
 $$\begin{eqnarray}\displaystyle \bar{\unicode[STIX]{x1D707}}_{1}=\unicode[STIX]{x1D6FE}+\frac{1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}-\left(\text{P}(\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})+\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}\right)}{2(\unicode[STIX]{x1D6FE}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))} & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \bar{\unicode[STIX]{x1D707}}_{1}=\unicode[STIX]{x1D6FE}+\frac{1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}-\left(\text{P}(\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})+\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}\right)}{2(\unicode[STIX]{x1D6FE}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))} & & \displaystyle \nonumber\end{eqnarray}$$
and
 $$\begin{eqnarray}\displaystyle \text{r}(\unicode[STIX]{x1D709})-\text{r}(E_{-\unicode[STIX]{x1D6FE}}^{m_{1}})=\frac{\text{r}(\unicode[STIX]{x1D709})\left\{1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}-\left(\text{P}(\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})+\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}\right)\right\}}{1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}}. & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \text{r}(\unicode[STIX]{x1D709})-\text{r}(E_{-\unicode[STIX]{x1D6FE}}^{m_{1}})=\frac{\text{r}(\unicode[STIX]{x1D709})\left\{1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}-\left(\text{P}(\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})+\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}\right)\right\}}{1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}}. & & \displaystyle \nonumber\end{eqnarray}$$
From these equations, we see that the cases (1-i), (1-ii) and (1-iii) correspond to
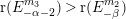 $\text{r}(E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}})>\text{r}(E_{-\unicode[STIX]{x1D6FD}}^{m_{2}})$
,
$\text{r}(E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}})>\text{r}(E_{-\unicode[STIX]{x1D6FD}}^{m_{2}})$
,
 $\text{r}(E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}})=\text{r}(E_{-\unicode[STIX]{x1D6FD}}^{m_{2}})$
and
$\text{r}(E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}})=\text{r}(E_{-\unicode[STIX]{x1D6FD}}^{m_{2}})$
and
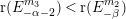 $\text{r}(E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}})<\text{r}(E_{-\unicode[STIX]{x1D6FD}}^{m_{2}})$
, respectively.
$\text{r}(E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}})<\text{r}(E_{-\unicode[STIX]{x1D6FD}}^{m_{2}})$
, respectively.
Let
![]() $\unicode[STIX]{x1D702}$
be the K-class
$\unicode[STIX]{x1D702}$
be the K-class
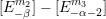 $[E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}]-[E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}]$
.
$[E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}]-[E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}]$
.
Case (1-ii). In this case, we can apply Proposition 5.9 for the K-class
![]() $\unicode[STIX]{x1D702}$
and conclude
$\unicode[STIX]{x1D702}$
and conclude
 $M^{\star s}\neq \emptyset$
.
$M^{\star s}\neq \emptyset$
.
In cases (1-i) and (1-iii), put
 $\bar{\unicode[STIX]{x1D707}}_{2}=\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D702})$
and
$\bar{\unicode[STIX]{x1D707}}_{2}=\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D702})$
and
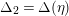 $\unicode[STIX]{x1D6E5}_{2}=\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D702})$
. Since the point
$\unicode[STIX]{x1D6E5}_{2}=\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D702})$
. Since the point
 $(\bar{\unicode[STIX]{x1D707}}_{2},\unicode[STIX]{x1D6E5}_{2})$
in the
$(\bar{\unicode[STIX]{x1D707}}_{2},\unicode[STIX]{x1D6E5}_{2})$
in the
![]() $(\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5})$
-plane lies on the parabolas
$(\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5})$
-plane lies on the parabolas
 $$\begin{eqnarray}\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\bar{\unicode[STIX]{x1D707}}_{1})-\unicode[STIX]{x1D6E5}_{1}\qquad \text{and}\qquad \unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}},\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\bar{\unicode[STIX]{x1D707}}_{1})-\unicode[STIX]{x1D6E5}_{1}\qquad \text{and}\qquad \unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}},\end{eqnarray}$$
we have, by calculation,
 $$\begin{eqnarray}\bar{\unicode[STIX]{x1D707}}_{2}=-\unicode[STIX]{x1D6FE}+\frac{1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}}{2(\bar{\unicode[STIX]{x1D707}}_{1}-\unicode[STIX]{x1D6FE})}.\end{eqnarray}$$
$$\begin{eqnarray}\bar{\unicode[STIX]{x1D707}}_{2}=-\unicode[STIX]{x1D6FE}+\frac{1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}}{2(\bar{\unicode[STIX]{x1D707}}_{1}-\unicode[STIX]{x1D6FE})}.\end{eqnarray}$$
Case (1-iii). In this case, we have
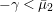 $-\unicode[STIX]{x1D6FE}<\bar{\unicode[STIX]{x1D707}}_{2}$
. So we can apply Proposition 5.7 for the K-class
$-\unicode[STIX]{x1D6FE}<\bar{\unicode[STIX]{x1D707}}_{2}$
. So we can apply Proposition 5.7 for the K-class
![]() $\unicode[STIX]{x1D702}$
and conclude that
$\unicode[STIX]{x1D702}$
and conclude that
 $M^{\star s}\neq \emptyset$
.
$M^{\star s}\neq \emptyset$
.
Case (1-i). In this case, we consider the dual map
 $$\begin{eqnarray}f^{\ast }:E_{\unicode[STIX]{x1D6FD}}^{m_{2}}\rightarrow E_{\unicode[STIX]{x1D6FC}+2}^{m_{3}}.\end{eqnarray}$$
$$\begin{eqnarray}f^{\ast }:E_{\unicode[STIX]{x1D6FD}}^{m_{2}}\rightarrow E_{\unicode[STIX]{x1D6FC}+2}^{m_{3}}.\end{eqnarray}$$
Let
![]() $\unicode[STIX]{x1D702}^{\dagger }$
be the K-class
$\unicode[STIX]{x1D702}^{\dagger }$
be the K-class
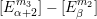 $[E_{\unicode[STIX]{x1D6FC}+2}^{m_{3}}]-[E_{\unicode[STIX]{x1D6FD}}^{m_{2}}]$
. We have
$[E_{\unicode[STIX]{x1D6FC}+2}^{m_{3}}]-[E_{\unicode[STIX]{x1D6FD}}^{m_{2}}]$
. We have
 $$\begin{eqnarray}\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D702}^{\dagger })=-\bar{\unicode[STIX]{x1D707}}_{2}=\unicode[STIX]{x1D6FE}+\frac{1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}}{2(\unicode[STIX]{x1D6FE}-\bar{\unicode[STIX]{x1D707}}_{1})}>\unicode[STIX]{x1D6FE}+\frac{1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}}{2x_{\unicode[STIX]{x1D6FE}}}=\unicode[STIX]{x1D6FE}+2-x_{\unicode[STIX]{x1D6FE}}.\end{eqnarray}$$
$$\begin{eqnarray}\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D702}^{\dagger })=-\bar{\unicode[STIX]{x1D707}}_{2}=\unicode[STIX]{x1D6FE}+\frac{1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}}{2(\unicode[STIX]{x1D6FE}-\bar{\unicode[STIX]{x1D707}}_{1})}>\unicode[STIX]{x1D6FE}+\frac{1-2\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}}{2x_{\unicode[STIX]{x1D6FE}}}=\unicode[STIX]{x1D6FE}+2-x_{\unicode[STIX]{x1D6FE}}.\end{eqnarray}$$
Then we can apply Proposition 5.7 for the K-class
![]() $\unicode[STIX]{x1D702}^{\dagger }$
and conclude that
$\unicode[STIX]{x1D702}^{\dagger }$
and conclude that
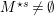 $M^{\star s}\neq \emptyset$
.◻
$M^{\star s}\neq \emptyset$
.◻
9.3 Moving curves
Let us see that there are moving curves in the domain of definition of the rational map
![]() $\unicode[STIX]{x1D70B}$
.
$\unicode[STIX]{x1D70B}$
.
Proposition 9.2. In Case (1) or Case (3), for a general point
![]() $x$
of
$x$
of
![]() $M(\unicode[STIX]{x1D709})$
, there exists a complete curve
$M(\unicode[STIX]{x1D709})$
, there exists a complete curve
![]() $C$
passing through
$C$
passing through
![]() $x$
in
$x$
in
![]() $M(\unicode[STIX]{x1D709})^{\circ \unicode[STIX]{x1D70E}}$
such that
$M(\unicode[STIX]{x1D709})^{\circ \unicode[STIX]{x1D70E}}$
such that
![]() $\unicode[STIX]{x1D70B}(C)$
is a point.
$\unicode[STIX]{x1D70B}(C)$
is a point.
Proposition 9.3. In Case (2), the map
![]() $\unicode[STIX]{x1D70B}$
is birational. More precisely, it gives rise to an open immersion of
$\unicode[STIX]{x1D70B}$
is birational. More precisely, it gives rise to an open immersion of
![]() $M(\unicode[STIX]{x1D709})^{\circ }$
to
$M(\unicode[STIX]{x1D709})^{\circ }$
to
![]() $M^{\star s}$
. For a general point
$M^{\star s}$
. For a general point
![]() $x$
of
$x$
of
![]() $M(\unicode[STIX]{x1D709})$
, there exists a complete curve in
$M(\unicode[STIX]{x1D709})$
, there exists a complete curve in
![]() $M(\unicode[STIX]{x1D709})^{\circ }$
passing through
$M(\unicode[STIX]{x1D709})^{\circ }$
passing through
![]() $x$
.
$x$
.
Proof of Proposition 9.2.
We give a proof for Case (1). We first check that the dimension of the fiber of the rational map
![]() $\unicode[STIX]{x1D70B}$
is positive. By calculation, the dimension of the fiber of the rational map
$\unicode[STIX]{x1D70B}$
is positive. By calculation, the dimension of the fiber of the rational map
![]() $\unicode[STIX]{x1D70B}$
is
$\unicode[STIX]{x1D70B}$
is
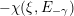 $-\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709},E_{-\unicode[STIX]{x1D6FE}})$
. We need to show
$-\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709},E_{-\unicode[STIX]{x1D6FE}})$
. We need to show
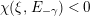 $\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709},E_{-\unicode[STIX]{x1D6FE}})<0$
.
$\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709},E_{-\unicode[STIX]{x1D6FE}})<0$
.
When
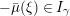 $-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})\in I_{\unicode[STIX]{x1D6FE}}$
, we have
$-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})\in I_{\unicode[STIX]{x1D6FE}}$
, we have
 $$\begin{eqnarray}\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})>\text{P}(\unicode[STIX]{x1D6FE}-2+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})>\text{P}(\unicode[STIX]{x1D6FE}-2+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}\end{eqnarray}$$
since the height of the moduli space
![]() $M(\unicode[STIX]{x1D709})$
is positive. From this, we have
$M(\unicode[STIX]{x1D709})$
is positive. From this, we have
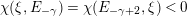 $\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709},E_{-\unicode[STIX]{x1D6FE}})=\unicode[STIX]{x1D712}(E_{-\unicode[STIX]{x1D6FE}+2},\unicode[STIX]{x1D709})<0$
.
$\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709},E_{-\unicode[STIX]{x1D6FE}})=\unicode[STIX]{x1D712}(E_{-\unicode[STIX]{x1D6FE}+2},\unicode[STIX]{x1D709})<0$
.
When
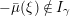 $-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})\notin I_{\unicode[STIX]{x1D6FE}}$
, we have
$-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})\notin I_{\unicode[STIX]{x1D6FE}}$
, we have
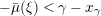 $-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})<\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}}$
. Moving upward the graph
$-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})<\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}}$
. Moving upward the graph
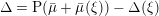 $\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})$
in the
$\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})$
in the
![]() $(\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5})$
-plane, we can find a positive number
$(\bar{\unicode[STIX]{x1D707}},\unicode[STIX]{x1D6E5})$
-plane, we can find a positive number
![]() $\unicode[STIX]{x1D70C}$
such that the graph
$\unicode[STIX]{x1D70C}$
such that the graph
 $\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})+\unicode[STIX]{x1D70C}$
passes through the point
$\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})+\unicode[STIX]{x1D70C}$
passes through the point
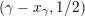 $(\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}},1/2)$
. Then by calculation,
$(\unicode[STIX]{x1D6FE}-x_{\unicode[STIX]{x1D6FE}},1/2)$
. Then by calculation,
 $$\begin{eqnarray}\displaystyle \text{P}(-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})-\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}+\unicode[STIX]{x1D70C} & = & \displaystyle (x_{\unicode[STIX]{x1D6FE}}-2)(2(\unicode[STIX]{x1D6FE}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-x_{\unicode[STIX]{x1D6FE}})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}+1/2\nonumber\\ \displaystyle & {<} & \displaystyle x_{\unicode[STIX]{x1D6FE}}(x_{\unicode[STIX]{x1D6FE}}-2)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}+1/2=0.\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \text{P}(-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})-\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}+\unicode[STIX]{x1D70C} & = & \displaystyle (x_{\unicode[STIX]{x1D6FE}}-2)(2(\unicode[STIX]{x1D6FE}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-x_{\unicode[STIX]{x1D6FE}})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}+1/2\nonumber\\ \displaystyle & {<} & \displaystyle x_{\unicode[STIX]{x1D6FE}}(x_{\unicode[STIX]{x1D6FE}}-2)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}+1/2=0.\nonumber\end{eqnarray}$$
By this, we have
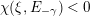 $\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709},E_{-\unicode[STIX]{x1D6FE}})<0$
.
$\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709},E_{-\unicode[STIX]{x1D6FE}})<0$
.
We turn to the existence of a complete curve in
![]() $M(\unicode[STIX]{x1D709})^{\circ \unicode[STIX]{x1D70E}}$
passing through a general point. Recall that we put
$M(\unicode[STIX]{x1D709})^{\circ \unicode[STIX]{x1D70E}}$
passing through a general point. Recall that we put
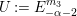 $U:=E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}$
and
$U:=E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}$
and
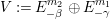 $V:=E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}\oplus E_{-\unicode[STIX]{x1D6FE}}^{m_{1}}$
. Put
$V:=E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}\oplus E_{-\unicode[STIX]{x1D6FE}}^{m_{1}}$
. Put
 $$\begin{eqnarray}\text{H}_{1}:=\text{Hom}(U,E_{-\unicode[STIX]{x1D6FE}}^{m_{1}})\qquad \text{and}\qquad \text{H}_{2}:=\text{Hom}(U,E_{-\unicode[STIX]{x1D6FD}}^{m_{2}})\end{eqnarray}$$
$$\begin{eqnarray}\text{H}_{1}:=\text{Hom}(U,E_{-\unicode[STIX]{x1D6FE}}^{m_{1}})\qquad \text{and}\qquad \text{H}_{2}:=\text{Hom}(U,E_{-\unicode[STIX]{x1D6FD}}^{m_{2}})\end{eqnarray}$$
so that
 $\text{Hom}(U,V)\simeq \text{H}_{2}\oplus \text{H}_{1}$
. Take a general
$\text{Hom}(U,V)\simeq \text{H}_{2}\oplus \text{H}_{1}$
. Take a general
![]() $f\in \text{H}_{2}$
. Then the representation of the quiver corresponding to
$f\in \text{H}_{2}$
. Then the representation of the quiver corresponding to
![]() $f$
is
$f$
is
![]() $\unicode[STIX]{x1D703}$
-stable. Consider the open subset
$\unicode[STIX]{x1D703}$
-stable. Consider the open subset
 $Y_{f}\subset \text{H}_{1}$
consisting of
$Y_{f}\subset \text{H}_{1}$
consisting of
![]() $g\in \text{H}_{1}$
such that the morphism
$g\in \text{H}_{1}$
such that the morphism
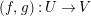 $(f,g):U\rightarrow V$
is injective with stable cokernel. Since the open subset
$(f,g):U\rightarrow V$
is injective with stable cokernel. Since the open subset
![]() $Y_{f}$
is invariant under the action of
$Y_{f}$
is invariant under the action of
![]() $\mathbb{C}^{\times }$
by multiplication, we can consider the projectivization
$\mathbb{C}^{\times }$
by multiplication, we can consider the projectivization
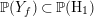 $\mathbb{P}(Y_{f})\subset \mathbb{P}(\text{H}_{1})$
. Since
$\mathbb{P}(Y_{f})\subset \mathbb{P}(\text{H}_{1})$
. Since
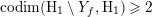 $\text{codim}(\text{H}_{1}\setminus Y_{f},\text{H}_{1})\geqslant 2$
by the explanation before Remark 8.2, a general line in
$\text{codim}(\text{H}_{1}\setminus Y_{f},\text{H}_{1})\geqslant 2$
by the explanation before Remark 8.2, a general line in
![]() $\text{P}(\text{H}_{1})$
lies inside
$\text{P}(\text{H}_{1})$
lies inside
![]() $\text{P}(Y_{f})$
and determines a complete curve in
$\text{P}(Y_{f})$
and determines a complete curve in
![]() $M(\unicode[STIX]{x1D709})^{\circ \unicode[STIX]{x1D70E}}$
. This curve is mapped to a point by
$M(\unicode[STIX]{x1D709})^{\circ \unicode[STIX]{x1D70E}}$
. This curve is mapped to a point by
![]() $\unicode[STIX]{x1D70B}$
because
$\unicode[STIX]{x1D70B}$
because
![]() $f$
is fixed.
$f$
is fixed.
The argument for Case (3) is similar (and easier). ◻
Proof of Proposition 9.3.
The argument goes as in the proof of Proposition 9.2. We employ the same notation used there. In Case (2), we have
![]() $m_{1}=0$
. In the last paragraph of Section 8, we considered the open subset
$m_{1}=0$
. In the last paragraph of Section 8, we considered the open subset
 $Y^{\prime }\subset \text{Hom}(U,V)$
consisting of injective morphisms with stable cokernel. By the action of
$Y^{\prime }\subset \text{Hom}(U,V)$
consisting of injective morphisms with stable cokernel. By the action of
![]() $\mathbb{C}^{\times }$
by multiplication,
$\mathbb{C}^{\times }$
by multiplication,
![]() $Y^{\prime }$
is invariant. Consider the projectivization
$Y^{\prime }$
is invariant. Consider the projectivization
 $\mathbb{P}(Y^{\prime })\subset \mathbb{P}\text{Hom}(U,V)$
. Since the complement of
$\mathbb{P}(Y^{\prime })\subset \mathbb{P}\text{Hom}(U,V)$
. Since the complement of
![]() $\mathbb{P}(Y^{\prime })$
in
$\mathbb{P}(Y^{\prime })$
in
 $\mathbb{P}\text{Hom}(U,V)$
has codimension at least
$\mathbb{P}\text{Hom}(U,V)$
has codimension at least
![]() $2$
, a general line in
$2$
, a general line in
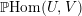 $\mathbb{P}\text{Hom}(U,V)$
lies in
$\mathbb{P}\text{Hom}(U,V)$
lies in
![]() $\mathbb{P}(Y^{\prime })$
, which determines a complete curve in
$\mathbb{P}(Y^{\prime })$
, which determines a complete curve in
![]() $M(\unicode[STIX]{x1D709})^{\circ }$
.◻
$M(\unicode[STIX]{x1D709})^{\circ }$
.◻
Remark 9.4. Let
![]() $M(\unicode[STIX]{x1D709})^{\unicode[STIX]{x1D707}s}$
be the open subscheme of
$M(\unicode[STIX]{x1D709})^{\unicode[STIX]{x1D707}s}$
be the open subscheme of
![]() $M(\unicode[STIX]{x1D709})$
consisting of
$M(\unicode[STIX]{x1D709})$
consisting of
![]() $\unicode[STIX]{x1D707}$
-stable bundles. When
$\unicode[STIX]{x1D707}$
-stable bundles. When
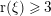 $\text{r}(\unicode[STIX]{x1D709})\geqslant 3$
, substituting
$\text{r}(\unicode[STIX]{x1D709})\geqslant 3$
, substituting
![]() $Y^{\prime \prime }$
in Remark 8.2 for
$Y^{\prime \prime }$
in Remark 8.2 for
![]() $Y^{\prime }$
in the above proof, we can find a complete curve passing through a general point in Propositions 9.2 and 9.3 inside
$Y^{\prime }$
in the above proof, we can find a complete curve passing through a general point in Propositions 9.2 and 9.3 inside
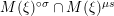 $M(\unicode[STIX]{x1D709})^{\circ \unicode[STIX]{x1D70E}}\cap M(\unicode[STIX]{x1D709})^{\unicode[STIX]{x1D707}s}$
.
$M(\unicode[STIX]{x1D709})^{\circ \unicode[STIX]{x1D70E}}\cap M(\unicode[STIX]{x1D709})^{\unicode[STIX]{x1D707}s}$
.
10 Effective cone of the moduli space
10.1 Line bundles on moduli spaces
We fix notation for line bundles on a moduli space of sheaves.
For a flat family
![]() ${\mathcal{E}}$
of coherent sheaves on
${\mathcal{E}}$
of coherent sheaves on
![]() $S$
parametrized by a scheme
$S$
parametrized by a scheme
![]() $T$
, we let
$T$
, we let
 $\unicode[STIX]{x1D706}_{{\mathcal{E}}}:K(S)\rightarrow \text{Pic}(T)$
be the composition of homomorphisms
$\unicode[STIX]{x1D706}_{{\mathcal{E}}}:K(S)\rightarrow \text{Pic}(T)$
be the composition of homomorphisms
 $$\begin{eqnarray}K(S)\xrightarrow[{}]{q^{\ast }}K^{0}(S\times T)\xrightarrow[{}]{\cdot [{\mathcal{E}}]}K^{0}(S\times T)\xrightarrow[{}]{p_{!}}K^{0}(T)\xrightarrow[{}]{\text{det}}\text{Pic}(T),\end{eqnarray}$$
$$\begin{eqnarray}K(S)\xrightarrow[{}]{q^{\ast }}K^{0}(S\times T)\xrightarrow[{}]{\cdot [{\mathcal{E}}]}K^{0}(S\times T)\xrightarrow[{}]{p_{!}}K^{0}(T)\xrightarrow[{}]{\text{det}}\text{Pic}(T),\end{eqnarray}$$
where
 $p:S\times T\rightarrow T$
and
$p:S\times T\rightarrow T$
and
 $q:S\times T\rightarrow S$
are projections, and
$q:S\times T\rightarrow S$
are projections, and
![]() $K^{0}(?)$
is the Grothendieck group of locally free sheaves on
$K^{0}(?)$
is the Grothendieck group of locally free sheaves on
![]() $?$
$?$
Fix a semistable class
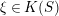 $\unicode[STIX]{x1D709}\in K(S)$
. Let
$\unicode[STIX]{x1D709}\in K(S)$
. Let
![]() $H$
be a smooth divisor in the very ample linear system
$H$
be a smooth divisor in the very ample linear system
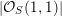 $|{\mathcal{O}}_{S}(1,1)|$
and let
$|{\mathcal{O}}_{S}(1,1)|$
and let
 $h=[{\mathcal{O}}_{H}]$
.
$h=[{\mathcal{O}}_{H}]$
.
As in [Reference Huybrechts and LehnHL, Definition 8.1.4], we put
 $$\begin{eqnarray}K_{\unicode[STIX]{x1D709}}:=\unicode[STIX]{x1D709}^{\bot }\qquad \text{and}\qquad K_{\unicode[STIX]{x1D709},H}:=\unicode[STIX]{x1D709}^{\bot }\cap \{1,h,h^{2}\}^{\bot \bot }.\end{eqnarray}$$
$$\begin{eqnarray}K_{\unicode[STIX]{x1D709}}:=\unicode[STIX]{x1D709}^{\bot }\qquad \text{and}\qquad K_{\unicode[STIX]{x1D709},H}:=\unicode[STIX]{x1D709}^{\bot }\cap \{1,h,h^{2}\}^{\bot \bot }.\end{eqnarray}$$
Since a class
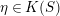 $\unicode[STIX]{x1D702}\in K(S)$
is in
$\unicode[STIX]{x1D702}\in K(S)$
is in
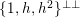 $\{1,h,h^{2}\}^{\bot \bot }$
if and only if
$\{1,h,h^{2}\}^{\bot \bot }$
if and only if
![]() $\unicode[STIX]{x1D702}$
has symmetric
$\unicode[STIX]{x1D702}$
has symmetric
![]() $c_{1}$
, we can also describe
$c_{1}$
, we can also describe
![]() $K_{\unicode[STIX]{x1D709},H}$
as
$K_{\unicode[STIX]{x1D709},H}$
as
 $$\begin{eqnarray}K_{\unicode[STIX]{x1D709},H}=\left\{\unicode[STIX]{x1D702}\in K_{\unicode[STIX]{x1D709}}\mid \unicode[STIX]{x1D702}\text{ has symmetric }c_{1}\right\}\!.\end{eqnarray}$$
$$\begin{eqnarray}K_{\unicode[STIX]{x1D709},H}=\left\{\unicode[STIX]{x1D702}\in K_{\unicode[STIX]{x1D709}}\mid \unicode[STIX]{x1D702}\text{ has symmetric }c_{1}\right\}\!.\end{eqnarray}$$
So, from now on, we write
![]() $K_{\unicode[STIX]{x1D709}}^{\text{sym}}$
for
$K_{\unicode[STIX]{x1D709}}^{\text{sym}}$
for
![]() $K_{\unicode[STIX]{x1D709},H}$
.
$K_{\unicode[STIX]{x1D709},H}$
.
By [Reference Huybrechts and LehnHL, Theorem 8.1.5], we have a homomorphism
 $$\begin{eqnarray}\unicode[STIX]{x1D706}:K_{\unicode[STIX]{x1D709}}^{\text{sym}}\rightarrow \text{Pic}\left(M(\unicode[STIX]{x1D709})\right)\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D706}:K_{\unicode[STIX]{x1D709}}^{\text{sym}}\rightarrow \text{Pic}\left(M(\unicode[STIX]{x1D709})\right)\end{eqnarray}$$
such that if
![]() ${\mathcal{E}}$
is a flat family of semistable sheaves with K-class
${\mathcal{E}}$
is a flat family of semistable sheaves with K-class
![]() $\unicode[STIX]{x1D709}$
on
$\unicode[STIX]{x1D709}$
on
![]() $S$
parametrized by a scheme
$S$
parametrized by a scheme
![]() $T$
, then
$T$
, then
 $\unicode[STIX]{x1D719}_{{\mathcal{E}}}^{\ast }\circ \unicode[STIX]{x1D706}=\unicode[STIX]{x1D706}_{{\mathcal{E}}}$
, where
$\unicode[STIX]{x1D719}_{{\mathcal{E}}}^{\ast }\circ \unicode[STIX]{x1D706}=\unicode[STIX]{x1D706}_{{\mathcal{E}}}$
, where
 $\unicode[STIX]{x1D719}_{{\mathcal{E}}}:T\rightarrow M(\unicode[STIX]{x1D709})$
is the classifying morphism.
$\unicode[STIX]{x1D719}_{{\mathcal{E}}}:T\rightarrow M(\unicode[STIX]{x1D709})$
is the classifying morphism.
As in [Reference Huybrechts and LehnHL, Definition 8.1.9], we define a line bundle
![]() ${\mathcal{L}}_{i}$
by
${\mathcal{L}}_{i}$
by
 $$\begin{eqnarray}{\mathcal{L}}_{i}:=\unicode[STIX]{x1D706}\left(u_{i}(\unicode[STIX]{x1D709})\right)\!,\end{eqnarray}$$
$$\begin{eqnarray}{\mathcal{L}}_{i}:=\unicode[STIX]{x1D706}\left(u_{i}(\unicode[STIX]{x1D709})\right)\!,\end{eqnarray}$$
where
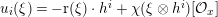 $u_{i}(\unicode[STIX]{x1D709})=-\text{r}(\unicode[STIX]{x1D709})\cdot h^{i}+\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709}\otimes h^{i})[{\mathcal{O}}_{x}]$
with
$u_{i}(\unicode[STIX]{x1D709})=-\text{r}(\unicode[STIX]{x1D709})\cdot h^{i}+\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709}\otimes h^{i})[{\mathcal{O}}_{x}]$
with
![]() $x\in S$
a closed point.
$x\in S$
a closed point.
For
![]() $m\gg 0$
,
$m\gg 0$
,
 ${\mathcal{L}}_{0}\otimes {\mathcal{L}}_{1}^{m}$
is an ample line bundle on
${\mathcal{L}}_{0}\otimes {\mathcal{L}}_{1}^{m}$
is an ample line bundle on
![]() $M(\unicode[STIX]{x1D709})$
(cf. [Reference Huybrechts and LehnHL, Remark 8.1.12]).
$M(\unicode[STIX]{x1D709})$
(cf. [Reference Huybrechts and LehnHL, Remark 8.1.12]).
10.2 Edges of the cone
In the rest of this section, we assume that the semistable class
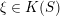 $\unicode[STIX]{x1D709}\in K(S)$
has symmetric
$\unicode[STIX]{x1D709}\in K(S)$
has symmetric
![]() $c_{1}$
such that the height of the moduli space
$c_{1}$
such that the height of the moduli space
![]() $M(\unicode[STIX]{x1D709})$
is positive. Let
$M(\unicode[STIX]{x1D709})$
is positive. Let
![]() $\unicode[STIX]{x1D6FE}$
be the symmetric exceptional slope determined by Lemma 8.1.
$\unicode[STIX]{x1D6FE}$
be the symmetric exceptional slope determined by Lemma 8.1.
We denote also by
![]() $\unicode[STIX]{x1D706}$
the map
$\unicode[STIX]{x1D706}$
the map
 $$\begin{eqnarray}\unicode[STIX]{x1D706}:K_{\unicode[STIX]{x1D709}}^{\text{sym}}\otimes \mathbb{R}\rightarrow \text{NS}\left(M(\unicode[STIX]{x1D709})\right)_{\mathbb{R}}\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D706}:K_{\unicode[STIX]{x1D709}}^{\text{sym}}\otimes \mathbb{R}\rightarrow \text{NS}\left(M(\unicode[STIX]{x1D709})\right)_{\mathbb{R}}\end{eqnarray}$$
induced by (10.1).
Lemma 10.1. The map
![]() $\unicode[STIX]{x1D706}$
in (10.2) is injective.
$\unicode[STIX]{x1D706}$
in (10.2) is injective.
Proof. When
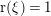 $\text{r}(\unicode[STIX]{x1D709})=1$
, the exceptional divisor of the Hilbert–Chow morphism is effective but not ample, so
$\text{r}(\unicode[STIX]{x1D709})=1$
, the exceptional divisor of the Hilbert–Chow morphism is effective but not ample, so
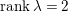 $\text{rank}\,\unicode[STIX]{x1D706}=2$
.
$\text{rank}\,\unicode[STIX]{x1D706}=2$
.
When
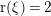 $\text{r}(\unicode[STIX]{x1D709})=2$
, the divisor of
$\text{r}(\unicode[STIX]{x1D709})=2$
, the divisor of
![]() $M(\unicode[STIX]{x1D709})$
consisting of nonlocally free sheaves is effective but not ample, so
$M(\unicode[STIX]{x1D709})$
consisting of nonlocally free sheaves is effective but not ample, so
 $\text{rank}\,\unicode[STIX]{x1D706}=2$
.
$\text{rank}\,\unicode[STIX]{x1D706}=2$
.
The proof for the case
 $\text{r}(\unicode[STIX]{x1D709})\geqslant 3$
is postponed after the proof of Theorem 10.2.◻
$\text{r}(\unicode[STIX]{x1D709})\geqslant 3$
is postponed after the proof of Theorem 10.2.◻
Put
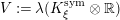 $V:=\unicode[STIX]{x1D706}(K_{\unicode[STIX]{x1D709}}^{\text{sym}}\otimes \mathbb{R})$
. In this section, we determine the cone
$V:=\unicode[STIX]{x1D706}(K_{\unicode[STIX]{x1D709}}^{\text{sym}}\otimes \mathbb{R})$
. In this section, we determine the cone
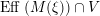 $\text{Eff}\left(M(\unicode[STIX]{x1D709})\right)\cap V$
.
$\text{Eff}\left(M(\unicode[STIX]{x1D709})\right)\cap V$
.
We define
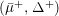 $(\bar{\unicode[STIX]{x1D707}}^{+},\unicode[STIX]{x1D6E5}^{+})$
as follows (see the paragraph after Lemma 8.1 for Cases (1), (2) and (3)):
$(\bar{\unicode[STIX]{x1D707}}^{+},\unicode[STIX]{x1D6E5}^{+})$
as follows (see the paragraph after Lemma 8.1 for Cases (1), (2) and (3)):
In Case (1), we define
 $(\bar{\unicode[STIX]{x1D707}}^{+},\unicode[STIX]{x1D6E5}^{+})$
to be the intersection of the parabolas
$(\bar{\unicode[STIX]{x1D707}}^{+},\unicode[STIX]{x1D6E5}^{+})$
to be the intersection of the parabolas
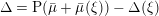 $\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})$
and
$\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})$
and
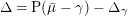 $\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}$
.
$\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FE})-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}$
.
In Case (2), we define
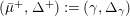 $(\bar{\unicode[STIX]{x1D707}}^{+},\unicode[STIX]{x1D6E5}^{+}):=(\unicode[STIX]{x1D6FE},\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}})$
.
$(\bar{\unicode[STIX]{x1D707}}^{+},\unicode[STIX]{x1D6E5}^{+}):=(\unicode[STIX]{x1D6FE},\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}})$
.
In Case (3), we define
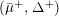 $(\bar{\unicode[STIX]{x1D707}}^{+},\unicode[STIX]{x1D6E5}^{+})$
to be the intersection of the parabolas
$(\bar{\unicode[STIX]{x1D707}}^{+},\unicode[STIX]{x1D6E5}^{+})$
to be the intersection of the parabolas
 $\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})$
and
$\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}+\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}))-\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709})$
and
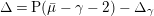 $\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FE}-2)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}$
.
$\unicode[STIX]{x1D6E5}=\text{P}(\bar{\unicode[STIX]{x1D707}}-\unicode[STIX]{x1D6FE}-2)-\unicode[STIX]{x1D6E5}_{\unicode[STIX]{x1D6FE}}$
.
Let
![]() $r^{+}$
be the smallest positive integer such that
$r^{+}$
be the smallest positive integer such that
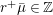 $r^{+}\bar{\unicode[STIX]{x1D707}}\in \mathbb{Z}$
and
$r^{+}\bar{\unicode[STIX]{x1D707}}\in \mathbb{Z}$
and
 $r^{+}(\text{P}(\bar{\unicode[STIX]{x1D707}}^{+})-\unicode[STIX]{x1D6E5}^{+})\in \mathbb{Z}$
. We define
$r^{+}(\text{P}(\bar{\unicode[STIX]{x1D707}}^{+})-\unicode[STIX]{x1D6E5}^{+})\in \mathbb{Z}$
. We define
![]() $\unicode[STIX]{x1D709}^{+}$
to be an element of
$\unicode[STIX]{x1D709}^{+}$
to be an element of
![]() $K_{\unicode[STIX]{x1D709}}^{\text{sym}}$
such that
$K_{\unicode[STIX]{x1D709}}^{\text{sym}}$
such that
 $\text{r}(\unicode[STIX]{x1D709}^{+})=r^{+}$
,
$\text{r}(\unicode[STIX]{x1D709}^{+})=r^{+}$
,
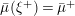 $\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}^{+})=\bar{\unicode[STIX]{x1D707}}^{+}$
and
$\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}^{+})=\bar{\unicode[STIX]{x1D707}}^{+}$
and
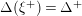 $\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709}^{+})=\unicode[STIX]{x1D6E5}^{+}$
.
$\unicode[STIX]{x1D6E5}(\unicode[STIX]{x1D709}^{+})=\unicode[STIX]{x1D6E5}^{+}$
.
Theorem 10.2.
-
(a) The ray spanned by
 $\unicode[STIX]{x1D706}(-\unicode[STIX]{x1D709}^{+})$
in
$\unicode[STIX]{x1D706}(-\unicode[STIX]{x1D709}^{+})$
in
 $\text{NS}(M(\unicode[STIX]{x1D709}))_{\mathbb{R}}$
is an edge of
$\text{NS}(M(\unicode[STIX]{x1D709}))_{\mathbb{R}}$
is an edge of
 $\text{Eff}(M(\unicode[STIX]{x1D709}))\cap V$
.
$\text{Eff}(M(\unicode[STIX]{x1D709}))\cap V$
. -
(b) The other edge of
 $\text{Eff}(M(\unicode[STIX]{x1D709}))\cap V$
is spanned by
$\text{Eff}(M(\unicode[STIX]{x1D709}))\cap V$
is spanned by
 $\unicode[STIX]{x1D706}(\unicode[STIX]{x1D702})$
. Here
$\unicode[STIX]{x1D706}(\unicode[STIX]{x1D702})$
. Here
 $\unicode[STIX]{x1D702}$
is the element of
$\unicode[STIX]{x1D702}$
is the element of
 $K_{\unicode[STIX]{x1D709}}^{\text{sym}}\otimes \mathbb{R}$
with
$K_{\unicode[STIX]{x1D709}}^{\text{sym}}\otimes \mathbb{R}$
with
 $\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D702})=-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})$
and
$\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D702})=-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})$
and
 $\text{r}(\unicode[STIX]{x1D702})>0$
when
$\text{r}(\unicode[STIX]{x1D702})>0$
when
 $\text{r}(\unicode[STIX]{x1D709})=1$
;
$\text{r}(\unicode[STIX]{x1D709})=1$
;
 $\unicode[STIX]{x1D702}\in K_{\unicode[STIX]{x1D709}}^{\text{sym}}\otimes \mathbb{R}$
is such that
$\unicode[STIX]{x1D702}\in K_{\unicode[STIX]{x1D709}}^{\text{sym}}\otimes \mathbb{R}$
is such that
 $\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D702})=-1-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})$
and
$\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D702})=-1-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})$
and
 $\text{r}(\unicode[STIX]{x1D702})>0$
when
$\text{r}(\unicode[STIX]{x1D702})>0$
when
 $\text{r}(\unicode[STIX]{x1D709})=2$
; and
$\text{r}(\unicode[STIX]{x1D709})=2$
; and
 $\unicode[STIX]{x1D702}=((\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{+})^{\ast }$
when
$\unicode[STIX]{x1D702}=((\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{+})^{\ast }$
when
 $\text{r}(\unicode[STIX]{x1D709})\geqslant 3$
.
$\text{r}(\unicode[STIX]{x1D709})\geqslant 3$
.
Sketch of Proof.
The argument is the same as in [Reference Coskun, Huizenga and WoolfCHW].
We let
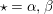 $\star =\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD}$
or
$\star =\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD}$
or
![]() $\unicode[STIX]{x1D6FE}$
depending on whether
$\unicode[STIX]{x1D6FE}$
depending on whether
![]() $\unicode[STIX]{x1D6FC}$
,
$\unicode[STIX]{x1D6FC}$
,
![]() $\unicode[STIX]{x1D6FD}$
or
$\unicode[STIX]{x1D6FD}$
or
![]() $\unicode[STIX]{x1D6FE}$
is even.
$\unicode[STIX]{x1D6FE}$
is even.
(a) Case
![]() $(1)$
. To show
$(1)$
. To show
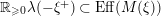 $\mathbb{R}_{{\geqslant}0}\unicode[STIX]{x1D706}(-\unicode[STIX]{x1D709}^{+})\subset \text{Eff}(M(\unicode[STIX]{x1D709}))$
, it suffices to find
$\mathbb{R}_{{\geqslant}0}\unicode[STIX]{x1D706}(-\unicode[STIX]{x1D709}^{+})\subset \text{Eff}(M(\unicode[STIX]{x1D709}))$
, it suffices to find
 $E\in M(\unicode[STIX]{x1D709})$
and
$E\in M(\unicode[STIX]{x1D709})$
and
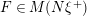 $F\in M(N\unicode[STIX]{x1D709}^{+})$
for some
$F\in M(N\unicode[STIX]{x1D709}^{+})$
for some
![]() $N>0$
such that
$N>0$
such that
 $\text{H}^{i}(E\otimes F)=0$
for all
$\text{H}^{i}(E\otimes F)=0$
for all
![]() $i$
. In fact, then,
$i$
. In fact, then,
 $\unicode[STIX]{x1D706}(-N\unicode[STIX]{x1D709}^{+})$
is the class of the effective divisor
$\unicode[STIX]{x1D706}(-N\unicode[STIX]{x1D709}^{+})$
is the class of the effective divisor
 $\unicode[STIX]{x1D6E9}_{F}:=\{E\in M(\unicode[STIX]{x1D709})\mid \text{H}^{0}(E\otimes F)\neq 0\}$
.
$\unicode[STIX]{x1D6E9}_{F}:=\{E\in M(\unicode[STIX]{x1D709})\mid \text{H}^{0}(E\otimes F)\neq 0\}$
.
Consider
 $E\in M(\unicode[STIX]{x1D709})^{\circ \unicode[STIX]{x1D70E}}$
fitting in a short exact sequence
$E\in M(\unicode[STIX]{x1D709})^{\circ \unicode[STIX]{x1D70E}}$
fitting in a short exact sequence
 $$\begin{eqnarray}0\rightarrow E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}\xrightarrow[{}]{(f,g)}E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}\oplus E_{-\unicode[STIX]{x1D6FE}}^{m_{1}}\rightarrow E\rightarrow 0,\end{eqnarray}$$
$$\begin{eqnarray}0\rightarrow E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}\xrightarrow[{}]{(f,g)}E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}\oplus E_{-\unicode[STIX]{x1D6FE}}^{m_{1}}\rightarrow E\rightarrow 0,\end{eqnarray}$$
and
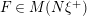 $F\in M(N\unicode[STIX]{x1D709}^{+})$
fitting in a short exact sequence
$F\in M(N\unicode[STIX]{x1D709}^{+})$
fitting in a short exact sequence
 $$\begin{eqnarray}0\rightarrow E_{\unicode[STIX]{x1D6FD}-2}^{m}\xrightarrow[{}]{h}E_{\unicode[STIX]{x1D6FC}}^{n}\rightarrow F\rightarrow 0,\end{eqnarray}$$
$$\begin{eqnarray}0\rightarrow E_{\unicode[STIX]{x1D6FD}-2}^{m}\xrightarrow[{}]{h}E_{\unicode[STIX]{x1D6FC}}^{n}\rightarrow F\rightarrow 0,\end{eqnarray}$$
where
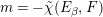 $m=-\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FD}},F)$
and
$m=-\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FD}},F)$
and
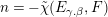 $n=-\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},F)$
. Define two-term complexes
$n=-\tilde{\unicode[STIX]{x1D712}}(E_{\unicode[STIX]{x1D6FE}.\unicode[STIX]{x1D6FD}},F)$
. Define two-term complexes
![]() $C_{1}^{\bullet }$
,
$C_{1}^{\bullet }$
,
![]() $C_{2}^{\bullet }$
and
$C_{2}^{\bullet }$
and
![]() $D^{\bullet }$
with terms in degree
$D^{\bullet }$
with terms in degree
![]() $-1$
,
$-1$
,
![]() $0$
by
$0$
by
 $$\begin{eqnarray}\displaystyle & & \displaystyle C_{1}^{\bullet }=[E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}\xrightarrow[{}]{(f,g)}E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}\oplus E_{-\unicode[STIX]{x1D6FE}}^{m_{1}}],\quad C_{2}^{\bullet }=[E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}\xrightarrow[{}]{f}E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}],\nonumber\\ \displaystyle & & \displaystyle D^{\bullet }=[E_{\unicode[STIX]{x1D6FD}-2}^{m}\xrightarrow[{}]{h}E_{\unicode[STIX]{x1D6FC}}^{n}].\nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle & & \displaystyle C_{1}^{\bullet }=[E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}\xrightarrow[{}]{(f,g)}E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}\oplus E_{-\unicode[STIX]{x1D6FE}}^{m_{1}}],\quad C_{2}^{\bullet }=[E_{-\unicode[STIX]{x1D6FC}-2}^{m_{3}}\xrightarrow[{}]{f}E_{-\unicode[STIX]{x1D6FD}}^{m_{2}}],\nonumber\\ \displaystyle & & \displaystyle D^{\bullet }=[E_{\unicode[STIX]{x1D6FD}-2}^{m}\xrightarrow[{}]{h}E_{\unicode[STIX]{x1D6FC}}^{n}].\nonumber\end{eqnarray}$$
We have
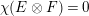 $\unicode[STIX]{x1D712}(E\otimes F)=0$
and
$\unicode[STIX]{x1D712}(E\otimes F)=0$
and
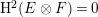 $\text{H}^{2}(E\otimes F)=0$
. Moreover, we have
$\text{H}^{2}(E\otimes F)=0$
. Moreover, we have
 $$\begin{eqnarray}\displaystyle \text{H}^{1}(E\otimes F) & \simeq & \displaystyle \text{H}^{1}(C_{1}^{\bullet }\otimes F)\nonumber\\ \displaystyle & \simeq & \displaystyle \text{H}^{1}(C_{2}^{\bullet }\otimes F)\nonumber\\ \displaystyle & \simeq & \displaystyle \text{Hom}_{\text{D}(S)}(D^{\bullet \vee },C_{2}^{\bullet }[1])\nonumber\\ \displaystyle & \simeq & \displaystyle \text{Hom}_{\text{D}(S)}(C_{2}^{\bullet },D^{\bullet \vee }\otimes K_{S}[1])^{\ast },\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \text{H}^{1}(E\otimes F) & \simeq & \displaystyle \text{H}^{1}(C_{1}^{\bullet }\otimes F)\nonumber\\ \displaystyle & \simeq & \displaystyle \text{H}^{1}(C_{2}^{\bullet }\otimes F)\nonumber\\ \displaystyle & \simeq & \displaystyle \text{Hom}_{\text{D}(S)}(D^{\bullet \vee },C_{2}^{\bullet }[1])\nonumber\\ \displaystyle & \simeq & \displaystyle \text{Hom}_{\text{D}(S)}(C_{2}^{\bullet },D^{\bullet \vee }\otimes K_{S}[1])^{\ast },\end{eqnarray}$$
where the second isomorphism follows from
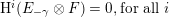 $\text{H}^{i}(E_{-\unicode[STIX]{x1D6FE}}\otimes F)=0,\text{for all }i$
, and the last isomorphism from the duality.
$\text{H}^{i}(E_{-\unicode[STIX]{x1D6FE}}\otimes F)=0,\text{for all }i$
, and the last isomorphism from the duality.
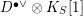 $D^{\bullet \vee }\otimes K_{S}[1]$
is the complex
$D^{\bullet \vee }\otimes K_{S}[1]$
is the complex
 $$\begin{eqnarray}E_{-\unicode[STIX]{x1D6FC}-2}^{n}\xrightarrow[{}]{h^{\prime }}E_{-\unicode[STIX]{x1D6FD}}^{m}\end{eqnarray}$$
$$\begin{eqnarray}E_{-\unicode[STIX]{x1D6FC}-2}^{n}\xrightarrow[{}]{h^{\prime }}E_{-\unicode[STIX]{x1D6FD}}^{m}\end{eqnarray}$$
with terms in degree
![]() $-1,0$
, where
$-1,0$
, where
![]() $h^{\prime }$
is the morphism induced by
$h^{\prime }$
is the morphism induced by
![]() $h$
. The complex
$h$
. The complex
![]() $C_{2}^{\bullet }$
corresponds to the representation
$C_{2}^{\bullet }$
corresponds to the representation
![]() $\unicode[STIX]{x1D70B}(E)$
of the quiver
$\unicode[STIX]{x1D70B}(E)$
of the quiver
![]() $R^{\star }$
, we let
$R^{\star }$
, we let
![]() $\unicode[STIX]{x1D70F}(F)$
be the representation of
$\unicode[STIX]{x1D70F}(F)$
be the representation of
![]() $R^{\star }$
corresponding to the complex (10.4). Using [Reference Derksen and WeymanDW, Theorem 1], we can see that for some
$R^{\star }$
corresponding to the complex (10.4). Using [Reference Derksen and WeymanDW, Theorem 1], we can see that for some
![]() $N>0$
and general
$N>0$
and general
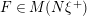 $F\in M(N\unicode[STIX]{x1D709}^{+})$
, we have
$F\in M(N\unicode[STIX]{x1D709}^{+})$
, we have
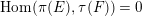 $\text{Hom}(\unicode[STIX]{x1D70B}(E),\unicode[STIX]{x1D70F}(F))=0$
, hence
$\text{Hom}(\unicode[STIX]{x1D70B}(E),\unicode[STIX]{x1D70F}(F))=0$
, hence
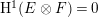 $\text{H}^{1}(E\otimes F)=0$
.
$\text{H}^{1}(E\otimes F)=0$
.
By Proposition 9.2, we have a moving complete curve
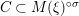 $C\subset M(\unicode[STIX]{x1D709})^{\circ \unicode[STIX]{x1D70E}}$
. From (10.3), it follows that
$C\subset M(\unicode[STIX]{x1D709})^{\circ \unicode[STIX]{x1D70E}}$
. From (10.3), it follows that
 $C\subset \unicode[STIX]{x1D6E9}_{F}$
or
$C\subset \unicode[STIX]{x1D6E9}_{F}$
or
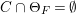 $C\cap \unicode[STIX]{x1D6E9}_{F}=\emptyset$
because
$C\cap \unicode[STIX]{x1D6E9}_{F}=\emptyset$
because
![]() $\unicode[STIX]{x1D70B}(C)$
is a point. Then the intersection number
$\unicode[STIX]{x1D70B}(C)$
is a point. Then the intersection number
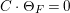 $C\cdot \unicode[STIX]{x1D6E9}_{F}=0$
. This implies that the ray
$C\cdot \unicode[STIX]{x1D6E9}_{F}=0$
. This implies that the ray
 $\mathbb{R}_{{\geqslant}0}\unicode[STIX]{x1D706}(-\unicode[STIX]{x1D709}^{+})$
is an edge of
$\mathbb{R}_{{\geqslant}0}\unicode[STIX]{x1D706}(-\unicode[STIX]{x1D709}^{+})$
is an edge of
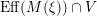 $\text{Eff}(M(\unicode[STIX]{x1D709}))\cap V$
.
$\text{Eff}(M(\unicode[STIX]{x1D709}))\cap V$
.
Case
![]() $(2)$
. In this case we have
$(2)$
. In this case we have
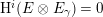 $\text{H}^{i}(E\otimes E_{\unicode[STIX]{x1D6FE}})=0$
, for all
$\text{H}^{i}(E\otimes E_{\unicode[STIX]{x1D6FE}})=0$
, for all
![]() $i$
, for any
$i$
, for any
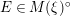 $E\in M(\unicode[STIX]{x1D709})^{\circ }$
. The effective divisor
$E\in M(\unicode[STIX]{x1D709})^{\circ }$
. The effective divisor
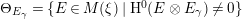 $\unicode[STIX]{x1D6E9}_{E_{\unicode[STIX]{x1D6FE}}}=\{E\in M(\unicode[STIX]{x1D709})\mid \text{H}^{0}(E\otimes E_{\unicode[STIX]{x1D6FE}})\neq 0\}$
has the class
$\unicode[STIX]{x1D6E9}_{E_{\unicode[STIX]{x1D6FE}}}=\{E\in M(\unicode[STIX]{x1D709})\mid \text{H}^{0}(E\otimes E_{\unicode[STIX]{x1D6FE}})\neq 0\}$
has the class
![]() $\unicode[STIX]{x1D706}(-\unicode[STIX]{x1D709}^{+})$
. Using Proposition 9.3 instead of Proposition 9.2, arguing as in Case (1), we can see that the ray
$\unicode[STIX]{x1D706}(-\unicode[STIX]{x1D709}^{+})$
. Using Proposition 9.3 instead of Proposition 9.2, arguing as in Case (1), we can see that the ray
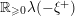 $\mathbb{R}_{{\geqslant}0}\unicode[STIX]{x1D706}(-\unicode[STIX]{x1D709}^{+})$
is an edge of
$\mathbb{R}_{{\geqslant}0}\unicode[STIX]{x1D706}(-\unicode[STIX]{x1D709}^{+})$
is an edge of
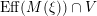 $\text{Eff}(M(\unicode[STIX]{x1D709}))\cap V$
.
$\text{Eff}(M(\unicode[STIX]{x1D709}))\cap V$
.
Case
![]() $(3)$
. We can argue as in Case (1).
$(3)$
. We can argue as in Case (1).
(b) In the case
 $\text{r}(\unicode[STIX]{x1D709})=1$
(resp.
$\text{r}(\unicode[STIX]{x1D709})=1$
(resp.
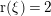 $\text{r}(\unicode[STIX]{x1D709})=2$
), we can see, by calculation, that the ray
$\text{r}(\unicode[STIX]{x1D709})=2$
), we can see, by calculation, that the ray
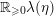 $\mathbb{R}_{{\geqslant}0}\unicode[STIX]{x1D706}(\unicode[STIX]{x1D702})$
is spanned by the exceptional divisor of the Hilbert–Chow morphism (resp. the morphism to the Donaldson–Uhlenbeck compactification). So it is an edge of
$\mathbb{R}_{{\geqslant}0}\unicode[STIX]{x1D706}(\unicode[STIX]{x1D702})$
is spanned by the exceptional divisor of the Hilbert–Chow morphism (resp. the morphism to the Donaldson–Uhlenbeck compactification). So it is an edge of
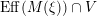 $\text{Eff}(M(\unicode[STIX]{x1D709}))\cap V$
.
$\text{Eff}(M(\unicode[STIX]{x1D709}))\cap V$
.
Consider the case
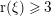 $\text{r}(\unicode[STIX]{x1D709})\geqslant 3$
. By (a), for general
$\text{r}(\unicode[STIX]{x1D709})\geqslant 3$
. By (a), for general
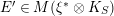 $E^{\prime }\in M(\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})$
and
$E^{\prime }\in M(\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})$
and
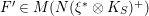 $F^{\prime }\in M(N(\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{+})$
for some
$F^{\prime }\in M(N(\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{+})$
for some
![]() $N>0$
, we have
$N>0$
, we have
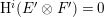 $\text{H}^{i}(E^{\prime }\otimes F^{\prime })=0$
for all
$\text{H}^{i}(E^{\prime }\otimes F^{\prime })=0$
for all
![]() $i$
. This implies, by considering the dual, that for general
$i$
. This implies, by considering the dual, that for general
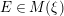 $E\in M(\unicode[STIX]{x1D709})$
and
$E\in M(\unicode[STIX]{x1D709})$
and
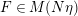 $F\in M(N\unicode[STIX]{x1D702})$
we have
$F\in M(N\unicode[STIX]{x1D702})$
we have
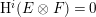 $\text{H}^{i}(E\otimes F)=0$
for all
$\text{H}^{i}(E\otimes F)=0$
for all
![]() $i$
. Then
$i$
. Then
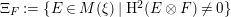 $\unicode[STIX]{x1D6EF}_{F}:=\{E\in M(\unicode[STIX]{x1D709})\mid \text{H}^{2}(E\otimes F)\neq 0\}$
is an effective divisor with the class
$\unicode[STIX]{x1D6EF}_{F}:=\{E\in M(\unicode[STIX]{x1D709})\mid \text{H}^{2}(E\otimes F)\neq 0\}$
is an effective divisor with the class
![]() $\unicode[STIX]{x1D706}(N\unicode[STIX]{x1D702})$
. Let us show that the ray
$\unicode[STIX]{x1D706}(N\unicode[STIX]{x1D702})$
. Let us show that the ray
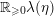 $\mathbb{R}_{{\geqslant}0}\unicode[STIX]{x1D706}(\unicode[STIX]{x1D702})$
is an edge of
$\mathbb{R}_{{\geqslant}0}\unicode[STIX]{x1D706}(\unicode[STIX]{x1D702})$
is an edge of
 $\text{Eff}(M(\unicode[STIX]{x1D709}))\cap V$
. Let
$\text{Eff}(M(\unicode[STIX]{x1D709}))\cap V$
. Let
![]() $M(\unicode[STIX]{x1D709})^{\unicode[STIX]{x1D707}s}$
and
$M(\unicode[STIX]{x1D709})^{\unicode[STIX]{x1D707}s}$
and
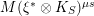 $M(\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{\unicode[STIX]{x1D707}s}$
be the open subschemes of
$M(\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{\unicode[STIX]{x1D707}s}$
be the open subschemes of
![]() $M(\unicode[STIX]{x1D709})$
and
$M(\unicode[STIX]{x1D709})$
and
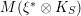 $M(\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})$
, respectively consisting of
$M(\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})$
, respectively consisting of
![]() $\unicode[STIX]{x1D707}$
-stable bundles. We have an isomorphism
$\unicode[STIX]{x1D707}$
-stable bundles. We have an isomorphism
 $$\begin{eqnarray}f:M(\unicode[STIX]{x1D709})^{\unicode[STIX]{x1D707}s}\rightarrow M(\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{\unicode[STIX]{x1D707}s}\end{eqnarray}$$
$$\begin{eqnarray}f:M(\unicode[STIX]{x1D709})^{\unicode[STIX]{x1D707}s}\rightarrow M(\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{\unicode[STIX]{x1D707}s}\end{eqnarray}$$
by
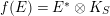 $f(E)=E^{\ast }\otimes K_{S}$
. By Remark 9.4, we can find a moving complete curve
$f(E)=E^{\ast }\otimes K_{S}$
. By Remark 9.4, we can find a moving complete curve
![]() $C$
in
$C$
in
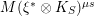 $M(\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{\unicode[STIX]{x1D707}s}$
that is orthogonal to the class
$M(\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{\unicode[STIX]{x1D707}s}$
that is orthogonal to the class
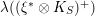 $\unicode[STIX]{x1D706}((\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{+})$
. Then the moving curve
$\unicode[STIX]{x1D706}((\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{+})$
. Then the moving curve
![]() $f^{-1}(C)$
is orthogonal to
$f^{-1}(C)$
is orthogonal to
![]() $\unicode[STIX]{x1D706}(\unicode[STIX]{x1D702})$
. So the ray
$\unicode[STIX]{x1D706}(\unicode[STIX]{x1D702})$
. So the ray
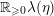 $\mathbb{R}_{{\geqslant}0}\unicode[STIX]{x1D706}(\unicode[STIX]{x1D702})$
is extremal. ◻
$\mathbb{R}_{{\geqslant}0}\unicode[STIX]{x1D706}(\unicode[STIX]{x1D702})$
is extremal. ◻
Proof of Lemma 10.1 in the case
 $\text{r}(\unicode[STIX]{x1D709})\geqslant 3$
.
$\text{r}(\unicode[STIX]{x1D709})\geqslant 3$
.
We first claim that
![]() $\unicode[STIX]{x1D709}^{+}$
and
$\unicode[STIX]{x1D709}^{+}$
and
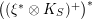 $((\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{+})^{\ast }$
are linearly independent in
$((\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{+})^{\ast }$
are linearly independent in
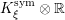 $K_{\unicode[STIX]{x1D709}}^{\text{sym}}\otimes \mathbb{R}$
. Indeed, by definition, we have
$K_{\unicode[STIX]{x1D709}}^{\text{sym}}\otimes \mathbb{R}$
. Indeed, by definition, we have
 $$\begin{eqnarray}\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}^{+})>-1-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})\qquad \text{and}\qquad \bar{\unicode[STIX]{x1D707}}\left((\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{+}\right)>-1-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}^{\ast }\otimes K_{S}).\end{eqnarray}$$
$$\begin{eqnarray}\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}^{+})>-1-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})\qquad \text{and}\qquad \bar{\unicode[STIX]{x1D707}}\left((\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{+}\right)>-1-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}^{\ast }\otimes K_{S}).\end{eqnarray}$$
From this, we have
 $$\begin{eqnarray}\bar{\unicode[STIX]{x1D707}}(((\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{+})^{\ast })<-1-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})<\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}^{+}).\end{eqnarray}$$
$$\begin{eqnarray}\bar{\unicode[STIX]{x1D707}}(((\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{+})^{\ast })<-1-\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709})<\bar{\unicode[STIX]{x1D707}}(\unicode[STIX]{x1D709}^{+}).\end{eqnarray}$$
So
![]() $\unicode[STIX]{x1D709}^{+}$
and
$\unicode[STIX]{x1D709}^{+}$
and
 $((\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{+})^{\ast }$
are linearly independent.
$((\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{+})^{\ast }$
are linearly independent.
Now suppose that
![]() $\unicode[STIX]{x1D706}$
is not injective. Then
$\unicode[STIX]{x1D706}$
is not injective. Then
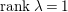 $\text{rank}\,\unicode[STIX]{x1D706}=1$
because
$\text{rank}\,\unicode[STIX]{x1D706}=1$
because
![]() $\text{Im}\,\unicode[STIX]{x1D706}$
contains an ample class. For
$\text{Im}\,\unicode[STIX]{x1D706}$
contains an ample class. For
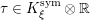 $\unicode[STIX]{x1D70F}\in K_{\unicode[STIX]{x1D709}}^{\text{sym}}\otimes \mathbb{R}$
,
$\unicode[STIX]{x1D70F}\in K_{\unicode[STIX]{x1D709}}^{\text{sym}}\otimes \mathbb{R}$
,
![]() $\unicode[STIX]{x1D706}(\unicode[STIX]{x1D70F})$
is proportional to an ample class. So, if there exists a complete curve
$\unicode[STIX]{x1D706}(\unicode[STIX]{x1D70F})$
is proportional to an ample class. So, if there exists a complete curve
 $C\subset M(\unicode[STIX]{x1D709})$
such that the intersection number
$C\subset M(\unicode[STIX]{x1D709})$
such that the intersection number
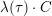 $\unicode[STIX]{x1D706}(\unicode[STIX]{x1D70F})\cdot C$
is zero, then
$\unicode[STIX]{x1D706}(\unicode[STIX]{x1D70F})\cdot C$
is zero, then
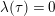 $\unicode[STIX]{x1D706}(\unicode[STIX]{x1D70F})=0$
. In the proof of Theorem 10.2, we showed that for
$\unicode[STIX]{x1D706}(\unicode[STIX]{x1D70F})=0$
. In the proof of Theorem 10.2, we showed that for
![]() $\unicode[STIX]{x1D709}^{+}$
and
$\unicode[STIX]{x1D709}^{+}$
and
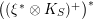 $((\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{+})^{\ast }$
, such a curve exists. So
$((\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{+})^{\ast }$
, such a curve exists. So
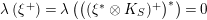 $\unicode[STIX]{x1D706}\left(\unicode[STIX]{x1D709}^{+}\right)=\unicode[STIX]{x1D706}\left(((\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{+})^{\ast }\right)=0$
. But then, we have
$\unicode[STIX]{x1D706}\left(\unicode[STIX]{x1D709}^{+}\right)=\unicode[STIX]{x1D706}\left(((\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{+})^{\ast }\right)=0$
. But then, we have
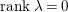 $\text{rank}\,\unicode[STIX]{x1D706}=0$
because
$\text{rank}\,\unicode[STIX]{x1D706}=0$
because
![]() $\unicode[STIX]{x1D709}^{+}$
and
$\unicode[STIX]{x1D709}^{+}$
and
 $((\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{+})^{\ast }$
span
$((\unicode[STIX]{x1D709}^{\ast }\otimes K_{S})^{+})^{\ast }$
span
![]() $K_{\unicode[STIX]{x1D709}}^{\text{sym}}$
. This is a contradiction.◻
$K_{\unicode[STIX]{x1D709}}^{\text{sym}}$
. This is a contradiction.◻
Remark 10.3. I mention related works. The effective and ample cones of the Hilbert scheme of Del Pezzo surfaces are discussed in [Reference Bertram and CoskunBC]. Ryan [Reference RyanRy] studies the effective cone of the moduli space of sheaves on a quadric surface without the assumption
![]() $c_{1}$
symmetric. The ample and movable cone of the moduli space of sheaves on a surface is also studied by several authors [Reference Bayer and MacrBM], [Reference Coskun and HuizengaCH1], [Reference Coskun and HuizengaCH2], [Reference Li and ZhaoLZ], [Reference YoshiokaY].
$c_{1}$
symmetric. The ample and movable cone of the moduli space of sheaves on a surface is also studied by several authors [Reference Bayer and MacrBM], [Reference Coskun and HuizengaCH1], [Reference Coskun and HuizengaCH2], [Reference Li and ZhaoLZ], [Reference YoshiokaY].
11 Strange duality
Let
 $\unicode[STIX]{x1D709},\unicode[STIX]{x1D709}^{\prime }\in \text{K}(S)$
be semistable class with symmetric
$\unicode[STIX]{x1D709},\unicode[STIX]{x1D709}^{\prime }\in \text{K}(S)$
be semistable class with symmetric
![]() $c_{1}$
. Assume that
$c_{1}$
. Assume that
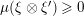 $\unicode[STIX]{x1D707}(\unicode[STIX]{x1D709}\otimes \unicode[STIX]{x1D709}^{\prime })\geqslant 0$
and
$\unicode[STIX]{x1D707}(\unicode[STIX]{x1D709}\otimes \unicode[STIX]{x1D709}^{\prime })\geqslant 0$
and
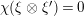 $\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709}\otimes \unicode[STIX]{x1D709}^{\prime })=0$
. Define a subscheme
$\unicode[STIX]{x1D712}(\unicode[STIX]{x1D709}\otimes \unicode[STIX]{x1D709}^{\prime })=0$
. Define a subscheme
![]() $\unicode[STIX]{x1D6E9}$
of
$\unicode[STIX]{x1D6E9}$
of
 $M(\unicode[STIX]{x1D709})\times M(\unicode[STIX]{x1D709}^{\prime })$
by
$M(\unicode[STIX]{x1D709})\times M(\unicode[STIX]{x1D709}^{\prime })$
by
 $$\begin{eqnarray}\unicode[STIX]{x1D6E9}=\left\{(F,F^{\prime })\in M(\unicode[STIX]{x1D709})\times M(\unicode[STIX]{x1D709}^{\prime })\left|\text{H}^{0}(S,F\otimes F^{\prime })\neq 0\right.\right\}\!.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D6E9}=\left\{(F,F^{\prime })\in M(\unicode[STIX]{x1D709})\times M(\unicode[STIX]{x1D709}^{\prime })\left|\text{H}^{0}(S,F\otimes F^{\prime })\neq 0\right.\right\}\!.\end{eqnarray}$$
Assume that
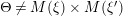 $\unicode[STIX]{x1D6E9}\neq M(\unicode[STIX]{x1D709})\times M(\unicode[STIX]{x1D709}^{\prime })$
. Then
$\unicode[STIX]{x1D6E9}\neq M(\unicode[STIX]{x1D709})\times M(\unicode[STIX]{x1D709}^{\prime })$
. Then
![]() $\unicode[STIX]{x1D6E9}$
is a Cartier divisor and we have
$\unicode[STIX]{x1D6E9}$
is a Cartier divisor and we have
 $$\begin{eqnarray}{\mathcal{O}}(\unicode[STIX]{x1D6E9})\simeq {\mathcal{D}}\boxtimes {\mathcal{D}}^{\prime },\end{eqnarray}$$
$$\begin{eqnarray}{\mathcal{O}}(\unicode[STIX]{x1D6E9})\simeq {\mathcal{D}}\boxtimes {\mathcal{D}}^{\prime },\end{eqnarray}$$
where
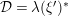 ${\mathcal{D}}=\unicode[STIX]{x1D706}(\unicode[STIX]{x1D709}^{\prime })^{\ast }$
and
${\mathcal{D}}=\unicode[STIX]{x1D706}(\unicode[STIX]{x1D709}^{\prime })^{\ast }$
and
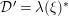 ${\mathcal{D}}^{\prime }=\unicode[STIX]{x1D706}(\unicode[STIX]{x1D709})^{\ast }$
. The section defining
${\mathcal{D}}^{\prime }=\unicode[STIX]{x1D706}(\unicode[STIX]{x1D709})^{\ast }$
. The section defining
![]() $\unicode[STIX]{x1D6E9}$
induces a linear map
$\unicode[STIX]{x1D6E9}$
induces a linear map
 $$\begin{eqnarray}\text{H}^{0}(M(\unicode[STIX]{x1D709}),{\mathcal{D}})^{\ast }\rightarrow \text{H}^{0}(M(\unicode[STIX]{x1D709}^{\prime }),{\mathcal{D}}^{\prime }).\end{eqnarray}$$
$$\begin{eqnarray}\text{H}^{0}(M(\unicode[STIX]{x1D709}),{\mathcal{D}})^{\ast }\rightarrow \text{H}^{0}(M(\unicode[STIX]{x1D709}^{\prime }),{\mathcal{D}}^{\prime }).\end{eqnarray}$$
Theorem 11.1. Assume that either
![]() $M(\unicode[STIX]{x1D709})$
or
$M(\unicode[STIX]{x1D709})$
or
![]() $M(\unicode[STIX]{x1D709}^{\prime })$
is of height zero. Then the map (11.1) is an isomorphism.
$M(\unicode[STIX]{x1D709}^{\prime })$
is of height zero. Then the map (11.1) is an isomorphism.
Once we have Proposition 6.1, and a rational map to moduli spaces of quiver representations constructed in Section 9, the proof of the above theorem is quite parallel to that of [Reference AbeA15, Theorem 1.1]. So we omit the proof.
Appendix
From a full exceptional collection, one obtains a Beilinson-type spectral sequence (cf. [Reference Gorodentsev and KuleshovGK, Section 4.5]). For readers’ convenience, we present a spectral sequence obtained from a full
![]() $d$
-block exceptional collection.
$d$
-block exceptional collection.
We let
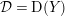 ${\mathcal{D}}=\text{D}(Y)$
for a smooth projective variety
${\mathcal{D}}=\text{D}(Y)$
for a smooth projective variety
![]() $Y$
. Let
$Y$
. Let
 $$\begin{eqnarray}\mathbb{E}=(\mathbb{E}^{(1)},\ldots ,\mathbb{E}^{(d)})\end{eqnarray}$$
$$\begin{eqnarray}\mathbb{E}=(\mathbb{E}^{(1)},\ldots ,\mathbb{E}^{(d)})\end{eqnarray}$$
be a full
![]() $d$
-block exceptional collection, where
$d$
-block exceptional collection, where
 $\mathbb{E}^{(k)}=(E_{1}^{(k)},\ldots ,E_{N_{k}}^{(k)})$
. We define a block
$\mathbb{E}^{(k)}=(E_{1}^{(k)},\ldots ,E_{N_{k}}^{(k)})$
. We define a block
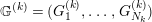 $\mathbb{G}^{(k)}=(G_{1}^{(k)},\ldots ,G_{N_{k}}^{(k)})$
of exceptional objects by
$\mathbb{G}^{(k)}=(G_{1}^{(k)},\ldots ,G_{N_{k}}^{(k)})$
of exceptional objects by
 $$\begin{eqnarray}\mathbb{G}^{(k)}=R_{\mathbb{ E}^{(d)}}\ldots R_{\mathbb{E}^{(k+1)}}(\mathbb{E}^{(k)}).\end{eqnarray}$$
$$\begin{eqnarray}\mathbb{G}^{(k)}=R_{\mathbb{ E}^{(d)}}\ldots R_{\mathbb{E}^{(k+1)}}(\mathbb{E}^{(k)}).\end{eqnarray}$$
Then we have
 $$\begin{eqnarray}\text{Hom}^{m}(G_{i}^{(k)},E_{j}^{(l)})=\left\{\begin{array}{@{}ll@{}}\mathbb{C}\quad & (k,i)=(l,j)\text{ and }m=0,\\ 0\quad & \text{otherwise}.\end{array}\right.\end{eqnarray}$$
$$\begin{eqnarray}\text{Hom}^{m}(G_{i}^{(k)},E_{j}^{(l)})=\left\{\begin{array}{@{}ll@{}}\mathbb{C}\quad & (k,i)=(l,j)\text{ and }m=0,\\ 0\quad & \text{otherwise}.\end{array}\right.\end{eqnarray}$$
Let
![]() $F\in {\mathcal{D}}$
and put
$F\in {\mathcal{D}}$
and put
 $F_{1}:=F$
. Since the pair
$F_{1}:=F$
. Since the pair
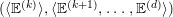 $(\langle \mathbb{E}^{(k)}\rangle ,\langle \mathbb{E}^{(k+1)},\ldots ,\mathbb{E}^{(d)}\rangle )$
is a semi-orthogonal decomposition of
$(\langle \mathbb{E}^{(k)}\rangle ,\langle \mathbb{E}^{(k+1)},\ldots ,\mathbb{E}^{(d)}\rangle )$
is a semi-orthogonal decomposition of
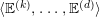 $\langle \mathbb{E}^{(k)},\ldots ,\mathbb{E}^{(d)}\rangle$
, we can define
$\langle \mathbb{E}^{(k)},\ldots ,\mathbb{E}^{(d)}\rangle$
, we can define
![]() $F_{k}$
(
$F_{k}$
(
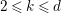 $2\leqslant k\leqslant d$
), and
$2\leqslant k\leqslant d$
), and
![]() $C_{k}$
(
$C_{k}$
(
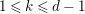 $1\leqslant k\leqslant d-1$
) inductively by a triangle
$1\leqslant k\leqslant d-1$
) inductively by a triangle
 $$\begin{eqnarray}F_{k+1}\rightarrow F_{k}\rightarrow C_{k}\rightarrow F_{k+1}[1],\end{eqnarray}$$
$$\begin{eqnarray}F_{k+1}\rightarrow F_{k}\rightarrow C_{k}\rightarrow F_{k+1}[1],\end{eqnarray}$$
where
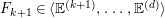 $F_{k+1}\in \langle \mathbb{E}^{(k+1)},\ldots ,\mathbb{E}^{(d)}\rangle$
and
$F_{k+1}\in \langle \mathbb{E}^{(k+1)},\ldots ,\mathbb{E}^{(d)}\rangle$
and
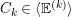 $C_{k}\in \langle \mathbb{E}^{(k)}\rangle$
. Put
$C_{k}\in \langle \mathbb{E}^{(k)}\rangle$
. Put
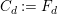 $C_{d}:=F_{d}$
. Using the triangle (A2), we can see that
$C_{d}:=F_{d}$
. Using the triangle (A2), we can see that
 $$\begin{eqnarray}C_{k}=\bigoplus _{1\leqslant \unicode[STIX]{x1D6FC}\leqslant N_{k},\;j\in \mathbb{Z}}\text{Ext}^{j}(G_{\unicode[STIX]{x1D6FC}}^{(k)},F)\otimes E_{\unicode[STIX]{x1D6FC}}^{(k)}[-j].\end{eqnarray}$$
$$\begin{eqnarray}C_{k}=\bigoplus _{1\leqslant \unicode[STIX]{x1D6FC}\leqslant N_{k},\;j\in \mathbb{Z}}\text{Ext}^{j}(G_{\unicode[STIX]{x1D6FC}}^{(k)},F)\otimes E_{\unicode[STIX]{x1D6FC}}^{(k)}[-j].\end{eqnarray}$$
From the triangle (A2), we see that
![]() $F_{k}$
is quasi-isomorphic to the mapping cone of
$F_{k}$
is quasi-isomorphic to the mapping cone of
 $C_{k}[-1]\rightarrow F_{k+1}$
. By considering inductively, we can see that
$C_{k}[-1]\rightarrow F_{k+1}$
. By considering inductively, we can see that
![]() $F$
is quasi-isomorphic to a complex
$F$
is quasi-isomorphic to a complex
![]() $A^{\bullet }$
with filtration
$A^{\bullet }$
with filtration
 $$\begin{eqnarray}A^{\bullet }=A_{1}^{\bullet }\supset \cdots \supset A_{d}^{\bullet }\supset A_{d+1}^{\bullet }=0\end{eqnarray}$$
$$\begin{eqnarray}A^{\bullet }=A_{1}^{\bullet }\supset \cdots \supset A_{d}^{\bullet }\supset A_{d+1}^{\bullet }=0\end{eqnarray}$$
such that the graded complex
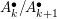 $A_{k}^{\bullet }/A_{k+1}^{\bullet }$
is quasi-isomorphic to
$A_{k}^{\bullet }/A_{k+1}^{\bullet }$
is quasi-isomorphic to
![]() $C_{k}$
. So we have a spectral sequence
$C_{k}$
. So we have a spectral sequence
 $$\begin{eqnarray}E_{1}^{p,q}=\bigoplus _{1\leqslant \unicode[STIX]{x1D6FC}\leqslant N_{p},\;j\in \mathbb{Z}}\text{Ext}^{j}(G_{\unicode[STIX]{x1D6FC}}^{(p)},F)\otimes \text{H}^{p+q-j}(E_{\unicode[STIX]{x1D6FC}}^{(p)})\end{eqnarray}$$
$$\begin{eqnarray}E_{1}^{p,q}=\bigoplus _{1\leqslant \unicode[STIX]{x1D6FC}\leqslant N_{p},\;j\in \mathbb{Z}}\text{Ext}^{j}(G_{\unicode[STIX]{x1D6FC}}^{(p)},F)\otimes \text{H}^{p+q-j}(E_{\unicode[STIX]{x1D6FC}}^{(p)})\end{eqnarray}$$
converging to
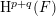 $\text{H}^{p+q}(F)$
.
$\text{H}^{p+q}(F)$
.
Now suppose in addition that
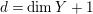 $d=\dim Y+1$
and all
$d=\dim Y+1$
and all
![]() $E_{i}^{(k)}$
are exceptional sheaves. Put
$E_{i}^{(k)}$
are exceptional sheaves. Put
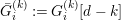 $\bar{G}_{i}^{(k)}:=G_{i}^{(k)}[d-k]$
. Then
$\bar{G}_{i}^{(k)}:=G_{i}^{(k)}[d-k]$
. Then
![]() $\bar{G}_{i}^{(k)}$
are sheaves by [Reference Bridgeland and SternBS, Theorem 4.5 and Lemma 5.2]. We have a spectral sequence
$\bar{G}_{i}^{(k)}$
are sheaves by [Reference Bridgeland and SternBS, Theorem 4.5 and Lemma 5.2]. We have a spectral sequence
 $$\begin{eqnarray}E_{1}^{p,q}=\bigoplus _{1\leqslant \unicode[STIX]{x1D6FC}\leqslant N_{p}}\text{Ext}^{q}(\bar{G}_{\unicode[STIX]{x1D6FC}}^{(p+d)},F)\otimes E_{\unicode[STIX]{x1D6FC}}^{(p+d)}\end{eqnarray}$$
$$\begin{eqnarray}E_{1}^{p,q}=\bigoplus _{1\leqslant \unicode[STIX]{x1D6FC}\leqslant N_{p}}\text{Ext}^{q}(\bar{G}_{\unicode[STIX]{x1D6FC}}^{(p+d)},F)\otimes E_{\unicode[STIX]{x1D6FC}}^{(p+d)}\end{eqnarray}$$
converging to
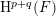 $\text{H}^{p+q}(F)$
.
$\text{H}^{p+q}(F)$
.
We apply the above spectral sequence to symmetric exceptional triples. Let
 ${\mathcal{E}}=(E^{(1)},E^{(2)},E^{(3)})$
be a symmetric exceptional triple on
${\mathcal{E}}=(E^{(1)},E^{(2)},E^{(3)})$
be a symmetric exceptional triple on
![]() $S$
. Put
$S$
. Put
 $(G^{(3)},G^{(2)},G^{(1)}):=\unicode[STIX]{x1D70F}_{1}^{R}\unicode[STIX]{x1D70F}_{2}^{R}\unicode[STIX]{x1D70F}_{1}^{R}({\mathcal{E}})$
. Note the parity of
$(G^{(3)},G^{(2)},G^{(1)}):=\unicode[STIX]{x1D70F}_{1}^{R}\unicode[STIX]{x1D70F}_{2}^{R}\unicode[STIX]{x1D70F}_{1}^{R}({\mathcal{E}})$
. Note the parity of
![]() $E^{(k)}$
and
$E^{(k)}$
and
![]() $G^{(k)}$
is the same. Then for
$G^{(k)}$
is the same. Then for
![]() $F\in {\mathcal{D}}$
, we have a spectral sequence
$F\in {\mathcal{D}}$
, we have a spectral sequence
 $$\begin{eqnarray}E_{1}^{p,q}=\text{Ext}^{q}(G^{(p+3)},F)\hat{\otimes }E^{(p+3)}\end{eqnarray}$$
$$\begin{eqnarray}E_{1}^{p,q}=\text{Ext}^{q}(G^{(p+3)},F)\hat{\otimes }E^{(p+3)}\end{eqnarray}$$
converging to
 $\text{H}^{p+q}(F)$
, where
$\text{H}^{p+q}(F)$
, where
 $$\begin{eqnarray}\text{Ext}^{q}(G^{(k)},F)\hat{\otimes }E^{(k)}=\left\{\begin{array}{@{}ll@{}}\text{Ext}^{q}(G^{(k)},F)\otimes E^{(k)}\quad & \hspace{-3.0pt}\text{if }G^{(k)}\text{ and }E^{(k)}\text{ are odd},\\ \begin{array}{@{}c@{}}\text{Ext}^{q}(G^{(k)\prime },F)\otimes E^{(k)\prime }\\ \oplus \\ \text{Ext}^{q}(G^{(k)\prime \prime },F)\otimes E^{(k)\prime \prime }\end{array}\quad & \hspace{-3.0pt}\begin{array}{@{}l@{}}\text{if }G^{(k)}=G^{(k)\prime }\oplus G^{(k)\prime \prime }\\ \text{and }E^{(k)}=E^{(k)\prime }\oplus E^{(k)\prime \prime }\\ \text{are even.}\end{array}\end{array}\right.\end{eqnarray}$$
$$\begin{eqnarray}\text{Ext}^{q}(G^{(k)},F)\hat{\otimes }E^{(k)}=\left\{\begin{array}{@{}ll@{}}\text{Ext}^{q}(G^{(k)},F)\otimes E^{(k)}\quad & \hspace{-3.0pt}\text{if }G^{(k)}\text{ and }E^{(k)}\text{ are odd},\\ \begin{array}{@{}c@{}}\text{Ext}^{q}(G^{(k)\prime },F)\otimes E^{(k)\prime }\\ \oplus \\ \text{Ext}^{q}(G^{(k)\prime \prime },F)\otimes E^{(k)\prime \prime }\end{array}\quad & \hspace{-3.0pt}\begin{array}{@{}l@{}}\text{if }G^{(k)}=G^{(k)\prime }\oplus G^{(k)\prime \prime }\\ \text{and }E^{(k)}=E^{(k)\prime }\oplus E^{(k)\prime \prime }\\ \text{are even.}\end{array}\end{array}\right.\end{eqnarray}$$