1 Introduction
Liquids evaporate far below their boiling point if the atmosphere is not saturated with their vapour. In this case, the rate of evaporation is limited by the transport of vapour through the gas phase, while liquid–vapour equilibrium is maintained at the droplet surface. A sessile droplet of a pure liquid evaporating into quiescent air is a prototype of great importance. Its idealized continuum treatment exhibits a diverging evaporation rate at the contact line, and many studies have been devoted to this problem. The literature has been reviewed e.g. by Cazabat & Guéna (Reference Cazabat and Guéna2010), Brutin (Reference Brutin2015).
Most common, however, is the case where droplets consist of more than a single pure liquid. In daily life or technological applications, multiple components are required for their specific functionalities. Alcoholic beverages, for instance, contain at least three ingredients: water, alcohol and a flavour. In general, different liquids evaporate at different rates, leading to an enrichment of the residual component near the contact line. Evaporating droplets of Ouzo, a popular Greek liquor, are a particularly visual example: Anise oil precipitates and the liquid turns pale in alcohol-depleted regions, starting near the contact line (Tan et al.
Reference Tan, Diddens, Lv, Kuerten, Zhang and Lohse2016). Enrichment of residuals can also cause Marangoni convection (Pesach & Marmur Reference Pesach and Marmur1987; Guéna, Poulard & Cazabat Reference Guéna, Poulard and Cazabat2007) which may influence the pattern left behind by a drying drop. Utilizing this to control the deposit pattern (Kim et al.
Reference Kim, Boulogne, Um, Jacobi, Button and Stone2016) is relevant e.g. in ink-jet printing. Precipitates of evaporating salt water droplets are important in stainless steel corrosion (Soulie et al.
Reference Soulie, Karpitschka, Lequien, Prené, Zemb, Möhwald and Riegler2015), and drying drops of blood form manifold patterns with potential use in disease diagnostics (Brutin et al.
Reference Brutin, Sobac, Loquet and Sampol2011). But even for the seemingly simple case of a pure water droplet, theory predicts strong thermal Marangoni flows that are absent in experiments. This discrepancy has been attributed to trace impurities (Hu & Larson Reference Hu and Larson2005; Marin et al.
Reference Marin, Liepelt, Rossi and Khler2016), clearly indicating the fundamental importance of multi-component droplet evaporation.
One of the major challenges in the field is determining the spatio-temporal compositional evolution of the droplet. Most previous studies resolved it indirectly from numerical models that were calibrated against accessible observables (Diddens et al.
Reference Diddens, Tan, Lv, Versluis, Kuerten, Zhang and Lohse2017; Karpitschka, Liebig & Riegler Reference Karpitschka, Liebig and Riegler2017). Direct, non-intrusive measurements have so far not been available in the literature.
2 Overview
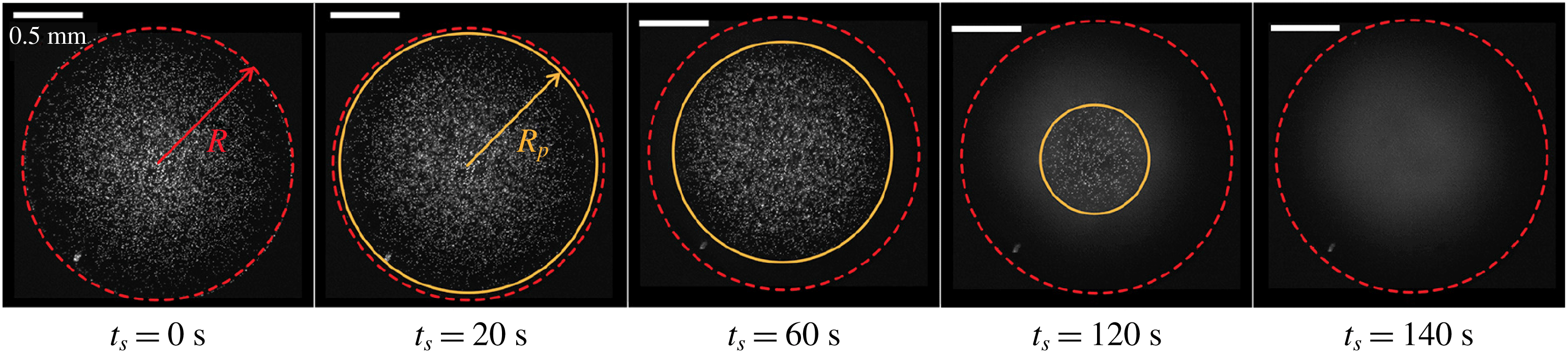
In their recent paper, Kim & Stone present an unconventional yet charmingly simple approach for determining local droplet compositions. They have used the instability of fluorescently labelled microparticles with respect to dissolution in specific solvent mixtures. During evaporation, the solvent composition changes, and disappearing particles indicate where a critical composition has been reached (see figure 1).
Figure 1. Fluorescence signal of particles in an evaporating sessile multi-component droplet. When a critical concentration of the residual component is reached, here indicated by the radius
 $R_{p}$
, particles disappear. Adapted from Kim & Stone (Reference Kim and Stone2018).
$R_{p}$
, particles disappear. Adapted from Kim & Stone (Reference Kim and Stone2018).
In the present case, the particles were made of polystyrene (PS), which is stable in most polar solvents and has a density close to that of water. Dispersed in the liquid under investigation, such particles are routinely used to trace flow velocities. Conventionally, they are considered inert, merely following the streamlines in the fluid. Non-polar solvents, however, typically swell or dissolve PS particles. Kim & Stone have used 1-Methyl-2-Pyrrolidinone (1M2P), an organic solvent that is miscible with water, but dissolves PS particles. It has a much lower vapour pressure than water, and therefore evaporates more slowly. The authors have systematically determined the stability of their particles in 1M2P–water mixtures. They find that above a 1M2P mass fraction of
 ${\approx}0.88$
, the fluorescence signal of the particles disappears.
${\approx}0.88$
, the fluorescence signal of the particles disappears.
This enables unprecedented insight into the compositional evolution of an evaporating droplet of a water/1M2P mixture: in those regions of a droplet where the mass fraction of 1M2P exceeds
 ${\approx}0.88$
, particles dissolve and their fluorescence signal disappears. Thus, by determining the boundary of the region where particles are still visible, an equicompositional surface can be reconstructed in the droplet.
${\approx}0.88$
, particles dissolve and their fluorescence signal disappears. Thus, by determining the boundary of the region where particles are still visible, an equicompositional surface can be reconstructed in the droplet.
Kim & Stone deposited small droplets of water/1M2P mixtures onto glass surfaces. The droplets had contact angles below
 ${\approx}60^{\circ }$
, pinned contact lines and the ambient relative humidity was 10 % or below. Enrichment of 1M2P near the contact line, expected under such conditions, took the form of an outer ring without visible particles, expanding toward the centre of the droplet. By assuming that dissolution occurs much faster than compositional changes, they determined the location where the 1M2P mass fraction exceeded its critical value.
${\approx}60^{\circ }$
, pinned contact lines and the ambient relative humidity was 10 % or below. Enrichment of 1M2P near the contact line, expected under such conditions, took the form of an outer ring without visible particles, expanding toward the centre of the droplet. By assuming that dissolution occurs much faster than compositional changes, they determined the location where the 1M2P mass fraction exceeded its critical value.
Precipitation of sodium chloride (Soulie et al.
Reference Soulie, Karpitschka, Lequien, Prené, Zemb, Möhwald and Riegler2015) or liquid–liquid phase separation (Tan et al.
Reference Tan, Diddens, Lv, Kuerten, Zhang and Lohse2016; Li et al.
Reference Li, Lv, Diddens, Tan, Wijshoff, Versluis and Lohse2018) have been previously used to determine saturation conditions. In these cases, however, the indicating phenomenon strongly affects the fluid physics. A dissolved polymer will also influence the liquid properties. However, its amount remains small, below a volume fraction of
 $10^{-5}$
, so the method of Kim & Stone can be considered much less intrusive.
$10^{-5}$
, so the method of Kim & Stone can be considered much less intrusive.
Kim & Stone compare the measured evolution of the critical composition to the prediction of an analytical model. Their model neglects convection and diffusion in the liquid, which they estimated to be much slower than evaporative enrichment. Further, they assume that the evaporation of water is not influenced by the presence of 1M2P. This is true for the gas phase diffusion, but the evaporation rate also depends on the liquid–vapour equilibrium at the droplet surface. According to Raoult’s law for ideal mixtures, the vapour pressure is proportional to the molar fraction of the substance in the liquid (Atkins & de Paula Reference Atkins and de Paula2009). In the present case, deviations from Raoult’s law remain well below 20 % (Noll, Fischer & Gmehling Reference Noll, Fischer and Gmehling1996) and the evaporation rate should scale with the molar fraction of water. Despite such strong simplifications, Kim & Stone find a good match between their model and the measurements. Deviations for large 1M2P mass fractions, and for the onset of particle dissolution, can most likely be attributed to these simplifications.
3 Future
The present study is an important first step toward a direct measurement of droplet composition. Its main drawback is the limitation to resolving a single equicompositional plane at a time. However, since colloid chemistry offers a plethora of materials with different dissolution properties, it appears feasible to extend this method to other solvent combinations or to simultaneously determine dissolution points of different particles.
Another drawback is the irreversibility of particle dissolution, which would obscure non-monotonic concentration changes. Only very recently, Li et al. (Reference Li, Lv, Diddens, Tan, Wijshoff, Versluis and Lohse2018) have used the selective solubility of dyes to resolve evaporation-induced phase separation by confocal microscopy. A similar approach should be possible for a homogeneous system, using a dye with a calibrated solvatochromic shift. Whispering gallery mode sensors, which recently gained significant attention in biomolecule detection (Foreman, Swaim & Vollmer Reference Foreman, Swaim and Vollmer2015), could provide an even less intrusive access to the local composition. Their optical mode structure depends on the refractive index of the surrounding medium, and PS particles have successfully been used in that context (Francois & Himmelhaus Reference Francois and Himmelhaus2009).
The liquid vapour equilibrium, or Raoult’s law provides a coupling mechanism between the boundary conditions of the gas phase and the liquid composition. This, in turn, affects the evaporation rate through a non-local relation that follows from the diffusion in the gas phase (Eggers & Pismen Reference Eggers and Pismen2010). As predicted by numerical simulations, such a coupling provides a regularization mechanism of the evaporation rate singularity (Diddens et al.
Reference Diddens, Tan, Lv, Versluis, Kuerten, Zhang and Lohse2017; Karpitschka et al.
Reference Karpitschka, Liebig and Riegler2017). Adaptations of the new method by Kim & Stone could provide the missing link between theory and experiment. Despite its limitations, the value of this method lies in its simplicity, requiring only standard fluid physics laboratory equipment.
$R_{p}$, particles disappear. Adapted from Kim & Stone (2018).
1 Introduction
Liquids evaporate far below their boiling point if the atmosphere is not saturated with their vapour. In this case, the rate of evaporation is limited by the transport of vapour through the gas phase, while liquid–vapour equilibrium is maintained at the droplet surface. A sessile droplet of a pure liquid evaporating into quiescent air is a prototype of great importance. Its idealized continuum treatment exhibits a diverging evaporation rate at the contact line, and many studies have been devoted to this problem. The literature has been reviewed e.g. by Cazabat & Guéna (Reference Cazabat and Guéna2010), Brutin (Reference Brutin2015).
Most common, however, is the case where droplets consist of more than a single pure liquid. In daily life or technological applications, multiple components are required for their specific functionalities. Alcoholic beverages, for instance, contain at least three ingredients: water, alcohol and a flavour. In general, different liquids evaporate at different rates, leading to an enrichment of the residual component near the contact line. Evaporating droplets of Ouzo, a popular Greek liquor, are a particularly visual example: Anise oil precipitates and the liquid turns pale in alcohol-depleted regions, starting near the contact line (Tan et al. Reference Tan, Diddens, Lv, Kuerten, Zhang and Lohse2016). Enrichment of residuals can also cause Marangoni convection (Pesach & Marmur Reference Pesach and Marmur1987; Guéna, Poulard & Cazabat Reference Guéna, Poulard and Cazabat2007) which may influence the pattern left behind by a drying drop. Utilizing this to control the deposit pattern (Kim et al. Reference Kim, Boulogne, Um, Jacobi, Button and Stone2016) is relevant e.g. in ink-jet printing. Precipitates of evaporating salt water droplets are important in stainless steel corrosion (Soulie et al. Reference Soulie, Karpitschka, Lequien, Prené, Zemb, Möhwald and Riegler2015), and drying drops of blood form manifold patterns with potential use in disease diagnostics (Brutin et al. Reference Brutin, Sobac, Loquet and Sampol2011). But even for the seemingly simple case of a pure water droplet, theory predicts strong thermal Marangoni flows that are absent in experiments. This discrepancy has been attributed to trace impurities (Hu & Larson Reference Hu and Larson2005; Marin et al. Reference Marin, Liepelt, Rossi and Khler2016), clearly indicating the fundamental importance of multi-component droplet evaporation.
One of the major challenges in the field is determining the spatio-temporal compositional evolution of the droplet. Most previous studies resolved it indirectly from numerical models that were calibrated against accessible observables (Diddens et al. Reference Diddens, Tan, Lv, Versluis, Kuerten, Zhang and Lohse2017; Karpitschka, Liebig & Riegler Reference Karpitschka, Liebig and Riegler2017). Direct, non-intrusive measurements have so far not been available in the literature.
2 Overview
In their recent paper, Kim & Stone present an unconventional yet charmingly simple approach for determining local droplet compositions. They have used the instability of fluorescently labelled microparticles with respect to dissolution in specific solvent mixtures. During evaporation, the solvent composition changes, and disappearing particles indicate where a critical composition has been reached (see figure 1).
Figure 1. Fluorescence signal of particles in an evaporating sessile multi-component droplet. When a critical concentration of the residual component is reached, here indicated by the radius $R_{p}$
, particles disappear. Adapted from Kim & Stone (Reference Kim and Stone2018).
$R_{p}$
, particles disappear. Adapted from Kim & Stone (Reference Kim and Stone2018).
In the present case, the particles were made of polystyrene (PS), which is stable in most polar solvents and has a density close to that of water. Dispersed in the liquid under investigation, such particles are routinely used to trace flow velocities. Conventionally, they are considered inert, merely following the streamlines in the fluid. Non-polar solvents, however, typically swell or dissolve PS particles. Kim & Stone have used 1-Methyl-2-Pyrrolidinone (1M2P), an organic solvent that is miscible with water, but dissolves PS particles. It has a much lower vapour pressure than water, and therefore evaporates more slowly. The authors have systematically determined the stability of their particles in 1M2P–water mixtures. They find that above a 1M2P mass fraction of ${\approx}0.88$
, the fluorescence signal of the particles disappears.
${\approx}0.88$
, the fluorescence signal of the particles disappears.
This enables unprecedented insight into the compositional evolution of an evaporating droplet of a water/1M2P mixture: in those regions of a droplet where the mass fraction of 1M2P exceeds ${\approx}0.88$
, particles dissolve and their fluorescence signal disappears. Thus, by determining the boundary of the region where particles are still visible, an equicompositional surface can be reconstructed in the droplet.
${\approx}0.88$
, particles dissolve and their fluorescence signal disappears. Thus, by determining the boundary of the region where particles are still visible, an equicompositional surface can be reconstructed in the droplet.
Kim & Stone deposited small droplets of water/1M2P mixtures onto glass surfaces. The droplets had contact angles below ${\approx}60^{\circ }$
, pinned contact lines and the ambient relative humidity was 10 % or below. Enrichment of 1M2P near the contact line, expected under such conditions, took the form of an outer ring without visible particles, expanding toward the centre of the droplet. By assuming that dissolution occurs much faster than compositional changes, they determined the location where the 1M2P mass fraction exceeded its critical value.
${\approx}60^{\circ }$
, pinned contact lines and the ambient relative humidity was 10 % or below. Enrichment of 1M2P near the contact line, expected under such conditions, took the form of an outer ring without visible particles, expanding toward the centre of the droplet. By assuming that dissolution occurs much faster than compositional changes, they determined the location where the 1M2P mass fraction exceeded its critical value.
Precipitation of sodium chloride (Soulie et al. Reference Soulie, Karpitschka, Lequien, Prené, Zemb, Möhwald and Riegler2015) or liquid–liquid phase separation (Tan et al. Reference Tan, Diddens, Lv, Kuerten, Zhang and Lohse2016; Li et al. Reference Li, Lv, Diddens, Tan, Wijshoff, Versluis and Lohse2018) have been previously used to determine saturation conditions. In these cases, however, the indicating phenomenon strongly affects the fluid physics. A dissolved polymer will also influence the liquid properties. However, its amount remains small, below a volume fraction of $10^{-5}$
, so the method of Kim & Stone can be considered much less intrusive.
$10^{-5}$
, so the method of Kim & Stone can be considered much less intrusive.
Kim & Stone compare the measured evolution of the critical composition to the prediction of an analytical model. Their model neglects convection and diffusion in the liquid, which they estimated to be much slower than evaporative enrichment. Further, they assume that the evaporation of water is not influenced by the presence of 1M2P. This is true for the gas phase diffusion, but the evaporation rate also depends on the liquid–vapour equilibrium at the droplet surface. According to Raoult’s law for ideal mixtures, the vapour pressure is proportional to the molar fraction of the substance in the liquid (Atkins & de Paula Reference Atkins and de Paula2009). In the present case, deviations from Raoult’s law remain well below 20 % (Noll, Fischer & Gmehling Reference Noll, Fischer and Gmehling1996) and the evaporation rate should scale with the molar fraction of water. Despite such strong simplifications, Kim & Stone find a good match between their model and the measurements. Deviations for large 1M2P mass fractions, and for the onset of particle dissolution, can most likely be attributed to these simplifications.
3 Future
The present study is an important first step toward a direct measurement of droplet composition. Its main drawback is the limitation to resolving a single equicompositional plane at a time. However, since colloid chemistry offers a plethora of materials with different dissolution properties, it appears feasible to extend this method to other solvent combinations or to simultaneously determine dissolution points of different particles.
Another drawback is the irreversibility of particle dissolution, which would obscure non-monotonic concentration changes. Only very recently, Li et al. (Reference Li, Lv, Diddens, Tan, Wijshoff, Versluis and Lohse2018) have used the selective solubility of dyes to resolve evaporation-induced phase separation by confocal microscopy. A similar approach should be possible for a homogeneous system, using a dye with a calibrated solvatochromic shift. Whispering gallery mode sensors, which recently gained significant attention in biomolecule detection (Foreman, Swaim & Vollmer Reference Foreman, Swaim and Vollmer2015), could provide an even less intrusive access to the local composition. Their optical mode structure depends on the refractive index of the surrounding medium, and PS particles have successfully been used in that context (Francois & Himmelhaus Reference Francois and Himmelhaus2009).
The liquid vapour equilibrium, or Raoult’s law provides a coupling mechanism between the boundary conditions of the gas phase and the liquid composition. This, in turn, affects the evaporation rate through a non-local relation that follows from the diffusion in the gas phase (Eggers & Pismen Reference Eggers and Pismen2010). As predicted by numerical simulations, such a coupling provides a regularization mechanism of the evaporation rate singularity (Diddens et al. Reference Diddens, Tan, Lv, Versluis, Kuerten, Zhang and Lohse2017; Karpitschka et al. Reference Karpitschka, Liebig and Riegler2017). Adaptations of the new method by Kim & Stone could provide the missing link between theory and experiment. Despite its limitations, the value of this method lies in its simplicity, requiring only standard fluid physics laboratory equipment.