Glycaemic index (GI) is the ratio of postprandial glycaemic response following ingestion of a test and a reference food containing equivalent amounts of available carbohydrate, expressed as a percentage(Reference Wolever, Jenkins, Jenkins and Josse1). Originally the GI concept was developed to assist individuals with diabetes in choosing carbohydrate-rich foods(Reference Jenkins, Wolever, Taylor, Barker, Fielden, Baldwin, Bowling, Newman, Jenkins and Goff2), as the lower postprandial glycaemic response following low-GI foods was considered to improve overall glycaemic control. Low-GI diets are now often recommended in the general population based on the assumption that they promote a healthy body weight and reduce the risk of several chronic diseases(Reference Ludwig3). Glycaemic load (GL), which is calculated as the product of the amount of carbohydrate and GI, has been suggested as a potentially more useful concept than GI since it provides an indication of glycaemic response to a typically consumed quantity of carbohydrate. Positive associations between risk of diabetes and CHD and dietary GI and/or GL have been found in some, but not all, epidemiological data(Reference Pi-Sunyer4). Thus, despite scientific and public interest in GI and GL, their usefulness in disease prevention and diabetes management remains controversial, although some benefit in blood glucose control for individuals with diabetes is indicated(Reference Sheard, Clark, Brand-Miller, Franz, Pi-Sunyer, Mayer-Davis, Kulkarni and Geil5).
A criticism of GI is that its measurement lacks accuracy and precision(Reference Pi-Sunyer4). The GI of foods are usually estimated in a small number of subjects (n 6–12) and presented with standard errors typically ranging from 3 to 15, indicating that the CI for the GI of some foods are large(Reference Wolever, Vorster and Björck6). For example, if the sample estimate of GI of a particular food were 70 with a standard error of 10, the population estimate of GI would have a 95 % chance of being between 50 and 90, a very imprecise estimate. The estimate of 70 would be regarded as accurate or valid if it differed from the ‘true’ or the underlying ‘error-free’ value of the parameter of interest by an acceptably small amount, and precise if the 95 % CI were narrow. Such estimates are essential if GI is to be used in examining diet and disease relationships(Reference Salmeron, Manson, Stampfer, Colditz, Wing and Willett7) or as a strategy in diabetes prevention and management(Reference Ludwig3, Reference Kalergis, Pytka, Yale, Mayo and Strychar8, 9).
If an individual's response to a given food is measured repeatedly, in conditions as uniform as possible, it varies because of random error or because of the inherent lability of the measure. An indication of the consistency or repeatability of these responses in a sample of participants can be obtained using the intra-class coefficent (ICC) of reliability (ICC or reliability for short) described in the Appendix(Reference Fleiss10). The reliability of a measure depends on both the between- and within-person variability. It can have values between 0 and 1. Better reliability, a value closer to 1, is obtained when the within-person variability is small when compared with the between-person variability. A consistent response to a particular food, or minimal within-person variability, is required for good reliability or an ICC of an acceptable level. This is important if GI is to be used as a strategy in the management of diabetes.
If GI is regarded as simply a property of food it seems advantageous to use an individual's response to a reference food to adjust the glycaemic response to a particular food in the interest of reducing the between-person variation. Estimates of the proportion of total variance attributable to the within- and between-person variation show that adjusting for a reference food in this way reduces the proportion of variance attributable to between-person variation at the expense of the within-person variance(Reference Wolover11). The within-person variance of the GI is an indication of the extent to which estimates of GI depend on the participants' day-to-day responses and it or the CV has been used as a measure of repeatability. The CV is a measure of relative variability as it depends on both the mean and the scale of measurement, making comparisons of the CV for the area under the curve (AUC) of a food and its GI, for instance, difficult(Reference Allison12).
The purpose of the present study was to estimate the reliability of the components of GI (the incremental AUC (iAUC)), and of GI itself, by determining the within- and between-person variation in glycaemic response. The reliability is presented as the ICC which has well-recognised criteria for qualitative interpretation(Reference Fleiss10, Reference Bartko and Carpenter13, Reference Dunn14).
Subjects and methods
Subjects
Twenty individuals, eleven women and nine men, with a normal response to a 2 h oral glucose tolerance test (OGTT) participated in the study. The age and BMI of the group were 23·3 (sd 3·5) years and 23·4 (sd 3·3) kg/m2 respectively. The Human Ethics Committee of the University of Otago approved the study and all participants gave informed consent.
Study design
This was part of a larger study carried out over a 4-month period in which participants completed a series of thirty-three tests following a predetermined balanced randomisation of all products whose sequence differed for each individual(Reference Venn, Wallace, Monro, Perry, Brown, Frampton and Green15). The iAUC was calculated for beverages containing 0, 12·5, 25, 50 and 75 g anhydrous glucose, with tests carried out in triplicate except for the 50 g glucose test, which was repeated on four occasions. Five foods were tested at three serving sizes. These included white bread providing 50 g available carbohydrate tested on three occasions, potato providing 50 g available carbohydrate and chickpeas providing 25 g available carbohydrate studied on a single occasion. The iAUC for the repeated tests for the 50 g glucose beverage, a 25 g glucose beverage and white bread providing 50 g available carbohydrate as well as the response to potato, and chickpeas were used to examine the reliability of GI. The amount of available carbohydrate in the test foods was determined by measuring the total starch and total sugars content of the food. Total starch was determined using Association of Official Analytical Chemists method 996.11(16). Total sugars were determined as the sum of sucrose, lactose, maltose, glucose and fructose, each measured by a GLC method.
Participants were instructed to consume an evening meal containing a large proportion of carbohydrate-rich food the night before each test. It was suggested that the meal be based on rice, bread or potato. Participants were asked not to drink alcohol or consume food or beverages (other than water) after 22.00 hours the night before the tests. On the mornings of the tests the participants were asked to refrain from physical exercise and to report to the clinic in a fasting state. Capillary blood samples were taken using a lancet. A drop of blood was collected into a HemoCue® cuvette and blood glucose concentration measured using a Hemocue® Glucose 201 Analyzer (Aktiebolaget Leo, Helsingborg, Sweden). Each morning the instrument was checked using its own internal system to confirm that it was functioning correctly. The mean of two fasting blood glucose concentrations determined 5 min apart was used as a baseline measure. The time of the second fasting sample was recorded as the start time of the test. White bread or glucose were consumed at an even pace over a period of 15 min and capillary blood samples were taken at 15, 30, 45, 60, 90 and 120 min after the start time. If blood glucose concentrations were >0·2 mmol/l above baseline at 2 h, further blood samples were taken at 150 and 180 min. Participants were asked to remain seated for the duration of the tests. The iAUC was calculated using the method described in FAO/WHO Food and Nutrition paper 66(9).
Before the study, we carried out a validation of the Hemocue® Glucose 201 Analyzer against our standard method. For the standard method, capillary fingerprick blood was collected in micro-centrifuge tubes containing anticoagulant and held on ice until centrifuged at 3000 rpm at 4°C. Blood plasma was pipetted off the packed erythrocytes and stored at − 18°C until analysis. The plasma glucose concentration was analysed using an enzymic UV test with hexokinase (Glucose HK Unikit III; Roche Diagnostica, Basle, Switzerland). All glucose analyses were performed on a Cobas II Fara autoanalyser (Roche Diagnostica). We measured iAUC for glucose ( × 3) and two test foods (yoghurt and bread) in twelve test subjects using the Hemocue® analyser and our standard method. Mean GI using the Hemocue method and our standard method for yoghurt were 37 (se 5) and 37 (se 4) %, respectively. For bread the corresponding means were 57 (se 3) and 58 (se 5) %.
Statistics
The means and standard deviations for all the foods and naive within-subject CV were calculated as the mean of each individual's CV obtained from the standard deviation/mean where there were replicates(Reference Wolever, Vorster and Björck6, Reference Quan and Shih17). Because the variance increased as the mean increased the data were log-transformed before analysis. A single-factor repeated-measures ANOVA was used to estimate the between- and within-person standard deviations and the ICC of reliability of the log-transformed iAUC for glucose and white bread and the reliability of the GI of each food. A random coefficient model, which accounted for the correlation between the estimates for each individual using the log-transformed values, was used to estimate the GI and its 95 % CI for each food and compare the GI of the different foods. Person was used as the random effect. As the values for iAUC were log-transformed the GI of each food was estimated as the difference between the average(log(iAUC test food)) and the average(log(iAUC glucose)). This estimate and its CI were back-transformed to obtain the GI of the test food and its CI.
The reliability of a single observation is defined as 
, where 
is the between-person variance and 
is the within-person variance. The reliability of the mean of m independent replicates is given by: 
(Reference Fleiss10).
Any observation made on an individual is unreliable in the sense that if the observation were repeated under similar conditions it would differ to some extent from the first. If many hypothetical replicate measurements were made under as close to uniform conditions as possible the mean of these measurement would represent an individual's ‘true score’ or underlying ‘error-free score’ for a particular characteristic(Reference Fleiss10). The ICC of an observation is estimated in the context of a study where each participant is measured several times(Reference Fleiss10). The sample estimate of the mean GI and its reliability can be used to estimate the ‘true underlying GI’ or error-free estimate of each individual's GI as well as its 95 % CI(Reference Cook18, Reference Irwig, Glasziou, Wilson and Macaskill19) (see Appendix).
The data were analysed with Stata Release 9 (StataCorp LP, College Station, TX, USA)(20).
Results
The means, standard deviations, geometric means and ranges of the iAUC for glucose and the three foods tested are shown in Table 1. All participants consumed 118 g white bread (50 g available carbohydrate) on three occasions, and a beverage containing 50 g glucose on four occasions. The data, ordered according to participants' mean glucose iAUC, are illustrated in Fig. 1. The postprandial glycaemic responses in some individuals are reasonably consistent, while in others day-to-day responses are markedly different. For example, the minimum and maximum iAUC for participant no. 8 were comparatively consistent, 83–121 for three bread tests, and 151–200 mmol/l × min for four glucose tests. For participant no. 14, the ranges for bread and glucose were wider, 83–218 and 167–287 mmol/l × min, respectively. There was seldom a consistent separation in iAUC between white bread and glucose, with considerable overlap occurring in some cases, for example participants no. 3 and no. 5.
Table 1 Incremental area under the curve (mmol/l×min) for glucose and foods
(Mean values and standard deviations for twenty subjects)
NE, not estimatable.
* The standard deviation is based on the distribution across individuals after averaging the within-person replicates.
† Calculated as the mean of each individual's CV obtained from the standard deviation/mean where there were replicates.
‡ Available carbohydrate.
Fig. 1 (A) The incremental blood glucose area under the curve (iAUC; mmol/l × min) estimates for three replicates of 118 g white bread (●) and four replicates of 50 g glucose beverages (□) measured in twenty participants. (B) The glycaemic index (GI) of white bread for each individual (●) and the mean GI and 95 % CI for the sample (——). The data have been ordered by mean of values for glucose.
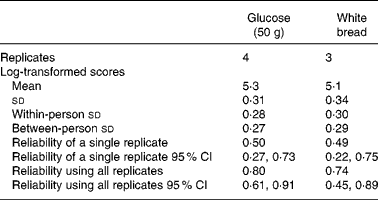
The data in Fig. 1 show that the variability of the iAUC values for both glucose and white bread increase as the mean increases. The data were log-transformed so that the variance did not increase with the mean. The mean and standard deviations (on the log scale) are shown in Table 2. The between- and within-person standard deviations for glucose, also shown in Table 2, were 0·27 and 0·28, indicating that the reliability of a single observation of the iAUC is 0·50 (95 % CI 0·27, 0·73), and 0·66, 0·75 and 0·80 for the mean of two, three and four replicates, respectively. The between- and within-person standard deviations for white bread were 0·29 and 0·30. The reliability of the iAUC values for a single measure of white bread was 0·49 (95 % CI 0·22, 0·75), and 0·65 and 0·74 for the mean of two and three replicates, respectively. The estimate of the GI of white bread was 81 (95 % CI 74, 90). The reliability or ICC of the GI based on four replicates of glucose and three of white bread was 0·27, from which between- and within-standard deviations of 0·13 and 0·22 on the log scale, respectively, can be inferred.
Table 2 Means, standard deviations and reliability for log-transformed values of the incremental area under the curve for glucose and white bread for twenty subjects
The GI for white bread and its 95 % CI (width 16 units) and the GI values for each individual are shown in Fig. 1. Interestingly, the participant with the highest GI had one of the lowest and most consistent responses to both glucose and white bread. If GI is regarded as an estimate of an individual's response to white bread, with random day-to-day fluctuations, future estimates would be expected to be closer to the mean. The width of the 95 % CI for an individual's score, centred on the ‘true’ GI (the GI measured without error), ranged from 32 to 44. These differ from individual to individual because the variance increases as the mean increases.
The data for each individual for both potato and the 50 g glucose beverage are presented in Fig. 2 (A). The individual values for the GI along with the sample mean and 95 % CI are shown Fig. 2 (B). The GI for potato was 87 (95 % CI 76, 101). The reliability, assuming that the reliability of the iAUC of potato was similar to that obtained for glucose, was 0·02. This means that the wide CI for the GI of potato can be attributed almost entirely to within-person variability. The ‘true score’ for individuals depends on the sample mean. The ratio of the GI of potato to white bread was 1·07 (95 % CI 0·88, 1·31) and not statistically different (P = 0·47) from 1.
Fig. 2 (A) The incremental blood glucose area under the curve (iAUC; mmol/l × min) estimates for potato (♦) and four replicates of 50 g glucose beverages (□) measured in twenty participants. (B) The glycaemic index (GI) of potato for each individual (●) and the mean GI and 95 % CI for the sample (——). The participants are in the same order as Fig. 1.
The iAUC values for glucose (25 g) and chickpeas are shown in Fig. 3. There is much less overlap between the values in this case. The reliability for the log-transformed values for glucose was 0·47 (95 % CI 0·21, 0·74), with between- and within-person standard deviations of 0·28 and 0·27 respectively. The GI for chickpeas was 28 (95 % CI 22, 37). The reliability or ICC of the GI of chickpeas was 0·40, assuming that the reliability of the iAUC of chickpeas was the same as that for 25 g glucose. The mean and its 95 % CI (width 15) are shown in Fig. 3 along with the estimate of GI for each individual. Although future values of the GI for chickpeas for an individual would be pulled towards the population mean, the width of the 95 % CI for an individual's score would be between 16 and 97. Thus, a wide range of plausible values for individuals' responses is possible. The ratio of the GI of chickpeas to white bread was 0·34 (95 % CI 0·27, 0·44), which was statistically significant (P < 0·001).
Fig. 3 (A) The incremental blood glucose area under the curve (iAUC; mmol/l × min) estimates for replicates of chickpeas (▲) and three replicates of 25 g glucose beverages (□) measured in twenty individuals. (B) The glycaemic index (GI) of chickpeas for each individual (●) and the mean GI and 95 % CI for the sample (——). The participants are in the same order as Fig. 1.
Discussion
The measures of reliability of the GI of foods described in the present report indicate that their repeatability was unacceptably low. The ICC, which has been recommended for use in human nutrition(Reference Allison12), indicates that the repeatability of the iAUC used in the present study was moderate and those of GI fair to poor(Reference Fleiss10, Reference Dunn14). Values of reliability in the region of 0·5 indicate that the within-person and between-person variances are similar in magnitude; fair or poor reliability, as is the case for the GI of potato, is the result of the within-person variability being much larger than the between-person variability. Although the use of a reference food reduced the between-person variability for GI the within-person variability was still considerable. The magnitude of the overall error, accumulated from the large errors associated with the iAUC, is indicated by the wide CI for the GI means. Modest improvements in the reliability of glucose and white bread could be obtained by increasing the number of replicates, though as the law of diminishing returns applies most gain in reliability comes with the first two or three replicates, with little extra being realised from adding further replicates.
The iAUC in the present study were based on glucose measurements taken at the recommended time points. Increasing the frequency of the observations would capture the glucose profile more accurately and could increase its reliability. More frequent observations would also mean that other ways of summarising the glucose profile such as the maximum glucose concentration could be considered(Reference Matthews, Altman, Campbell and Royston21). However, more frequent observations would add to the cost and perhaps make recruiting and retaining volunteers more difficult. The reliability of GI could be affected by the time interval between repeat tests. If the GI of a food is be used for practical advice in real life, however, it is important that its reliability does not depend on the time interval between the tests. If population estimates are the main concern, problems created by poor reliability could be overcome by increasing the sample size.
The data for the twenty participants shown in Fig. 1 (A) illustrate both the considerable overlap and the wide variation for the values of the iAUC for glucose and white bread. The wide CI indicating that plausible values of the population mean for the GI of white bread were between 74 and 90 were a consequence of this. The CI for the GI of potato and chickpeas were also wide, making the ranking of foods according to their GI, a cornerstone for delivering advice to the population at large, extremely difficult. The CI for individuals' values for the GI of white bread were also very broad, indicating a very unstable response in some participants. If the variability can be attributed to day-to-day random fluctuations, a larger number of replicates would minimise the effect of the within-person variance on the overall variance, and provide more precise CI for the estimate of the GI of a particular food. In real life an individual's response to a food would average out over many eating occasions, so the estimates of the GI of foods such as potato and chickpeas used in the present study may be an indicator of the GI of a long-term diet. If, on the other hand, the different iAUC values for glucose and the test food mark genuine differences in physiological response to carbohydrate on different days, the validity of GI and its usefulness in clinical practice must be questioned. As the present study was based on a relatively homogeneous group of young individuals it is possible that the variability could be even larger if the participants were older, of different ethnic origin or diabetic.
Food is often classified as low, medium or high GI for convenience(Reference Brand-Miller, Wolever, Foster-Powell and Colaguiri22). The error inherent with GI measurement based on small samples means that misclassification is likely to occur. In an interlaboratory study, rice would have been classified as low by one laboratory, medium by three, and high GI by three laboratories(Reference Wolever, Vorster and Björck6). Assigning food a category of GI using small samples implies a level of precision unwarranted by the experimental procedure.
The reliability of an OGTT in 111 participants without diabetes in a longitudinal study of an elderly population in the Netherlands was 0·38 for the iAUC, a value comparable with that obtained in the present study(Reference Feskens, Bowles and Kromhout23). That study also reported a large within-person standard deviation of 87·5 mmol/l × min for the iAUC for the non-diabetic participants. Although the mean value of an OGTT could be different in an older population the reliability, which is a property of the test, should be comparable. Several studies have shown that the reproducibility of OGTT tests is only moderate(Reference McDonald, Fisher and Burnham24, Reference Mooy, Grootenhuis, de Vries, Kostense, Popp-Snijders, Bouter and Heine25) and an article arguing that the OGTT is superfluous suggests that its most serious drawback from the clinical point of view is its lack of reproducibility(Reference Davidson26). If the iAUC in studies of GI are analogous to the OGTT tests, we should not be surprised to find that the reliability is unsatisfactory. The reliabilities reported in the present study were similar to those that can be obtained from data presented in another report(Reference Wolever, Csima, Jenkins, Wong and Josse27) and other authors have alluded to the problems associated with imprecise tests such as glucose tolerance tests(Reference Levy, Morris, Hammersley and Turner28).
The mean and standard deviation for AUC of glucose obtained in the present study were similar in magnitude with those of an interlaboratory study and a more recent Finnish study(Reference Wolever, Vorster and Björck6, Reference Hatonen, Simila, Virtamo, Eriksson, Hannila, Sinkko, Sundvall, Mykkanen and Valstra29). Individuals' GI for white rice ranged from 60 to 131 in a Japanese study using ten participants, a range in estimates of 71 GI units(Reference Sugiyama, Tang, Wakaki and Koyama30), comparable in magnitude with those in the present study. The Japanese study excluded the largest value because it was more than two standard deviations above the mean although there was no evidence to suggest that the responses to glucose and white rice were not genuine. We did not exclude the participant with the highest GI value from our calculations for the GI of bread because replicate tests of glucose and white bread showed very good consistency in iAUC (Fig. 1 (A); participant no. 1), indicating that the GI calculated for this participant was probably a valid representation of their physiological response.
The present study included twenty participants rather than the recommended six to twelve participants. It also used log-transformed values to overcome the strong relationship between the mean and the standard deviation, a practice recommended for AUC(Reference Matthews, Altman, Campbell and Royston21, Reference Keene31). This led to a more normal distribution of the data, a requirement of statistical tests such as ANOVA and t tests(Reference Bland and Altman32). Reliability coefficients as well as estimates of the within-person CV have also been presented. It has been suggested that the CV, the ratio of the standard deviation to the mean, provides a useful statistic for comparing the precision of different variables(Reference Hulley and Cummings33). It is also understood that large CV, as is the case in this and other studies of iAUC, imply imprecise measurement methods. Stronger arguments are made for the use of the ICC, which relates the between-person variance to the total variance comparing like with like as it were(Reference Fleiss10, Reference Allison12). The reliability of GI is poor partly because the reliability of the iAUC for glucose and foods is only moderate, although this can be improved by replication. Furthermore, individuals' responses to glucose and food are correlated, all be it modestly, which makes the total variance less than it would be if the measures were independent.
In many studies poor reliability is overcome by using very large samples, as is the case in many epidemiological studies, which have examined the relationship between GI or GL and the onset of diabetes or CHD. Larger samples with more replicates would provide more precise and possibly more accurate estimates of the GI of foods, making ranking and classifying foods more dependable. Nonetheless, as samples of more than thirty subjects using three replicates for both foods are required to provide CI within 10 % of the GI estimate, it is unrealistic to think that foods with GI differing by 15 or 20 points, derived from samples of six to twelve subjects are necessarily different. The results of the present study do show that the GI of chickpeas is one-third that of white bread, and that this difference is statistically significant, so GI estimated from small samples may be a useful way of signalling major differences in foods.
One of the practical problems associated with small samples and poor reliability is varying estimates of GI even for the same food. In an interlaboratory study in which the same foods were supplied to GI-testing laboratories each using eight to twelve subjects and three replicates for glucose with no repeat testing of the food, mean GI values ranged from 86 to 99 (instant potato), 55 to 85 (rice), 39 to 70 (spaghetti) and 25 to 46 (barley)(Reference Wolever, Vorster and Björck6). Similarly, in the international tables of GI and GL, the GI of Russet baked potatoes (item 603) are reported to range from 56 to 111(Reference Foster-Powell, Holt and Brand-Miller34). The reasons for such large differences in GI for the same food are not understood, but improving reliability by increasing the number of replicates and sample size should help to reduce differences.
The GI and GL concepts have been used in several contexts. Prospective epidemiological studies have examined the relationship between the GL of diet and subsequent chronic disease. Clinical trials demonstrating the potential of low-GI foods to favourably influence lipoprotein profiles and glycaemic control in individuals with diabetes form the basis of recommendations to encourage the use of low-GI foods. The large number of individuals in prospective studies may justify conclusions relating to the protective role of low-GI foods, despite the lack of reliability demonstrated here. On the other hand the low reliability suggests that individual responses are likely to be unpredictable when using GI to guide food choices in the clinical context. The use of GI in dietary prescriptions may be further limited by the variable nature of foods.
Acknowledgements
T. G. and T. P. offer a commercial glycaemic index testing service through the University of Otago.
Funding was provided jointly by The New Zealand Institute for Crop and Food Research Limited and the University of Otago.
The contributions of authors were: S. W. and B. V. wrote the first draft; S. W. carried out the statistical analysis; J. M. and T. G. provided major editorial assistance. R. B., T. P. and A. W. reviewed the manuscript.
Appendix
To quantify the within- and between-person variance of a measure we considered the classical linear model:
where X is the observed value for a particular person, T the error-free score and e the error or the difference between the observed and error-free scores. In a population the error-free score T will vary about some mean with a variance of 
(between-person variation). For a particular person the random error e varies about a mean zero (within-person variation). Assuming that the distribution of the error is independent of T, e has variance 
regardless of the value of T so the variance of X is:
The reliability of X is defined as(Reference Fleiss10):
Thus, the reliability of a measure depends on both between- and within-person error and will be high when (the within-person error) is small in comparison with (the between-person error). The within-person variability can be controlled by replication as the variance of the mean of m replicates is:
So the reliability of the average of m replications is(Reference Fleiss10):
This can also be expressed as:
The reliability of the difference between two measures, in this case averages of the log-transformed values of the AUC for the test food and glucose is:
where 
and 
are the variances of the two measures, r12 is the correlation between them, and R1 and R2 are their reliabilities(Reference Peter, Churchill and Brown35).
The ‘true score’ or the score, which would be obtained if there were no error of measurement, and its standard error, are estimated as follows(Reference Cook18, Reference Irwig, Glasziou, Wilson and Macaskill19):
where Xi is an observation from an individual and μ is the sample mean with variance:
