Introduction
The transformation of snow to ice in polar glaciers proceeds via two principal mechanisms, densification and recrystallization. Densification has to do with the elimination of pore space until the firn is no longer permeable, whereas recrystallization is involved with changes in the granular and crystalline structure of the snow as it densifies. The overall transformation has been described variously as diagenesis (Reference PaulckePaulcke, 1934; Reference BensonBenson, 1959, Reference Benson1962; Reference Anderson, Benson and KingeryAnderson and Benson, 1963), metamorphism (Reference BaderBader and others, 1939) and sintering. So far, studies of this transformation have tended to concentrate on densification, and detailed investigations of the structure of polar firn have been limited to a few locations only. These include observations to 100 m depth at Maudheim (Reference SchyttSchytt, 1958) and to 45 m depth at Southice (Reference Stephenson and ListerStephenson and Lister, 1959; Reference Stephenson and OuraStephenson, 1967) in Antarctica, and the snow-structure studies conducted by Reference FuchsFuchs (1959) and Reference Nakaya, Kuroiwa and ŌuraNakaya and Kuroiwa (1967) to depths of 46 m and 26 m, respectively, at Site 2, Greenland.
In the present study, measurements have been made of the growth of crystals and grains in the top 49 m of the firn at Amundsen-Scott (South Pole) station. The objectives of this study were to (1) ascertain the time rate of growth of crystals and grains at the very low temperatures prevailing at the South Pole, and (2) evaluate the temperature dependence of this growth in conjunction with data obtained at other locations in Antarctica and Greenland.
Sampling
Samples were obtained from a 28 m deep snow mine and from cores from a hole drilled at the bottom of the mine to a depth of 49 m below the surface. All samples were selected randomly, without regard to grain-size or visible stratigraphic structure. Densities of samples ranged from 0.32 Mg m−3 near the surface to 0.65 Mg m−3 at 49 m. The current rate of snow accumulation at the South Pole is 70 kg m−2 year−1 and the age of the firn at 49 m depth is estimated at 388 years. The temperature below 10 m is virtually constant at −51°C. Detailed results of these and related glaciological studies at the South Pole are to be found in Reference GiovinettoGiovinetto (1960), Reference Gow and RamseierGow and Ramseier (1963), Reference PicciottoPicciotto and others (1964), Reference EpsteinEpstein and others (1965) and Reference GowGow (1965).
Preparation of Thin Sections
In firn, as in other well-bonded aggregates, e.g. sedimentary rocks, and metallurgical and ceramic sinters, the only feasible method of investigating the size and structural arrangement of particles is to prepare thin or polished sections. Most methods of preparing thin sections of snow require preliminary filling of the pore spaces to facilitate mounting and to reinforce the structure against breakage during sectioning. A number of liquid fillers have been used for this purpose, including tetrabromoethane (Reference BaderBader and others, 1939), diethylphthalate (Reference SchyttSchytt, 1958; Reference Stephenson and ListerStephenson and Lister, 1959) and aniline (Reference Kinosita and WakahamaKinosita and Wakahama, 1959; Reference Nakaya, Kuroiwa and ŌuraNakaya and Kuroiwa, 1967). Aniline was used in the present study. Water-saturated aniline freezes at −11 °C, does not noticeably deform the snow structure on freezing and melts out to a transparent isotropic liquid. Other advantages are its low solubility for water, low viscosity and good wettability. The principal disadvantage of aniline is that it is a toxic material that must be handled with care even at low temperatures. It should be pointed out here that this direct use of aniline is in no way associated with the staining properties of aniline dye, a derivative of aniline.
Thin sections were prepared in essentially the same manner as described by Reference Kinosita and WakahamaKinosita and Wakahama (1959). Small blocks of firn measuring 3×3×1 cm were immersed in water-saturated aniline at −7°C and when fully impregnated were allowed to congeal at −20°C. After freezing a 5×7.5 cm microscope slide to one side of the block, the other side was planed smooth with a heavy sledge-type microtome and a second glass slide frozen onto the prepared surface. The block was cut in two on a band saw and the final sectioning was performed on the microtome. The remainder of the sample (with glass slide attached) was retained for making additional sections as needed. The thickness of the finished section measured approximately one-half the average grain diameter of the specimen. Sections could be prepared in as little as half-an-hour with this technique, which yields much better material for examining firn structure than either the replica method (Reference FuchsFuchs, 1956, Reference Fuchs1959) or the “red paste” technique used by Reference ShimizuShimizu (1958). A Bausch and Lomb extension camera fitted with polarizers was used to obtain structure photographs of each thin section. Best results are obtained if the temperature is raised to about −7°C to allow all interstitial aniline to melt. Photographs were taken at ×10 magnification with fine-grained panchromatic sheet film measuring 17.5×12.5 cm. A single photograph of this size generally contains enough crystals for analyzing the average crystal size of a sample. Actual measurements were made on contact prints which also constitute a ready source of “structure pictures” that can be compared and evaluated at a moment’s notice.
Distinction between Grains and Crystals
Snow is a granular material in which the individual grains may be composed of more than one crystal. This distinction between grains and crystals is best observed in thin sections placed between crossed polaroids; different crystals in the same grain exhibit different bire fringence colors because of variations in orientation. Whereas crystals were found to retain relatively simple outlines at all depths at the South Pole, grains did not, principally because of the growth of intergranular bonds which cause virtually complete obscuration of individual outlines at about 10 m depth. Only crystals could be distinguished satisfactorily for size analysis below 10 m.
Grain- and Crystal-size Measurements
The determination of grain and crystal size in thin or polished sections usually involves measurements of either the diameter or the cross-sectional area of particles. Reference SeligmanSeligman (1949), for example, employed the method of the least-circle diameter using a set of root-mean-square diameters ranging from 0.25 to 10 cm to measure grain-size in rubbings of ice. Reference Ahlmann and DroesslerAhlmann and Droessler (1949) preferred to measure the longest and shortest axes of ice grains and to compute cross-sectional areas from these data. Reference SchyttSchytt (1958) and Reference Stephenson and OuraStephenson (1967) employed much the same method in their studies of crystal growth in Antarctic firn. In general, however, the average particle size obtained from thin-section analysis will be less than the true value simply because a section seldom cuts crystals at their maximum diameter. Reference KrumbeinKrumbein (1935), for example, found with sections of uniform spherical grains that the most frequent diameter (or mode) was only about 80% of the true modal diameter, i.e. the modal cross-section will measure less than 64% of its true value. Variations in particle shape also complicate the problem which is further compounded by the lack of knowledge concerning the actual distribution of particle sizes within the original sample.
A cursory examination of the grains obtained by disaggregating several individual snow layers at the South Pole indicated a certain uniformity of size and shape of the constituent grains in each layer. From these observations it might be deduced that the smaller cross-sections in a thin slice or section are due more to under-average cuts than to under-sized particles (grains or crystals). Since under-sized cross-sections exert a disproportionate influence on the average particle size it was decided to restrict measurements of crystal size at the South Pole to the 50 largest crystals in each section. With the exception of very fine-grained layers in the top 2 m, these 50 largest crystals constitute at least 25% of the total number of crystals in a section measuring 1.75×1.25 cm. The mean crystal size obtained in this way is believed to yield a more realistic value than that based on measurements of all the crystals in a section or a random selection of crystals. Crystal cross-sectional areas were computed from measurements of the length and breadth made with a “pocket comparator” (simply a magnifying glass enscribed with suitable scales) on large contact prints. Crystal-size distributions of a number of representative samples of South Pole firn are shown in Figure 1. All curves are characterized by a relatively narrow range of crystal sizes or high degree of sorting. Crystal-size data for all samples arc presented in Table 1 and Figure 2. Measurements of grain cross-sections to 10 m depth are given in Table II.

Fig. 1. Representative cumulative frequency curves of crystal areas in South Pole firn.
Table I Crystal-Size Measurements in Firn at the South Pole. (Ages Calculated on the Basis of a Constant Accumulation Rate of 70 kg m−2 Year−1)


Fig. 2. Fist crystal size versus depth at the South Pole.
Table II Grain-Size and Grain–Crystal-Size Relationships in Firn at the South Pole. Results Based on Measurements of Cross-Sectional Areas of 50 Largest Crystals and Grains in a Section Measuring 1.95×1.25 cm

Results and Discussion
Grain cross-sections at the South Pole were observed to increase in size from approximately 0.24 mm2 at 0.1 m to 0.63 mm2 at 10 m. Crystal cross-sections, as distinct from grains, also increased between two- and three-fold from 0.18 to 0.43 mm2 over the same depth interval. These data (see Table II for grain–crystal relations) would indicate that grains and crystals tend to maintain a fairly constant growth ratio (1.4 : 1) during the early stages of recrystallization and that most grains are composed of just one or two crystals and not several as is frequently contended. Reference SchyttSchytt (1958) also recorded a three-fold growth of crystals in the top 10 m of firn at Maudheim. The fact that he failed to detect any significant increase in grain-size between the surface and 10 m depth clearly indicates that the crystals must have grown at the expense of grains. The ratio of grain cross-section to crystal cross-section at 10 m at Maudheim was 1.36 : 1, very similar to that observed at the South Pole. Reference Stephenson and OuraStephenson’s (1967) data from Southice also yield a grainFootnote * to crystal ratio of approximately 1.4 : 1 at 10 m depth. Grains, for all practical purposes, appear to reach a limiting size at about 10 m depth. Crystals of comparable cross-sectional area occur at 16–18 m depth at Maudheim and Southice but not until nearly 50 m at the South Pole. However, it seems worth noting that the density of the firn at these levels is much the same at all three locations, 0.62–0.64 Mg m−3.
The much greater rates of growth of grains and crystals in the top 4 m at the South Pole can probably be attributed to the effects of sustained temperature gradients in a snowpack that is accumulating very slowly. These dampen out rather rapidly below 4 m. Virtually constant temperatures (these approximate closely the mean annual surface air temperature) prevail below 10 m, so that the growth of firn crystals at the South Pole can be considered analogous to the process of “crystal” growth in isothermally sintered aggregates in metals and ceramic materials (Reference Burke and TurnbullBurke and Turnbull, 1952; Reference Burke and KingeryBurke, 1958).
Crystals only could be distinguished below 10 m, and over the interval 10 to 49 m the size of crystals increased approximately linearly at the rate of 0.005 mm2 per m. At 49 m the average crystal cross-section measured 0.63 mm2. The mean value of the deviations from the straight line in Figure 2 is less than 0.06 mm2 and the maximum deviation (at 48 m) is 0.4 mm2. Extrapolation of this straight line to the estimated depth (110 m) of the firn-ice transition would give a mean crystal size of nearly 1.00 mm2 at 110 m. A comparison of the South Pole data with other crystal-size measurements from Antarctica and Greenland (Fig. 3) demonstrates clearly the retarding effect on crystal growth of the very low temperatures prevailing at the South Pole. This occurs in spite of the fact that the firn is considerably older and has, as a consequence, been recrystallizing for a longer period of time at the South Pole than at corresponding depths at the other locations.

Fig. 3. Crystal-size variations with depth of firn in Greenland and Antarctica. Data sources: Site 2, Greenland (Reference FuchsFuchs, 1959); Southice (Stephenson and Lister, 1958); Maudheim (Schytt, 1951); Wilkes (Reference HollinHollin and others, 1961).
It is interesting to note that all growth curves except the one for Site 2, Greenland, in Figure 3 intersect at 5 m depth where the mean crystal size is about 0.45 mm2. Virtually identical profiles at Maudheim and Southice probably reflect a balance between temperature (much higher temperatures at Maudheim) on the one hand and the time factor (much older snow at Southice) on the other.
A plot of crystal size versus depth is useful for characterizing firn-structure changes in a glacier. However, with regards growth rates and growth mechanisms, a much more meaningful set of data is likely to be provided by a plot of crystal size versus time. The elapsed time (or age of a sample) will depend on the rate of accumulation. If the effect of load per se is ignored and it is assumed for simplicity that the rate of accumulation remains constant at a particular location, then the snow load (σ) at any depth will be directly proportional to the elapsed time (t), i.e. σ = At, where A is the mean accumulation rate. In order to determine the age of a sample at any particular depth the snow load σ (obtained by integrating the depth–density curve to the appropriate depth) is simply divided by the rate of accumulation A.
Assuming a constant value of accumulation of 70 kg m−2 year−1 at the South Pole, it was found (Fig. 4) on recalculating data from Figure 2 that the mean crystal cross-section below 4 m increased essentially linearly with the age of the firn. This growth relationship can be expressed in the form
where D 2 is the measured mean cross-sectional area (mm2) of crystals in a firn sample of age t (years’ and

Fig. 4. Time rate of growth of crystals in polar firn. Ages calculated on the basis of constant accumulation rates of: 70 kg m−2 year−1 at South Pole; 100 kg m−2 year−1 at Southice; 400 kg m−2 year−1 at Site 2, Greenland; 130 kg m−2 year−1 at Wilkes; 370 kg m−2 year−1 at Maudheim. 10 m temperatures at each location are indicated in the figure.

Fig. 5. Thin-section photographs of the structure of South Pole firn. The clear areas in each section represent pore spaces (filled with melted aniline). The photographs at 0.1, 37 and 47 m were taken in ordinary light. The remaining sections were photographed between crossed polaroids to reveal the crystals. The crystalline nature of the grain structure at 47 in is also demonstrated. ×6.7.

A recalculation of crystal-growth data from other locations in Antarctica and Greenland also reveals a strong linear relationship between the cross-sectional areas of crystals and their ages. It is clear also from Figure 4 that crystal-growth rates are strongly temperature dependent; the higher the firn temperature, the faster the growth. As indicated in Table III, this growth increases more than 30-fold from 0.0006 mm2 year−1 at the South Pole to nearly 0.019 mm2 year−1 at Maudheim. Furthermore, it appears that the temperature dependence of the growth of crystals in polar firn can be expressed in an equation of the form
where K is the observed rate of growth of crystals

Fig. 6. Temperature dependence of crystal growth rates in polar firn.
Table III Crystal-Growth Rates (K) of Polar Firn; Determined According to the Relation

The temperature range of crystal-growth observations made so far in Antarctica, −51° to −17°C, comes close to spanning the entire temperature range of the dry snow facies (−60° to −15°C). An extrapolation of data in Figure 6 indicates that crystal-growth rates in Antarctica could be expected to vary by at least two orders of magnitude—from a rate of 0.0003 mm2 year−1 at −60°C to 0.03 mm2 year−1 at −15°C.
An important by-product of crystal-size measurements is that they provide data that could be used to obtain estimates of accumulation rates. The only change in presenting the data would be to replace the crystal size—age plot (which was obtained originally by assuming a constant accumulation rate) with a plot of crystal size versus snow load. From such a plot, it is possible to determine a load rate of growth which can be expressed in the units mm2 per kg m−2. Knowing the firn temperature (to m temperature), Figure 6 can now be used to pick off the growth rate, mm2 year−1, appropriate to that temperature. To determine the mean accumulation rate, the time rate of growth of crystals obtained from Figure 6 is simply divided by the measured load rate of growth, i.e.

Acknowledgements
This study was sponsored by the Office of Antarctic Programs, U.S. Antarctic Research Program, and was funded by a grant from the National Science Foundation. The support of these institutions and the assistance in the field of various elements of Task Force 43, U.S. Navy, is gratefully acknowledged. I am grateful to Mr L. W. Stanley of U.S. Army CRREL for assisting with data analysis and to Dr W. F. Weeks for critically reviewing the manuscript.


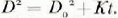 . The fact that the temperature dependence of the crystal growth rate
. The fact that the temperature dependence of the crystal growth rate 








