1. Introduction
One of the most important questions in fluid mechanics is how, and under what conditions, flows transition to a turbulent state; this determines the topological, dissipative and mixing properties of these flows. Besides its fundamental interest as a unique physical process, it is central to every application where fluid flows through a conduit: turbulent mixing promotes heat exchange in cooling applications, whereas turbulent dissipation drastically increases energy consumption. As discovered by Reynolds (Reference Reynolds1883), the flow of water through a pipe became turbulent only for sufficiently high values of the eponymous Reynolds number ![]() $Re=U_0 L/\nu$. In the present work,
$Re=U_0 L/\nu$. In the present work, ![]() $Re$ is built out of the typical streamwise velocity
$Re$ is built out of the typical streamwise velocity ![]() $U_0$ and kinematic viscosity
$U_0$ and kinematic viscosity ![]() $\nu$ of the fluid, and the transverse conduit length scale
$\nu$ of the fluid, and the transverse conduit length scale ![]() $2L$. Since then, the question of transition was often tackled by seeking the conditions necessary for perturbations growing from the laminar base flow to ignite turbulence.
$2L$. Since then, the question of transition was often tackled by seeking the conditions necessary for perturbations growing from the laminar base flow to ignite turbulence.
In pipes and other shear flows, the most distinctive property of the transition to turbulence is that it is subcritical. If ![]() $Re_c$ is the critical Reynolds number beyond which some perturbation grows exponentially from an infinitely small amplitude through a linear mechanism, turbulence can develop at
$Re_c$ is the critical Reynolds number beyond which some perturbation grows exponentially from an infinitely small amplitude through a linear mechanism, turbulence can develop at ![]() $Re< Re_c$, provided the flow is seeded with a sufficiently energised perturbation. The process is nonlinear and amplifies finite amplitude perturbations through an intrinsically 3-D ‘lift-up’ mechanism, enacted by the growth of streamwise streaks (Schmid & Henningson Reference Schmid and Henningson2001). It also becomes active at Reynolds numbers well below
$Re< Re_c$, provided the flow is seeded with a sufficiently energised perturbation. The process is nonlinear and amplifies finite amplitude perturbations through an intrinsically 3-D ‘lift-up’ mechanism, enacted by the growth of streamwise streaks (Schmid & Henningson Reference Schmid and Henningson2001). It also becomes active at Reynolds numbers well below ![]() $Re_c$, where infinitesimal perturbations are severely damped. For convenience we define
$Re_c$, where infinitesimal perturbations are severely damped. For convenience we define ![]() $r_{c}=Re/Re_c$, where subcritical Reynolds numbers correspond to
$r_{c}=Re/Re_c$, where subcritical Reynolds numbers correspond to ![]() $r_{c}<1$. The least damped perturbations are the 2-D transverse-invariant Tollmien–Schlichting waves (TS waves), which manifest in plane shear flows. For instance, Beneitez et al. (Reference Beneitez, Duguet, Schlatter and Henningson2019) showed that TS waves were not found to partake in the 3-D transition to turbulence below
$r_{c}<1$. The least damped perturbations are the 2-D transverse-invariant Tollmien–Schlichting waves (TS waves), which manifest in plane shear flows. For instance, Beneitez et al. (Reference Beneitez, Duguet, Schlatter and Henningson2019) showed that TS waves were not found to partake in the 3-D transition to turbulence below ![]() $r_{c} \simeq 0.98$ in Blasius boundary layers, while Zammert & Eckhardt (Reference Zammert and Eckhardt2019) showed that they could not be detected in plane Poiseuille flow for
$r_{c} \simeq 0.98$ in Blasius boundary layers, while Zammert & Eckhardt (Reference Zammert and Eckhardt2019) showed that they could not be detected in plane Poiseuille flow for ![]() $r_c \lesssim 0.84$.
$r_c \lesssim 0.84$.
However, in rapidly rotating or stratified flows, or in an electrically conducting fluid subjected to a high magnetic field, fluid motion can be prevented from becoming 3-D if the respective Coriolis, buoyancy or Lorentz forces are sufficiently intense. Hydraulic circuits in rotating machines, atmospheres, oceans and some models of planetary interiors subject to planetary rotation, and the liquid metal blankets cooling fusion reactors, all occupy this category. Real flows can never be fully 2-D (Paret et al. Reference Paret, Marteau, Paireau and Tabeling1997; Akkermans et al. Reference Akkermans, Kamp, Clercx and van Heijst2008); three-dimensionality subsists either in asymptotically small measure or in asymptotically small regions such as boundary layers (Sansón & van Heijst Reference Sansón and van Heijst2000; Pothérat Reference Pothérat2012). The resulting flows are quasi-two-dimensional (quasi-2-D). Since the lift-up mechanism driving transition in 3-D shear flows cannot manifest in quasi-2-D flows, can subcritical quasi-2-D turbulence exist, and if so via which alternative transition mechanism?
Traditionally, quasi-2-D turbulence has ‘only’ been considered as a limit-state of its 3-D counterpart (Moffatt Reference Moffatt1967; Sommeria & Moreau Reference Sommeria and Moreau1982; Shats, Byrne & Xia Reference Shats, Byrne and Xia2010), perhaps because both very often coexist (Celani, Musacchio & Vincenzi Reference Celani, Musacchio and Vincenzi2010). For example, atmospheric flows are quasi-2-D at large, continental scales, but 3-D nearer to topographic scales (Lindborg Reference Lindborg1999). A similar spectral split exists in magnetohydrodynamic turbulence (Baker et al. Reference Baker, Pothérat, Davoust and Debray2018) and in turbulence in thin channels (Benavides & Alexakis Reference Benavides and Alexakis2017). Quasi-2-D turbulence appears progressively rather than through a bifurcation, and is controlled by the constraint driving two-dimensionality (Sommeria & Moreau Reference Sommeria and Moreau1982; Pothérat & Klein Reference Pothérat and Klein2014; Benavides & Alexakis Reference Benavides and Alexakis2017). Turbulent transition in quasi-2-D shear flows differs from the switch between 3-D and 2-D turbulence as it is expected to arise suddenly, out of (quasi-)2-D finite amplitude instabilities. Since 3-D mechanisms are excluded, the question is whether there exists a quasi-2-D subcritical transition pathway from the laminar to the turbulent state. This is particularly crucial in shear flows as the subcritical lift-up transition is progressively suppressed as two-dimensionality is established (Cassells et al. Reference Cassells, Vo, Pothérat and Sheard2019). The key result presented in this paper is the discovery of a transition from the quasi-2-D laminar state to subcritical quasi-2-D turbulence in shear flows, and that the lack of a 3-D bypass mechanism gives way to an alternative nonlinear 2-D mechanism which, unlike its 3-D counterpart, relies on TS waves.
2. Physical model
All calculations are performed on a rectangular incompressible duct flow, with walls in the ![]() $(x,z)$ plane moving at a constant velocity
$(x,z)$ plane moving at a constant velocity ![]() $U_0\boldsymbol {e}_x$ (
$U_0\boldsymbol {e}_x$ (![]() $\boldsymbol e_{x,y,z}$ are unit vectors in the respective
$\boldsymbol e_{x,y,z}$ are unit vectors in the respective ![]() $x,y,z$ directions). The base flow is streamwise invariant and periodic boundary conditions are imposed in the streamwise direction (
$x,y,z$ directions). The base flow is streamwise invariant and periodic boundary conditions are imposed in the streamwise direction (![]() $x$). The flow is assumed quasi-2-D, i.e. all quantities are invariant in
$x$). The flow is assumed quasi-2-D, i.e. all quantities are invariant in ![]() $z$ except in thin boundary layers near the fixed walls in
$z$ except in thin boundary layers near the fixed walls in ![]() $(x,y)$ planes (see figure 1). Such flows are well described by the 2-D,
$(x,y)$ planes (see figure 1). Such flows are well described by the 2-D, ![]() $z$-averaged Navier–Stokes equations for
$z$-averaged Navier–Stokes equations for ![]() $z$-averaged velocity and pressure supplemented by a linear friction term accounting for the friction these layers impart. With length, velocity, time and pressure respectively scaled by
$z$-averaged velocity and pressure supplemented by a linear friction term accounting for the friction these layers impart. With length, velocity, time and pressure respectively scaled by ![]() $L$,
$L$, ![]() $U_0$,
$U_0$, ![]() $L/U_0$ and
$L/U_0$ and ![]() $\rho U_0^2$, these equations are
$\rho U_0^2$, these equations are
with dimensionless boundary conditions ![]() $\boldsymbol {u}_\perp (y=\pm 1) = (1,0)$, where
$\boldsymbol {u}_\perp (y=\pm 1) = (1,0)$, where ![]() $\boldsymbol {u}_\perp =(u_\perp,v_\perp )$,
$\boldsymbol {u}_\perp =(u_\perp,v_\perp )$, ![]() $\boldsymbol {\nabla }_\perp = (\partial _x,\partial _y)$,
$\boldsymbol {\nabla }_\perp = (\partial _x,\partial _y)$, ![]() $\boldsymbol {\nabla }_\perp ^2=\partial _x^2+\partial _y^2$,
$\boldsymbol {\nabla }_\perp ^2=\partial _x^2+\partial _y^2$, ![]() $\rho$ is the fluid density, and
$\rho$ is the fluid density, and ![]() $2L$ the distance between the moving walls. Friction parameter
$2L$ the distance between the moving walls. Friction parameter ![]() $H$ may be defined as appropriate to describe systems including duct flows under a strong transverse magnetic field
$H$ may be defined as appropriate to describe systems including duct flows under a strong transverse magnetic field ![]() $B\boldsymbol {e}_z$ (Sommeria & Moreau Reference Sommeria and Moreau1982), thin films (Bühler Reference Bühler1996), or flows with background rotation (with the addition of the Coriolis force) (Pedlosky Reference Pedlosky1987).
$B\boldsymbol {e}_z$ (Sommeria & Moreau Reference Sommeria and Moreau1982), thin films (Bühler Reference Bühler1996), or flows with background rotation (with the addition of the Coriolis force) (Pedlosky Reference Pedlosky1987).

Figure 1. An example of a quasi-2-D flow is shown, namely a lateral wall-driven laminar duct flow under a transverse magnetic field. Here the solution (green profile, ![]() $H=10$) obtained from the quasi-2-D equations (2.1) precisely approximates the
$H=10$) obtained from the quasi-2-D equations (2.1) precisely approximates the ![]() $z$-average of the 3-D streamwise velocity profile
$z$-average of the 3-D streamwise velocity profile ![]() $u_{3D}$ (grey isosurface, black contour lines) satisfying the quasi-static magnetohydrodynamic equations (Müller & Bühler Reference Müller and Bühler2001). Beyond this example, the results derived in this paper apply to a much wider variety of flows.
$u_{3D}$ (grey isosurface, black contour lines) satisfying the quasi-static magnetohydrodynamic equations (Müller & Bühler Reference Müller and Bühler2001). Beyond this example, the results derived in this paper apply to a much wider variety of flows.
We investigate perturbations ![]() $\hat {\boldsymbol {u}}_\perp$ about the base flow
$\hat {\boldsymbol {u}}_\perp$ about the base flow ![]() $\boldsymbol {U}_\perp (y) = (\cosh [H^{1/2}y]/ \cosh [H^{1/2}],0)$ at
$\boldsymbol {U}_\perp (y) = (\cosh [H^{1/2}y]/ \cosh [H^{1/2}],0)$ at ![]() $H=10$. Here linear perturbations become unstable at
$H=10$. Here linear perturbations become unstable at ![]() ${{Re}}_{c} = 79\,123.2$. At
${{Re}}_{c} = 79\,123.2$. At ![]() $r_{c} = 0.9$, the maximum linear transient growth occurs at a streamwise wavenumber
$r_{c} = 0.9$, the maximum linear transient growth occurs at a streamwise wavenumber ![]() $\alpha _{opt} = 1.49$, whereas the minimum exponential decay occurs at
$\alpha _{opt} = 1.49$, whereas the minimum exponential decay occurs at ![]() $\alpha _{max} = 0.979651$. The linearly optimised initial perturbation maximising growth in the functional
$\alpha _{max} = 0.979651$. The linearly optimised initial perturbation maximising growth in the functional ![]() $G=\lVert \hat {\boldsymbol {u}}_\perp (t=\tau )\rVert /\lVert \hat {\boldsymbol {u}}_\perp (t=0)\rVert$ is sought following Barkley, Blackburn & Sherwin (Reference Barkley, Blackburn and Sherwin2008) for a prescribed target time
$G=\lVert \hat {\boldsymbol {u}}_\perp (t=\tau )\rVert /\lVert \hat {\boldsymbol {u}}_\perp (t=0)\rVert$ is sought following Barkley, Blackburn & Sherwin (Reference Barkley, Blackburn and Sherwin2008) for a prescribed target time ![]() $\tau$ and wavenumber
$\tau$ and wavenumber ![]() $\alpha$. Here
$\alpha$. Here ![]() $G$ represents the gain in perturbation kinetic energy under the norm
$G$ represents the gain in perturbation kinetic energy under the norm ![]() $\lVert \hat {\boldsymbol {u}}_\perp \rVert = \int \hat {\boldsymbol {u}}_\perp \boldsymbol {\cdot } \hat {\boldsymbol {u}}_\perp \,\mathrm {d}\varOmega$, over computational domain
$\lVert \hat {\boldsymbol {u}}_\perp \rVert = \int \hat {\boldsymbol {u}}_\perp \boldsymbol {\cdot } \hat {\boldsymbol {u}}_\perp \,\mathrm {d}\varOmega$, over computational domain ![]() $\varOmega$. Optimisation is performed on the linearised rather than the full nonlinear equation, though both return practically identical results for this problem (Camobreco, Pothérat & Sheard Reference Camobreco, Pothérat and Sheard2020). The choice of
$\varOmega$. Optimisation is performed on the linearised rather than the full nonlinear equation, though both return practically identical results for this problem (Camobreco, Pothérat & Sheard Reference Camobreco, Pothérat and Sheard2020). The choice of ![]() $\alpha$ is based on the decay rate of the leading direct eigenmode, obtained by decomposing perturbations into normal modes
$\alpha$ is based on the decay rate of the leading direct eigenmode, obtained by decomposing perturbations into normal modes ![]() $(\boldsymbol{\tilde{u}}_\perp, \tilde {p}_\perp ) \exp (\mathrm {i}[\alpha x-\omega t])$ of complex frequency
$(\boldsymbol{\tilde{u}}_\perp, \tilde {p}_\perp ) \exp (\mathrm {i}[\alpha x-\omega t])$ of complex frequency ![]() $\omega$, and complex velocity and pressure distributions
$\omega$, and complex velocity and pressure distributions ![]() $\tilde {\boldsymbol u}_\perp$ and
$\tilde {\boldsymbol u}_\perp$ and ![]() $\tilde{p}_\perp$. A discretised direct eigenvalue problem
$\tilde{p}_\perp$. A discretised direct eigenvalue problem ![]() $-\mathrm {i}\omega \tilde {v}_\perp = \boldsymbol {L}\tilde {v}_\perp$ is solved in MATLAB via eigs(
$-\mathrm {i}\omega \tilde {v}_\perp = \boldsymbol {L}\tilde {v}_\perp$ is solved in MATLAB via eigs(![]() $\mathrm {i}\boldsymbol {L}$). The linear evolution operator
$\mathrm {i}\boldsymbol {L}$). The linear evolution operator ![]() $\boldsymbol {L}$ is constructed following Trefethen et al. (Reference Trefethen, Trefethen, Reddy and Driscoll1993) and Schmid & Henningson (Reference Schmid and Henningson2001). The discretised adjoint eigenvalue problem
$\boldsymbol {L}$ is constructed following Trefethen et al. (Reference Trefethen, Trefethen, Reddy and Driscoll1993) and Schmid & Henningson (Reference Schmid and Henningson2001). The discretised adjoint eigenvalue problem ![]() $\mathrm {i}\omega^{\ddagger} \tilde {\xi }_\perp = \boldsymbol {L}^{\ddagger} \tilde {\xi }_\perp$ for complex adjoint eigenvector
$\mathrm {i}\omega^{\ddagger} \tilde {\xi }_\perp = \boldsymbol {L}^{\ddagger} \tilde {\xi }_\perp$ for complex adjoint eigenvector ![]() $^{\ddagger}\tilde{\xi}_\perp$ is also considered, where the linear adjoint operator
$^{\ddagger}\tilde{\xi}_\perp$ is also considered, where the linear adjoint operator ![]() $\boldsymbol {L}^{\ddagger}$ is derived following Schmid & Henningson (Reference Schmid and Henningson2001).
$\boldsymbol {L}^{\ddagger}$ is derived following Schmid & Henningson (Reference Schmid and Henningson2001).
To support the classification of initial conditions realising turbulence, streamwise Fourier spectra of kinetic energy are computed at selected instants in time at ![]() $21$ equi-spaced
$21$ equi-spaced ![]() $y$-values spanning the channel. At each
$y$-values spanning the channel. At each ![]() $y$-location, a Fourier transform is obtained along
$y$-location, a Fourier transform is obtained along ![]() $x$, with coefficients for the streamwise wavector
$x$, with coefficients for the streamwise wavector ![]() $\kappa$,
$\kappa$, ![]() $c_\kappa (y) = N_{f}^{-1}\sum _{n=0}^{N_{f}-1}\lvert \hat {\boldsymbol {u}}_\perp (x_n,y)\rvert ^2\exp (-2{\rm \pi} \mathrm {i} \kappa n/N_{f})$, where the
$c_\kappa (y) = N_{f}^{-1}\sum _{n=0}^{N_{f}-1}\lvert \hat {\boldsymbol {u}}_\perp (x_n,y)\rvert ^2\exp (-2{\rm \pi} \mathrm {i} \kappa n/N_{f})$, where the ![]() $n^{\rm th}$ coefficient
$n^{\rm th}$ coefficient ![]() $x_n=2{\rm \pi} n/(\alpha N_f)$ spans the streamwise-periodic domain. Here for convenience the coefficient
$x_n=2{\rm \pi} n/(\alpha N_f)$ spans the streamwise-periodic domain. Here for convenience the coefficient ![]() $N_{f}^{-1}$ is applied to the forward transform rather than its inverse. Instantaneous mean Fourier coefficients
$N_{f}^{-1}$ is applied to the forward transform rather than its inverse. Instantaneous mean Fourier coefficients ![]() $\bar {c}_\kappa$ are then obtained by averaging
$\bar {c}_\kappa$ are then obtained by averaging ![]() $\lvert c_\kappa \rvert$ over
$\lvert c_\kappa \rvert$ over ![]() $y$.
$y$.
Time evolution of the full quasi-2-D equations or the linear forward and adjoint systems is computed numerically using a primitive variable spectral element solver (Hussam, Thompson & Sheard Reference Hussam, Thompson and Sheard2012; Cassells et al. Reference Cassells, Vo, Pothérat and Sheard2019; Camobreco et al. Reference Camobreco, Pothérat and Sheard2020; Camobreco, Pothérat & Sheard Reference Camobreco, Pothérat and Sheard2021b). The ![]() $(x,y)$ plane is discretised with quadrilateral elements (12 by 48) featuring polynomial basis functions of order
$(x,y)$ plane is discretised with quadrilateral elements (12 by 48) featuring polynomial basis functions of order ![]() $N_{p}=19$ (Camobreco et al. Reference Camobreco, Pothérat and Sheard2020, Reference Camobreco, Pothérat and Sheard2021b; Camobreco, Pothérat & Sheard Reference Camobreco, Pothérat and Sheard2021a). Time integration is via third-order backward differencing, with operator splitting (Karniadakis, Israeli & Orszag Reference Karniadakis, Israeli and Orszag1991). The time step size is initially set to
$N_{p}=19$ (Camobreco et al. Reference Camobreco, Pothérat and Sheard2020, Reference Camobreco, Pothérat and Sheard2021b; Camobreco, Pothérat & Sheard Reference Camobreco, Pothérat and Sheard2021a). Time integration is via third-order backward differencing, with operator splitting (Karniadakis, Israeli & Orszag Reference Karniadakis, Israeli and Orszag1991). The time step size is initially set to ![]() $\Delta t = 1.25\times 10^{-3}$, and is reduced once turbulence emerges to maintain stability. The initial condition is composed of the laminar base flow and a perturbation computed via linear transient growth optimisation. The domain length is matched to the wavelength minimising the decay rate of the leading eigenmode. The perturbation amplitude is normalised to the energy
$\Delta t = 1.25\times 10^{-3}$, and is reduced once turbulence emerges to maintain stability. The initial condition is composed of the laminar base flow and a perturbation computed via linear transient growth optimisation. The domain length is matched to the wavelength minimising the decay rate of the leading eigenmode. The perturbation amplitude is normalised to the energy ![]() $E_0$ required for each simulation.
$E_0$ required for each simulation.
3. Evidence of subcritical turbulence
The starting point is to determine whether subcritical turbulence in quasi-2-D shear flows exists (for ![]() ${{Re}}<{{Re}}_{c}$). In the subcritical regime, turbulence originates from perturbations that are sufficiently intense to activate nonlinear amplification mechanisms that infinitesimal ones cannot. Unlike 3-D flows, there is evidence that seeding the subcritical laminar shear flow with even high levels of noise does not ignite turbulence (Camobreco et al. Reference Camobreco, Pothérat and Sheard2021b). Seeking turbulence, but not necessarily its most efficient trigger, the laminar state is seeded with optimal transient growth perturbations of different energies, and evolved until the flow either returns to its initial laminar state or becomes turbulent.
${{Re}}<{{Re}}_{c}$). In the subcritical regime, turbulence originates from perturbations that are sufficiently intense to activate nonlinear amplification mechanisms that infinitesimal ones cannot. Unlike 3-D flows, there is evidence that seeding the subcritical laminar shear flow with even high levels of noise does not ignite turbulence (Camobreco et al. Reference Camobreco, Pothérat and Sheard2021b). Seeking turbulence, but not necessarily its most efficient trigger, the laminar state is seeded with optimal transient growth perturbations of different energies, and evolved until the flow either returns to its initial laminar state or becomes turbulent.
Figure 2(a) depicts a representative set of the aforementioned simulations at a Reynolds number ![]() $r_{c}=0.9$. A turbulent state is reached for any normalised initial energy
$r_{c}=0.9$. A turbulent state is reached for any normalised initial energy ![]() $E_0>E_{D}$, where
$E_0>E_{D}$, where ![]() $E_0=E/E_{B}$. Here
$E_0=E/E_{B}$. Here ![]() $E$ is the kinetic energy of the disturbance, and
$E$ is the kinetic energy of the disturbance, and ![]() $E_{B}$ is the energy of the laminar base flow. The delineation energy
$E_{B}$ is the energy of the laminar base flow. The delineation energy ![]() $E_{D}$, found when seeding the flow with optimal perturbations from linear transient growth analysis, lies within
$E_{D}$, found when seeding the flow with optimal perturbations from linear transient growth analysis, lies within ![]() $3.0576\times 10^{-6}< E_{D}<3.0577\times 10^{-6}$. Evidence of a turbulent state is found in the energy spectra
$3.0576\times 10^{-6}< E_{D}<3.0577\times 10^{-6}$. Evidence of a turbulent state is found in the energy spectra ![]() $\bar {c}_\kappa (\kappa )$ of figure 2(b): while low energy states contain energy above the noise floor (around
$\bar {c}_\kappa (\kappa )$ of figure 2(b): while low energy states contain energy above the noise floor (around ![]() $10^{-13}$) in only a few of the lower wavenumber modes, all modes are energised in the turbulent cases (Grossmann Reference Grossmann2000), with an extended inertial range following
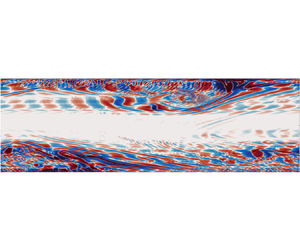
$10^{-13}$) in only a few of the lower wavenumber modes, all modes are energised in the turbulent cases (Grossmann Reference Grossmann2000), with an extended inertial range following ![]() $\bar {c}_\kappa (\kappa )\sim \kappa ^{-5/3}$ (Tabeling Reference Tabeling2002). These features are characteristic of turbulence, a snapshot of which is visualised in figure 2(c). This visualisation employs a streamwise high-pass filter to remove the otherwise occluding large-scale TS wave structures. This filter reveals smaller-scale structures being entrained from the sidewall boundary layers into the channel interior. Instances of this are visible near the bottom wall at the upstream end of the domain, and further downstream near the top wall. The respective locations of these features align with the entrainment regions of the underlying TS wave.
$\bar {c}_\kappa (\kappa )\sim \kappa ^{-5/3}$ (Tabeling Reference Tabeling2002). These features are characteristic of turbulence, a snapshot of which is visualised in figure 2(c). This visualisation employs a streamwise high-pass filter to remove the otherwise occluding large-scale TS wave structures. This filter reveals smaller-scale structures being entrained from the sidewall boundary layers into the channel interior. Instances of this are visible near the bottom wall at the upstream end of the domain, and further downstream near the top wall. The respective locations of these features align with the entrainment regions of the underlying TS wave.

Figure 2. (a) Kinetic energy time history showing the nonlinear evolution of linear optimals at ![]() $r_{c} = 0.9$,
$r_{c} = 0.9$, ![]() $\alpha _{max}$: when
$\alpha _{max}$: when ![]() $E_0< E_{D}$ (blue) the flow visits the edge but relaminarises, whereas it becomes turbulent for
$E_0< E_{D}$ (blue) the flow visits the edge but relaminarises, whereas it becomes turbulent for ![]() $E_0>E_{D}$ (red). (b) Fourier spectra at select instants in time at
$E_0>E_{D}$ (red). (b) Fourier spectra at select instants in time at ![]() $E_0=3.0577\times 10^{-6}>E_{D}$. Dash-dotted line,
$E_0=3.0577\times 10^{-6}>E_{D}$. Dash-dotted line, ![]() $\exp (-3\kappa /2)$ trend. Dashed line,
$\exp (-3\kappa /2)$ trend. Dashed line, ![]() $\kappa ^{-5/3}$ trend. (c) Streamwise high-pass filtered snapshot of spanwise vorticity
$\kappa ^{-5/3}$ trend. (c) Streamwise high-pass filtered snapshot of spanwise vorticity ![]() $\lvert \hat {\omega }_{z,\lvert \kappa \rvert \geq 10}\rvert$ from DNS at
$\lvert \hat {\omega }_{z,\lvert \kappa \rvert \geq 10}\rvert$ from DNS at ![]() $r_{c} = 0.9$,
$r_{c} = 0.9$, ![]() $\alpha _{max}$, indicating quasi-2-D turbulence at
$\alpha _{max}$, indicating quasi-2-D turbulence at ![]() $t=1.1\times 10^4$. (d) Data from (a) re-plotted under the framework of the Stuart–Landau model. The instantaneous growth rate of the perturbation amplitude
$t=1.1\times 10^4$. (d) Data from (a) re-plotted under the framework of the Stuart–Landau model. The instantaneous growth rate of the perturbation amplitude ![]() $A$ is plotted against
$A$ is plotted against ![]() $A^2$. The data exhibits a collapse onto a common curve exhibiting the signature of a subcritical bifurcation, i.e. approaching the eigenmode growth rate
$A^2$. The data exhibits a collapse onto a common curve exhibiting the signature of a subcritical bifurcation, i.e. approaching the eigenmode growth rate ![]() $\mathrm {Im}(\omega )=-5.94084\times 10^{-4}$ as
$\mathrm {Im}(\omega )=-5.94084\times 10^{-4}$ as ![]() $A\rightarrow 0^+$ with a positive gradient. For guidance, the dot-dashed line is tangent to this curve at
$A\rightarrow 0^+$ with a positive gradient. For guidance, the dot-dashed line is tangent to this curve at ![]() $A=0$.
$A=0$.
With the existence of subcritical turbulence in quasi-2-D flows now established, two remarkable features emerge. First, the turbulence is intermittent in time, exhibiting sporadic regressions to a low energy state differing from the original laminar state (figure 2a). This is reminiscent of the spatial intermittency in various 3-D shear flows (Cros & Gal Reference Cros and Gal2002; Barkley & Tuckerman Reference Barkley and Tuckerman2007; Moxey & Barkley Reference Moxey and Barkley2010; Brethouwer, Duguet & Schlatter Reference Brethouwer, Duguet and Schlatter2012; Khapko et al. Reference Khapko, Duguet, Kreilos, Schlatter, Eckhardt and Henningson2014). Second, transition to indefinitely sustained turbulence was only found for ![]() ${{Re}} \gtrsim 0.8{{Re}}_{c}$. Hence the Reynolds numbers required to sustain turbulence are much higher than in 3-D flows. Over
${{Re}} \gtrsim 0.8{{Re}}_{c}$. Hence the Reynolds numbers required to sustain turbulence are much higher than in 3-D flows. Over ![]() $0.4\lesssim r_{c} \lesssim 0.8$, only a single turbulent episode was observed, with finite lifetime proportional to
$0.4\lesssim r_{c} \lesssim 0.8$, only a single turbulent episode was observed, with finite lifetime proportional to ![]() ${{Re}}$.
${{Re}}$.
Verification that the turbulence reported herein originates from a subcritical instability is determined using the Stuart–Landau model (Drazin & Reid Reference Drazin and Reid2004) following the approach detailed in Sapardi et al. (Reference Sapardi, Hussam, Pothérat and Sheard2017), a brief outline of which is explained here. The time history of a measure of the disturbance amplitude ![]() $A(t) = \sqrt {\int _\varOmega \lvert \hat {\boldsymbol {u}}_\perp \rvert ^2\,\mathrm {d}\varOmega }$ is taken, with subcritical bifurcation evolution characterised by an increase in
$A(t) = \sqrt {\int _\varOmega \lvert \hat {\boldsymbol {u}}_\perp \rvert ^2\,\mathrm {d}\varOmega }$ is taken, with subcritical bifurcation evolution characterised by an increase in ![]() $\mathrm {d}(\log A)/\mathrm {d}t$ with increasing amplitude near
$\mathrm {d}(\log A)/\mathrm {d}t$ with increasing amplitude near ![]() $A=0$. Beyond the critical Reynolds number, an infinitesimal disturbance achieves super-exponential growth before saturating. Below the critical Reynolds number, small disturbances decay, while larger-amplitude disturbances grow. This behaviour is observed in figure 2(d). Cases bracketing
$A=0$. Beyond the critical Reynolds number, an infinitesimal disturbance achieves super-exponential growth before saturating. Below the critical Reynolds number, small disturbances decay, while larger-amplitude disturbances grow. This behaviour is observed in figure 2(d). Cases bracketing ![]() $E_{D}$ approach a common curve having the expected subcritical profile; cases with
$E_{D}$ approach a common curve having the expected subcritical profile; cases with ![]() $E< E_{D}$ then decay (
$E< E_{D}$ then decay (![]() $\mathrm {d}(\log A)/\mathrm {d}t<0$,
$\mathrm {d}(\log A)/\mathrm {d}t<0$, ![]() $A\rightarrow 0$), while
$A\rightarrow 0$), while ![]() $E>E_{D}$ cases grow towards turbulence.
$E>E_{D}$ cases grow towards turbulence.
4. Nonlinear Tollmien–Schlichting waves are the tipping point between laminar and turbulent states
Having established that subcritical turbulence exists, we consider the pathway from the laminar base flow to the turbulent state. We now seek edge states. These act as tipping points from which the flow can either revert to its original laminar state, or become turbulent (Skufca, Yorke & Eckhardt Reference Skufca, Yorke and Eckhardt2006). The edge state is reached for the initial perturbation delineation energy ![]() $E_{D}$, separating perturbations triggering turbulence from those decaying. As the initial energy approaches
$E_{D}$, separating perturbations triggering turbulence from those decaying. As the initial energy approaches ![]() $E_{D}$, the edge state persists for a longer duration. Here
$E_{D}$, the edge state persists for a longer duration. Here ![]() $E_{D}$ is found iteratively with a bisection method (Itano & Toh Reference Itano and Toh2001). The edge state from figure 2(a) is visualised in figure 3(a). It consists of a travelling wave of very similar topology to the infinitesimal TS wave, suggesting they may play a role in the quasi-2-D transition to turbulence.
$E_{D}$ is found iteratively with a bisection method (Itano & Toh Reference Itano and Toh2001). The edge state from figure 2(a) is visualised in figure 3(a). It consists of a travelling wave of very similar topology to the infinitesimal TS wave, suggesting they may play a role in the quasi-2-D transition to turbulence.

Figure 3. (a) Flooded contours of spanwise velocity ![]() $\hat {v}_\perp$ from DNS at
$\hat {v}_\perp$ from DNS at ![]() $r_{c} = 0.9$,
$r_{c} = 0.9$, ![]() $\alpha _{max}$, representing the edge state at
$\alpha _{max}$, representing the edge state at ![]() $t=7.48\times 10^3$ overlaid with contour lines showing the linear TS wave
$t=7.48\times 10^3$ overlaid with contour lines showing the linear TS wave ![]() $\hat {v}_{\perp,1,1}$. Both sets of contours are equi-spaced between the minimum and maximum values of the respective fields. (b) Weakly nonlinear modes
$\hat {v}_{\perp,1,1}$. Both sets of contours are equi-spaced between the minimum and maximum values of the respective fields. (b) Weakly nonlinear modes ![]() $\hat {u}_{\perp,0,2}$,
$\hat {u}_{\perp,0,2}$, ![]() $\hat {v}_{\perp,1,1}$,
$\hat {v}_{\perp,1,1}$, ![]() $\hat {v}_{\perp,2,2}$ (dashed black lines) and corresponding Fourier components from DNS at different times (coloured lines) at
$\hat {v}_{\perp,2,2}$ (dashed black lines) and corresponding Fourier components from DNS at different times (coloured lines) at ![]() $\kappa =0$ (streamwise),
$\kappa =0$ (streamwise), ![]() $1$ (spanwise) and
$1$ (spanwise) and ![]() $2$ (spanwise) with
$2$ (spanwise) with ![]() $E_0 = 3.0577\times 10^{-6}>E_{D}$. The solution departs the edge at
$E_0 = 3.0577\times 10^{-6}>E_{D}$. The solution departs the edge at ![]() $t \approx 9\times 10^3$.
$t \approx 9\times 10^3$.
To investigate this possibility, we calculated a weakly nonlinear flow state in which nonlinearities only arise out of combinations of the leading TS wave. The weakly nonlinear equations are a more precise version of the perturbation equations compared with the linearised version used to calculate the leading eigenmode and the perturbation maximising transient growth. They are obtained by approximating the perturbation by the leading eigenmode, and truncating its governing equations to the third order in its amplitude. For example, for a leading eigenmode of amplitude ![]() $\epsilon$, the
$\epsilon$, the ![]() $n\mathrm {th}$ harmonic of the spanwise velocity component is written as
$n\mathrm {th}$ harmonic of the spanwise velocity component is written as
 \begin{equation} \hat{v}_{{\perp},n} = \sum_{m=0}^{\infty}\epsilon^{\lvert n\rvert+2m}\tilde{A}^{\lvert n\rvert}\lvert\tilde{A}\rvert^{2m}\hat{v}_{{\perp},n,\lvert n\rvert+2m}, \end{equation}
\begin{equation} \hat{v}_{{\perp},n} = \sum_{m=0}^{\infty}\epsilon^{\lvert n\rvert+2m}\tilde{A}^{\lvert n\rvert}\lvert\tilde{A}\rvert^{2m}\hat{v}_{{\perp},n,\lvert n\rvert+2m}, \end{equation}
where ![]() $\hat {v}_{\perp,n,\lvert n\rvert +2m}$ denotes a perturbation (
$\hat {v}_{\perp,n,\lvert n\rvert +2m}$ denotes a perturbation (![]() $n$ refers to the harmonic,
$n$ refers to the harmonic, ![]() $\lvert n\rvert +2m$ to the amplitude order) and
$\lvert n\rvert +2m$ to the amplitude order) and ![]() $\tilde {A} = A/\epsilon$ is the normalised amplitude. Nonlinear self-interaction of the linear mode
$\tilde {A} = A/\epsilon$ is the normalised amplitude. Nonlinear self-interaction of the linear mode ![]() $\hat {v}_{\perp,1,1}$ excites a second harmonic
$\hat {v}_{\perp,1,1}$ excites a second harmonic ![]() $\hat {v}_{\perp,2,2}$, which is compared to the
$\hat {v}_{\perp,2,2}$, which is compared to the ![]() $\kappa =2$ harmonic from DNS. Nonlinear interaction between the linear mode
$\kappa =2$ harmonic from DNS. Nonlinear interaction between the linear mode ![]() $\hat {v}_{\perp,1,1}$ and its complex conjugate
$\hat {v}_{\perp,1,1}$ and its complex conjugate ![]() $\hat {v}_{\perp,-1,1}$ generates a modification to the base flow
$\hat {v}_{\perp,-1,1}$ generates a modification to the base flow ![]() $\hat {u}_{\perp,0,2}$, which is compared to the
$\hat {u}_{\perp,0,2}$, which is compared to the ![]() $\kappa =0$ harmonic from DNS. The full equations governing the
$\kappa =0$ harmonic from DNS. The full equations governing the ![]() $n$th harmonic of the base flow and perturbation follow from insertion of this decomposition into (2.1); they are expressed in full in Camobreco et al. (Reference Camobreco, Pothérat and Sheard2021b) and the general method is detailed in Hagan & Priede (Reference Hagan and Priede2013). The full nonlinear evolution of the flow is obtained by solving the system (2.1) numerically.
$n$th harmonic of the base flow and perturbation follow from insertion of this decomposition into (2.1); they are expressed in full in Camobreco et al. (Reference Camobreco, Pothérat and Sheard2021b) and the general method is detailed in Hagan & Priede (Reference Hagan and Priede2013). The full nonlinear evolution of the flow is obtained by solving the system (2.1) numerically.
This technique facilitates a comparison between the fully nonlinear flow state (with all possible modes) obtained from DNS, with its asymptotic approximation to second order in the perturbation amplitude. The streamwise ![]() $\kappa =1$ Fourier mode extracted from DNS is directly compared to the linearly computed modal instability in figure 3(a), showing close agreement.
$\kappa =1$ Fourier mode extracted from DNS is directly compared to the linearly computed modal instability in figure 3(a), showing close agreement.
Figure 3(b) compares velocity profiles from each of the modes accounted for in the weakly nonlinear analysis with the corresponding Fourier components of the same wavelength extracted from the full DNS evolved from the linear optimal with ![]() $\alpha =\alpha _{max}$ with
$\alpha =\alpha _{max}$ with ![]() $E_0$ close to
$E_0$ close to ![]() $E_{D}$. Both the leading eigenmode (
$E_{D}$. Both the leading eigenmode (![]() $\kappa =1$) and its nonlinear interaction (
$\kappa =1$) and its nonlinear interaction (![]() $\kappa =2$) matched their DNS counterpart to high precision in the early stage of evolution. The modulated streamwise-independent (
$\kappa =2$) matched their DNS counterpart to high precision in the early stage of evolution. The modulated streamwise-independent (![]() $\kappa =0$) component exhibited small differences at this stage, that vanished as the influence of our particular choice of initial condition did. Additionally, the cumulated kinetic energy of all three components forming the weakly nonlinear approximation represents over
$\kappa =0$) component exhibited small differences at this stage, that vanished as the influence of our particular choice of initial condition did. Additionally, the cumulated kinetic energy of all three components forming the weakly nonlinear approximation represents over ![]() $93.7\,\%$ of the total energy in the DNS while on the edge. This proves that the build-up of the edge state originates almost exclusively from the dynamics of the TS wave. As such, this transition mechanism differs radically from its counterpart in 3-D flows (Zammert & Eckhardt Reference Zammert and Eckhardt2019), where a bypass transition involving rapidly growing streamwise structures takes place at such low Reynolds numbers that TS waves are too damped to contribute.
$93.7\,\%$ of the total energy in the DNS while on the edge. This proves that the build-up of the edge state originates almost exclusively from the dynamics of the TS wave. As such, this transition mechanism differs radically from its counterpart in 3-D flows (Zammert & Eckhardt Reference Zammert and Eckhardt2019), where a bypass transition involving rapidly growing streamwise structures takes place at such low Reynolds numbers that TS waves are too damped to contribute.
With the pathway to the edge state and then to turbulence now clarified, the question remains as to its robustness against the choice of initial condition. Thus, DNS were performed with the initial conditions chosen as the modes optimising growth at increasingly large times ![]() $T = \tau /\tau _{opt}$ (where
$T = \tau /\tau _{opt}$ (where ![]() $\tau _{opt}$ is the time of optimal growth for a given wavenumber
$\tau _{opt}$ is the time of optimal growth for a given wavenumber ![]() $\alpha$, i.e.
$\alpha$, i.e. ![]() $\tau _{opt}\simeq 31.0$ for
$\tau _{opt}\simeq 31.0$ for ![]() $\alpha =\alpha _{max}$ at
$\alpha =\alpha _{max}$ at ![]() $r_{c}=0.9$). The corresponding delineation energy provides a measure of how easily these transients ignite turbulence and therefore of their role in doing so.
$r_{c}=0.9$). The corresponding delineation energy provides a measure of how easily these transients ignite turbulence and therefore of their role in doing so.
In these simulations, all optimals for a given ![]() $\alpha$ evolved into the same edge state. Further, as
$\alpha$ evolved into the same edge state. Further, as ![]() $T$ increases, the profiles of spanwise velocity of the initial condition optimising growth with
$T$ increases, the profiles of spanwise velocity of the initial condition optimising growth with ![]() $\alpha =\alpha _{max}$ converge toward the leading adjoint mode (figure 4a), with a corresponding monotonic reduction in the delineation energy with increasing
$\alpha =\alpha _{max}$ converge toward the leading adjoint mode (figure 4a), with a corresponding monotonic reduction in the delineation energy with increasing ![]() $T$ (figure 4b). By
$T$ (figure 4b). By ![]() $T=8$, the delineation energy of the optimal matches that of the leading adjoint to approximately
$T=8$, the delineation energy of the optimal matches that of the leading adjoint to approximately ![]() $\pm 0.01\,\%$. Figure 4(b) further shows that these initial conditions lead to reduced transient growth, yet are more efficient at triggering turbulence: that is to say, the delineation energy
$\pm 0.01\,\%$. Figure 4(b) further shows that these initial conditions lead to reduced transient growth, yet are more efficient at triggering turbulence: that is to say, the delineation energy ![]() $E_D$ decreased approximately twofold as the initial condition morphed from the linear optimal mode to the linear adjoint mode. This was found to be consistent when the streamwise wavenumber was varied. Duguet, Brandt & Larsson (Reference Duguet, Brandt and Larsson2010) similarly showed that maximising transient growth does not necessarily imply an easier transition. Hence the leading adjoint eigenmode, which by construction optimally energises the TS wave, is a more efficient initial condition to reach turbulence than any initial condition producing optimal transient growth. Consequently, optimal growth does not favour the transition, unlike in 3-D shear flows where the transient growth associated with the lift-up mechanism is an essential element of the transition process (Reddy et al. Reference Reddy, Schmid, Baggett and Henningson1998; Pringle, Willis & Kerswell Reference Pringle, Willis and Kerswell2012).
$E_D$ decreased approximately twofold as the initial condition morphed from the linear optimal mode to the linear adjoint mode. This was found to be consistent when the streamwise wavenumber was varied. Duguet, Brandt & Larsson (Reference Duguet, Brandt and Larsson2010) similarly showed that maximising transient growth does not necessarily imply an easier transition. Hence the leading adjoint eigenmode, which by construction optimally energises the TS wave, is a more efficient initial condition to reach turbulence than any initial condition producing optimal transient growth. Consequently, optimal growth does not favour the transition, unlike in 3-D shear flows where the transient growth associated with the lift-up mechanism is an essential element of the transition process (Reddy et al. Reference Reddy, Schmid, Baggett and Henningson1998; Pringle, Willis & Kerswell Reference Pringle, Willis and Kerswell2012).

Figure 4. (a) Comparison between linear transient growth initial conditions with various ![]() $\tau =T\tau _{opt}$ (solid lines) and the leading adjoint mode (dashed lines), horizontally shifted for clarity. (b) Delineation energies from DNS and energy growth ratios from linear analysis for each
$\tau =T\tau _{opt}$ (solid lines) and the leading adjoint mode (dashed lines), horizontally shifted for clarity. (b) Delineation energies from DNS and energy growth ratios from linear analysis for each ![]() $T$.
$T$.
The same procedures applied at lower ![]() $r_{c}$ produced the same reduction in delineation energy with increasing
$r_{c}$ produced the same reduction in delineation energy with increasing ![]() $T$ and exhibited edge states independent of
$T$ and exhibited edge states independent of ![]() $T$. For
$T$. For ![]() $0.3 \lesssim r_{c} \lesssim 0.8$, after departing the edge, a secondary stable state formed (Jiménez Reference Jiménez1990; Falkovich & Vladimirova Reference Falkovich and Vladimirova2018), again independent of the initial condition.
$0.3 \lesssim r_{c} \lesssim 0.8$, after departing the edge, a secondary stable state formed (Jiménez Reference Jiménez1990; Falkovich & Vladimirova Reference Falkovich and Vladimirova2018), again independent of the initial condition.
5. Discussion and concluding remarks
In conclusion, subcritical turbulence exists in quasi-2-D shear flows and can be reached directly, rather than via an intermediate 3-D state. The 2-D transition mechanism bears important similarities with its 3-D cousin: it is ignited by a perturbation of finite amplitude and first reaches an edge state that is seemingly independent of this initial perturbation. The edge state subsequently breaks down into a turbulent state if the initial perturbation energy exceeds the delineation energy ![]() $E_{D}$ for that particular perturbation. As in the 3-D problem, the attained turbulence is not yet fully developed (Wygnanski & Champagne Reference Wygnanski and Champagne1973; Wygnanski, Sokolov & Friedman Reference Wygnanski, Sokolov and Friedman1975). Departure from fully established turbulence in quasi-2-D shear flows expresses as time intermittency, with sporadic retreats to a low energy state different from the base laminar flow.
$E_{D}$ for that particular perturbation. As in the 3-D problem, the attained turbulence is not yet fully developed (Wygnanski & Champagne Reference Wygnanski and Champagne1973; Wygnanski, Sokolov & Friedman Reference Wygnanski, Sokolov and Friedman1975). Departure from fully established turbulence in quasi-2-D shear flows expresses as time intermittency, with sporadic retreats to a low energy state different from the base laminar flow.
Conversely, the subcritical transition in quasi-2-D shear flows exhibits specificities that distinguish it sharply from the 3-D one. Chiefly, the lift-up mechanism that underpins transitions in 3-D can be ignited at criticality so low that the TS waves are strongly suppressed by the linear dynamics, despite being the least damped infinitesimal perturbation. Thus, they are not observed in the 3-D transition. In quasi-2-D flows by contrast, the 3-D mechanism is absent and our study demonstrates that the dynamics is dominated by the TS waves, with the edge state resulting directly from their weakly nonlinear evolution. In a quasi-2-D flow, the TS wave instability directly connects the base flow to turbulence via a subcritical bifurcation, in stark contrast to a 3-D flow in which the saddle-node bifurcation is disconnected from the base state (Khapko et al. Reference Khapko, Duguet, Kreilos, Schlatter, Eckhardt and Henningson2014). This may also explain why transition in quasi-2-D flows is relatively weakly subcritical: at lower ![]() $r_{c}$, TS waves are so strongly linearly damped that their nonlinear growth is stifled.
$r_{c}$, TS waves are so strongly linearly damped that their nonlinear growth is stifled.
This new transition mechanism reopens many questions resolved in the 3-D case: How does the intermittency or localisation of the turbulence evolve into the supercritical regime, e.g. following the mechanism outlined by Mellibovsky & Meseguer (Reference Mellibovsky and Meseguer2015)? Does the transition to the fully turbulent state obey a second-order phase transition of the universality class of directed percolations as for other shear flows (Lemoult et al. Reference Lemoult, Shi, Avila, Jalikop, Avila and Hof2016)? While the thermodynamic formalism used by Wang, Li & Weinan (Reference Wang, Li and Weinan2015) indicates that two-dimensional Poiseuille flow loses stability in a manner consistent with a continuous phase transition, in the quasi-2-D case linear friction may impact the development and interaction of unstable travelling waves, and so this remains an open question. Separately, much remains to be discovered on the subcritical response to finite amplitude perturbations: How does the delineation energy vary with criticality, especially considering the relatively short subcritical range in which turbulence can be sustained? Can this subcritical response be manipulated to prevent or promote turbulence (for example, to enhance heat transfer in the heat exchangers of plasma fusion reactors)? These questions call for expensive numerical simulations, but also for experiments with well-controlled perturbations, since this first evidence of subcritical transitions in quasi-2-D shear flows is currently purely numerical.
While we have established a scenario for transition involving a purely 2-D mechanism, 3-D mechanisms could still compete with this scenario and trigger a subcritical 3-D transition. Whether one of the other scenarios dominates cannot be determined on the basis of (2.1) only as the 3-D mechanisms are specific to the physical process promoting the emergence of quasi-2-D dynamics. As such, whether a purely quasi-2-D subcritical transition to turbulence can be observed in practice remains to be determined in particular cases. The question could be addressed either with 3-D numerical methods or experiments on magnetohydrodynamics or rotating flows, or in Hele-Shaw cells, for example.
Acknowledgements
The authors thank Dr S. Horn and Dr C. Pringle for their helpful feedback.
Funding
C.J.C. was supported by the Australian Government Research Training Program (RTP). This research was supported by Australian Research Council Discovery Grant DP180102647 and Royal Society International Exchanges Grant IE170034. Computations were possible thanks to the National Computational Infrastructure (NCI), Pawsey Supercomputing Centre, and the Monash e-Research Centre.
Declaration of interest
The authors report no conflict of interest.