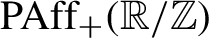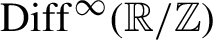Distortion in the group of circle homeomorphisms

Abstract

Let G be the group $\text {PAff}_{+}(\mathbb R/\mathbb Z)$ of piecewise affine circle homeomorphisms or the group ${\operatorname {\mathrm {Diff}}}^{{\kern1pt}\infty }(\mathbb R/\mathbb Z)$ of smooth circle diffeomorphisms. A constructive proof that all irrational rotations are distorted in G is given.

1 Introduction

Let G be a group with some finite generating set $\mathcal G$ . We define the metric $d_{\mathcal G}$ on G by taking $d_{\mathcal G} (g_{1}, g_{2})$ to be the infimum over all $k\ge 0$ such that there exist $f_{1},\ldots , f_{k}\in \mathcal G$ and $\epsilon _{1},\ldots ,\epsilon _{k}\in \{-1, 1\}$ satisfying $g_{2}=f_{1}^{\epsilon _{1}}\cdots f_{k}^{\epsilon _{k}}g_{1}$ .

Now let H be an arbitrary group. An element $f\in H$ is called distorted in H if there exists a finitely generated subgroup $G\subset H$ containing f such that

$$ \begin{align*} \lim_{n\to\infty}\frac{d_{\mathcal G}(f^{n}, \text{id})}{n}=0 \end{align*} $$

for some (and hence every) generating set $\mathcal G$ . Since the limit always exists, it is enough to verify it for some subsequence. The notion of distortion comes from geometric group theory and was introduced by Gromov in [7].

The problem of the existence of distorted elements in some groups of homeomorphisms has been intensively studied for many years (see [2, 3–6, 8, 10, 11]). Substantial progress has been achieved for groups of diffeomorphisms of manifolds. In particular, Avila [1] proved that rotations with irrational rotation number are distorted in the group of smooth diffeomorphisms of the circle. In this note we give a constructive proof that all irrational rotations are distorted both in the group of piecewise affine circle homeomorphisms, $\text {PAff}_{+}({\mathbb R/\mathbb Z})$ , and in the group of smooth circle diffeomorphisms, ${\operatorname {\mathrm {Diff}}}^{{\kern1pt}\infty }(\mathbb R/\mathbb Z)$ . The result gives an answer to Question 11 in [9] (see also Question 2.5 in [5]). So far it has not even been known whether there exist distorted elements in $\text {PAff}_{+}(\mathbb R/\mathbb Z)$ . Now from [8] it follows that each distorted element is conjugate to a rotation.

From now on let G be either $\text {PAff}_{+}(\mathbb R/\mathbb Z)$ or ${\operatorname {\mathrm {Diff}}}^{{\kern1pt}\infty }(\mathbb R/\mathbb Z)$ . We say that $g\in G$ is trivial on some set if there exists a non-empty open set $I\subset [0,1)$ such that $g(x)=x$ for $x\in I$ . The set of all homeomorphisms in G which are trivial on some set will be denoted by $G_{\text {triv}}$ . By $\text {T}$ we denote the set of all rotations, and let $T_{\alpha }$ be the rotation with rotation number $\alpha $ .

This paper is devoted to the proof of the following theorem.

Theorem. All irrational rotations are distorted in G.

2 Proofs

We first formulate two lemmas and deduce the theorem. The proofs of the lemmas will be given at the end of the paper.

Lemma 1. For any irrational rotation $T_{\alpha }$ and $g\in G_{\text {triv}}\cup \text {T}$ there exist a finite generating set $\mathcal G_{g}\subset G$ and a constant $C>0$ such that

$$ \begin{align*} d_{\mathcal G_{g}}(T_{\alpha}^{n} g T_{\alpha}^{-n}, \textrm{id})\le C\log n\quad\text{for all }n\ge 1. \end{align*} $$

Lemma 2. In G there exist $g_{1},\ldots , g_{l}\in G_{\text {triv}}\cup \text {T}$ and $k, k_{1},\ldots , k_{l}\in {\mathbb Z}$ with $k\neq k_{1}+\cdots +k_{l}$ , such that for each sufficiently small $\beta>0$ the element $x=T_{\beta }$ satisfies

(1) $$ \begin{align} x^{k_{1}}g_{1}x^{k_{2}}g_{2}\cdots x^{k_{l}}g_{l}=x^{k}. \end{align} $$

Proof of the theorem

Fix an irrational rotation $T_{\alpha }$ . From Lemma 2 it follows that in G there exists an equation of the form (1) such that $x=T_{\beta }$ , for all sufficiently small $\beta $ , is its solution. Let $\mathcal G=\mathcal G_{g_{1}}\cup \cdots \cup \mathcal G_{g_{l}}$ , where $\mathcal G_{g_{i}}$ , $i=1,\ldots , l$ , are finite generating sets derived from Lemma 1 for $T_{\alpha }$ . We may rewrite equation (1) in the form

(2) $$ \begin{align} x^{k_{1}}g_{1}x^{-k_{1}} x^{k_{2}+k_{1}}g_{2}x^{-k_{2}-k_{1}}\cdots x^{k_{1}+\cdots+k_{l}}g_{l} x^{-k_{1}-\cdots-k_{l}}=x^{k-k_{1}-\cdots-k_{l}}. \end{align} $$

Let $\beta _{0}$ be a positive constant such that $x=T_{\beta }$ for $\beta \in (0, \beta _{0})$ satisfies (2). Set $m:=k-k_{1}-\cdots -k_{l}$ , and let $(n_{i})$ be an increasing sequence of integers such that $n_{i}\alpha \in (0, \beta _{0})\, (\text {mod}\, 1)$ . From Lemma 1 it follows that

$$ \begin{align*} d_{\mathcal G}(T_{\alpha}^{n_{i}(k_{1}+\cdots+k_{j})} g_{j} T_{\alpha}^{-n_{i}(k_{1}+\cdots+k_{j})}, \text{id})\le C_{j}\log n_{i}\quad\text{for all }i\ge 1\text{ and }j=1,\ldots, l. \end{align*} $$

Since $x=T_{n_{i}\alpha }$ satisfies (2), we obtain

$$ \begin{align*} d_{\mathcal G}(T_{\alpha}^{n_{i} m}, \text{id})\le \sum_{j=1}^{l} C_{j}\log n_{i}:= C \log n_{i}\quad\text{for all }i\ge 1\text{.} \end{align*} $$

Hence

$$ \begin{align*} \lim_{n\to\infty}\frac{d_{\mathcal G}(T_{\alpha}^{n}, \text{id})}{n}= \lim_{i\to\infty}\frac{d_{\mathcal G}(T_{\alpha}^{n_{i} m}, \text{id})}{n_{i} m}\le \frac{C}{m}\lim_{i\to\infty} \frac{\log n_{i}}{n_{i}}=0 \end{align*} $$

and the proof is complete.

Proof of Lemma 1

The proof relies on the observation that for a given interval $I\subset (0, 1)$ there exists a finite generating set $\mathcal G\subset G$ such that for any $n\ge 1$ there exists a homeomorphism $h_{n}$ with $d_{\mathcal G}(h_{n}, \text {id})\le C\log n$ for some constant $C>0$ independent of n, and $h_{n}(x)=T_{\alpha }^{n}(x)$ for $x\notin I$ . Without loss of generality we may assume that $I=(a, 1).$ Let $m\ge 1$ be an integer such that $a+2/m<1$ . Let $h\in G $ be any homeomorphism such that $h(x)=x/2$ for $x\in [0, a+2/m)$ , and let $r(x)=x+1/m$ .

We shall define $h_{n}$ by induction. Set $h_{0}=\text {id}$ . If n is odd we put $h_{n}=T_{\alpha } h_{n-1}$ . If n is even, we take $s_{n}:=h_{n/2} h$ and observe that $s_{n}((0, a))=(n\alpha /2, a/2+n\alpha /2)$ . Let $k\in \{1,\ldots , m\}$ be such that $n\alpha /2+k/m\in [0, 1/m)$ (mod $1$ ). Then $r^{k} s_{n}((0, a))\subset (0, a/2+1/m)$ . Therefore

(3) $$ \begin{align} h^{-1} r^{k} h_{n/2} h(x)=2(x/2+n\alpha/2+k/m)=x+n\alpha+2k/m=T_{\alpha}^{n}(x)+2k/m \end{align} $$

for $x\in (0, a)$ . Put $h_{n}:=r^{-2k}h^{-1} r^{k} h_{n/2} h$ , and let $\mathcal G:=\{T_{\alpha }, h, r\}$ . Note that

$$ \begin{align*} d_{\mathcal G}(h_{n}, \text{id})\le 3m+3+d_{\mathcal G}(h_{\lfloor n/2\rfloor}, \text{id}). \end{align*} $$

Thus we obtain $d_{\mathcal G}(h_{n}, \text {id})\le C\log n$ . Finally, observe that for any $g\in G_{\text {triv}}$ such that $g(x)=\text {id}$ on I we have

(4) $$ \begin{align} T_{\alpha}^{n} g T_{\alpha}^{-n}=h_{n} g h_{n}^{-1}. \end{align} $$

Indeed, from (3) and the definition of $h_{n}$ and r it follows that $h_{n}(x)=T_{\alpha }^{n}(x)$ for $x\in (0, a)$ , and

(5) $$ \begin{align} h_{n}((0, a))=T_{\alpha}^{n}((0, a))=(n\alpha, a+n\alpha). \end{align} $$

Therefore, we have

$$ \begin{align*} h_{n}^{-1}(x)=T_{\alpha}^{-n}(x)\in (0, a)\quad\text{for }x\in (n\alpha, a+n\alpha). \end{align*} $$

Since $g(x)=x$ for $x\in (a, 1)$ and g is a homeomorphism, we have $g((0, a))=(0, a)$ .

To justify equality (4), first fix $x\in (n\alpha , a+n\alpha )$ . Then we have

$$ \begin{align*} h_{n}^{-1}(x)=T_{\alpha}^{-n}(x)\in (0, a) \end{align*} $$

and

$$ \begin{align*} (gh_{n}^{-1})(x)=(gT_{\alpha}^{-n})(x)\in (0, a). \end{align*} $$

Consequently, we obtain

$$ \begin{align*} h_{n}gh_{n}^{-1}(x)=T_{\alpha}^{n}gT_{\alpha}^{-n}(x)\quad\text{for }x\in (n\alpha, a+n\alpha), \end{align*} $$

by the fact that $h_{n}(x)=T_{\alpha }^{n}(x)$ for $x\in (0, a)$ . On the other hand, if $x\notin (n\alpha , a+n\alpha )$ , from (5) and the fact that $T_{\alpha }^{n}$ and $h_{n}$ are homeomorphisms, we obtain

$$ \begin{align*} T_{\alpha}^{-n}(x)\in (a, 1]\quad\text{and}\quad h_{n}^{-1}(x)\in(a, 1]. \end{align*} $$

Since $g(x)=x$ for $x\in (a, 1]$ , we have

$$ \begin{align*} (T_{\alpha}^{n}gT_{\alpha}^{-n})(x)=(T_{\alpha}^{n}T_{\alpha}^{-n})(x)=x \end{align*} $$

and

$$ \begin{align*} (h_{n} g h_{n}^{-1})(x)=(h_{n} h_{n}^{-1})(x)=x. \end{align*} $$

Thus equality (4) holds true.

Finally, we obtain

$$ \begin{align*} d_{\mathcal G}(T_{\alpha}^{n} g T_{\alpha}^{-n}, \text{id})\le C\log n. \end{align*} $$

In the case where g is a rotation the conclusion of the lemma is obvious.

Proof of Lemma 2

Let $\beta \in (0, 10^{-3})$ , and let $f_{1}\in G_{\text {triv}}$ be arbitrary such that

$$ \begin{align*} f_{1}(x)=0.4+2(x-0.4) \text{ for }x\in [0.4, 0.6]\quad\text{and}\quad f_{1}(x)=x \text{ for }x\in [0.9, 1.1]. \end{align*} $$

Set

$$ \begin{align*} H_{1}=T_{2\beta}^{-1} f_{1} T_{2\beta} f_{1}^{-1}. \end{align*} $$

It is obvious that

$$ \begin{align*} H_{1}(x)=x+2\beta \text{ for }x\in [0.41, 0.79]\quad\text{and}\quad H_{1}(x)=x \text{ for }x\in [0.91, 1.09]. \end{align*} $$

Define

$$ \begin{align*} H_{2}=T_{1/2} H_{1}^{-1} T_{1/2} H_{1}, \end{align*} $$

and observe that

$$ \begin{align*} H_{2}(x)=x-2\beta\quad\text{for }x\in [0.95, 1]. \end{align*} $$

Simple computation gives

$$ \begin{align*} T_{1/2} H_{2}T_{1/2} H_{2}=\text{id}. \end{align*} $$

Set

$$ \begin{align*} H_{3} =T_{2\beta} H_{2}. \end{align*} $$

Then we have

$$ \begin{align*} H_{3}(x)=x\quad\text{for }x\in [0.95, 1] \end{align*} $$

and

(6) $$ \begin{align} T_{2\beta+1/2}H_{3} T_{-2\beta-1/2} H_{3}=T_{4\beta}. \end{align} $$

Take an arbitrary $f_{2}\in G_{\text {triv}}$ satisfying

$$ \begin{align*} f_{2}(x)=2x\quad\text{for }x\in [0, 0.49], \end{align*} $$

and define

$$ \begin{align*} H_{4}=f_{2}^{-1} H_{3}f_{2}. \end{align*} $$

It is easy to see that

$$ \begin{align*} H_{4}(x)= \begin{cases} H_{3}(2x)/2 & \text{for } x\in [0, 1/2),\\ x & \text{for } x\in [1/2, 1). \end{cases} \end{align*} $$

Let

(7) $$ \begin{align} H_{5}=T_{1/2} H_{4} T_{1/2}H_{4}. \end{align} $$

Observe that the graph of $H_{5}$ is built from two scaled copies of $H_{3}$ , that is,

$$ \begin{align*} H_{5}(x)= \begin{cases} H_{3}(2x)/2 & \text{for } x\in [0, 1/2),\\ H_{3}(2x-1)/2+1/2 & \text{for } x\in [1/2, 1). \end{cases} \end{align*} $$

Therefore, by (6) and (7), we finally obtain

(8) $$ \begin{align} T_{\beta+1/4}H_{5} T_{-\beta-1/4}H_{5}=T_{2\beta}. \end{align} $$

Indeed, this is easy to see if we realize that (8) is simply equation (6) rewritten in the new coordinates $(x/2, y/2)$ . Subsequently plugging $H_{5}, H_{4}, H_{3}, H_{2}$ and $H_{1}$ into formula (8), we have

$$ \begin{align*} \begin{aligned} &T_{\beta} T_{1/4}T_{1/2} f_{2}^{-1} T_{\beta}^{2} T_{1/2} f_{1}T_{\beta}^{-2} f_{1}^{-1} T_{\beta}^{2} T_{1/2} T_{\beta}^{-2} f_{1} T_{\beta}^{2} f_{1}^{-1}f_{2} T_{1/2}f_{2}^{-1} T_{\beta}^{2} T_{1/2} f_{1}T_{\beta}^{-2}\\[3pt] &\cdot f_{1}^{-1} T_{\beta}^{2} T_{1/2} T_{\beta}^{-2} f_{1} T_{\beta}^{2} f_{1}^{-1}f_{2} T_{\beta}^{-1} T_{-1/4}T_{1/2} f_{2}^{-1} T_{\beta}^{2} T_{1/2} f_{1}T_{\beta}^{-2} f_{1}^{-1} T_{\beta}^{2} T_{1/2} T_{\beta}^{-2} f_{1} T_{\beta}^{2} \\[3pt] &\cdot f_{1}^{-1}f_{2} T_{1/2}f_{2}^{-1} T_{\beta}^{2} T_{1/2} f_{1}T_{\beta}^{-2} f_{1}^{-1} T_{\beta}^{2} T_{1/2} T_{\beta}^{-2} f_{1} T_{\beta}^{2} f_{1}^{-1}f_{2}=T_{\beta}^{2}. \end{aligned} \end{align*} $$

Since $\beta \in (0, 10^{-3})$ was arbitrary, we obtain that each $T_{\beta }$ sufficiently small satisfies equation (1) with the functions $g_{1},\ldots , g_{l}\in \{f_{1}, f_{2}, f_{1}^{-1}, f_{2}^{-1}, T_{1/2}, T_{-1/2}, T_{1/4}, T_{-1/4}\}\subset G_{\text {triv}}\cup \text {T}$ and $k_{1},\ldots , k_{l}\in {\mathbb Z}$ . Obviously, some of the $k_{i}$ are equal to $0$ ( $k_{2}$ , for instance) but $k_{1}+\cdots +k_{l}=8$ . Since $k=2$ , the proof of the lemma is complete.