1 Introduction
The fluid dynamics of underwater propulsion is an area of research that has stimulated vigorous collaborations between biologists and engineers, and fostered numerous mathematical treatments, experimental investigations and numerical simulations. It has contributed to a deeper understanding of the underlying biology of fish and aquatic mammals, and provided inspiration for developing innovative new underwater systems. This level of attention is no doubt driven at least in part by the grace and beauty displayed by many swimming animals. There is also the possibility that evolution has led to propulsive mechanisms that are inherently more efficient, manoeuvrable and quieter than those currently in use in marine applications.
The literature surrounding this field is extensive. The books by Gray (Reference Gray1968), Blake (Reference Blake1983a ) and Videler (Reference Videler1993) provide a comprehensive portrait of fish swimming, while more specific topics, such as the hydrodynamics and energetics of fish propulsion, dolphin hydrodynamics and performance, fish swimming mechanics and behaviour in altered flows, passive and active flow control by swimming fishes and mammals and the mechanics and control of swimming, are considered by Webb (Reference Webb1975), Fish & Rohr (Reference Fish and Rohr1999), Colgate & Lynch (Reference Colgate and Lynch2004), Fish & Lauder (Reference Fish and Lauder2006) and Liao (Reference Liao2007), respectively, and Lauder & Tytell (Reference Lauder and Tytell2005) present a particularly insightful review of the hydrodynamics of undulatory propulsion. The analyses by Lighthill (Reference Lighthill1969) for slender bodies and Wu (Reference Wu1961) for thin flexible membranes continue to be influential, as do the considerations on hydrodynamic scaling by Triantafyllou, Triantafyllou & Yue (Reference Triantafyllou, Triantafyllou and Yue2000) and Triantafyllou et al. (Reference Triantafyllou, Hover, Techet and Yue2005). There are also three notable popular articles that have helped introduce a broad audience to the subject (Webb Reference Webb1984; Triantafyllou & Triantafyllou Reference Triantafyllou and Triantafyllou1995; Fish & Lauder Reference Fish and Lauder2013). This is by no means a comprehensive list.
Our aim here is to supplement this body of work with a contemporary perspective on our understanding of the fundamental fluid dynamic aspects of oscillatory and undulatory swimming, and to consider the implications for novel propulsors for future underwater vehicles. We will primarily consider motions at cruise condition, where the animal is moving in a straight line at an average speed that is constant. This is expected to be the most efficient condition, in that it is the mode chosen by fish for sustained swimming (Fish & Rohr Reference Fish and Rohr1999; Triantafyllou et al. Reference Triantafyllou, Hover, Techet and Yue2005).
1.1 Swimming styles
The biology is evidently rich with complexity, encompassing a vast range of body morphologies and swimming styles. A primary sorting can be made on the basis of Reynolds number. When viscous forces dominate, as they do for micro-organisms such as bacteria, propulsion requires non-reciprocal motion that breaks symmetry as a consequence of the linearity of the governing equations. Very small fish, with lengths of a millimetre or so, also live in this world. However, for somewhat larger fish, say of length 10 mm, swimming at one body length per second, the Reynolds number will exceed 100, and reciprocal motions become the dominant form of propulsion.
For such organisms, we can identify four major types of swimmers: undulatory, oscillatory, pulsatile and drag-based, as illustrated in figure 1. Undulatory swimmers are animals that generate a travelling wave along their body or propulsive fins to push fluid backwards. Examples include eels, lampreys and some rays. Oscillatory swimmers, such as salmon, tuna, dolphins and sharks, propel themselves primarily using a semi-rigid caudal fin or fluke that is oscillated periodically. Animals that periodically ingest a volume of water and then discharge it impulsively to produce thrust by reaction are called pulsatile swimmers, and examples include jellyfish, squid, frogfish and some molluscs (Fish Reference Fish1987; Dabiri Reference Dabiri2009). Finally, drag-based swimmers such as humans, turtles, seals and ducks propel a bluff body such as a rigid flipper through the water to generate thrust (Fish Reference Fish1996).
Our principal consideration here will be undulatory and oscillatory swimmers that use body and/or caudal fin locomotion. In the conventional view, anguilliform swimmers such as eels and lampreys pass a travelling wave of increasing amplitude along the whole body, whereas for thunniform swimmers such as tuna and mackerel the anterior part of the body is held to be relatively stiff, and only the posterior third of the body is used for propulsion, with the notable presence of a high-aspect-ratio caudal fin (Lindsey Reference Lindsey, Hoar and Randall1978; Sfakiotakis, Lane & Davies Reference Sfakiotakis, Lane and Davies1999). We shall see that this distinction is not entirely accurate, and also less useful than one based on the wavelength of actuation, in that, broadly speaking, undulatory swimmers display a wavelength shorter than their body length, and oscillatory swimmers display a wavelength longer than their body length.

Figure 1. Examples of four swimming types: (a) oscillatory – tuna; (b) undulatory – ray; (c) pulsatile jet – jellyfish; and (d) drag based – duck. Reproduction with permission from Van Buren, Floryan & Smits (Reference Van Buren, Floryan, Smits, Daniel and Soboyejo2019a ).
1.2 Wake structure
The hydrodynamic structure of the wake is determined by the Reynolds number, the non-dimensional frequency and the non-dimensional amplitude of the motion. The non-dimensional frequency is typically expressed either as the Strouhal number
![]() $St$
, or the reduced frequency
$St$
, or the reduced frequency
![]() $k$
. Here,
$k$
. Here,
![]() $St=2af/U$
and
$St=2af/U$
and
![]() $k=fc/U$
, where
$k=fc/U$
, where
![]() $a$
is the amplitude of oscillation of the tail,
$a$
is the amplitude of oscillation of the tail,
![]() $f$
is the frequency of oscillation (Hz),
$f$
is the frequency of oscillation (Hz),
![]() $U$
is the speed of swimming and
$U$
is the speed of swimming and
![]() $c$
is a characteristic length such as the length of the body or the chord of the caudal fin or fluke.
$c$
is a characteristic length such as the length of the body or the chord of the caudal fin or fluke.
Triantafyllou, Triantafyllou & Grosenbaugh (Reference Triantafyllou, Triantafyllou and Grosenbaugh1993) analysed the swimming performance of a wide variety of fishes and mammals, from bream to sharks to dolphins, and found that they swam broadly within the range of Strouhal numbers from 0.2 to 0.35. This observation echoes a number of earlier studies (for example, Bainbridge Reference Bainbridge1958) that showed that fish tend to increase their swimming speed by increasing their tail beat frequency while keeping their amplitude of motion more or less constant (typically 15–20 % or so of the body length), in effect keeping their Strouhal number fixed. Similar results were found for cetacean swimming by Fish & Rohr (Reference Fish and Rohr1999) (see figure 2), although for rainbow trout Webb, Kostecki & Stevens (Reference Webb, Kostecki and Stevens1984) found that the amplitude of the tail beat relative to the body size decreased with the size of the specimen.

Figure 2. (a) Fluke-beat frequency and (b) non-dimensional fluke-beat amplitude as functions of length-specific swimming speed for several odontocete cetaceans. Original data from Rohr & Fish (Reference Rohr and Fish2004), replotted as in Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a ), reproduced with permission.
Triantafyllou et al. (Reference Triantafyllou, Triantafyllou and Grosenbaugh1993) made two additional observations. First, the efficiency of a heaving and pitching foil displayed a peak in this ‘optimal’ range (
![]() $0.2\leqslant St\leqslant 0.35$
). Second, the average velocity profile in the wake resembles a jet, and they showed that the jet profile is convectively unstable over a narrow range of frequencies that corresponds well to this Strouhal number range. Triantafyllou et al. (Reference Triantafyllou, Triantafyllou and Grosenbaugh1993) therefore concluded that fish swim in this particular Strouhal number range because it corresponds to the frequency of maximum amplification and therefore it is expected to be most efficient. In addition, the associated wake structure resembled a reverse von Kármán street pattern (Weihs Reference Weihs1972), that is, a pattern akin to the von Kármán vortex street but with the signs of the vortices reversed so that thrust is produced instead of drag. No Reynolds number effects were considered, presumably because the Reynolds numbers for their data set were relatively high (
$0.2\leqslant St\leqslant 0.35$
). Second, the average velocity profile in the wake resembles a jet, and they showed that the jet profile is convectively unstable over a narrow range of frequencies that corresponds well to this Strouhal number range. Triantafyllou et al. (Reference Triantafyllou, Triantafyllou and Grosenbaugh1993) therefore concluded that fish swim in this particular Strouhal number range because it corresponds to the frequency of maximum amplification and therefore it is expected to be most efficient. In addition, the associated wake structure resembled a reverse von Kármán street pattern (Weihs Reference Weihs1972), that is, a pattern akin to the von Kármán vortex street but with the signs of the vortices reversed so that thrust is produced instead of drag. No Reynolds number effects were considered, presumably because the Reynolds numbers for their data set were relatively high (
![]() $10^{4}$
to about
$10^{4}$
to about
![]() $10^{6}$
based on body length). However, as we will show, viscous effects may continue be important even at very high Reynolds numbers (see § 3.1).
$10^{6}$
based on body length). However, as we will show, viscous effects may continue be important even at very high Reynolds numbers (see § 3.1).
This view, that the reverse von Kármán street wake pattern corresponds to the most efficient swimming mode, and that it is typical of cruising fish, is still widely held. At the time, however, evidence for the ubiquity in nature of the reverse von Kármán street wake structure was rather scant, and it was not really until the advent of particle image velocimetry (PIV) that the vortex patterns produced by fish could be examined in detail (Müller et al. Reference Müller, Van Den Heuvel, Stamhuis and Videler1997; Drucker & Lauder Reference Drucker and Lauder2001; Lauder & Tytell Reference Lauder and Tytell2005). An early example showing the formation of a reverse von Kármán street wake, as visualized in the plane of the tail motion, is shown in figure 3. This type of structure is also called 2S, that is, two vortices are shed into the wake per shedding cycle, after Williamson & Roshko (Reference Williamson and Roshko1988) who coined the phrase with respect to wakes generated by oscillating cylinders.

Figure 3. Representative flow fields in the wake of the oscillating caudal fin of sunfish during steady swimming at 1.1
![]() $L/s$
, where
$L/s$
, where
![]() $L$
is total body length. Adapted with permission from Drucker & Lauder (Reference Drucker and Lauder2001).
$L$
is total body length. Adapted with permission from Drucker & Lauder (Reference Drucker and Lauder2001).
A different example is given in figure 4(a) for an American eel in steady swimming. Large vortices are shed by the flapping tail, but when viewed in the plane of the tail motion this wake pattern does not resemble that of a reverse von Kármán street. The wake of a robotic lamprey, another undulatory swimmer, shown in figure 4(b), displays a very similar vortex pattern, as do alligators (Fish & Lauder Reference Fish and Lauder2013), and although dolphins typically generate 2S wakes they also sometimes generate this type of wake (Fish et al. Reference Fish, Legac, Williams and Wei2014). We see a structure that is more reminiscent of Williamson and Rosko’s 2P wake structure, where two vortex pairs are shed per cycle. It is clear that different wake patterns are possible, and we shall see that efficient swimming is not necessarily restricted to one particular wake pattern.

Figure 4. Phase-averaged velocity fields in the wake of (a) an American eel moving at constant speed, adapted with permission from Tytell & Lauder (Reference Tytell and Lauder2004), and (b) a robotic lamprey, adapted with permission from Hultmark, Leftwich & Smits (Reference Hultmark, Leftwich and Smits2007). Contours give levels of spanwise vorticity. The views represent the flow field at a similar phase in the motion.
These visualizations in the plane of the tail motion tend to ignore the fact that fish wakes will naturally be highly three-dimensional, even if the caudal fin has a large aspect ratio. As anticipated by Lighthill (Reference Lighthill1969), the spanwise vorticity shed from the central part of the caudal fin is much greater than what can be shed from the tapered tips, and so the vortex lines shed from the central part must bend downstream. Further downstream, they must turn inwards, and then close up when they reach the vorticity of opposite sign shed by the fin half a period earlier. This line of thinking suggests that the wake consists of a series of vortex rings or loops of alternate sign, and that the reverse von Kármán street is seen only in a particular cross-section through this structure (see also Videler Reference Videler1993). Nauen & Lauder (Reference Nauen and Lauder2002) make this description more explicit by direct observations on chub mackerel at Strouhal numbers of approximately 0.4. Thus, the stability argument advanced by Triantafyllou et al. (Reference Triantafyllou, Triantafyllou and Grosenbaugh1993) for two-dimensional flows is unlikely to translate directly to actual fish wakes.
In addition, we should note that for a free swimming fish moving at constant speed no resultant force is acting, and the overall wake in the frame of the fish motion is momentumless. So even if the cross-sectional view of the wake resembles a 2S or a 2P structure the vortices appear aligned in such a way so that there is no net axial jet. This is illustrated by the wakes shown in figure 4(a,b), visualized under free swimming conditions.
We will show here that we should treat the wake structure and the swimming performance (thrust, power and efficiency), as two separate but inter-related topics, in that there is no obvious one-to-one connection between them. Zhang (Reference Zhang2017), in the context of a flapping wing, cautioned that examining the flow pattern is much like looking at the footprints of terrestrial animals; although some information can be gleaned from the wake, it does not tell the whole story. Similarly, Taylor (Reference Taylor2018) writes that an explanation of propulsive efficiency given only in terms of the wake feels a little incomplete, and notes that Eloy (Reference Eloy2012) suggested that efficient development of the wake may be a consequence, rather than a cause, of efficient propulsion. See also Floryan, Van Buren & Smits (Reference Floryan, Van Buren and Smits2019).
1.3 Measures of performance
We have a particular interest in the thrust, power and efficiency of a wide variety of ‘swimmers’, a term that may include animals, robots, as well as isolated propulsors such as oscillating foils and flexible plates. As indicated earlier, the principal non-dimensional variables governing the performance are the Strouhal number,
![]() $St$
, the reduced frequency,
$St$
, the reduced frequency,
![]() $k$
, and the chord-based Reynolds number,
$k$
, and the chord-based Reynolds number,
![]() $Re=cU/\unicode[STIX]{x1D708}$
, where
$Re=cU/\unicode[STIX]{x1D708}$
, where
![]() $\unicode[STIX]{x1D708}$
is the fluid kinematic viscosity. We also have the aspect ratio
$\unicode[STIX]{x1D708}$
is the fluid kinematic viscosity. We also have the aspect ratio ![]() , where
, where
![]() $s$
is the span and
$s$
is the span and
![]() $A$
is the planform area of the propulsor, as well as some measure of the body flexibility such as the effective stiffness. The results on propulsive performance are usually presented in terms of the non-dimensional thrust output coefficient, input power coefficient and Froude efficiency, conventionally defined according to
$A$
is the planform area of the propulsor, as well as some measure of the body flexibility such as the effective stiffness. The results on propulsive performance are usually presented in terms of the non-dimensional thrust output coefficient, input power coefficient and Froude efficiency, conventionally defined according to
where
![]() $T$
is the net streamwise (
$T$
is the net streamwise (
![]() $x$
-direction) component of the force developed by the motion,
$x$
-direction) component of the force developed by the motion,
![]() $P$
is the power expended and
$P$
is the power expended and
![]() $\unicode[STIX]{x1D70C}$
is the fluid density. Unless otherwise indicated, we consider only time averaged values.
$\unicode[STIX]{x1D70C}$
is the fluid density. Unless otherwise indicated, we consider only time averaged values.
What about the drag on the body? The net thrust,
![]() $T$
, is given by the thrust produced by the motion,
$T$
, is given by the thrust produced by the motion,
![]() $F_{x}$
, minus the drag force,
$F_{x}$
, minus the drag force,
![]() $D$
, so that
$D$
, so that
![]() $T=F_{x}-D$
. For a body in steady self-propelled motion (free swimming), the total thrust is equal to the total drag, so that
$T=F_{x}-D$
. For a body in steady self-propelled motion (free swimming), the total thrust is equal to the total drag, so that
![]() $T=0$
,
$T=0$
,
![]() $C_{T}=0$
and the efficiency can no longer be defined. In such cases the cost of transport
$C_{T}=0$
and the efficiency can no longer be defined. In such cases the cost of transport
![]() $CoT$
is often used, as first introduced by Von Kármán & Gabrielli (Reference Von Kármán and Gabrielli1950). That is, for an animal of weight
$CoT$
is often used, as first introduced by Von Kármán & Gabrielli (Reference Von Kármán and Gabrielli1950). That is, for an animal of weight
![]() $mg$
,
$mg$
,
![]() $CoT=P/(mgU)$
. This is a useful quantity to help compare the performance of dissimilar animals where
$CoT=P/(mgU)$
. This is a useful quantity to help compare the performance of dissimilar animals where
![]() $P$
is given by the metabolic rate, and the minimum
$P$
is given by the metabolic rate, and the minimum
![]() $CoT$
is assumed to occur at the velocity at which the animal can cover the largest distance for the smallest energy cost. Fish & Rohr (Reference Fish and Rohr1999) note that fish have the lowest
$CoT$
is assumed to occur at the velocity at which the animal can cover the largest distance for the smallest energy cost. Fish & Rohr (Reference Fish and Rohr1999) note that fish have the lowest
![]() $CoT$
for any vertebrate, and that cetaceans have values two or three times higher than similar sized fish, probably because of the higher maintenance costs. Intriguingly, they found that the minimum value of the
$CoT$
for any vertebrate, and that cetaceans have values two or three times higher than similar sized fish, probably because of the higher maintenance costs. Intriguingly, they found that the minimum value of the
![]() $CoT$
for fish and mammals is a function of body mass
$CoT$
for fish and mammals is a function of body mass
![]() $m$
, scaling approximately according to
$m$
, scaling approximately according to
![]() $m^{-0.25}$
.
$m^{-0.25}$
.
In some animals, we can separate the sources of drag from the sources of thrust. If, for example, we can identify the caudal fin as the main source of propulsive force and the body as the main source of drag, then the caudal fin needs to generate a net positive force (its thrust
![]() $F_{x}$
minus its drag
$F_{x}$
minus its drag
![]() $D_{p}$
) in order to overcome the drag offered by the body
$D_{p}$
) in order to overcome the drag offered by the body
![]() $D_{b}$
. In this case, the fin can be considered in isolation, and we can define the efficiency of the propulsor as
$D_{b}$
. In this case, the fin can be considered in isolation, and we can define the efficiency of the propulsor as
![]() $TU/P$
, where
$TU/P$
, where
![]() $P$
is the power necessary to generate the net propulsor thrust
$P$
is the power necessary to generate the net propulsor thrust
![]() $T=F_{x}-D_{p}$
, ignoring the question of how efficiently the input power was generated to begin with (that is, we are concerned with fluid mechanical efficiency and not metabolic efficiency). Furthermore, the net propulsor thrust
$T=F_{x}-D_{p}$
, ignoring the question of how efficiently the input power was generated to begin with (that is, we are concerned with fluid mechanical efficiency and not metabolic efficiency). Furthermore, the net propulsor thrust
![]() $T$
then determines the steady swimming speed of the body plus propulsor, through the force balance
$T$
then determines the steady swimming speed of the body plus propulsor, through the force balance
![]() $T=F_{x}-D_{p}=D_{b}$
. These broad assumptions are often used in propulsive models for oscillatory swimmers such as tuna and dolphins (see § 3).
$T=F_{x}-D_{p}=D_{b}$
. These broad assumptions are often used in propulsive models for oscillatory swimmers such as tuna and dolphins (see § 3).
1.4 Undulatory and oscillatory swimming
To compare the swimming styles of undulatory and oscillatory swimmers, we consider how the shape of the body midline changes during an actuation cycle. The results are shown for a number of different species in figure 5. The distinction among swimmer types is obviously less clear than that implied by the conventional view that there are distinct morphological differences between undulatory and oscillatory swimmers (Lindsey Reference Lindsey, Hoar and Randall1978; Sfakiotakis et al. Reference Sfakiotakis, Lane and Davies1999). That is, all four types shown here display a midline motion that grows similarly in amplitude along the body length. Although the anguilliform motion grows more uniformly along the body compared to other species, there is no great distinction among the other three types where the motion is largely restricted to the posterior one third of the body.

Figure 5. Four classical categories of fish undulatory propulsion illustrated with fish outlines and midlines derived from recent experimental data. Outlines of swimming fishes are shown above with displacements that to illustrate forward progression, while midlines at equally spaced time intervals throughout a tail beat are superimposed at right, aligned at the tip of the snout; each time is shown in a distinct colour. Anguilliform mode based on Anguilla, subcarangiform mode based on Lepomis, carangiform mode based on Scomber and thunniform mode based on Euthynnus. All fishes were between 20 and 25 cm total length (
![]() $L$
), and swam at a similar speed of 1.6 to 1.8
$L$
), and swam at a similar speed of 1.6 to 1.8
![]() $L/s$
. Times shown indicate duration of the tail beat. Scale bars
$L/s$
. Times shown indicate duration of the tail beat. Scale bars
![]() $=$
2 cm. Adapted with permission from Lauder & Tytell (Reference Lauder and Tytell2005).
$=$
2 cm. Adapted with permission from Lauder & Tytell (Reference Lauder and Tytell2005).
Instead of using body morphology to distinguish oscillatory and undulatory swimmers, we therefore use a criterion based on the mechanism that generates thrust. In the first group we have animals that pass a travelling wave along the body such as eels, lampreys, snakes. We call these undulatory swimmers because the wavelength of the undulation is equal to or shorter than the length of the body. Some rajiform species such as the blue spotted stingray and Atlantic stingray similarly undulate their pectoral fins to generate thrust, and amiiform and gymnotiform swimmers such as the bowfin and knifefish may also be included in this group, since they move by propagating undulations along their elongated anal or dorsal fin, with a wavelength shorter than the length of the fin (Fish Reference Fish2001).
In the second group we have carangiform and thunniform swimmers where the primary mechanism for producing thrust is a prominent caudal fin. Here, the wavelength of the undulation is longer than the body length, and so the caudal fin describes a combination of heaving and pitching motions. Some species of ray such as the manta ray and cownose ray move their pectoral fins in a similar motion, which happens when the wavelength of the undulation is longer than the chord of the pectoral fin (called mobuliform swimming). We also include cetaceans in this group, because their primary propulsive force is due to the oscillatory motion of the fluke in the vertical plane. The swimming kinematics of cetaceans are characteristic of the thunniform mode, also known as carangiform with lunate tail (Lighthill Reference Lighthill1969, Reference Lighthill1970; Webb Reference Webb1975; Lindsey Reference Lindsey, Hoar and Randall1978). The role of flexibility varies widely, in that the caudal fin of tuna are stiff compared to the rather flexible flukes of dolphins, and manta rays can actively control the flexibility of their pectoral fins by muscle action.
In some species, however, it is not always possible to make a clear demarcation between undulatory and oscillatory swimming. Sharks, for example, display motions ranging from anguilliform to thunniform (Wilga & Lauder Reference Wilga, Lauder, Carrier, Musick and Heithaus2004). They also have a distinctive heterocercal tail, that is, an asymmetric caudal fin shape, and so are expected to generate asymmetric wake structures (Flammang et al. Reference Flammang, Lauder, Troolin and Strand2011). Nevertheless, the broad distinction based on the propulsive mechanism proves to be useful as an organizing principle.
In what follows, we first consider undulatory swimming, and then oscillatory swimming. Mobuliform swimmers like manta rays will be considered separately, in that they are a good example of animals that actively control the shape of their fins to change the waveform of actuation. Some basic themes will emerge. One, there is no one-to-one correspondence between wake structure and efficiency. Two, the primary velocity scale for propulsion is the characteristic velocity of the trailing edge. Three, the drag of the propulsor is crucially important in determining its thrust and efficiency. Four, flexibility (either passive or active) can have a major effect on performance, and a proper exploitation of flexibility seems essential to attain high thrust and efficiency.
2 Undulatory swimming
Taylor (Reference Taylor1952) was the first to estimate the thrust produced by long and narrow animals such as snakes, eels and marine worms, by ‘considering the equilibrium of a flexible cylinder immersed in water when waves of bending of constant amplitude travel down it at constant speed. The force on each element of the cylinder is assumed to be the same as that which would act on a corresponding element of a long straight cylinder moving at the same speed and inclination to the direction of motion’. This approach is now known as the ‘resistive model’ of thrust production, and the total thrust is found by integrating the streamwise contributions from all the segments along the body. By using an appropriate estimate for the drag coefficient on each segment, and by allowing the amplitude of the bending wave to vary along the body, the model can be adapted to non-circular cross-sections, varying locomotion profiles, and a wide range of Reynolds numbers. For example, it was used by Gray & Hancock (Reference Gray and Hancock1955) to analyse very low Reynolds number swimmers using a linear drag dependence. An important result is that for high Reynolds numbers the bending wave needs to move down the body at a speed greater than the swim velocity to achieve positive thrust.
As pointed out by Lauder & Tytell (Reference Lauder and Tytell2005) and many others, the theory is limited by the assumption of small-amplitude bending waves, and the assumption that the resistive force experienced by each segment is quasi-steady and independent of the adjacent segments. In addition, the drag coefficients used in the theory are determined from experiments on steady cylinders, and in unsteady flow added mass forces will appear, and the circulatory forces would also change from their steady flow values, at least for high Reynolds numbers. Therefore, although it is still widely used for low Reynolds number applications (see, for example, Zhong et al. (Reference Zhong, Moored, Pinedo, Garcia-Gonzalez and Smits2013)), for high Reynolds number flows where accelerations (such as those associated with added mass effects) need to be considered, resistive models have largely been replaced by approaches based on some form of slender body theory.
2.1 Slender body theory
Lighthill (Reference Lighthill1960) was the first to apply slender body theory (originally developed in the context of airships and supersonic flow by Munk Reference Munk1924; Tsien Reference Tsien1938) to the swimming of slender fish, that is, ‘either a fish or a swimming mammal, whose dimensions and movements at right angles to its direction of locomotion are small compared with its length, while its cross-section varies along it only gradually’. It is similar to thin airfoil theory in the sense that the derivatives are evaluated at the body centreline without consideration to its thickness.
Several other assumptions are made in the basic theory. First, the flow is taken to be inviscid, so that the instantaneous energy conservation in aquatic animal self-locomotion is given by
where
![]() $P$
is the power exerted by the animal,
$P$
is the power exerted by the animal,
![]() $E$
is the rate of change of the kinetic energy of the fluid and
$E$
is the rate of change of the kinetic energy of the fluid and
![]() $TU$
is the rate of work done to produce thrust
$TU$
is the rate of work done to produce thrust
![]() $T$
. In evaluating the efficiency, the drag is taken to be zero, and therefore the efficiency in slender body theory measures the part of the power that goes to produce thrust, compared to the total power input that includes the loss of energy to the fluid (that is, the wake). Second, the theory assumes that the force on each segment of the body is given by the reactive force due to the acceleration of the added mass per unit length of the segment, which is why it is sometimes called a ‘reactive’ model. This reactive force is taken to act only in the
$T$
. In evaluating the efficiency, the drag is taken to be zero, and therefore the efficiency in slender body theory measures the part of the power that goes to produce thrust, compared to the total power input that includes the loss of energy to the fluid (that is, the wake). Second, the theory assumes that the force on each segment of the body is given by the reactive force due to the acceleration of the added mass per unit length of the segment, which is why it is sometimes called a ‘reactive’ model. This reactive force is taken to act only in the
![]() $y$
-direction, which is normal to the direction of the forward progress of the animal (taken to be the
$y$
-direction, which is normal to the direction of the forward progress of the animal (taken to be the
![]() $x$
-direction). Circulatory forces are not considered. Third, it assumes that each segment is independent of the next, which is the same infinite cylinder approximation used in resistive theory. Fourth, motions are taken to be small (although the theory was extended to large-amplitude motions by Lighthill Reference Lighthill1971).
$x$
-direction). Circulatory forces are not considered. Third, it assumes that each segment is independent of the next, which is the same infinite cylinder approximation used in resistive theory. Fourth, motions are taken to be small (although the theory was extended to large-amplitude motions by Lighthill Reference Lighthill1971).
We now summarize the salient results. The notation and approach follow Wu (Reference Wu2011), and the theory applies equally well to long slender bodies and thin membranes (that is, flexible ribbon plates of constant width where the width is small compared to its length). We give more details than might be wise, but the results will find a number of applications in the rest of this paper.
For a travelling wave described by
![]() $h(x,t)$
, the local velocity in the
$h(x,t)$
, the local velocity in the
![]() $y$
-direction is given by
$y$
-direction is given by
![]() $V(x,t)=(\unicode[STIX]{x2202}/\unicode[STIX]{x2202}t+U\unicode[STIX]{x2202}/\unicode[STIX]{x2202}x)h$
. The associated force per unit length is called the specific lift
$V(x,t)=(\unicode[STIX]{x2202}/\unicode[STIX]{x2202}t+U\unicode[STIX]{x2202}/\unicode[STIX]{x2202}x)h$
. The associated force per unit length is called the specific lift
![]() ${\mathcal{L}}$
(Wu Reference Wu2011), where
${\mathcal{L}}$
(Wu Reference Wu2011), where
![]() ${\mathcal{L}}=(\unicode[STIX]{x2202}/\unicode[STIX]{x2202}t+U\unicode[STIX]{x2202}/\unicode[STIX]{x2202}x)(mV)$
. Note that
${\mathcal{L}}=(\unicode[STIX]{x2202}/\unicode[STIX]{x2202}t+U\unicode[STIX]{x2202}/\unicode[STIX]{x2202}x)(mV)$
. Note that
![]() ${\mathcal{L}}$
depends entirely on the added mass per unit length
${\mathcal{L}}$
depends entirely on the added mass per unit length
![]() $m$
, and that there is no contribution due to circulatory forces. Typically, the added mass model is very simple, in that it is taken to be proportional to the area of the body cross-section. Then, instantaneously,
$m$
, and that there is no contribution due to circulatory forces. Typically, the added mass model is very simple, in that it is taken to be proportional to the area of the body cross-section. Then, instantaneously,
where
![]() $\ell$
is the body length, and
$\ell$
is the body length, and
![]() $E$
is evaluated as the rate of shedding of kinetic energy of lateral fluid motions into the fluid. The thrust
$E$
is evaluated as the rate of shedding of kinetic energy of lateral fluid motions into the fluid. The thrust
![]() $T$
is found from the energy balance in (2.1), although it can be formally shown that
$T$
is found from the energy balance in (2.1), although it can be formally shown that
![]() $T$
is the projection in the
$T$
is the projection in the
![]() $x$
-direction of the forces due to pressure differences acting on the body.
$x$
-direction of the forces due to pressure differences acting on the body.
For a body in periodic motion with
![]() $m(x)=0$
and
$m(x)=0$
and
![]() $m(\ell )=m_{\ell }$
, the time-averaged values are given by
$m(\ell )=m_{\ell }$
, the time-averaged values are given by
and
Then, from the energy balance given in (2.1),
We see that the mean thrust and power depend only on the conditions at the trailing edge where
![]() $x=\ell$
. In addition, the lateral velocity of the tail,
$x=\ell$
. In addition, the lateral velocity of the tail,
![]() $V$
, plays a crucial role in determining the performance.
$V$
, plays a crucial role in determining the performance.
Following Lighthill (Reference Lighthill1960) and Wu (Reference Wu1971b
), we consider the special case of a distally propagating wave
![]() $g(x)\unicode[STIX]{x1D6FE}(x-c_{w}t)$
, where
$g(x)\unicode[STIX]{x1D6FE}(x-c_{w}t)$
, where
![]() $c_{w}$
is the wave velocity and the wave amplitude
$c_{w}$
is the wave velocity and the wave amplitude
![]() $g$
depends on
$g$
depends on
![]() $x$
(
$x$
(
![]() $|\unicode[STIX]{x1D6FE}|=1$
). It will be assumed that such a motion can be made to satisfy the restrictions on the lateral and angular momenta embodied in the evaluation of the specific lift (the so-called ‘recoil’ constraint). Lighthill (Reference Lighthill1960) argues, on the basis of efficiency, that it is best to have
$|\unicode[STIX]{x1D6FE}|=1$
). It will be assumed that such a motion can be made to satisfy the restrictions on the lateral and angular momenta embodied in the evaluation of the specific lift (the so-called ‘recoil’ constraint). Lighthill (Reference Lighthill1960) argues, on the basis of efficiency, that it is best to have
![]() $g_{x}(\ell )=0$
, and if this condition is satisfied then
$g_{x}(\ell )=0$
, and if this condition is satisfied then
where
![]() $k_{\unicode[STIX]{x1D706}}=c_{w}/U=f\unicode[STIX]{x1D706}/U$
. The thrust is therefore proportional to the mean square lateral velocity of the trailing edge, and for a given
$k_{\unicode[STIX]{x1D706}}=c_{w}/U=f\unicode[STIX]{x1D706}/U$
. The thrust is therefore proportional to the mean square lateral velocity of the trailing edge, and for a given
![]() $k_{\unicode[STIX]{x1D706}}$
it is independent of
$k_{\unicode[STIX]{x1D706}}$
it is independent of
![]() $U$
. Also, the travelling wave needs to move down the body at a speed greater than the swim velocity to achieve positive thrust; that is,
$U$
. Also, the travelling wave needs to move down the body at a speed greater than the swim velocity to achieve positive thrust; that is,
![]() $T$
is only positive for
$T$
is only positive for
![]() $c_{w}>U$
, the same conclusion reached by Taylor (Reference Taylor1952) using resistive theory.
$c_{w}>U$
, the same conclusion reached by Taylor (Reference Taylor1952) using resistive theory.
To find the steady swimming speed, we balance the thrust against the body drag. If it is assumed that the drag depends only on the body wetted area
![]() $A_{b}$
so that
$A_{b}$
so that
![]() $D_{b}=\unicode[STIX]{x1D70C}U^{2}A_{b}C_{D}/2$
, where
$D_{b}=\unicode[STIX]{x1D70C}U^{2}A_{b}C_{D}/2$
, where
![]() $C_{D}$
is the drag coefficient, then
$C_{D}$
is the drag coefficient, then
For a given body at a fixed value of
![]() $k_{\unicode[STIX]{x1D706}}$
, the swim speed is therefore proportional to the root-mean-square (r.m.s.) lateral velocity of the trailing edge. Furthermore, since the thrust only depends on the conditions at the trailing edge, it would be ‘wasteful’ (Lighthill’s word) to keep
$k_{\unicode[STIX]{x1D706}}$
, the swim speed is therefore proportional to the root-mean-square (r.m.s.) lateral velocity of the trailing edge. Furthermore, since the thrust only depends on the conditions at the trailing edge, it would be ‘wasteful’ (Lighthill’s word) to keep
![]() $g(x)$
constant along the length of the body, and it is more desirable to let it increase from zero at the snout to its maximum at the tail.
$g(x)$
constant along the length of the body, and it is more desirable to let it increase from zero at the snout to its maximum at the tail.
Such observations follow from the model, which is entirely based on added mass considerations. Neglecting the circulatory force seems reasonable for long slender bodies because these forces tend to be important mostly near the leading edge. In this respect, the assumption that the lateral motion of the leading edge motion was zero is an important ingredient of the model; relaxing this assumption might also introduce a significant contribution from lift.
Finally, Lighthill considered how the angular and transverse momenta affected the thrust and efficiency, and concluded that minimizing the angular ‘recoil’ due to the body motion would be particularly desirable. This could be achieved by confining the motions to the rear part of the fish where the fish mass is low, and by letting the waveform have both a positive and negative phase in the region of substantial motion amplitude. This accurately describes anguilliform motion. For example, Tytell & Lauder (Reference Tytell and Lauder2004) investigated the kinematics of a steadily swimming American eel and found that the motion of the body midline was described well by an exponentially growing travelling wave,
where
![]() $y$
is the lateral position of the midline,
$y$
is the lateral position of the midline,
![]() $s$
is the coordinate following the midline,
$s$
is the coordinate following the midline,
![]() $\unicode[STIX]{x1D706}$
is the wavelength,
$\unicode[STIX]{x1D706}$
is the wavelength,
![]() $a$
is the tail beat amplitude and
$a$
is the tail beat amplitude and
![]() $\unicode[STIX]{x1D6FC}$
is the amplitude growth rate. Equation (2.8) also describes lamprey motion (Hultmark et al.
Reference Hultmark, Leftwich and Smits2007), and it appears to satisfy Lighthill’s conditions for high thrust production and minimal recoil but not the condition for high efficiency, in that
$\unicode[STIX]{x1D6FC}$
is the amplitude growth rate. Equation (2.8) also describes lamprey motion (Hultmark et al.
Reference Hultmark, Leftwich and Smits2007), and it appears to satisfy Lighthill’s conditions for high thrust production and minimal recoil but not the condition for high efficiency, in that
![]() $y_{s}\neq 0$
at the tail.
$y_{s}\neq 0$
at the tail.
The important role played by the lateral tail velocity in undulatory swimming was also confirmed by studying the thrust production of lampreys. Hultmark et al. (Reference Hultmark, Leftwich and Smits2007) used a robotic lamprey that was actuated to copy the lamprey motion described by (2.8). The near-wake structure for the lamprey robot and the American eel were shown in figure 4(a,b) and figure 6 gives the instantaneous vorticity fields generated by the robot at three phases in its motion. A band of positive vorticity is evident in the region near the body, confined to a relatively thin boundary layer rather than being shed and convected downstream. The undulatory motion of the robot generates alternating favourable and unfavourable pressure gradients along the body, seen in the streamwise varying strength of the near-surface vorticity, but there is no evidence of separation. The flow in the vicinity of the body, therefore, is unlikely to contribute little to either drag or thrust. As the tail moves to the right (figure 6 b), the maximum strength of the near-surface vorticity increases, and as the tail changes direction the pressure gradients change sign and the region of vorticity becomes weaker, as seen in figure 6(c), indicating that a significant amount of vorticity has been shed into the wake. As might be expected, the tail motions and the vortices shed into the wake are closely correlated to the fluctuations in pressure on the body near the tail (Leftwich & Smits Reference Leftwich and Smits2011).

Figure 6. Phase-averaged out-of-plane vorticity fields along the body and in the wake for a steadily swimming robot. The flow is from top to bottom, and the body of the robot is indicated by the black shape. Reproduction with permission from Hultmark et al. (Reference Hultmark, Leftwich and Smits2007).
To determine the contributions to the thrust, the momentum flux was integrated over the boundaries of a two-dimensional control volume containing the wake and parts of the tail. By varying the size of the box to include progressively more of the body, it was found that the region very close to the tail (
![]() $x/\ell \geqslant 0.99$
) was responsible for the majority of the thrust production. For the region
$x/\ell \geqslant 0.99$
) was responsible for the majority of the thrust production. For the region
![]() $0.99\geqslant x/\ell \geqslant 0.9$
the thrust was essentially balanced by the drag, and at locations upstream of
$0.99\geqslant x/\ell \geqslant 0.9$
the thrust was essentially balanced by the drag, and at locations upstream of
![]() $x/\ell =0.9$
the drag was found to be greater than the thrust. The dominant role of the tail in producing thrust is in complete accordance with slender body theory. In addition, Leftwich & Smits (Reference Leftwich and Smits2011) found that the net mean thrust produced by their lamprey robot at
$x/\ell =0.9$
the drag was found to be greater than the thrust. The dominant role of the tail in producing thrust is in complete accordance with slender body theory. In addition, Leftwich & Smits (Reference Leftwich and Smits2011) found that the net mean thrust produced by their lamprey robot at
![]() $St=0.53$
was only approximately 20 % less than that given by (2.5). That would imply a drag coefficient for the robot of about 0.08, which seems like a reasonable value.
$St=0.53$
was only approximately 20 % less than that given by (2.5). That would imply a drag coefficient for the robot of about 0.08, which seems like a reasonable value.
2.2 Flexible panels in undulatory motion
Slender body theory gives estimates for the forces acting on the body and the surrounding fluid under a prescribed motion. It does not generally consider the mechanical response of the body itself, although Wu (Reference Wu1971b ) considered the internal forces generated by an elastic membrane in prescribed motion in terms of the attendant recoil. In fish, the amplitude and wavelength of their undulation can generally be controlled by muscle action. In laboratory studies of undulatory motion, this sort of active control is rarely imitated (for an exception, see Clark & Smits Reference Clark and Smits2006). Instead, experiments typically rely on a predetermined motion input (such as heave or pitch) and allow the flexibility of the foil, membrane or body to determine its response. Computations generally go further, and prescribe the full motion, typically determined directly from animal studies, without accounting for any fluid–structure interaction.
To understand the effects of flexibility and the mechanical response of the body more generally, Quinn, Lauder & Smits (Reference Quinn, Lauder and Smits2014) examined the behaviour of flexible, rectangular panels, actuated in heave at their leading edge with an amplitude
![]() $a^{\prime }$
. For small deflections
$a^{\prime }$
. For small deflections
![]() $h$
of a flexible panel with constant thickness
$h$
of a flexible panel with constant thickness
![]() $\unicode[STIX]{x1D6FF}$
and span
$\unicode[STIX]{x1D6FF}$
and span
![]() $s$
, the panel response can be modelled by the Euler–Bernoulli beam equation,
$s$
, the panel response can be modelled by the Euler–Bernoulli beam equation,
where
![]() $\unicode[STIX]{x1D70C}_{p}$
is the density of the panel,
$\unicode[STIX]{x1D70C}_{p}$
is the density of the panel,
![]() $E$
is the elastic modulus,
$E$
is the elastic modulus,
![]() $I$
is the area moment of inertia and
$I$
is the area moment of inertia and
![]() $F_{ext}$
is the external force per unit length (Allen & Smits Reference Allen and Smits2001). Since
$F_{ext}$
is the external force per unit length (Allen & Smits Reference Allen and Smits2001). Since
![]() $\unicode[STIX]{x1D70C}_{p}/\unicode[STIX]{x1D70C}=O(1)$
and
$\unicode[STIX]{x1D70C}_{p}/\unicode[STIX]{x1D70C}=O(1)$
and
![]() $\unicode[STIX]{x1D6FF}/c\ll 1$
, the added mass forces are expected to dominate over the inertia of the panel, and so the mass per length of the panel,
$\unicode[STIX]{x1D6FF}/c\ll 1$
, the added mass forces are expected to dominate over the inertia of the panel, and so the mass per length of the panel,
![]() $\unicode[STIX]{x1D70C}_{p}s\unicode[STIX]{x1D6FF}$
, is replaced with an effective (added) mass per length,
$\unicode[STIX]{x1D70C}_{p}s\unicode[STIX]{x1D6FF}$
, is replaced with an effective (added) mass per length,
![]() $\unicode[STIX]{x1D70C}sc$
, which is assumed constant along the chord. For long, narrow panels
$\unicode[STIX]{x1D70C}sc$
, which is assumed constant along the chord. For long, narrow panels ![]() , the added mass per unit length will vary as
, the added mass per unit length will vary as
![]() $\unicode[STIX]{x1D70C}s^{2}$
, and for short, wide panels
$\unicode[STIX]{x1D70C}s^{2}$
, and for short, wide panels ![]() it varies as
it varies as
![]() $\unicode[STIX]{x1D70C}cs$
. For panels with
$\unicode[STIX]{x1D70C}cs$
. For panels with ![]() of
of
![]() $O(1)$
, the appropriate added mass term is less clear. Quinn et al. used panels with
$O(1)$
, the appropriate added mass term is less clear. Quinn et al. used panels with ![]() , so their choice of
, so their choice of
![]() $\unicode[STIX]{x1D70C}sc$
is somewhat arbitrary but since the aspect ratio was fixed it does not affect any of their conclusions (see also Allen & Smits Reference Allen and Smits2001; Thiria & Godoy-Diana Reference Thiria and Godoy-Diana2010; Dewey et al.
Reference Dewey, Boschitsch, Moored, Stone and Smits2013; Ramananarivo, Godoy-Diana & Thiria Reference Ramananarivo, Godoy-Diana and Thiria2013). This issue is addressed in more detail in § 3.6.
$\unicode[STIX]{x1D70C}sc$
is somewhat arbitrary but since the aspect ratio was fixed it does not affect any of their conclusions (see also Allen & Smits Reference Allen and Smits2001; Thiria & Godoy-Diana Reference Thiria and Godoy-Diana2010; Dewey et al.
Reference Dewey, Boschitsch, Moored, Stone and Smits2013; Ramananarivo, Godoy-Diana & Thiria Reference Ramananarivo, Godoy-Diana and Thiria2013). This issue is addressed in more detail in § 3.6.
Introducing the dimensionless variables
![]() $x^{\ast }\equiv x/c$
,
$x^{\ast }\equiv x/c$
,
![]() $h^{\ast }\equiv h/a$
, and
$h^{\ast }\equiv h/a$
, and
![]() $t^{\ast }\equiv tf$
, where
$t^{\ast }\equiv tf$
, where
![]() $a$
is (as usual) the amplitude of the trailing edge motion, gives
$a$
is (as usual) the amplitude of the trailing edge motion, gives
where
![]() $F_{ext}^{\prime }\equiv F_{ext}\,c^{4}/(EIa)$
is the dimensionless external force per unit length, except for added mass, which is now incorporated into the left-hand side as the effective mass per length. These external forces could include, for example, circulatory forces and internal damping or viscous drag. Here,
$F_{ext}^{\prime }\equiv F_{ext}\,c^{4}/(EIa)$
is the dimensionless external force per unit length, except for added mass, which is now incorporated into the left-hand side as the effective mass per length. These external forces could include, for example, circulatory forces and internal damping or viscous drag. Here,
and it is the ratio of added mass forces to internal bending forces, called the effective flexibility. We also recognize it as a non-dimensional frequency
![]() $f^{\ast }$
, that is, the ratio of the driving frequency to the first resonant frequency of the panel when added mass forces are considered.
$f^{\ast }$
, that is, the ratio of the driving frequency to the first resonant frequency of the panel when added mass forces are considered.
The effect of resonances for pitching foils was examined with a more exact, though two-dimensional fluid dynamical model by Alben (Reference Alben2008), who found that the resonances were distributed as integers to the
![]() $-5/2$
power, and the input power and thrust power were proportional to the parameter
$-5/2$
power, and the input power and thrust power were proportional to the parameter
![]() $R_{2}=\unicode[STIX]{x1D6F1}_{1}^{\prime }$
at the resonances. The Froude efficiency tended to 1 at small thrust power (when many wavelengths are present on the body), then dropped to a fixed fraction at large thrust power. No peaks were seen in the efficiency, as was also found by Floryan & Rowley (Reference Floryan and Rowley2018). Alben et al. (Reference Alben, Witt, Baker, Anderson and Lauder2012) later studied (theoretically and experimentally) a freely swimming flexible foil in heave, and observed that the scaling of swimming speed with bending modulus and foil length was given as power laws at resonant peaks (separate power laws in terms of dimensional and dimensionless parameters).
$R_{2}=\unicode[STIX]{x1D6F1}_{1}^{\prime }$
at the resonances. The Froude efficiency tended to 1 at small thrust power (when many wavelengths are present on the body), then dropped to a fixed fraction at large thrust power. No peaks were seen in the efficiency, as was also found by Floryan & Rowley (Reference Floryan and Rowley2018). Alben et al. (Reference Alben, Witt, Baker, Anderson and Lauder2012) later studied (theoretically and experimentally) a freely swimming flexible foil in heave, and observed that the scaling of swimming speed with bending modulus and foil length was given as power laws at resonant peaks (separate power laws in terms of dimensional and dimensionless parameters).
Quinn et al. (Reference Quinn, Lauder and Smits2014) conducted experiments on four panels with bending stiffnesses ranging from
![]() $EI=320\times 10^{-3}$
(Panel
$EI=320\times 10^{-3}$
(Panel
![]() $A$
, rigid) to
$A$
, rigid) to
![]() $0.069\times 10^{-3}~\text{N}~\text{m}^{-2}$
(Panel
$0.069\times 10^{-3}~\text{N}~\text{m}^{-2}$
(Panel
![]() $D$
, the most flexible), as listed in table 1. The modal contributions to the panel undulatory motion were expressed in terms of the eigenfunctions for the homogenous form of (2.10), that is, where
$D$
, the most flexible), as listed in table 1. The modal contributions to the panel undulatory motion were expressed in terms of the eigenfunctions for the homogenous form of (2.10), that is, where
![]() $F_{ext}^{\prime }=0$
. The natural frequencies of these modes,
$F_{ext}^{\prime }=0$
. The natural frequencies of these modes,
![]() $\hat{f}_{i}$
, are such that
$\hat{f}_{i}$
, are such that
![]() $f/\hat{f}_{i}=\unicode[STIX]{x1D6F1}_{1}\unicode[STIX]{x1D706}_{i}^{2}$
, where
$f/\hat{f}_{i}=\unicode[STIX]{x1D6F1}_{1}\unicode[STIX]{x1D706}_{i}^{2}$
, where
![]() $\unicode[STIX]{x1D706}_{i}$
is the corresponding wavelength. Flexibility essentially adds resonances to the system. The two most rigid panels displayed only the first mode, while the most flexible panel exhibited the first four modes. As the heaving frequency of the more flexible panels increased, the modal contributions passed through a series of peaks as successively higher modes were activated, and with increasing flow speed these peaks shifted to higher frequencies. Resonance occurred at certain driving frequencies where the trailing edge amplitude was locally maximized, and Quinn et al. (Reference Quinn, Lauder and Smits2014) found that the frequency at which this resonance occurs was a strong function of
$\unicode[STIX]{x1D706}_{i}$
is the corresponding wavelength. Flexibility essentially adds resonances to the system. The two most rigid panels displayed only the first mode, while the most flexible panel exhibited the first four modes. As the heaving frequency of the more flexible panels increased, the modal contributions passed through a series of peaks as successively higher modes were activated, and with increasing flow speed these peaks shifted to higher frequencies. Resonance occurred at certain driving frequencies where the trailing edge amplitude was locally maximized, and Quinn et al. (Reference Quinn, Lauder and Smits2014) found that the frequency at which this resonance occurs was a strong function of
![]() $f^{\ast }$
, but relatively independent of flow speed (for a factor of 5 variation).
$f^{\ast }$
, but relatively independent of flow speed (for a factor of 5 variation).
Table 1. Panel physical properties. Panels
![]() $A$
to
$A$
to
![]() $D$
from Quinn et al. (Reference Quinn, Lauder and Smits2014); Panels
$D$
from Quinn et al. (Reference Quinn, Lauder and Smits2014); Panels
![]() $P_{1}$
to
$P_{1}$
to
![]() $P_{\infty }$
from Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013). Here,
$P_{\infty }$
from Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013). Here,
![]() $\unicode[STIX]{x1D6F1}_{1}^{\prime }=k^{2}/\unicode[STIX]{x1D6F1}_{1}$
(see (3.19)). The effective stiffness for fish is likely in the range
$\unicode[STIX]{x1D6F1}_{1}^{\prime }=k^{2}/\unicode[STIX]{x1D6F1}_{1}$
(see (3.19)). The effective stiffness for fish is likely in the range
![]() $1.4<\unicode[STIX]{x1D6F1}_{1}^{\prime }<4$
(panels
$1.4<\unicode[STIX]{x1D6F1}_{1}^{\prime }<4$
(panels
![]() $P_{3}$
to
$P_{3}$
to
![]() $P_{5}$
).
$P_{5}$
).

From slender body theory, we know that the conditions at the trailing edge are paramount. For flexible panels, the trailing edge amplitude
![]() $a$
is an output of the system since it depends on the panel response to the input
$a$
is an output of the system since it depends on the panel response to the input
![]() $a^{\prime }$
. The amplitudes of the resonant peaks in
$a^{\prime }$
. The amplitudes of the resonant peaks in
![]() $a/a^{\prime }$
decrease with increasing flexibility as the deflections are more easily suppressed by fluid forces when the panel is more flexible. They also decrease with increasing flow speed as the form drag at higher flow speeds suppresses panel deflections and thereby reduces the amplitude of the wave along the panel. It appears that the first-order effect of form and viscous drag is to stretch the shape of the panel in the streamwise direction but leave the resonant frequencies relatively unaffected.
$a/a^{\prime }$
decrease with increasing flexibility as the deflections are more easily suppressed by fluid forces when the panel is more flexible. They also decrease with increasing flow speed as the form drag at higher flow speeds suppresses panel deflections and thereby reduces the amplitude of the wave along the panel. It appears that the first-order effect of form and viscous drag is to stretch the shape of the panel in the streamwise direction but leave the resonant frequencies relatively unaffected.
The time-averaged thrust behaviour is shown in figure 7. In general,
![]() $\overline{T}$
increases with heaving frequency, but it does so at different rates, and in some ranges even decreases with frequency. The resulting plateaus are directly related to resonance at the trailing edge. Since the more flexible panels pass through multiple resonant frequencies, they exhibit multiple plateaus (for (b) the first resonance is close to 1 Hz, and the second resonance occurs beyond 3 Hz).
$\overline{T}$
increases with heaving frequency, but it does so at different rates, and in some ranges even decreases with frequency. The resulting plateaus are directly related to resonance at the trailing edge. Since the more flexible panels pass through multiple resonant frequencies, they exhibit multiple plateaus (for (b) the first resonance is close to 1 Hz, and the second resonance occurs beyond 3 Hz).
Higher flow speeds reduce
![]() $\overline{T}$
in two main ways. One way is by shifting the curves downward due to viscous drag producing an offset between them (see also § 3.1). At higher frequencies, the curves differ by more than just an offset, especially for the more flexible panels. This trend suggests that variations beyond the simple offset are due to the different kinematics brought on by higher flow speeds. A similar behaviour was seen by Floryan & Rowley (Reference Floryan and Rowley2018) who analysed the response of two-dimensional, flexible panels in heave and pitch. When the flow speed increases, circulatory forces start to become important, the natural frequencies of the system begin to shift away from their quiescent behaviour, and the eigenvalues become more damped.
$\overline{T}$
in two main ways. One way is by shifting the curves downward due to viscous drag producing an offset between them (see also § 3.1). At higher frequencies, the curves differ by more than just an offset, especially for the more flexible panels. This trend suggests that variations beyond the simple offset are due to the different kinematics brought on by higher flow speeds. A similar behaviour was seen by Floryan & Rowley (Reference Floryan and Rowley2018) who analysed the response of two-dimensional, flexible panels in heave and pitch. When the flow speed increases, circulatory forces start to become important, the natural frequencies of the system begin to shift away from their quiescent behaviour, and the eigenvalues become more damped.

Figure 7. Time-averaged thrust. (a,b,c,d) ▿,
![]() $u_{\infty }=40~\text{mm}~\text{s}^{-1}$
; ◃,
$u_{\infty }=40~\text{mm}~\text{s}^{-1}$
; ◃,
![]() $u_{\infty }=110~\text{mm}~\text{s}^{-1}$
; ▵,
$u_{\infty }=110~\text{mm}~\text{s}^{-1}$
; ▵,
![]() $u_{\infty }=170~\text{mm}~\text{s}^{-1}$
; ▹,
$u_{\infty }=170~\text{mm}~\text{s}^{-1}$
; ▹,
![]() $u_{\infty }=240~\text{mm}~\text{s}^{-1}$
. Adapted with permission from Quinn et al. (Reference Quinn, Lauder and Smits2014).
$u_{\infty }=240~\text{mm}~\text{s}^{-1}$
. Adapted with permission from Quinn et al. (Reference Quinn, Lauder and Smits2014).
Quinn et al. (Reference Quinn, Lauder and Smits2014) used slender body theory to help understand the formation of the plateaus in thrust. From (2.5) we can write
Consider a panel experiencing a sinusoidal travelling wave with a wavelength equal to the chord, that is,
![]() $h^{\ast }=\sin (2\unicode[STIX]{x03C0}(x^{\ast }+t^{\ast }))$
. Figure 8 plots the predicted variation of the thrust coefficient for the case where
$h^{\ast }=\sin (2\unicode[STIX]{x03C0}(x^{\ast }+t^{\ast }))$
. Figure 8 plots the predicted variation of the thrust coefficient for the case where
![]() $a/c$
is constant, and for the case where
$a/c$
is constant, and for the case where
![]() $a/c$
varies sinusoidally with the reduced frequency
$a/c$
varies sinusoidally with the reduced frequency
![]() $k$
. The local maxima in the trailing edge amplitude increase the net thrust coefficient such that plateaus appear in the
$k$
. The local maxima in the trailing edge amplitude increase the net thrust coefficient such that plateaus appear in the
![]() $k$
–
$k$
–
![]() $\overline{C_{T}}$
curve. In addition, if the heaving panel were perfectly rigid (
$\overline{C_{T}}$
curve. In addition, if the heaving panel were perfectly rigid (
![]() $EI\rightarrow \infty$
),
$EI\rightarrow \infty$
),
![]() $h^{\ast }$
would have no spatial variation. For finite rigidities, however,
$h^{\ast }$
would have no spatial variation. For finite rigidities, however,
![]() $h^{\ast }$
varies with
$h^{\ast }$
varies with
![]() $x^{\ast }$
, and (2.12) predicts that this spatial variation will reduce
$x^{\ast }$
, and (2.12) predicts that this spatial variation will reduce
![]() $\overline{C_{T}}$
, which is consistent with the data shown in figure 7.
$\overline{C_{T}}$
, which is consistent with the data shown in figure 7.

Figure 8. Predictions of the Lighthill model for a sinusoidal propulsor. Thin line:
![]() $a/c=0.1$
; thick line:
$a/c=0.1$
; thick line:
![]() $a/c=0.1(1+0.15\,\sin ^{2}k)$
. Adapted with permission from Quinn et al. (Reference Quinn, Lauder and Smits2014).
$a/c=0.1(1+0.15\,\sin ^{2}k)$
. Adapted with permission from Quinn et al. (Reference Quinn, Lauder and Smits2014).
The efficiency can be quantified for thrust-producing conditions (
![]() $\overline{T}>0$
) where the propulsor is accelerating or overcoming the drag on a body to which it is attached. Following Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013), the results for the flexible propulsors with
$\overline{T}>0$
) where the propulsor is accelerating or overcoming the drag on a body to which it is attached. Following Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013), the results for the flexible propulsors with
![]() $\overline{T}>0$
are shown in figure 9 as
$\overline{T}>0$
are shown in figure 9 as
![]() $\hat{\unicode[STIX]{x1D702}}=\unicode[STIX]{x1D702}St$
(this scaling will be addressed further in § 3.6). Peaks in
$\hat{\unicode[STIX]{x1D702}}=\unicode[STIX]{x1D702}St$
(this scaling will be addressed further in § 3.6). Peaks in
![]() $\hat{\unicode[STIX]{x1D702}}$
occur at or just above the
$\hat{\unicode[STIX]{x1D702}}$
occur at or just above the
![]() $f^{\ast }$
values where the trailing edge amplitude was maximal. The efficiency is maximized at low speeds and high flexibilities. At high flow speeds, flow visualizations indicated the presence of flow separation on the membrane itself, undoubtedly resulting in significantly higher drag and lower efficiency.
$f^{\ast }$
values where the trailing edge amplitude was maximal. The efficiency is maximized at low speeds and high flexibilities. At high flow speeds, flow visualizations indicated the presence of flow separation on the membrane itself, undoubtedly resulting in significantly higher drag and lower efficiency.

Figure 9. Efficiency peaks of heaving flexible panels at multiple resonance modes. Panels
![]() $A$
,
$A$
,
![]() $B$
,
$B$
,
![]() $C$
and
$C$
and
![]() $D$
have stiffnesses
$D$
have stiffnesses
![]() $EI=3.2\times 10^{-1}$
,
$EI=3.2\times 10^{-1}$
,
![]() $1.1\times 10^{-2}$
,
$1.1\times 10^{-2}$
,
![]() $8.1\times 10^{-4}$
,
$8.1\times 10^{-4}$
,
![]() $6.9\times 10^{-5}$
, and are coloured red, orange, green and blue respectively. Adapted with permission from Quinn et al. (Reference Quinn, Lauder and Smits2014).
$6.9\times 10^{-5}$
, and are coloured red, orange, green and blue respectively. Adapted with permission from Quinn et al. (Reference Quinn, Lauder and Smits2014).
Since the value of the Strouhal number in the vicinity of the peaks varies from approximately 0.5 to 1, it is evident from figure 9 that the corresponding values of the efficiency
![]() $\unicode[STIX]{x1D702}$
for a flexible heaving panel are quite low. In a later study focusing on panel
$\unicode[STIX]{x1D702}$
for a flexible heaving panel are quite low. In a later study focusing on panel
![]() $D$
(see table 1), Quinn, Lauder & Smits (Reference Quinn, Lauder and Smits2015) found that adding a pitching motion enhanced the efficiency considerably. Although the net thrust and power scaled as expected with the frequency and amplitude of the leading edge, the efficiency showed a complex multimodal response. For heave only motions, two optimal conditions were found, one with
$D$
(see table 1), Quinn, Lauder & Smits (Reference Quinn, Lauder and Smits2015) found that adding a pitching motion enhanced the efficiency considerably. Although the net thrust and power scaled as expected with the frequency and amplitude of the leading edge, the efficiency showed a complex multimodal response. For heave only motions, two optimal conditions were found, one with
![]() $\unicode[STIX]{x1D702}=0.23$
at
$\unicode[STIX]{x1D702}=0.23$
at
![]() $St=0.53$
, and one with
$St=0.53$
, and one with
![]() $\unicode[STIX]{x1D702}=0.21$
at
$\unicode[STIX]{x1D702}=0.21$
at
![]() $St=0.40$
, as illustrated in figure 10. For pitch and heave motions combined, two optimal conditions were also found, one for
$St=0.40$
, as illustrated in figure 10. For pitch and heave motions combined, two optimal conditions were also found, one for
![]() $St=0.26$
,
$St=0.26$
,
![]() $\unicode[STIX]{x1D702}=0.38$
, and the other for
$\unicode[STIX]{x1D702}=0.38$
, and the other for
![]() $St=0.33$
,
$St=0.33$
,
![]() $\unicode[STIX]{x1D702}=0.37$
, so the addition of pitch increased the efficiency by a factor of approximately 1.7. The conditions correspond almost exactly to a doubling of the parameter
$\unicode[STIX]{x1D702}=0.37$
, so the addition of pitch increased the efficiency by a factor of approximately 1.7. The conditions correspond almost exactly to a doubling of the parameter
![]() $\unicode[STIX]{x1D6F1}_{1}=f^{\ast }$
, that is, the non-dimensional frequency describing the resonant modes.
$\unicode[STIX]{x1D6F1}_{1}=f^{\ast }$
, that is, the non-dimensional frequency describing the resonant modes.

Figure 10. Contour plot of propulsive efficiency, heave only motions,
![]() $a^{\prime }/c=0.07$
. Adapted with permission from Quinn et al. (Reference Quinn, Lauder and Smits2015).
$a^{\prime }/c=0.07$
. Adapted with permission from Quinn et al. (Reference Quinn, Lauder and Smits2015).
In this respect, Floryan & Rowley (Reference Floryan and Rowley2018) showed that linear inviscid theory predicts that local resonant maxima in thrust and power appear, but it does not predict any local resonant maxima in efficiency, whereas such efficiency peaks are clearly observed in experiments. Therefore, the resonant peaks in efficiency are due to either finite Reynolds number effects, nonlinear effects, or both. Floryan & Rowley (Reference Floryan and Rowley2018) found that the presence of drag will always create resonant peaks in efficiency, while Ramananarivo, Godoy-Diana & Thiria (Reference Ramananarivo, Godoy-Diana and Thiria2011) showed that nonlinearities can create non-resonant peaks in efficiency for flexible flapping wings.
Figure 11 shows the phase-averaged vorticity plots corresponding to the two optima for heave and pitch combined. Although the efficiencies are almost the same, they occur at two different mode shapes, and generate very different wakes; whereas the first optimum resembles a 2S (reverse von Kármán street) structure, the second optimum is more a 2P structure. For simple flexible panels, therefore, efficient swimming can be achieved for (at least) two different wake structures.
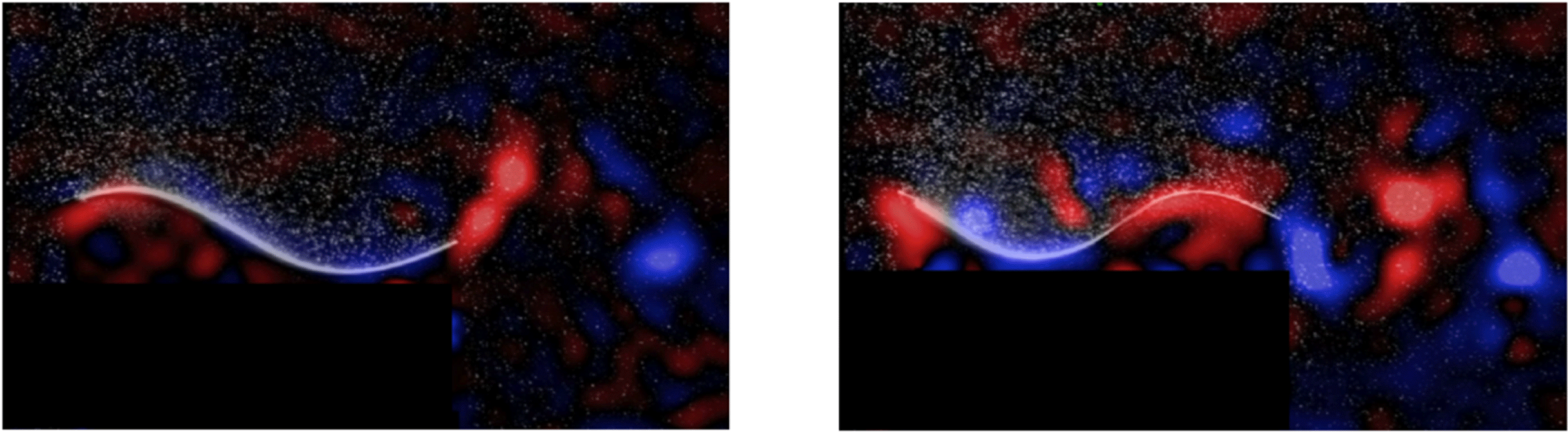
Figure 11. Phase-averaged spanwise vorticity (red is positive, blue is negative). (a) First optimum,
![]() $St=0.26$
,
$St=0.26$
,
![]() $f^{\ast }=24.8$
,
$f^{\ast }=24.8$
,
![]() $a^{\prime }/c=0.07$
,
$a^{\prime }/c=0.07$
,
![]() $\unicode[STIX]{x1D6FC}=30^{\circ }$
,
$\unicode[STIX]{x1D6FC}=30^{\circ }$
,
![]() $\unicode[STIX]{x1D719}=76^{\circ }$
,
$\unicode[STIX]{x1D719}=76^{\circ }$
,
![]() $\unicode[STIX]{x1D702}=0.38$
. (b) Second optimum,
$\unicode[STIX]{x1D702}=0.38$
. (b) Second optimum,
![]() $St=0.33$
,
$St=0.33$
,
![]() $f^{\ast }=50.8$
,
$f^{\ast }=50.8$
,
![]() $a^{\prime }/c=0.07$
,
$a^{\prime }/c=0.07$
,
![]() $\unicode[STIX]{x1D6FC}=30^{\circ }$
,
$\unicode[STIX]{x1D6FC}=30^{\circ }$
,
![]() $\unicode[STIX]{x1D719}=96^{\circ }$
,
$\unicode[STIX]{x1D719}=96^{\circ }$
,
![]() $\unicode[STIX]{x1D702}=0.37$
. Adapted with permission from Quinn et al. (Reference Quinn, Lauder and Smits2015).
$\unicode[STIX]{x1D702}=0.37$
. Adapted with permission from Quinn et al. (Reference Quinn, Lauder and Smits2015).
In general, Quinn et al. (Reference Quinn, Lauder and Smits2015) concluded that efficiency is globally optimized when (i) the Strouhal number is high enough that the flow does not separate over the peaks and troughs in the panel waveform, but low enough that the vortex cores in the wake remain tightly packed and coherent; (ii) the panel is actuated at a resonant frequency of the fluid–panel system; (iii) heave amplitude is tuned such that trailing edge amplitude is maximized while the flow along the body remains attached; and (iv) the maximum pitch angle and phase lag are chosen so that the effective angle of attack is minimized.
We now turn to oscillatory swimming.
3 Oscillatory Swimming
For fast, efficient swimming in sustained cruise, the prototypical animal is the tuna (Webb Reference Webb1984). The tuna has a streamlined (fusiform) shape that is deepest about halfway between the head and the tail (see figure 12), and its particular swimming motion is called thunniform, as described earlier. As pointed out by Lighthill (Reference Lighthill1969), the body morphology also helps to minimize recoil. For anguilliform swimmers, the unsteady side forces and yawing moments tend to cancel because they have at least one wavelength present along their body length. Carangiform and thunniform swimmers do not, but the recoil motions are minimized by having a deep anterior body and a narrowing posterior.

Figure 12. Clockwise from top left: Kawakawa (Euthynnus affinis, or mackerel tuna, License: by Attribution-Noncommercial Australian National Fish Collection, CSIRO); Dace (Leuciscus leuciscus, reproduced by attribution from http://www.fishinginireland.info/index.htm); Bream (Abramis brama, reproduced with permission from www.sommen.nu); Goldfish (Carassius auratus, reproduced by attribution from https://archive.usgs.gov/archive/sites/fl.biology.usgs.gov/Carp_ID/html/carassius_auratus.html).
Because the posterior part tapers down to a narrow peduncle, and the caudal fin planform area is relatively large by comparison, it is expected that most of the thrust production is due to the caudal fin motion, and that the contribution by the body motion is secondary. Bainbridge (Reference Bainbridge1963) analysed the swimming performance of dace, bream and goldfish, and estimated that the contribution of the caudal fin to the total thrust varied from a value of 45 % for the bream, to 65 % for the goldfish, to 84 % for the dace. He also quotes Gray (Reference Gray1933), who estimated 40 % for the whiting (Gadus merlangu). We will show that these estimates are probably low (see § 3.10), and so a useful approximation for the purposes of modelling is to assume that all of the thrust is provided by the motion of the caudal fin (or fluke, as the case may be), and, by extension, that the body is the principal (but not the only) source of drag.
We therefore focus on the hydrodynamic performance of the caudal fin. In essence, it describes a combination of heaving and pitching, naively described by sinusoidal motions at a common frequency, so that
where
![]() $h_{0}$
is the heave amplitude,
$h_{0}$
is the heave amplitude,
![]() $\unicode[STIX]{x1D703}_{0}$
is the pitch amplitude and
$\unicode[STIX]{x1D703}_{0}$
is the pitch amplitude and
![]() $\unicode[STIX]{x1D719}$
is the phase difference between pitch and heave. For reference, figure 16 illustrates the motion of a swimming foil for phase differences
$\unicode[STIX]{x1D719}$
is the phase difference between pitch and heave. For reference, figure 16 illustrates the motion of a swimming foil for phase differences
![]() $\unicode[STIX]{x1D719}=0^{\circ },90^{\circ },180^{\circ }$
and
$\unicode[STIX]{x1D719}=0^{\circ },90^{\circ },180^{\circ }$
and
![]() $270^{\circ }$
.
$270^{\circ }$
.
We now describe some simple models for thrust and efficiency of heaving and pitching foils, based on experiments and analytical considerations. We mainly consider rectangular foils as an abstraction of a caudal fin, but some aspects of the fin planform shape including the aspect ratio and the shape of the trailing edge will also be discussed. The coefficients of thrust and power are given by
where
![]() $F_{x}$
is time-averaged thrust in the streamwise direction produced by the foil motion,
$F_{x}$
is time-averaged thrust in the streamwise direction produced by the foil motion,
![]() $F_{y}$
is the force perpendicular to the free-stream direction and
$F_{y}$
is the force perpendicular to the free-stream direction and
![]() $M$
is the moment taken about the leading edge of the foil.
$M$
is the moment taken about the leading edge of the foil.
3.1 Pitching foils
We begin with a simple example that captures some of the salient characteristics of oscillatory propulsion. Consider a two-dimensional NACA0012 airfoil pitching about its quarter chord in a uniform stream of speed
![]() $U$
so that its pitch angle
$U$
so that its pitch angle
![]() $\unicode[STIX]{x1D703}$
varies as
$\unicode[STIX]{x1D703}$
varies as
![]() $\unicode[STIX]{x1D703}_{0}\sin (2\unicode[STIX]{x03C0}ft)$
. There is no heaving motion. The results for the thrust, power and efficiency shown in figure 13 were computed using the direct numerical simulations (DNS) method described by Sentürk & Smits (Reference Sentürk and Smits2018) and Sentürk et al. (Reference Sentürk, Brunner, Jasak, Herzog, Rowley and Smits2019). The Reynolds number was varied from 500 to 32 000. To put that range into perspective, consider a fish with a caudal fin that has a chord length 10 % of its overall length. A chord Reynolds number of 500 would then correspond to a fish of length 70 mm, swimming at about one body length per second, which is a typical speed for the kind of fish we are interested in. Similarly, a chord Reynolds number of 32 000 would correspond to a fish of length 560 mm, also swimming at one body length per second. The corresponding fluke Reynolds numbers for dolphins can reach
$\unicode[STIX]{x1D703}_{0}\sin (2\unicode[STIX]{x03C0}ft)$
. There is no heaving motion. The results for the thrust, power and efficiency shown in figure 13 were computed using the direct numerical simulations (DNS) method described by Sentürk & Smits (Reference Sentürk and Smits2018) and Sentürk et al. (Reference Sentürk, Brunner, Jasak, Herzog, Rowley and Smits2019). The Reynolds number was varied from 500 to 32 000. To put that range into perspective, consider a fish with a caudal fin that has a chord length 10 % of its overall length. A chord Reynolds number of 500 would then correspond to a fish of length 70 mm, swimming at about one body length per second, which is a typical speed for the kind of fish we are interested in. Similarly, a chord Reynolds number of 32 000 would correspond to a fish of length 560 mm, also swimming at one body length per second. The corresponding fluke Reynolds numbers for dolphins can reach
![]() $10^{6}$
, with a body length Reynolds number of
$10^{6}$
, with a body length Reynolds number of
![]() $10^{7}$
, and the corresponding numbers for whales could be 10 times higher.
$10^{7}$
, and the corresponding numbers for whales could be 10 times higher.

Figure 13. NACA0012 foil pitching about quarter chord from the leading edge (
![]() $\unicode[STIX]{x1D703}_{0}=8^{\circ }$
). DNS by Sentürk & Smits (Reference Sentürk and Smits2019). All data averaged over one pitching cycle. Adapted with permission from Sentürk & Smits (Reference Sentürk and Smits2019).
$\unicode[STIX]{x1D703}_{0}=8^{\circ }$
). DNS by Sentürk & Smits (Reference Sentürk and Smits2019). All data averaged over one pitching cycle. Adapted with permission from Sentürk & Smits (Reference Sentürk and Smits2019).
We first note that the mean thrust and power coefficients increase nonlinearly with Strouhal number. The thrust varies approximately as
![]() $St^{2}$
, and power varies approximately as
$St^{2}$
, and power varies approximately as
![]() $St^{3}$
(the actual scaling is considered in § 3.3). Second, we see that the thrust coefficient and the efficiency display a strong Reynolds number dependence. Third, the thrust coefficient has a negative offset at small values of the Strouhal number, which corresponds to the drag offset at very low Strouhal number (quasi-steady flow), averaged over a pitching cycle. This offset is similar to that seen by Quinn et al. (Reference Quinn, Lauder and Smits2014) for a flexible pitching panel in heave (see figure 7). Fourth, the efficiency tends to negative values at low Strouhal number (corresponding to a negative thrust coefficient), rising quickly as the Strouhal number increases, before falling more slowly at higher Strouhal numbers. The curves display a maximum value of efficiency
$St^{3}$
(the actual scaling is considered in § 3.3). Second, we see that the thrust coefficient and the efficiency display a strong Reynolds number dependence. Third, the thrust coefficient has a negative offset at small values of the Strouhal number, which corresponds to the drag offset at very low Strouhal number (quasi-steady flow), averaged over a pitching cycle. This offset is similar to that seen by Quinn et al. (Reference Quinn, Lauder and Smits2014) for a flexible pitching panel in heave (see figure 7). Fourth, the efficiency tends to negative values at low Strouhal number (corresponding to a negative thrust coefficient), rising quickly as the Strouhal number increases, before falling more slowly at higher Strouhal numbers. The curves display a maximum value of efficiency
![]() $\unicode[STIX]{x1D702}_{m}$
at a particular Strouhal number
$\unicode[STIX]{x1D702}_{m}$
at a particular Strouhal number
![]() $St^{\ast }$
, where both
$St^{\ast }$
, where both
![]() $\unicode[STIX]{x1D702}_{m}$
and
$\unicode[STIX]{x1D702}_{m}$
and
![]() $St^{\ast }$
depend strongly on Reynolds number; increasing the Reynolds number tends to increase the efficiency at all Strouhal numbers, while also notably increasing the maximum efficiency. Similar peaks in efficiency were seen for flexible panels in undulatory motion (figure 10).
$St^{\ast }$
depend strongly on Reynolds number; increasing the Reynolds number tends to increase the efficiency at all Strouhal numbers, while also notably increasing the maximum efficiency. Similar peaks in efficiency were seen for flexible panels in undulatory motion (figure 10).
These DNS results are in broad agreement with the experiments of Buchholz & Smits (Reference Buchholz and Smits2008) on rigid rectangular panels pitching about their leading edge. Buchholz et al. found that decreasing the aspect ratio of the panel monotonically decreased the thrust coefficient, while the efficiency was largely unaffected except for the smallest aspect ratio ![]() where it decreased somewhat. As to the effects of Reynolds number, their measurements were conducted over two Reynolds number ranges with average values of approximately 10 000 and 21 000. Small differences in the thrust and efficiency were observed, but conclusive trends were difficult to discern, which is perhaps not surprising given the results shown in figure 13, where
where it decreased somewhat. As to the effects of Reynolds number, their measurements were conducted over two Reynolds number ranges with average values of approximately 10 000 and 21 000. Small differences in the thrust and efficiency were observed, but conclusive trends were difficult to discern, which is perhaps not surprising given the results shown in figure 13, where
![]() $C_{D}$
is seen to vary slowly for Reynolds numbers larger than approximately 10 000, and its effects on thrust and efficiency are also seen to diminish.
$C_{D}$
is seen to vary slowly for Reynolds numbers larger than approximately 10 000, and its effects on thrust and efficiency are also seen to diminish.
It is important to note that the drag coefficient for a given foil depends on its profile shape as well as its Reynolds number. Maximizing thrust and efficiency at a given Reynolds number therefore poses an optimization problem to find the foil shape with the lowest drag coefficient for a given motion profile (Van Buren et al. Reference Van Buren, Floryan, Smits, Pan, Bode-Oke and Dong2019b ) (see also § 3.4).

Figure 14. (a,b) Dye flow visualizations. Flow is from left to right. (c,d) Vortex skeleton models of the wake for ![]() ,
,
![]() $A/s=0.31$
and
$A/s=0.31$
and
![]() $Re_{c}=640$
. (a,c)
$Re_{c}=640$
. (a,c)
![]() $St=0.23$
; (b,d)
$St=0.23$
; (b,d)
![]() $St=0.43$
. Adapted with permission from Buchholz & Smits (Reference Buchholz and Smits2005, Reference Buchholz and Smits2006).
$St=0.43$
. Adapted with permission from Buchholz & Smits (Reference Buchholz and Smits2005, Reference Buchholz and Smits2006).
In addition to considering the thrust and efficiency, Buchholz & Smits (Reference Buchholz and Smits2005, Reference Buchholz and Smits2006) visualized the wake using different colour dyes, and suggested vortex skeleton models for the wake structure (see figure 14). For these low Reynolds number wakes (
![]() $Re_{c}=640$
), three distinct and highly three-dimensional wake structures were observed as the Strouhal number was varied. For approximately
$Re_{c}=640$
), three distinct and highly three-dimensional wake structures were observed as the Strouhal number was varied. For approximately
![]() $0.20<St<0.25$
, two horseshoe vortices were shed per pitching cycle, which interacted with neighbouring structures to form a three-dimensional chain of vortex loops. When viewed along the spanwise axis, the wake resembles a transversely growing von Kármán vortex street. Two critical attributes are that the streamwise legs of the structures increase in strength (circulation) toward the trailing edge, as well as changing in strength with the phase of the motion. This influences the dynamics of the wake in that the interaction between horseshoes is dominated by the most recently created structure. It also implies the existence of spanwise vorticity bridging the legs which is a salient feature of the wakes observed at higher Strouhal numbers and is consistent with the dynamic stall vortex shed by other unsteady propulsors. For all wakes, there is a strong compression of the wake in the spanwise direction, and an expansion in the plane of the motion.
$0.20<St<0.25$
, two horseshoe vortices were shed per pitching cycle, which interacted with neighbouring structures to form a three-dimensional chain of vortex loops. When viewed along the spanwise axis, the wake resembles a transversely growing von Kármán vortex street. Two critical attributes are that the streamwise legs of the structures increase in strength (circulation) toward the trailing edge, as well as changing in strength with the phase of the motion. This influences the dynamics of the wake in that the interaction between horseshoes is dominated by the most recently created structure. It also implies the existence of spanwise vorticity bridging the legs which is a salient feature of the wakes observed at higher Strouhal numbers and is consistent with the dynamic stall vortex shed by other unsteady propulsors. For all wakes, there is a strong compression of the wake in the spanwise direction, and an expansion in the plane of the motion.
For approximately
![]() $St>0.25$
, the wake bifurcates into two oblique trains of vortex structures. At
$St>0.25$
, the wake bifurcates into two oblique trains of vortex structures. At
![]() $St=0.43$
, the individual structures are topologically similar to the structures observed at
$St=0.43$
, the individual structures are topologically similar to the structures observed at
![]() $St=0.23$
except that a portion of the spanwise shear layer found at
$St=0.23$
except that a portion of the spanwise shear layer found at
![]() $St=0.23$
is shed from the trailing edge as a discrete spanwise vortex. The resulting fundamental structure is therefore a vortex ring that is partly entrained into the tip of a horseshoe vortex. These vortex rings move away from the centreline under their own induced velocity, and the wake is seen to bifurcate. At
$St=0.23$
is shed from the trailing edge as a discrete spanwise vortex. The resulting fundamental structure is therefore a vortex ring that is partly entrained into the tip of a horseshoe vortex. These vortex rings move away from the centreline under their own induced velocity, and the wake is seen to bifurcate. At
![]() $St=0.64$
(not shown here), the wake contains an additional feature in which streamwise vortices undergo a perturbation near the trailing edge of the panel which leads to the generation of hairpin or horseshoe vortices that convect outward in the spanwise direction. This transition from a kind of 2S structure to a 2P structure with increasing Strouhal number is very similar to that seen in other cases, such as pitching and heaving plates (Guglielmini Reference Guglielmini2004; Dong et al.
Reference Dong, Mittal, Bozkurttas and Najjar2005).
$St=0.64$
(not shown here), the wake contains an additional feature in which streamwise vortices undergo a perturbation near the trailing edge of the panel which leads to the generation of hairpin or horseshoe vortices that convect outward in the spanwise direction. This transition from a kind of 2S structure to a 2P structure with increasing Strouhal number is very similar to that seen in other cases, such as pitching and heaving plates (Guglielmini Reference Guglielmini2004; Dong et al.
Reference Dong, Mittal, Bozkurttas and Najjar2005).
The wake structure was found to depend on the Reynolds number, although a similar global wake behaviour was observed at moderate Reynolds numbers of
![]() $O(10^{4})$
. Of course, the effects of Strouhal number and Reynolds number are intertwined, as made clear by the results shown in figure 13 for the NACA0012 airfoil. That is, at a given Strouhal number (for example, 0.3), the efficiency at low Reynolds number is negative, meaning the drag is larger than the thrust, whereas at higher Reynolds numbers the efficiency is positive because the thrust is larger than the drag. In this regard, the orientation of the vortices in the plane of motion for
$O(10^{4})$
. Of course, the effects of Strouhal number and Reynolds number are intertwined, as made clear by the results shown in figure 13 for the NACA0012 airfoil. That is, at a given Strouhal number (for example, 0.3), the efficiency at low Reynolds number is negative, meaning the drag is larger than the thrust, whereas at higher Reynolds numbers the efficiency is positive because the thrust is larger than the drag. In this regard, the orientation of the vortices in the plane of motion for
![]() $St=0.23$
in figure 14 suggests that for this panel at this Strouhal number and this Reynolds number the wake is drag producing. Increasing the Reynolds number will reduce the drag, lead to a net positive thrust, and a re-orientation of the vortices.
$St=0.23$
in figure 14 suggests that for this panel at this Strouhal number and this Reynolds number the wake is drag producing. Increasing the Reynolds number will reduce the drag, lead to a net positive thrust, and a re-orientation of the vortices.
3.2 Heaving and pitching foils
The results shown in figure 13 also demonstrate that the peak efficiency for this particular pitching foil does not exceed about 25 %, even at the largest Reynolds number considered. Adding a heave motion, with an appropriate phase difference can improve this result substantially. For example, figure 15 displays the time-averaged thrust coefficient and efficiency of a pitching foil with incremental increases in heave amplitude while keeping the pitch amplitude fixed at
![]() $\unicode[STIX]{x1D703}_{0}=15^{\circ }$
. Similar increases in thrust and efficiency for undulatory swimming were seen in the case of a flexible plate, where adding pitch to heave increased the optimal efficiency by a factor of about 1.7 (see figure 10). For the rigid foil shown in figure 15 the efficiency curves also exhibit a clear maximum value, suggesting that an optimum efficiency exists for any heave/pitch combination. For reduced frequencies below the optimum, the efficiency decreases sharply as the effects of the viscous drag on the propulsor become important.
$\unicode[STIX]{x1D703}_{0}=15^{\circ }$
. Similar increases in thrust and efficiency for undulatory swimming were seen in the case of a flexible plate, where adding pitch to heave increased the optimal efficiency by a factor of about 1.7 (see figure 10). For the rigid foil shown in figure 15 the efficiency curves also exhibit a clear maximum value, suggesting that an optimum efficiency exists for any heave/pitch combination. For reduced frequencies below the optimum, the efficiency decreases sharply as the effects of the viscous drag on the propulsor become important.

Figure 15. Pitching foil with incremental increases in heave amplitude for
![]() $\unicode[STIX]{x1D719}=270^{\circ }$
(
$\unicode[STIX]{x1D719}=270^{\circ }$
(
![]() $f^{\ast }=k$
). (a) Thrust coefficient; (b) efficiency. Reproduction with permission from Van Buren, Floryan & Smits (Reference Van Buren, Floryan and Smits2018a
).
$f^{\ast }=k$
). (a) Thrust coefficient; (b) efficiency. Reproduction with permission from Van Buren, Floryan & Smits (Reference Van Buren, Floryan and Smits2018a
).
When combining sinusoidal heaving and pitching motions the phase offset is a critical parameter. Figure 16 illustrates the motion of a swimming foil for phase differences
![]() $\unicode[STIX]{x1D719}=0^{\circ },90^{\circ },180^{\circ }$
and
$\unicode[STIX]{x1D719}=0^{\circ },90^{\circ },180^{\circ }$
and
![]() $270^{\circ }$
. When heave and pitch are in phase (
$270^{\circ }$
. When heave and pitch are in phase (
![]() $\unicode[STIX]{x1D719}=0$
), the motion appears to an observer moving with the foil as if the foil is pitching about some point upstream of the leading edge. For phase angles around
$\unicode[STIX]{x1D719}=0$
), the motion appears to an observer moving with the foil as if the foil is pitching about some point upstream of the leading edge. For phase angles around
![]() $\unicode[STIX]{x1D719}=90^{\circ }$
, the trailing edge leads the leading edge, and when
$\unicode[STIX]{x1D719}=90^{\circ }$
, the trailing edge leads the leading edge, and when
![]() $\unicode[STIX]{x1D719}=180^{\circ }$
the foil appears to pitch about a point behind the leading edge. For
$\unicode[STIX]{x1D719}=180^{\circ }$
the foil appears to pitch about a point behind the leading edge. For
![]() $\unicode[STIX]{x1D719}=270^{\circ }$
the motion seems to be the most ‘fish-like’, cleanly slicing through the water with the lowest angles of attack (the angle between the foil and its instantaneous direction of motion). This case is further illustrated in figure 17. Compared to heave-only motions, greater heave velocities can be achieved for the same angle of attack by adding the appropriate pitch. The increased heave velocity increases the thrust component and rotates more of the lift vector in the thrust direction, increasing the efficiency. As a useful measure of the relative magnitudes of pitching and heaving, Lighthill (Reference Lighthill1969, Reference Lighthill1970) introduced the proportional-feathering parameter,
$\unicode[STIX]{x1D719}=270^{\circ }$
the motion seems to be the most ‘fish-like’, cleanly slicing through the water with the lowest angles of attack (the angle between the foil and its instantaneous direction of motion). This case is further illustrated in figure 17. Compared to heave-only motions, greater heave velocities can be achieved for the same angle of attack by adding the appropriate pitch. The increased heave velocity increases the thrust component and rotates more of the lift vector in the thrust direction, increasing the efficiency. As a useful measure of the relative magnitudes of pitching and heaving, Lighthill (Reference Lighthill1969, Reference Lighthill1970) introduced the proportional-feathering parameter,
![]() $\unicode[STIX]{x1D6E9}$
defined so that
$\unicode[STIX]{x1D6E9}$
defined so that
![]() $\unicode[STIX]{x1D6FC}_{m}=\unicode[STIX]{x1D6E9}V/U$
, where
$\unicode[STIX]{x1D6FC}_{m}=\unicode[STIX]{x1D6E9}V/U$
, where
![]() $\unicode[STIX]{x1D6FC}_{m}$
is the maximum angle of attack. Therefore
$\unicode[STIX]{x1D6FC}_{m}$
is the maximum angle of attack. Therefore
![]() $\unicode[STIX]{x1D6E9}$
is a parameter that measures the reduction in the instantaneous angle of attack from its maximum value, and decreasing
$\unicode[STIX]{x1D6E9}$
is a parameter that measures the reduction in the instantaneous angle of attack from its maximum value, and decreasing
![]() $\unicode[STIX]{x1D6E9}$
tends to increase thrust while decreasing the efficiency.
$\unicode[STIX]{x1D6E9}$
tends to increase thrust while decreasing the efficiency.

Figure 16. Motion of a foil swimming from left to right via heave and pitch motions with a phase offset (a)
![]() $\unicode[STIX]{x1D719}=0^{\circ }$
, (b)
$\unicode[STIX]{x1D719}=0^{\circ }$
, (b)
![]() $90^{\circ }$
, (c)
$90^{\circ }$
, (c)
![]() $180^{\circ }$
and (d)
$180^{\circ }$
and (d)
![]() $270^{\circ }$
. In this example,
$270^{\circ }$
. In this example,
![]() $h_{0}/c=0.375$
,
$h_{0}/c=0.375$
,
![]() $\unicode[STIX]{x1D703}_{0}=15^{\circ }$
and
$\unicode[STIX]{x1D703}_{0}=15^{\circ }$
and
![]() $f^{\ast }=0.16$
. Reproduction with permission from Van Buren et al. (Reference Van Buren, Floryan and Smits2018a
).
$f^{\ast }=0.16$
. Reproduction with permission from Van Buren et al. (Reference Van Buren, Floryan and Smits2018a
).

Figure 17. Heaving foil at the same instantaneous angle of attack (a) without and (b) with added pitch motion (
![]() $\unicode[STIX]{x1D719}=270^{\circ }$
). Streamwise, heave and effective velocities shown in red, resulting lift-based forces shown in blue. Reproduction with permission from Van Buren et al. (Reference Van Buren, Floryan and Smits2018a
).
$\unicode[STIX]{x1D719}=270^{\circ }$
). Streamwise, heave and effective velocities shown in red, resulting lift-based forces shown in blue. Reproduction with permission from Van Buren et al. (Reference Van Buren, Floryan and Smits2018a
).
The performance of submerged foils in combined heaving and pitching motion has been studied relatively extensively (Lighthill Reference Lighthill1970; Dickinson Reference Dickinson1996; Sfakiotakis et al.
Reference Sfakiotakis, Lane and Davies1999; Triantafyllou et al.
Reference Triantafyllou, Triantafyllou and Yue2000; Von Ellenrieder, Parker & Soria Reference Von Ellenrieder, Parker and Soria2003). In a particularly influential work, Anderson et al. (Reference Anderson, Streitlien, Barrett and Triantafyllou1998) obtained efficiencies as high as 87 % using a heaving and pitching two-dimensional NACA0012 airfoil in sinusoidal motion. They connected the wake structure to the performance of the foil, arguing that for maximum efficiency the leading edge vortex pair needs to interact beneficially with the trailing edge vorticity. The flow visualization was performed at
![]() $Re=1000$
, whereas their force measurements were at
$Re=1000$
, whereas their force measurements were at
![]() $Re=40\,000$
. Subsequent research suggests that it is necessary to avoid leading edge vortices altogether in order to maximize efficiency (Tuncer & Kaya Reference Tuncer and Kaya2005; Young et al.
Reference Young, Lai, Kaya and Tuncer2006; Young & Lai Reference Young and Lai2007). In related work, Read, Hover & Triantafyllou (Reference Read, Hover and Triantafyllou2003) recognized the importance of the peak angle of attack when considering performance, although they reported lower values of efficiency (55 %–70 %) than the 87 % reported by Anderson et al. (Reference Anderson, Streitlien, Barrett and Triantafyllou1998) in the same laboratory under similar experimental conditions. We take that to indicate the crucial role played by drag in determining the efficiency, as we will see. Experiments on large amplitude motions by Scherer (Reference Scherer1968) showed similar peak efficiency values to those found by Read et al. (Reference Read, Hover and Triantafyllou2003), over a wide range of parameters. We now present some simple models for the thrust production, power expenditure, and efficiency of heaving and pitching foils, to help explain these trends.
$Re=40\,000$
. Subsequent research suggests that it is necessary to avoid leading edge vortices altogether in order to maximize efficiency (Tuncer & Kaya Reference Tuncer and Kaya2005; Young et al.
Reference Young, Lai, Kaya and Tuncer2006; Young & Lai Reference Young and Lai2007). In related work, Read, Hover & Triantafyllou (Reference Read, Hover and Triantafyllou2003) recognized the importance of the peak angle of attack when considering performance, although they reported lower values of efficiency (55 %–70 %) than the 87 % reported by Anderson et al. (Reference Anderson, Streitlien, Barrett and Triantafyllou1998) in the same laboratory under similar experimental conditions. We take that to indicate the crucial role played by drag in determining the efficiency, as we will see. Experiments on large amplitude motions by Scherer (Reference Scherer1968) showed similar peak efficiency values to those found by Read et al. (Reference Read, Hover and Triantafyllou2003), over a wide range of parameters. We now present some simple models for the thrust production, power expenditure, and efficiency of heaving and pitching foils, to help explain these trends.
3.3 Analysis of heaving or pitching foils
The analysis of heaving and pitching plates and foils has a distinguished history, dating back to Theodorsen (Reference Theodorsen1935), who first derived the linearized expressions for the forces generated by an oscillating foil in the context of aerodynamic flutter. His analysis included the contributions due to circulatory and added mass forces, and Garrick (Reference Garrick1936) used his results to develop expressions for thrust and power for a two-dimensional, rigid propulsor. We now follow Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a ), Floryan, Van Buren & Smits (Reference Floryan, Van Buren and Smits2018) and Van Buren et al. (Reference Van Buren, Floryan and Smits2018a ) to consider a foil moving in heave and pitch as a simplified model of an isolated propulsor. The unsteady lift model of Theodorsen (Reference Theodorsen1935) will be combined with the added mass force model by Sedov (Reference Sedov1965) to construct scaling relations for the thrust and efficiency. The added mass model given by Sedov (Reference Sedov1965) is preferred to that given by Theodorsen (Reference Theodorsen1935) because Sedov’s model includes both the normal and tangential contributions. In this section, we examine either heaving or pitching foils in sinusoidal motion, as described by (3.1). Combined motions are studied in the following section.
The only circulatory (lift-based) forces considered are those that arise when the foil is at an instantaneous angle of attack to the free stream given by
![]() $\unicode[STIX]{x1D6FC}$
. The effective flow velocity seen by the foil has a magnitude
$\unicode[STIX]{x1D6FC}$
. The effective flow velocity seen by the foil has a magnitude
![]() $U_{eff}=\sqrt{U^{2}+{\dot{h}}^{2}}$
, and an angle relative to the free-stream velocity of
$U_{eff}=\sqrt{U^{2}+{\dot{h}}^{2}}$
, and an angle relative to the free-stream velocity of
![]() $\arctan ({\dot{h}}/U)$
, so that
$\arctan ({\dot{h}}/U)$
, so that
![]() $\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D703}-\arctan ({\dot{h}}/U)$
(notation as given in figure 18). Hence, for a foil of chord
$\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D703}-\arctan ({\dot{h}}/U)$
(notation as given in figure 18). Hence, for a foil of chord
![]() $c$
and span
$c$
and span
![]() $s$
,
$s$
,
Here,
![]() $F_{x}$
is the thrust,
$F_{x}$
is the thrust,
![]() $F_{y}$
is the lateral force,
$F_{y}$
is the lateral force,
![]() $L$
is the lift on the foil given by
$L$
is the lift on the foil given by
![]() $L=(1/2)\unicode[STIX]{x1D70C}U_{eff}^{2}scC_{L}$
and the lift coefficient
$L=(1/2)\unicode[STIX]{x1D70C}U_{eff}^{2}scC_{L}$
and the lift coefficient
![]() $C_{L}=2\unicode[STIX]{x03C0}\sin \unicode[STIX]{x1D6FC}+(3/2)\unicode[STIX]{x03C0}\dot{\unicode[STIX]{x1D6FC}}c/U$
(Theodorsen Reference Theodorsen1935). The moment about the leading edge is
$C_{L}=2\unicode[STIX]{x03C0}\sin \unicode[STIX]{x1D6FC}+(3/2)\unicode[STIX]{x03C0}\dot{\unicode[STIX]{x1D6FC}}c/U$
(Theodorsen Reference Theodorsen1935). The moment about the leading edge is
![]() $M=-cL/4$
. The wake correction term due to downwash (Theodorsen’s lift deficiency factor) is neglected as it is approximately constant under the conditions considered here.
$M=-cL/4$
. The wake correction term due to downwash (Theodorsen’s lift deficiency factor) is neglected as it is approximately constant under the conditions considered here.

Figure 18. (a) Notation. (b) Lift-based thrust generation for a foil in pure heave. Adapted from the original shown in Katz & Plotkin (Reference Katz and Plotkin2001).
Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a ) combined circulatory and added mass-based contributions to the thrust and power for pitching or heaving foils. They showed that. the mean thrust generated by heaving motions is entirely lift-based (see figure 18), whereas mean thrust generated by pitching motions is from added mass alone. However, for both heave and pitch motions, the mean input power (and thus efficiency) depends on lift-based and added mass forces. In contrast, slender body theory, and the analysis of undulatory motions in general, assumes that the only forces acting are those due to added mass.
We can anticipate some later results by first doing a simple scaling analysis (see also Dewey et al.
Reference Dewey, Boschitsch, Moored, Stone and Smits2013, Quinn et al.
Reference Quinn, Lauder and Smits2015). We expect that the thrust generated by a purely pitching motion will scale as the component in the streamwise direction of the added mass (
![]() ${\sim}\unicode[STIX]{x1D70C}c^{2}s$
for two-dimensional panels, that is,
${\sim}\unicode[STIX]{x1D70C}c^{2}s$
for two-dimensional panels, that is, ![]() ) times the acceleration (
) times the acceleration (
![]() ${\sim}c\ddot{\unicode[STIX]{x1D703}}$
). Hence,
${\sim}c\ddot{\unicode[STIX]{x1D703}}$
). Hence,
so that the mean thrust scales as
where
![]() $V$
is the amplitude of the trailing edge velocity.
$V$
is the amplitude of the trailing edge velocity.
For a purely heaving motion, the thrust is expected to scale as the component in the streamwise direction of the instantaneous lift force. That is, from (3.4),
If we assume that the contribution to the lift is quasi-steady, and that for small angles of attack
![]() $\unicode[STIX]{x1D6FC}\approx {\dot{h}}/U_{eff}$
, then
$\unicode[STIX]{x1D6FC}\approx {\dot{h}}/U_{eff}$
, then
so that the mean thrust scales as
We see that for both pitch and heave, to this level of approximation, the time-averaged thrust depends on the velocity of the trailing edge, and it is independent of the flow velocity. The experimental results given by Van Buren et al. (Reference Van Buren, Floryan, Wei and Smits2018b
) demonstrated that this scaling with
![]() $V$
for heaving and pitching foils holds well for a twofold change in mean velocity, and oscillations in the flow velocity of up to 38 %. That is, the most important velocity scale for describing the thrust performance is not the flow velocity but the characteristic velocity of the trailing edge. This is completely in accord with the conclusions drawn from slender body theory (§ 2.1), although here we considered both circulatory and added mass forces. It is also not unexpected. The early work by Garrick (Reference Garrick1936) on flapping and oscillating airfoils had already indicated that the mean thrust should depend approximately on
$V$
for heaving and pitching foils holds well for a twofold change in mean velocity, and oscillations in the flow velocity of up to 38 %. That is, the most important velocity scale for describing the thrust performance is not the flow velocity but the characteristic velocity of the trailing edge. This is completely in accord with the conclusions drawn from slender body theory (§ 2.1), although here we considered both circulatory and added mass forces. It is also not unexpected. The early work by Garrick (Reference Garrick1936) on flapping and oscillating airfoils had already indicated that the mean thrust should depend approximately on
![]() $V^{2}$
(Garrick’s equation 29 simplified), and in the context of fish swimming Bainbridge (Reference Bainbridge1963) indicated that the thrust should depend on ‘the square of its speed of transverse movement’, although his reasoning is unclear. More recently, Gazzola, Argentina & Mahadevan (Reference Gazzola, Argentina and Mahadevan2014) offered a mechanistic basis for the importance of the transverse tail velocity, and they showed that for added mass forces the thrust should scale as
$V^{2}$
(Garrick’s equation 29 simplified), and in the context of fish swimming Bainbridge (Reference Bainbridge1963) indicated that the thrust should depend on ‘the square of its speed of transverse movement’, although his reasoning is unclear. More recently, Gazzola, Argentina & Mahadevan (Reference Gazzola, Argentina and Mahadevan2014) offered a mechanistic basis for the importance of the transverse tail velocity, and they showed that for added mass forces the thrust should scale as
![]() $V^{2}$
. However, aerodynamic forces are important when heaving motions are present, and so considerations of pitching and heaving propulsors need to take into account both added mass and lift-based forces (Floryan et al.
Reference Floryan, Van Buren, Rowley and Smits2017a
).
$V^{2}$
. However, aerodynamic forces are important when heaving motions are present, and so considerations of pitching and heaving propulsors need to take into account both added mass and lift-based forces (Floryan et al.
Reference Floryan, Van Buren, Rowley and Smits2017a
).
Equations (3.6) and (3.9) also suggest that the thrust coefficient
![]() $C_{T}$
(as conventionally defined) scales with
$C_{T}$
(as conventionally defined) scales with
![]() $St^{2}$
. However, this simple scaling argument neglects the drag on the propulsor, and as we saw earlier (as in figure 13) the drag can have an important effect on the total thrust. Thus the conclusions embodied in (3.6) and (3.9) will only hold when the drag is small relative to the total thrust. As suggested by the results shown in figure 13, this requires the Reynolds number and Strouhal number to be sufficiently large.
$St^{2}$
. However, this simple scaling argument neglects the drag on the propulsor, and as we saw earlier (as in figure 13) the drag can have an important effect on the total thrust. Thus the conclusions embodied in (3.6) and (3.9) will only hold when the drag is small relative to the total thrust. As suggested by the results shown in figure 13, this requires the Reynolds number and Strouhal number to be sufficiently large.
A more complete treatment, as given by Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a
), includes the unsteady effects embodied by
![]() $\dot{\unicode[STIX]{x1D6FC}}$
, the interaction between lift-based and added mass forces, and the drag on the propulsor. In addition, high-frequency and large-amplitude motions will undoubtedly strengthen the nonlinearities in the response; the work of Liu et al. (Reference Liu, Wang, Zhang and He2014) suggests that this will alter the phase differences between forces and motions. As such, terms that are expected to be
$\dot{\unicode[STIX]{x1D6FC}}$
, the interaction between lift-based and added mass forces, and the drag on the propulsor. In addition, high-frequency and large-amplitude motions will undoubtedly strengthen the nonlinearities in the response; the work of Liu et al. (Reference Liu, Wang, Zhang and He2014) suggests that this will alter the phase differences between forces and motions. As such, terms that are expected to be
![]() $90^{\circ }$
out of phase (for example, displacement and velocity, or velocity and acceleration) may develop in-phase components. These phase shifts are included in the analysis that follows, although they will be assumed to remain fixed for simplicity. In this way, some degree of nonlinearity is retained in an otherwise linear model.
$90^{\circ }$
out of phase (for example, displacement and velocity, or velocity and acceleration) may develop in-phase components. These phase shifts are included in the analysis that follows, although they will be assumed to remain fixed for simplicity. In this way, some degree of nonlinearity is retained in an otherwise linear model.
Following Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a ), we obtain for purely heaving motions
 $$\begin{eqnarray}\displaystyle \left.\begin{array}{@{}c@{}}C_{T}=c_{1}St_{h}^{2}+c_{2}St_{h}^{2}\,k\,U^{\ast }-C_{Dh},\\ C_{P}=c_{3}St_{h}^{2}+c_{4}St_{h}^{2}\,k+c_{5}St_{h}^{2}\,k\,U^{\ast },\\ \displaystyle \unicode[STIX]{x1D702}=\frac{c_{1}+c_{2}kU^{\ast }}{c_{3}+c_{4}k+c_{5}kU^{\ast }},\end{array}\right\} & & \displaystyle\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \left.\begin{array}{@{}c@{}}C_{T}=c_{1}St_{h}^{2}+c_{2}St_{h}^{2}\,k\,U^{\ast }-C_{Dh},\\ C_{P}=c_{3}St_{h}^{2}+c_{4}St_{h}^{2}\,k+c_{5}St_{h}^{2}\,k\,U^{\ast },\\ \displaystyle \unicode[STIX]{x1D702}=\frac{c_{1}+c_{2}kU^{\ast }}{c_{3}+c_{4}k+c_{5}kU^{\ast }},\end{array}\right\} & & \displaystyle\end{eqnarray}$$
where
![]() $C_{Dh}$
is the drag coefficient on the propulsor for heaving motions. Here,
$C_{Dh}$
is the drag coefficient on the propulsor for heaving motions. Here,
![]() $U^{\ast }=U_{eff}/U$
, and
$U^{\ast }=U_{eff}/U$
, and
![]() $St_{h}=2fh_{0}/U$
. The constants
$St_{h}=2fh_{0}/U$
. The constants
![]() $c_{i}$
need to be determined by experiment. The thrust coefficient demonstrates a
$c_{i}$
need to be determined by experiment. The thrust coefficient demonstrates a
![]() $St_{h}^{2}$
scaling, but the reduced frequency and the effective velocity also make an appearance (however, for small motions
$St_{h}^{2}$
scaling, but the reduced frequency and the effective velocity also make an appearance (however, for small motions
![]() $U^{\ast }\approx 1$
). The expression for the efficiency neglects the drag force, and this inviscid estimate depends only on
$U^{\ast }\approx 1$
). The expression for the efficiency neglects the drag force, and this inviscid estimate depends only on
![]() $k$
and
$k$
and
![]() $U^{\ast }$
. Furthermore, for large
$U^{\ast }$
. Furthermore, for large
![]() $k$
, the inviscid efficiency tends to a constant value.
$k$
, the inviscid efficiency tends to a constant value.
Similarly, for purely pitching motions, Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a ) finds
 $$\begin{eqnarray}\displaystyle \left.\begin{array}{@{}c@{}}C_{T}=c_{6}St_{\unicode[STIX]{x1D703}}^{2}+c_{7}St_{\unicode[STIX]{x1D703}}\unicode[STIX]{x1D703}_{0}-C_{Dp},\\ C_{P}=c_{8}St_{\unicode[STIX]{x1D703}}^{2}+c_{9}St_{\unicode[STIX]{x1D703}}^{2}\,k,\\ \displaystyle \unicode[STIX]{x1D702}=\frac{1}{k}\frac{c_{6}k+c_{7}/2}{c_{9}k+c_{8}},\end{array}\right\} & & \displaystyle\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \left.\begin{array}{@{}c@{}}C_{T}=c_{6}St_{\unicode[STIX]{x1D703}}^{2}+c_{7}St_{\unicode[STIX]{x1D703}}\unicode[STIX]{x1D703}_{0}-C_{Dp},\\ C_{P}=c_{8}St_{\unicode[STIX]{x1D703}}^{2}+c_{9}St_{\unicode[STIX]{x1D703}}^{2}\,k,\\ \displaystyle \unicode[STIX]{x1D702}=\frac{1}{k}\frac{c_{6}k+c_{7}/2}{c_{9}k+c_{8}},\end{array}\right\} & & \displaystyle\end{eqnarray}$$
where
![]() $C_{Dp}$
is the drag coefficient for pitching motions, and
$C_{Dp}$
is the drag coefficient for pitching motions, and
![]() $St_{\unicode[STIX]{x1D703}}=2fc\unicode[STIX]{x1D703}_{0}/U$
. Note that the expression for efficiency only holds in the limit of negligible drag, and that this inviscid estimate is independent of the Strouhal number, and depends only on the reduced frequency, falling off as
$St_{\unicode[STIX]{x1D703}}=2fc\unicode[STIX]{x1D703}_{0}/U$
. Note that the expression for efficiency only holds in the limit of negligible drag, and that this inviscid estimate is independent of the Strouhal number, and depends only on the reduced frequency, falling off as
![]() $k^{-1}$
for large
$k^{-1}$
for large
![]() $k$
.
$k$
.
These results on efficiency may be compared to those of two-dimensional small-amplitude theory. Wu (Reference Wu1971c
) considered the problem of a rigid plate in sinusoidal pitching and heaving motions in inviscid flow. For pure pitch, the efficiency was found to increase monotonically from a value of zero at
![]() $k=1.781/\unicode[STIX]{x03C0}$
(for smaller values of the reduced frequency the thrust was negative) to a value of 0.5 at
$k=1.781/\unicode[STIX]{x03C0}$
(for smaller values of the reduced frequency the thrust was negative) to a value of 0.5 at
![]() $k=\infty$
. For pure heave, he found that the efficiency monotonically decreased from a value of one at
$k=\infty$
. For pure heave, he found that the efficiency monotonically decreased from a value of one at
![]() $k=0$
to a value of about 0.5 at
$k=0$
to a value of about 0.5 at
![]() $k=\infty$
. These predictions are rather different from those given by (3.11) and (3.10), so we now consider the comparisons with experiment.
$k=\infty$
. These predictions are rather different from those given by (3.11) and (3.10), so we now consider the comparisons with experiment.
The results for a heaving foil are given in figures 19 and 20. The thrust data collapse well onto a single curve, suggesting that the simplified physics used in the model are sufficient to explain the behaviour of the thrust. In (3.10), the
![]() $St_{h}^{2}$
term is rooted in circulatory forces due to the angle of attack, and the
$St_{h}^{2}$
term is rooted in circulatory forces due to the angle of attack, and the
![]() $St_{h}^{2}\,kU^{\ast }$
term corresponds to the rate of change of angle of attack. We see therefore that the thrust for heaving motions is entirely due to lift-based forces, and that the effects of unsteadiness on the mean thrust appear to be well captured by the rate of change of angle of attack. The data also suggest that the drag coefficient
$St_{h}^{2}\,kU^{\ast }$
term corresponds to the rate of change of angle of attack. We see therefore that the thrust for heaving motions is entirely due to lift-based forces, and that the effects of unsteadiness on the mean thrust appear to be well captured by the rate of change of angle of attack. The data also suggest that the drag coefficient
![]() $C_{Dh}$
is small (of
$C_{Dh}$
is small (of
![]() $O(0.1)$
) and approximately constant over the conditions explored in the experiment.
$O(0.1)$
) and approximately constant over the conditions explored in the experiment.

Figure 19. Heaving motions of a tear-drop shaped foil. Time-averaged (a) thrust and (b) power coefficients as functions of the scaling parameters (3.10) for various
![]() $h^{\ast }=h_{0}/c$
. Adapted with permission from Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a
).
$h^{\ast }=h_{0}/c$
. Adapted with permission from Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a
).

Figure 20. Heaving motions of a tear-drop shaped foil. Efficiency as a function of (a) Strouhal number
![]() $St$
, and (b) reduced frequency
$St$
, and (b) reduced frequency
![]() $k$
. In (b) solid lines indicate the scaling given by (3.10); short dashed line indicates the scaling with
$k$
. In (b) solid lines indicate the scaling given by (3.10); short dashed line indicates the scaling with
![]() $C_{Dh}=0$
and large
$C_{Dh}=0$
and large
![]() $k$
. Adapted with permission from Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a
).
$k$
. Adapted with permission from Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a
).
Likewise, the power data collapse well onto a single curve, although there is some spread in the data for the stronger motions. The angle of attack, the rate of change of angle of attack and added mass contribute to the power scaling. Power for heaving motions is thus affected by both lift-based and added mass forces, and the essential effects of unsteadiness on the mean power are well captured by the rate of change of angle of attack and added mass. The fact that the mean power is a weakly nonlinear function of the scaling parameters suggests the limits of our model; this is likely caused by the modification of the added mass (Liu et al. Reference Liu, Wang, Zhang and He2014), although Moored & Quinn (Reference Moored and Quinn2018) propose an alternative explanation that considers the energy shed into the wake.
The efficiency data are given in figure 20, presented both as a function of Strouhal number and the reduced frequency. For heaving motions, the scaling arguments indicate that the efficiency in the absence of drag should be approximately constant (for these constants and this range of parameters). For higher values of the reduced frequency we see that the efficiency data indeed approach a constant, marked by the dashed line. The efficiency deviates from this trend for lower values of the reduced frequency and for smaller heave amplitudes due to the viscous drag on the foil. As motions become weaker, they produce less thrust. The drag, however, remains essentially constant. Thus, as the motions become weaker, the drag will constitute a larger portion of the net streamwise force, eventually overtaking any thrust produced and leading to a negative efficiency. The solid lines in figure 20(b) show the curve fits of our model after taking the drag into account, where
![]() $C_{Dh}$
was estimated from the thrust measurements at zero frequency.
$C_{Dh}$
was estimated from the thrust measurements at zero frequency.
The results on pitch-only motions are similar to those for heave-only motions, in that the scaling given here collapses the data onto a single curve for thrust and power (see Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a
) for details). The drag coefficient
![]() $C_{Dp}$
was found to be small (
$C_{Dp}$
was found to be small (
![]() ${\approx}0.08$
) and the efficiency data for large values of the reduced frequency followed the
${\approx}0.08$
) and the efficiency data for large values of the reduced frequency followed the
![]() $k^{-1}$
scaling predicted by the model.
$k^{-1}$
scaling predicted by the model.
3.4 Analysis of heave and pitch motions combined
To find the total thrust and power expended by the foil in a combined heaving and pitching motion, Van Buren et al. (Reference Van Buren, Floryan and Smits2018a
) used the same scaling advanced in the previous section. All the motions were assumed to be small, which also implies that
![]() $U^{\ast }\approx 1$
, and the out-of-phase terms were retained, as in Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a
). Van Buren et al. (Reference Van Buren, Floryan and Smits2018a
) gives
$U^{\ast }\approx 1$
, and the out-of-phase terms were retained, as in Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a
). Van Buren et al. (Reference Van Buren, Floryan and Smits2018a
) gives
The total motion Strouhal number is defined by
![]() $St=2fa/U$
, and
$St=2fa/U$
, and
![]() $St^{2}=St_{h}^{2}+St_{\unicode[STIX]{x1D703}}^{2}+2St_{h}St_{\unicode[STIX]{x1D703}}\cos \unicode[STIX]{x1D719}$
. In the special cases of pure pitch (
$St^{2}=St_{h}^{2}+St_{\unicode[STIX]{x1D703}}^{2}+2St_{h}St_{\unicode[STIX]{x1D703}}\cos \unicode[STIX]{x1D719}$
. In the special cases of pure pitch (
![]() $h_{0}=0$
) and pure heave (
$h_{0}=0$
) and pure heave (
![]() $\unicode[STIX]{x1D703}_{0}=0$
), these expressions for thrust and power reduce to those given in (3.11) and (3.10), as required.
$\unicode[STIX]{x1D703}_{0}=0$
), these expressions for thrust and power reduce to those given in (3.11) and (3.10), as required.

Figure 21. Scaling of the time-averaged (a) thrust and (b) power coefficients for all motion amplitudes and phases for the tear-drop shaped foil tested by Van Buren et al. (Reference Van Buren, Floryan and Smits2018a ). Adapted with permission from Van Buren et al. (Reference Van Buren, Floryan and Smits2018a ).
To verify these scaling relationships, Van Buren et al. (Reference Van Buren, Floryan and Smits2018a
) performed experiments over a wide range of motion amplitudes and phase differences
![]() $[0^{\circ },360^{\circ }]$
, for the same tear-drop shaped foil used by Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a
). The results shown in figure 21 display the expected linear relationship between the data and the model thrust, but again the power shows a slight nonlinear behaviour. To make the power scaling linear, we would need to add a higher-order term (one in
$[0^{\circ },360^{\circ }]$
, for the same tear-drop shaped foil used by Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a
). The results shown in figure 21 display the expected linear relationship between the data and the model thrust, but again the power shows a slight nonlinear behaviour. To make the power scaling linear, we would need to add a higher-order term (one in
![]() $St^{3}$
), but that is beyond the level of the current analysis. The coefficients were determined via linear regression over the entire experimental dataset.
$St^{3}$
), but that is beyond the level of the current analysis. The coefficients were determined via linear regression over the entire experimental dataset.
A number of useful approximations can now be made (Van Buren et al.
Reference Van Buren, Floryan and Smits2018a
; Floryan et al.
Reference Floryan, Van Buren and Smits2018). The heave-based term in the power,
![]() $kSt_{h}^{2}$
, which originates from the out-of-phase
$kSt_{h}^{2}$
, which originates from the out-of-phase
![]() $\ddot{h}{\dot{h}}$
term, made an important contribution to the power, and the drag term
$\ddot{h}{\dot{h}}$
term, made an important contribution to the power, and the drag term
![]() $\overline{C_{D}}$
was found to have a linear dependence on the pitch amplitude
$\overline{C_{D}}$
was found to have a linear dependence on the pitch amplitude
![]() $\unicode[STIX]{x1D703}_{0}$
, that is, a linear dependence on the projected frontal area for slow motions (
$\unicode[STIX]{x1D703}_{0}$
, that is, a linear dependence on the projected frontal area for slow motions (
![]() $f\rightarrow 0$
). Furthermore, the pitch-based terms in the thrust and power (associated with constants
$f\rightarrow 0$
). Furthermore, the pitch-based terms in the thrust and power (associated with constants
![]() $c_{3}$
,
$c_{3}$
,
![]() $c_{9}$
and
$c_{9}$
and
![]() $c_{10}$
) can be neglected without much penalty on the data collapse. Such terms are all of order
$c_{10}$
) can be neglected without much penalty on the data collapse. Such terms are all of order
![]() $O(\unicode[STIX]{x1D703}_{0}^{2})$
, so this is not surprising.
$O(\unicode[STIX]{x1D703}_{0}^{2})$
, so this is not surprising.
Neglecting these terms, and noting that for biologically relevant motions where phase angles are confined to the range
![]() $0^{\circ }$
–
$0^{\circ }$
–
![]() $270^{\circ }$
the terms associated with
$270^{\circ }$
the terms associated with
![]() $c_{2}$
and
$c_{2}$
and
![]() $c_{7}$
are small compared to the other terms, Van Buren et al. (Reference Van Buren, Floryan and Smits2018a
) found that
$c_{7}$
are small compared to the other terms, Van Buren et al. (Reference Van Buren, Floryan and Smits2018a
) found that
where
![]() $a_{i}$
are new empirical constants. We see that the thrust and power are now independent of phase. Furthermore, based on the values of the constants in (3.14)–(3.15) as determined from the experiment, we can propose an approximate model where
$a_{i}$
are new empirical constants. We see that the thrust and power are now independent of phase. Furthermore, based on the values of the constants in (3.14)–(3.15) as determined from the experiment, we can propose an approximate model where
Plotting the thrust and power data against expressions (3.16)–(3.17) yields figure 22. The collapse using these reduced models is similar to that seen using the full expressions (compare with figure 21). Hence,
where the constant
![]() $c_{4}$
sets the relative importance of the drag term compared to the thrust term (in general, we expect
$c_{4}$
sets the relative importance of the drag term compared to the thrust term (in general, we expect
![]() $c_{4}$
to be a function of Reynolds number). Also,
$c_{4}$
to be a function of Reynolds number). Also,
![]() $A^{\ast }=a/c$
,
$A^{\ast }=a/c$
,
![]() $H^{\ast }=H=h_{0}/a$
and
$H^{\ast }=H=h_{0}/a$
and
![]() $\unicode[STIX]{x1D6E9}^{\ast }=c\unicode[STIX]{x1D703}_{0}/a$
. Parenthetically, at a given Strouhal number, the thrust in combined heave and pitch was consistently higher than the thrust in pitch only by a factor of approximately 5 (this observation will become useful in § 3.10).
$\unicode[STIX]{x1D6E9}^{\ast }=c\unicode[STIX]{x1D703}_{0}/a$
. Parenthetically, at a given Strouhal number, the thrust in combined heave and pitch was consistently higher than the thrust in pitch only by a factor of approximately 5 (this observation will become useful in § 3.10).

Figure 22. Thrust and power data plotted against expressions (3.16)–(3.17) for
![]() $\unicode[STIX]{x1D719}=0^{\circ }$
(blue) and
$\unicode[STIX]{x1D719}=0^{\circ }$
(blue) and
![]() $\unicode[STIX]{x1D719}=270^{\circ }$
(orange). The coefficients are
$\unicode[STIX]{x1D719}=270^{\circ }$
(orange). The coefficients are
![]() $c_{1}=4.65$
,
$c_{1}=4.65$
,
![]() $c_{4}=0.49$
,
$c_{4}=0.49$
,
![]() $a_{2}=62.51$
. Adapted with permission from Floryan et al. (Reference Floryan, Van Buren and Smits2018).
$a_{2}=62.51$
. Adapted with permission from Floryan et al. (Reference Floryan, Van Buren and Smits2018).
We see immediately that to achieve high efficiency, the dimensionless amplitude
![]() $A^{\ast }$
should be large. This observation is consistent with the argument that large-amplitude motions are more efficient than small-amplitude motions (Alexander Reference Alexander2003). However, there are two potential limiting factors. First, as
$A^{\ast }$
should be large. This observation is consistent with the argument that large-amplitude motions are more efficient than small-amplitude motions (Alexander Reference Alexander2003). However, there are two potential limiting factors. First, as
![]() $A^{\ast }$
becomes larger the instantaneous angle of attack increases, so that leading edge vortex formation and dynamic stall effects may become important and the drag model given here would be invalidated. Second, animal morphology naturally sets a limit as to how large they can make
$A^{\ast }$
becomes larger the instantaneous angle of attack increases, so that leading edge vortex formation and dynamic stall effects may become important and the drag model given here would be invalidated. Second, animal morphology naturally sets a limit as to how large they can make
![]() $A^{\ast }$
. For efficient cruising, therefore,
$A^{\ast }$
. For efficient cruising, therefore,
![]() $A^{\ast }$
should be as large as an animal’s morphology allows, while avoiding dynamic stall at all times.
$A^{\ast }$
should be as large as an animal’s morphology allows, while avoiding dynamic stall at all times.
What about the optimal Strouhal number? Equation (3.18) gives negative efficiencies at low
![]() $St$
, a rapid increase with
$St$
, a rapid increase with
![]() $St$
to achieve a positive peak value at
$St$
to achieve a positive peak value at
![]() $St=\sqrt{3c_{4}\unicode[STIX]{x1D703}_{0}}$
, and a subsequent slow decrease with further increases in
$St=\sqrt{3c_{4}\unicode[STIX]{x1D703}_{0}}$
, and a subsequent slow decrease with further increases in
![]() $St$
as the influence of drag becomes weaker. The comparison between the form given by (3.18) and the data for a heaving and pitching airfoil makes this clear, as displayed in figure 23. The offset drag is critical in determining the low
$St$
as the influence of drag becomes weaker. The comparison between the form given by (3.18) and the data for a heaving and pitching airfoil makes this clear, as displayed in figure 23. The offset drag is critical in determining the low
![]() $St$
behaviour and in setting the particular
$St$
behaviour and in setting the particular
![]() $St$
at which the peak efficiency occurs. In addition, the maximum value of the efficiency is directly related to the value of the drag constant
$St$
at which the peak efficiency occurs. In addition, the maximum value of the efficiency is directly related to the value of the drag constant
![]() $c_{4}$
, which further emphasizes the critical role of the drag term in determining the efficiency behaviour.
$c_{4}$
, which further emphasizes the critical role of the drag term in determining the efficiency behaviour.

Figure 23. Efficiency
![]() $\unicode[STIX]{x1D702}$
as a function of
$\unicode[STIX]{x1D702}$
as a function of
![]() $St$
. Data are as given for a heaving and pitching NACA0012 foil (Quinn et al.
Reference Quinn, Lauder and Smits2015). Solid lines are given by (3.18) with a fixed proportionality constant of 0.155. The drag constant,
$St$
. Data are as given for a heaving and pitching NACA0012 foil (Quinn et al.
Reference Quinn, Lauder and Smits2015). Solid lines are given by (3.18) with a fixed proportionality constant of 0.155. The drag constant,
![]() $c_{4}$
, is set to 0.5, 0.35, 0.23, 0.15, 0.1 and 0.05 as the colours vary from dark to light. Reproduction with permission from Floryan et al. (Reference Floryan, Van Buren and Smits2018).
$c_{4}$
, is set to 0.5, 0.35, 0.23, 0.15, 0.1 and 0.05 as the colours vary from dark to light. Reproduction with permission from Floryan et al. (Reference Floryan, Van Buren and Smits2018).
We expect that the relative importance of the drag, captured by
![]() $c_{4}$
, will depend on the Reynolds number. Our drag model is similar to that for a bluff body, such as a sphere or cylinder, so we expect
$c_{4}$
, will depend on the Reynolds number. Our drag model is similar to that for a bluff body, such as a sphere or cylinder, so we expect
![]() $c_{4}$
will be large at small Reynolds numbers, and decrease as the Reynolds number increases until it reaches approximately 1000, above which the drag will be almost constant (at least for
$c_{4}$
will be large at small Reynolds numbers, and decrease as the Reynolds number increases until it reaches approximately 1000, above which the drag will be almost constant (at least for
![]() $Re<2\times 10^{5}$
, although biological measurements imply that the drag may remain constant up to
$Re<2\times 10^{5}$
, although biological measurements imply that the drag may remain constant up to
![]() $Re=10^{8}$
) (Gazzola et al.
Reference Gazzola, Argentina and Mahadevan2014). Our conclusion is consistent with biological measurements (at least for swimmers), where the preferred Strouhal number appears to decrease as the Reynolds number increases, until it reaches an asymptotic value (Gazzola et al.
Reference Gazzola, Argentina and Mahadevan2014). The drag on the propulsor is the crucial factor in creating an efficiency peak which dictates the cruising conditions of swimming animals. In other words, energetic considerations set the kinematics of the propulsor to the most efficient one, and the net thrust of the propulsor at peak efficiency balances the drag of the body to set the cruising speed.
$Re=10^{8}$
) (Gazzola et al.
Reference Gazzola, Argentina and Mahadevan2014). Our conclusion is consistent with biological measurements (at least for swimmers), where the preferred Strouhal number appears to decrease as the Reynolds number increases, until it reaches an asymptotic value (Gazzola et al.
Reference Gazzola, Argentina and Mahadevan2014). The drag on the propulsor is the crucial factor in creating an efficiency peak which dictates the cruising conditions of swimming animals. In other words, energetic considerations set the kinematics of the propulsor to the most efficient one, and the net thrust of the propulsor at peak efficiency balances the drag of the body to set the cruising speed.
As noted earlier, the drag behaviour will depend on the profile shape of the propulsor as well as the Reynolds number, and it is likely that there exists an optimum profile for a given Reynolds number and set of operating conditions. Van Buren et al. (Reference Van Buren, Floryan, Smits, Pan, Bode-Oke and Dong2019b
) used variety of techniques to address this optimization problem, and figure 24 shows the path of optimization from the starting foil, NACA0012, to the optimized foil, on a map of the efficiency and thrust coefficient for two reduced frequencies,
![]() $k=0.4$
and 1.0. The pitch and heave amplitudes were chosen to match experiments, with
$k=0.4$
and 1.0. The pitch and heave amplitudes were chosen to match experiments, with
![]() $c=80$
mm,
$c=80$
mm,
![]() $h_{0}=30$
mm and
$h_{0}=30$
mm and
![]() $\unicode[STIX]{x1D703}_{0}=15^{\circ }$
. Although the optimization was aimed only at improving efficiency, the optimization improves both the efficiency and thrust by approximately 30 % and 15 %, respectively, at
$\unicode[STIX]{x1D703}_{0}=15^{\circ }$
. Although the optimization was aimed only at improving efficiency, the optimization improves both the efficiency and thrust by approximately 30 % and 15 %, respectively, at
![]() $k=1$
. The optimized foil performance exceeds other common foil shapes as well, including the EPPLER 836 and a dolphin fluke profile. Figure 25 shows the efficiency as it varies with Reynolds number. At
$k=1$
. The optimized foil performance exceeds other common foil shapes as well, including the EPPLER 836 and a dolphin fluke profile. Figure 25 shows the efficiency as it varies with Reynolds number. At
![]() $k=1$
the foil is in the regime of high thrust but far from having peak efficiency, and the impact of Reynolds number is small. However, at
$k=1$
the foil is in the regime of high thrust but far from having peak efficiency, and the impact of Reynolds number is small. However, at
![]() $k=0.4$
the foil is near peak efficiency and the Reynolds number becomes much more important, and the benefits of having an optimized foil increase with Reynolds number. Hence, the foil kinematics and shape are tightly intertwined and must be simultaneously taken into consideration to truly maximize performance.
$k=0.4$
the foil is near peak efficiency and the Reynolds number becomes much more important, and the benefits of having an optimized foil increase with Reynolds number. Hence, the foil kinematics and shape are tightly intertwined and must be simultaneously taken into consideration to truly maximize performance.

Figure 24. Efficiency and thrust for optimized two-dimensional foil, as they develop during optimization, relative to the NACA0012 reference foil. (a)
![]() $k=0.4$
; (b)
$k=0.4$
; (b)
![]() $k=1.0$
. Other common foil shapes are also shown. Reproduction with permission from Van Buren et al. (Reference Van Buren, Floryan, Smits, Pan, Bode-Oke and Dong2019b
).
$k=1.0$
. Other common foil shapes are also shown. Reproduction with permission from Van Buren et al. (Reference Van Buren, Floryan, Smits, Pan, Bode-Oke and Dong2019b
).

Figure 25. Reynolds number dependence of efficiency for optimized two-dimensional foil, compared to the NACA0012 reference foil. (a)
![]() $k=0.4$
; (b)
$k=0.4$
; (b)
![]() $k=1.0$
. Reproduction with permission from Van Buren et al. (Reference Van Buren, Floryan, Smits, Pan, Bode-Oke and Dong2019b
).
$k=1.0$
. Reproduction with permission from Van Buren et al. (Reference Van Buren, Floryan, Smits, Pan, Bode-Oke and Dong2019b
).
There remains the question of setting an operating point where reasonable efficiency and thrust can be achieved at the same time. To illustrate this challenge, we use the data obtained by Van Buren et al. (Reference Van Buren, Floryan and Smits2018a
) and plot the efficiency versus the thrust coefficient for the cases where
![]() $210^{\circ }\leqslant \unicode[STIX]{x1D719}\leqslant 330^{\circ }$
, as in figure 26. The data appear to display a Pareto front, where the maximum instantaneous angle of attack is less than about
$210^{\circ }\leqslant \unicode[STIX]{x1D719}\leqslant 330^{\circ }$
, as in figure 26. The data appear to display a Pareto front, where the maximum instantaneous angle of attack is less than about
![]() $30^{\circ }$
. This limit of the angle of attack suggests that leading edge vortex formation and dynamic stall effects need to be avoided to achieve the best performance (Tuncer & Kaya Reference Tuncer and Kaya2005; Young et al.
Reference Young, Lai, Kaya and Tuncer2006; Young & Lai Reference Young and Lai2007). The presence of this front indicates that for rigid propulsors, even at optimum conditions, high efficiency motions occur at low thrust, and increasing the thrust reduces the efficiency.
$30^{\circ }$
. This limit of the angle of attack suggests that leading edge vortex formation and dynamic stall effects need to be avoided to achieve the best performance (Tuncer & Kaya Reference Tuncer and Kaya2005; Young et al.
Reference Young, Lai, Kaya and Tuncer2006; Young & Lai Reference Young and Lai2007). The presence of this front indicates that for rigid propulsors, even at optimum conditions, high efficiency motions occur at low thrust, and increasing the thrust reduces the efficiency.

Figure 26. Combined heave and pitch motions of a rigid foil. Data points are coloured by maximum angle of attack. The Pareto front is broadly defined by
![]() $\unicode[STIX]{x1D6FC}_{m}$
between
$\unicode[STIX]{x1D6FC}_{m}$
between
![]() $20^{\circ }$
and
$20^{\circ }$
and
![]() $30^{\circ }$
. Data obtained by Van Buren et al. (Reference Van Buren, Floryan and Smits2018a
).
$30^{\circ }$
. Data obtained by Van Buren et al. (Reference Van Buren, Floryan and Smits2018a
).
3.5 Flexibility in oscillatory motions
In § 2.2 we examined the performance of flexible panels in undulatory motion, mainly by reference to the results obtained by Quinn et al. (Reference Quinn, Lauder and Smits2014, Reference Quinn, Lauder and Smits2015). We now consider flexible panels in oscillatory motion by considering the work by Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013) on flexible pitching panels. The flexibility was passive, in the sense that the foils were actuated in pitch at the leading edge, and the resulting foil shape was set by the interaction between the panel and the fluid flow. This passive flexibility might be a reasonable model for the flukes of mammals, where muscles appear to be absent. Seven panels with different flexibilities were considered, including one rigid panel (
![]() $P_{1}$
–
$P_{1}$
–
![]() $P_{\infty }$
in table 1). The flexibility of the panel was defined by the effective stiffness of the panel
$P_{\infty }$
in table 1). The flexibility of the panel was defined by the effective stiffness of the panel
![]() $\unicode[STIX]{x1D6F1}_{1}^{\prime }$
, where
$\unicode[STIX]{x1D6F1}_{1}^{\prime }$
, where
This definition of the effective stiffness differs from the bending stiffness coefficient of an isotropic plate
![]() $E\unicode[STIX]{x1D6FF}^{3}/[12(1-\unicode[STIX]{x1D708}_{p}^{2})]$
used by Shyy et al. (Reference Shyy, Aono, Chimakurthi, Trizila, Kang, Cesnik and Liu2010). See also Alben (Reference Alben2008), where
$E\unicode[STIX]{x1D6FF}^{3}/[12(1-\unicode[STIX]{x1D708}_{p}^{2})]$
used by Shyy et al. (Reference Shyy, Aono, Chimakurthi, Trizila, Kang, Cesnik and Liu2010). See also Alben (Reference Alben2008), where
![]() $R_{2}=\unicode[STIX]{x1D6F1}_{1}^{\prime }$
. In addition,
$R_{2}=\unicode[STIX]{x1D6F1}_{1}^{\prime }$
. In addition,
![]() $\unicode[STIX]{x1D6F1}_{1}^{\prime }=k_{1}^{2}/\unicode[STIX]{x1D6F1}_{1}^{2}$
, where
$\unicode[STIX]{x1D6F1}_{1}^{\prime }=k_{1}^{2}/\unicode[STIX]{x1D6F1}_{1}^{2}$
, where
![]() $\unicode[STIX]{x1D6F1}_{1}$
is the effective flexibility used by Quinn et al. (Reference Quinn, Lauder and Smits2014) (see § 2.2). It is difficult to assign a corresponding value of the effective stiffness for fish, but it is likely in the range
$\unicode[STIX]{x1D6F1}_{1}$
is the effective flexibility used by Quinn et al. (Reference Quinn, Lauder and Smits2014) (see § 2.2). It is difficult to assign a corresponding value of the effective stiffness for fish, but it is likely in the range
![]() $1.4<\unicode[STIX]{x1D6F1}_{1}^{\prime }<4$
(panels
$1.4<\unicode[STIX]{x1D6F1}_{1}^{\prime }<4$
(panels
![]() $P_{3}$
–
$P_{3}$
–
![]() $P_{5}$
).
$P_{5}$
).
Figure 27 shows the thrust coefficients as a function of reduced frequency. For the flexible panels, the thrust coefficient initially increases with
![]() $k$
until a maximum is reached after which it declines slightly with a further increase in
$k$
until a maximum is reached after which it declines slightly with a further increase in
![]() $k$
. The peaks occur at a frequency that is approximately 50 % higher than the structural resonance frequency
$k$
. The peaks occur at a frequency that is approximately 50 % higher than the structural resonance frequency
![]() $f_{1}$
for each panel, as measured when the panel is immersed in water (for Panels
$f_{1}$
for each panel, as measured when the panel is immersed in water (for Panels
![]() $P_{1}$
and
$P_{1}$
and
![]() $P_{2}$
the resonant frequency of the first beam bending mode is lower than the frequencies examined here). Each of the flexible panels generates a higher thrust coefficient than its rigid counterpart over a certain frequency range. For example, at
$P_{2}$
the resonant frequency of the first beam bending mode is lower than the frequencies examined here). Each of the flexible panels generates a higher thrust coefficient than its rigid counterpart over a certain frequency range. For example, at
![]() $k=10$
, the results in figure 27(a) indicate that panels
$k=10$
, the results in figure 27(a) indicate that panels
![]() $P_{5}$
and
$P_{5}$
and
![]() $P_{6}$
yield thrust coefficients that are twice as large as the rigid panel (
$P_{6}$
yield thrust coefficients that are twice as large as the rigid panel (
![]() $P_{\infty }$
). The coefficient of power
$P_{\infty }$
). The coefficient of power
![]() $C_{P}$
(figure 27
b) demonstrates qualitatively similar trends.
$C_{P}$
(figure 27
b) demonstrates qualitatively similar trends.
Figure 27(c) indicates that the moderately flexible panels (
![]() $P_{3}$
and
$P_{3}$
and
![]() $P_{4}$
) yield the highest propulsive efficiencies, and that there is an optimal stiffness for maximum efficiency. The global maximum efficiency for the rigid panel reaches only 16 % at
$P_{4}$
) yield the highest propulsive efficiencies, and that there is an optimal stiffness for maximum efficiency. The global maximum efficiency for the rigid panel reaches only 16 % at
![]() $k=6.3$
, but for the flexible panels it reaches about 38 % (panel
$k=6.3$
, but for the flexible panels it reaches about 38 % (panel
![]() $P_{3}$
) at the same reduced frequency. In addition, the flexible panels exhibit high efficiency across a wide range of frequencies whereas the rigid panels display sharper peaks. As the frequency is increased, therefore, the performance benefit of the flexible panels over the rigid panels becomes even more pronounced. This trend may be contrasted with the efficiency curve of a typical propeller, which shows a relatively slow rise with the advance ratio up to its peak value followed by a sharp decline.
$P_{3}$
) at the same reduced frequency. In addition, the flexible panels exhibit high efficiency across a wide range of frequencies whereas the rigid panels display sharper peaks. As the frequency is increased, therefore, the performance benefit of the flexible panels over the rigid panels becomes even more pronounced. This trend may be contrasted with the efficiency curve of a typical propeller, which shows a relatively slow rise with the advance ratio up to its peak value followed by a sharp decline.

Figure 27. Coefficients of thrust, power and efficiency as a function of reduced frequency for nominally two-dimensional pitching panels. Panel properties given in table 1. Adapted with permission from Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013).
Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013) concluded that the global maximum in propulsive efficiency across a range of panel flexibilities is achieved when two conditions are simultaneously satisfied: (i) the oscillation of the panel yields a Strouhal number in the range
![]() $0.25<St<0.35$
; and (ii) the frequency of motion is tuned to the structural resonant frequency of the panel.
$0.25<St<0.35$
; and (ii) the frequency of motion is tuned to the structural resonant frequency of the panel.
These conclusions were made with respect to panels in pitch only. To demonstrate the effects of flexibility on the performance of panels in combined pitch and heave motions, consider the results shown in figure 28. These panels had effective stiffnesses similar to those used by Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013) – see table 1. For certain flexibilities, there is a clear envelope where the thrust production is up to 1.5 times higher than the thrust of a rigid panel. Here, the peak efficiency is less influenced by flexibility than thrust, but the efficiency tends to remain higher over a larger Strouhal number range, which also means higher thrust levels. Thus, those animals that can control their fin stiffness using muscle action may be able to maintain high efficiency at higher cruising speeds by actively changing the stiffness.

Figure 28. Effects of flexibility on time-averaged (a) thrust and (b) efficiency for a pitching and heaving panels separated by phase
![]() $\unicode[STIX]{x1D719}=270^{\circ }$
. Pitch amplitudes
$\unicode[STIX]{x1D719}=270^{\circ }$
. Pitch amplitudes
![]() $\unicode[STIX]{x1D703}=\{6^{\circ },9^{\circ },\ldots ,15^{\circ }\}$
; heave amplitudes
$\unicode[STIX]{x1D703}=\{6^{\circ },9^{\circ },\ldots ,15^{\circ }\}$
; heave amplitudes
![]() $h_{0}/c=\{0.083,0.167,\ldots ,0.33\}$
; and frequencies
$h_{0}/c=\{0.083,0.167,\ldots ,0.33\}$
; and frequencies
![]() $f=\{0.2,0.25,\ldots ,1~\text{Hz}\}$
. The rigid panel corresponds to
$f=\{0.2,0.25,\ldots ,1~\text{Hz}\}$
. The rigid panel corresponds to
![]() $\unicode[STIX]{x1D6F1}_{1}^{\prime }\sim \infty$
. See table 1. Adapted with permission from Van Buren et al. (Reference Van Buren, Floryan, Smits, Daniel and Soboyejo2019a
).
$\unicode[STIX]{x1D6F1}_{1}^{\prime }\sim \infty$
. See table 1. Adapted with permission from Van Buren et al. (Reference Van Buren, Floryan, Smits, Daniel and Soboyejo2019a
).
3.6 Effects of aspect ratio
So far, we have analysed rectangular foils or panels in oscillatory motion that were effectively two-dimensional, as in Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a ) and Van Buren et al. (Reference Van Buren, Floryan and Smits2018a ), or the panels had a fixed aspect ratio, as in Quinn et al. (Reference Quinn, Lauder and Smits2014). The effects of aspect ratio have not yet been considered, but it is obviously an important consideration given the range of caudal fin and fluke shapes seen in nature. These effects were explored systematically for rectangular panels by Buchholz & Smits (Reference Buchholz and Smits2008), Green & Smits (Reference Green and Smits2008), Dai et al. (Reference Dai, Luo, de Sousa and Doyle2012), Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013) and, more recently, Ayancik et al. (Reference Ayancik, Zhong, Quinn, Brandes, Bart-Smith and Moored2018). Chopra (Reference Chopra1974) extended Lighthill’s (Reference Lighthill1970) and Wu’s (Reference Wu and Yih1971a ) inviscid flow theory for a thin plate to the three-dimensional case of finite aspect ratio, for combined pitch and heave motion. He concluded that reducing the aspect ratio would lead to lower thrust and efficiency.
Buchholz & Smits (Reference Buchholz and Smits2008) studied rectangular rigid panels in pitch with ![]() , 0.83. 1.11 and 2.38. For the highest aspect ratio, the panel spanned the working section, and so was taken to represent the two-dimensional case
, 0.83. 1.11 and 2.38. For the highest aspect ratio, the panel spanned the working section, and so was taken to represent the two-dimensional case ![]() . At a given Strouhal number, the thrust coefficient (defined on the basis of panel area
. At a given Strouhal number, the thrust coefficient (defined on the basis of panel area
![]() $sc$
, as usual) decreased with increasing aspect ratio, but the effects on efficiency were generally rather minor. Green & Smits (Reference Green and Smits2008) analysed the same data, and showed that the thrust results could be collapsed by the scaling
$sc$
, as usual) decreased with increasing aspect ratio, but the effects on efficiency were generally rather minor. Green & Smits (Reference Green and Smits2008) analysed the same data, and showed that the thrust results could be collapsed by the scaling
![]() $C_{T}^{\ast }=C_{T}(1+c^{\prime }a/s)$
where
$C_{T}^{\ast }=C_{T}(1+c^{\prime }a/s)$
where
![]() $c^{\prime }$
is an empirical constant, and noted that this scaling is similar to the that used to account for the effects of aspect ratio on the lift coefficient in finite wing theory. This last observation is somewhat misleading in that the thrust produced by a pitching panel is primarily due to added mass forces, not circulatory forces, but as we shall see the added mass scaling with aspect ratio follows a somewhat similar variation.
$c^{\prime }$
is an empirical constant, and noted that this scaling is similar to the that used to account for the effects of aspect ratio on the lift coefficient in finite wing theory. This last observation is somewhat misleading in that the thrust produced by a pitching panel is primarily due to added mass forces, not circulatory forces, but as we shall see the added mass scaling with aspect ratio follows a somewhat similar variation.
Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013) considered rectangular flexible panels in pitch with ![]() , 1.0, 1.5 and 2.0, and found that flexible panels did not exhibit a monotonic trend with aspect ratio (see figure 29
a). For panel
, 1.0, 1.5 and 2.0, and found that flexible panels did not exhibit a monotonic trend with aspect ratio (see figure 29
a). For panel
![]() $P_{4}$
, for example, the coefficient of thrust increases with aspect ratio at lower frequencies, while it decreases with aspect ratio at higher frequencies. The results for
$P_{4}$
, for example, the coefficient of thrust increases with aspect ratio at lower frequencies, while it decreases with aspect ratio at higher frequencies. The results for
![]() $C_{P}$
demonstrate qualitatively similar trends as those of
$C_{P}$
demonstrate qualitatively similar trends as those of
![]() $C_{T}$
(figure 29
b). Whereas for the rigid panels the coefficient of power monotonically increases with reduced frequency, for the flexible panels it initially increases with reduced frequency until a maximum is reached at which point the coefficient of power tends to level off. The peak in
$C_{T}$
(figure 29
b). Whereas for the rigid panels the coefficient of power monotonically increases with reduced frequency, for the flexible panels it initially increases with reduced frequency until a maximum is reached at which point the coefficient of power tends to level off. The peak in
![]() $C_{P}$
occurs at a frequency that is approximately 50 % higher than the structural resonance frequency, which is the same point where the thrust reaches its maximum. In addition, for each flexibility examined, the efficiency increases with aspect ratio as observed by Buchholz & Smits (Reference Buchholz and Smits2008) for rigid panels (figure 29
c), although this effect is rather small compared to the effects of flexibility, where there is a clear optimal stiffness to achieve maximum efficiency.
$C_{P}$
occurs at a frequency that is approximately 50 % higher than the structural resonance frequency, which is the same point where the thrust reaches its maximum. In addition, for each flexibility examined, the efficiency increases with aspect ratio as observed by Buchholz & Smits (Reference Buchholz and Smits2008) for rigid panels (figure 29
c), although this effect is rather small compared to the effects of flexibility, where there is a clear optimal stiffness to achieve maximum efficiency.

Figure 29. Coefficients of thrust, power and efficiency as a function of reduced frequency for finite-aspect-ratio pitching panels. Panel properties given in table 1. Adapted with permission from Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013).
For all the flexible panels considered by Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013), only the first mode was excited, so that
![]() $f_{1}$
was the single resonant frequency of interest. In such cases, the elastic forces in bending become important, so that the dynamics will be governed by the balance between inertial, fluid forces and elastic forces acting on the panel. The inertial force
$f_{1}$
was the single resonant frequency of interest. In such cases, the elastic forces in bending become important, so that the dynamics will be governed by the balance between inertial, fluid forces and elastic forces acting on the panel. The inertial force
![]() $F_{i}$
is the mass of the panel times its characteristic acceleration, so that
$F_{i}$
is the mass of the panel times its characteristic acceleration, so that
![]() $F_{i}\sim \unicode[STIX]{x1D70C}_{p}sc\unicode[STIX]{x1D6FF}(f^{2}c)$
, and for thin panels in water this is expected to be small compared to the other forces. The fluid force
$F_{i}\sim \unicode[STIX]{x1D70C}_{p}sc\unicode[STIX]{x1D6FF}(f^{2}c)$
, and for thin panels in water this is expected to be small compared to the other forces. The fluid force
![]() $F_{v}$
is taken to be due to added mass, so that
$F_{v}$
is taken to be due to added mass, so that
![]() $F_{v}\sim \unicode[STIX]{x1D70C}s^{2}c(f^{2}c)$
. Here, Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013), chose to use
$F_{v}\sim \unicode[STIX]{x1D70C}s^{2}c(f^{2}c)$
. Here, Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013), chose to use
![]() $\unicode[STIX]{x1D70C}s^{2}c$
for the added mass, which is the form appropriate to panels with small aspect ratios, even though the aspect ratios in their experiments varied from 0.5 to effectively infinite. The elastic forces depend on the flexural stiffness of the panel (
$\unicode[STIX]{x1D70C}s^{2}c$
for the added mass, which is the form appropriate to panels with small aspect ratios, even though the aspect ratios in their experiments varied from 0.5 to effectively infinite. The elastic forces depend on the flexural stiffness of the panel (
![]() $EI\sim Es\unicode[STIX]{x1D6FF}^{3}$
), and so the characteristic elastic force is
$EI\sim Es\unicode[STIX]{x1D6FF}^{3}$
), and so the characteristic elastic force is
![]() $F_{e}=Es\unicode[STIX]{x1D6FF}^{3}/c^{2}$
.
$F_{e}=Es\unicode[STIX]{x1D6FF}^{3}/c^{2}$
.
When flexibility is the dominant effect, we expect that the thrust will scale with
![]() $F_{e}$
, and the characteristic power expended in bending is then expressed by the product of
$F_{e}$
, and the characteristic power expended in bending is then expressed by the product of
![]() $F_{e}$
and a characteristic velocity. Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013) chose
$F_{e}$
and a characteristic velocity. Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013) chose
![]() $fc$
for this velocity scale, whereas a more natural choice might be
$fc$
for this velocity scale, whereas a more natural choice might be
![]() $fa$
, since that represents the characteristic velocity of the trailing edge. However, the pitch amplitude at the leading edge was fixed (
$fa$
, since that represents the characteristic velocity of the trailing edge. However, the pitch amplitude at the leading edge was fixed (
![]() $\unicode[STIX]{x1D703}_{0}=7.2^{\circ }$
), and if we ignore Panels
$\unicode[STIX]{x1D703}_{0}=7.2^{\circ }$
), and if we ignore Panels
![]() $P_{1}$
and
$P_{1}$
and
![]() $P_{2}$
, the amplitude of the trailing edge motion compared to its value for the rigid panel was only approximately 20 %–30 % larger for the infinite-aspect-ratio cases, and 25 %–40 % larger for the finite-aspect-ratio cases. Hence the difference between choosing
$P_{2}$
, the amplitude of the trailing edge motion compared to its value for the rigid panel was only approximately 20 %–30 % larger for the infinite-aspect-ratio cases, and 25 %–40 % larger for the finite-aspect-ratio cases. Hence the difference between choosing
![]() $fc$
and
$fc$
and
![]() $fa$
is generally rather small for these experiments. If we accept this scaling, the non-dimensional thrust production and power input for flexible panels are then given by
$fa$
is generally rather small for these experiments. If we accept this scaling, the non-dimensional thrust production and power input for flexible panels are then given by
This suggests a new efficiency parameter given by
![]() $\widetilde{\unicode[STIX]{x1D702}}=T(fc)/P=k\unicode[STIX]{x1D702}$
. The thrust, power and efficiency data in this new scaling are shown in figure 30, where
$\widetilde{\unicode[STIX]{x1D702}}=T(fc)/P=k\unicode[STIX]{x1D702}$
. The thrust, power and efficiency data in this new scaling are shown in figure 30, where
![]() $f_{1}^{\ast }=f/f_{1}$
. This scaling collapses the data remarkably well across all aspect ratios examined. The effects of aspect ratio for these flexible panels therefore seem to be well accounted for by the elastic response of the panel.
$f_{1}^{\ast }=f/f_{1}$
. This scaling collapses the data remarkably well across all aspect ratios examined. The effects of aspect ratio for these flexible panels therefore seem to be well accounted for by the elastic response of the panel.

Figure 30. Flexible pitching panels with ![]() . (a) Scaled thrust behaviour; (b) scaled power behaviour; and (c) scaled efficiency behaviour. Symbols are the same used in figure 27, but only the flexible panel data for panels
. (a) Scaled thrust behaviour; (b) scaled power behaviour; and (c) scaled efficiency behaviour. Symbols are the same used in figure 27, but only the flexible panel data for panels
![]() $P_{3}$
to
$P_{3}$
to
![]() $P_{6}$
are presented here. Reproduction with permission from Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013).
$P_{6}$
are presented here. Reproduction with permission from Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013).
For the rigid panels examined by Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013) we expect that the data should scale with the characteristic fluid force
![]() $F_{v}$
. That is, we can define new thrust and power coefficients according to
$F_{v}$
. That is, we can define new thrust and power coefficients according to
and, as before,
![]() $\widetilde{\unicode[STIX]{x1D702}}=T(fc)/P=k\unicode[STIX]{x1D702}$
. The collapse of the data shown in figure 31 is quite remarkable, particularly in that it is independent of aspect ratio.
$\widetilde{\unicode[STIX]{x1D702}}=T(fc)/P=k\unicode[STIX]{x1D702}$
. The collapse of the data shown in figure 31 is quite remarkable, particularly in that it is independent of aspect ratio.
To see why, remember that the characteristic fluid force
![]() $F_{v}$
is due to added mass. For small-aspect-ratio panels we would expect the added mass to vary as
$F_{v}$
is due to added mass. For small-aspect-ratio panels we would expect the added mass to vary as
![]() $\unicode[STIX]{x1D70C}s^{2}c$
, that is, the circumscribing circle that is at the basis of added mass models is defined by the span. This is also used in slender body theory where the circumscribing circle is defined by the cross-sectional area of the body. However, for large-aspect-ratio panels we would expect the added mass term to look like
$\unicode[STIX]{x1D70C}s^{2}c$
, that is, the circumscribing circle that is at the basis of added mass models is defined by the span. This is also used in slender body theory where the circumscribing circle is defined by the cross-sectional area of the body. However, for large-aspect-ratio panels we would expect the added mass term to look like
![]() $\unicode[STIX]{x1D70C}sc^{2}$
, that is, the circumscribing circle is defined by the chord. The force
$\unicode[STIX]{x1D70C}sc^{2}$
, that is, the circumscribing circle is defined by the chord. The force
![]() $F_{v}$
was defined using
$F_{v}$
was defined using
![]() $\unicode[STIX]{x1D70C}s^{2}c$
for the added mass term, but given the range of aspect ratios covered in the experiments, the added mass term should depend on aspect ratio, something like
$\unicode[STIX]{x1D70C}s^{2}c$
for the added mass term, but given the range of aspect ratios covered in the experiments, the added mass term should depend on aspect ratio, something like ![]() , where the function
, where the function ![]() for
for ![]() and
and
![]() $g=1$
for
$g=1$
for ![]() . A suitable form would be
. A suitable form would be ![]() , where the exponent
, where the exponent
![]() $n$
needs to be determined. The form with
$n$
needs to be determined. The form with
![]() $n=1$
was proposed by Brennen (Reference Brennen1982), but the comparison with the data given in figure 32 is not encouraging. Rather unexpectedly, the data suggest that the variation with aspect ratio is not smooth and that they seem better fitted by a discontinuous function
$n=1$
was proposed by Brennen (Reference Brennen1982), but the comparison with the data given in figure 32 is not encouraging. Rather unexpectedly, the data suggest that the variation with aspect ratio is not smooth and that they seem better fitted by a discontinuous function ![]() for
for ![]() , and
, and
![]() $g=1$
for
$g=1$
for ![]() . That is, the preferred added mass representation seems to be
. That is, the preferred added mass representation seems to be
![]() $m=\unicode[STIX]{x1D70C}s^{2}c$
for
$m=\unicode[STIX]{x1D70C}s^{2}c$
for ![]() , and
, and
![]() $\unicode[STIX]{x1D70C}sc^{2}$
for
$\unicode[STIX]{x1D70C}sc^{2}$
for ![]() . Therefore Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013)’s choice for the added mass term appears to embody precisely the correct scaling for the effects of aspect ratio, for the range of values considered in their experiments.
. Therefore Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013)’s choice for the added mass term appears to embody precisely the correct scaling for the effects of aspect ratio, for the range of values considered in their experiments.

Figure 31. Rigid pitching panels (
![]() $P_{5}$
) with
$P_{5}$
) with ![]() . (a) Scaled thrust behaviour; (b) scaled power behaviour; and (c) scaled efficiency behaviour. Symbols are the same used in figure 27. Adapted with permission from Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013).
. (a) Scaled thrust behaviour; (b) scaled power behaviour; and (c) scaled efficiency behaviour. Symbols are the same used in figure 27. Adapted with permission from Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013).

Figure 32. Added mass variation with aspect ratio, ![]() . Dotted line is the discontinuous function proposed here. Chain-dotted line is from Brennen (Reference Brennen1982), apparently a smoothed curve fitted to the experimental data from Patton (Reference Patton1965), as reproduced here.
. Dotted line is the discontinuous function proposed here. Chain-dotted line is from Brennen (Reference Brennen1982), apparently a smoothed curve fitted to the experimental data from Patton (Reference Patton1965), as reproduced here.
We conclude that the data for the thrust production and power input to the fluid for rigid and flexible panels in pitch collapse using scalings for the characteristic fluid force and the characteristic elastic force, respectively. In particular, the effects of aspect ratio for the flexible panels is contained within the characteristic elastic force, and for the rigid panels it is contained in a judicious choice for the added mass term in the characteristic fluid force. For those panels that have stiffnesses between what we have called ‘rigid’ or ‘flexible’ the scaling will undoubtedly depend on both the elastic force and the fluid force.
So far we have only discussed the effects of aspect ratio on panels in pitch. For panels in heave, the circulatory forces will be important, and so it seems likely that that the circulatory forces should vary with aspect ratio as indicated by finite wing theory, that is, they would reduce by the factor ![]() . Recent results by Ayancik et al. (Reference Ayancik, Zhong, Quinn, Brandes, Bart-Smith and Moored2018) support this expectation, although the results for finite wing theory are probably not reliable for
. Recent results by Ayancik et al. (Reference Ayancik, Zhong, Quinn, Brandes, Bart-Smith and Moored2018) support this expectation, although the results for finite wing theory are probably not reliable for ![]() , and for more complicated planform shapes.
, and for more complicated planform shapes.
3.7 Effects of planform
All the foils considered so far have been rectangular in planform. Caudal fins, of course, exhibit a wide range of shapes, as shown in figure 33 (see also figure 12). The shape of the caudal fin clearly affects the fish flow kinematics, swimming efficiency, acceleration and manoeuvrability (Sambilay Jr Reference Sambilay1990; Lauder Reference Lauder2000; Sumich & Morrissey Reference Sumich and Morrissey2004). To understand the effects on performance, a number of investigations have systematically altered the planform of fin-like propulsors. For example, Webb (Reference Webb1973) employed caudal fin amputation as a tool to examine drag and thrust relations for a swimming fish, using the Lighthill (Reference Lighthill1969) model to analyse the thrust mechanics. Karpouzian, Spedding & Cheng (Reference Karpouzian, Spedding and Cheng1990) analytically compared the performance of fins with varying aspect ratio and sweep, and both parameters were found to have a significant impact on thrust and efficiency. The effects of planform shape as it applies to many fish-like propulsors were studied analytically and numerically for inviscid flow by Chopra & Kambe (Reference Chopra and Kambe1977), who explored the complex relationship between thrust and (inviscid) efficiency when the sweep, taper and aspect ratio were varied. They found that ‘compared with a rectangular tail, a curved leading edge as in lunate tails gives a reduced thrust contribution from the leading edge suction for the same total thrust; however, a sweep angle of the leading edge exceeding about
![]() $30^{\circ }$
leads to a marked reduction of efficiency’. The reduction in leading edge suction would help to reduce the incidence of dynamic stall, as well as the possible formation of a strong leading edge vortex, so it seems likely that a curved leading edge would help push the performance envelope to higher angles of attack. The reduced efficiency at high sweep angles is associated with the reduced thrust developed by such planforms.
$30^{\circ }$
leads to a marked reduction of efficiency’. The reduction in leading edge suction would help to reduce the incidence of dynamic stall, as well as the possible formation of a strong leading edge vortex, so it seems likely that a curved leading edge would help push the performance envelope to higher angles of attack. The reduced efficiency at high sweep angles is associated with the reduced thrust developed by such planforms.
In addition, Li, Luodin & Lu (Reference Li, Luodin and Lu2012) and Liu & Bose (Reference Liu and Bose1993) compared the performance of three whale fins using a quasi-vortex-lattice numerical method. By varying pitch and heave amplitude, it was found that the tail shape significantly altered the conditions for maximum efficiency. Lauder et al. (Reference Lauder, Lim, Shelton, Witt, Anderson and Tangorra2011) isolated the effects of trailing edge shape for highly flexible panels of very low aspect ratio ![]() akin to eel-like swimmers, and found experimentally that the trailing edge could be manipulated to improve the panel self-propelled swimming speed.
akin to eel-like swimmers, and found experimentally that the trailing edge could be manipulated to improve the panel self-propelled swimming speed.

Figure 33. The variety of fish caudal fin types. Reproduction with permission from Van Buren et al. (Reference Van Buren, Floryan, Brunner, Şentürk and Smits2017a ).
Part of the complexity introduced by a change in planform is that it usually changes more than one fin parameter at the same time. For example, changing the sweep alters the angle of the leading and trailing edges simultaneously, and changing the planform of the panel also affects its flexibility. Even for rigid panels the planform can assume many different shapes, so that a multitude of geometric parameters come into play. Three studies that have addressed these issues to some extent are Green, Rowley & Smits (Reference Green, Rowley and Smits2011) and King, Kumar & Green (Reference King, Kumar and Green2018) who studied the behaviour of low aspect ratio trapezoidal panels with square trailing edges (an approximation to the truncate caudal fin shape), and Van Buren et al. (Reference Van Buren, Floryan, Brunner, Şentürk and Smits2017a ) who investigated foils with planforms that varied systematically from a shape that approximated a forked tail to that of a lanceolate tail.
King et al. (Reference King, Kumar and Green2018) focused primarily on the wake structure that develops downstream of the trapezoidal foil with increasing Strouhal number. Isometric views of the phase-averaged three-dimensional isosurfaces of
![]() $Q$
are shown in figure 34, where the
$Q$
are shown in figure 34, where the
![]() $Q$
criterion proposed by Hunt, Wray & Moin (Reference Hunt, Wray and Moin1988) helps to identify the vortex structure. They observed that the spanwise vortices shed from the trailing edge of the panel formed a reverse von Kármán vortex street in at least some sections of the wake at all Strouhal numbers considered. Streamwise vortices were created near the spanwise tips of the panel, connecting with spanwise vortices to create vortex rings, and a connected chain of vortex rings was shown to exist at each Strouhal number, prior to the onset of wake breakdown. The induced velocities of the vortex rings were consistent with the transverse expansion and a spanwise compression of the wake, and increasing the Strouhal number produced a greater amount of transverse wake expansion, together with a greater amount of spanwise wake compression. Such observations are consistent with those of Buchholz & Smits (Reference Buchholz and Smits2006) for a pitching rectangular panel with aspect ratio
$Q$
criterion proposed by Hunt, Wray & Moin (Reference Hunt, Wray and Moin1988) helps to identify the vortex structure. They observed that the spanwise vortices shed from the trailing edge of the panel formed a reverse von Kármán vortex street in at least some sections of the wake at all Strouhal numbers considered. Streamwise vortices were created near the spanwise tips of the panel, connecting with spanwise vortices to create vortex rings, and a connected chain of vortex rings was shown to exist at each Strouhal number, prior to the onset of wake breakdown. The induced velocities of the vortex rings were consistent with the transverse expansion and a spanwise compression of the wake, and increasing the Strouhal number produced a greater amount of transverse wake expansion, together with a greater amount of spanwise wake compression. Such observations are consistent with those of Buchholz & Smits (Reference Buchholz and Smits2006) for a pitching rectangular panel with aspect ratio ![]() (see figure 14), who demonstrated that the wake formation is dominated by the streamwise vorticity generated at the panel edges, driving the wake towards the panel centre, very much like the axis switching phenomena of non-axisymmetric jets (Dhanak & Bernardinis Reference Dhanak and Bernardinis1981).
(see figure 14), who demonstrated that the wake formation is dominated by the streamwise vorticity generated at the panel edges, driving the wake towards the panel centre, very much like the axis switching phenomena of non-axisymmetric jets (Dhanak & Bernardinis Reference Dhanak and Bernardinis1981).

Figure 34. Wake development for pitching trapezoidal foil. Isometric views of
![]() $Q$
isosurfaces at a value of 1 %
$Q$
isosurfaces at a value of 1 %
![]() $Q_{max}$
at
$Q_{max}$
at
![]() $t/T=0$
.
$t/T=0$
.
![]() $Q$
isosurfaces are coloured by the local value of
$Q$
isosurfaces are coloured by the local value of
![]() $\unicode[STIX]{x1D714}_{z}$
. (a–c)
$\unicode[STIX]{x1D714}_{z}$
. (a–c)
![]() $St=0.17$
, 0.37 and 0.56. Adapted with permission from King et al. (Reference King, Kumar and Green2018).
$St=0.17$
, 0.37 and 0.56. Adapted with permission from King et al. (Reference King, Kumar and Green2018).
The experiments by Van Buren et al. (Reference Van Buren, Floryan, Brunner, Şentürk and Smits2017a
) were directed more to understanding the effects of changing the trailing edge shape, which was varied from concave to convex (see figure 35). The aspect ratio was held constant ![]() as was the foil planform area, so that the propulsive performance among the different foils could be compared directly. The wake visualizations displayed in figure 35 show that the angle of the trailing edge bends the trailing edge vortices so that the natural vortex bending and wake compression could be promoted (convex) or delayed (concave). This change in vortex formation had a significant impact on the time-averaged velocity field, with a single jet-like wake structure appearing for the concave panels, and a four jet structure for the convex panels. In terms of performance, the thrust and efficiency generally improved going from concave to convex trailing edges. For instance, the most convex panel produced a thrust that was approximately 15 % higher than the square panel. For concave panels, the peak efficiency was reduced by a maximum of approximately 3 % compared to the square trailing edge case, while for convex panels the peak efficiency was improved by a maximum of approximately 2 %. These were significant differences in that the average efficiency of the square panel was only approximately 10 %.
as was the foil planform area, so that the propulsive performance among the different foils could be compared directly. The wake visualizations displayed in figure 35 show that the angle of the trailing edge bends the trailing edge vortices so that the natural vortex bending and wake compression could be promoted (convex) or delayed (concave). This change in vortex formation had a significant impact on the time-averaged velocity field, with a single jet-like wake structure appearing for the concave panels, and a four jet structure for the convex panels. In terms of performance, the thrust and efficiency generally improved going from concave to convex trailing edges. For instance, the most convex panel produced a thrust that was approximately 15 % higher than the square panel. For concave panels, the peak efficiency was reduced by a maximum of approximately 3 % compared to the square trailing edge case, while for convex panels the peak efficiency was improved by a maximum of approximately 2 %. These were significant differences in that the average efficiency of the square panel was only approximately 10 %.
We take these results to imply that the evolution of the various fish caudal fin shapes may include other purposes than simply improving thrust and efficiency; most fish have concave tails, but the data suggest that this may not generate the optimum performance. It is possible that the diversity in caudal fin trailing edge shape fulfils other evolutionary purposes, or it may be that trailing edge shape works together with another parameter to improve performance, for example, sweep angle. It is also possible that the relatively small benefits due to trailing edge shape seen here might be outweighed by the much larger effects due to flexibility or an adaptation to the motion profile.

Figure 35. Isosurfaces of phase-averaged spanwise vorticity
![]() $\langle \unicode[STIX]{x1D714}_{z}^{\ast }\rangle$
, for
$\langle \unicode[STIX]{x1D714}_{z}^{\ast }\rangle$
, for
![]() $St=0.2$
. Red is positive, blue is negative. Spanwise (i) and panel-normal (ii) views are shown for three cases. (a–c) concave, square and convex. Adapted with permission from Van Buren et al. (Reference Van Buren, Floryan, Brunner, Şentürk and Smits2017a
).
$St=0.2$
. Red is positive, blue is negative. Spanwise (i) and panel-normal (ii) views are shown for three cases. (a–c) concave, square and convex. Adapted with permission from Van Buren et al. (Reference Van Buren, Floryan, Brunner, Şentürk and Smits2017a
).
3.8 Intermittent motions
Many aquatic animals, such as sharks and seals as well as small schooling fish, exhibit an intermittent swimming behaviour, sometimes called burst-and-coast swimming (Videler & Weihs Reference Videler and Weihs1982; Blake Reference Blake1983b ; Fish, Fegely & Xanthopoulos Reference Fish, Fegely and Xanthopoulos1991; Chung Reference Chung2009). Fish practice intermittent swimming while hunting, fleeing a predator or pursuing a mate, and exhibit a wide range of ratios of burst-to-coast times (Kramer & McLaughlin Reference Kramer and McLaughlin2001). Of interest here is the possible energy benefit of intermittent swimming versus continuous swimming.
To help answer this question, Floryan, Van Buren & Smits (Reference Floryan, Van Buren and Smits2017b
) conducted experiments on a two-dimensional tear-drop foil in pitch, and varied the swimming duty cycle
![]() $\unicode[STIX]{x1D6E5}$
from 0.2 to 1, where 1 represents continuous swimming. The results show that the mean thrust and power scale linearly with the duty cycle (figure 36), indicating that individual bursts of activity in intermittent motions are independent of each other (Akoz & Moored Reference Akoz and Moored2018 arrived at a similar result). This conclusion was corroborated by flow visualizations, which show that the main vortical structures in the wake did not change with duty cycle, at least for the range of Strouhal numbers considered. The results also indicate that wake vortex proximity to the propulsor itself does appear to be important. We expect that at higher Strouhal numbers these conclusions may not continue to hold because the interaction among the wake vortices would become more intense.
$\unicode[STIX]{x1D6E5}$
from 0.2 to 1, where 1 represents continuous swimming. The results show that the mean thrust and power scale linearly with the duty cycle (figure 36), indicating that individual bursts of activity in intermittent motions are independent of each other (Akoz & Moored Reference Akoz and Moored2018 arrived at a similar result). This conclusion was corroborated by flow visualizations, which show that the main vortical structures in the wake did not change with duty cycle, at least for the range of Strouhal numbers considered. The results also indicate that wake vortex proximity to the propulsor itself does appear to be important. We expect that at higher Strouhal numbers these conclusions may not continue to hold because the interaction among the wake vortices would become more intense.

Figure 36. (a) Thrust and (b) power coefficients as functions of Strouhal number. (a,b) Conventional scaling. (c,d) Coefficients normalized by duty cycle. Dark to light symbols represent increasing duty cycles, ranging from
![]() $\unicode[STIX]{x1D6E5}=0.2$
to 1 every 0.1. Symbols identify pitch amplitudes of
$\unicode[STIX]{x1D6E5}=0.2$
to 1 every 0.1. Symbols identify pitch amplitudes of
![]() $\unicode[STIX]{x1D703}_{0}=5^{\circ }$
(circle),
$\unicode[STIX]{x1D703}_{0}=5^{\circ }$
(circle),
![]() $10^{\circ }$
(square) and
$10^{\circ }$
(square) and
![]() $15^{\circ }$
(triangle). Adapted with permission from Floryan et al. (Reference Floryan, Van Buren and Smits2017b
).
$15^{\circ }$
(triangle). Adapted with permission from Floryan et al. (Reference Floryan, Van Buren and Smits2017b
).
Of particular interest is the free swimming performance during intermittent swimming, that is, the point where the mean thrust is equal to the mean drag. Does the duty cycle decrease the energy required to travel a certain distance? Floryan et al. (Reference Floryan, Van Buren and Smits2017b
) argue that over a fixed distance, the energy ratio
![]() $\unicode[STIX]{x1D719}=E/E_{0}$
should scale according to
$\unicode[STIX]{x1D719}=E/E_{0}$
should scale according to
![]() $\sqrt{\unicode[STIX]{x1D6E5}}$
, where
$\sqrt{\unicode[STIX]{x1D6E5}}$
, where
![]() $E$
is the energy expended by intermittent motion, and
$E$
is the energy expended by intermittent motion, and
![]() $E_{0}$
is the energy expended by continuous motion with the same actuation. Their data generally follow this trend, and so for this simple case it would appear that intermittent motions are always energetically favourable. However, apart from energy spent on swimming, aquatic animals also expend energy on metabolic processes,
$E_{0}$
is the energy expended by continuous motion with the same actuation. Their data generally follow this trend, and so for this simple case it would appear that intermittent motions are always energetically favourable. However, apart from energy spent on swimming, aquatic animals also expend energy on metabolic processes,
![]() $E_{m}$
. When the mean power spent on metabolic processes is assumed the same for continuous and intermittent motion, and that it remains a constant fraction
$E_{m}$
. When the mean power spent on metabolic processes is assumed the same for continuous and intermittent motion, and that it remains a constant fraction
![]() $c_{m}$
of the power lost in continuous swimming, Floryan et al. find that
$c_{m}$
of the power lost in continuous swimming, Floryan et al. find that
From figure 37(a) we see that the data and the scaling tell the same story: even though intermittent motions expend less energy on swimming, the metabolic losses can play a significant role because intermittent motions take more time to traverse a given distance than continuous motions. The extra time to travel will increase the metabolic energy losses, and this effect may dominate the benefits gained in reducing swimming energy losses. Estimates for
![]() $c_{m}$
from nature are difficult to obtain, but it is likely in the range shown in figure 37(a), and so the possible benefits of intermittent swimming will vary with species.
$c_{m}$
from nature are difficult to obtain, but it is likely in the range shown in figure 37(a), and so the possible benefits of intermittent swimming will vary with species.

Figure 37. (a) Ratio of energy expended by intermittent motions to energy expended by continuous motions, including metabolic energy losses, as a function of duty cycle for
![]() $\unicode[STIX]{x1D703}_{0}=15^{\circ }$
, all frequencies, with
$\unicode[STIX]{x1D703}_{0}=15^{\circ }$
, all frequencies, with
![]() $C_{Db}=0.1$
. Each point is an average over all frequencies. The shading denotes the value of the metabolic power fraction
$C_{Db}=0.1$
. Each point is an average over all frequencies. The shading denotes the value of the metabolic power fraction
![]() $c_{m}$
, 0 to 2 in intervals of 0.25 (dark to light). (b) Ratio of energy expended by intermittent motions to energy expended by continuous motions, restricted to equal mean speeds. Dashed lines correspond to the model. The symbol grey scale corresponds to three values of (dimensional) mean speed chosen,
$c_{m}$
, 0 to 2 in intervals of 0.25 (dark to light). (b) Ratio of energy expended by intermittent motions to energy expended by continuous motions, restricted to equal mean speeds. Dashed lines correspond to the model. The symbol grey scale corresponds to three values of (dimensional) mean speed chosen,
![]() $U_{mean}=0.2,0.25,0.3$
(dark to light). The frequency of the intermittent motion was chosen so that it would have a speed equal to the continuous motion. Reproduction with permission from Floryan et al. (Reference Floryan, Van Buren and Smits2017b
).
$U_{mean}=0.2,0.25,0.3$
(dark to light). The frequency of the intermittent motion was chosen so that it would have a speed equal to the continuous motion. Reproduction with permission from Floryan et al. (Reference Floryan, Van Buren and Smits2017b
).
It is also possible to restrict ourselves to motions which produce the same mean speed, that is,
![]() $\unicode[STIX]{x1D719}|_{U_{mean}}$
. For example, it may be that a continuous motion produces the same mean speed as an intermittent motion with a duty cycle of 0.5 actuating at twice the frequency. Which strategy is best if a swimmer wants to traverse a given distance in a given amount of time? The results are plotted in figure 37(b), where the lines in the figure are derived using the propulsive model derived in § 3.1 to make estimates for the thrust and power exerted in the free swimming condition. It appears that intermittent motions continue to be energetically favourable with the added time restriction. Energetically optimal duty cycles exist, and savings are greater for lower speeds, at least for the data considered here, despite having to increase the frequency of actuation in order to match the mean speed of continuous motions.
$\unicode[STIX]{x1D719}|_{U_{mean}}$
. For example, it may be that a continuous motion produces the same mean speed as an intermittent motion with a duty cycle of 0.5 actuating at twice the frequency. Which strategy is best if a swimmer wants to traverse a given distance in a given amount of time? The results are plotted in figure 37(b), where the lines in the figure are derived using the propulsive model derived in § 3.1 to make estimates for the thrust and power exerted in the free swimming condition. It appears that intermittent motions continue to be energetically favourable with the added time restriction. Energetically optimal duty cycles exist, and savings are greater for lower speeds, at least for the data considered here, despite having to increase the frequency of actuation in order to match the mean speed of continuous motions.
3.9 Non-sinusoidal motions
Thus far, we have always assumed that the foil is actuated sinusoidally, but there may be times when animals use non-sinusoidal motions, and there may be benefits to doing so. In terms of optimization, Kaya & Tuncer (Reference Kaya and Tuncer2007) numerically studied heaving and pitching airfoil performance in laminar air flow, and used gradient-based optimization of the motion paths to show that there were significant benefits to thrust by moving non-sinusoidally. They found that motions that maintain a constant angle of attack for longer periods of time, a topic also considered by Read et al. (Reference Read, Hover and Triantafyllou2003), produced higher thrust than purely sinusoidal motions. Similar results were reported by Van Buren et al. (Reference Van Buren, Floryan, Quinn and Smits2017b
), who used Jacobi elliptic functions to vary the shape of the actuation from more triangular (
![]() $\unicode[STIX]{x1D705}=-0.99$
), to sinusoidal (
$\unicode[STIX]{x1D705}=-0.99$
), to sinusoidal (
![]() $\unicode[STIX]{x1D705}=0$
), to more square-like motions (
$\unicode[STIX]{x1D705}=0$
), to more square-like motions (
![]() $\unicode[STIX]{x1D705}=+0.99$
), as shown in figure 38.
$\unicode[STIX]{x1D705}=+0.99$
), as shown in figure 38.

Figure 38. Jacobi elliptic functions produce varying actuation waveform shape based on the elliptic modulus,
![]() $\unicode[STIX]{x1D705}$
. Coloured circles represent points of vortex production in the cycle based on PIV measurements, and smaller circles correspond to secondary vortices (see figure 41). Adapted with permission from Van Buren et al. (Reference Van Buren, Floryan, Quinn and Smits2017b
).
$\unicode[STIX]{x1D705}$
. Coloured circles represent points of vortex production in the cycle based on PIV measurements, and smaller circles correspond to secondary vortices (see figure 41). Adapted with permission from Van Buren et al. (Reference Van Buren, Floryan, Quinn and Smits2017b
).
Their results for heaving motions of a tear-drop shaped foil are shown in figure 39. We see that square-like motions exhibit much higher thrust and power than the triangular-like and sinusoidal motions. Similar results were found for pitch. This variation of thrust with
![]() $\unicode[STIX]{x1D705}$
suggested that the thrust may scale with waveform peak velocity, especially for heaving motions. Empirically, a function
$\unicode[STIX]{x1D705}$
suggested that the thrust may scale with waveform peak velocity, especially for heaving motions. Empirically, a function
![]() $P_{2}$
was found that was only a function of the peak velocity. When the scaling of Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a
) is combined with this peak velocity scaling parameter, there is an encouraging collapse of the thrust and power coefficients for a large range of motion parameters, as shown in figure 39. The empirical constants used in the scaling were identical to those found by Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a
) for purely sinusoidal motions.
$P_{2}$
was found that was only a function of the peak velocity. When the scaling of Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a
) is combined with this peak velocity scaling parameter, there is an encouraging collapse of the thrust and power coefficients for a large range of motion parameters, as shown in figure 39. The empirical constants used in the scaling were identical to those found by Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a
) for purely sinusoidal motions.

Figure 39. Performance as a function of Strouhal number, for heave. (a,b) (i) Thrust coefficient; (ii) power coefficient versus Strouhal number. (c,d) (i) Thrust and (ii) power modified by the lateral velocity scale plotted against the scaling proposed by Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a
). Symbol colour identifies the waveform shape, and tone represents amplitude of motion ranging from low (dark) to high (light). For heave,
![]() $h/c=6.25\,\%{-}18.75\,\%$
every 2.5 %. For pitch
$h/c=6.25\,\%{-}18.75\,\%$
every 2.5 %. For pitch
![]() $\unicode[STIX]{x1D703}=3^{\circ }{-}15^{\circ }$
every
$\unicode[STIX]{x1D703}=3^{\circ }{-}15^{\circ }$
every
![]() $2^{\circ }$
. Adapted with permission from Van Buren et al. (Reference Van Buren, Floryan, Quinn and Smits2017b
).
$2^{\circ }$
. Adapted with permission from Van Buren et al. (Reference Van Buren, Floryan, Quinn and Smits2017b
).
The efficiency as a function of thrust is given in figure 40. For heave, the effects of waveform shape are rather minor (within the range of amplitudes studied), but for pitch increasing
![]() $\unicode[STIX]{x1D705}$
tends to decrease the efficiency at a given thrust, and the highest peak efficiency is achieved with sinusoidal motions. We see that actuation waveform can be used to regulate thrust/efficiency trade-offs during locomotion, for a given frequency and amplitude.
$\unicode[STIX]{x1D705}$
tends to decrease the efficiency at a given thrust, and the highest peak efficiency is achieved with sinusoidal motions. We see that actuation waveform can be used to regulate thrust/efficiency trade-offs during locomotion, for a given frequency and amplitude.

Figure 40. Efficiency versus thrust coefficient for (a) heave, and (b) pitch. Symbols and colours as in figure 39. Adapted with permission from Van Buren et al. (Reference Van Buren, Floryan, Quinn and Smits2017b ).

Figure 41. Heaving foil. (i) Phase- and (ii) time-averaged change in streamwise velocity (
![]() $h/c=12.5\,\%$
,
$h/c=12.5\,\%$
,
![]() $St=0.4$
). Waveform: (a) triangular-like
$St=0.4$
). Waveform: (a) triangular-like
![]() $\unicode[STIX]{x1D705}=-0.99$
; (b) sinusoidal
$\unicode[STIX]{x1D705}=-0.99$
; (b) sinusoidal
![]() $\unicode[STIX]{x1D705}=0$
; (c) square-like
$\unicode[STIX]{x1D705}=0$
; (c) square-like
![]() $\unicode[STIX]{x1D705}=0.99$
. Reproduction with permission from Van Buren et al. (Reference Van Buren, Floryan, Quinn and Smits2017b
).
$\unicode[STIX]{x1D705}=0.99$
. Reproduction with permission from Van Buren et al. (Reference Van Buren, Floryan, Quinn and Smits2017b
).
In addition to its effect on the performance, the waveform shape has a dramatic impact on the vortex structure produced in the wake of the foil. The rapid start and stop of square-like wave type motions produces vortex pairs instead of the typical vortex street, resulting in a dual-jet velocity wake (see figure 41). This wake structure was associated with large increases in the thrust, producing up to four times higher forces than sinusoidal motions. These effects are more pronounced in heave than in pitch, though similar trends were found in both cases and across all Strouhal numbers studied (
![]() $St=0.2$
, 0.3, and 0.4). Triangular-like and sinusoidal motions showed more typical behaviour, with a reverse von Kármán vortex street and a single jet-like wake.
$St=0.2$
, 0.3, and 0.4). Triangular-like and sinusoidal motions showed more typical behaviour, with a reverse von Kármán vortex street and a single jet-like wake.
As Van Buren et al. (Reference Van Buren, Floryan, Quinn and Smits2017b ) indicate, these results imply that animals and vehicles could use non-sinusoidal propulsive motions to increase thrust, efficiency or swimming speed, depending on the need. A square wave motion, for example, could be used to accelerate quickly, whereas a sinusoidal motion could be used for efficient cruise. These ideas are consistent with the diversity of motion types seen in biological swimmers, and they suggest new strategies for effective motor control in swimming robots.
3.10 Contribution of caudal fin to total thrust
Our aim in modelling the propulsive forces produced by an oscillating foil was to help understand the swimming of fish and mammals. At the outset (§ 3), we made the assumption that for oscillatory swimming the caudal fin is the principal source of thrust, and the body is the principal source of drag. Now that we have some reasonable models for the production of thrust by heaving and pitching foils we can examine the plausibility of this approximation, at least for the case of the tuna. To do so, we revisit the analysis by Bainbridge (Reference Bainbridge1963) of the swimming performance of dace, bream and goldfish (see figure 12). In particular, Bainbridge estimated the contribution of the caudal fin to the total thrust by using biological data on lateral velocity and planform area distributions. He assumed that for a segment of planform area
![]() $\text{d}A$
the local thrust contribution is proportional to
$\text{d}A$
the local thrust contribution is proportional to
![]() $V^{2}\,\text{d}A$
, so that
$V^{2}\,\text{d}A$
, so that
where
![]() $T$
is the mean total thrust in steady swimming,
$T$
is the mean total thrust in steady swimming,
![]() $V$
is the local transverse velocity and the first integral is taken over the body (from the snout to the fork) while the second integral is taken over the caudal fin. This scaling with the lateral velocity is entirely in accord with many of the observations we have made so far. As indicated earlier, Bainbridge found that the proportion of the total thrust contributed by the caudal fin varied from 45 % for the bream, 65 % for the goldfish, to 84 % for the dace, while Gray (Reference Gray1933) estimated 40 % for the whiting.
$V$
is the local transverse velocity and the first integral is taken over the body (from the snout to the fork) while the second integral is taken over the caudal fin. This scaling with the lateral velocity is entirely in accord with many of the observations we have made so far. As indicated earlier, Bainbridge found that the proportion of the total thrust contributed by the caudal fin varied from 45 % for the bream, 65 % for the goldfish, to 84 % for the dace, while Gray (Reference Gray1933) estimated 40 % for the whiting.
A similar analysis may be performed for a member of the tuna family, in particular the kawakawa (Euthynnus affinis, or mackerel tuna, see figure 12). Donley & Dickson (Reference Donley and Dickson2000) measured the mean maximum intervertebral lateral displacement of juvenile kawakawa during steady swimming, which together with measurements of the tail beat frequency and the assumption of sinusoidal actuation gives an estimate of
![]() $V$
. Taking the planform aspect from the literature (as in figure 12) allows us to estimate the terms in (3.23), and the result indicates that for this member of the tuna family the caudal fin contributes approximately 40 % of the total thrust.
$V$
. Taking the planform aspect from the literature (as in figure 12) allows us to estimate the terms in (3.23), and the result indicates that for this member of the tuna family the caudal fin contributes approximately 40 % of the total thrust.
These analyses, however, overlook an important distinction between the thrust due to body movement and that due to caudal fin oscillation: the former is primarily a flapping or pitching motion, whereas the latter is primarily a combination of heave and pitch motions with a phase difference of approximately
![]() $270^{\circ }$
. The difference is that heave is a much more effective means of generating thrust compared to pitch. For rigid, rectangular panels, for example, Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a
) found that at a Strouhal number
$270^{\circ }$
. The difference is that heave is a much more effective means of generating thrust compared to pitch. For rigid, rectangular panels, for example, Floryan et al. (Reference Floryan, Van Buren, Rowley and Smits2017a
) found that at a Strouhal number
![]() $St=0.35$
a heaving motion gives
$St=0.35$
a heaving motion gives
![]() $C_{T}=0.6$
compared to a value of 0.2 for a pitching motion, at comparable amplitudes of the trailing edge motion (
$C_{T}=0.6$
compared to a value of 0.2 for a pitching motion, at comparable amplitudes of the trailing edge motion (
![]() $h/c=0.1875$
for heave, and
$h/c=0.1875$
for heave, and
![]() $\unicode[STIX]{x1D703}=10^{\circ }=0.1745$
rad for pitch), see § 3.1. That is, under similar conditions heave produces about three times the thrust produced by pitch. The difference is even larger in combined pitch and heave, where our earlier observations indicated a factor closer to five (see § 3.4). Therefore, in estimating the thrust contributions of the body motion compared to the caudal fin motion, we need to take these differences into account by weighting the caudal fin contribution given by
$\unicode[STIX]{x1D703}=10^{\circ }=0.1745$
rad for pitch), see § 3.1. That is, under similar conditions heave produces about three times the thrust produced by pitch. The difference is even larger in combined pitch and heave, where our earlier observations indicated a factor closer to five (see § 3.4). Therefore, in estimating the thrust contributions of the body motion compared to the caudal fin motion, we need to take these differences into account by weighting the caudal fin contribution given by
![]() $I_{2}$
in (3.23) by a factor close to five (for similar amplitudes of motion). When this is done for the kawakawa example, we find that the contribution by the caudal fin to the total thrust increases from 40 % to 77 %.
$I_{2}$
in (3.23) by a factor close to five (for similar amplitudes of motion). When this is done for the kawakawa example, we find that the contribution by the caudal fin to the total thrust increases from 40 % to 77 %.
We also need to take into account the effects of aspect ratio, ![]() . The measurements on rectangular foils by Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013) and theoretical considerations by Ayancik et al. (Reference Ayancik, Zhong, Quinn, Brandes, Bart-Smith and Moored2018) indicate that the fall-off in thrust due to the contribution made by added mass forces is important only at small values of
. The measurements on rectangular foils by Dewey et al. (Reference Dewey, Boschitsch, Moored, Stone and Smits2013) and theoretical considerations by Ayancik et al. (Reference Ayancik, Zhong, Quinn, Brandes, Bart-Smith and Moored2018) indicate that the fall-off in thrust due to the contribution made by added mass forces is important only at small values of ![]() (figure 32), and the fall-off due to circulatory forces depends approximately on
(figure 32), and the fall-off due to circulatory forces depends approximately on ![]() . For pitching motions, the thrust is due primarily to added mass forces, so that it needs to be discounted by a factor similar to that shown in figure 32. For heaving motions, the thrust is primarily due to circulatory forces, so that it needs to be discounted by the factor approximately given by
. For pitching motions, the thrust is due primarily to added mass forces, so that it needs to be discounted by a factor similar to that shown in figure 32. For heaving motions, the thrust is primarily due to circulatory forces, so that it needs to be discounted by the factor approximately given by ![]() . For the kawakawa caudal fin, we have
. For the kawakawa caudal fin, we have ![]() , and for the body we use the local aspect ratio defined as
, and for the body we use the local aspect ratio defined as
![]() $s^{2}/\text{d}A$
, where
$s^{2}/\text{d}A$
, where
![]() $s$
is the local span of the fish in side view. The contribution by the caudal fin to the total thrust then increases from 77 % to 86 %.
$s$
is the local span of the fish in side view. The contribution by the caudal fin to the total thrust then increases from 77 % to 86 %.
We see that in modelling the propulsive forces produced by swimming fish of the kind considered here (low predation, steady swimming, high flow; Langerhans & Reznick Reference Langerhans and Reznick2010), it seems reasonable to assume that the caudal fin is the principal source of thrust, and the contribution made by the body motion can be discounted.
4 Mobuliform swimming
For undulatory swimming, we used eels and lampreys as prototypical examples, and for oscillatory swimming we put forward tuna and dolphins. Batoid swimmers like rays use a different propulsive mechanism, in that they use their greatly enlarged pectoral fins to propel themselves, and can display either undulatory or oscillatory swimming behaviours, called rajiform and mobuliform, respectively (Rosenberger & Westneat Reference Rosenberger and Westneat1999; Rosenberger Reference Rosenberger2001; Fish et al.
Reference Fish, Schreiber, Moored, Liu, Dong and Bart-Smith2016, Reference Fish, Dong, Zhu and Bart-Smith2017). Rays are fish of the order Batoidei. They are related to sharks, and the order encompasses more than 500 species. Most species live on the ocean floor, but a few, such as the manta ray, live in the open sea. Rays such as the blue spotted and southern stingray are undulatory swimmers and display at least a full wavelength of activation on their pectoral fin (
![]() $\unicode[STIX]{x1D706}^{\ast }=\unicode[STIX]{x1D706}/c<1$
), while manta rays and cownose rays are oscillatory swimmers and display less than a full wavelength of activation (
$\unicode[STIX]{x1D706}^{\ast }=\unicode[STIX]{x1D706}/c<1$
), while manta rays and cownose rays are oscillatory swimmers and display less than a full wavelength of activation (
![]() $\unicode[STIX]{x1D706}^{\ast }>1$
). One of the important aspects of mobuliform swimming is that rays precisely control the shape of their fins to swim and manoeuver. While this is also true for undulatory swimmers such as eels and knifefish, manta rays provide a good example of an oscillatory swimmer that uses active rather than passive flexibility to control the amplitude and wavelength of the actuating waveform.
$\unicode[STIX]{x1D706}^{\ast }>1$
). One of the important aspects of mobuliform swimming is that rays precisely control the shape of their fins to swim and manoeuver. While this is also true for undulatory swimmers such as eels and knifefish, manta rays provide a good example of an oscillatory swimmer that uses active rather than passive flexibility to control the amplitude and wavelength of the actuating waveform.
To help understand the swimming of rays that use oscillatory propulsion, Clark & Smits (Reference Clark and Smits2006) performed experiments on a flexible fin that replicated some features of the pectoral fin of a manta ray or cownose ray. The cross-section of the fin was approximated by a NACA0020 airfoil section, which is close to that seen in nature, and the planform was semi-elliptic, with the root chord assumed to act as a plane of symmetry (![]() for the half-span). The fin was aligned with the flow direction, and it was actuated in a travelling wave motion, with the wave amplitude increasing linearly along the span from root to tip (see figure 42). By changing the phase angle
for the half-span). The fin was aligned with the flow direction, and it was actuated in a travelling wave motion, with the wave amplitude increasing linearly along the span from root to tip (see figure 42). By changing the phase angle
![]() $\unicode[STIX]{x1D719}$
between the individual actuators from 0, 30, 60, 90, 120 and
$\unicode[STIX]{x1D719}$
between the individual actuators from 0, 30, 60, 90, 120 and
![]() $240^{\circ }$
, the wavelength could be varied from
$240^{\circ }$
, the wavelength could be varied from
![]() $\unicode[STIX]{x1D706}^{\ast }=\infty$
, to 12, 6, 4, 3 and 2. Since the wavelength was always larger than the chord of the fin, the motion was oscillatory in nature, with the added feature of having a fin with a chord-wise profile that conformed to the prescribed actuation.
$\unicode[STIX]{x1D706}^{\ast }=\infty$
, to 12, 6, 4, 3 and 2. Since the wavelength was always larger than the chord of the fin, the motion was oscillatory in nature, with the added feature of having a fin with a chord-wise profile that conformed to the prescribed actuation.
The thrust and efficiency results are shown in figure 43. Thrust generally increases with Strouhal number, as expected, but local peaks are present in the vicinity of
![]() $St=0.3$
–0.35, where the Strouhal number is based on the amplitude of the trailing edge motion at midspan. The highest thrust was found to occur at either
$St=0.3$
–0.35, where the Strouhal number is based on the amplitude of the trailing edge motion at midspan. The highest thrust was found to occur at either
![]() $\unicode[STIX]{x1D706}^{\ast }=4$
or 6 (
$\unicode[STIX]{x1D706}^{\ast }=4$
or 6 (
![]() $\unicode[STIX]{x1D719}=90^{\circ }$
or
$\unicode[STIX]{x1D719}=90^{\circ }$
or
![]() $60^{\circ }$
). These wavelengths also gave the highest efficiencies, with a global maximum efficiency of approximately 54 % for
$60^{\circ }$
). These wavelengths also gave the highest efficiencies, with a global maximum efficiency of approximately 54 % for
![]() $\unicode[STIX]{x1D706}^{\ast }=6$
, which is impressive for such a relatively crude approximation to manta ray locomotion. Also, the optimal wavelength of
$\unicode[STIX]{x1D706}^{\ast }=6$
, which is impressive for such a relatively crude approximation to manta ray locomotion. Also, the optimal wavelength of
![]() $\unicode[STIX]{x1D706}^{\ast }=4$
to 6 is in accord with observations of manta rays in nature by Rosenberger (Reference Rosenberger2001). Most intriguingly, the efficiencies displayed two local peaks; one at approximately
$\unicode[STIX]{x1D706}^{\ast }=4$
to 6 is in accord with observations of manta rays in nature by Rosenberger (Reference Rosenberger2001). Most intriguingly, the efficiencies displayed two local peaks; one at approximately
![]() $St=0.2$
to 0.225, and another at approximately
$St=0.2$
to 0.225, and another at approximately
![]() $St=0.3$
.
$St=0.3$
.

Figure 42. Fin actuation mechanism. A DC motor with a speed controller turns a shaft at frequency
![]() $f$
which powers a gear train. The rotation of the gears actuates rods which impose a travelling wave along the fin through rigid spars. PIV was used to investigate the structure of the wake. Reproduction with permission from Dewey, Carriou & Smits (Reference Dewey, Carriou and Smits2012).
$f$
which powers a gear train. The rotation of the gears actuates rods which impose a travelling wave along the fin through rigid spars. PIV was used to investigate the structure of the wake. Reproduction with permission from Dewey, Carriou & Smits (Reference Dewey, Carriou and Smits2012).

Figure 43. (a) Coefficient of thrust for different traveling wave phase differentials
![]() $\unicode[STIX]{x1D719}$
. (b) Efficiency at
$\unicode[STIX]{x1D719}$
. (b) Efficiency at
![]() $\unicode[STIX]{x1D719}=60^{\circ }$
and
$\unicode[STIX]{x1D719}=60^{\circ }$
and
![]() $90^{\circ }$
(
$90^{\circ }$
(
![]() $\unicode[STIX]{x1D706}^{\ast }=6$
and 4). For the fin, the Strouhal number
$\unicode[STIX]{x1D706}^{\ast }=6$
and 4). For the fin, the Strouhal number
![]() $St_{A}$
is based on
$St_{A}$
is based on
![]() $\bar{A}$
, the trailing edge displacement at the mid-chord; for the other cases,
$\bar{A}$
, the trailing edge displacement at the mid-chord; for the other cases,
![]() $A=\bar{A}$
. Reproduction with permission from Clark & Smits (Reference Clark and Smits2006).
$A=\bar{A}$
. Reproduction with permission from Clark & Smits (Reference Clark and Smits2006).
Dewey et al. (Reference Dewey, Carriou and Smits2012) used PIV to investigate the wake structure corresponding to these thrust and efficiency trends. The highly three-dimensional wake exhibited a structure that varied with both the spanwise location and the streamwise location. Because the circulation distribution at any point in the flapping cycle varies along the span of the fin, with it being zero at the root and tip, the trailing edge vortex varies in strength along the span. It also varies in time, of course, because the motion is periodic. At
![]() $St=0.25$
and
$St=0.25$
and
![]() $\unicode[STIX]{x1D706}^{\ast }=4$
(highest efficiency condition), both a 2S and a 2P wake were observed at different spanwise locations (2S at midspan), as also seen by Heathcote, Wang & Gursul (Reference Heathcote, Wang and Gursul2008) for flexible foils where the peak-to-peak amplitude increased with span. The wavelength of actuation had a significant impact, in that it caused a transition from a 2S to a 2P structure at a particular downstream location (see figure 44). This transition appeared to be directly linked to the increasing phase delay of the trailing edge of the fin with decreasing wavelength, and indicates that for a given Strouhal number (or non-dimensional wavelength) there is a specific non-dimensional wavelength (or Strouhal number) such that a transition from a 2S to 2P wake structure occurs. In fact, a transition from 2P to 2S always occurs with increasing Strouhal number, and at a fixed Strouhal number the distance from the trailing edge to the location of the bifurcation increases with decreasing wavelength. Similarly, for a fixed bifurcation distance, the longest wavelength (
$\unicode[STIX]{x1D706}^{\ast }=4$
(highest efficiency condition), both a 2S and a 2P wake were observed at different spanwise locations (2S at midspan), as also seen by Heathcote, Wang & Gursul (Reference Heathcote, Wang and Gursul2008) for flexible foils where the peak-to-peak amplitude increased with span. The wavelength of actuation had a significant impact, in that it caused a transition from a 2S to a 2P structure at a particular downstream location (see figure 44). This transition appeared to be directly linked to the increasing phase delay of the trailing edge of the fin with decreasing wavelength, and indicates that for a given Strouhal number (or non-dimensional wavelength) there is a specific non-dimensional wavelength (or Strouhal number) such that a transition from a 2S to 2P wake structure occurs. In fact, a transition from 2P to 2S always occurs with increasing Strouhal number, and at a fixed Strouhal number the distance from the trailing edge to the location of the bifurcation increases with decreasing wavelength. Similarly, for a fixed bifurcation distance, the longest wavelength (
![]() $\unicode[STIX]{x1D706}^{\ast }=\infty$
) causes the bifurcation to occur at the lowest Strouhal number.
$\unicode[STIX]{x1D706}^{\ast }=\infty$
) causes the bifurcation to occur at the lowest Strouhal number.

Figure 44. Wake transition from 2P to 2S with increasing Strouhal number at the midspan: (a,b)
![]() $\unicode[STIX]{x1D706}^{\ast }=6$
,
$\unicode[STIX]{x1D706}^{\ast }=6$
,
![]() $St=0.15$
(a) and
$St=0.15$
(a) and
![]() $St=0.25$
(b); (c,d)
$St=0.25$
(b); (c,d)
![]() $\unicode[STIX]{x1D706}^{\ast }=3$
,
$\unicode[STIX]{x1D706}^{\ast }=3$
,
![]() $St=0.2$
(c) and
$St=0.2$
(c) and
![]() $St=0.3$
(d). Flow is from left to right, and the trailing edge of the fin is outlined in black. Adapted with permission from Dewey et al. (Reference Dewey, Carriou and Smits2012).
$St=0.3$
(d). Flow is from left to right, and the trailing edge of the fin is outlined in black. Adapted with permission from Dewey et al. (Reference Dewey, Carriou and Smits2012).
5 Wake resonance
Moored et al. (Reference Moored, Dewey, Smits and Haj-Hariri2012) sought to gain a better understanding of the conditions necessary for efficient propulsion, and how these conditions make themselves evident in the flow field. They introduced the concept of wake resonance, which is based on a linear stability analysis of the mean velocity profile in the wake. The work built on that originally done by Triantafyllou et al. (Reference Triantafyllou, Triantafyllou and Grosenbaugh1993), who performed a linear stability analysis of the time-averaged wake behind a two-dimensional foil pitching about its quarter chord point with a small amplitude. They found that the maximum spatial growth of the instabilities of the velocity jet occurred over a range of Strouhal numbers of 0.25 to 0.35, corresponding to the range of Strouhal numbers observed for fish and mammals in nature. Hence, they inferred that efficient swimming occurs at the frequency of maximum amplification (see also § 1.2). That is, the perturbation waves will have the largest amplification (per unit input energy) at the ‘resonant’ frequency of the jet profile (where there is maximum spatial growth), and that this will cause an expedient shear layer roll up and entrainment, and result in the strongest momentum jet for a given input energy. Thus a peak in the propulsive efficiency is expected when the fin is driven at the resonant frequency of the jet. By inference, this peak was associated with a 2S (reverse von Kármán vortex street), and an ‘optimal’ Strouhal number range of
![]() $0.25<St<0.35$
.
$0.25<St<0.35$
.
Although many animals swim in this Strouhal number range, we have seen that there are many cases where the peak efficiency falls outside this range (Anderson et al.
Reference Anderson, Streitlien, Barrett and Triantafyllou1998; Taylor, Nudds & Thomas Reference Taylor, Nudds and Thomas2003; Clark & Smits Reference Clark and Smits2006; Buchholz & Smits Reference Buchholz and Smits2008). Furthermore, elongated fish such as the eel and lamprey typically generate
![]() $2P$
wakes meaning that two pairs of vortices are shed per cycle (see figure 4
a), and fish with low aspect ratio propulsors such as rays can exhibit both
$2P$
wakes meaning that two pairs of vortices are shed per cycle (see figure 4
a), and fish with low aspect ratio propulsors such as rays can exhibit both
![]() $2S$
and
$2S$
and
![]() $2P$
wakes depending upon the fin kinematics (see figure 44). Other more elaborate wake structures have also been observed in the wakes of heaving and pitching airfoils (Lewin & Haj-Hariri Reference Lewin and Haj-Hariri2003; Lentink et al.
Reference Lentink, Muijres, Donker-Duyvis and van Leeuwen2008).
$2P$
wakes depending upon the fin kinematics (see figure 44). Other more elaborate wake structures have also been observed in the wakes of heaving and pitching airfoils (Lewin & Haj-Hariri Reference Lewin and Haj-Hariri2003; Lentink et al.
Reference Lentink, Muijres, Donker-Duyvis and van Leeuwen2008).
For the model of mobuliform locomotion studied by Clark & Smits (Reference Clark and Smits2006) and Dewey et al. (Reference Dewey, Carriou and Smits2012), the instantaneous flow field at any spanwise location is characterized by the formation of wavy jet structures (figure 45
b), while the time-averaged flow field exhibits a jet-like behaviour (figure 45
c). In most cases, the jet can be considered weakly non-parallel, meaning that the characteristic length scale of a velocity profile changes slowly in the downstream direction as compared to the instability wavelength (Chomaz Reference Chomaz2005), and so the flow may be analysed using a local spatial stability analysis. Moored et al. (Reference Moored, Dewey, Smits and Haj-Hariri2012) found that when the driving frequency is close to the jet resonant frequency, a peak in efficiency is expected. This may occur at more than one Strouhal number. With
![]() $\unicode[STIX]{x1D706}^{\ast }=4$
, for example, the driving frequency is nearly coincident with the resonant frequency at two Strouhal numbers:
$\unicode[STIX]{x1D706}^{\ast }=4$
, for example, the driving frequency is nearly coincident with the resonant frequency at two Strouhal numbers:
![]() $St=0.2$
and 0.3 (see figure 46). Moreover, the two wake resonant frequencies from the linear stability analysis match closely to the two peaks observed in efficiency.
$St=0.2$
and 0.3 (see figure 46). Moreover, the two wake resonant frequencies from the linear stability analysis match closely to the two peaks observed in efficiency.

Figure 45. (a) Schematic of a ray-like robotic pectoral fin and the vortex wake structure at a laser illuminated plane, (b) reverse von Kármán vortex street inducing a wavy jet and (c) time-average velocity field. The
![]() $x$
- and
$x$
- and
![]() $y$
-coordinates are non-dimensionalized with the chord length,
$y$
-coordinates are non-dimensionalized with the chord length,
![]() $L$
. Adapted with permission from Moored et al. (Reference Moored, Dewey, Smits and Haj-Hariri2012).
$L$
. Adapted with permission from Moored et al. (Reference Moored, Dewey, Smits and Haj-Hariri2012).

Figure 46. (a) Propulsive efficiency data of the elliptical fin measured by Clark & Smits (Reference Clark and Smits2006) for
![]() $\unicode[STIX]{x1D706}^{\ast }=4$
. Dashed lines denote the resonant frequencies found by the linear stability analysis. The solid lines denote the regions of uncertainty in the wake resonant frequencies. (b) Stability curves for 5 velocity profiles taken from DPIV data measured by Dewey et al. (Reference Dewey, Carriou and Smits2012), where
$\unicode[STIX]{x1D706}^{\ast }=4$
. Dashed lines denote the resonant frequencies found by the linear stability analysis. The solid lines denote the regions of uncertainty in the wake resonant frequencies. (b) Stability curves for 5 velocity profiles taken from DPIV data measured by Dewey et al. (Reference Dewey, Carriou and Smits2012), where
![]() $\unicode[STIX]{x1D6FC}_{i}$
is the imaginary part of the complex wavenumber. The
$\unicode[STIX]{x1D6FC}_{i}$
is the imaginary part of the complex wavenumber. The
![]() $\times$
mark the resonant frequency of a stability curve while the ○ mark the driving frequency used to generate the velocity profile. Reproduction with permission from Moored et al. (Reference Moored, Dewey, Smits and Haj-Hariri2012).
$\times$
mark the resonant frequency of a stability curve while the ○ mark the driving frequency used to generate the velocity profile. Reproduction with permission from Moored et al. (Reference Moored, Dewey, Smits and Haj-Hariri2012).
To connect the analysis to the wake structure, Moored et al. (Reference Moored, Dewey, Smits and Haj-Hariri2012) then examined the eigenfunction,
![]() $\unicode[STIX]{x1D719}(y)$
, associated with each eigenvalue. The eigenfunctions relate to stream function perturbations and thus velocity perturbations that must be superimposed onto the base flow. The linear vorticity perturbation can also be obtained from
$\unicode[STIX]{x1D719}(y)$
, associated with each eigenvalue. The eigenfunctions relate to stream function perturbations and thus velocity perturbations that must be superimposed onto the base flow. The linear vorticity perturbation can also be obtained from
![]() $\unicode[STIX]{x1D719}(y)$
and captures many of the features of the observed wake structures. For example, the vorticity eigenmodes for
$\unicode[STIX]{x1D719}(y)$
and captures many of the features of the observed wake structures. For example, the vorticity eigenmodes for
![]() $\unicode[STIX]{x1D706}^{\ast }=4$
are compared with the wake structure at the midspan at three Strouhal numbers in figure 47. At the first peak in efficiency (at
$\unicode[STIX]{x1D706}^{\ast }=4$
are compared with the wake structure at the midspan at three Strouhal numbers in figure 47. At the first peak in efficiency (at
![]() $St=0.2$
for this wavelength), the vorticity perturbation exhibits a characteristic
$St=0.2$
for this wavelength), the vorticity perturbation exhibits a characteristic
![]() $2P$
pattern, although some asymmetry occurs and the eigenfunction has a slightly different spatial wavelength compared to the experimentally observed vortices. The experimentally observed wake displays a
$2P$
pattern, although some asymmetry occurs and the eigenfunction has a slightly different spatial wavelength compared to the experimentally observed vortices. The experimentally observed wake displays a
![]() $2P$
structure in the near wake, but for
$2P$
structure in the near wake, but for
![]() $x>0.5$
a transition from
$x>0.5$
a transition from
![]() $2P$
to
$2P$
to
![]() $2S$
is occurring, which is reflected in the time-averaged velocity field (not shown here). When the Strouhal number increases, as in figure 47(c–f), the wake structures and vorticity eigenmodes begin to display the characteristics of a
$2S$
is occurring, which is reflected in the time-averaged velocity field (not shown here). When the Strouhal number increases, as in figure 47(c–f), the wake structures and vorticity eigenmodes begin to display the characteristics of a
![]() $2S$
wake. Each of the wake resonant frequencies can now be associated with a specific wake mode. There is a resonant frequency for the
$2S$
wake. Each of the wake resonant frequencies can now be associated with a specific wake mode. There is a resonant frequency for the
![]() $2P$
wake mode and a resonant frequency for the
$2P$
wake mode and a resonant frequency for the
![]() $2S$
wake mode (as labelled in figure 46
a).
$2S$
wake mode (as labelled in figure 46
a).

Figure 47. Observed wake structures for
![]() $\unicode[STIX]{x1D706}^{\ast }=4$
at (a)
$\unicode[STIX]{x1D706}^{\ast }=4$
at (a)
![]() $St=0.2$
, (c)
$St=0.2$
, (c)
![]() $St=0.25$
and (e)
$St=0.25$
and (e)
![]() $St=0.3$
. The vorticity perturbations (b), (d) and (f) are at the same parameters, respectively. A transition from a
$St=0.3$
. The vorticity perturbations (b), (d) and (f) are at the same parameters, respectively. A transition from a
![]() $2P$
wake to a
$2P$
wake to a
![]() $2S$
wake (dashed line) is observed. Reproduction with permission from Moored et al. (Reference Moored, Dewey, Smits and Haj-Hariri2012).
$2S$
wake (dashed line) is observed. Reproduction with permission from Moored et al. (Reference Moored, Dewey, Smits and Haj-Hariri2012).
These wake resonance results, although derived in the context of manta ray locomotion, are expected to hold more generally. When combined with the experiments of Clark & Smits (Reference Clark and Smits2006) and Dewey et al. (Reference Dewey, Carriou and Smits2012), we now have a preliminary prescription for efficient unsteady propulsion. First, tuning the driving frequency to a wake resonant frequency results in a local peak in efficiency. Second, there may be multiple wake resonant frequencies relating to multiple peaks in efficiency. Third, some observed wake structures will transition when the wake instability modes transition. We see that a 2S wake structure is not the only wake mode that leads to efficient locomotion and other wake modes such as a 2P mode can also lead to locally efficient propulsion. The global maximum in efficiency will be based on the kinematics of motion as well as the shape of the fin or device. That is, for one set of kinematics and one propulsor shape a 2P mode may be the most efficient wake mode to utilize, while for another set of kinematics and a different propulsor shape a 2S mode may be the optimally efficient wake mode.
In this respect, Linden & Turner (Reference Linden and Turner2001) and Dabiri (Reference Dabiri2009) have interpreted the structure of wakes generated by swimmers in terms of optimal vortex formation, a concept that was originally proposed in terms of vortex ring formation Gharib, Rambod & Shariff (Reference Gharib, Rambod and Shariff1998). There may well be a connection between this concept and the idea of wake resonance, and this may be a profitable direction to explore in future work.
6 General observations
This may be a good place to end. I have attempted to give a perspective on undulatory and oscillatory swimming mechanisms, from a reasonably fundamental point of view. In doing so I have not addressed much of the other research that is relevant to swimming. I have largely ignored the strong connections to the fluid–structure interaction community, and to the extensive work on non-steady swimming, gust response, or other aggressive manoeuvers. Similarly, I have not highlighted the experiments done on entire fish, either in vivo or on robotic imitations, and passed over many of the computations of the flow field developed by swimming animals. Nor have I addressed the possible benefits of coordinated swimming, or swimming near the sea bottom, fin–fin interactions and many other interesting features of swimming as seen in nature. These are among the topics that I have neglected in order to focus on more basic theory and modelling efforts.
Within this restricted focus, I find that theory and modelling remain central to developing our understanding of swimming, and that observations on the fluid dynamics of simple membranes and foils in unsteady motion can illustrate much of the underlying physics seen. In particular, experiments and theory agree that the more important velocity scale is the characteristic lateral velocity of the tail motion rather than the swimming speed. Although the swimming speed is the most important factor in biology, in that it relates to survival, it is the characteristic lateral velocity of the tail motion that largely governs performance. The primacy of the lateral velocity over the swimming speed as the correct velocity scale erases to a large extent the difference between the results obtained in a tethered mode, compared to those obtained using a free swimming condition.
More particular observations are as follows. First, there is no one-to-one connection between the integrated swimming performance and the details of the wake structure. In this respect, wake resonance theory reveals the possibility of multiple wake structures that exhibit high efficiency, while also helping to identify the conditions for efficient swimming. Second, there are fundamental differences between the study of rigid propulsors, and flexible ones. Animal locomotion usually involves fins with some level of flexibility, passive or active, and the distinction from rigid foils is important to remember since flexibility can be used to improve efficiency. Third, animals propel themselves using a wide variety of actions, which may combine undulatory motion with heaving and pitching motions. Studying heaving or pitching motions by themselves will only give limited insight into actual animal locomotion, and combined heave and pitch motions with an appropriate phase difference seem essential to achieve high performance. Fourth, the effects of Reynolds number are often ignored, based on the assumption that the unsteadiness (characterized by the Strouhal number or the reduced frequency), is the major physical phenomenon, and that viscous effects are secondary. Although we have been guilty of making this assumption at various times ourselves, it turns out that viscous effects play a critical role in determining the thrust and efficiency, and so any assumptions about Reynolds number effects need to be carefully substantiated (Floryan et al. Reference Floryan, Van Buren and Smits2018). Likewise, the shape of the foil influences its drag performance, and we have seen that optimizing the foil shape can result in substantial improvements in performance. In the context of fish swimming, the recent computations by Haibo Dong and his colleagues at UVA have shown that oscillation of the head and front body region in tuna acts to reduce the bending body coefficient of drag by 22 % (private communication). In addition, George Lauder and his colleagues at Harvard are finding very consistent head yaw patterns across species (private communication), and Gemmell et al. (Reference Gemmell, Colin, Costello and Dabiri2015) and Gemmell et al. (Reference Gemmell, Fogerson, Costello, Morgan, Dabiri and Colin2016) have reported that the negative pressures associated with head movements in lampreys can lead to a strong ‘pulling’ contribution to thrust. Hence, there are undoubtedly other factors in addition to reducing drag that contribute to enhancing fish swimming efficiency. Fifth, cautionary remarks should also be made regarding changing aspect ratio, although we are close to a reasonable understanding of these effects. In contrast, we are far from understanding the effects of changing the planform shape. Sixth, almost all laboratory and computational work has been done on foils that are sinusoidally actuated, although non-sinusoidal gaits may be beneficial in some circumstances. In this context, the positive effects of intermittent swimming are now well recognized.
As a final remark, while it is true that the investigation of the mechanics of swimming is inspired by biological organisms, it is also true that such engineering studies have broadened our understanding of the performance of animals. In this area of research, biology and engineering are tightly intertwined, to the benefit of all.
Acknowledgements and a personal note
One of the first opportunities I ever had for snorkelling on a world-class reef was at Coral Bay on the western coast of Australia in about 2002. As part of an excursion to nearby Exmouth, I went swimming with manta rays. I had never seen such fluid grace and precision in any underwater animal before, and upon my return to Princeton I resolved to learn more about the mechanics of manta swimming. I was able to interest a talented senior, R. Clark, in designing and performing some water channel experiments on a robotic imitation of manta pectoral fin motion. This work turned into a masters thesis, and eventually led to the publication of Clark & Smits (Reference Clark and Smits2006). These experiences stimulated in me a new interest in underwater propulsion, using flexible and rigid foils of low aspect ratio. I found an abstract beauty in the formation and development of the wake, and it is a remarkable experience to see the wake generated in real time, and watch the exquisite detail present in the vortex formation and interaction. I was hooked, and so I was determined to find ways to continue this line of research. Fortunately, I became part of a team led by A. Cohen to study lamprey propulsion, which eventually led me back to manta rays, and then on to tuna, through two separate ONR-MURI efforts, both led by H. Bart-Smith. These efforts introduced me to F. Fish and G. Lauder, two of the key biologists who study swimming, who continue to be sources of inspiration to me. The research presented in this article was primarily supported by ONR through Program Manager R. Brizzolara. I would like to thank F. Fish for providing the biological data used in figure 2, and D. Floryan for preparing figure 32. D. Floryan, F. Fish, G. Lauder, T. Van Buren, L. Ding and T. Saxton-Fox gave constructive comments on an earlier draft and suggested some additional reference material, and T. Van Buren helped in preparing the figures. I am very grateful for their assistance, but of course I am solely responsible for any errors or omissions. H. Bart-Smith has been an amazing team leader and colleague, and a steadfast believer in the beautiful example given by manta rays. I am also deeply indebted to A. Cohen and L. Fauci who kept the flame burning on the NSF-NIH lamprey project. Finally, this article is based for the most part on the efforts of my graduate and undergraduate students, postdocs and visiting scholars. Thanks for the fun ride.



















































