Article contents
Multiplicity-Free Schubert Calculus
Published online by Cambridge University Press: 20 November 2018
Abstract
Multiplicity-free algebraic geometry is the study of subvarieties 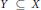 $Y\,\subseteq \,X$ with the “smallest invariants” as witnessed by a multiplicity-free Chow ring decomposition of
$Y\,\subseteq \,X$ with the “smallest invariants” as witnessed by a multiplicity-free Chow ring decomposition of 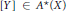 $\left[ Y \right]\,\in \,{{A}^{*}}\left( X \right)$ into a predetermined linear basis.
$\left[ Y \right]\,\in \,{{A}^{*}}\left( X \right)$ into a predetermined linear basis.
This paper concerns the case of Richardson subvarieties of the Grassmannian in terms of the Schubert basis. We give a nonrecursive combinatorial classification of multiplicity-free Richardson varieties, i.e., we classify multiplicity-free products of Schubert classes. This answers a question of W. Fulton.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2010
References
- 8
- Cited by


