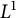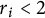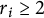Global existence of a weak solution for a reaction–diffusion system in a porous medium with membrane conditions and mass control

Abstract

In this paper, we prove the global exstence of weak solutions for a porous medium dynamics of m species moving between two domains separated by a zero-thickness membrane. On this membrane, Kedem–Katchalsky conditions are considered, and the study is characterized by natural structural conditions applied to the nonlinear reactive terms. The global existence is established under the assumption that these reactive terms are bounded in $L^1$. This problem has already been analyzed in the linear diffusion case by Ciavolella and Perthame in Ciavolella and Perthame (2021, Journal of Evolution Equations 21, 1513–1540). The present work constitutes an extension for nonlinear diffusion, particularly of the porous medium type, in the form $\partial _t v_i - \Delta v_i^{r_i} = R_i$, for an exponent $r_i < 2$. The case $r_i \geq 2$ remains an open problem. This paper is an adaptation of the ideas from Ciavolella and Perthame (2021, Journal of Evolution Equations 21, 1513–1540), with new strategies to overcome the appearance of nonlinearity and degeneracy in the diffusion term.

1 Introduction

The study of diffusion in porous medium is of great importance in modeling transport phenomena that are ubiquitous in fields such as hydrology, geology, biology, and materials engineering. A particularly relevant phenomenon is osmosis, the process by which a solvent or species diffuses through a semi-permeable membrane. The modeling of such a process, generally governed by reaction–diffusion systems, often integrates so-called Kedem–Katchalsky conditions [16]. For instance, in [10], the authors studied such a model for linear diffusion.

In this paper, we consider a nonlinear reaction–diffusion model of the porous medium type, incorporating a Kedem–Katchalsky condition, given by the following system:

(1.1) $$ \begin{align} \begin{cases} \text{for} \ i=1,\ldots, m,\\ \partial_tv_i - \Delta \varphi_i( v_i )= R_i(v_1, \ldots, v_m ) & \text{in} \hspace{1cm} Q_T:=(0,T)\times \Omega, \\ v_i =0 & \text{in} \hspace{1cm} \Sigma_{T}:=(0,T)\times (\Gamma_1 \cup \Gamma_2), \\ \partial_{\nu_1} {{}^1\varphi_i( v_i)}=\partial_{\nu_1} {{}^2\varphi_i( v_i)}= k_i({}^2\varphi_i( v_i) - {}^1\varphi_i( v_i)) & \text{in} \hspace{1cm}\Sigma_{T,\Gamma}:=(0,T)\times \Gamma,\\ v_i(0,x)= v_{0,i}(x) \geq 0 & \text{in} \hspace{1cm} \Omega, \end{cases} \end{align} $$

where

$\blacklozenge $ $\Omega $ is a bounded open spatial domain of $\mathbb {R}^{d}$ , $d \geq 2$ , and $\partial \Omega $ denotes its boundary supposed smooth,

$\blacklozenge $ $\Omega _1$ and $\Omega _2$ are open and bounded spatial subdomains of $\Omega $ , with respective boundaries $\partial \Omega _1$ and $\partial \Omega _2$ which are assumed to be sufficiently regular. And let us put

$$ \begin{align*} \Omega= \Omega_1 \cup \Omega_2, \hspace{0.5cm} \Gamma= \partial \Omega_1 \cap \partial \Omega_2, \hspace{0.5cm} \Gamma_1= \partial \Omega_1 \setminus \Gamma, \hspace{0.5cm} \Gamma_2= \partial \Omega_2 \setminus \Gamma,\\[-30pt] \end{align*} $$

$\blacklozenge $ We also denote by $\nu _1$ and $\nu _2$ the exterior normals to $ \Omega _1$ and $ \Omega _2$ , respectively.

$\blacklozenge $ $\varphi _i(v_i)=D_i v_{i}^{r_i}$ , $D_i>0, r_i >0$ , $i=1, \ldots ,m$ are continuous increasing functions from $[0,+\infty )$ into $[0,+\infty )$ with $\varphi _i(0) = 0$ and the nonlinearities $R_i$ are regular functions satisfying the following two main properties:

$\ast $ (P): the nonnegativity of the solutions is preserved for all time;

$\ast $ (M): the total mass of the components is controlled at all times (sometimes even exactly conserved). We will come back to this later for more details.

$\blacklozenge $ We designate the density of each species i $(i=1, \ldots , m)$ by

$$ \begin{align*} v_i= \begin{cases} {}^1v_i, \hspace{0,2cm} \text{if species } i \text{ live in } \Omega_1,\\ {}^2v_i, \hspace{0,2cm} \text{if species } i \text{ live in }\Omega_2 \end{cases} \hspace{0,25cm} \!\!\!\text{such as} \hspace{0,25cm} \varphi_i(v_i)=\begin{cases} \varphi_i({}^1v_i):= \ {}^1\varphi_i(v_i), \hspace{0,2cm} \text{if species } i \text{ live in } \Omega_1,\\ \varphi_i({}^2v_i):= \ {}^2\varphi_i(v_i), \hspace{0,2cm} \text{if species } i \text{ live in } \Omega_2. \end{cases} \end{align*} $$

This choice is justified by the fact that each of the species i $(i=1,\ldots , m)$ lives only in one of the separate domains $\Omega _1$ or $\Omega _2$ and can move from one domain to the other across the permeable transverse membrane $\Gamma $ . There is a jump of species $v_i, \ i=1, \ldots , m$ across the $\Gamma $ membrane which we designate by

$$ \begin{align*} {}^2v_i- {}^1v_i :=[\![v_i]\!]. \end{align*} $$

To be more precise, for $x \in \Gamma $ and for $i=1,\ldots ,m $ , the trace in the sense of Sobolev allows us to pose

$$ \begin{align*} ^1v_i(x)=\lim\limits_{h \to 0^-}v_i(x+h\nu_1(x)) \hspace{0,5cm} and \hspace{0,5cm} {}^2v_i(x)=\lim\limits_{h \to 0^-}v_i(x+h\nu_2(x)). \end{align*} $$

In this paper, we analyze a nonlinear reaction–diffusion model of the porous medium type with membrane conditions called Kedem–Katchalsky conditions [10]. Our main goal is to prove the global existence in time of a weak solution for the system (1.1) under an a priori estimate $L^1$ with $r_i \in \left ( (d-2)^{+}/d; 2 \right )$ , $i=1, \ldots ,m$ . We exploit here the “ $L^1$ ” framework offered by the properties (M) and (P), by the fact that just as in the semilinear case, the operator $v_i \mapsto \partial _t v_i - D_iv_i^{r_i}$ has favorable compactness properties in $L^1$ when $r_i> \dfrac {(d-2)^{+}}{d}$ . Concerning the restriction $r_i < 2$ , we will detail its natural appearance in the rest of the work, more precisely in the proof of Lemma 3.5. Recently, Ciavolella and Perthame in [10] studied a similar model for linear diffusion ( $r_i=1$ ). The authors proved the global existence of weak solutions for $L^1$ data, by adapting to membrane conditions an $L^1$ theory for reaction–diffusion systems initiated by M. Pierre and his collaborators (see [3, 5, 19, 20, 28]).

We aim to extend the main results on this global existence of weak solutions from the semilinear case [10] to the case where the $\varphi _i$ are nonlinear, particularly of the porous medium type, i.e., $\varphi _i(v_i) = D_i v_i^{r_i}$ , $r_i \geq 1$ , with Kedem–Katchalsky conditions. Two principles are fundamental for this:

1. – the conservation of mass, which leads to the continuity of the density flux,

2. – the dissipation principle such that the $L^2$ -norm of the solution decreases over time.

From these properties, it follows that the density flux is proportional to the jump $[\![ v_i ]\!]$ across the membrane with a proportionality coefficient $k_i \geq 0$ , $i=1, \ldots , m$ , representing the permeability constants of the membrane $\Gamma $ for each species density $v_i, \ i = 1, \ldots , m$ .

Over the last two decades, the study of biological models with membrane boundary problems describing diffusion phenomena has attracted many authors at various scales (see [4, 6, 7, 9, 13, 21, 31, 34]).

The existence of bounded regular solutions on the interval $(0,+\infty )$ can be found in several references, notably [8, 11, 14, 15, 17, 24–26, 30], as well as in many other articles listed in the survey [28] or in the book [32]. However, it is well known that solutions can blow up in $L^{\infty }(\Omega )$ -norm in finite time, as demonstrated in [29], where explicit finite-time blowups in $L^{\infty }(\Omega )$ -norm are presented. Thus, even in the semilinear or nonlinear case, it is necessary to deal with weak solutions to ensure global existence in time.

Our paper is structured into three distinct sections, each making a specific contribution to our research. Section 1 presents the context and issues of the study. Section 2 is devoted to the presentation of our main result, preceded by a preliminary phase which establishes the foundations and hypotheses necessary to prove the global existence of a weak solution for equation (1.1). Section 3 demonstrates our main result by means of an approximation model, applying crucial estimates, and proving the existence of a weak solution in two steps: first an supersolution, then a subsolution. This structure allows for a clear and logical presentation, providing a comprehensive overview of our contribution to this area of research.

2 Preliminaries and main results

2.1 Preliminaries and notations

The purpose of this part is to introduce some notations and recall some basic mathematical results. we denote by $\textbf {H}^1$ the Hilbert space of functions defined by

$$ \begin{align*} \textbf{H}^1= \left\lbrace u \in H^1(\Omega_1)\times H^1(\Omega_2), u=0 \ in \ \Gamma_1 \ \textrm{and} \ \Gamma_2 \right\rbrace. \end{align*} $$

We endow it with the norm

$$ \begin{align*} \Vert u \Vert_{\textbf{H}^1}=\left( \Vert u \Vert_{H^1(\Omega_1)}^2 + \Vert u \Vert_{H^1(\Omega_2)}^2 \right)^{\frac{1}{2}}. \end{align*} $$

We designate $(\cdot , \cdot )$ as the inner product in $\textbf {H}^1$ and $\left \langle \cdot , \cdot \right \rangle $ denote the duality bracket of $\textbf {H}^1$ with its dual space $(\textbf {H}^{1})^{\star }$ .

2.2 Assumptions

For further work in this paper, we formulate the following hypotheses:

For $i= 1,\ldots , m$ , we assume that

(2.1) $$ \begin{align} k_1= \cdots=k_m=k, \hspace{1cm} \varphi_i(v_i)=D_i v_i^{r_i},\ \dfrac{(d-2)^{+}}{d} <r_i < 2. \end{align} $$

For $i= 1,\ldots , m$ , $R_i:Q_T \times [0,+\infty )^m \rightarrow \mathbb {R}$ be such as

(2.2) $$ \begin{align} &\textbf{Regularity}: \nonumber\\ &\begin{cases} R_i \ \text{is measurable},\\ \forall T>0, R_i(\cdot,\cdot, 0) \in L^1(Q_T),\\ \exists K : [0,+\infty)\rightarrow [0,+\infty) \ \text{nondecreasing such that: }\\ \vert R_i(x,t, v) - R_i(x,t, \tilde{v}) \vert \leq K(M) \sum \limits_{j=1}^{m} \vert v_j - \tilde{v}_j \vert \\ \text{for all} \ M>0 \ \text{for all} \ v, \tilde{v} \in (0,M)^m \ \text{and a.e.} \ (x,t)\in Q_T. \end{cases} \end{align} $$

We assume that the nonlinearities $R_i$ satisfy the properties:

(2.3) $$ \begin{align} &\hspace{-12pt}\textbf{Quasi-positivity:} \nonumber\\ &\hspace{-12pt}\textbf{(P):} \begin{cases} R_i(t,x,v_1, \ldots, v_{i-1},0,v_{i+1}, \ldots,v_m) \geq 0 \\ \text{for all} \ v=(v_1,\ldots,v_m)\in [0,+\infty)^m \ \mathrm{a.e.} \ (t,x)\in Q_T. \end{cases} \end{align} $$

(2.4) $$ \begin{align} &\quad\ \kern1.5pt\textbf{Control of mass:} \nonumber\\ &\kern1.5pt\ \quad \textbf{(M):} \begin{cases} \text{there exists} \ (\xi_1, \ldots,\xi_m) \in (0, +\infty)^m \ \text{such as} \\ \forall v=(v_1,\cdot,v_m)\in [0,+\infty)^m, \ \text{ for a.e.} \ (t,x)\in Q_T, \ \sum \limits_{j=1}^{m} \xi_jR_j(x,t,v) \leq 0. \end{cases} \end{align} $$

(2.5) $$ \begin{align} &\hspace{-63pt}\textbf{Sub-quadratic growth:} \nonumber\\ &\hspace{-63pt}\begin{cases} \forall i = 1, \ldots, m, \forall v= (v_1,\cdot,v_m)\in [0,+\infty)^m, \\ \vert R_i(v) \vert \leq C\left( 1 + \sum \limits_{j=1}^{m} v_j^{r_i+1} \right). \end{cases} \end{align} $$

Remark 1 Note that all our given results extend immediately if (M) is replaced by

(2.6) $$ \begin{align} \textbf{(M')} \begin{cases} \forall \ v=(v_1,\ldots,v_m)\in [0,+\infty)^m, \text{for a.e.} \ (x,t)\in Q_T, \ \sum \limits_{j=1}^{m} R_j(x,t,v) \leq C\sum \limits_{j=1}^{m} v_j + h(x,t), \\ \text{for some} \ C>0 \, h \in L^1_{loc}([0,+\infty); L^2(\Omega)^+). \end{cases} \end{align} $$

The properties (P) and (M) or (M’) exist naturally in applications. In fact, evolutionary reaction–diffusion systems are mathematical models for evolutionary phenomena undergoing both spatial diffusion and (bio)chemical reactions. In these models, the unknown functions are generally densities, concentrations, and temperatures, so their nonnegativity is required. In addition, it is often necessary to control the total mass, sometimes even the preservation of the total mass is naturally guaranteed by the model. Interest in these models has grown recently, particularly for applications in biology, ecology, and population dynamics. We refer to [29] for examples of reaction–diffusion systems with properties (P) and (M) or (M’).

We now present the notion of solution and also the main result that is the subject of our mathematical analysis in this paper.

2.3 Main result

We define our space of test functions as

$$ \begin{align*} W_T& =\left\lbrace ({}^1 \Psi,^2 \Psi)\in C^{\infty}([0,T]\times \overline{\Omega_1}) \times C^{\infty} ([0,T]\times \overline{\Omega_2}), \Psi \geq 0 ,\right. \\ &\left. \hspace{1cm} \Psi(\cdot,T)=0, \Psi =0 \ in \ \Sigma_T, \nabla {}^1\Psi.\nu_1 = \nabla {}^2\Psi.\nu_1 =k_i({}^2\Psi - {}^1\Psi) \ in \ [0,T]\times \Gamma \right\rbrace, \end{align*} $$

where $\Psi = \begin {cases} {}^1\Psi , \hspace {0,5cm} in \ \Omega _1, \\ {}^2\Psi , \hspace {0,5cm} in \ \Omega _2\end {cases}. $ We now introduce the notion of weak solution of problem (1.1) and also the existence and regularity result of this solution.

Definition 1 Given $v_{0,i}\in L^1(\Omega )\cap (\textbf {H}^1)^{\star }, v_{0,i} \geq 0$ , $i = 1,\ldots ,m$ , a global weak solution of system (1.1) is a nonnegative function $\textbf {v}=(v_1,\ldots , v_m)$ such that for all $T> 0$ and $i=1, \ldots , m$ , $v_i \in C([0,T],L^1(\Omega )),\ \varphi _i(v_i) \in L^1(0,T,W^{1,1}), \ R_i(v) \in L^1(Q_T)$ , and

(2.7) $$ \begin{align} - \int_{\Omega} v_i(\cdot,0)\Psi(\cdot,0)dx - \int_{0}^{T}\int_{\Omega} v_i\partial_t\Psi+ \varphi_i(v_i).\Delta\Psi dxdt = \int_{0}^{T}\int_{\Omega} R_i(v)\Psi dxdt \end{align} $$

for all $\Psi \in W_T$ .

Theorem 1 Assume that (2.1)–(2.3) and (2.6) hold and $k_i=k, i=1,\ldots ,m$ . Assume that $L^1$ -estimate (3.6) holds. Then, for all $v_0=(v_{0,1}, \ldots , v_{0,n})$ , such as $v_0 \in (L^1(\Omega )\cap (\textbf {H}^1)^{\star })^m$ , $v_0 \geq 0$ , the system (1.1) has a nonnegative global weak solution in the sense of Definition 1.

3 Proof of the existence result

3.1 The approximate reaction–diffusion system

In this subsection, we introduce an approximation of the system (1.1).

We first approximate the initial data and the reaction terms as follows:

(3.1) $$ \begin{align} v^n_{0,i}:=\inf\left\lbrace v_{0,i},n \right\rbrace \hspace{1cm} \text{and} \hspace{1cm} R^{n }_i :=\dfrac{R_i}{1 + \frac{1}{n }\sum_{1\leq j \leq m} \vert R_j \vert}. \end{align} $$

For each fixed n, $v^{n }_{0,i}(x)\in L^{\infty }(\Omega )$ , $i=1,\ldots ,m$ , and converges to $v_{i,0}$ in $L^1(\Omega )\cap (\textbf {H}^1)^{\star }$ . We consider the following regularised system:

(3.2) $$ \begin{align} &\begin{cases} \textrm{for } i=1,\ldots,m,\\ \textrm{for all } T> 0, v_i^n \in L^{\infty}(Q_T)^{+}, \varphi_i(v_i^n) \in L^{2}(0,T;H^{1}(\Omega)), \\ \partial_tv^{n }_i - \Delta \varphi_i( v^{n }_i )= R^{n }_i(v^{n }_1, \ldots, v^{n }_m ) & \text{in} \hspace{1cm} Q_T:=(0,T)\times \Omega, \\ v^{n }_i =0 & \text{in} \hspace{1cm} \Sigma_{T}:=(0,T)\times (\Gamma_1 \cup \Gamma_2), \\ \partial_{\nu_1} {{}^1\varphi_i( v^{n }_i)}=\partial_{\nu_1} {{}^2\varphi_i( v^{n }_i)}= k_i({}^2\varphi_i( v^{n }_i) - {}^1\varphi_i( v^{n }_i)) & \text{in} \hspace{1cm} \Sigma_{T,\Gamma}:=(0,T)\times \Gamma,\\ v^{n }_i(0,x)= v^{n }_{0,i}(x) \geq 0 & \text{in} \hspace{1cm} \Omega, \end{cases} \end{align} $$

where the approximate nonlinearities $R_i^n$ are essentially “truncations” of the $R_i$ ’s. More precisely, we will assume that $R^n_i$ is locally Lipschitz continuous and satisfies (2.2) with $K(\cdot )$ independent of n, and (2.3)–(2.5) with h independent of n, and is in $L^{\infty }(Q_T \times \mathbb {R}^{m})$ . Moreover, thanks to our choice, we have $\Vert R^n_i \Vert _{L^{\infty }} \leq n$ for each fixed n. Therefore, the approximate system (3.2) has a nonnegative bounded global solution (see, e.g., [20, Lemma 2.3] and [18] or [35] for more details). Let us denote

(3.3) $$ \begin{align} \epsilon^n_M:=\max \limits_{1 \leq i \leq n} \sup \limits_{v \in [0,M]^m}\vert R^n_i(v) - R_i(v) \vert, \end{align} $$

where $ v=(v_1,\ldots ,v_m)$ . Then, we check that

(3.4) $$ \begin{align} \epsilon^n_M \leq \dfrac{C_M m}{n} \textrm{ and } \epsilon^n_M \longrightarrow 0 \ \textrm{in } L^1(Q_T) \text{ and a.e. in } Q_T \textrm{ as } n\rightarrow +\infty. \end{align} $$

For $i=1,\ldots ,m$ , $v^{n }_{0,i}(x)\in L^{\infty }(\Omega )$ and converges to $v_{i,0}$ in $L^1(\Omega )\cap (\textbf {H}^1)^{\star }$ .

3.2 The key estimate

Lemma 3.1 Assume that, for $1 \leq i \leq m$ , $v_{i,0} \in L^{1}(\Omega )\cap (\textbf {H}^1)^{\star }$ and $h \in L^1_{loc}([0,+\infty ); L^2(\Omega ))$ under the assumption (2.6). Then, for all nonnegative regular functions $v_i$ solution of (3.2) with $k_i=k, i= 1,\ldots , m$ , there exists $C(T)>0$ such that

(3.5) $$ \begin{align} \Vert v^n_i \Vert_{L^{r_i+1}(Q_T)} \leq C(T)\left( 1+ \Vert v_{i,0}^n \Vert_{(\textbf{H}^1)^{\star} } \right). \end{align} $$

Moreover, with the assumption (2.5), the $R_i^{n}(v^n)$ are uniformly bounded in $L^1(Q_T)$ , more precisely, for all $T>0$ , there exists a constant $C'> 0$ independent of n such that

(3.6) $$ \begin{align} \Vert R_i^{n}(v^n) \Vert_{L^1(Q_T)} \leq C'. \end{align} $$

Proof By summing the m equations of the regularized system (3.2) and then using (2.6) of Remark 1, we obtain

(3.7) $$ \begin{align} \partial_t \left( \sum \limits_{i=1}^{m} v_i^n \right) - \Delta \left( \sum \limits_{i=1}^{m} \varphi_i( v_i^n) \right) \leq C \sum \limits_{i=1}^{m} v_i^n +h. \end{align} $$

By multiplying this inequality by $e^{-Ct}$ , and observing that

$ e^{-Ct} \sum \limits _{i=1}^{m} v_i^n = \partial _t \left ( e^{-Ct} \sum \limits _{i=1}^{m} v_i^n \right ) + C \sum \limits _{i=1}^{m} v_i^n $ , we obtain

(3.8) $$ \begin{align} \partial_t \left( e^{-Ct} \sum \limits_{i=1}^{m} v_i^n \right) - \Delta \left( e^{-Ct} \sum \limits_{i=1}^{m} \varphi_i( v_i^n) \right) \leq e^{-Ct}h \leq h. \end{align} $$

By integrating from 0 to t, we obtain

(3.9) $$ \begin{align} e^{-Ct} \sum \limits_{i=1}^{m} v_i^n - \Delta \left(\int_0^t e^{-Cs} \sum \limits_{i=1}^{m} \varphi_i( v_i^n(s,\cdot)ds \right)\leq \sum \limits_{i=1}^{m} v_{i,0}^n + \int_0^t h(s,\cdot)ds. \end{align} $$

Let us set $\widehat {U}(t)= \displaystyle e^{-Ct} \sum \limits _{i=1}^{m} v_i^n(t,\cdot )$ and $\displaystyle \widehat {V}(t)= \int _0^t e^{-Cs} \sum \limits _{i=1}^{m} \varphi _i( v_i^n(s,\cdot )ds $ , then we obtain the following problem:

(3.10) $$ \begin{align} \begin{cases} \displaystyle \widehat{U} - \Delta \widehat{V} \leq \widehat{U}_0 + \int_0^t h(s,\cdot)ds, & \text { in } Q_{T},\\ \widehat{V}=0, & \text { in } \Sigma_{T}, \\ \partial_{\boldsymbol{\nu}_{1}} {{}^{1}\widehat{V}}=\partial_{\boldsymbol{\nu}_{1}} {{}^{2}\widehat{V}}=k\left({}^{2}\widehat{V}-^{1}\widehat{V}\right), & \text { in } \Sigma_{T, \Gamma}, \\ \widehat{V}(0, x)= 0, & \text { in } \Omega,\end{cases} \end{align} $$

multiplying the inequation of (3.10) by $\partial _t \widehat {V}\geq 0 $ , then integrating over $Q_T$ , we get

(3.11) $$ \begin{align} \int_{Q_T}(\partial_t \widehat{V}) \widehat{U} - \int_{Q_T}(\partial_t \widehat{V}) \Delta\widehat{V} \leq \int_{Q_T}(\partial_t \widehat{V})\left[\widehat{U}_0 + \int_0^t h(s,\cdot)ds\right], \end{align} $$

where $\widehat {U}_0:= \widehat {U}(0)= \displaystyle \sum \limits _{i=1}^{m} v_{i,0}^n $ . Integrating by parts and majorating the right-hand side, we get

(3.12) $$ \begin{align} \int_{Q_T}(\partial_t \widehat{V}) \widehat{U} + \int_{Q_T}\nabla (\partial_t \widehat{V}) \nabla\widehat{V} - \int_0^T\int_{\partial \Omega }\partial_{\nu} \widehat{V} \partial_t \widehat{V} \leq \int_{Q_T} (\partial_t \widehat{V})\left[\widehat{U}_0 + \int_0^T h(s,\cdot)ds\right]. \end{align} $$

Now, we can see that

$$ \begin{align*} \int_0^T \int_{\partial \Omega} \partial_\nu \widehat{V} \partial_t \widehat{V} &=\int_0^T \int_{\Gamma} \partial_{\nu_1} {{}^1\widehat{V}}\partial_t {{}^1\widehat{V}} + \int_0^T \int_{\Gamma} \partial_{\nu_2} {{}^2\widehat{V}}\partial_t {{}^2\widehat{V}}\\ &= - \int_0^T\int_{\Gamma} k([\![\widehat{V}]\!]) \partial_t([\![\widehat{V}]\!])\\ &= -\frac{k}{2}\int_0^T \frac{d}{d t} \int_{\Gamma}([\![\widehat{V}]\!])^2\\ &= -\frac{k}{2} \int_{\Gamma}([\![\widehat{V}(T)]\!])^2,\end{align*} $$

since $ \widehat {V}(0)=0$ . So, from (3.12), it follows that

$$ \begin{align*} \int_{Q_T}(\partial_t \widehat{V}) \widehat{U} + \dfrac{1}{2}\int_0^T \dfrac{d}{dt} \int_{\Omega} \vert \nabla\widehat{V} \vert^2 +\frac{k}{2} \int_{\Gamma}([\![\widehat{V}(T)]\!])^2 \leq& \int_{\Omega}\left[\widehat{U}_0 + \int_0^T h(s,\cdot)ds\right]\widehat{V}(T),\\ \int_{Q_T}(\partial_t \widehat{V}) \widehat{U} + \dfrac{1}{2}\int_{\Omega} \vert \nabla\widehat{V}(T) \vert^2 +\frac{k}{2} \int_{\Gamma}([\![\widehat{V}(T)]\!])^2 \leq& \int_{\Omega}\left[\widehat{U}_0 + \int_0^T h(s,\cdot)ds\right]\widehat{V}(T). \end{align*} $$

Therefore,

(3.13) $$ \begin{align} \int_{Q_T}(\partial_t \widehat{V}) \widehat{U} \leq \int_{\Omega}\left[\widehat{U}_0 + \int_0^T h(s,\cdot)ds\right]\widehat{V}(T). \end{align} $$

Furthermore, the second term of the right-hand side of the above inequality is bounded for all $T>0$ . To see this, we introduce, thanks to the Lax–Milgram theorem, the solution of

(3.14) $$ \begin{align} \begin{cases} W \in \textbf{H}^1, \quad W \geq 0, \\ - \displaystyle \Delta W = \widehat{U}_0 + \int_0^T h(s,\cdot)ds, & \text { in } Q_{T},\\ W=0, & \text { in } \Sigma_T, \\ \partial_{\boldsymbol{\nu}_{1}} {{}^{1}\widehat{W}}=\partial_{\boldsymbol{\nu}_{1}} {{}^{2}\widehat{W}}=k\left({}^{2}\widehat{W}-^{1}\widehat{W}\right), & \text { in } \Sigma_{T, \Gamma},\\ W(0,x)=W_0(x)\geq 0 & \text { in } \Omega. \end{cases} \end{align} $$

Consider the inequality from (3.10) at time $t=T$ . Multiplying by W and using the growth of the integral, we integrate over $\Omega $ to obtain

(3.15) $$ \begin{align} \int_{\Omega} \widehat{U}(T)W - \int_{\Omega} \Delta \widehat{V}(T)W \leq \int_{\Omega} \widehat{U}_{0} W + \int_{\Omega} W \int_{0}^{T}h(s,\cdot)ds := -\int_{\Omega}W \Delta W. \end{align} $$

Using two integrations by parts on the second term of the left-hand side, and exploiting equation (3.14), we observe that

(3.16) $$ \begin{align} \int_{\Omega} \Delta \widehat{V}(T)W = \int_{\Omega} V(T)\Delta W := -\int_{\Omega} \left[ \widehat{U}_0 + \int_0^T h(s,\cdot)ds \right]\widehat{V}(T), \end{align} $$

and with an integration by parts on the right-hand side term, we have

(3.17) $$ \begin{align} -\int_{\Omega} W\Delta W = \left( \int_{\Gamma} k [\![W]\!]^2 + \int_{\Omega} |\nabla W|^2 \right). \end{align} $$

Taking W as the test function in the weak formulation of (3.14), it follows that

(3.18) $$ \begin{align} \left( \int_{\Gamma} k [\![W]\!]^2 + \int_{\Omega} |\nabla W|^2 \right)&:= \int_{\Omega} \left( \widehat{U}_0 + \int_{0}^{T}h(s,\cdot)ds \right)W \nonumber\\ &\leq \|W\|^2_{\textbf{H}^1} \left( \|\widehat{U}_0 \|^2_{(\textbf{H}^1)^{\star} } + \left \| \int_{0}^{T} h(s,\cdot)ds \right\|^2_{L^2(\Omega)} \right) \nonumber \\ &\leq \|W\|^2_{\textbf{H}^1} \left( \sum \limits_{i=1}^{m} \|v^n_{i,0} \|^2_{(\textbf{H}^1)^{\star} } + \left \| \int_{0}^{T} h(s,\cdot)ds \right\|^2_{L^2(\Omega)} \right) \nonumber \\ &\leq \max\left\lbrace 1, \left \| \int_{0}^{T} h(s,\cdot)ds \right\|^2_{L^2(\Omega)} \right\rbrace \|W\|^2_{\textbf{H}^1} \left( 1+ \sum \limits_{i=1}^{m} \|v^n_{i,0} \|^2_{(\textbf{H}^1)^{\star} } \right) \nonumber \\ &\leq C \left( 1+ \sum \limits_{i=1}^{m} \|v^n_{i,0} \|^2_{(\textbf{H}^1)^{\star} } \right), \end{align} $$

where $C= \max \left \lbrace 1, \left \| \int _{0}^{T} h(s,\cdot )ds \right \|^2_{L^2(\Omega )} \right \rbrace \|W\|^2_{\textbf {H}^1} $ . From (3.15)–(3.18), we obtain

$$ \begin{align*} \int_{\Omega} \widehat{U}(T)W + \int_{\Omega} \left[ \widehat{U}_0 + \int_0^T h(s,\cdot)ds \right]\widehat{V}(T) \leq C \left( 1+ \sum \limits_{i=1}^{m} \|v^n_{i,0} \|^2_{(\textbf{H}^1)^{\star} } \right). \end{align*} $$

Thanks to the positivity of W and $v^n_{i}$ , it follows that

(3.19) $$ \begin{align} \int_{\Omega} \left[ \widehat{U}_0 + \int_0^T h(s,\cdot)ds \right]\widehat{V}(T) \leq C \left( 1+ \sum \limits_{i=1}^{m} \|v^n_{i,0} \|^2_{(\textbf{H}^1)^{\star} } \right). \end{align} $$

From (3.13) and (3.19), it comes that

$$ \begin{align*} \int_{Q_T}(\partial_t \widehat{V}) \widehat{U} \leq C \left( 1+ \sum \limits_{i=1}^{m} \|v^n_{i,0} \|^2_{(\textbf{H}^1)^{\star} } \right), \end{align*} $$

which can be rewritten as

$$ \begin{align*} \int_{Q_T} \sum\limits_{i=1}^{m} v_{i}^{n}(t,\cdot) \sum\limits_{i=1}^{m} \varphi_{i}(v_{i}^{n}(t,\cdot))\leq {e}^{2CT}C \left( 1+ \sum \limits_{i=1}^{m} \|v^n_{i,0} \|^2_{(\textbf{H}^1)^{\star} } \right). \end{align*} $$

Finally, from (3.1) and the fact that $\varphi _i(v_i)=D_i v_i^{r_i}$ , it follows that

$$ \begin{align*} \min \limits_{i} \left\lbrace D_i \right\rbrace \sum\limits_{i=1}^{m} \int_{Q_T}\vert v_i^n\vert^{r_i+1} \leq {e}^{2CT}C \left( 1+ \sum \limits_{i=1}^{m} \|v^n_{i,0} \|^2_{(\textbf{H}^1)^{\star} } \right) \leq {e}^{2CT}C \sum \limits_{i=1}^{m} \left( 1+ \|v^n_{i,0} \|^2_{(\textbf{H}^1)^{\star} } \right). \end{align*} $$

This implies

(3.20) $$ \begin{align} \int_{Q_T}\vert v_i^n\vert^{r_i+1} \leq \textbf{C} \left( 1+ \|v^n_{i,0} \|^2_{(\textbf{H}^1)^{\star} } \right), \quad \text{ where } \textbf{C}=\dfrac{{e}^{2CT}C}{\min \limits_{i} \left\lbrace D_i \right\rbrace}. \end{align} $$

This proves (3.5).

Noting that $\vert R_i^{n} \vert \leq \vert R_i \vert $ , then from (2.5) and (3.20), we have

(3.21) $$ \begin{align} \int_{Q_T}\vert R_i^{n}(\textbf{v}^n)\vert \leq C \left[ T\vert \Omega \vert + \sum \limits_{i=1}^{m} \int_{Q_T} \vert v_i^n \vert^{r_i+1} \right] \leq C(T, \Omega,\textbf{C},m) \left( 1+ \sum \limits_{i=1}^{m} \Vert v_{i,0}^n \Vert^2_{(\textbf{H}^1)^{\star} } \right). \end{align} $$

Hence, $\left \lbrace R_i^n(v^n)\right \rbrace $ is bounded in $L^1(Q_T)$ . This proves (3.6) and completes the proof of Lemma 3.1.

Let us now recall the main compactness properties of the solutions of (1.1). We start with the following compactness lemma (see [2, 3, 5]; for the compactness of the trace, we use the continuity of the trace operator from $W^{1,1}$ into $L^{1}(\partial \Omega )$ ). Let $(w_0,F)\in L^1(\Omega )\times L^1(Q_T)$ . We consider w the solution of the problem in dimension $d \geq 2$

(3.22) $$ \begin{align} \begin{cases} \partial_tw - D\Delta w^{\alpha} = F & \text{in} \hspace{1cm} Q_T:=(0,T)\times \Omega, \\ w =0 & \text{in} \hspace{1cm} \Sigma_{T}:=(0,T)\times (\Gamma_1 \cup \Gamma_2), \\ \partial_{\nu_1} {{}^1\varphi(w)}=\partial_{\nu_1} {{}^2\varphi(w)}= k_i({}^2\varphi(w) - {{}^1\varphi(w)}) & \text{in} \hspace{1cm} \Sigma_{T,\Gamma}:=(0,T)\times \Gamma,\\ w(0,x)= w_{0}(x) \geq 0 & \text{in} \hspace{1cm} \Omega. \end{cases} \end{align} $$

• • The mapping $(w_0,f) \mapsto w$ is compact from $ L^1(\Omega )\times L^1(Q_T)$ to $L^1(Q_T)$ for all $\alpha> \dfrac {(d-2)^{+}}{d}$ .

• • The trace mapping $(w_0,f) \mapsto w_{\vert \Gamma } \in L^{1}\left ( \Gamma \right )$ is also compact.

Lemma 3.2 (see [22])

Let $(w_0,F)\in L^1(\Omega )\times L^1(Q_T)$ and $\alpha> \dfrac {(d-2)^{+}}{d}$ . We consider w the solution of the problem (3.22) in dimension $d \geq 2$ . Then,

(3.23) $$ \begin{align} \int_{Q_T} \vert w \vert^{\alpha \gamma} &\leq C \text{ for } 0<\gamma< \dfrac{2+\alpha d}{\alpha d}, \end{align} $$

(3.24) $$ \begin{align} \int_{Q_T} \vert \nabla w^{\alpha} \vert^{\beta} &\leq C \text{ for } 0<\beta< 1+ \dfrac{1}{1+ \alpha d}. \end{align} $$

The constant C depends only on $\vert Q_T \vert $ , $\Vert w_0 \Vert _{L^1(\Omega )},\Vert F \Vert _{L^1(Q_T)}, \gamma , \beta , \alpha \textrm { and of dimension } d $ .

Proof For a proof, see Lukari, for the case $\alpha> 1$ and [23, Lemma 3.5] for the case $\dfrac {(d - 2)^{+}}{d} < \alpha < 1$ . In these two references, the proof is given with zero initial data, but with right-hand side a bounded measure. We may use the measure $\delta _{t=0}\otimes w_0 dx$ include the case of initial data $w_0$ . We may also use the results in [1, Theorem 2.9]. The estimate in the nondegenerate case may be obtained in a similar way.

In the rest of the demonstrations, we will need to use Vitali’s lemma, which we recall below.

Lemma 3.3 (see [12, 33])

Let $(E,\mu )$ be a measured space such that $\mu (E)< +\infty $ , let $1 \leq p < +\infty $ , and let $\left \lbrace f_n\right \rbrace _n \subset L^p(E)$ such that $f_n \to f$ a.e. If $\left \lbrace f{{}^p}_n\right \rbrace _n$ is uniformly integrate over E, then $f \in L^p(E)$ and $f_n \to f$ in $L^p(E)$ .

3.3 Existence of global weak supersolution

Thanks to Lemma 3.1, we know that the reaction term $R^n$ is bounded in $L^1(Q_T)$ . Thus, we can assert the existence of a supersolution of the system (1.1) through the following theorem.

Theorem 2 (Existence of a supersolution)

Assume that the $L^1$ -estimate (3.6) holds for the solution $v^n$ of (3.2) with $\dfrac {(d-2)^{+}}{d}<r_i<2 $ . Assume that, for $1 \leq i \leq m$ , $R_i$ satisfy (2.5). Let $ v^n=(v^n_1,\ldots ,v^n_m)$ be a nonnegative solution of approximate system (3.2). Let us consider that $k_i= k,$ for $i=1,\ldots ,m$ . Then, up to a subsequence, $v^n$ converges in $L^1(Q_T)$ and a.e. in $Q_T$ to a supersolution $v=(v_1,\ldots ,v_m)$ of system (1.1), which means that for $i=1, \ldots ,m$ ,

(3.25) $$ \begin{align} -\int_{\Omega} v_i(\cdot,0)\Psi(\cdot,0)dx &+ \int_{0}^{T}\int_{\Omega} - v_i\partial_t\Psi + \nabla \varphi_i(v_i).\nabla\Psi dxdt \nonumber\\ &+k \int_{0}^{T}\int_{\Gamma}[\![ \Psi ]\!] [\![ \varphi_i(v_i) ]\!] \geq \int_{0}^{T}\int_{\Omega} R_i(v)\Psi dxdt \end{align} $$

for all $\Psi \in W_T$ .

Proof To prove this theorem, we will proceed in several steps. We start with compactness results for the approximate solution $v^n$ using the following lemma.

Lemma 3.4 Assume that the $L^1$ -estimate (3.6) holds for the solution $v^n$ of (3.2) with $r_i> \dfrac {(d-2)^{+}}{d} $ . Then, up to a subsequence, and for all $T>0$ and $1 \leq i \leq m$ , we have

• • $v_i^n \to v_i$ in $L^1(Q_T)$ and a.e. in $Q_T$ ,

• • $\varphi _i(v_i^n) \to \varphi _i(v_i)$ in $L^{\gamma }(Q_T)$ and a.e. in $Q_T$ for all $\gamma \in \left [1, 1+\dfrac {2}{r_i d}\right )$ ,

• • $\varphi _i(v_i) \in L^{\beta }(0,T;W^{1,\beta }(\Omega ))$ for all $\beta \in \left [1, 1+\dfrac {1}{1+r_i d}\right )$ ,

• • $R_i^n(v^n) \to R_i(v)$ a.e. in $Q_T$ and $R_i(v) \in L^1(Q_T)$ .

Proof Thanks to Lemma 3.1, $\left \lbrace R_i^n(v^n) \right \rbrace $ is bounded in $L^1(Q_T)$ , and according to Lemma 3.2, $\left \lbrace v_i^n \right \rbrace $ is relatively compact in $L^1(Q_T)$ .Therefore, up to a subsequence,

(3.26) $$ \begin{align} v_i^n \to v_i \textrm{ in } L^1(Q_T) \textrm{ and a.e. } Q_T. \end{align} $$

Thanks to the choice of $R_i^n(v^n)$ , and the a.e. convergence of $ \left \lbrace v_i^n \right \rbrace $ (3.26), it follows that

$$ \begin{align*} R_i^n(v^n) \to R_i(v) \textrm{ a.e. in } Q_T. \end{align*} $$

By Fatou’s lemma, we have

$$ \begin{align*} \int_{Q_T} \vert R_i(v) \vert \leq \liminf_{n \to +\infty} \int_{Q_T} \vert R_i^n(v^n) \vert, \end{align*} $$

which implies

$$ \begin{align*} R_i(v) \in L^1(Q_T)^m. \end{align*} $$

Thanks to the estimation (3.6), we can apply (3.23) of Lemma 3.2 to the $ith$ equation of (3.2). Then, it comes that $\left \lbrace \varphi _i(v_i^n) \right \rbrace _n$ is bounded in $L^{\gamma }(Q_T)$ for all $1 \leq \gamma < 1+ \dfrac {2}{r_id}$ and for all $T> 0$ , i.e., there is a constant $C> 0$ such that

$$ \begin{align*} \int_{Q_T} \vert \varphi_i(v_i^n) \vert^{\gamma} \leq C, \end{align*} $$

which implies that $(\varphi _i(v_i^n))^{\gamma } \textrm {is bounded in } L^1(Q_T)$ . By arbitrarity nature of $\gamma $ in $\left [1, 1+ \dfrac {2}{r_id} \right )$ , $\left (\varphi _i(v_i^n)\right )^{\gamma }$ is even uniformly integrable. Since it also converges a.e. to $\varphi _i(v_i)$ , by Vitali’s Lemma 3.3, the convergence holds strongly in $L^{\gamma }(Q_T)$ to $\varphi _i(v_i)$ .

Next, according to the estimation (3.6), we can apply (3.24) of Lemma 3.2 to the ith equation of (3.2). Then, it comes that $\left \lbrace \varphi _i(v_i^n) \right \rbrace _n$ is bounded in $L^{\beta }(0,T; W^{1,\beta }(\Omega ))$ for all $1 \leq \beta < 1+ \dfrac {1}{1+r_id}$ . These space being reflexive (for $\beta> 1$ ), it follows that $\varphi _i(v_i)$ also belongs to these same spaces.

Since $v_i^n$ satisfies (3.2), then for all $i=1,\ldots , m$ , we have

(3.27) $$ \begin{align} - \int_{\Omega} v_i^n(\cdot,0)\Psi(\cdot,0)dx &+ \int_{0}^{T}\int_{\Omega} - v_i^n\partial_t\Psi+ \nabla \varphi_i(v_i^n).\nabla\Psi dxdt \nonumber\\ &+ k \int_{0}^{T}\int_{\Gamma}[\![ \Psi ]\!] [\![ \varphi_i(v_i^n) ]\!]= \int_{0}^{T}\int_{\Omega} R_i^n(v_i^n)\Psi dxdt \end{align} $$

for all $\Psi \in W_T$ .

Now, we want to take the limit as $ n \to \infty $ in (3.27). But first, let us make the following remark.

Remark 2 So far we only have the a.e. convergence of $R_i^n(v_i^n)$ to $R_i(v_i)$ . As a result, we are unable to go to the limit in (3.27) in order to obtain the weak formulation in the sense of Definition 1. To do this, we need to show that the convergence of $R_i^n(v^n)$ to $R_i(v)$ occurs in the sense of the distributions. Herein lies the main difficulty of the proof. Indeed, $R_i^n(v^n)$ is bounded in $L^1(Q_T)$ . Consequently, it converges in the sense of measures to $R_i(v)+ \mu $ , where $\mu $ is a bounded measure. The challenge is to prove that this measure is equal to zero. For this, we use the truncation method, as in [27], to prove that the limit v is first a supersolution in the sense of (3.25). And later we prove that v is a subsolution in order to obtain the weak formulation desired in Definition 1.

3.4 Truncation method

Here, the main idea is that if $v_i^n$ is a weak solution of (3.2), then the regular approximation of the truncation function $T_b(v_i)$ (with $T_b$ defined below (3.28) and $v_i$ being the limit of $v_i^n$ as $n \rightarrow +\infty $ ) constitutes a supersolution of (1.1). To demonstrate this, we let $n \rightarrow +\infty $ in the inequality satisfied by an appropriate approximation of $T_b(v_i^n)$ (specifically (3.45) in Lemma 3.7).

In the semilinear case, the method consisted of writing, for each index i, the inequality satisfied by $T_b(v_i^n + \eta \sum _{j \neq i} v_j^n)$ with $\eta> 0$ , then letting $n \rightarrow +\infty $ for fixed $\eta $ and b, then $\eta \rightarrow 0$ , and finally $b \rightarrow +\infty $ (see [10, 27]).

Here, the ideas remain the same but need to be adapted to nonlinear diffusions. Therefore, to prepare the proof of existence of supersolution, let us introduce the truncating functions $T_b :[0, +\infty ) \to [0, +\infty )$ of class $C^3$ which satisfy the following for all $b \geq 1$ :

(3.28) $$ \begin{align} \begin{cases} T_b(\sigma)=\sigma \textrm{ if } \sigma \in [0,b-1],\\ T_b(\sigma) \leq b, \textrm{ for all } \sigma \geq 0. \\ T^{\prime}_b(\sigma) =0 \textrm{ if } \sigma \geq b,\\ 0\leq T^{\prime}_b(\sigma)\leq 1 \textrm{ and } -1\leq T^{\prime \prime}_b(\sigma)\leq 0 \textrm{ for all } \sigma \geq 0. \end{cases} \end{align} $$

For example, we may choose $T_b$ as

(3.29) $$ \begin{align} T_b(\sigma)= \begin{cases} \sigma, &\textrm{ if } \sigma \in [0,b-1], \\ \dfrac{\left( \sigma -b+1 \right)^{4}}{2} -\left( \sigma -b+1 \right)^{3} + \sigma, &\textrm{ if } \sigma \in [b-1,b], \\ b-\dfrac{1}{2}, &\textrm{ if } \sigma \in ]b,+\infty). \end{cases} \end{align} $$

Next, for all $i=1,\ldots , m$ , and for all $(n,\eta ,b) \in \mathbb {N}^{\star }\times (0,1)\times [1,+\infty )$ , we introduce

(3.30) $$ \begin{align} A^{n}_{i,\eta,b}= \partial_t \left[ T_b(v_i^n)T^{\prime}_b(\eta V_{i}^{n}) \right] - \nabla.\left[T^{\prime}_b (v_i^n)T^{\prime}_b(\eta V_i^n)\nabla \varphi_i(v_i^n)\right], \end{align} $$

where $T_b(v_i^n)$ is such that $\vert v_i^n \vert = \min \limits _{ j } \left \lbrace \vert vj \vert \right \rbrace $ and $V_i^n= \sum \limits _{j \neq i}v_j^n$ , $j \in \left \lbrace 1, \ldots ,m \right \rbrace $ .

Note that the operator $A^{n}_{i,\eta ,b} \to \partial _tv_i^n - \nabla .(\nabla \varphi _i(v_i^n))= \partial _tv_i^n - \Delta \varphi _i(v_i^n) $ as $\eta \to 1 $ and $b \to +\infty $ .

Using a computation, we can prove that

(3.31) $$ \begin{align} A^{n}_{i,\eta,b}= T^{\prime}_b(v_i^n)T_b(\eta V_i^n)R_i^n(v^n) + A_i^n + B_i^n, \end{align} $$

where

$$ \begin{align*} A_i^n= - \nabla \varphi_i(v_i^n).\nabla [T^{\prime}_b(v_i^n)T^{\prime}_b(\eta V_i^n)] \ \textrm{ and }\hspace{3cm}\\ B_i^n= \eta T_b(v_i^n)T^{\prime\prime}_b(\eta V_i^n)\partial_t V_i^n = \eta T_b(v_i^n)T^{\prime\prime}_b(\eta V_i^n)\sum \limits_{j \neq i}\left( \Delta \varphi_j(v_j^n) + R_j^n(v^n) \right). \end{align*} $$

By multiplying $A^{n}_{i,\eta ,b}$ by $\Psi \in W_T$ and then integrating over $Q_T$ , we obtain

(3.32) $$ \begin{align} \int_{Q_T} A^{n}_{i,\eta,b} \Psi = \int_{Q_T} T^{\prime}_b(v_i^n)T_b(\eta V_i^n)R_i^n(v^n) \Psi + \int_{Q_T} A_i^n \Psi + \int_{Q_T} B_i^n \Psi. \end{align} $$

Now we are going to bound the last two terms of (3.32). To arrive at this, we need the following lemma.

Lemma 3.5 Let $F \in L^{1}\left (Q_{T}\right )^{+}, w_{0} \in L^{1}(\Omega )^{+}$ . Then w the solution of (3.22) satisfies the following: there exists $C=C\left (\int _{Q_{T}} F, \int _{\Omega } w_{0}\right )$ such that, for all nondecreasing $\theta :(0,+\infty ) \rightarrow (0,+\infty )$ of class $\mathcal {C}^{1}$ and with $\theta \left (0^{+}\right )=0$ ,

(3.33) $$ \begin{align} \int_{[\theta(w) \leq b]}|\nabla \theta(w)||\nabla \varphi(w)|=\int_{[\theta(w) \leq b]} \nabla \theta(w) \nabla \varphi(w) \leq C b. \end{align} $$

In particular,

(3.34) $$ \begin{align} \int_{[\varphi(w) \leq b]}|\nabla \varphi(w)|^{2} \leq C b, \int_{[w \leq b]}|\nabla w|^{2} \leq C b^{2-r_i} \end{align} $$

with $r_{i}<2$ .

Remark 3 The main restriction $r_{i}<2$ discussed in the introduction appears in the above statement. The proof of Theorem 1 requires to control the $L^{2}$ -norm of $\nabla v_{i}^{n}$ on the level sets $\left [v_{i}^{n} \leq b\right ]$ . This $L^{2}$ -norm is not bounded if $r_{i} \geq 2$ because of the degeneracy around the points where $v_{i}^{n}=0$ . It is, however, valid for the large values of $v_{i}^{n}$ . But this does not seem to be sufficient for the proof.

Proof of Lemma 3.5

As usual, we make the computations for regular enough solutions and they are preserved by approximation for all semigroup solutions.

Multiplying the equation $\partial _{t} w-\Delta \varphi (w)=F$ by $T_{b+1}(\theta (w))$ . We obtain

(3.35) $$ \begin{align} \partial_{t} w T_{b+1}(\theta(w))-\Delta \varphi(w)T_{b+1}(\theta(w))=FT_{b+1}(\theta(w)). \end{align} $$

Let us set $j_b(w)=\displaystyle \int _0^w T_{b+1}(\theta (s))ds $ , then $J_{b}(0)=0$ and $\partial _t J_{b}(w)=\partial _t w T_{b+1}(\theta (w)).$ Integrating (3.35) over $Q_T$ , we obtain

$$ \begin{align*} \int_{\Omega} J_{b}(w)(T)&+ k\int_{0}^{T}\int_{\Gamma} [\![T_{b+1}(\theta(w))]\!][\![\varphi(w)]\!]+\int_{Q_{T}} T_{b+1}^{\prime}(\theta(w)) \nabla \theta(w) \nabla \varphi(w)\\ &\quad =\int_{Q_{T}} T_{b+1}(\theta(w)) F+\int_{\Omega} J_{b}\left(w_{0}\right). \end{align*} $$

Exploiting the increase in $T_{b+1}$ , $\theta $ and $\varphi $ , we find that $[\![T_{b+1}(\theta (w))]\!][\![\varphi (w)]\!] \geq 0$ . Since $T_{b+1} \leq b+1$ , we have $0\leq J_{b}(r) \leq (b+1) r$ for all $r \geq 0$ so that

$$ \begin{align*}\int_{[\theta(w) \leq b]}|\nabla \theta(w)||\nabla \varphi(w)| \leq(b+1)\left(\int_{Q_{T}} F+\int_{\Omega} w_{0}\right) \leq C (b). \end{align*} $$

Choosing $\theta :=\varphi $ gives the first estimate of (3.34). If $r_{i}<2$ in the expression of $\varphi $ (2.1), we choose $\theta (w):=w^{2-r_{i}}$ to obtain

$$ \begin{align*}d_{i}\left(2-r_{i}\right) r_{i} \int_{\left[w^{(2-r_{i})} \leq b\right]}|\nabla w|^{2} \leq C_1 b, \end{align*} $$

and by substituting b for $b^{2-r_{i}}$ , we get

$$ \begin{align*}\int_{\left[w \leq b\right]}|\nabla w|^{2} \leq C b^{2-r_{i}}, \end{align*} $$

which gives the second estimate of (3.34).

Thanks to the results of the previous lemma (Lemma 3.5), we are able to state the following results.

Lemma 3.6 There exist $\delta>0, C \geq 0$ independent of n and $\eta $ such that, for all $i=1, \ldots , m$ and for all $\Psi \in W_{T}$ ,

(3.36) $$ \begin{align} \int_{Q_{T}} \Psi A_{i}^{n} \geq -\eta^{\delta} C D(\Psi) \end{align} $$

and

(3.37) $$ \begin{align} \int_{Q_{T}} \Psi B_{i}^{n} \geq -\eta^{\delta} CD(\Psi), \end{align} $$

where $D(\Psi )=\|\Psi \|_{L^{\infty }\left (Q_{T}\right )}+\|\nabla \Psi \|_{L^{\infty }\left (Q_{T}\right )}$ .

Proof We have

$$ \begin{align*}\begin{aligned} \int_{Q_{T}} \Psi A_{i}^{n} & =-\int_{Q_{T}} \Psi \nabla \varphi_{i}\left(v_{i}^{n}\right) \nabla\left[T_{b}^{\prime}\left(v_{i}^{n}\right) T_{b}^{\prime}\left(\eta V_{i}^{n}\right)\right] \\ & =-\int_{Q_{T}} \Psi \nabla \varphi_{i}\left(v_{i}^{n}\right).\left[ T_{b}^{\prime \prime}\left(v_{i}^{n}\right) T_{b}^{\prime}\left(\eta V_{i}^{n}\right)\nabla v_{i}^{n} + \eta T_{b}^{\prime}\left(v_{i}^{n}\right) T_{b}^{\prime \prime}\left(\eta V_{i}^{n}\right) \nabla V_{i}^{n}\right] \\ & =-\int_{Q_{T}} \Psi \varphi^{\prime}_{i}\left(v_{i}^{n}\right) T_{b}^{\prime \prime}\left(v_{i}^{n}\right) T_{b}^{\prime}\left(\eta V_{i}^{n}\right)\vert \nabla v_{i}^{n} \vert^{2} - \eta\int_{Q_{T}} \Psi T_{b}^{\prime}\left(v_{i}^{n}\right) T_{b}^{\prime \prime}\left(\eta V_{i}^{n}\right) \nabla \varphi_{i}\left(v_{i}^{n}\right).\nabla V_{i}^{n} \\ & \geq-\eta \int_{Q_{T}} \Psi T_{b}^{\prime}\left(v_{i}^{n}\right) T_{b}^{\prime \prime}\left(\eta V_{i}^{n}\right) \nabla \varphi_{i}\left(v_{i}^{n}\right). \nabla V_{i}^{n}, \end{aligned} \end{align*} $$

the last inequality is obtained thanks to the positivity of $\varphi _{i}^{\prime }, \Psi $ and conditions on $T_b$ in (3.28). We can see that apart from $ \left \lbrace (x,t) \in Q_T, v_{i}^{n} \leq b \, \ \eta V_{i}^{n} \leq b \right \rbrace $ , $ T_{b}^{\prime }\left (v_{i}^{n}\right ) T_{b}^{\prime } \left (\eta V_{i}^{n}\right )=0$ .

To simplify notation, let us write $ \left [v_{i}^{n} \leq b\right ] \cap \left [\eta V_{i}^{n} \leq b\right ] $ instead of $ \left \lbrace (x,t) \in Q_T, v_{i}^{n} \leq b \, \ \eta V_{i}^{n} \leq b \right \rbrace $ . From this observation, it follows that

$$ \begin{align*}\begin{aligned} \int_{Q_{T}}\left|\Psi T_{b}^{\prime}\left(v_{i}^{n}\right) T_{b}^{\prime \prime}\left(\eta V_{i}^{n}\right) \nabla \varphi_{i}\left(v_{i}^{n}\right) \nabla V_{i}^{n}\right| & := \int_{\left[v_{i}^{n} \leq b\right] \cap \left[\eta V_{i}^{n} \leq b\right] }\left|\Psi T_{b}^{\prime}\left(v_{i}^{n}\right) T_{b}^{\prime \prime}\left(\eta V_{i}^{n}\right) \nabla \varphi_{i}\left(v_{i}^{n}\right) \nabla V_{i}^{n}\right|. \end{aligned} \end{align*} $$

By the Schwarz inequality and conditions on $T_b$ in (3.28), there exists a constant C depending on b, and we have the following result:

$$ \begin{align*}\begin{aligned} \int_{Q_{T}}&\left|\Psi T_{b}^{\prime}\left(v_{i}^{n}\right) T_{b}^{\prime \prime}\left(\eta V_{i}^{n}\right) \nabla \varphi_{i}\left(v_{i}^{n}\right) \nabla V_{i}^{n}\right| \\ & \leq C\|\Psi\|_{L^{\infty}\left(Q_{T}\right)}\left(\int_{\left[v_{i}^{n} \leq b\right] \cap \left[\eta V_{i}^{n} \leq b\right] }\left|\nabla \varphi_{i}\left(v_{i}^{n}\right)\right|{}^{2}\right)^{1 / 2}\left(\int_{\left[v_{i}^{n} \leq b\right] \cap \left[\eta V_{i}^{n} \leq b\right] }\left|\nabla V_{i}^{n}\right|{}^{2}\right)^{1 / 2} \\ & \leq C\|\Psi\|_{L^{\infty}\left(Q_{T}\right)}\left(\int_{\left[v_{i}^{n} \leq b\right]}\left|\nabla \varphi_{i}\left(v_{i}^{n}\right)\right|{}^{2}\right)^{1 / 2}\left(\int_{\left[\eta V_{i}^{n} \leq b\right]}\left|\nabla V_{i}^{n}\right|{}^{2}\right)^{1 / 2} \\ & \leq C\|\Psi\|_{L^{\infty}\left(Q_{T}\right)}\left(\int_{\left[\varphi_i(v_{i}^{n}) \leq \varphi_i(b)\right]}\left|\nabla \varphi_{i}\left(v_{i}^{n}\right)\right|{}^{2}\right)^{1 / 2}\left(\int_{\left[V_{i}^{n} \leq \frac{b}{\eta}\right]}\left|\nabla V_{i}^{n}\right|{}^{2}\right)^{1 / 2}. \end{aligned} \end{align*} $$

By Lemma 3.5, we obtain

$$ \begin{align*}\begin{aligned} \int_{Q_{T}}\left|\Psi T_{b}^{\prime}\left(v_{i}^{n}\right) T_{b}^{\prime \prime}\left(\eta V_{i}^{n}\right) \nabla \varphi_{i}\left(v_{i}^{n}\right) \nabla V_{i}^{n}\right| &\leq C\|\Psi\|_{L^{\infty}\left(Q_{T}\right)} \sqrt{\varphi_{i}(b)}[b / \eta]^{1-r_i / 2}\\ &\leq C\|\Psi\|_{L^{\infty}\left(Q_{T}\right)} \sqrt{\varphi_{i}(b)}[b / \eta]^{1-M / 2};\\ &\quad M:=\max \left\{1, \max \limits_{i} r_{i}\right\}\\ &\leq C\|\Psi\|_{L^{\infty}\left(Q_{T}\right)} b^{1+\frac{(r_i-M)}{2}} \eta^{\frac{M}{2}-1} \\ &\leq C(b) D(\Psi) \eta^{\frac{M}{2}-1}, \end{aligned} \end{align*} $$

where $C(b)= b $ and $D(\Psi )= \Vert \Psi \Vert _{L^{\infty }\left (Q_{T}\right )} + \Vert \nabla \Psi \Vert _{L^{\infty }\left (Q_{T}\right )}$ . This implies

$$ \begin{align*}\begin{aligned} \eta \int_{Q_{T}}\Psi T_{b}^{\prime}\left(v_{i}^{n}\right) T_{b}^{\prime \prime}\left(\eta V_{i}^{n}\right) \nabla \varphi_{i}\left(v_{i}^{n}\right) \nabla V_{i}^{n} \leq C(b) D(\Psi) \eta^{\frac{M}{2}}. \end{aligned} \end{align*} $$

Therefore,

(3.38) $$ \begin{align} \int_{Q_{T}} \Psi A_{i}^{n} \geq-C D(\Psi) \eta^{\delta} \end{align} $$

for some $C=C(b)$ and $\delta =M / 2$ , hence the result (3.36) of Lemma 3.6. To prove the second estimate of Lemma 3.6, we will proceed in several steps. We have

$$ \begin{align*} \int_{Q_{T}}\Psi B_{i}^{n}= \sum \limits_{j \neq i}\left(\eta \int_{Q_{T}}\Psi T_b(v_i^n)T^{\prime\prime}_b(\eta V_i^n) \Delta \varphi_j(v_j^n) + \eta\int_{Q_{T}}\Psi T_b(v_i^n)T^{\prime\prime}_b(\eta V_i^n) R_j^n(v^n) \right). \end{align*} $$

Let us put $I_n= \eta \displaystyle \int _{Q_{T}}\Psi T_b(v_i^n)T^{\prime \prime }_b(\eta V_i^n) \Delta \varphi _j(v_j^n) $ and $J_n= \eta \displaystyle \int _{Q_{T}}\Psi T_b(v_i^n) T^{\prime \prime }_b(\eta V_i^n) R_j^n(v^n)$ .

• • Let us bound $J_n$ . We have:

using the fact that $-1 \leq T^{\prime \prime }_b(\sigma ) \leq 0$ , $0 \leq T_b(\sigma ) \leq b$ for all $\sigma \geq 0$ , and the bound $L^{1}$ on $R_{i}^{n}$ , we obtain

(3.39) $$ \begin{align} J_n \geq -\eta C(b)\Vert \Psi \Vert_{L^{\infty}(Q_{T})}.\\[-24pt]\nonumber \end{align} $$

• • Let us bound $I_n$ . We have:

$$ \begin{align*} I_n &= \eta\int_{Q_{T}} \Psi T_{b}\left(v_{i}^{n}\right) T_{b}^{\prime \prime}\left(\eta V_{i}^{n}\right) \Delta \varphi_{j}\left(v_{j}^{n}\right)\\ &= -\eta\int_{0}^{T}\int_{\Gamma} k[\![\Psi T_{b}(v_{i}^{n}) T_{b}^{\prime \prime}\left(\eta V_{i}^{n}\right)]\!] [\![ \varphi_{j}(v_{j}^{n})]\!] \\ &\quad - \eta\int_{Q_{T}} \nabla\left(\Psi T_{b}(v_{i}^{n}) T_{b}^{\prime \prime}(\eta V_{i}^{n}) \right).\nabla \varphi_{j}(v_{j}^{n}) \\ &= -K_{n} - I_{1,n}-I_{2,n}-I_{3,n}, \end{align*} $$

where

$$ \begin{align*} &K_{n}= \eta\int_{0}^{T}\int_{\Gamma} k[\![\Psi T_{b}(v_{i}^{n}) T_{b}^{\prime \prime}\left(\eta V_{i}^{n}\right)]\!] [\![ \varphi_{j}(v_{j}^{n})]\!], \\ &I_{1,n}= \eta\int_{Q_T} T_{b}\left(v_{i}^{n}\right) T_{b}^{\prime \prime}\left(\eta V_{i}^{n}\right) \nabla \varphi_{j}\left(v_{j}^{n}\right).\nabla \Psi,\\ & I_{2,n}= \eta\int_{Q_T} \Psi T_{b}^{\prime}\left(v_{i}^{n}\right) T_{b}^{\prime \prime}\left(\eta V_{i}^{n}\right) \nabla \varphi_{j}(v_{j}^{n}).\nabla v_{i}^{n}, \\ & I_{3,n}=\eta^{2} \int_{Q_T} \Psi T_{b}\left(v_{i}^{n}\right) T_{b}^{\prime \prime \prime}\left(\eta V_{i}^{n}\right) \nabla \varphi_{j}(v_{j}^{n}).\nabla V_{i}^{n}.\\[-18pt] \end{align*} $$

• • Let us bound $K_{n}$ .

(3.40) $$ \begin{align} \vert K_n \vert =& \eta\left\vert \int_{0}^{T}\int_{\Gamma} k\left[{}^2\Psi T_{b}({}^2v_{i}^{n}) T_{b}^{\prime \prime}\left(\eta {{}^2V}_{i}^{n}\right) - {{}^1\Psi} T_{b}({}^1v_{i}^{n}) T_{b}^{\prime \prime}\left(\eta {{}^1V}_{i}^{n}\right) \right] \left[ \varphi_{j}({}^2v_{j}^{n}) - \varphi_{j}({}^1v_{j}^{n})\right] \right\vert \nonumber\\ &\leq 4b\eta \Vert \Psi \Vert_{L^{\infty}(Q_T)}\int_{\left[v_{i}^{n} \leq b\right] \cap\Gamma} \vert v_i^n \vert \vert \varphi_j(v_j^n) \vert \nonumber\\ &\leq 4b\eta D \Vert \Psi \Vert_{L^{\infty}(Q_T)}\int_{\left[v_{i}^{n} \leq b\right] \cap\Gamma} \vert v_j^n \vert^{rj+1} \nonumber\\ &\leq C(b,T)\eta\Vert \Psi \Vert_{L^{\infty}(Q_T)}. \end{align} $$

With $D=\max \limits _{j}\left \lbrace Dj \right \rbrace $ , we have used Lemma 3.1.

• • Let us bound $I_{1,n}$ . By (3.24) (with $\beta =1$ ) and Lemma 3.5, we obtain

(3.41) $$ \begin{align} \vert I_{1,n}\vert \leq b \eta \Vert \nabla \Psi \Vert_{L^{\infty}(Q_{T})} \int_{Q_{T}}\left|\nabla \varphi_{j}\left(v_{j}^{n}\right)\right| \leq C(b)\eta \Vert \nabla \Psi \Vert_{L^{\infty}(Q_{T})}.\\[-28pt]\nonumber \end{align} $$

• • Let us bound $I_{2, n}$ .

$$ \begin{align*} \vert I_{2,n} \vert&= \eta\left \vert \int_{Q_T} \Psi T_{b}^{\prime}\left(v_{i}^{n}\right) T_{b}^{\prime \prime}\left(\eta V_{i}^{n}\right) \nabla \varphi_{j}(v_{j}^{n}).\nabla v_{i}^{n} \right\vert \\ &=\eta \left \vert \int_{\left[\eta V_{i}^{n} \leq b\right] \cap\left[v_{i}^{n} \leq b\right]} \Psi T_{b}^{\prime}\left(v_{i}^{n}\right) T_{b}^{\prime \prime}\left(\eta V_{i}^{n}\right) \nabla \varphi_{j}(v_{j}^{n}).\nabla v_{i}^{n} \right\vert \\ &\leq \eta\int_{\left[\eta V_{i}^{n} \leq b\right] \cap\left[v_{i}^{n} \leq b\right]} \Psi T_{b}^{'}(v_{i}^{n})\vert T_{b}^{"}(\eta V_{i}^{n})\vert \vert \nabla \varphi_{j}(v_{j}^{n})\vert \vert \nabla v_{i}^{n}. \vert \end{align*} $$

By noticing that $\left \lbrace \eta V_{i}^{n} \leq b \right \rbrace \subset \left \lbrace \eta v_{j}^{n} \leq b \right \rbrace $ and by Schwarz’s inequality, it follows that

(3.42) $$ \begin{align} \vert I_{2,n} \vert &\leq \eta \int_{\left[\eta v_{j}^{n} \leq b\right] \cap\left[v_{i}^{n} \leq b\right]} \Psi T_{b}^{'}(v_{i}^{n})\vert T_{b}^{"}(\eta V_{i}^{n})\vert \vert \nabla \varphi_{j}(v_{j}^{n})\vert \vert \nabla v_{i}^{n} \vert \nonumber\\ & \leq \eta \Vert \Psi \Vert_{L^{\infty}(Q_{T})} \left(\int_{\left[\eta v_{j}^{n} \leq b\right]}\vert \nabla \varphi_{j}(v_{j}^{n})\vert^{2}\right)^{1 / 2}\left(\int_{\left[v_{i}^{n} \leq b\right]}\vert \nabla v_{i}^{n}\vert^{2}\right)^{1 / 2} \nonumber\\ & \leq \eta \Vert \Psi \Vert_{L^{\infty}(Q_{T})} \left(\int_{\left[\varphi_j (v_{j}^{n}) \leq \varphi_j( \frac{b}{\eta})\right]}\vert \nabla \varphi_{j}(v_{j}^{n})\vert^{2}\right)^{1 / 2}\left(\int_{\left[v_{i}^{n} \leq b\right]}\vert \nabla v_{i}^{n}\vert^{2}\right)^{1 / 2} \nonumber\\ & \leq \eta C \Vert \Psi \Vert_{L^{\infty}(Q_{T})} \sqrt{\varphi_j ( \frac{b}{\eta})} b^{1- \frac{r_{i}}{2}} \nonumber\\ &\leq C(b,D) \Vert \Psi \Vert_{L^{\infty}(Q_{T})}\eta^{1- \frac{r_{j}}{2}}, \end{align} $$

with $r_j< 2$ , $D=\max \limits _{j} \left \lbrace D_j \right \rbrace $ and $C(b,D) =C b^{1+ (r_{j}-r_{i})/2} \sqrt {D}$ , $i,j=1,\ldots ,m, j\neq i$ , and we have used Lemma 3.5.

• • Let us bound $I_{3, n}$ . Using again Schwarz’s inequality, Lemma 3.5, and $\left [\eta V_{i}^{n} \leq b\right ] \subset \left [\eta v_{j}^{n} \leq b\right ]$ , we obtain

(3.43) $$ \begin{align} \vert I_{3, n}\vert & \leq \eta^{2} \left( \int_{\left[\eta V_{i}^{n} \leq b\right]} \Psi \vert T_{b}(v_{i}^{n})\vert \vert T_{b}^{\prime \prime \prime}(\eta V_{i}^{n}) \vert \vert \nabla \varphi_{j}(v_{j}^{n})\vert \vert \nabla V_{i}^{n}\vert \right) \nonumber \\ & \leq C \eta^{2} \|\Psi\|_{L^{\infty}\left(Q_{T}\right)}\left[\int_{\left[\eta v_{j}^{n} \leq b\right]}\left|\nabla \varphi_{j}\left(v_{j}^{n}\right)\right|{}^{2}\right]^{1 / 2}\left[\left.\int_{\left[\eta V_{i}^{n} \leq b\right]}\left|\nabla V_{i}^{n}\right|{}^{2}\right]^{1 / 2}\right. \nonumber \\ & \leq C \eta^{2} \|\Psi\|_{L^{\infty}\left(Q_{T}\right)}\left[\int_{\left[\varphi_j (v_{j}^{n}) \leq \varphi_j(\frac{b}{\eta})\right]}\left|\nabla \varphi_{j}\left(v_{j}^{n}\right)\right|{}^{2}\right]^{1 / 2}\left[\left.\int_{\left[\eta V_{i}^{n} \leq b\right]}\left|\nabla V_{i}^{n}\right|{}^{2}\right]^{1 / 2}\right.\nonumber \\ & \leq C \eta^{2} \Vert \Psi\Vert_{L^{\infty}\left(Q_{T}\right)} \sqrt{\varphi_{j}(b / \eta)}\left[\frac{b}{\eta}\right]^{1-M / 2} \nonumber \\ & \leq C \eta^{2} \Vert \Psi\Vert_{L^{\infty}\left(Q_{T}\right)}\sqrt{D}\left[\frac{b}{\eta}\right]^{1+\frac{r_j-M}{2}} \nonumber \\ & \leq C(b,D) \Vert \Psi\Vert_{L^{\infty}\left(Q_{T}\right)}\eta^{1+\frac{M-r_j}{2}} \nonumber\\ & \leq C(b,D) \Vert \Psi\Vert_{L^{\infty}\left(Q_{T}\right)}\eta, \end{align} $$

where $C(b,D)=C\sqrt {D}b^{1+\frac {r_j-M}{2}}$ and $M=\max \limits _{j}\left \lbrace 1,\max \limits _{j}\left \lbrace r_j \right \rbrace \right \rbrace , j=1,\ldots ,m, \ j\neq i. $

From (3.40)–(3.43), we obtain

(3.44) $$ \begin{align} I_{n} \geq C(b,T,D)\eta^{\delta}D(\Psi), \end{align} $$

where $ \delta = 1-\frac {M}{2}, $ and $ D(\Psi )= \Vert \Psi \Vert _{L^{\infty }\left (Q_{T}\right )} + \Vert \nabla \Psi \Vert _{L^{\infty }\left (Q_{T}\right )} $ .

By (3.39) and (3.44), we obtain the estimation (3.37) of Lemma 3.6.

This proves Lemma 3.6.

Now, thanks to Lemma 3.6, we have the following result.

Lemma 3.7 There exist $\delta>0, C>0$ independent of n and $\eta $ such that, for all $i=1, \ldots $ , m and for all $\Psi \in W_{T}$ ,

(3.45) $$ \begin{align} \int_{Q_{T}} A_{i, \eta, b}^{n} \Psi \geq \int_{Q_{T}} T_{b}^{\prime}\left(v_{i}^{n}\right) T_{b}^{\prime}\left(\eta V_{i}^{n}\right) R_{i}^{n}\left(v^{n}\right) \Psi-C D(\Psi) \eta^{\delta}, \end{align} $$

where $ A_{i, \eta , b}^{n}=\partial _{t}\left (T_{b}\left (v_{i}^{n}\right ) T_{b}^{\prime }\left (\eta V_{i}^{n}\right )\right )-\nabla \cdot \left (T_{b}^{\prime }\left (v_{i}^{n}\right ) T_{b}^{\prime }\left (\eta V_{i}^{n}\right ) \nabla \varphi _{i}\left (v_{i}^{n}\right )\right ), \quad V_{i}^{n}=\sum \limits _{j \neq i} v_{j}^{n} $ and $D(\Psi )=\|\Psi \|_{L^{\infty }\left (Q_{T}\right )}+\|\nabla \Psi \|_{L^{\infty }\left (Q_{T}\right )}$ .

Proof It is a direct consequence of formula (3.32) and of Lemmas 3.5 and 3.6 above. We can choose $\delta = \min \left \lbrace \dfrac {M}{2}, 1- \dfrac {M}{2} \right \rbrace $ .

Note also that

(3.46) $$ \begin{align} \int_{Q_{T}} A_{i, n, b}^{n} \Psi=&-\int_{\Omega} T_{b}\left(v_{i 0}^{n}\right) T_{b}^{\prime}\left(\eta V_{i}^{n}(0)\right) \Psi(0) + k\int_{0}^{T}\int_{\Gamma} [\![ \Psi T^{\prime}_{b}(v_{i}^{n})T^{\prime}_{b}(\eta V_{i}^{n}) ]\!] [\![ \varphi_{i}(v_{i}^{n}) ]\!]\nonumber \\ &+\int_{Q_{T}}-T_{b}\left(v_{i}^{n}\right) T_{b}^{\prime}\left(\eta V_{i}^{n}\right) \partial_{t} \Psi+T_{b}^{\prime}\left(v_{i}^{n}\right) T_{b}^{\prime}\left(\eta V_{i}^{n}\right) \nabla \varphi_{i}\left(v_{i}^{n}\right) \nabla \Psi. \end{align} $$

Our aim now is to pass to the limit between (3.45) and (3.46). We do it in the following order: first $n \rightarrow +\infty $ , then $\eta \rightarrow 0$ , finally $b \rightarrow +\infty $ .

• • We make $n \rightarrow +\infty $ along the subsequence introduced in Lemma 3.4 ( $\eta $ and b are fixed). Since $v_{i 0}^{n} \rightarrow v_{i 0}$ in $L^{1}(\Omega )$ and $T_{b}, T_{b}^{\prime }$ are Lipschitz continuous, it follows that

$$ \begin{align*}\int_{\Omega} T_{b}\left(v_{i 0}^{n}\right) T_{b}^{\prime}\left(\eta V_{i}^{n}(0)\right) \Psi(0) \rightarrow \int_{\Omega} T_{b}\left(v_{i 0}\right) T_{b}^{\prime}\left(\eta V_{i}(0)\right) \Psi(0), \end{align*} $$

and thanks to Lemmas 3.2 and 3.4, it follows that

$$ \begin{align*}\int_{0}^{T}\int_{\Gamma} [\![ \Psi T^{\prime}_{b}(v_{i}^{n})T^{\prime}_{b}(\eta V_{i}^{n}) ]\!] [\![ \varphi_{i}(v_{i}^{n}) ]\!] \to \int_{0}^{T}\int_{\Gamma} [\![ \Psi T^{\prime}_{b}(v_{i})T^{\prime}_{b}(\eta V_{i}) ]\!] [\![ \varphi_{i}(v_{i}) ]\!]. \end{align*} $$

Concerning the last integral in (3.46), since, for all $j=1, \ldots , m, v_{j}^{n}$ converges in $L^{1}\left (Q_{T}\right )$ and a.e. to $v_{j}$ , we have

$$ \begin{align*}T_{b}\left(v_{i}^{n}\right) T_{b}^{\prime}\left(\eta V_{i}^{n}\right) \rightarrow T_{b}\left(v_{i}\right) T_{b}^{\prime}\left(\eta V_{i}\right) \text{ in } L^{1}\left(Q_{T}\right), \end{align*} $$

where we set $V_{i}:=\sum \limits _{j \neq i} v_{j}$ . It also follows that

$$ \begin{align*}T_{b}^{\prime}\left(\eta V_{i}^{n}\right) \to T_{b}^{\prime}\left(\eta V_{i}\right) \text{ in } L^{2}\left(Q_{T}\right). \end{align*} $$

Next, $T_{b}^{\prime }\left (v_{i}^{n}\right ) \nabla \varphi \left (v_{i}^{n}\right )$ is bounded in $L^{2}\left (Q_{T}\right )$ by (3.33) in Lemma 3.5. Therefore,

$$ \begin{align*}T_{b}^{\prime}\left(v_{i}^{n}\right) \nabla \varphi\left(v_{i}^{n}\right) \to T_{b}^{\prime}\left(v_{i}\right) \nabla \varphi\left(v_{i}\right) \textrm{ weakly in } L^{2}\left(Q_{T}\right). \end{align*} $$

Indeed, let us set $ S_{b}(r):=\displaystyle \int _{0}^{r} T_{b}^{\prime }(s) \varphi _{i}^{\prime }(s) d s$ , then $ \nabla S_{b}\left (v_{i}^{n}\right )=T_{b}^{\prime }\left (v_{i}^{n}\right ) \nabla \varphi \left (v_{i}^{n}\right )$ . Since $S_{b}\left (v_{i}^{n}\right )$ converges a.e. to $S_{b}\left (v_{i}\right )$ and is bounded, the convergence holds in the sense of distributions. Therefore, the distribution limit of $\nabla S_{b}\left (v_{i}^{n}\right )$ is $\nabla S_{b}\left (v_{i}\right )=T_{b}^{\prime }\left (v_{i}\right ) \nabla \varphi _{i}\left (v_{i}\right )$ .

This ends the proof of the passing to the limit in (3.46).

Now we will go to the limit in the right-hand side of the inequality (3.45). Let us put

$$ \begin{align*}W_{n}:=T_{b}^{\prime}\left(v_{i}^{n}\right) T_{b}^{\prime}\left(\eta V_{i}^{n}\right) R_{i}^{n}\left(v^{n}\right), \quad W : =T_{b}^{\prime}\left(v_{i}\right) T_{b}^{\prime}\left(\eta V_{i}\right) R_{i}(v) \end{align*} $$

and show that $W_{n}$ converges to W in $L^{1}\left (Q_{T}\right )$ . Since $W_{n}=0$ outside the set

$\left [v_{i}^{n} \leq b\right ] \cup \left [V_{i}^{n} \leq b / \eta \right ]$ , if $M: =\max \{b, b / \eta \}$ , then, from the definition of $\epsilon _{M}^{n}$ in (3.3), the regularity property (2.2), and the fact that $\left |T_{b}^{\prime }\right | \leq 1$ , we obtain

$$ \begin{align*}\left|W_{n}\right| \leq\left|R_{i}^{n}\left( v^{n}\right)\right| \leq\left|R_{i}(0)\right|+\epsilon_{M}^{n}+K(M)|| v^{n}(t, x)||, \textrm{ where } ||r||:= \sum \limits_{i=1}^{m} \vert r_{i} \vert. \end{align*} $$

By assumption (see (3.4)), as $n \rightarrow +\infty , \epsilon _{M}^{n}$ tends to 0 in $L^{1}\left (Q_{T}\right )$ . Moreover, $v^{n}$ converges in $L^{1}\left (Q_{T}\right )^{m}$ to v. Therefore, to prove the convergence of $W_{n}$ in $L^{1}\left (Q_{T}\right )$ , it is sufficient to prove that it converges a.e. We know that, for all $j, v_{j}^{n} \to v_{j}$ a.e. in $Q_T$ . Therefore, from the continuity of $T^{\prime }_b$ , it follows that

$$ \begin{align*}T_{b}^{\prime}\left(v_{i}^{n}\right) T_{b}^{\prime}\left(\eta V_{i}^{n}\right) \to T_{b}^{\prime}\left(v_{i}\right) T_{b}^{\prime}\left(\eta V_{i}\right) \textrm{ a.e. in } Q_T. \end{align*} $$

It remains to check that

(3.47) $$ \begin{align} R_{i}^{n}\left(v^{n}(t, x)\right) \to R_{i}( v(t, x)) \textrm{ a.e. in } Q_T. \end{align} $$

Let D be the subset of $Q_{T}$ such that, at the same time, $v^{n}(t, x)$ converges to $v(t, x)$ with $\|v(t, x)\|<+\infty $ and $\epsilon _{p}^{n}(t, x)$ converges to 0 for all positive integer p as $n \rightarrow +\infty $ along the subsequence introduced in Lemma 3.4. We know that $Q_{T}\setminus D$ is of zero Lebesgue measure. Now let $(t, x) \in D$ and let $p>\|v(t, x)\|$ . For n large enough, $\left \|v^{n}(t, x)\right \|<p$ and we may write for all $i=1, \ldots , m$ (using the definition de $\epsilon _{p}^{n}$ (3.3) and property (2.2)):

(3.48) $$ \begin{align} \left|R_{i}^{n}\left(v^{n}(t, x)\right)-R_{i}(v(t, x))\right| &\leq \epsilon_{p}(t, x)+\left|R_{i}\left(v^{n}(t, x)\right)-R_{i}(v(t, x))\right| \nonumber \\ & \leq \epsilon_{p}(t, x)+K(p) \|v^{n}(t, x)-v(t, x)||. \end{align} $$

The right-hand side of this inequality tends to 0 by definition of D.

According to the above analysis, we can pass to the limit as $n \rightarrow +\infty $ in (3.45) and (3.46) and we obtain that

(3.49) $$ \begin{align} -\int_{\Omega} T_{b}\left(v_{i 0}\right) T_{b}^{\prime}\left(\eta V_{i}(0)\right) \Psi(0) \,{+}\, & k\int_{0}^{T}\!\int_{\Gamma} [\![ \Psi T^{\prime}_{b}(v_{i})T^{\prime}_{b}(\eta V_{i}) ]\!] [\![ \varphi_{i}(v_{i}) ]\!]\nonumber \\ {+}\, &\int_{Q_{T}}\!\kern-1.3pt -T_{b}\left(v_{i}\right) T_{b}^{\prime}\left(\eta V_{i}\right) \partial_{t} \Psi+T_{b}^{\prime}\left(v_{i}\right) T_{b}^{\prime}\left(\eta V_{i}\right) \nabla \varphi_{i}\left(v_{i}\right) \nabla \Psi \nonumber \\ &\geq \int_{Q_{T}} T_{b}^{\prime}\left(v_{i}\right) T_{b}^{\prime}\left(\eta V_{i}\right) R_{i}(v) \Psi-C D(\Psi) \eta^{\delta}.\\[-24pt]\nonumber \end{align} $$

• • Now we make $\eta \rightarrow 0$ for b fixed in (3.49). Since $R_{i}^{n}\left (v^{n}\right )$ converges a.e. to $R_{i}(v)$ (see (3.47) and is bounded in $L^{1}\left (Q_{T}\right )$ , Fatou’s lemma implies that $R_{i}(v) \in L^{1}\left (Q_{T}\right )$ . We can observe that $T_b^{\prime }(r)= \chi _{[0,b-1]}(r)$ . Therefore, by continuity of $T_b^{\prime }$ (recall that $T_b \in C^3 $ ), when $\eta \rightarrow 0$ , $T_b^{\prime }\left (\eta V_i\right ) \rightarrow \chi _{[0,b-1]}(0):=1$ a.e. in $Q_T$ and remains bounded by 1, then by dominated convergence and thanks to the positivity of $\delta $ , we obtain

(3.50) $$ \begin{align} -\int_{\Omega} T_{b}\left(v_{i 0}\right) \Psi(0)&+ k\int_{0}^{T}\int_{\Gamma} [\![ \Psi T^{\prime}_{b}(v_{i})]\!] [\![ \varphi_{i}(v_{i}) ]\!] \nonumber\\ &+\int_{Q_{T}}-T_{b}\left(v_{i}\right) \partial_{t} \Psi+T_{b}^{\prime}\left(v_{i}\right) \nabla \varphi_{i}\left(v_{i}\right) \nabla \Psi \geq \int_{Q_{T}} T_{b}^{\prime}\left(v_{i}\right) R_{i}(v) \Psi. \end{align} $$

• • Finally, we let $b \rightarrow +\infty $ in this inequality (3.50). Then $T_{b}\left (v_{i}\right )$ increases to $v_{i}$ and $T_{b}^{\prime }\left (v_{i}\right )$ increases to $1, \nabla \varphi _{i}\left (v_{i}\right )$ is at least in $L^{1}\left (Q_{T}\right )$ (see (3.24) of Lemma 3.2 and $R_{i}(v) \in L^{1}\left (Q_{T}\right )$ . Therefore, we easily pass to the limit in (3.50) to obtain

(3.51) $$ \begin{align} -\int_{\Omega} v_{i 0} \Psi(0)+ k\int_{0}^{T}\int_{\Gamma} [\![ \Psi]\!] [\![ \varphi_{i}(v_{i}) ]\!] + \int_{Q_{T}}-v_{i} \partial_{t} \Psi+\nabla \varphi_{i}\left(v_{i}\right) \nabla \Psi \geq \int_{Q_{T}} R_{i}(v) \Psi. \end{align} $$

And this ends the proof of Theorem 2.

3.5 Global existence of weak solution

In this section, we finalize the proof of Theorem 1. We use the approximate system constructed earlier in Section 3.1. From Theorem 2, we already know that the limit v is a supersolution. In order to conclude the proof of Theorem 1, it is necessary to show that this supersolution is also a subsolution. To do this, we use the mass control structure property (2.6).

Proof of Theorem 1

By Theorem 2, up to subsequence, the approximate solution $v_{i}^{n}$ of the system (3.2) converges to a weak supersolution. We will show using the property of the mass control structure (2.6) that the inverse inequality of (3.51) is satisfied for the sum of its m expressions, i.e.,

(3.52) $$ \begin{align} -\int_{\Omega}\left[\sum_{i} v_{i 0}\right] \Psi(0) &+ k\int_{0}^{T}\int_{\Gamma} \left[ \sum_{i}[\![ \varphi_{i}(v_{i}) ]\!] \right][\![ \Psi]\!] \nonumber \\ &+\int_{Q_{T}}-\left[\sum_{i} v_{i}\right] \partial_{t} \Psi+\left[\sum_{i} \nabla \varphi_{i}\left(v_{i}\right)\right] \nabla \Psi \leq \int_{Q_{T}}\left[\sum_{i} R_{i}(v)\right] \Psi. \end{align} $$

This will imply that equality holds in each of the inequalities (3.51).

First, we recall some convergence results obtained above in the previous subsection. When $n \to + \infty $ , we have

(3.53) $$ \begin{align} \begin{cases} v_{i}^{n} \to v_{i} \textrm{ in } L^{1}(Q_{T}) \textrm{ and a.e. in } Q_{T},\\ \nabla \varphi_{i}(v_{i}^{n}) \rightharpoonup \nabla \varphi_{i}(v_{i}) \textrm{ weakly in } L^{2}(Q_{T}),\\ {v_{i}^{n}}_{\vert \Gamma} \to {v_{i}}_{\vert \Gamma} \textrm{ in } L^{1}(\Gamma). \end{cases} \end{align} $$

Let us look again at the approximate system (3.2) and add up the m equations. We then obtain that, for all $\Psi \in W_T$ ,

(3.54) $$ \begin{align} -\int_{\Omega}\left[\sum_{i} v_{i 0}^{n}\right] \Psi(0) &+ k\int_{0}^{T}\int_{\Gamma} \left[ \sum_{i}[\![ \varphi_{i}(v_{i}^{n}) ]\!] \right][\![ \Psi]\!] \nonumber\\ &+\int_{Q_{T}}-\left[\sum_{i} v_{i}^{n}\right] \partial_{t} \Psi+\left[\sum_{i} \nabla \varphi_{i}\left(v_{i}^{n}\right)\right] \nabla \Psi=\int_{Q_{T}}\left[\sum_{i} R_{i}^{n}\left(v^{n}\right)\right] \Psi. \end{align} $$

For the right-hand side of (3.54), thanks to the hypothesis (2.6) on $R_{i}^{n}$ , it follows that

$$ \begin{align*}C\left\|v^{n}\right\|+h-\sum_{i} R_{i}^{n}\left(v^{n}\right) \geq 0. \end{align*} $$

By a.e. convergence of all function $R_i, i=1,\ldots ,m$ (see 3.47), by $L^{1}(Q_{T})$ -convergence of $v^{n}$ and by Fatou’s lemma, we have

$$ \begin{align*}\int_{Q_{T}}\left[C\|v\|+h-\sum_{i} R_{i}(v)\right] \Psi \leq \int_{Q_{T}}(C\|v\|+h) \Psi+\liminf _{n \rightarrow+\infty} \int_{Q_{T}}-\left[\sum_{i} R_{i}^{n}\left(v^{n}\right)\right] \Psi. \end{align*} $$

Therefore,

$$ \begin{align*}\liminf _{n \rightarrow \infty} \int_{Q_{T}}\left[\sum_{i} R_{i}^{n}\left(v^{n}\right)\right] \Psi \leq \int_{Q_{T}}\left[\sum_{i} R_{i}(v)\right] \Psi. \end{align*} $$

Thus, by passing to the limit in equation (3.54) and using the convergence results (3.53), we arrive at the inequality (3.52). And, as explained above, this implies that the equality holds in (3.51), i.e.,

(3.55) $$ \begin{align} -\int_{\Omega} v_{i 0} \Psi(0)+ k\int_{0}^{T}\int_{\Gamma} [\![ \Psi]\!] [\![ \varphi_{i}(v_{i}) ]\!] + \int_{Q_{T}}-v_{i} \partial_{t} \Psi+\nabla \varphi_{i}\left(v_{i}\right) \nabla \Psi = \int_{Q_{T}} R_{i}(v) \Psi. \end{align} $$

And finally, thanks to an integration from, we arrive at the following formulation:

(3.56) $$ \begin{align} -\int_{\Omega} v_{i 0} \Psi(0) - \int_{Q_{T}} v_{i} \partial_{t} \Psi + \varphi_{i}\left(v_{i}\right) \Delta \Psi=\int_{Q_{T}} R_{i}(v) \Psi.\\[-36pt]\nonumber \end{align} $$