Hostname: page-component-745bb68f8f-b95js Total loading time: 0 Render date: 2025-02-05T16:06:26.839Z Has data issue: false hasContentIssue false
  • English
  • Français

A spectral refinement of the Bergelson–Host–Kra decomposition and new multiple ergodic theorems

Published online by Cambridge University Press:  07 September 2017

JOEL MOREIRA  and
FLORIAN KARL RICHTER
JOEL MOREIRA
Affiliation:
Department of Mathematics, Northwestern University, Evanston, Illinois, USA email [email protected]
FLORIAN KARL RICHTER
Affiliation:
Department of Mathematics, The Ohio State University, Columbus, Ohio, USA email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We investigate how spectral properties of a measure-preserving system $(X,{\mathcal{B}},\unicode[STIX]{x1D707},T)$ are reflected in the multiple ergodic averages arising from that system. For certain sequences $a:\mathbb{N}\rightarrow \mathbb{N}$, we provide natural conditions on the spectrum 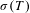$\unicode[STIX]{x1D70E}(T)$ such that, for all 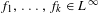$f_{1},\ldots ,f_{k}\in L^{\infty }$,

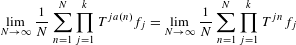$$\begin{eqnarray}\lim _{N\rightarrow \infty }\frac{1}{N}\mathop{\sum }_{n=1}^{N}\mathop{\prod }_{j=1}^{k}T^{ja(n)}f_{j}=\lim _{N\rightarrow \infty }\frac{1}{N}\mathop{\sum }_{n=1}^{N}\mathop{\prod }_{j=1}^{k}T^{jn}f_{j}\end{eqnarray}$$
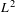in $L^{2}$-norm. In particular, our results apply to infinite arithmetic progressions,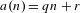$a(n)=qn+r$, Beatty sequences, 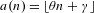$a(n)=\lfloor \unicode[STIX]{x1D703}n+\unicode[STIX]{x1D6FE}\rfloor$, the sequence of squarefree numbers, $a(n)=q_{n}$, and the sequence of prime numbers, 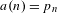$a(n)=p_{n}$. We also obtain a new refinement of Szemerédi’s theorem via Furstenberg’s correspondence principle.

Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 39 , Issue 4 , April 2019 , pp. 1042 - 1070
Copyright
© Cambridge University Press, 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Auslander, L., Green, L. and Hahn, F.. Flows on Homogeneous Spaces (Annals of Mathematics Studies, 53) . Princeton University Press, Princeton, NJ, 1963, with the assistance of L. Markus and W. Massey, and an appendix by L. Greenberg.Google Scholar
Bellow, A. and Losert, V.. The weighted pointwise ergodic theorem and the individual ergodic theorem along subsequences. Trans. Amer. Math. Soc. 288 (1985), 307–345.Google Scholar
Bergelson, V., Host, B. and Kra, B.. Multiple recurrence and nilsequences. Invent. Math. 160 (2005), 261–303, with an appendix by Imre Ruzsa.Google Scholar
Besicovitch, A. S.. Almost Periodic Functions. Dover Publications, New York, 1955.Google Scholar
Bohr, H.. Zur theorie der fast periodischen funktionen: I. Eine verallgemeinerung der theorie der fourierreihen. Acta Math. 45 (1925), 29–127.Google Scholar
Bohr, H.. Zur Theorie der Fastperiodischen Funktionen: II. Zusammenhang der fastperiodischen Funktionen mit Funktionen von unendlich vielen Variabeln; gleichmässige Approximation durch trigonometrische Summen. Acta Math. 46 (1925), 101–214.Google Scholar
Durrett, R.. Probability: Theory and Examples (Cambridge Series in Statistical and Probabilistic Mathematics) . 4th edn. Cambridge University Press, Cambridge, 2010.Google Scholar
Frantzikinakis, N.. The structure of strongly stationary systems. J. Anal. Math. 93 (2004), 359–388.Google Scholar
Frantzikinakis, N.. Multiple ergodic averages for three polynomials and applications. Trans. Amer. Math. Soc. 360 (2008), 5435–5475.Google Scholar
Frantzikinakis, N. and Host, B.. Higher order Fourier analysis of multiplicative functions and applications. J. Amer. Math. Soc. 30 (2017), 67–157.Google Scholar
Frantzikinakis, N., Host, B. and Kra, B.. Multiple recurrence and convergence for sequences related to the prime numbers. J. Reine Angew. Math. 611 (2007), 131–144.Google Scholar
Frantzikinakis, N., Host, B. and Kra, B.. The polynomial multidimensional Szemerédi theorem along shifted primes. Israel J. Math. 194 (2013), 331–348.Google Scholar
Furstenberg, H.. Disjointness in ergodic theory, minimal sets, and a problem in Diophantine approximation. Math. Systems Theory 1 (1967), 1–49.Google Scholar
Furstenberg, H.. Ergodic behavior of diagonal measures and a theorem of Szemerédi on arithmetic progressions. J. Analyse Math. 31 (1977), 204–256.Google Scholar
Glasner, E.. Ergodic Theory via Joinings (Mathematical Surveys and Monographs, 101) . American Mathematical Society, Providence, RI, 2003.Google Scholar
Gowers, W. T.. A new proof of Szemerédi’s theorem. Geom. Funct. Anal. 11 (2001), 465–588.Google Scholar
Green, B. and Tao, T.. Linear equations in primes. Ann. of Math. (2) 171 (2010), 1753–1850.Google Scholar
Green, B. and Tao, T.. The Möbius function is strongly orthogonal to nilsequences. Ann. of Math. (2) 175 (2012), 541–566.Google Scholar
Green, B., Tao, T. and Ziegler, T.. An inverse theorem for the Gowers U s+1[N]-norm. Ann. of Math. (2) 176 (2012), 1231–1372.Google Scholar
Green, L. W.. Spectra of nilflows. Bull. Amer. Math. Soc. 67 (1961), 414–415.Google Scholar
Host, B. and Kra, B.. An odd Furstenberg–Szemerédi theorem and quasi-affine systems. J. Anal. Math. 86 (2002), 183–220.Google Scholar
Host, B. and Kra, B.. Convergence of polynomial ergodic averages. Israel J. Math. 149 (2005), 1–19.Google Scholar
Host, B. and Kra, B.. Nonconventional ergodic averages and nilmanifolds. Ann. of Math. (2) 161 (2005), 397–488.Google Scholar
Host, B. and Kra, B.. Uniformity seminorms on ℓ ∞ and applications. J. Anal. Math. 108 (2009), 219–276.Google Scholar
Leibman, A.. Multiple recurrence theorem for measure preserving actions of a nilpotent group. Geom. Funct. Anal. 8 (1998), 853–931.Google Scholar
Leibman, A.. Pointwise convergence of ergodic averages for polynomial actions of ℤ d by translations on a nilmanifold. Ergod. Th. & Dynam. Sys. 25 (2005), 215–225.Google Scholar
Leibman, A.. Pointwise convergence of ergodic averages for polynomial sequences of translations on a nilmanifold. Ergod. Th. & Dynam. Sys. 25 (2005), 201–213.Google Scholar
Leibman, A.. Rational sub-nilmanifolds of a compact nilmanifold. Ergod. Th. & Dynam. Sys. 26 (2006), 787–798.Google Scholar
Leibman, A.. Multiple polynomial correlation sequences and nilsequences. Ergod. Th. & Dynam. Sys. 30 (2010), 841–854.Google Scholar
Leibman, A.. Orbit of the diagonal in the power of a nilmanifold. Trans. Amer. Math. Soc. 362 (2010), 1619–1658.Google Scholar
Lesigne, E.. Sur une nil-variété, les parties minimales associées à une translation sont uniquement ergodiques. Ergod. Th. & Dynam. Sys. 11 (1991), 379–391.Google Scholar
Parry, W.. Ergodic properties of affine transformations and flows on nilmanifolds. Amer. J. Math. 91 (1969), 757–771.Google Scholar
Parry, W.. Dynamical systems on nilmanifolds. Bull. Lond. Math. Soc. 2 (1970), 37–40.Google Scholar
Raghunathan, M. S.. Discrete Subgroups of Lie Groups (Ergebnisse der Mathematik und ihrer Grenzgebiete, 68) . Springer, New York–Heidelberg, 1972.Google Scholar
Rauzy, G.. Propriétés statistiques de suites arithmétiques (Le Mathématicien, 15, Collection SUP) . Presses Universitaires de France, Paris, 1976.Google Scholar
Ribenboim, P.. The New Book of Prime Number Records. Springer, New York, 1996.Google Scholar
Sun, W.. Multiple recurrence and convergence for certain averages along shifted primes. Ergod. Th. & Dynam. Sys. 35 (2015), 1592–1609.Google Scholar
Vinogradov, I. M.. The Method of Trigonometrical Sums in the Theory of Numbers. Interscience Publishers, London and New York, 1947, translated, revised and annotated by K. F. Roth and Anne Davenport.Google Scholar
Ziegler, T.. A non-conventional ergodic theorem for a nilsystem. Ergod. Th. & Dynam. Sys. 25 (2005), 1357–1370.Google Scholar
Ziegler, T.. Universal characteristic factors and Furstenberg averages. J. Amer. Math. Soc. 20 (2007), 53–97 (electronic).Google Scholar