No CrossRef data available.
Article contents
Asymptotic persistence time formulae for multitype birth–death processes
Published online by Cambridge University Press: 21 March 2023
Abstract
We consider a class of processes describing a population consisting of k types of individuals. The process is almost surely absorbed at the origin within finite time, and we study the expected time taken for such extinction to occur. We derive simple and precise asymptotic estimates for this expected persistence time, starting either from a single individual or from a quasi-equilibrium state, in the limit as a system size parameter N tends to infinity. Our process need not be a Markov process on 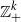 $ {\mathbb Z}_+^k$; we allow the possibility that individuals’ lifetimes may follow more general distributions than the exponential distribution.
$ {\mathbb Z}_+^k$; we allow the possibility that individuals’ lifetimes may follow more general distributions than the exponential distribution.
MSC classification
- Type
- Original Article
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press on behalf of Applied Probability Trust



