1. Introduction
The temperature-composition phase diagram of a partially miscible liquid mixture at a given pressure exhibits two distinct regions: a stable sector where the mixture is homogeneous, indicating complete miscibility of the liquids, and a two-phase region where two homogeneous phases coexist. These regions are separated by the equilibrium curve, obtained through the minimization of the mixture's free energy. When a partially miscible binary mixture is brought from the single-phase to the two-phase region by altering its composition and/or temperature, a phase separation process occurs. This process involves the formation of droplets of the minority phase, which subsequently grow by diffusion and coalescence. Close to the equilibrium curve within the two-phase region of the phase diagram, the mixture exists in a metastable state, necessitating a finite activation energy to initiate the phase separation process. Conversely, far from the equilibrium curve, the mixture becomes unstable, leading to phase separation via spinodal decomposition (Gunton, San Miguel & Sahni Reference Gunton, San Miguel and Sahni1983; Gunton Reference Gunton1984; Binder Reference Binder2005). This process entails the growth of fluctuations, even infinitesimal ones, exceeding a critical wavelength.
The growth of droplets of the minority phase in the context of phase separation is due to the relative movement of the two phases, induced by diffusion and convection. Diffusion arises from molecular velocity fluctuations at equilibrium, wherein molecules undergo incoherent, random motion without any specific preferential direction. In contrast, convection is a collective phenomenon that occurs over larger distances and is influenced by both outer and inner force fields. The latter is described by the diffuse interface model (also known as the phase field model) (Hohenberg & Halperin Reference Hohenberg and Halperin1977; Anderson, McFadden & Wheeler Reference Anderson, McFadden and Wheeler1998; Lowengrub & Truskinovsky Reference Lowengrub and Truskinovsky1998; Lamorgese, Mauri & Sagis Reference Lamorgese, Mauri and Sagis2017; Bertei, Chueh & Mauri Reference Bertei, Chueh and Mauri2021) as the gradient of a non-equilibrium chemical potential. Under equilibrium conditions, this, so-called, Korteweg force reduces to the Marangoni force (Jasnow & Viñals Reference Jasnow and Viñals1996; Jacqmin Reference Jacqmin2000), allowing the phase field approach to be viewed as a generalized model based on surface tension (McGuire et al. Reference McGuire, Laxminarayan, Martula and Lloyd1996; Martula et al. Reference Martula, Hasegawa, Lloyd and Bonnecaze2000), whereby the force acting on droplets linearly increases with their interface area. In the absence of an outer force field, both diffusion and convection occur in random directions, resulting in the formation of isotropic morphological structures. The ratio between convection and diffusion magnitudes is captured by the fluidity number, which distinguishes low viscous mixtures from high viscous ones. Notably, when diffusion dominates, as in the case of highly viscous systems or shallow temperature quenches, the average radius of the drops grows over time following a t 1/3 (Siggia Reference Siggia1979; deGennes Reference deGennes1980; Lifshitz & Pitaevskii Reference Lifshitz and Pitaevskii1984) or more generalized power law (Binder & Stauffer Reference Binder and Stauffer1974; Binder Reference Binder1977; Midya & Das Reference Midya and Das2017). Conversely, when convection drives the process, as seen in low-viscosity liquid mixtures or deep temperature quenches, the mean droplet radius grows linearly with time (Siggia Reference Siggia1979; Tanaka Reference Tanaka1996), in agreement with numerous experimental results (Chou & Goldburg Reference Chou and Goldburg1979; Wong & Knobler Reference Wong and Knobler1981; Guenoun et al. Reference Guenoun, Gastaud, Perrot and Beysens1987; Cumming et al. Reference Cumming, Wiltzius, Bates and Rosedale1992; White & Wiltzius Reference White and Wiltzius1995; Gupta, Mauri & Shinnar Reference Gupta, Mauri and Shinnar1999; Mauri et al. Reference Mauri, Califano, Calvi, Gupta and Shinnar2003; Califano & Mauri Reference Califano and Mauri2004).
The force exerted on droplets by an outer force field exhibits a linear growth with respect to the droplet volume, in contrast to the force resulting from the inner force field, which depends on the droplet surface area. The ratio between these two forces is referred to as the Bond number, which exhibits a linear dependence on the droplet radius. Consequently, for small droplets characterized by small Bond numbers, the outer force can be disregarded, resulting in isotropic morphological structures. As the droplets grow, they eventually reach a critical size corresponding to an O(1) Bond number, known as the capillary length. At this point, the influence of the outer force field becomes significant, causing a breakdown in the process symmetry. Recent research has demonstrated that when the mixture is initially unstable, phase separation occurs rapidly through spinodal decomposition, leading to a complete segregation of the two phases. In contrast, for initially metastable mixtures, the external force primarily induces species stratification, followed by a much slower nucleation process (Bertei & Mauri Reference Bertei and Mauri2022).
This study focuses on examining the phase separation process and its progression towards complete segregation. Numerical simulations are performed for different Bond and fluidity numbers, thus investigating the dynamics of segregation for both diffusion-dominated and convection-dominated regimes at different extents of the external force field. After presenting the model equations in § 2, numerical results are first discussed qualitatively in § 3.1, highlighting the principal phenomena of formation, coalescence and progression towards thermodynamic equilibrium with and without an external force field. A quantitative analysis of segregation, formation and floating times is then presented in § 3.2, revealing that phase segregation cannot be strictly interpreted as a series of elementary phenomena, although some patterns for the segregation time as a function of Bond number emerge in the diffusion-dominated regime.
2. Physical and mathematical model descriptions
The dynamic behaviour of a regular binary mixture under the influence of an external force field has been studied in previous works (Bertei & Mauri Reference Bertei and Mauri2022; Chueh, Mauri & Bertei Reference Chueh, Mauri and Bertei2022) for Mauri's non-equilibrium multiphase flow group. The physical model is developed based on the principles of mass conservation, momentum conservation and conservation of chemical species. The model assumes isothermal conditions and makes the following assumptions: (i) constant density, viscosity and diffusion coefficient that are independent of composition; and (ii) a regular and symmetric mixture, whose excess enthalpy depends on the Margules parameter ![]() $\varPsi $ (Bertei et al. Reference Bertei, Chueh and Mauri2021), where the two species possess equal molecular weights. This assumption is not overly restrictive and serves as a simplification to reduce the number of parameters. It is worth noting that for polymeric mixtures with high molecular weight, the average molecular weight can be used. As a result, the governing equations can be simplified as follows:
$\varPsi $ (Bertei et al. Reference Bertei, Chueh and Mauri2021), where the two species possess equal molecular weights. This assumption is not overly restrictive and serves as a simplification to reduce the number of parameters. It is worth noting that for polymeric mixtures with high molecular weight, the average molecular weight can be used. As a result, the governing equations can be simplified as follows:
where ![]() $\rho $ is the density of the mixture and η is its viscosity, while
$\rho $ is the density of the mixture and η is its viscosity, while ![]() $\boldsymbol{v}$, p and ϕ are respectively the mass-averaged velocity, the pressure and the mass fraction of species 1, which are functions of position, r, and time, t. Furthermore, f and Jϕ are the force per unit mass and the diffusive flux of species 1, respectively. In the present study, f is the sum of the outer (gravitational or electric) force, f(ext), and the inner Korteweg non-equilibrium force, f(K) (Bertei & Mauri Reference Bertei and Mauri2022):
$\boldsymbol{v}$, p and ϕ are respectively the mass-averaged velocity, the pressure and the mass fraction of species 1, which are functions of position, r, and time, t. Furthermore, f and Jϕ are the force per unit mass and the diffusive flux of species 1, respectively. In the present study, f is the sum of the outer (gravitational or electric) force, f(ext), and the inner Korteweg non-equilibrium force, f(K) (Bertei & Mauri Reference Bertei and Mauri2022):
where ![]() $\psi _{12}^{(K)} =- R^{\prime}T{a^2}{\nabla ^2}\phi $ is the Korteweg potential difference and
$\psi _{12}^{(K)} =- R^{\prime}T{a^2}{\nabla ^2}\phi $ is the Korteweg potential difference and ![]() $\psi _{12}^{(ext)} =- {\chi _{12}}bz$ is the external potential difference. Here,
$\psi _{12}^{(ext)} =- {\chi _{12}}bz$ is the external potential difference. Here, ![]() $R^{\prime} = R/{M_w}$ (with R denoting the universal gas constant and Mw the molecular weight), T is the temperature (that here is assumed to be uniform), a is a characteristic length (which is proportional to the surface tension at equilibrium),
$R^{\prime} = R/{M_w}$ (with R denoting the universal gas constant and Mw the molecular weight), T is the temperature (that here is assumed to be uniform), a is a characteristic length (which is proportional to the surface tension at equilibrium), ![]() $b = |\boldsymbol{b}|$ is the external force field magnitude, acting along the z-direction, and
$b = |\boldsymbol{b}|$ is the external force field magnitude, acting along the z-direction, and ![]() ${\chi _{12}}$ is the susceptibility difference, indicating how differently the two components of the mixture respond to the applied field. Note that a total energy potential
${\chi _{12}}$ is the susceptibility difference, indicating how differently the two components of the mixture respond to the applied field. Note that a total energy potential ![]() $\psi _{12}^{(cons)} = \psi _{12}^{(K)} + \psi _{12}^{(ext)}$ can be defined as the sum of all the conservative body forces (namely, Korteweg and external forces).
$\psi _{12}^{(cons)} = \psi _{12}^{(K)} + \psi _{12}^{(ext)}$ can be defined as the sum of all the conservative body forces (namely, Korteweg and external forces).
According to non-equilibrium thermodynamics, the diffusive flux, Jϕ, can be written as (Bertei & Mauri Reference Bertei and Mauri2022)
where ![]() ${\mu _{12}}$ is the thermodynamic chemical potential (which can be derived from the free energy of the mixture), while D* is the effective diffusivity. Imposing that for ideal mixtures (2.5) must reduce to Fick's law, then we obtain
${\mu _{12}}$ is the thermodynamic chemical potential (which can be derived from the free energy of the mixture), while D* is the effective diffusivity. Imposing that for ideal mixtures (2.5) must reduce to Fick's law, then we obtain ![]() ${D^\ast } = (\rho D/R^{\prime}T)\phi (1 - \phi )$, where D is a constant diffusivity.
${D^\ast } = (\rho D/R^{\prime}T)\phi (1 - \phi )$, where D is a constant diffusivity.
It is important to mention that the energy balance equation has been omitted in this analysis. This decision is based on the assumption that, similar to liquids, heat propagates significantly faster than mass. Consequently, it is assumed that the temperature of the mixture remains constant and uniform, mimicking an instantaneous quench.
After substituting the expressions for the non-equilibrium Korteweg force, the external force and the diffusion flux into the governing equations (2.1)–(2.3), we can transform them into a dimensionless form using the following scaling:
Here, ![]() $\hat{a}$ stands for the characteristic length,
$\hat{a}$ stands for the characteristic length, ![]() ${\hat{a}^2}/D$ denotes a characteristic diffusion time,
${\hat{a}^2}/D$ denotes a characteristic diffusion time, ![]() $D/\hat{a}$ is a characteristic diffusion velocity, while
$D/\hat{a}$ is a characteristic diffusion velocity, while ![]() $\eta D/{\hat{a}^2}$ represents a scaling factor for pressure. Consequently, the governing equations that describe the conservation of mass, momentum and chemical species are as follows:
$\eta D/{\hat{a}^2}$ represents a scaling factor for pressure. Consequently, the governing equations that describe the conservation of mass, momentum and chemical species are as follows:
 \begin{gather}\frac{{\partial \phi }}{{\partial \tilde{t}}} + \tilde{\boldsymbol{\nabla }}\boldsymbol{\cdot }(\tilde{\boldsymbol{v}}\phi + {\tilde{\boldsymbol{J}}_\phi }) = 0;\notag\\ {\tilde{\boldsymbol{J}}_\phi } =- \left[ {(1 - 2\varPsi \phi (1 - \phi ))\tilde{\nabla }\phi - \frac{\varPsi }{2}\phi (1 - \phi )\tilde{\nabla }({{\tilde{\nabla }}^2}\phi + {N_{Bo}}\tilde{z})} \right],\end{gather}
\begin{gather}\frac{{\partial \phi }}{{\partial \tilde{t}}} + \tilde{\boldsymbol{\nabla }}\boldsymbol{\cdot }(\tilde{\boldsymbol{v}}\phi + {\tilde{\boldsymbol{J}}_\phi }) = 0;\notag\\ {\tilde{\boldsymbol{J}}_\phi } =- \left[ {(1 - 2\varPsi \phi (1 - \phi ))\tilde{\nabla }\phi - \frac{\varPsi }{2}\phi (1 - \phi )\tilde{\nabla }({{\tilde{\nabla }}^2}\phi + {N_{Bo}}\tilde{z})} \right],\end{gather}where the following dimensionless parameters are defined: the Schmidt number, the fluidity number and the Bond (or Eotvos) number:
The Schmidt number, ![]() ${N_{Sc}}$, represents the ratio of momentum diffusivity
${N_{Sc}}$, represents the ratio of momentum diffusivity ![]() $(\eta /\rho )$ to material diffusivity (D). Note that in (2.6),
$(\eta /\rho )$ to material diffusivity (D). Note that in (2.6), ![]() ${N_{Sc}}$ assumes a role similar to that of the inverse Reynolds number, so that for liquid mixtures, where
${N_{Sc}}$ assumes a role similar to that of the inverse Reynolds number, so that for liquid mixtures, where ![]() ${N_{Sc}} \gg 1$, the inertial term in (2.8) is negligible. In this work, as we consider liquid mixtures, we assume
${N_{Sc}} \gg 1$, the inertial term in (2.8) is negligible. In this work, as we consider liquid mixtures, we assume ![]() ${N_{Sc}} = {10^3}$. The fluidity number, referred to as Nα, can be interpreted as an intrinsic Péclet number. It provides insight into whether the process is predominantly governed by mass diffusion
${N_{Sc}} = {10^3}$. The fluidity number, referred to as Nα, can be interpreted as an intrinsic Péclet number. It provides insight into whether the process is predominantly governed by mass diffusion ![]() $({N_\alpha } \le 1)$ or by convection
$({N_\alpha } \le 1)$ or by convection ![]() $({N_\alpha } \gg 1)$. Lastly, the Bond (or Eotvos) number, denoted as
$({N_\alpha } \gg 1)$. Lastly, the Bond (or Eotvos) number, denoted as ![]() ${N_{Bo}}$, expresses the ratio between external and capillary forces. The representative external force is given by
${N_{Bo}}$, expresses the ratio between external and capillary forces. The representative external force is given by ![]() $\rho {\chi _{12}}b{\hat{a}^3}$, while the reference capillary force is
$\rho {\chi _{12}}b{\hat{a}^3}$, while the reference capillary force is ![]() $\hat{\sigma }\hat{a}$, where
$\hat{\sigma }\hat{a}$, where ![]() $\hat{\sigma }$ represents a reference surface tension equal to
$\hat{\sigma }$ represents a reference surface tension equal to ![]() $\hat{\sigma } = \rho R^{\prime}{T_c}\hat{a}$ (Chueh et al. Reference Chueh, Mauri and Bertei2022), where
$\hat{\sigma } = \rho R^{\prime}{T_c}\hat{a}$ (Chueh et al. Reference Chueh, Mauri and Bertei2022), where ![]() ${T_c}$ is the critical temperature of the mixture. Both forces are expressed relative to the intrinsic characteristic length,
${T_c}$ is the critical temperature of the mixture. Both forces are expressed relative to the intrinsic characteristic length, ![]() $\hat{a}$. The Bond number can also be viewed as the ratio between the characteristic interface length
$\hat{a}$. The Bond number can also be viewed as the ratio between the characteristic interface length ![]() $\hat{a}$ and the capillary length,
$\hat{a}$ and the capillary length, ![]() ${l_c} = R^{\prime}{T_c}/{\chi _{12}}b$.
${l_c} = R^{\prime}{T_c}/{\chi _{12}}b$.
The system of (2.7)–(2.9) is solved numerically in a two-dimensional domain ![]() ${\varOmega = (\tilde{x},\tilde{z})}$, which represents a rectangular region with lateral periodic boundaries with two dimensionless side lengths of
${\varOmega = (\tilde{x},\tilde{z})}$, which represents a rectangular region with lateral periodic boundaries with two dimensionless side lengths of ![]() $\tilde{H}$ and
$\tilde{H}$ and ![]() $\tilde{L}$, as depicted in figure 1. The initial conditions of the mixture are characterized by a state of rest, indicated by
$\tilde{L}$, as depicted in figure 1. The initial conditions of the mixture are characterized by a state of rest, indicated by ![]() $\tilde{\boldsymbol{v}} = 0$, and uniform pressure, represented by
$\tilde{\boldsymbol{v}} = 0$, and uniform pressure, represented by ![]() $\tilde{p} = 0$.
$\tilde{p} = 0$.

Figure 1. Initial and boundary conditions considered in the present study.
An initial mass fraction lying within the spinodal unstable region (Sandler Reference Sandler2006) is set to ![]() ${\phi _0} = 0.4$, accompanied by a superimposed random white noise ε with statistical properties
${\phi _0} = 0.4$, accompanied by a superimposed random white noise ε with statistical properties ![]() $\langle \delta \phi \rangle = 0$ and
$\langle \delta \phi \rangle = 0$ and ![]() ${\langle \delta {\phi ^2}\rangle ^{1/2}} = {10^{ - 2}}$ at
${\langle \delta {\phi ^2}\rangle ^{1/2}} = {10^{ - 2}}$ at ![]() $t = 0$. These conditions indicate an abrupt and uniform quenching of the mixture below its critical temperature, with a Margules parameter Ψ set equal to 2.62.
$t = 0$. These conditions indicate an abrupt and uniform quenching of the mixture below its critical temperature, with a Margules parameter Ψ set equal to 2.62.
3. Results and discussion
In § 3.1, we explore the detailed complexities related to representing phase transition patterns, specifically focusing on the changes in ![]() ${N_{Bo}}$ and
${N_{Bo}}$ and ![]() ${N_\alpha }$ at a given
${N_\alpha }$ at a given ![]() ${N_{Sc}} = {10^3}$. Here,
${N_{Sc}} = {10^3}$. Here, ![]() ${N_\alpha }$ is the ratio between convection- and diffusion-driven material fluxes, and therefore the process is convection driven when
${N_\alpha }$ is the ratio between convection- and diffusion-driven material fluxes, and therefore the process is convection driven when ![]() ${N_\alpha } \gg 1$, while it is diffusion driven when
${N_\alpha } \gg 1$, while it is diffusion driven when ![]() ${N_\alpha } < 1$. In fact, in the absence of external forces, it has been shown (Vladimirova, Malagoli & Mauri Reference Vladimirova, Malagoli and Mauri1999) that the mean droplet size, d, grows linearly with time, i.e. d ∝ t when
${N_\alpha } < 1$. In fact, in the absence of external forces, it has been shown (Vladimirova, Malagoli & Mauri Reference Vladimirova, Malagoli and Mauri1999) that the mean droplet size, d, grows linearly with time, i.e. d ∝ t when ![]() ${N_\alpha } \gg 1$, while d ∝ t 1/3 when
${N_\alpha } \gg 1$, while d ∝ t 1/3 when ![]() ${N_\alpha } < 1$, in agreement with experimental results (Gupta et al. Reference Gupta, Mauri and Shinnar1999; Mauri et al. Reference Mauri, Califano, Calvi, Gupta and Shinnar2003). Conversely,
${N_\alpha } < 1$, in agreement with experimental results (Gupta et al. Reference Gupta, Mauri and Shinnar1999; Mauri et al. Reference Mauri, Califano, Calvi, Gupta and Shinnar2003). Conversely, ![]() ${N_{Bo}}$ plays a crucial role in determining the strength of an external force field, which is powerful enough to cause upward movement of the droplets along the z-axis. In § 3.2, correlations for phase separation and phase segregation as functions of domain height,
${N_{Bo}}$ plays a crucial role in determining the strength of an external force field, which is powerful enough to cause upward movement of the droplets along the z-axis. In § 3.2, correlations for phase separation and phase segregation as functions of domain height, ![]() ${N_{Bo}}$ and
${N_{Bo}}$ and ![]() ${N_\alpha }$, are presented. It is important to note that simulations were carried out using the same code already validated by Chueh, Bertei & Mauri (Reference Chueh, Bertei and Mauri2020) and Bertei & Mauri (Reference Bertei and Mauri2022).
${N_\alpha }$, are presented. It is important to note that simulations were carried out using the same code already validated by Chueh, Bertei & Mauri (Reference Chueh, Bertei and Mauri2020) and Bertei & Mauri (Reference Bertei and Mauri2022).
3.1. Effect of  ${\boldsymbol{N}_{\boldsymbol{Bo}}}$ and
${\boldsymbol{N}_{\boldsymbol{Bo}}}$ and  ${\boldsymbol{N}_{\boldsymbol{\alpha }}}$ on phase segregation
${\boldsymbol{N}_{\boldsymbol{\alpha }}}$ on phase segregation
Figure 2 illustrates a scenario where both ![]() ${N_\alpha }$ and
${N_\alpha }$ and ![]() ${N_{Bo}}$ are notably small, namely
${N_{Bo}}$ are notably small, namely ![]() ${N_\alpha } = 0.01$ and
${N_\alpha } = 0.01$ and ![]() ${N_{Bo}} = {10^{ - 3}}$. Given the asymmetric initial composition
${N_{Bo}} = {10^{ - 3}}$. Given the asymmetric initial composition ![]() ${\phi _0} = 0.4$, phase separation proceeds via the formation of droplets of the minority phase. We see that drops form and start growing due to the coalescence induced by diffusion; However, the convection induced by external forces is initially very weak, since the drops are much smaller than the capillary length
${\phi _0} = 0.4$, phase separation proceeds via the formation of droplets of the minority phase. We see that drops form and start growing due to the coalescence induced by diffusion; However, the convection induced by external forces is initially very weak, since the drops are much smaller than the capillary length ![]() ${l_c}$, where external forces are balanced by surface forces (i.e. the corresponding macroscopic Bond number is O(1)). In the last part of the process, as the drops increase in size, the effect of the external force starts to become relevant, thus facilitating droplet growth through convection-induced coalescence. Note that, despite the seemingly small value of
${l_c}$, where external forces are balanced by surface forces (i.e. the corresponding macroscopic Bond number is O(1)). In the last part of the process, as the drops increase in size, the effect of the external force starts to become relevant, thus facilitating droplet growth through convection-induced coalescence. Note that, despite the seemingly small value of ![]() ${N_{Bo}}$, the external force always plays a significant role, since at the end, drops will always reach the capillary length size and start moving upward; while in the absence of external forces, this floating process does not take place. At the end, we observe the formation of an upper rich phase (in red) with a horizontal interface that moves downward, until complete phase segregation is reached. It is interesting to note that, due to a 90° contact angle, some droplets occasionally remain attached to both the bottom and the upper walls. However, the magnitude of the Bond number,
${N_{Bo}}$, the external force always plays a significant role, since at the end, drops will always reach the capillary length size and start moving upward; while in the absence of external forces, this floating process does not take place. At the end, we observe the formation of an upper rich phase (in red) with a horizontal interface that moves downward, until complete phase segregation is reached. It is interesting to note that, due to a 90° contact angle, some droplets occasionally remain attached to both the bottom and the upper walls. However, the magnitude of the Bond number, ![]() ${N_{Bo}} = {10^{ - 3}}$, exceeds a critical threshold identified in previous works (Chueh et al. Reference Chueh, Mauri and Bertei2022), corresponding to the point at which droplets detach from the confining walls. Furthermore, the observed behaviour and dynamics align notably well with the patterns depicted in figure 4 of Califano, Mauri & Shinnar (Reference Califano, Mauri and Shinnar2005), further corroborating the agreement between the experimental findings and the current study.
${N_{Bo}} = {10^{ - 3}}$, exceeds a critical threshold identified in previous works (Chueh et al. Reference Chueh, Mauri and Bertei2022), corresponding to the point at which droplets detach from the confining walls. Furthermore, the observed behaviour and dynamics align notably well with the patterns depicted in figure 4 of Califano, Mauri & Shinnar (Reference Califano, Mauri and Shinnar2005), further corroborating the agreement between the experimental findings and the current study.

Figure 2. Time evolution of phase segregation for ![]() ${N_\alpha } = 0.01$ and
${N_\alpha } = 0.01$ and ![]() ${N_{Bo}} = {10^{ - 3}}$ in a rectangular domain with two dimensionless side lengths of
${N_{Bo}} = {10^{ - 3}}$ in a rectangular domain with two dimensionless side lengths of ![]() $\tilde{H} = 200$ and
$\tilde{H} = 200$ and ![]() $\tilde{L} = 100$.
$\tilde{L} = 100$.
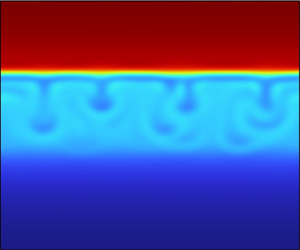
It is particularly intriguing to conduct a comparative analysis of the phase separation patterns in the presence and absence of an external force field, as visually demonstrated in figure 3. In the absence of any external force (as depicted in the first row of figure 3), the growth of the droplets is primarily influenced by the effect of the internal force field, which is typically proportional to the area of the interface. As we observe the impact of increasing values of NBo, ranging from 10−5 to 10−3, we see that the external force field, whose effect is proportional to the volume of the drops and grows linearly with their size, becomes increasingly influential. Consequently, the acceleration in the drop formation process becomes more pronounced (notice the different dimensionless times above each panel), thereby expediting the attainment of complete segregation within the system.

Figure 3. Time evolution of phase segregation for ![]() ${N_\alpha } = 0.01$ and
${N_\alpha } = 0.01$ and ![]() ${N_{Bo}}$, ranging from 0 to 10−3, in a square domain with the dimensionless side length of
${N_{Bo}}$, ranging from 0 to 10−3, in a square domain with the dimensionless side length of ![]() $\tilde{H} = \tilde{L} = 100$.
$\tilde{H} = \tilde{L} = 100$.
A noteworthy transformation is shown in figure 4, as the value of ![]() ${N_\alpha }$ is set to 100, ushering in a distinct dominance of convection within the process at hand. As time progresses, the droplets exhibit a tendency to either emerge or coalesce with one another due to the vigorous influence of convection in a nearly ballistic motion. Consequently, the phenomenon of diffusion-induced growth, observed in previous instances, becomes irrelevant under such circumstances. In a parallel development, when
${N_\alpha }$ is set to 100, ushering in a distinct dominance of convection within the process at hand. As time progresses, the droplets exhibit a tendency to either emerge or coalesce with one another due to the vigorous influence of convection in a nearly ballistic motion. Consequently, the phenomenon of diffusion-induced growth, observed in previous instances, becomes irrelevant under such circumstances. In a parallel development, when ![]() ${N_{Bo}} < {10^{ - 4}}$, the red drops remain attached to the bottom wall through the capillary force, and the external force is not strong enough to detach them. However, for larger
${N_{Bo}} < {10^{ - 4}}$, the red drops remain attached to the bottom wall through the capillary force, and the external force is not strong enough to detach them. However, for larger ![]() ${N_{Bo}}$, the rich red phase embarks on an upward trajectory, progressively forming an interface that moves in a downward direction over time. Overall, the dynamics of the process becomes much faster than in the absence of convection (compare the dimensionless times above each panel in figures 3 and 4).
${N_{Bo}}$, the rich red phase embarks on an upward trajectory, progressively forming an interface that moves in a downward direction over time. Overall, the dynamics of the process becomes much faster than in the absence of convection (compare the dimensionless times above each panel in figures 3 and 4).

Figure 4. Time evolution of phase segregation for ![]() ${N_\alpha } = 100$ and
${N_\alpha } = 100$ and ![]() ${N_{Bo}}$, ranging from 0 to 10−3, in a square domain with the dimensionless side length of
${N_{Bo}}$, ranging from 0 to 10−3, in a square domain with the dimensionless side length of ![]() $\tilde{H} = \tilde{L} = 100$.
$\tilde{H} = \tilde{L} = 100$.
A comparative analysis of the droplet size evolution is shown in figure 5. The figure reports the characteristic droplet size (Bertei, Tellini & Mauri Reference Bertei, Tellini and Mauri2019; Chueh, Bertei & Mauri Reference Chueh, Bertei and Mauri2020) along the horizontal ![]() $({\tilde{d}_x})$ and vertical direction
$({\tilde{d}_x})$ and vertical direction ![]() $({\tilde{d}_z})$ as a function of dimensionless time for a selection of different Bond numbers in the two regimes of fluidity numbers, corresponding to the cases represented in figures 3 and 4. Figure 5(a), corresponding to
$({\tilde{d}_z})$ as a function of dimensionless time for a selection of different Bond numbers in the two regimes of fluidity numbers, corresponding to the cases represented in figures 3 and 4. Figure 5(a), corresponding to ![]() ${N_\alpha } = 0.01$ and
${N_\alpha } = 0.01$ and ![]() ${N_{Bo}} = {10^{ - 3}}$, shows that droplets grow according to a scaling
${N_{Bo}} = {10^{ - 3}}$, shows that droplets grow according to a scaling ![]() $\tilde{d} \propto {\tilde{t}^{1/3}}$ as long as they are sufficiently small to be unaffected by the external force field; then, as the droplet size increases, the external force adds a coherent vertical motion that causes coalescence in both directions and speeds up the droplet growth. Nevertheless, the convection-dominated regime, characterized by
$\tilde{d} \propto {\tilde{t}^{1/3}}$ as long as they are sufficiently small to be unaffected by the external force field; then, as the droplet size increases, the external force adds a coherent vertical motion that causes coalescence in both directions and speeds up the droplet growth. Nevertheless, the convection-dominated regime, characterized by ![]() $\tilde{d} \propto \tilde{t}$, is not reached; this is consistent with the qualitative description provided at the beginning of this section. For
$\tilde{d} \propto \tilde{t}$, is not reached; this is consistent with the qualitative description provided at the beginning of this section. For ![]() ${N_\alpha } = 100$, reported in figure 5(b), in addition to the irregular spikes that correspond to coalescence events, there is a faster growth along the vertical direction as the Bond number increases. The growth rate never scales as
${N_\alpha } = 100$, reported in figure 5(b), in addition to the irregular spikes that correspond to coalescence events, there is a faster growth along the vertical direction as the Bond number increases. The growth rate never scales as ![]() $\tilde{d} \propto {\tilde{t}^{1/3}}$ as expected, because the dynamics is dominated by convection for
$\tilde{d} \propto {\tilde{t}^{1/3}}$ as expected, because the dynamics is dominated by convection for ![]() ${N_\alpha } = 100$, but a clear and sustained linear regime cannot be claimed either as
${N_\alpha } = 100$, but a clear and sustained linear regime cannot be claimed either as ![]() ${N_{Bo}}$ varies. As also stressed in the next section, identifying general laws for the convection-driven regime is challenging and requires larger computational domains and statistical analysis which are beyond the scope of the present study.
${N_{Bo}}$ varies. As also stressed in the next section, identifying general laws for the convection-driven regime is challenging and requires larger computational domains and statistical analysis which are beyond the scope of the present study.
Coming back to the qualitative description of phenomena, it is important to acknowledge that a mixture may initially attain a state of local equilibrium, wherein the compositions of the droplets and the continuous phase align with the equilibrium compositions of the respective phases. However, the complete segregation of the two phases occurs much later in the process. Consequently, we introduce the concept of separation depth to measure the extent to which the system deviates from local equilibrium on a 0–1 scale. The separation depth, S, was defined by Vladimirova, Malagoli & Mauri (Reference Vladimirova, Malagoli and Mauri1999) as
 \begin{equation}S = \left\{ {\begin{array}{*{20}{@{}ll@{}}} {\dfrac{{\phi - {\phi_0}}}{{\phi_e^{(B\,)} - {\phi_0}}}}&{\textrm{for}\;\phi > {\phi_0}}\\ {\dfrac{{\phi - {\phi_0}}}{{\phi_e^{(A)} - {\phi_0}}}}&{\textrm{for}\;\phi \le {\phi_0}} \end{array}} \right.,\end{equation}
\begin{equation}S = \left\{ {\begin{array}{*{20}{@{}ll@{}}} {\dfrac{{\phi - {\phi_0}}}{{\phi_e^{(B\,)} - {\phi_0}}}}&{\textrm{for}\;\phi > {\phi_0}}\\ {\dfrac{{\phi - {\phi_0}}}{{\phi_e^{(A)} - {\phi_0}}}}&{\textrm{for}\;\phi \le {\phi_0}} \end{array}} \right.,\end{equation} where ![]() $\phi _e^{(A)}$ and
$\phi _e^{(A)}$ and ![]() $\phi _e^{(B)}$ denote the equilibrium compositions of the poor (blue) and rich (red) phases, equal to 0.12 and 0.88, respectively, for the conditions simulated in this study. For
$\phi _e^{(B)}$ denote the equilibrium compositions of the poor (blue) and rich (red) phases, equal to 0.12 and 0.88, respectively, for the conditions simulated in this study. For ![]() ${N_\alpha }\; = 100$, the separation depth maps shown in figure 6 provide a vivid portrayal of the droplets quickly achieving local equilibrium, while the encompassing continuum phase situated outside lingers in a state of disequilibrium. The diffuse interface separating the two phases inherently shows S < 1 since the mass fraction varies smoothly from inside to outside a droplet according to the phase field approach. The discrepancy between internal droplet equilibration versus external disequilibrium of the continuum phase, along with the interfacial composition gradient (Kumaran Reference Kumaran1998, Reference Kumaran2000), serve as crucial factors for the rapid dynamics observed within the system. Although barely noticeable, the external force induces a little variation in composition along the vertical direction in both the continuum and droplet phases, which corresponds to the stratification phenomenon discussed elsewhere (Bertei & Mauri Reference Bertei and Mauri2022). In any case, it is clear that in a convection-dominated regime, droplets equilibrate quickly after emergence and initial growth, while further coalescence induced by the external force field takes place at a later stage when the droplets are locally at thermodynamic equilibrium.
${N_\alpha }\; = 100$, the separation depth maps shown in figure 6 provide a vivid portrayal of the droplets quickly achieving local equilibrium, while the encompassing continuum phase situated outside lingers in a state of disequilibrium. The diffuse interface separating the two phases inherently shows S < 1 since the mass fraction varies smoothly from inside to outside a droplet according to the phase field approach. The discrepancy between internal droplet equilibration versus external disequilibrium of the continuum phase, along with the interfacial composition gradient (Kumaran Reference Kumaran1998, Reference Kumaran2000), serve as crucial factors for the rapid dynamics observed within the system. Although barely noticeable, the external force induces a little variation in composition along the vertical direction in both the continuum and droplet phases, which corresponds to the stratification phenomenon discussed elsewhere (Bertei & Mauri Reference Bertei and Mauri2022). In any case, it is clear that in a convection-dominated regime, droplets equilibrate quickly after emergence and initial growth, while further coalescence induced by the external force field takes place at a later stage when the droplets are locally at thermodynamic equilibrium.

Figure 6. Time evolution of separation depth contour corresponding to figure 4.
In figure 7, when ![]() ${N_{Bo}} = {10^{ - 1}}$ and
${N_{Bo}} = {10^{ - 1}}$ and ![]() ${N_\alpha } = 100$, the strong convection induced by the external force field causes a stratification of the two species, with the formation of two interfaces. Therefore, during the process of phase separation,
${N_\alpha } = 100$, the strong convection induced by the external force field causes a stratification of the two species, with the formation of two interfaces. Therefore, during the process of phase separation, ![]() $\phi \approx \phi _e^{(B)}\; $ at the top and
$\phi \approx \phi _e^{(B)}\; $ at the top and ![]() $\phi \approx \phi _e^{(A)}$ at the bottom, while between the two interfaces, a random convective motion takes place (with no local equilibrium), with a rapid process of spinodal decomposition. Then, the two interfaces gradually move towards each other until they merge and a complete phase segregation is reached. The phase separation process described in figure 7 corresponds to the experimental data by Califano & Mauri (Reference Califano and Mauri2018).
$\phi \approx \phi _e^{(A)}$ at the bottom, while between the two interfaces, a random convective motion takes place (with no local equilibrium), with a rapid process of spinodal decomposition. Then, the two interfaces gradually move towards each other until they merge and a complete phase segregation is reached. The phase separation process described in figure 7 corresponds to the experimental data by Califano & Mauri (Reference Califano and Mauri2018).

Figure 7. Time evolution of phase segregation for ![]() ${N_\alpha } = 100$ and
${N_\alpha } = 100$ and ![]() ${N_{Bo}} = {10^{ - 1}}$, in a square domain with the dimensionless side length of
${N_{Bo}} = {10^{ - 1}}$, in a square domain with the dimensionless side length of ![]() $\tilde{H} = \tilde{L} = 100$.
$\tilde{H} = \tilde{L} = 100$.
3.2. Quantitative analysis of segregation time
In this section, we present a quantitative analysis of the processes that were qualitatively described in the previous section. In particular, so far, we loosely defined segregation as the process that leads to a complete separation of the two equilibrated phases, A and B, under an external force. Being more specific, we mark the segregation time, ![]() ${\tilde{t}_{segr}}$, as the time at which there is, within the domain, only one interface that separates the two equilibrated phases. Thus,
${\tilde{t}_{segr}}$, as the time at which there is, within the domain, only one interface that separates the two equilibrated phases. Thus, ![]() ${\tilde{t}_{segr}}$ characterizes the segregation process at the macroscale, by identifying the moment when the system transitions from multiple interfaces to a single interface. Arguably, the segregation process may be decoupled into two phenomena: (i) formation, which is the process of emergence of droplets until their internal composition achieves thermodynamic equilibrium; and (ii) floating, which refers to the rising and coalescence of (equilibrated) droplets under the external force field (obviously, we would call this process sedimentation if droplets moved downwards, which depends only on the orientation of the external force field). Each of these two processes takes place in a finite time that we call
${\tilde{t}_{segr}}$ characterizes the segregation process at the macroscale, by identifying the moment when the system transitions from multiple interfaces to a single interface. Arguably, the segregation process may be decoupled into two phenomena: (i) formation, which is the process of emergence of droplets until their internal composition achieves thermodynamic equilibrium; and (ii) floating, which refers to the rising and coalescence of (equilibrated) droplets under the external force field (obviously, we would call this process sedimentation if droplets moved downwards, which depends only on the orientation of the external force field). Each of these two processes takes place in a finite time that we call ![]() ${\tilde{t}_{form}}$ and
${\tilde{t}_{form}}$ and ![]() ${\tilde{t}_{floa}}$ for formation and floating, respectively.
${\tilde{t}_{floa}}$ for formation and floating, respectively.
For large Bond numbers, we cannot refer to independent formation and floating as distinct processes, as shown in figure 7 for ![]() ${N_{Bo}} = \; {10^{ - 1}}$, where no droplets form and two well-separated regions grow from the top and the bottom at the expense of the central mixed region. For smaller Bond numbers, instead, we may speculate that formation and floating take place in series. In fact, while phase separation initially occurs on a small scale and does not depend on the presence of an external force, corresponding to the formation stage, the rise and progressive coalescence of droplets is mainly dictated by the external force field during the floating stage, eventually leading to complete segregation of the phases. We may also speculate that formation, being a process occurring locally and dictated by how far the system is from equilibrium, is independent of the domain dimension, namely the domain height
${N_{Bo}} = \; {10^{ - 1}}$, where no droplets form and two well-separated regions grow from the top and the bottom at the expense of the central mixed region. For smaller Bond numbers, instead, we may speculate that formation and floating take place in series. In fact, while phase separation initially occurs on a small scale and does not depend on the presence of an external force, corresponding to the formation stage, the rise and progressive coalescence of droplets is mainly dictated by the external force field during the floating stage, eventually leading to complete segregation of the phases. We may also speculate that formation, being a process occurring locally and dictated by how far the system is from equilibrium, is independent of the domain dimension, namely the domain height ![]() $\tilde{H}$, so that any dependence of the segregation time from the domain size has to be attributed to the floating time. Thus, it seems reasonable to assume that the segregation time can be obtained as the sum of the formation time and floating time, that is,
$\tilde{H}$, so that any dependence of the segregation time from the domain size has to be attributed to the floating time. Thus, it seems reasonable to assume that the segregation time can be obtained as the sum of the formation time and floating time, that is,
with the brackets denoting the dependence of a term from the domain height. This ansatz is critically investigated in this section by addressing first the formation time and then the floating time.
Since the formation refers to the emergence of droplets and their thermodynamic equilibration, it seems natural to use the separation depth, S, as a useful metric to mark the formation time. According to its definition in (3.1), the separation depth is a scalar quantity that measures the deviation from equilibrium locally in each point of the domain. To obtain a global descriptor of the formation within the domain, we adopt the integral average of the separation depth, ![]() $\langle S\rangle = (1/\tilde{H}\tilde{L})\int_\varOmega {S\,\textrm{d}\kern0.06em \tilde{x}\,\textrm{d}\tilde{z}} $. Figure 8 reports the average separation depth as a function of dimensionless time for different domain heights for two representative fluidity numbers at
$\langle S\rangle = (1/\tilde{H}\tilde{L})\int_\varOmega {S\,\textrm{d}\kern0.06em \tilde{x}\,\textrm{d}\tilde{z}} $. Figure 8 reports the average separation depth as a function of dimensionless time for different domain heights for two representative fluidity numbers at ![]() ${N_{Bo}} = {10^{ - 3}}$. The average separation depth increases with time in every case indicating that the system evolves towards equilibrium, albeit more slowly for
${N_{Bo}} = {10^{ - 3}}$. The average separation depth increases with time in every case indicating that the system evolves towards equilibrium, albeit more slowly for ![]() ${N_\alpha } = 0.01$ since system evolution is ruled by diffusion. Notably,
${N_\alpha } = 0.01$ since system evolution is ruled by diffusion. Notably, ![]() $\langle S\rangle $ never becomes equal to one in a finite system because, even though S = 1 within the bulk of the phases, the diffuse interface separating the two phases is characterized by a gradient of composition ϕ and, as such, by S < 1 (see figure 6). Nonetheless,
$\langle S\rangle $ never becomes equal to one in a finite system because, even though S = 1 within the bulk of the phases, the diffuse interface separating the two phases is characterized by a gradient of composition ϕ and, as such, by S < 1 (see figure 6). Nonetheless, ![]() $\langle S\rangle $ approaches an asymptotic maximum value at the end of the segregation. Figure 8 also shows that
$\langle S\rangle $ approaches an asymptotic maximum value at the end of the segregation. Figure 8 also shows that ![]() $\langle S\rangle $ is basically independent of the domain height
$\langle S\rangle $ is basically independent of the domain height ![]() $\tilde{H}$ since, for each value of
$\tilde{H}$ since, for each value of ![]() ${N_\alpha }$, all the curves tend to collapse one onto the others. This indicates that
${N_\alpha }$, all the curves tend to collapse one onto the others. This indicates that ![]() $\langle S\rangle $ contains information about the system pathway towards equilibrium without being affected by the domain size, which is exactly what the formation process and the formation time aim to mark, satisfying part of the ansatz in (3.2). As such,
$\langle S\rangle $ contains information about the system pathway towards equilibrium without being affected by the domain size, which is exactly what the formation process and the formation time aim to mark, satisfying part of the ansatz in (3.2). As such, ![]() $\langle S\rangle $ is adopted to identify the formation time
$\langle S\rangle $ is adopted to identify the formation time ![]() ${\tilde{t}_{form}}$.
${\tilde{t}_{form}}$.

Figure 8. Change in average separation depth over dimensionless time for different dimensionless domain heights for (a) Nα = 100, (b) Nα = 0.01, given ![]() ${N_{Bo}} = {10^{ - 3}}$.
${N_{Bo}} = {10^{ - 3}}$.
Despite setting a threshold value of ![]() $\langle S\rangle $ contains a certain degree of arbitrariness, an analysis of figure 6 at
$\langle S\rangle $ contains a certain degree of arbitrariness, an analysis of figure 6 at ![]() ${N_\alpha } = 100$ reveals that droplets are internally equilibrated (i.e. S = 1) at
${N_\alpha } = 100$ reveals that droplets are internally equilibrated (i.e. S = 1) at ![]() $\tilde{t} \approx 260$, which corresponds to
$\tilde{t} \approx 260$, which corresponds to ![]() $\langle S\rangle = 0.8$ in figure 8(a). Thus, we identify the formation time
$\langle S\rangle = 0.8$ in figure 8(a). Thus, we identify the formation time ![]() ${\tilde{t}_{form}}$ as the time at which
${\tilde{t}_{form}}$ as the time at which ![]() $\langle S\rangle $ equals 0.8, which is
$\langle S\rangle $ equals 0.8, which is ![]() ${\tilde{t}_{form}} \approx 260$ for
${\tilde{t}_{form}} \approx 260$ for ![]() ${N_\alpha } = 100$. Clearly, at the formation time,
${N_\alpha } = 100$. Clearly, at the formation time, ![]() $\langle S\rangle $ has not yet approached its asymptotic maximum value because the continuum blue phase is not equilibrated yet at
$\langle S\rangle $ has not yet approached its asymptotic maximum value because the continuum blue phase is not equilibrated yet at ![]() $\phi _e^{(A)} = 0.12$, as denoted by the colourmap in the last row of figure 6 at
$\phi _e^{(A)} = 0.12$, as denoted by the colourmap in the last row of figure 6 at ![]() ${N_{Bo}} = {10^{ - 3}}$. By using the same threshold
${N_{Bo}} = {10^{ - 3}}$. By using the same threshold ![]() $\langle S\rangle = 0.8$ in figure 8(b), we find
$\langle S\rangle = 0.8$ in figure 8(b), we find ![]() ${\tilde{t}_{form}} \approx 2000$ for
${\tilde{t}_{form}} \approx 2000$ for ![]() ${N_\alpha } = 0.01$, independent of the domain height, which consistently agrees with the internal equilibration of the droplets in figure 3 for
${N_\alpha } = 0.01$, independent of the domain height, which consistently agrees with the internal equilibration of the droplets in figure 3 for ![]() ${N_{Bo}} = {10^{ - 3}}$ by judging their colour which is qualitatively similar to that of the droplets at
${N_{Bo}} = {10^{ - 3}}$ by judging their colour which is qualitatively similar to that of the droplets at ![]() $\tilde{t} \approx 260$ in figure 3 at
$\tilde{t} \approx 260$ in figure 3 at ![]() ${N_\alpha } = 100$. Therefore, marking
${N_\alpha } = 100$. Therefore, marking ![]() ${\tilde{t}_{form}}$, as the time at which
${\tilde{t}_{form}}$, as the time at which ![]() $\langle S\rangle = 0.8$, appears to be a sensible choice to identify the formation process for any value of
$\langle S\rangle = 0.8$, appears to be a sensible choice to identify the formation process for any value of ![]() ${N_\alpha }$ investigated, while satisfying the requirement of
${N_\alpha }$ investigated, while satisfying the requirement of ![]() ${\tilde{t}_{form}}$ being independent of
${\tilde{t}_{form}}$ being independent of ![]() $\tilde{H}$.
$\tilde{H}$.
Given these premises, the analysis shifts to the identification of the floating time and its additivity to the formation time to give the segregation time, which is a means to test the validity of (3.2). For the same set of simulations discussed in figure 8, figure 9 reports the segregation time ![]() ${\tilde{t}_{segr}}$ as a function of the dimensionless domain height
${\tilde{t}_{segr}}$ as a function of the dimensionless domain height ![]() $\tilde{H}$ for
$\tilde{H}$ for ![]() ${N_\alpha = 100}$ and
${N_\alpha = 100}$ and ![]() ${N_\alpha } = 0.01$ at
${N_\alpha } = 0.01$ at ![]() ${N_{Bo}} = {10^{ - 3}}$. If formation and floating really take place in series according to (3.2), with
${N_{Bo}} = {10^{ - 3}}$. If formation and floating really take place in series according to (3.2), with ![]() ${\tilde{t}_{form}}$ being independent of the domain height as shown above (see figure 8), any dependence of the segregation time with
${\tilde{t}_{form}}$ being independent of the domain height as shown above (see figure 8), any dependence of the segregation time with ![]() $\tilde{H}$ will scale directly to the floating time
$\tilde{H}$ will scale directly to the floating time ![]() ${\tilde{t}_{floa}}$, with
${\tilde{t}_{floa}}$, with ![]() ${\tilde{t}_{form}}$ representing an offset of
${\tilde{t}_{form}}$ representing an offset of ![]() ${\tilde{t}_{segr}}$ versus
${\tilde{t}_{segr}}$ versus ![]() $\tilde{H}$. The results in figure 9 indicate that, for both high and low fluidity numbers, which denote convection-driven and diffusion-driven phase separation and segregation, the segregation time increases with the domain height following a fairly linear trend, indicated by the dashed lines. However, in figure 9(a), the offset, which should correspond to
$\tilde{H}$. The results in figure 9 indicate that, for both high and low fluidity numbers, which denote convection-driven and diffusion-driven phase separation and segregation, the segregation time increases with the domain height following a fairly linear trend, indicated by the dashed lines. However, in figure 9(a), the offset, which should correspond to ![]() ${\tilde{t}_{form}}$, is equal to 1200, which is much larger than
${\tilde{t}_{form}}$, is equal to 1200, which is much larger than ![]() ${\tilde{t}_{form}} \approx 260$ identified above at
${\tilde{t}_{form}} \approx 260$ identified above at ![]() ${N_\alpha } = 100$ from the analysis of the separation depth at the threshold
${N_\alpha } = 100$ from the analysis of the separation depth at the threshold ![]() $\langle S\rangle = 0.8$. By looking at the last row in figure 4, it is clear that at
$\langle S\rangle = 0.8$. By looking at the last row in figure 4, it is clear that at ![]() $\tilde{t} = 1200$, the formation is completed and the floating has already started. However, figure 9(b) indicates that the extrapolation of the linear trend gives a negative offset for
$\tilde{t} = 1200$, the formation is completed and the floating has already started. However, figure 9(b) indicates that the extrapolation of the linear trend gives a negative offset for ![]() ${N_\alpha } = 0.01$, which would mean a negative
${N_\alpha } = 0.01$, which would mean a negative ![]() ${\tilde{t}_{form}}$, which has no physical meaning.
${\tilde{t}_{form}}$, which has no physical meaning.

Figure 9. Segregation time as a function of dimensionless domain heights for (a) Nα = 100, (b) Nα = 0.01, given ![]() ${N_{Bo}} = {10^{ - 3}}$. The dashed lines denote the best linear fit of data according to the least square method.
${N_{Bo}} = {10^{ - 3}}$. The dashed lines denote the best linear fit of data according to the least square method.
Hence, both these observations indicate that formation and floating are not strictly processes occurring in series and the latter may not scale strictly linearly with the domain height, which is the scaling one would expect in case the external force would pull the droplets at a constant terminal velocity. More importantly, the analysis of data in figure 9 disproves (3.2), meaning that the segregation time cannot be decoupled as a simple sum of formation and floating times, neither for large Bond numbers for which (3.2) is obviously inapplicable (see figure 7) nor for relatively small Bond numbers (namely, ![]() ${N_{Bo}} = {10^{ - 3}}$ as shown here). We conclude that formation and floating take place partly in series and partly in parallel in a convoluted manner. This result is independent from the few arbitrary choices made above, such as marking
${N_{Bo}} = {10^{ - 3}}$ as shown here). We conclude that formation and floating take place partly in series and partly in parallel in a convoluted manner. This result is independent from the few arbitrary choices made above, such as marking ![]() ${\tilde{t}_{form}}$ at
${\tilde{t}_{form}}$ at ![]() $\langle S\rangle = 0.8$, as any other threshold value would not make (3.2) to hold.
$\langle S\rangle = 0.8$, as any other threshold value would not make (3.2) to hold.
Having assessed that, in general, the segregation time cannot be decoupled as a sum of formation and floating times, figure 10 illustrates the relationship between ![]() ${\tilde{t}_{segr}}$ and Bond number for various values of
${\tilde{t}_{segr}}$ and Bond number for various values of ![]() ${N_\alpha }$ at a given domain height. Numerical results show that
${N_\alpha }$ at a given domain height. Numerical results show that ![]() ${\tilde{t}_{segr}}$ decreases as the Bond number and fluidity number increase, due to the larger pull of the external force field promoting floating in the first case and the higher mobility of droplets in a convective regime in the second case. Nonetheless, universal quantitative trends do not clearly emerge from figure 10. For
${\tilde{t}_{segr}}$ decreases as the Bond number and fluidity number increase, due to the larger pull of the external force field promoting floating in the first case and the higher mobility of droplets in a convective regime in the second case. Nonetheless, universal quantitative trends do not clearly emerge from figure 10. For ![]() ${N_\alpha } \le 0.1$, a linear dependence with a slope of ca. 0.9 in the log-log plot appears, so that the segregation time follows a power scaling with Bond number as
${N_\alpha } \le 0.1$, a linear dependence with a slope of ca. 0.9 in the log-log plot appears, so that the segregation time follows a power scaling with Bond number as ![]() ${\tilde{t}_{segr}} \approx N_{Bo}^{ - 0.9}$, being independent of
${\tilde{t}_{segr}} \approx N_{Bo}^{ - 0.9}$, being independent of ![]() ${N_\alpha }$ the more
${N_\alpha }$ the more ![]() ${N_{Bo}}$ approaches 10−1. However, for
${N_{Bo}}$ approaches 10−1. However, for ![]() ${N_\alpha } \ge 1$, such a power-law scaling does not hold anymore, probably due to an increased influence of convection. In fact, as discussed in § 3.1, the dynamics of phase segregation in the presence of an external force becomes very rich and complex in the convection-dominated regime, with arguably synergic nonlinear phenomena that contribute to phase segregation. The reproducibility and consistency observed across different simulations provide confidence in the validity (or absence thereof) of the scaling, emphasizing the importance of considering the interplay between convection, diffusion, external force and other factors on phase segregation dynamics.
${N_\alpha } \ge 1$, such a power-law scaling does not hold anymore, probably due to an increased influence of convection. In fact, as discussed in § 3.1, the dynamics of phase segregation in the presence of an external force becomes very rich and complex in the convection-dominated regime, with arguably synergic nonlinear phenomena that contribute to phase segregation. The reproducibility and consistency observed across different simulations provide confidence in the validity (or absence thereof) of the scaling, emphasizing the importance of considering the interplay between convection, diffusion, external force and other factors on phase segregation dynamics.

Figure 10. Segregation time as a function of Bond number for different values of Nα, given the dimensionless height ![]() $\tilde{H} = 100$.
$\tilde{H} = 100$.
4. Conclusions
The phase field approach was applied in this work to conduct numerical simulations of phase segregation in a partially miscible binary mixture subjected to an external force field. The presence of an external force breaks the symmetry of phase separation: although the formation of droplets is rather unaffected by the force field, the following coalescence process is no longer isotropic under an external force, which causes the segregation of equilibrated phases separated by a single interface. The dynamics of phase segregation is orders of magnitude slower for fluidity numbers smaller than one (i.e. in diffusion regime), while system evolution becomes much faster and richer in morphological patterns when convection dominates for fluidity number of the order of 100. In all the cases, an increase in Bond number reduces the segregation time. For small Bond numbers (e.g. ![]() ${N_{Bo}} = {10^{ - 3}}$), it is shown that the formation process is independent of the domain height for all the fluidity numbers. Nonetheless, the segregation time increases with the domain height. In any case, formation and floating cannot be regarded as strictly serial processes, so that the segregation time cannot be decoupled as a simple sum of a formation and a floating time. This is also corroborated in the extreme condition of high Bond numbers
${N_{Bo}} = {10^{ - 3}}$), it is shown that the formation process is independent of the domain height for all the fluidity numbers. Nonetheless, the segregation time increases with the domain height. In any case, formation and floating cannot be regarded as strictly serial processes, so that the segregation time cannot be decoupled as a simple sum of a formation and a floating time. This is also corroborated in the extreme condition of high Bond numbers ![]() $({N_{Bo}} = {10^{ - 1}})$, when droplet formation does not occur and phase segregation takes place via stratification of equilibrated phases at the boundaries of the domain with two interfaces that move one towards the other while the central mixed region shrinks. A universal scaling between segregation time, Bond number and fluidity number is not possible, although a power-law dependence
$({N_{Bo}} = {10^{ - 1}})$, when droplet formation does not occur and phase segregation takes place via stratification of equilibrated phases at the boundaries of the domain with two interfaces that move one towards the other while the central mixed region shrinks. A universal scaling between segregation time, Bond number and fluidity number is not possible, although a power-law dependence ![]() ${\tilde{t}_{segr}} \approx N_{Bo}^{ - 0.9}$ is attained for
${\tilde{t}_{segr}} \approx N_{Bo}^{ - 0.9}$ is attained for ![]() ${N_\alpha } \le 0.1$.
${N_\alpha } \le 0.1$.
The study and its results promote a deeper and mechanistic understanding of phase segregation, fostering relevant applications where an external force field is imposed to expedite the phase separation process. Future studies will be dedicated to investigating the effects of oscillating force fields on phase separation dynamics.
Supplemental movie
Supplementary movie is available at https://doi.org/10.1017/jfm.2024.841.
Funding
This research received partial funding from the Headquarters of University Advancement at the National Cheng Kung University, which was sponsored by the Ministry of Education, Taiwan, and the National Science and Technology Council (NSTC), Taiwan, under grant No. 111-2221-E-006-102-MY3.
Declaration of interests
The authors report no conflict of interest.
Nomenclature
- a
characteristic length of the diffuse interface [m]
- b
external field magnitude [N kg−1]
- dx
characteristic droplet size along direction x [m]
- dz
characteristic droplet size along direction z [m]
- D
composition-independent diffusivity [m2 s−1]
- f
conservative force per unit mass [N kg−1]
 $H$
$H$domain height [m]
 $L$
$L$domain length [m]
- lc
capillary length [m]
- Jϕ
diffusive mass flux of species 1 [kg (m2 s)−1]
 ${N_{Bo}}$
${N_{Bo}}$Bond (or Eotvos) number [−]
- NSc
Schmidt number [−]
- Nα
fluidity number [−]
- p
pressure [Pa]
- R′
ideal gas constant per unit mass [J (kg K)−1]
- S
separation depth [−]
- t
time [s]
- T
absolute temperature [K]
- Tc
critical temperature of the mixture [K]
- v
mass-average fluid velocity [m s−1]
- x
x coordinate [m]
- x
vector of spatial coordinate [m]
- z
z coordinate [m]
Greek letters
 $\varepsilon $
$\varepsilon $random white noise [−]
- η
mixture dynamic viscosity [kg (m s)−1]
- μ 12
chemical potential difference [J kg−1]
- ρ
mixture density [kg m−3]
- ϕ
mass fraction of species 1 [−]
- χ 12
susceptibility difference [−]
 $\varPsi $
$\varPsi $Margules coefficient [−]
- ψ 12
potential energy difference [J kg−1]
- Ω
computational domain [−]
Superscripts
 $\wedge $
$\wedge $characteristic value of temperature and composition-dependent quantities
- ∼
physical quantity recast in dimensionless form
- A
continuum (remainder) phase
- B
droplet phase
- cons
conservative
- ext
external
- K
Korteweg
Subscripts
- e
equilibrium
- i
species i
- floa
floating
- form
formation
- segr
segregation
- 0
initial