Hostname: page-component-669899f699-7tmb6 Total loading time: 0 Render date: 2025-04-29T05:14:55.753Z Has data issue: false hasContentIssue false
  • English
  • Français

Ideals with componentwise linear powers

Part of: General commutative ring theory Computational aspects and applications Algebraic combinatorics

Published online by Cambridge University Press:  12 March 2024

Takayuki Hibi  and
Somayeh Moradi
Takayuki Hibi
Affiliation:
Department of Pure and Applied Mathematics, Graduate School of Information Science and Technology, Osaka University, Suita, Osaka 565-0871, Japan e-mail: [email protected]
Somayeh Moradi*
Affiliation:
Department of Mathematics, Faculty of Science, Ilam University, P.O.Box 69315-516, Ilam, Iran
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Let 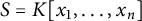$S=K[x_1,\ldots ,x_n]$ be the polynomial ring over a field K, and let A be a finitely generated standard graded S-algebra. We show that if the defining ideal of A has a quadratic initial ideal, then all the graded components of A are componentwise linear. Applying this result to the Rees ring 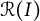$\mathcal {R}(I)$ of a graded ideal I gives a criterion on I to have componentwise linear powers. Moreover, for any given graph G, a construction on G is presented which produces graphs whose cover ideals 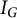$I_G$ have componentwise linear powers. This, in particular, implies that for any Cohen–Macaulay Cameron–Walker graph G all powers of $I_G$ have linear resolutions. Moreover, forming a cone on special graphs like unmixed chordal graphs, path graphs, and Cohen–Macaulay bipartite graphs produces cover ideals with componentwise linear powers.

Keywords

Rees algebracover ideal of a graphcomponentwise linear ideal

MSC classification

Primary: 13A02: Graded rings 13P10: Gröbner bases; other bases for ideals and modules (e.g., Janet and border bases)
Secondary: 05E40: Combinatorial aspects of commutative algebra
Type
Article
Information
Canadian Mathematical Bulletin , Volume 67 , Issue 3 , September 2024 , pp. 833 - 841
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press on behalf of Canadian Mathematical Society

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

Footnotes

Takayuki Hibi is partially supported by JSPS KAKENHI 19H00637. Somayeh Moradi is supported by the Alexander von Humboldt Foundation.

References

Aramova, A., Herzog, J., and Hibi, T., Ideals with stable Betti numbers . Adv. Math. 152(2000), 72–77.CrossRefGoogle Scholar
Erey, N., Powers of ideals associated to $\left({C}_4,2{K}_2\right)$ -free graphs . J. Pure Appl. Algebra 223(2019), no. 7, 3071–3080.CrossRefGoogle Scholar
Hà, H. T. and Van Tuyl, A., Powers of componentwise linear ideals: the Herzog–Hibi–Ohsugi conjecture and related problems . Res. Math. Sci. 9(2022), 22.CrossRefGoogle Scholar
Herzog, J. and Hibi, T., Componentwise linear ideals . Nagoya Math. J. 153(1999), 141–153.CrossRefGoogle Scholar
Herzog, J. and Hibi, T., Distributive lattices, bipartite graphs and Alexander duality . J. Algebraic Combin. 22(2005), 289–302.CrossRefGoogle Scholar
Herzog, J. and Hibi, T., Monomial ideals, Springer, London, 2011.CrossRefGoogle Scholar
Herzog, J., Hibi, T., and Moradi, S., Componentwise linear powers and the $x$ -condition . Math. Scand. 128(2022), 401–433.CrossRefGoogle Scholar
Herzog, J., Hibi, T., and Ohsugi, H., Powers of componentwise linear ideals . In: Fløystad, G., Johnsen, T., and Knutsen, A. (eds.), Combinatorial aspects of commutative algebra and algebraic geometry, Abel Symposia, 6, Springer, Berlin, 2011, pp. 49–60.CrossRefGoogle Scholar
Herzog, J., Hibi, T., and Ohsugi, H., Binomial ideals, Graduate Texts in Mathematics, 279, Springer, Cham, 2018.CrossRefGoogle Scholar
Herzog, J., Hibi, T., and Zheng, X., Monomial ideals whose powers have a linear resolution . Math. Scand. 95(2004), 23–32.CrossRefGoogle Scholar
Herzog, J., Reiner, V., and Welker, V., Componentwise linear ideals and Golod rings . Michigan Math. J. 46(1999), 211–223.CrossRefGoogle Scholar
Hibi, T., Higashitani, A., Kimura, K., and O’Keefe, A. B., Algebraic study on Cameron–Walker graphs . J. Algebra 422(2015), 257–269.CrossRefGoogle Scholar
Kumar, A. and Kumar, R., On the powers of vertex cover ideals . J. Pure. Appl. Algebra 226(2022), no. 1, 106808.CrossRefGoogle Scholar
Römer, T., On minimal graded free resolutions. Ph.D. dissertation, Universität Duisburg-Essen, 2001.Google Scholar
Yanagawa, K., Alexander duality for Stanley–Reisner rings and squarefree ${\mathbb{N}}^n$ -graded modules . J. Algebra 225(2000), 630–645.CrossRefGoogle Scholar