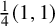1 Introduction
The analytic torsion, which is a certain combination of the determinants of Hodge Laplacians on differential forms, is an invariant of Riemannian manifolds defined by Ray and Singer [Reference Ray and Singer40] as an analytic analogue of the Reidemeister torsion, the first topological invariant that is not a homotopy invariant. It was proved independently by Cheeger [Reference Cheeger11] and Müller [Reference Müller38] that the analytic torsion and the Reidemeister torsion agree on closed manifolds (Ray-Singer conjecture). Ray and Singer [Reference Ray and Singer41] also introduced a version of the analytic torsion for complex manifolds, usually referred to as the holomorphic torsion. The holomorphic torsion has found significant applications in Arakelov theory, canonical metrics and mirror symmetry. Unlike its real analogue, it depends on the geometry and complex structure of the underlying complex manifold [Reference Bismut, Gillet and Soulé6] (the anomaly formula), which gives rise to interesting functions on moduli spaces. In this paper, we focus on this aspect of holomorphic torsion: that is, its connection with modular forms.
In fact, Ray and Singer already noticed the remarkable connection. Using Kronecker’s first limit formula, Ray and Singer [Reference Ray and Singer41] computed the analytic torsion for elliptic curves and found it to be given in terms of the Jacobi
![]() $\Delta $
-function, a modular form of weight
$\Delta $
-function, a modular form of weight
![]() $12$
on
$12$
on
![]() $\mathbf H/\textrm {SL}(2, \mathbf Z)$
. Their result has since then been extended to higher genus Riemann surfaces by Zograf [Reference Zograf51], McIntyre-Takhtajan [Reference McIntyre and Takhtajan35], Kokotov-Korotkin [Reference Kokotov and Korotkin28] and McIntyre-Park [Reference McIntyre and Park36]; Zograf and McIntyre-Takhtajan studied the analytic torsion of Riemann surfaces with respect to the hyperbolic metric, while Kokotov-Korotkin and McIntyre-Park studied it with respect to the (degenerate) flat metric attached to an abelian differential of the Riemann surface.
$\mathbf H/\textrm {SL}(2, \mathbf Z)$
. Their result has since then been extended to higher genus Riemann surfaces by Zograf [Reference Zograf51], McIntyre-Takhtajan [Reference McIntyre and Takhtajan35], Kokotov-Korotkin [Reference Kokotov and Korotkin28] and McIntyre-Park [Reference McIntyre and Park36]; Zograf and McIntyre-Takhtajan studied the analytic torsion of Riemann surfaces with respect to the hyperbolic metric, while Kokotov-Korotkin and McIntyre-Park studied it with respect to the (degenerate) flat metric attached to an abelian differential of the Riemann surface.
In dimension two, motivated by string duality, the second author [Reference Yoshikawa43] studied the case of
![]() $2$
-elementary
$2$
-elementary
![]() $K3$
surfaces – that is, pairs consisting of a
$K3$
surfaces – that is, pairs consisting of a
![]() $K3$
surface X and a holomorphic involution
$K3$
surface X and a holomorphic involution
![]() $\iota :\, X\longrightarrow X$
(acting nontrivially on holomorphic two forms) – and introduced an (equivariant) holomorphic torsion invariant for those surfaces. By the global Torelli theorem for
$\iota :\, X\longrightarrow X$
(acting nontrivially on holomorphic two forms) – and introduced an (equivariant) holomorphic torsion invariant for those surfaces. By the global Torelli theorem for
![]() $K3$
surfaces, the moduli space of 2-elementary K3 surfaces of fixed topological type is a modular variety of orthogonal type, so the holomorphic torsion invariant is viewed as a function on such modular varieties. On orthogonal modular varieties, Borcherds [Reference Borcherds8] constructed a class of automorphic forms with remarkable properties as singular theta lifts of elliptic modular forms. These automorphic forms are called Borcherds products. It is shown that the holomorphic torsion invariant of
$K3$
surfaces, the moduli space of 2-elementary K3 surfaces of fixed topological type is a modular variety of orthogonal type, so the holomorphic torsion invariant is viewed as a function on such modular varieties. On orthogonal modular varieties, Borcherds [Reference Borcherds8] constructed a class of automorphic forms with remarkable properties as singular theta lifts of elliptic modular forms. These automorphic forms are called Borcherds products. It is shown that the holomorphic torsion invariant of
![]() $2$
-elementary
$2$
-elementary
![]() $K3$
surfaces is given by the Petersson norm of a certain series of Borcherds products [Reference Yoshikawa45], [Reference Ma and Yoshikawa30].
$K3$
surfaces is given by the Petersson norm of a certain series of Borcherds products [Reference Yoshikawa45], [Reference Ma and Yoshikawa30].
If
![]() $\iota $
is fixed point free, then the quotient
$\iota $
is fixed point free, then the quotient
![]() $Y=X/\iota $
is an Enriques surface whose holomorphic torsion invariant is given by one of the most remarkable Borcherds products, the Borcherds
$Y=X/\iota $
is an Enriques surface whose holomorphic torsion invariant is given by one of the most remarkable Borcherds products, the Borcherds
![]() $\Phi $
-function of rank
$\Phi $
-function of rank
![]() $10$
. In this paper, we extend this result to a class of singular rational surfaces called log-Enriques surfaces introduced by D.-Q. Zhang [Reference Zhang47]. As in the case of Enriques surfaces, a log-Enriques surface Y is expressed as a quotient
$10$
. In this paper, we extend this result to a class of singular rational surfaces called log-Enriques surfaces introduced by D.-Q. Zhang [Reference Zhang47]. As in the case of Enriques surfaces, a log-Enriques surface Y is expressed as a quotient
![]() $Y=X/\iota $
, where X is a
$Y=X/\iota $
, where X is a
![]() $K3$
surface with rational double points called the canonical covering of Y, and
$K3$
surface with rational double points called the canonical covering of Y, and
![]() $\iota $
is an anti-symplectic involution on X free from fixed points outside the singular points. To be precise, our log-Enriques surfaces are those of index two in the sense of Zhang [Reference Zhang47]. To obtain a nice moduli space, we restrict ourselves to the case where X has only nodes as its singular points. A log-Enriques surface with this property is called good in this paper. Then a good log-Enriques surface can admit at most
$\iota $
is an anti-symplectic involution on X free from fixed points outside the singular points. To be precise, our log-Enriques surfaces are those of index two in the sense of Zhang [Reference Zhang47]. To obtain a nice moduli space, we restrict ourselves to the case where X has only nodes as its singular points. A log-Enriques surface with this property is called good in this paper. Then a good log-Enriques surface can admit at most
![]() $10$
singular points, any of which is a cyclic quotient singularity of type
$10$
singular points, any of which is a cyclic quotient singularity of type
![]() $\frac {1}{4}(1,1)$
. It turns out that the moduli space of good log-Enriques surfaces of k singular points is again a Zariski open subset of a modular variety of orthogonal type of dimension
$\frac {1}{4}(1,1)$
. It turns out that the moduli space of good log-Enriques surfaces of k singular points is again a Zariski open subset of a modular variety of orthogonal type of dimension
![]() $10-k$
attached to a unimodular lattice of signature
$10-k$
attached to a unimodular lattice of signature
![]() $(2,10-k)$
. Let us write
$(2,10-k)$
. Let us write
![]() ${\mathcal M}_{k}$
for this modular variety. When
${\mathcal M}_{k}$
for this modular variety. When
![]() $k=8$
, we have
$k=8$
, we have
![]() ${\mathcal M}_{8}^{\textrm {odd}}$
and
${\mathcal M}_{8}^{\textrm {odd}}$
and
![]() ${\mathcal M}_{8}^{\textrm {even}}$
, according to the parity of the unimodular lattice of signature
${\mathcal M}_{8}^{\textrm {even}}$
, according to the parity of the unimodular lattice of signature
![]() $(2,2)$
. For simplicity, we write
$(2,2)$
. For simplicity, we write
![]() ${\mathcal M}_{8}$
for
${\mathcal M}_{8}$
for
![]() ${\mathcal M}_{8}^{\textrm {odd}}$
and
${\mathcal M}_{8}^{\textrm {odd}}$
and
![]() ${\mathcal M}_{8}^{\textrm {even}}$
when there is no possibility of confusion. For a good log-Enriques surface Y with k singular points, we write
${\mathcal M}_{8}^{\textrm {even}}$
when there is no possibility of confusion. For a good log-Enriques surface Y with k singular points, we write
![]() ${\varpi }(Y)\in {\mathcal M}_k$
for the isomorphism class of Y. Interestingly enough,
${\varpi }(Y)\in {\mathcal M}_k$
for the isomorphism class of Y. Interestingly enough,
![]() ${\mathcal M}_{k}$
can be identified with a Zariski open subset of the Kähler moduli of a Del Pezzo surface V of degree
${\mathcal M}_{k}$
can be identified with a Zariski open subset of the Kähler moduli of a Del Pezzo surface V of degree
![]() $\deg V = k$
, the modular variety given by
$\deg V = k$
, the modular variety given by
![]() $\mathcal {KM}(V)=\Omega _{H(V, {\mathbf Z})}/O^{+}(H(V, {\mathbf Z}))$
, where
$\mathcal {KM}(V)=\Omega _{H(V, {\mathbf Z})}/O^{+}(H(V, {\mathbf Z}))$
, where
![]() $H(V, {\mathbf Z})$
is the total cohomology lattice of V,
$H(V, {\mathbf Z})$
is the total cohomology lattice of V,
![]() $O^{+}(H(V, {\mathbf Z}))$
is its automorphism group and
$O^{+}(H(V, {\mathbf Z}))$
is its automorphism group and
![]() $\Omega _{H(V, {\mathbf Z})}$
is the domain of type IV attached to
$\Omega _{H(V, {\mathbf Z})}$
is the domain of type IV attached to
![]() $H(V, {\mathbf Z})$
. (See Theorem 2.10.)
$H(V, {\mathbf Z})$
. (See Theorem 2.10.)
Analogously to the Enriques lattice, the Del Pezzo lattice
![]() $H(V, {\mathbf Z})$
admits a reflective modular form
$H(V, {\mathbf Z})$
admits a reflective modular form
![]() $\Phi _{V}$
on
$\Phi _{V}$
on
![]() $\Omega _{H(V, {\mathbf Z})}$
for
$\Omega _{H(V, {\mathbf Z})}$
for
![]() $O^{+}(H(V, {\mathbf Z}))$
of weight
$O^{+}(H(V, {\mathbf Z}))$
of weight
![]() $\deg V+4$
, which is nowhere vanishing on the Zariski open subset corresponding to
$\deg V+4$
, which is nowhere vanishing on the Zariski open subset corresponding to
![]() ${\mathcal M}_{k}$
and characterises the Heegner divisor of norm
${\mathcal M}_{k}$
and characterises the Heegner divisor of norm
![]() $(-1)$
-vectors [Reference Yoshikawa44]. In addition,
$(-1)$
-vectors [Reference Yoshikawa44]. In addition,
![]() $\Phi _{V}$
is the denominator function of a generalised Kac-Moody algebra with explicit Fourier series expansion by Gritsenko and Nikulin [Reference Gritsenko22], [Reference Gritsenko and Nikulin23]. (See Section 8 for more about
$\Phi _{V}$
is the denominator function of a generalised Kac-Moody algebra with explicit Fourier series expansion by Gritsenko and Nikulin [Reference Gritsenko22], [Reference Gritsenko and Nikulin23]. (See Section 8 for more about
![]() $\Phi _{V}$
.)
$\Phi _{V}$
.)
On the other hand, even though they are rational surfaces, every log-Enriques surface Y admits a Ricci flat Kähler orbifold metric [Reference Kobayash27]. Let
![]() $\tau (Y)$
denote the analytic torsion of Y in the sense of X. Ma [Reference Ma32] (suitably normalised by volume; see Section 8.1, especially Theorem 8.3 and Theorem 8.4 for the precise definition). Then our main result says that
$\tau (Y)$
denote the analytic torsion of Y in the sense of X. Ma [Reference Ma32] (suitably normalised by volume; see Section 8.1, especially Theorem 8.3 and Theorem 8.4 for the precise definition). Then our main result says that
![]() $\tau (Y)$
is given by some power of the Petersson norm of the Borcherds product
$\tau (Y)$
is given by some power of the Petersson norm of the Borcherds product
![]() $\Phi _{V}$
.
$\Phi _{V}$
.
Theorem 1.1. There exists a constant
![]() $C_{k}>0$
depending only on k such that for every good log-Enriques surface Y with k singular points,
$C_{k}>0$
depending only on k such that for every good log-Enriques surface Y with k singular points,
where V is a Del Pezzo surface of degree k.
It is important to note that our torsion invariant is essentially the complex
![]() $2$
-dimensional analogue of the BCOV invariant (See [Reference Bershadsky, Cecotti, Ooguri and Vafa3], [Reference Fang, Lu and Yoshikawa19], [Reference Eriksson, Freixas i Montplet and Mourougane17], [Reference Fu and Zhang20]). In higher dimensions, Bershadsky, Cecotti, Ooguri and Vafa [Reference Bershadsky, Cecotti, Ooguri and Vafa3] introduced a certain combination of holomorphic torsions, called the BCOV torsion, and predicted the mirror symmetry at genus one as an equivalence of the BCOV torsion and certain curve counting invariants at genus one. The corresponding holomorphic torsion invariant of Calabi-Yau threefolds, called the BCOV invariant, was introduced by Fang, Lu and the second author [Reference Fang, Lu and Yoshikawa19], who verified some predictions in [Reference Bershadsky, Cecotti, Ooguri and Vafa3]. Very recently, the BCOV invariant is extended to Calabi-Yau manifolds of arbitrary dimension by Eriksson, Freixas i Montplet and Mourougane [Reference Eriksson, Freixas i Montplet and Mourougane17], who have established the mirror symmetry at genus one for the Dwork family in arbitrary dimension [Reference Eriksson, Freixas i Montplet and Mourougane18]. The notion of the BCOV invariant is further extended to a certain class of pairs by Y. Zhang [Reference Zhang49], who, together with L. Fu, has established the birational invariance of the BCOV invariants [Reference Zhang50], [Reference Fu and Zhang20]. According to mirror symmetry, the BCOV invariants correspond to the topological string amplitudes whose modular properties are important features. In the final section, we will interpret Theorem 1.1 in terms of the BCOV torsion so that the BCOV invariant of good log-Enriques surfaces is expressed as the Borcherds product
$2$
-dimensional analogue of the BCOV invariant (See [Reference Bershadsky, Cecotti, Ooguri and Vafa3], [Reference Fang, Lu and Yoshikawa19], [Reference Eriksson, Freixas i Montplet and Mourougane17], [Reference Fu and Zhang20]). In higher dimensions, Bershadsky, Cecotti, Ooguri and Vafa [Reference Bershadsky, Cecotti, Ooguri and Vafa3] introduced a certain combination of holomorphic torsions, called the BCOV torsion, and predicted the mirror symmetry at genus one as an equivalence of the BCOV torsion and certain curve counting invariants at genus one. The corresponding holomorphic torsion invariant of Calabi-Yau threefolds, called the BCOV invariant, was introduced by Fang, Lu and the second author [Reference Fang, Lu and Yoshikawa19], who verified some predictions in [Reference Bershadsky, Cecotti, Ooguri and Vafa3]. Very recently, the BCOV invariant is extended to Calabi-Yau manifolds of arbitrary dimension by Eriksson, Freixas i Montplet and Mourougane [Reference Eriksson, Freixas i Montplet and Mourougane17], who have established the mirror symmetry at genus one for the Dwork family in arbitrary dimension [Reference Eriksson, Freixas i Montplet and Mourougane18]. The notion of the BCOV invariant is further extended to a certain class of pairs by Y. Zhang [Reference Zhang49], who, together with L. Fu, has established the birational invariance of the BCOV invariants [Reference Zhang50], [Reference Fu and Zhang20]. According to mirror symmetry, the BCOV invariants correspond to the topological string amplitudes whose modular properties are important features. In the final section, we will interpret Theorem 1.1 in terms of the BCOV torsion so that the BCOV invariant of good log-Enriques surfaces is expressed as the Borcherds product
![]() $\Phi _{V}$
, an infinite product of expected type in mirror symmetry. As log-Enriques surfaces are rational, the BCOV invariant is not a birational invariant in this case.
$\Phi _{V}$
, an infinite product of expected type in mirror symmetry. As log-Enriques surfaces are rational, the BCOV invariant is not a birational invariant in this case.
We remark that the equivalence of the analytic torsion of Ricci flat Enriques surfaces and the Borcherds
![]() $\Phi $
-function [Reference Yoshikawa43] may be viewed as the limiting case
$\Phi $
-function [Reference Yoshikawa43] may be viewed as the limiting case
![]() $k=0$
. Since
$k=0$
. Since
![]() $\tau (Y)$
is the analytic torsion of a resolution of Y with respect to a degenerate Ricci flat metric, our theorem may be viewed as a two-dimensional analogue of the theorems of Kokotov-Korotkin [Reference Kokotov and Korotkin28] and McIntyre-Park [Reference McIntyre and Park36], as mentioned above. Because of the isomorphism between the complex structure moduli of good log-Enriques surfaces and the Kähler moduli of Del Pezzo surfaces, in view of mirror symmetry at genus one as mentioned above, it may be worth asking if the Fourier coefficients of the elliptic modular form appearing in the infinite product expansion of
$\tau (Y)$
is the analytic torsion of a resolution of Y with respect to a degenerate Ricci flat metric, our theorem may be viewed as a two-dimensional analogue of the theorems of Kokotov-Korotkin [Reference Kokotov and Korotkin28] and McIntyre-Park [Reference McIntyre and Park36], as mentioned above. Because of the isomorphism between the complex structure moduli of good log-Enriques surfaces and the Kähler moduli of Del Pezzo surfaces, in view of mirror symmetry at genus one as mentioned above, it may be worth asking if the Fourier coefficients of the elliptic modular form appearing in the infinite product expansion of
![]() $\Phi _{V}$
are interpreted as some counting invariants of Del Pezzo surfaces. We also remark that by Theorem 1.1 and the recent result of S. Ma [Reference Ma29], the analytic torsion of good log-Enriques surfaces is obtained from the Borcherds
$\Phi _{V}$
are interpreted as some counting invariants of Del Pezzo surfaces. We also remark that by Theorem 1.1 and the recent result of S. Ma [Reference Ma29], the analytic torsion of good log-Enriques surfaces is obtained from the Borcherds
![]() $\Phi $
-function of rank
$\Phi $
-function of rank
![]() $10$
by manipulating quasi-pullbacks successively. See Section 8.3 for the details.
$10$
by manipulating quasi-pullbacks successively. See Section 8.3 for the details.
Our method of proof, which should have independent interest and which carries out the program proposed in [Reference Yoshikawa44, Question 5.18] for
![]() $2$
-elementary
$2$
-elementary
![]() $K3$
surfaces, is to de-singularise the double covering of Y via the Eguchi-Hanson instanton to obtain a
$K3$
surfaces, is to de-singularise the double covering of Y via the Eguchi-Hanson instanton to obtain a
![]() $2$
-elementary
$2$
-elementary
![]() $K3$
surface
$K3$
surface
![]() $(\widetilde {X},\theta )$
and study the limiting behaviour of the (equivariant) analytic torsion of
$(\widetilde {X},\theta )$
and study the limiting behaviour of the (equivariant) analytic torsion of
![]() $(\widetilde {X},\theta )$
, as well as other constituents of the invariant
$(\widetilde {X},\theta )$
, as well as other constituents of the invariant
![]() $\tau (\widetilde {X}, \theta )$
of
$\tau (\widetilde {X}, \theta )$
of
![]() $(\widetilde {X}, \theta )$
, as
$(\widetilde {X}, \theta )$
, as
![]() $\widetilde {X}$
degenerates into the orbifold double covering X of Y. As a result, the ratio
$\widetilde {X}$
degenerates into the orbifold double covering X of Y. As a result, the ratio
![]() $\tau (Y)/\tau (\widetilde {X}, \theta )^{1/2}$
may be viewed as the (equivariant) analytic torsion of the Eguchi-Hanson instanton (compare Theorem 7.12). In [Reference Bismut5], Bismut computed the behaviour of Quillen metrics when the exceptional divisor is blown down to a smooth point. In this paper, we study the same type of problem, where the blowing-up of
$\tau (Y)/\tau (\widetilde {X}, \theta )^{1/2}$
may be viewed as the (equivariant) analytic torsion of the Eguchi-Hanson instanton (compare Theorem 7.12). In [Reference Bismut5], Bismut computed the behaviour of Quillen metrics when the exceptional divisor is blown down to a smooth point. In this paper, we study the same type of problem, where the blowing-up of
![]() ${\mathbf C}^{2}$
will be replaced by the Eguchi-Hanson instanton. We remark that Theorem 1.1 would be proved in the same way as in [Reference Yoshikawa43] by making use of the fundamental theorems for Quillen metrics such as the curvature formula, anomaly formula and embedding formula [Reference Bismut4], [Reference Bismut, Gillet and Soulé6], [Reference Bismut and Lebeau7], [Reference Ma31], whose extensions to orbifolds were obtained by X. Ma [Reference Ma32], [Reference Ma33], if we could understand degenerations of log Enriques surfaces. On the other hand, it would be difficult to understand the geometric meaning of the ratio
${\mathbf C}^{2}$
will be replaced by the Eguchi-Hanson instanton. We remark that Theorem 1.1 would be proved in the same way as in [Reference Yoshikawa43] by making use of the fundamental theorems for Quillen metrics such as the curvature formula, anomaly formula and embedding formula [Reference Bismut4], [Reference Bismut, Gillet and Soulé6], [Reference Bismut and Lebeau7], [Reference Ma31], whose extensions to orbifolds were obtained by X. Ma [Reference Ma32], [Reference Ma33], if we could understand degenerations of log Enriques surfaces. On the other hand, it would be difficult to understand the geometric meaning of the ratio
![]() $\tau (Y)/\tau (\widetilde {X}, \theta )^{1/2}$
by this method. In the final section, we will observe that
$\tau (Y)/\tau (\widetilde {X}, \theta )^{1/2}$
by this method. In the final section, we will observe that
![]() $\tau (Y)/\tau (\widetilde {X}, \theta )^{1/2}$
is the key factor in the exact comparison formula for the BCOV invariants for certain Calabi-Yau orbifolds.
$\tau (Y)/\tau (\widetilde {X}, \theta )^{1/2}$
is the key factor in the exact comparison formula for the BCOV invariants for certain Calabi-Yau orbifolds.
This paper is organised as follows.
In Section 2, we recall log-Enriques surfaces and study their moduli space. In Section 3, we recall the notion of analytic torsion and also the holomorphic torsion invariant
![]() $\tau (\widetilde {X}, \theta )$
for
$\tau (\widetilde {X}, \theta )$
for
![]() $2$
-elementary
$2$
-elementary
![]() $K3$
surfaces [Reference Yoshikawa43]. In Theorem 3.2, we will give an explicit formula for the analytic torsion of a
$K3$
surfaces [Reference Yoshikawa43]. In Theorem 3.2, we will give an explicit formula for the analytic torsion of a
![]() $K3$
surface with respect to an arbitrary Kähler metric. In Section 4, we recall the Eguchi-Hanson instanton and construct a family of Kähler metrics
$K3$
surface with respect to an arbitrary Kähler metric. In Section 4, we recall the Eguchi-Hanson instanton and construct a family of Kähler metrics
![]() $\{ \gamma _{\epsilon ,\delta } \}$
on
$\{ \gamma _{\epsilon ,\delta } \}$
on
![]() $\widetilde {X}$
converging to an orbifold metric with uniformly bounded Ricci curvature. In Section 5, we study the behaviour of some constituents of the invariant
$\widetilde {X}$
converging to an orbifold metric with uniformly bounded Ricci curvature. In Section 5, we study the behaviour of some constituents of the invariant
![]() $\tau (\widetilde {X}, \theta )$
with respect to the metric
$\tau (\widetilde {X}, \theta )$
with respect to the metric
![]() $\gamma _{\epsilon ,\delta }$
as
$\gamma _{\epsilon ,\delta }$
as
![]() $\epsilon \to 0$
. In Section 6, we derive some estimates for the heat kernels of
$\epsilon \to 0$
. In Section 6, we derive some estimates for the heat kernels of
![]() $(\widetilde {X}, \gamma _{\epsilon ,\delta })$
. In Section 7, we determine the behaviour of (equivariant) analytic torsion of
$(\widetilde {X}, \gamma _{\epsilon ,\delta })$
. In Section 7, we determine the behaviour of (equivariant) analytic torsion of
![]() $(\widetilde {X}, \theta )$
with respect to the metric
$(\widetilde {X}, \theta )$
with respect to the metric
![]() $\gamma _{\epsilon ,\delta }$
as
$\gamma _{\epsilon ,\delta }$
as
![]() $\epsilon \to 0$
and
$\epsilon \to 0$
and
![]() $\delta \to 0$
. In Section 8, we introduce a holomorphic torsion invariant for good log-Enriques surfaces and prove the main theorem. In Section 9, we study the relation between the invariant
$\delta \to 0$
. In Section 8, we introduce a holomorphic torsion invariant for good log-Enriques surfaces and prove the main theorem. In Section 9, we study the relation between the invariant
![]() $\tau (Y)$
and the BCOV invariant.
$\tau (Y)$
and the BCOV invariant.
2 log-Enriques surfaces
2.1 log-Enriques surfaces
Following D.-Q. Zhang [Reference Zhang47], [Reference Zhang48], we recall the notion of log-Enriques surfaces (of index
![]() $2$
) and its basic properties.
$2$
) and its basic properties.
Definition 2.1. An irreducible normal projective complex surface Y is called a log-Enriques surface if the following conditions are satisfied:
-
(1) Y is singular and has at most quotient singularities except rational double points. In particular, Y has the structure of a compact complex orbifold.
-
(2) The irregularity of Y vanishes: that is,
 $H^{1}(Y,{\mathcal O}_{Y})=0$
.
$H^{1}(Y,{\mathcal O}_{Y})=0$
. -
(3) Let
 $K_{Y}$
be the canonical line bundle of Y in the sense of orbifolds. Then
$K_{Y}$
be the canonical line bundle of Y in the sense of orbifolds. Then  $$ \begin{align*}K_{Y}\not\cong{\mathcal O}_{Y}, \qquad K_{Y}^{\otimes2}={\mathcal O}_{Y}. \end{align*} $$
$$ \begin{align*}K_{Y}\not\cong{\mathcal O}_{Y}, \qquad K_{Y}^{\otimes2}={\mathcal O}_{Y}. \end{align*} $$
Remark 2.2. For
![]() ${\mathfrak p} \in \textrm {Sing}\,Y$
, there exist a neighbourhood
${\mathfrak p} \in \textrm {Sing}\,Y$
, there exist a neighbourhood
![]() $U_{\mathfrak p}$
of
$U_{\mathfrak p}$
of
![]() ${\mathfrak p}$
in Y, a finite group
${\mathfrak p}$
in Y, a finite group
![]() $G_{\mathfrak p} \subset \textrm {GL}({\mathbf C}^{2})$
and a
$G_{\mathfrak p} \subset \textrm {GL}({\mathbf C}^{2})$
and a
![]() $G_{\mathfrak p}$
-invariant neighbourhood V of
$G_{\mathfrak p}$
-invariant neighbourhood V of
![]() $0$
in
$0$
in
![]() ${\mathbf C}^{2}$
such that
${\mathbf C}^{2}$
such that
![]() $(U_{\mathfrak p}, {\mathfrak p}) \cong (V/G_{\mathfrak p}, 0)$
. Then
$(U_{\mathfrak p}, {\mathfrak p}) \cong (V/G_{\mathfrak p}, 0)$
. Then
![]() $K_{Y}|_{U_{\mathfrak p}}$
is defined as
$K_{Y}|_{U_{\mathfrak p}}$
is defined as
![]() $(V \times {\mathbf C})/G_{\mathfrak p}$
, where the
$(V \times {\mathbf C})/G_{\mathfrak p}$
, where the
![]() $G_{\mathfrak p}$
-action is given by
$G_{\mathfrak p}$
-action is given by
![]() $g\cdot (z,\zeta ) = (g\cdot z, \det (g)\zeta )$
.
$g\cdot (z,\zeta ) = (g\cdot z, \det (g)\zeta )$
.
Remark 2.3. Logarithmic Enriques surfaces in this paper are those of index two in Zhang’s papers [Reference Zhang47], [Reference Zhang48]. We only deal with log-Enriques surfaces of index two in this paper.
If a smooth complex surface satisfies conditions (2), (3), then it is an Enriques surface. For this reason, we impose that log-Enriques surfaces are singular. Then a log-Enriques surface is rational [Reference Zhang47, Lemma 3.4]. By Zhang [Reference Zhang47, Lemma 3.1], every singularity of a log-Enriques surface Y is the quotient of a rational double point by
![]() ${\mathbf Z}/2{\mathbf Z}$
and hence non-Gorenstein. Indeed, if
${\mathbf Z}/2{\mathbf Z}$
and hence non-Gorenstein. Indeed, if
![]() ${\mathfrak p}\in \textrm {Sing}\,Y$
, then there exists by (1) an isomorphism of germs of analytic spaces
${\mathfrak p}\in \textrm {Sing}\,Y$
, then there exists by (1) an isomorphism of germs of analytic spaces
![]() $(Y,{\mathfrak p})\cong ({\mathbf C}^{2}/G,0)$
, where
$(Y,{\mathfrak p})\cong ({\mathbf C}^{2}/G,0)$
, where
![]() $G\subset \textrm {GL}({\mathbf C}^{2})$
is a finite group. By (3), the image of the homomorphism
$G\subset \textrm {GL}({\mathbf C}^{2})$
is a finite group. By (3), the image of the homomorphism
![]() $\det \colon G\ni g\to \det g\in {\mathbf C}^{*}$
is
$\det \colon G\ni g\to \det g\in {\mathbf C}^{*}$
is
![]() $\pm 1$
. If
$\pm 1$
. If
![]() $G_{0}:=\ker \det \subset G$
, then
$G_{0}:=\ker \det \subset G$
, then
![]() $G_{0}\subset \textrm {SL}({\mathbf C}^{2})$
is a normal subgroup of G of index
$G_{0}\subset \textrm {SL}({\mathbf C}^{2})$
is a normal subgroup of G of index
![]() $2$
, so that
$2$
, so that
![]() $(X,0)=({\mathbf C}^{2}/G_{0},0)$
is a rational double point. If
$(X,0)=({\mathbf C}^{2}/G_{0},0)$
is a rational double point. If
![]() $p\colon (X,0)\to (Y,0)$
denotes the projection induced by the inclusion of groups
$p\colon (X,0)\to (Y,0)$
denotes the projection induced by the inclusion of groups
![]() $G_{0}\subset G$
, then p induces an isomorphism of germs
$G_{0}\subset G$
, then p induces an isomorphism of germs
![]() $(X/(G/G_{0}),0)\to (Y,0)$
, where
$(X/(G/G_{0}),0)\to (Y,0)$
, where
![]() $G/G_{0}\cong \{\pm 1\}\cong {\mathbf Z}/2{\mathbf Z}$
. By [Reference Zhang47, Lemma 3.1],
$G/G_{0}\cong \{\pm 1\}\cong {\mathbf Z}/2{\mathbf Z}$
. By [Reference Zhang47, Lemma 3.1],
![]() $(X, 0)$
is a rational double point of type
$(X, 0)$
is a rational double point of type
![]() $A_{2n-1}$
for some n. Since the homomorphism
$A_{2n-1}$
for some n. Since the homomorphism
![]() $\det ^{2} \colon G \to {\mathbf C}^{*}$
is trivial,
$\det ^{2} \colon G \to {\mathbf C}^{*}$
is trivial,
![]() $K_{Y}^{\otimes 2}$
is a holomorphic line bundle on Y in the ordinary sense.
$K_{Y}^{\otimes 2}$
is a holomorphic line bundle on Y in the ordinary sense.
2.2 The canonical double covering
Let Y be a log-Enriques surface, and let
![]() $\varXi \in H^{0}(Y,K_{Y}^{\otimes 2})\setminus \{0\}$
be a nowhere vanishing bicanonical form on Y in the sense of orbifolds. The canonical double covering of Y is defined as
$\varXi \in H^{0}(Y,K_{Y}^{\otimes 2})\setminus \{0\}$
be a nowhere vanishing bicanonical form on Y in the sense of orbifolds. The canonical double covering of Y is defined as
which is equipped with the projection
![]() $p\colon X\to Y$
induced from the projection
$p\colon X\to Y$
induced from the projection
![]() $K_{Y}\to Y$
. Then
$K_{Y}\to Y$
. Then
![]() $p\colon X\to Y$
is a double covering, which ramifies only over
$p\colon X\to Y$
is a double covering, which ramifies only over
![]() $\textrm {Sing}\,Y$
. (Since
$\textrm {Sing}\,Y$
. (Since
![]() $K_{Y, {\mathfrak p}} = {\mathbf C}/\pm 1$
for
$K_{Y, {\mathfrak p}} = {\mathbf C}/\pm 1$
for
![]() ${\mathfrak p} \in \textrm {Sing}(Y)$
,
${\mathfrak p} \in \textrm {Sing}(Y)$
,
![]() $p^{-1}({\mathfrak p})$
consists of a single point.) The canonical involution
$p^{-1}({\mathfrak p})$
consists of a single point.) The canonical involution
![]() $\iota \colon X\to X$
is defined as the nontrivial covering transformation:
$\iota \colon X\to X$
is defined as the nontrivial covering transformation:
Since the ramification locus of
![]() $p \colon X \to Y$
is
$p \colon X \to Y$
is
![]() $\textrm {Sing}\,X$
, we have
$\textrm {Sing}\,X$
, we have
![]() $X^{\iota } = \textrm {Sing}\,X$
and that
$X^{\iota } = \textrm {Sing}\,X$
and that
![]() $\iota $
has no fixed points on
$\iota $
has no fixed points on
![]() $X\setminus \textrm {Sing}\,X$
.
$X\setminus \textrm {Sing}\,X$
.
Let
![]() $\pi \colon \widetilde {X}\to X$
be the minimal resolution, and let
$\pi \colon \widetilde {X}\to X$
be the minimal resolution, and let
![]() ${\theta }\colon \widetilde {X}\to \widetilde {X}$
be the involution induced by the canonical involution
${\theta }\colon \widetilde {X}\to \widetilde {X}$
be the involution induced by the canonical involution
![]() $\iota $
. The involution
$\iota $
. The involution
![]() $\theta $
is also called the canonical involution on
$\theta $
is also called the canonical involution on
![]() $\widetilde {X}$
. We have the following commutative diagram:
$\widetilde {X}$
. We have the following commutative diagram:

Here the projection
![]() $p\colon X\to Y$
ramifies only at
$p\colon X\to Y$
ramifies only at
![]() $\textrm {Sing}\,Y$
. In what follows, we denote by
$\textrm {Sing}\,Y$
. In what follows, we denote by
![]() $X^{\iota }$
and
$X^{\iota }$
and
![]() $\widetilde {X}^{\theta }$
the sets of fixed points of
$\widetilde {X}^{\theta }$
the sets of fixed points of
![]() $\iota $
and
$\iota $
and
![]() $\theta $
, respectively. Since
$\theta $
, respectively. Since
![]() $\iota $
has no fixed points on
$\iota $
has no fixed points on
![]() $X\setminus \mathrm {Sing}\,X$
,
$X\setminus \mathrm {Sing}\,X$
,
![]() $\theta $
has no fixed points on
$\theta $
has no fixed points on
![]() $\widetilde {X}\setminus \pi ^{-1}(\mathrm {Sing}\,X)$
. Hence
$\widetilde {X}\setminus \pi ^{-1}(\mathrm {Sing}\,X)$
. Hence
![]() $\widetilde {X}\setminus \pi ^{-1}(\mathrm {Sing}\,X) \subset \widetilde {X} \setminus \widetilde {X}^{\theta }$
. In other words,
$\widetilde {X}\setminus \pi ^{-1}(\mathrm {Sing}\,X) \subset \widetilde {X} \setminus \widetilde {X}^{\theta }$
. In other words,
![]() $\widetilde {X}^{\theta } \subset \pi ^{-1}(\mathrm {Sing}\,X)$
.
$\widetilde {X}^{\theta } \subset \pi ^{-1}(\mathrm {Sing}\,X)$
.
Lemma 2.4. In the commutative diagram (2.1), the following hold:
-
(1) X is a
 $K3$
surface with rational double points, and
$K3$
surface with rational double points, and  $$ \begin{align*}X^{\iota}=\mathrm{Sing}\,X=p^{-1}(\mathrm{Sing}\,Y), \qquad \iota^{*}|_{H^{0}(X,K_{X})}=-1. \end{align*} $$
$$ \begin{align*}X^{\iota}=\mathrm{Sing}\,X=p^{-1}(\mathrm{Sing}\,Y), \qquad \iota^{*}|_{H^{0}(X,K_{X})}=-1. \end{align*} $$
-
(2)
 $(\widetilde {X},\theta )$
is a
$(\widetilde {X},\theta )$
is a
 $2$
-elementary
$2$
-elementary
 $K3$
surface. Namely,
$K3$
surface. Namely,
 $\theta $
acts nontrivially on holomorphic
$\theta $
acts nontrivially on holomorphic
 $2$
-forms on
$2$
-forms on
 $\widetilde {X}$
. Moreover, there exists an integer
$\widetilde {X}$
. Moreover, there exists an integer
 $k\in \{1,\ldots ,10\}$
such that The pair
$k\in \{1,\ldots ,10\}$
such that The pair $$ \begin{align*}\widetilde{X}^{\theta}=E_{1}\amalg\ldots\amalg E_{k}, \qquad E_{i}\cong\mathbf{P}^{1}. \end{align*} $$
$$ \begin{align*}\widetilde{X}^{\theta}=E_{1}\amalg\ldots\amalg E_{k}, \qquad E_{i}\cong\mathbf{P}^{1}. \end{align*} $$
 $(\widetilde {X},\theta )$
is called the
$(\widetilde {X},\theta )$
is called the
 $2$
-elementary
$2$
-elementary
 $K3$
surface associated to Y.
$K3$
surface associated to Y.
Proof. See [Reference Zhang47, Lemma 3.1, Th. 3.6] for (1) and [Reference Zhang48, Lemma 2.1] for (2).
Lemma 2.5. Let Y,
![]() $Y'$
be log Enriques surfaces with canonical double coverings
$Y'$
be log Enriques surfaces with canonical double coverings
![]() $p' \colon X' \to Y'$
and
$p' \colon X' \to Y'$
and
![]() $p \colon X \to Y$
, respectively. Let
$p \colon X \to Y$
, respectively. Let
![]() $\varphi \colon Y' \to Y$
be a birational holomorphic map. Then the following hold:
$\varphi \colon Y' \to Y$
be a birational holomorphic map. Then the following hold:
-
(1)
 $\varphi ^{*}$
induces an isomorphism from
$\varphi ^{*}$
induces an isomorphism from
 $H^{0}(Y, K_{Y}^{\otimes 2})$
to
$H^{0}(Y, K_{Y}^{\otimes 2})$
to
 $H^{0}(Y', K_{Y'}^{\otimes 2})$
.
$H^{0}(Y', K_{Y'}^{\otimes 2})$
. -
(2)
 $\varphi ( \mathrm {Sing}\,Y') \subset \mathrm {Sing}\,Y$
.
$\varphi ( \mathrm {Sing}\,Y') \subset \mathrm {Sing}\,Y$
. -
(3)
 $\varphi $
lifts to a holomorphic map
$\varphi $
lifts to a holomorphic map
 $f \colon X' \to X$
of canonical double coverings.
$f \colon X' \to X$
of canonical double coverings.
Proof. (1) Let
![]() $\Xi \in H^{0}(Y, K_{Y}^{\otimes 2})\setminus \{0\}$
and
$\Xi \in H^{0}(Y, K_{Y}^{\otimes 2})\setminus \{0\}$
and
![]() $\Xi ' \in H^{0}(Y', K_{Y'}^{\otimes 2})\setminus \{0\}$
. Then
$\Xi ' \in H^{0}(Y', K_{Y'}^{\otimes 2})\setminus \{0\}$
. Then
![]() $\varphi ^{*}\Xi $
is a bicanonical from on
$\varphi ^{*}\Xi $
is a bicanonical from on
![]() $Y'\setminus (\mathrm {Sing}\,Y' \cup \varphi ^{-1}(\mathrm {Sing}\,Y))$
, and
$Y'\setminus (\mathrm {Sing}\,Y' \cup \varphi ^{-1}(\mathrm {Sing}\,Y))$
, and
![]() $\Xi '$
is nowhere vanishing. We get
$\Xi '$
is nowhere vanishing. We get
![]() $\varphi ^{*}\Xi /\Xi ' \in {\mathcal O}(Y'\setminus (\mathrm {Sing}\,Y' \cup \varphi ^{-1}(\mathrm {Sing}\,Y))) = {\mathcal O}(Y'\setminus \varphi ^{-1}(\mathrm {Sing}\,Y)) = {\mathcal O}(Y\setminus \mathrm {Sing}\,Y) = {\mathcal O}(Y)={\mathbf C}$
, where the first and third equalities follow from the normality of
$\varphi ^{*}\Xi /\Xi ' \in {\mathcal O}(Y'\setminus (\mathrm {Sing}\,Y' \cup \varphi ^{-1}(\mathrm {Sing}\,Y))) = {\mathcal O}(Y'\setminus \varphi ^{-1}(\mathrm {Sing}\,Y)) = {\mathcal O}(Y\setminus \mathrm {Sing}\,Y) = {\mathcal O}(Y)={\mathbf C}$
, where the first and third equalities follow from the normality of
![]() $Y'$
and Y and the second equality follows from the Zariski Main Theorem. Hence
$Y'$
and Y and the second equality follows from the Zariski Main Theorem. Hence
![]() $\varphi ^{*}\Xi = c\,\Xi '$
with some
$\varphi ^{*}\Xi = c\,\Xi '$
with some
![]() $c \in {\mathbf C}\setminus \{0\}$
, and
$c \in {\mathbf C}\setminus \{0\}$
, and
![]() $\varphi ^{*}$
is an isomorphism.
$\varphi ^{*}$
is an isomorphism.
(2) Let
![]() $o \in \mathrm {Sing}\,Y'$
. Assume
$o \in \mathrm {Sing}\,Y'$
. Assume
![]() $\varphi (o) \in Y \setminus \mathrm {Sing}\,Y$
. There exist a neighbourhood U of
$\varphi (o) \in Y \setminus \mathrm {Sing}\,Y$
. There exist a neighbourhood U of
![]() $\varphi (o)$
and a nowhere vanishing canonical form
$\varphi (o)$
and a nowhere vanishing canonical form
![]() $\eta \in H^{0}(U, K_{Y})$
. We can express
$\eta \in H^{0}(U, K_{Y})$
. We can express
![]() $\Xi |_{U} = F\cdot \eta ^{\otimes 2}$
,
$\Xi |_{U} = F\cdot \eta ^{\otimes 2}$
,
![]() $F \in {\mathcal O}^{*}(U)$
. Since
$F \in {\mathcal O}^{*}(U)$
. Since
![]() $\varphi ^{*}\Xi $
and
$\varphi ^{*}\Xi $
and
![]() $\varphi ^{*}F$
are nowhere vanishing on
$\varphi ^{*}F$
are nowhere vanishing on
![]() $\varphi ^{-1}(U)$
, so is
$\varphi ^{-1}(U)$
, so is
![]() $\varphi ^{*}\eta ^{\otimes 2}$
. Hence
$\varphi ^{*}\eta ^{\otimes 2}$
. Hence
![]() $\varphi ^{*}\eta $
is nowhere vanishing. Since any singular point of
$\varphi ^{*}\eta $
is nowhere vanishing. Since any singular point of
![]() $Y'$
is non-Gorenstein, we get a contradiction. Thus
$Y'$
is non-Gorenstein, we get a contradiction. Thus
![]() $\varphi (o) \in \mathrm {Sing}\,Y$
.
$\varphi (o) \in \mathrm {Sing}\,Y$
.
(3) Since
![]() $\varphi ^{*}\Xi $
is nowhere vanishing on
$\varphi ^{*}\Xi $
is nowhere vanishing on
![]() $Y'\setminus \varphi ^{-1}(\mathrm {Sing}\,Y)$
,
$Y'\setminus \varphi ^{-1}(\mathrm {Sing}\,Y)$
,
![]() $\varphi $
has no critical points on
$\varphi $
has no critical points on
![]() $Y'\setminus \varphi ^{-1}(\mathrm {Sing}\,Y)$
. Since the restriction of
$Y'\setminus \varphi ^{-1}(\mathrm {Sing}\,Y)$
. Since the restriction of
![]() $\varphi $
to
$\varphi $
to
![]() $Y'\setminus \varphi ^{-1}(\mathrm {Sing}\,Y)$
is a closed map,
$Y'\setminus \varphi ^{-1}(\mathrm {Sing}\,Y)$
is a closed map,
![]() $\varphi \colon Y'\setminus \varphi ^{-1}(\mathrm {Sing}\,Y) \to Y \setminus \mathrm {Sing}\,Y$
is an étale covering of degree one: that is, an isomorphism.
$\varphi \colon Y'\setminus \varphi ^{-1}(\mathrm {Sing}\,Y) \to Y \setminus \mathrm {Sing}\,Y$
is an étale covering of degree one: that is, an isomorphism.
![]() $\varphi $
induces a holomorphic map
$\varphi $
induces a holomorphic map
![]() $f \colon X' \setminus (p')^{-1}\varphi ^{-1}(\mathrm {Sing}\,Y) \to X\setminus p^{-1}(\mathrm {Sing}\,Y)$
such that
$f \colon X' \setminus (p')^{-1}\varphi ^{-1}(\mathrm {Sing}\,Y) \to X\setminus p^{-1}(\mathrm {Sing}\,Y)$
such that
![]() $p\circ f = \varphi \circ p'$
. Since
$p\circ f = \varphi \circ p'$
. Since
![]() $p^{-1}(y)$
consists of a unique point for any
$p^{-1}(y)$
consists of a unique point for any
![]() $y\in \mathrm {Sing}\,Y$
, f extends to a map from
$y\in \mathrm {Sing}\,Y$
, f extends to a map from
![]() $X'$
to X by setting
$X'$
to X by setting
![]() $f(x') := p^{-1}(\varphi (p'(x')))$
for
$f(x') := p^{-1}(\varphi (p'(x')))$
for
![]() $x'\in (p')^{-1}\varphi ^{-1}(\mathrm {Sing}\,Y)$
. By construction,
$x'\in (p')^{-1}\varphi ^{-1}(\mathrm {Sing}\,Y)$
. By construction,
![]() $p\circ f = \varphi \circ p'$
. By this equality and the bijectivity of the map
$p\circ f = \varphi \circ p'$
. By this equality and the bijectivity of the map
![]() $p\colon \mathrm {Sing}\,X \to \mathrm {Sing}\,Y$
, f is continuous. Since f is holomorphic on a Zariski open subset,
$p\colon \mathrm {Sing}\,X \to \mathrm {Sing}\,Y$
, f is continuous. Since f is holomorphic on a Zariski open subset,
![]() $f \colon X'\to X$
is holomorphic by the normality of
$f \colon X'\to X$
is holomorphic by the normality of
![]() $X'$
.
$X'$
.
2.3 The good model of a log-Enriques surface
The group
![]() $\mathbf {Z}/4\mathbf {Z}$
acts on
$\mathbf {Z}/4\mathbf {Z}$
acts on
![]() $\mathbf {C}^{2}$
as the multiplication by
$\mathbf {C}^{2}$
as the multiplication by
![]() $i=\sqrt {-1}$
: that is,
$i=\sqrt {-1}$
: that is,
![]() $i(z_{1},z_{2}):=(iz_{1},iz_{2})$
. We define the cyclic quotient singularity of type
$i(z_{1},z_{2}):=(iz_{1},iz_{2})$
. We define the cyclic quotient singularity of type
![]() $\frac {1}{4}(1,1)$
by
$\frac {1}{4}(1,1)$
by
Its minimal resolution is the total space of the line bundle
![]() ${\mathcal O}_{\mathbf {P}^{1}}(-4)$
${\mathcal O}_{\mathbf {P}^{1}}(-4)$
where the exceptional divisor
![]() $E=\varpi ^{-1}(0)$
is a
$E=\varpi ^{-1}(0)$
is a
![]() $(-4)$
-curve: that is,
$(-4)$
-curve: that is,
![]() $E^{2}=-4$
.
$E^{2}=-4$
.
Definition 2.6. A log-Enriques surface Y is good if Y has only cyclic quotient singularities of type
![]() $\frac {1}{4}(1,1)$
.
$\frac {1}{4}(1,1)$
.
Let Y be a log-Enriques surface,
![]() $p\colon X\to Y$
be its canonical double covering and
$p\colon X\to Y$
be its canonical double covering and
![]() $\pi \colon \widetilde {X}\to X$
be the minimal resolution. Then X and
$\pi \colon \widetilde {X}\to X$
be the minimal resolution. Then X and
![]() $\widetilde {X}$
are equipped with the canonical involutions
$\widetilde {X}$
are equipped with the canonical involutions
![]() $\iota $
and
$\iota $
and
![]() $\theta $
, respectively. Let
$\theta $
, respectively. Let
![]() $E=\pi ^{-1}(\mathrm {Sing}\,X)$
be the exceptional divisor of
$E=\pi ^{-1}(\mathrm {Sing}\,X)$
be the exceptional divisor of
![]() $\pi \colon \widetilde {X}\to X$
. Then
$\pi \colon \widetilde {X}\to X$
. Then
![]() $E \supset \widetilde {X}^{\theta } =\amalg _{i=1}^{k}E_{i}$
with
$E \supset \widetilde {X}^{\theta } =\amalg _{i=1}^{k}E_{i}$
with
![]() $1\leq k\leq 10$
. Since
$1\leq k\leq 10$
. Since
![]() $E_{i}$
is a
$E_{i}$
is a
![]() $(-2)$
-curve of
$(-2)$
-curve of
![]() $\widetilde {X}$
, it is a
$\widetilde {X}$
, it is a
![]() $(-4)$
-curve of
$(-4)$
-curve of
![]() $\widetilde {X}/\theta $
, and its contraction produces a cyclic quotient singularity of type
$\widetilde {X}/\theta $
, and its contraction produces a cyclic quotient singularity of type
![]() $\frac {1}{4}(1,1)$
.
$\frac {1}{4}(1,1)$
.
Definition 2.7. The good model of a log-Enriques surface Y, denoted by
![]() $Y^{\natural }$
, is defined as the contraction of the disjoint union of
$Y^{\natural }$
, is defined as the contraction of the disjoint union of
![]() $(-4)$
-curves
$(-4)$
-curves
![]() $\widetilde {X}^{\theta }$
in
$\widetilde {X}^{\theta }$
in
![]() $\widetilde {X}/\theta $
, where
$\widetilde {X}/\theta $
, where
![]() $(\widetilde {X},\theta )$
is the
$(\widetilde {X},\theta )$
is the
![]() $2$
-elementary
$2$
-elementary
![]() $K3$
surface associated to Y.
$K3$
surface associated to Y.
Another construction of
![]() $Y^{\natural }$
from Y is as follows [Reference Zhang47, Th. 3.6], [Reference Zhang48, Lemmas 1.4 and 2.1]. Let
$Y^{\natural }$
from Y is as follows [Reference Zhang47, Th. 3.6], [Reference Zhang48, Lemmas 1.4 and 2.1]. Let
![]() $\widetilde {Y}$
be the minimal resolution of Y with exceptional divisor
$\widetilde {Y}$
be the minimal resolution of Y with exceptional divisor
![]() $D \subset \widetilde {Y}$
. Let
$D \subset \widetilde {Y}$
. Let
![]() $Y^{\#}$
be the blowing-up of
$Y^{\#}$
be the blowing-up of
![]() $\widetilde {Y}$
at
$\widetilde {Y}$
at
![]() $\mathrm {Sing}\,D$
. Then the proper transform of D consists of disjoint
$\mathrm {Sing}\,D$
. Then the proper transform of D consists of disjoint
![]() $(-4)$
-curves, say
$(-4)$
-curves, say
![]() $\widetilde {D}_{1},\ldots , \widetilde {D}_{k}$
. Then
$\widetilde {D}_{1},\ldots , \widetilde {D}_{k}$
. Then
![]() $Y^{\#} \cong \widetilde {X}/\theta $
and
$Y^{\#} \cong \widetilde {X}/\theta $
and
![]() $Y^{\natural }$
is obtained from
$Y^{\natural }$
is obtained from
![]() $Y^{\#}$
by contracting the
$Y^{\#}$
by contracting the
![]() $\widetilde {D}_{i}$
s. (Notice that
$\widetilde {D}_{i}$
s. (Notice that
![]() $\widetilde {Y}$
and
$\widetilde {Y}$
and
![]() $Y^{\#}$
are not log-Enriques surfaces.) As is verified easily, the composition of the rational map
$Y^{\#}$
are not log-Enriques surfaces.) As is verified easily, the composition of the rational map
![]() $Y^{\natural } \dashrightarrow Y^{\#}$
and the blowing-down
$Y^{\natural } \dashrightarrow Y^{\#}$
and the blowing-down
![]() $Y^{\#} \to Y$
extend to a holomorphic map from
$Y^{\#} \to Y$
extend to a holomorphic map from
![]() $Y^{\natural }$
to Y.
$Y^{\natural }$
to Y.
By construction,
![]() $Y^{\natural }$
has at most cyclic quotient singularities of type
$Y^{\natural }$
has at most cyclic quotient singularities of type
![]() $\frac {1}{4}(1,1)$
. If Y is a good log-Enriques surface, then
$\frac {1}{4}(1,1)$
. If Y is a good log-Enriques surface, then
![]() $Y=Y^{\natural }$
.
$Y=Y^{\natural }$
.
Proposition 2.8. Let Y be a log-Enriques surface. If there is a birational holomorphic map from a good log-Enriques surface
![]() $Y'$
to Y, then
$Y'$
to Y, then
![]() $Y'\cong Y^{\natural }$
.
$Y'\cong Y^{\natural }$
.
Proof. Let
![]() $X^{\natural }$
(respectively,
$X^{\natural }$
(respectively,
![]() $X'$
) be the canonical double covering of
$X'$
) be the canonical double covering of
![]() $Y^{\natural }$
(respectively,
$Y^{\natural }$
(respectively,
![]() $Y'$
), and let
$Y'$
), and let
![]() $\widetilde {X}^{\natural }$
(respectively,
$\widetilde {X}^{\natural }$
(respectively,
![]() $\widetilde {X'}$
) be the minimal resolution of
$\widetilde {X'}$
) be the minimal resolution of
![]() $X^{\natural }$
(respectively,
$X^{\natural }$
(respectively,
![]() $X'$
). The birational morphism
$X'$
). The birational morphism
![]() $Y'\to Y$
induces a birational morphism
$Y'\to Y$
induces a birational morphism
![]() $\psi \colon (X',\iota ') \to (X,\iota )$
by Lemma 2.5 (3), and this
$\psi \colon (X',\iota ') \to (X,\iota )$
by Lemma 2.5 (3), and this
![]() $\psi $
induces an isomorphism
$\psi $
induces an isomorphism
![]() $f\colon (\widetilde {X}',\theta ')\to (\widetilde {X},\theta )=(\widetilde {X}^{\natural },\theta )$
, by the minimality of
$f\colon (\widetilde {X}',\theta ')\to (\widetilde {X},\theta )=(\widetilde {X}^{\natural },\theta )$
, by the minimality of
![]() $K3$
surfaces. Hence
$K3$
surfaces. Hence
![]() $(\widetilde {X}'/\theta ',(\widetilde {X}')^{\theta '})\cong (\widetilde {X}^{\natural }/\theta ,(\widetilde {X}^{\natural })^{\theta })$
. Since the projection
$(\widetilde {X}'/\theta ',(\widetilde {X}')^{\theta '})\cong (\widetilde {X}^{\natural }/\theta ,(\widetilde {X}^{\natural })^{\theta })$
. Since the projection
![]() $\widetilde {X}'/\theta '\to Y'$
(respectively,
$\widetilde {X}'/\theta '\to Y'$
(respectively,
![]() $\widetilde {X}^{\natural }/\theta \to Y^{\natural }$
) is obtained by contracting every component of
$\widetilde {X}^{\natural }/\theta \to Y^{\natural }$
) is obtained by contracting every component of
![]() $(\widetilde {X}')^{\theta '}$
(respectively,
$(\widetilde {X}')^{\theta '}$
(respectively,
![]() $(\widetilde {X}^{\natural })^{\theta }$
) to a cyclic quotient singularity of type
$(\widetilde {X}^{\natural })^{\theta }$
) to a cyclic quotient singularity of type
![]() $\frac {1}{4}(1,1)$
, f induces an isomorphism from
$\frac {1}{4}(1,1)$
, f induces an isomorphism from
![]() $Y'$
to Y.
$Y'$
to Y.
By Proposition 2.8, every log-Enriques surface has a unique good model. By Zhang [Reference Zhang47, Th. 3.6], [Reference Zhang48, Th.4, Cor. 5, Lemma 2.3], one can associate to a log-Enriques surface another log-Enriques surface with a unique singular point in the canonical way. So log-Enriques surfaces of this type form another class to be studied. Because of the uniqueness (up to a scaling) of the Ricci-flat ALE hyperkähler metric on the minimal resolution of
![]() $A_{1}$
-singularity, in this paper, we focus on good log-Enriques surfaces.
$A_{1}$
-singularity, in this paper, we focus on good log-Enriques surfaces.
In the rest of this section, we study the moduli space of good log-Enriques surfaces. Throughout this paper, we mean by lattice a free
![]() $\mathbf {Z}$
-module of finite rank equipped with a nondegenerate integral symmetric bilinear form. We often identify a lattice with its Gram matrix.
$\mathbf {Z}$
-module of finite rank equipped with a nondegenerate integral symmetric bilinear form. We often identify a lattice with its Gram matrix.
2.4
 $2$
-elementary
$2$
-elementary
 $K3$
surfaces and log-Enriques surfaces
$K3$
surfaces and log-Enriques surfaces
A pair
![]() $(Z,\iota )$
is called a
$(Z,\iota )$
is called a
![]() $2$
-elementary
$2$
-elementary
![]() $K3$
surface if Z is a
$K3$
surface if Z is a
![]() $K3$
surface and
$K3$
surface and
![]() $\iota \colon Z\to Z$
is a holomorphic anti-symplectic involution. For a
$\iota \colon Z\to Z$
is a holomorphic anti-symplectic involution. For a
![]() $2$
-elementary
$2$
-elementary
![]() $K3$
surface
$K3$
surface
![]() $(Z,\iota )$
, we define
$(Z,\iota )$
, we define
which is equipped with the integral bilinear form induced from the intersection pairing on
![]() $H^{2}(Z,\mathbf {Z})$
. Then
$H^{2}(Z,\mathbf {Z})$
. Then
![]() $H^{2}(Z,\mathbf {Z})$
is isometric to the
$H^{2}(Z,\mathbf {Z})$
is isometric to the
![]() $K3$
-lattice (compare [Reference Barth, Hulek, Peters and Van de Ven1])
$K3$
-lattice (compare [Reference Barth, Hulek, Peters and Van de Ven1])
where
![]() ${\Bbb U}=\binom {0\,1}{1\,0}$
and
${\Bbb U}=\binom {0\,1}{1\,0}$
and
![]() ${\Bbb E}_{8}(-1)$
is the negative-definite even unimodular lattice of rank
${\Bbb E}_{8}(-1)$
is the negative-definite even unimodular lattice of rank
![]() $8$
whose Gram matrix is given by the Cartan matrix of type
$8$
whose Gram matrix is given by the Cartan matrix of type
![]() $E_{8}$
. If r denotes the rank of
$E_{8}$
. If r denotes the rank of
![]() $H^{2}(Z,\mathbf {Z})^{+}$
, then
$H^{2}(Z,\mathbf {Z})^{+}$
, then
![]() $H^{2}(Z,\mathbf {Z})^{+}$
(respectively,
$H^{2}(Z,\mathbf {Z})^{+}$
(respectively,
![]() $H^{2}(Z,\mathbf {Z})^{-}$
) has signature
$H^{2}(Z,\mathbf {Z})^{-}$
) has signature
![]() $(1,r-1)$
(respectively,
$(1,r-1)$
(respectively,
![]() $(2,20-r)$
). For a
$(2,20-r)$
). For a
![]() $2$
-elementary
$2$
-elementary
![]() $K3$
surface
$K3$
surface
![]() $(Z,\iota )$
, the topological type of
$(Z,\iota )$
, the topological type of
![]() $Z^{\iota }$
is determined by the isometry class of the lattice
$Z^{\iota }$
is determined by the isometry class of the lattice
![]() $H^{2}(Z,\mathbf {Z})^{-}$
.
$H^{2}(Z,\mathbf {Z})^{-}$
.
Let Y be a good log-Enriques surface, and let
![]() $(\widetilde {X},\theta )$
be the corresponding
$(\widetilde {X},\theta )$
be the corresponding
![]() $2$
-elementary
$2$
-elementary
![]() $K3$
surface. Hence
$K3$
surface. Hence
![]() $(\widetilde {X}/\theta ,\widetilde {X}^{\theta })\to (Y,\mathrm {Sing}(Y))$
is the minimal resolution of the cyclic quotient singularities of type
$(\widetilde {X}/\theta ,\widetilde {X}^{\theta })\to (Y,\mathrm {Sing}(Y))$
is the minimal resolution of the cyclic quotient singularities of type
![]() $\frac {1}{4}(1,1)$
of Y. We set
$\frac {1}{4}(1,1)$
of Y. We set
and define
![]() $\Lambda _{k}$
as the unimodular lattice of signature
$\Lambda _{k}$
as the unimodular lattice of signature
![]() $(2,10-k)$
(except when
$(2,10-k)$
(except when
![]() $k=8$
, which requires modification). Under the identification with a lattice with its Gram matrix, we have
$k=8$
, which requires modification). Under the identification with a lattice with its Gram matrix, we have
 $$ \begin{align*}\Lambda_{k} = \begin{pmatrix} I_{2}&0 \\ 0&-I_{10-k} \end{pmatrix} \quad (k\not=8), \qquad \Lambda_{8} = \begin{pmatrix} I_{2}&0 \\ 0&-I_{2} \end{pmatrix} \mbox{ or } {\mathbb U}\oplus{\mathbb U} \quad (k=8). \end{align*} $$
$$ \begin{align*}\Lambda_{k} = \begin{pmatrix} I_{2}&0 \\ 0&-I_{10-k} \end{pmatrix} \quad (k\not=8), \qquad \Lambda_{8} = \begin{pmatrix} I_{2}&0 \\ 0&-I_{2} \end{pmatrix} \mbox{ or } {\mathbb U}\oplus{\mathbb U} \quad (k=8). \end{align*} $$
According to the parity of
![]() $\Lambda _{8}$
, we set
$\Lambda _{8}$
, we set
![]() $\Lambda _{8}^{\mathrm {odd}} := I_{2}\oplus - I_{2}$
and
$\Lambda _{8}^{\mathrm {odd}} := I_{2}\oplus - I_{2}$
and
![]() $\Lambda _{8}^{\mathrm {even}} := {\mathbb U}\oplus {\mathbb U}$
. Since
$\Lambda _{8}^{\mathrm {even}} := {\mathbb U}\oplus {\mathbb U}$
. Since
![]() $\widetilde {X}^{\theta }$
consists of smooth rational curves, we deduce from Nikulin [Reference Nikulin39, Th. 4.2.2] that there is an isometry of lattices
$\widetilde {X}^{\theta }$
consists of smooth rational curves, we deduce from Nikulin [Reference Nikulin39, Th. 4.2.2] that there is an isometry of lattices
![]() $\alpha \colon H^{2}(\widetilde {X},\mathbf {Z}) \cong {\Bbb L}_{K3}$
with
$\alpha \colon H^{2}(\widetilde {X},\mathbf {Z}) \cong {\Bbb L}_{K3}$
with
Here
![]() $\Lambda _{k}(2)$
stands for the rescaling of
$\Lambda _{k}(2)$
stands for the rescaling of
![]() $\Lambda _{k}$
, whose bilinear form is the double of that of
$\Lambda _{k}$
, whose bilinear form is the double of that of
![]() $\Lambda _{k}$
. An isometry of lattices
$\Lambda _{k}$
. An isometry of lattices
![]() $\alpha \colon H^{2}(\widetilde {X},\mathbf {Z})\cong {\Bbb L}_{K3}$
satisfying equation (2.2) is called a marking of
$\alpha \colon H^{2}(\widetilde {X},\mathbf {Z})\cong {\Bbb L}_{K3}$
satisfying equation (2.2) is called a marking of
![]() $(\widetilde {X},\theta )$
. We set
$(\widetilde {X},\theta )$
. We set
where the orthogonal complement is considered in the
![]() $K3$
-lattice
$K3$
-lattice
![]() ${\Bbb L}_{K3}$
. A
${\Bbb L}_{K3}$
. A
![]() $2$
-elementary
$2$
-elementary
![]() $K3$
surface
$K3$
surface
![]() $(Z,\iota )$
is of type
$(Z,\iota )$
is of type
![]() $M_{k}$
if its invariant lattice
$M_{k}$
if its invariant lattice
![]() $H^{2}(Z,\mathbf {Z})^+$
is isometric to
$H^{2}(Z,\mathbf {Z})^+$
is isometric to
![]() $M_{k}$
.
$M_{k}$
.
We define
Then
![]() $\Omega _{k}$
consists of two connected components
$\Omega _{k}$
consists of two connected components
![]() $\Omega _{k}^{+}$
and
$\Omega _{k}^{+}$
and
![]() $\Omega _{k}^{-}$
, each of which is isomorphic to the bounded symmetric domain of type IV of dimension
$\Omega _{k}^{-}$
, each of which is isomorphic to the bounded symmetric domain of type IV of dimension
![]() $10-k$
. Let
$10-k$
. Let
![]() $O(\Lambda _{k})$
be the automorphism group of
$O(\Lambda _{k})$
be the automorphism group of
![]() $\Lambda _{k}$
, and let
$\Lambda _{k}$
, and let
![]() $O^{+}(\Lambda _{k})\subset O(\Lambda _{k})$
be the subgroup of index
$O^{+}(\Lambda _{k})\subset O(\Lambda _{k})$
be the subgroup of index
![]() $2$
consisting of elements preserving
$2$
consisting of elements preserving
![]() $\Omega _{k}^{\pm }$
. We define the orthogonal modular variety associated with
$\Omega _{k}^{\pm }$
. We define the orthogonal modular variety associated with
![]() $\Lambda _{k}$
by
$\Lambda _{k}$
by
When
![]() $k=8$
, we define
$k=8$
, we define
![]() ${\mathcal M}_{8}^{\mathrm {odd}} := \Omega _{8}/O(\Lambda _{8}^{\mathrm {odd}})$
and
${\mathcal M}_{8}^{\mathrm {odd}} := \Omega _{8}/O(\Lambda _{8}^{\mathrm {odd}})$
and
![]() ${\mathcal M}_{8}^{\mathrm {even}} := \Omega _{8}/O(\Lambda _{8}^{\mathrm {even}})$
. When there is no possibility of confusion, we write
${\mathcal M}_{8}^{\mathrm {even}} := \Omega _{8}/O(\Lambda _{8}^{\mathrm {even}})$
. When there is no possibility of confusion, we write
![]() ${\mathcal M}_{8}$
for
${\mathcal M}_{8}$
for
![]() ${\mathcal M}_{8}^{\mathrm {odd}}$
and
${\mathcal M}_{8}^{\mathrm {odd}}$
and
![]() ${\mathcal M}_{8}^{\mathrm {even}}$
.
${\mathcal M}_{8}^{\mathrm {even}}$
.
Since
![]() $\theta $
acts nontrivially on
$\theta $
acts nontrivially on
![]() $H^{0}(\widetilde {X},\Omega ^{2}_{\widetilde {X}})$
, we deduce the inclusion from the Hodge decomposition
$H^{0}(\widetilde {X},\Omega ^{2}_{\widetilde {X}})$
, we deduce the inclusion from the Hodge decomposition
![]() $H^{0}(\widetilde {X},\Omega ^{2}_{\widetilde {X}})\subset H^{2}(\widetilde {X},\mathbf {C})^{-}$
. Since
$H^{0}(\widetilde {X},\Omega ^{2}_{\widetilde {X}})\subset H^{2}(\widetilde {X},\mathbf {C})^{-}$
. Since
![]() $H^{0}(\widetilde {X},\Omega ^{2}_{\widetilde {X}})$
is a complex line, it follows from the Riemann-Hodge bilinear relations that
$H^{0}(\widetilde {X},\Omega ^{2}_{\widetilde {X}})$
is a complex line, it follows from the Riemann-Hodge bilinear relations that
The point
![]() $\varpi (\widetilde {X},\theta ,\alpha )\in \Omega _{k}$
is called the period of
$\varpi (\widetilde {X},\theta ,\alpha )\in \Omega _{k}$
is called the period of
![]() $(\widetilde {X},\theta ,\alpha )$
. We define the period of
$(\widetilde {X},\theta ,\alpha )$
. We define the period of
![]() $(\widetilde {X},\theta )$
as the
$(\widetilde {X},\theta )$
as the
![]() $O(\Lambda _{k})$
-orbit of
$O(\Lambda _{k})$
-orbit of
![]() $\varpi (\widetilde {X},\theta ,\alpha )$
: that is,
$\varpi (\widetilde {X},\theta ,\alpha )$
: that is,
By [Reference Yoshikawa43, Th. 1.8], the coarse moduli space of
![]() $2$
-elementary
$2$
-elementary
![]() $K3$
surfaces of type
$K3$
surfaces of type
![]() $M_{k}$
is isomorphic via the period map to the analytic space
$M_{k}$
is isomorphic via the period map to the analytic space
![]() ${\mathcal M}_{k}^{o}:={\mathcal M}_{k}\setminus {\mathcal D}_{k}$
, where
${\mathcal M}_{k}^{o}:={\mathcal M}_{k}\setminus {\mathcal D}_{k}$
, where
![]() ${\mathcal D}_{k}$
is the discriminant divisor
${\mathcal D}_{k}$
is the discriminant divisor
 $$ \begin{align*}{\mathcal D}_{k}=(\bigcup_{d\in\Lambda_{k},\,d^{2}=-1}d^{\perp})/O(\Lambda_{k}), \qquad d^{\perp}:=\{[\eta]\in\Omega_{k};\,\langle\eta,d\rangle=0\}. \end{align*} $$
$$ \begin{align*}{\mathcal D}_{k}=(\bigcup_{d\in\Lambda_{k},\,d^{2}=-1}d^{\perp})/O(\Lambda_{k}), \qquad d^{\perp}:=\{[\eta]\in\Omega_{k};\,\langle\eta,d\rangle=0\}. \end{align*} $$
2.5 The period mapping for log-Enriques surfaces
Definition 2.9. The period of a good log-Enriques surface Y with k singular points is defined as the period of the corresponding
![]() $2$
-elementary
$2$
-elementary
![]() $K3$
surface
$K3$
surface
![]() $(\widetilde {X},\theta )$
:
$(\widetilde {X},\theta )$
:
When
![]() $k=8$
, we define the parity of Y as that of the lattice
$k=8$
, we define the parity of Y as that of the lattice
![]() $\Lambda _{8}$
defined by equation (2.2).
$\Lambda _{8}$
defined by equation (2.2).
Theorem 2.10. The period mapping induces a bijection between the isomorphism classes of good log-Enriques surfaces with k singular points (and fixed parity when
![]() $k=8$
) and
$k=8$
) and
![]() ${\mathcal M}_{k}^{o}$
.
${\mathcal M}_{k}^{o}$
.
Proof. Let
![]() ${\mathcal N}_{k}$
be the isomorphism classes of good log-Enriques surfaces with k singular points (and fixed parity when
${\mathcal N}_{k}$
be the isomorphism classes of good log-Enriques surfaces with k singular points (and fixed parity when
![]() $k=8$
). By [Reference Yoshikawa43, Th. 1.8], we can identify
$k=8$
). By [Reference Yoshikawa43, Th. 1.8], we can identify
![]() ${\mathcal M}_{k}^{o}$
with the isomorphism classes of
${\mathcal M}_{k}^{o}$
with the isomorphism classes of
![]() $2$
-elementary
$2$
-elementary
![]() $K3$
surfaces of type
$K3$
surfaces of type
![]() $M_{k}$
via the period mapping. We define a map
$M_{k}$
via the period mapping. We define a map
![]() $f\colon {\mathcal N}_{k}\to {\mathcal M}_{k}^{o}$
by setting
$f\colon {\mathcal N}_{k}\to {\mathcal M}_{k}^{o}$
by setting
![]() $f(Y)=(\widetilde {X},\theta )$
, where
$f(Y)=(\widetilde {X},\theta )$
, where
![]() $(\widetilde {X},\theta )$
is the
$(\widetilde {X},\theta )$
is the
![]() $2$
-elementary
$2$
-elementary
![]() $K3$
surface associated to Y. Similarly, we define a map
$K3$
surface associated to Y. Similarly, we define a map
![]() $g \colon {\mathcal M}_{k}^{o} \to {\mathcal N}_{k}$
by sending
$g \colon {\mathcal M}_{k}^{o} \to {\mathcal N}_{k}$
by sending
![]() $(Z,\sigma ) \in {\mathcal M}_{k}^{o}$
to the surface obtained from
$(Z,\sigma ) \in {\mathcal M}_{k}^{o}$
to the surface obtained from
![]() $Z/\sigma $
by blowing down
$Z/\sigma $
by blowing down
![]() $Z^{\sigma }$
. Since
$Z^{\sigma }$
. Since
![]() $Z^{\sigma }$
consists of k disjoint
$Z^{\sigma }$
consists of k disjoint
![]() $(-2)$
-curves, its image in
$(-2)$
-curves, its image in
![]() $Z/\sigma $
consists of k disjoint
$Z/\sigma $
consists of k disjoint
![]() $(-4)$
-curves so that
$(-4)$
-curves so that
![]() $g(Z, \sigma )$
is a good log-Enriques surface with k singular points. Since
$g(Z, \sigma )$
is a good log-Enriques surface with k singular points. Since
![]() $g=f^{-1}$
by [Reference Zhang48, Lemmas 1.4 and 2.1], f is a bijection.
$g=f^{-1}$
by [Reference Zhang48, Lemmas 1.4 and 2.1], f is a bijection.
Since the (locally defined) family of
![]() $2$
-elementary
$2$
-elementary
![]() $K3$
surfaces of type
$K3$
surfaces of type
![]() $M_{k}$
associated to a holomorphic family of good log-Enriques surfaces with k-singular points is again holomorphic, the period mapping for any holomorphic family of good log-Enriques surfaces with k-singular points is holomorphic. In what follows, we regard
$M_{k}$
associated to a holomorphic family of good log-Enriques surfaces with k-singular points is again holomorphic, the period mapping for any holomorphic family of good log-Enriques surfaces with k-singular points is holomorphic. In what follows, we regard
![]() ${\mathcal M}_{k}^{o}$
as a coarse moduli space of good log-Enriques surfaces with k singular points (and fixed parity when
${\mathcal M}_{k}^{o}$
as a coarse moduli space of good log-Enriques surfaces with k singular points (and fixed parity when
![]() $k=8$
).
$k=8$
).
3 Analytic torsion for
 $K3$
surfaces and
$K3$
surfaces and
 $2$
-elementary
$2$
-elementary
 $K3$
surfaces
$K3$
surfaces
3.1 Analytic torsion
Let Z be a compact complex orbifold of dimension n, and let
![]() $\gamma $
be a Kähler form on Z in the sense of orbifolds. Let
$\gamma $
be a Kähler form on Z in the sense of orbifolds. Let
![]() $\iota \colon Z\to Z$
be a holomorphic involution, and assume that
$\iota \colon Z\to Z$
be a holomorphic involution, and assume that
![]() $\iota $
preserves
$\iota $
preserves
![]() $\gamma $
. Let
$\gamma $
. Let
![]() $A^{0,q}_{Z}$
be the space of smooth
$A^{0,q}_{Z}$
be the space of smooth
![]() $(0,q)$
-forms on Z in the sense of orbifolds. Let
$(0,q)$
-forms on Z in the sense of orbifolds. Let
![]() $\square _{q}=(\bar {\partial }+\bar {\partial }^{*})^{2}$
be the Hodge-Kodaira Laplacian acting on
$\square _{q}=(\bar {\partial }+\bar {\partial }^{*})^{2}$
be the Hodge-Kodaira Laplacian acting on
![]() $A^{0,q}_{Z}$
. Let
$A^{0,q}_{Z}$
. Let
 $$ \begin{align*}\zeta_{q}(s):=\sum_{\lambda\in\sigma(\square_{q})\setminus\{0\}}\lambda^{-s}\,\dim E(\lambda;\square_{q}) \end{align*} $$
$$ \begin{align*}\zeta_{q}(s):=\sum_{\lambda\in\sigma(\square_{q})\setminus\{0\}}\lambda^{-s}\,\dim E(\lambda;\square_{q}) \end{align*} $$
be the spectral zeta function of
![]() $\square _{q}$
, where
$\square _{q}$
, where
![]() $E(\lambda ;\square _{q})$
is the eigenspace of
$E(\lambda ;\square _{q})$
is the eigenspace of
![]() $\square _{q}$
corresponding to the eigenvalue
$\square _{q}$
corresponding to the eigenvalue
![]() $\lambda $
. Similarly, let
$\lambda $
. Similarly, let
 $$ \begin{align*}\zeta_{q}(s)(\iota):=\sum_{\lambda\in\sigma(\square_{q})\setminus\{0\}}\lambda^{-s}\,\mathrm{Tr}\left[\iota^{*}|_{E(\lambda;\square_{q})}\right] \end{align*} $$
$$ \begin{align*}\zeta_{q}(s)(\iota):=\sum_{\lambda\in\sigma(\square_{q})\setminus\{0\}}\lambda^{-s}\,\mathrm{Tr}\left[\iota^{*}|_{E(\lambda;\square_{q})}\right] \end{align*} $$
be the equivariant spectral zeta function of
![]() $\square _{q}$
. Since
$\square _{q}$
. Since
![]() $(Z,\gamma )$
is a Kähler orbifold,
$(Z,\gamma )$
is a Kähler orbifold,
![]() $\zeta _{q}(s)$
and
$\zeta _{q}(s)$
and
![]() $\zeta _{q}(s)(\iota )$
converge absolutely when
$\zeta _{q}(s)(\iota )$
converge absolutely when
![]() $\Re s>\dim Z$
, extend to meromorphic functions on
$\Re s>\dim Z$
, extend to meromorphic functions on
![]() $\mathbf {C}$
and are holomorphic at
$\mathbf {C}$
and are holomorphic at
![]() $s=0$
. After Ray-Singer [Reference Ray and Singer41] and Bismut [Reference Bismut4], we make the following:
$s=0$
. After Ray-Singer [Reference Ray and Singer41] and Bismut [Reference Bismut4], we make the following:
Definition 3.1. The analytic torsion of the Kähler orbilod
![]() $(Z,\gamma )$
is defined as
$(Z,\gamma )$
is defined as
 $$ \begin{align*}\tau(Z,\gamma):=\exp[-\sum_{q=0}^{n}(-1)^{q}q\,\zeta^{\prime}_{q}(0)]. \end{align*} $$
$$ \begin{align*}\tau(Z,\gamma):=\exp[-\sum_{q=0}^{n}(-1)^{q}q\,\zeta^{\prime}_{q}(0)]. \end{align*} $$
The equivariant analytic torsion of
![]() $(Z,\iota ,\gamma )$
is defined as
$(Z,\iota ,\gamma )$
is defined as
 $$ \begin{align*}\tau_{\mathbf{Z}_{2}}(Z,\gamma)(\iota):=\exp[-\sum_{q=0}^{n}(-1)^{q}q\,\zeta^{\prime}_{q}(0)(\iota)]. \end{align*} $$
$$ \begin{align*}\tau_{\mathbf{Z}_{2}}(Z,\gamma)(\iota):=\exp[-\sum_{q=0}^{n}(-1)^{q}q\,\zeta^{\prime}_{q}(0)(\iota)]. \end{align*} $$
3.2 Analytic torsion for
 $K3$
surfaces
$K3$
surfaces
Theorem 3.2. Let Z be a
![]() $K3$
surface, let
$K3$
surface, let
![]() $\eta \in H^{0}(Z,K_{Z})\setminus \{0\}$
, and let
$\eta \in H^{0}(Z,K_{Z})\setminus \{0\}$
, and let
![]() $\gamma $
be a Kähler form on Z. Then the following formula holds:
$\gamma $
be a Kähler form on Z. Then the following formula holds:
 $$ \begin{align*}\tau(Z,\gamma) = \exp \left[ -\frac{1}{24}\int_{Z} \log\left\{\frac{\eta\wedge\overline{\eta}}{\gamma^{2}/2!}\cdot\frac{\mathrm{Vol}(Z,\gamma)}{\|\eta\|_{L^{2}}^{2}}\right\}\,c_{2}(Z,\gamma) \right], \end{align*} $$
$$ \begin{align*}\tau(Z,\gamma) = \exp \left[ -\frac{1}{24}\int_{Z} \log\left\{\frac{\eta\wedge\overline{\eta}}{\gamma^{2}/2!}\cdot\frac{\mathrm{Vol}(Z,\gamma)}{\|\eta\|_{L^{2}}^{2}}\right\}\,c_{2}(Z,\gamma) \right], \end{align*} $$
where
![]() $c_{i}(Z,\gamma )$
denotes the ith Chern form of
$c_{i}(Z,\gamma )$
denotes the ith Chern form of
![]() $(TZ,\gamma )$
and
$(TZ,\gamma )$
and
![]() $\|\eta \|_{L^{2}}^{2}:=\int _{Z}\eta \wedge \overline {\eta }$
.
$\|\eta \|_{L^{2}}^{2}:=\int _{Z}\eta \wedge \overline {\eta }$
.
Proof. Let
![]() $\omega $
be a Ricci-flat Kähler form on Z such that
$\omega $
be a Ricci-flat Kähler form on Z such that
Since the
![]() $L^{2}$
-metric on
$L^{2}$
-metric on
![]() $H^{2}(Z,{\mathcal O}_{Z})=H^{0}(Z,K_{Z})^{\lor }$
is independent of the choice of a Kähler metric on Z, we get by the anomaly formula for Quillen metrics [Reference Bismut, Gillet and Soulé6]
$H^{2}(Z,{\mathcal O}_{Z})=H^{0}(Z,K_{Z})^{\lor }$
is independent of the choice of a Kähler metric on Z, we get by the anomaly formula for Quillen metrics [Reference Bismut, Gillet and Soulé6]
 $$ \begin{align} \log\left(\frac{\tau(Z,\gamma)\,\mathrm{Vol}(Z,\gamma)}{\tau(Z,\omega)\,\mathrm{Vol}(Z,\omega)}\right) = \frac{1}{24}\int_{Z}\widetilde{c_{1}c_{2}}(TZ;\gamma,\omega), \end{align} $$
$$ \begin{align} \log\left(\frac{\tau(Z,\gamma)\,\mathrm{Vol}(Z,\gamma)}{\tau(Z,\omega)\,\mathrm{Vol}(Z,\omega)}\right) = \frac{1}{24}\int_{Z}\widetilde{c_{1}c_{2}}(TZ;\gamma,\omega), \end{align} $$
where
![]() $\widetilde {c_{1}c_{2}}(TZ;\gamma ,\omega )$
is the Bott-Chern secondary class [Reference Bismut, Gillet and Soulé6] such that
$\widetilde {c_{1}c_{2}}(TZ;\gamma ,\omega )$
is the Bott-Chern secondary class [Reference Bismut, Gillet and Soulé6] such that
Since
![]() $c_{1}(Z,\omega )=0$
by the Ricci-flatness of
$c_{1}(Z,\omega )=0$
by the Ricci-flatness of
![]() $\omega $
and
$\omega $
and
![]() $\widetilde {c_{1}}(L;h,h')=\log (h/h')$
for a holomorphic line bundle L and Hermitian metrics h and
$\widetilde {c_{1}}(L;h,h')=\log (h/h')$
for a holomorphic line bundle L and Hermitian metrics h and
![]() $h'$
on L, and since
$h'$
on L, and since
by [Reference Gillet and Soulé21], we get by equation (3.1)
 $$ \begin{align} \widetilde{c_{1}c_{2}}(TZ;\gamma,\omega) = \widetilde{c_{1}}(TZ;\gamma,\omega)c_{2}(Z,\gamma) = \log\left(\frac{\gamma^{2}}{\omega^{2}}\right)c_{2}(Z,\gamma) = \log\left(\frac{\gamma^{2}/2!}{\eta\wedge\overline{\eta}}\right)c_{2}(Z,\gamma). \end{align} $$
$$ \begin{align} \widetilde{c_{1}c_{2}}(TZ;\gamma,\omega) = \widetilde{c_{1}}(TZ;\gamma,\omega)c_{2}(Z,\gamma) = \log\left(\frac{\gamma^{2}}{\omega^{2}}\right)c_{2}(Z,\gamma) = \log\left(\frac{\gamma^{2}/2!}{\eta\wedge\overline{\eta}}\right)c_{2}(Z,\gamma). \end{align} $$
Since
![]() $\mathrm {Vol}(Z,\gamma )/\mathrm {Vol}(Z,\omega )=\mathrm {Vol}(Z,\gamma )/\|\eta \|_{L^{2}}^{2}$
, we get by substituting equation (3.3) into equation (3.2)
$\mathrm {Vol}(Z,\gamma )/\mathrm {Vol}(Z,\omega )=\mathrm {Vol}(Z,\gamma )/\|\eta \|_{L^{2}}^{2}$
, we get by substituting equation (3.3) into equation (3.2)
 $$ \begin{align} \begin{aligned} \log\left(\frac{\tau(Z,\gamma)}{\tau(Z,\omega)}\right) &= -\log\left(\frac{\mathrm{Vol}(Z,\gamma)}{\|\eta\|_{L^{2}}^{2}}\right) - \frac{1}{24}\int_{Z} \log\left(\frac{\eta\wedge\overline{\eta}}{\gamma^{2}/2!}\right)\,c_{2}(Z,\gamma) \\ &= -\frac{1}{24}\int_{Z} \log\left\{\frac{\eta\wedge\overline{\eta}}{\gamma^{2}/2!}\cdot\frac{\mathrm{Vol}(Z,\gamma)}{\|\eta\|_{L^{2}}^{2}}\right\}\,c_{2}(Z,\gamma), \end{aligned} \end{align} $$
$$ \begin{align} \begin{aligned} \log\left(\frac{\tau(Z,\gamma)}{\tau(Z,\omega)}\right) &= -\log\left(\frac{\mathrm{Vol}(Z,\gamma)}{\|\eta\|_{L^{2}}^{2}}\right) - \frac{1}{24}\int_{Z} \log\left(\frac{\eta\wedge\overline{\eta}}{\gamma^{2}/2!}\right)\,c_{2}(Z,\gamma) \\ &= -\frac{1}{24}\int_{Z} \log\left\{\frac{\eta\wedge\overline{\eta}}{\gamma^{2}/2!}\cdot\frac{\mathrm{Vol}(Z,\gamma)}{\|\eta\|_{L^{2}}^{2}}\right\}\,c_{2}(Z,\gamma), \end{aligned} \end{align} $$
where we used the Gauss-Bonnet-Chern formula for Z to get the second equality.
Since
![]() $\omega $
is Ricci-flat, the Laplacians
$\omega $
is Ricci-flat, the Laplacians
![]() $\square _{0}$
and
$\square _{0}$
and
![]() $\square _{2}$
are isospectral via the map
$\square _{2}$
are isospectral via the map
![]() $A^{0,0}_{Y}\ni f\mapsto f\overline {\eta }\in A^{0,2}_{Y}$
. Hence, for the Ricci-flat metric
$A^{0,0}_{Y}\ni f\mapsto f\overline {\eta }\in A^{0,2}_{Y}$
. Hence, for the Ricci-flat metric
![]() $\omega $
, we get the equality of meromorphic functions
$\omega $
, we get the equality of meromorphic functions
Since the Dolbeault complex is exact on the orthogonal complement of harmonic forms, we get the equality of meromorphic functions
By equations (3.5) and (3.6), we get
3.3 Equivariant analytic torsion for
 $2$
-elementary
$2$
-elementary
 $K3$
surfaces
$K3$
surfaces
Let Z be a
![]() $K3$
surface, and let
$K3$
surface, and let
![]() $\iota \colon Z\to Z$
be an anti-symplectic holomorphic involution. Let
$\iota \colon Z\to Z$
be an anti-symplectic holomorphic involution. Let
![]() $Z^{\iota }=\amalg _{\alpha }C_{\alpha }$
be the decomposition into the connected components. By Nikulin [Reference Nikulin39, Th. 4.2.2], every
$Z^{\iota }=\amalg _{\alpha }C_{\alpha }$
be the decomposition into the connected components. By Nikulin [Reference Nikulin39, Th. 4.2.2], every
![]() $C_{\alpha }$
is a compact Riemann surface unless
$C_{\alpha }$
is a compact Riemann surface unless
![]() $Z^{\iota }=\emptyset $
.
$Z^{\iota }=\emptyset $
.
Let
![]() $\gamma $
be an
$\gamma $
be an
![]() $\iota $
-invariant Kähler form on Z, and let
$\iota $
-invariant Kähler form on Z, and let
![]() $\eta \in H^{2}(Z,K_{Z})\setminus \{0\}$
. Let
$\eta \in H^{2}(Z,K_{Z})\setminus \{0\}$
. Let
be the invariant sublattice of
![]() $H^{2}(Z,\mathbf {Z})$
with respect to the
$H^{2}(Z,\mathbf {Z})$
with respect to the
![]() $\iota $
-action. We define
$\iota $
-action. We define
where we define
and
 $$ \begin{align*}A_{M}(Z,\iota,\gamma) := \exp \left[ \frac{1}{8}\int_{Z^{\iota}} \left.\log\left\{\frac{\eta\wedge\overline{\eta}}{\gamma^{2}/2!}\cdot\frac{\mathrm{Vol}(Z,\gamma)}{\|\eta\|_{L^{2}}^{2}}\right\}\right|_{Z^{\iota}}\, c_{1}(Z^{\iota},\gamma|_{Z^{\iota}}) \right]. \end{align*} $$
$$ \begin{align*}A_{M}(Z,\iota,\gamma) := \exp \left[ \frac{1}{8}\int_{Z^{\iota}} \left.\log\left\{\frac{\eta\wedge\overline{\eta}}{\gamma^{2}/2!}\cdot\frac{\mathrm{Vol}(Z,\gamma)}{\|\eta\|_{L^{2}}^{2}}\right\}\right|_{Z^{\iota}}\, c_{1}(Z^{\iota},\gamma|_{Z^{\iota}}) \right]. \end{align*} $$
As before,
![]() $c_{1}(Z^{\iota },\gamma |_{Z^{\iota }})$
is the first Chern form of
$c_{1}(Z^{\iota },\gamma |_{Z^{\iota }})$
is the first Chern form of
![]() $(TZ^{\iota },\gamma |_{Z^{\iota }})$
.
$(TZ^{\iota },\gamma |_{Z^{\iota }})$
.
Theorem 3.3. The number
![]() $\tau _{M}(Z,\iota )$
is independent of the choice of an
$\tau _{M}(Z,\iota )$
is independent of the choice of an
![]() $\iota $
-invariant Kähler form on Z.
$\iota $
-invariant Kähler form on Z.
Proof. See [Reference Yoshikawa43, Th. 5.7].
For an explicit formula for
![]() $\tau _{M}$
as a function on the moduli space of
$\tau _{M}$
as a function on the moduli space of
![]() $2$
-elementary
$2$
-elementary
![]() $K3$
surfaces, see [Reference Yoshikawa43], [Reference Yoshikawa45], [Reference Ma and Yoshikawa30]. By Theorem 3.2, we can rewrite
$K3$
surfaces, see [Reference Yoshikawa43], [Reference Yoshikawa45], [Reference Ma and Yoshikawa30]. By Theorem 3.2, we can rewrite
![]() $\tau _{M}(Z,\iota )$
as follows:
$\tau _{M}(Z,\iota )$
as follows:
 $$ \begin{align} \begin{aligned} \tau_{M}(Z,\iota) &= \mathrm{Vol}(Z,\gamma)^{\frac{14-r(M)}{4}}\tau(Z,\gamma)\tau_{\mathbf{Z}_{2}}(Z,\gamma)(\iota)\, \mathrm{Vol}(Z^{\iota},\gamma|_{Z^{\iota}})\tau(Z^{\iota},\gamma|_{Z^{\iota}}) \\ &\quad\times A_{M}(Z,\iota,\gamma)\, \exp \left[ \frac{1}{24}\int_{Z} \log\left\{\frac{\eta\wedge\overline{\eta}}{\gamma^{2}/2!}\cdot\frac{\mathrm{Vol}(Z,\gamma)}{\|\eta\|_{L^{2}}^{2}}\right\}\,c_{2}(Z,\gamma) \right]. \end{aligned} \end{align} $$
$$ \begin{align} \begin{aligned} \tau_{M}(Z,\iota) &= \mathrm{Vol}(Z,\gamma)^{\frac{14-r(M)}{4}}\tau(Z,\gamma)\tau_{\mathbf{Z}_{2}}(Z,\gamma)(\iota)\, \mathrm{Vol}(Z^{\iota},\gamma|_{Z^{\iota}})\tau(Z^{\iota},\gamma|_{Z^{\iota}}) \\ &\quad\times A_{M}(Z,\iota,\gamma)\, \exp \left[ \frac{1}{24}\int_{Z} \log\left\{\frac{\eta\wedge\overline{\eta}}{\gamma^{2}/2!}\cdot\frac{\mathrm{Vol}(Z,\gamma)}{\|\eta\|_{L^{2}}^{2}}\right\}\,c_{2}(Z,\gamma) \right]. \end{aligned} \end{align} $$
4 A degenerating family of Kähler metrics
Let Y be a good log-Enriques surface. For an orbifold Kähler form
![]() $\gamma $
on Y, we write
$\gamma $
on Y, we write
![]() $\mathrm {Vol}(Y,\gamma )=\int _{Y}\gamma ^{2}/2!$
for the volume of
$\mathrm {Vol}(Y,\gamma )=\int _{Y}\gamma ^{2}/2!$
for the volume of
![]() $(Y,\gamma )$
. We set
$(Y,\gamma )$
. We set
Let
![]() $(\widetilde {X},\theta )$
be the
$(\widetilde {X},\theta )$
be the
![]() $2$
-elementary
$2$
-elementary
![]() $K3$
surface associated to Y such that
$K3$
surface associated to Y such that
Let
be the blowing-down of the disjoint union of
![]() $(-2)$
-curves. Then
$(-2)$
-curves. Then
In this section, we construct a two-parameter family of Kähler metrics
![]() $\{ \gamma _{\epsilon ,\delta } \}$
on
$\{ \gamma _{\epsilon ,\delta } \}$
on
![]() $\widetilde {X}$
converging to an orbifold Kähler metric on X, which is obtained by glueing the Eguchi-Hanson instanton at each
$\widetilde {X}$
converging to an orbifold Kähler metric on X, which is obtained by glueing the Eguchi-Hanson instanton at each
![]() ${\mathfrak p}$
and a Kähler metric on X. In the subsequent sections, we study the limiting behaviour of various geometric quantities of
${\mathfrak p}$
and a Kähler metric on X. In the subsequent sections, we study the limiting behaviour of various geometric quantities of
![]() $(\widetilde {X}, \gamma _{\epsilon ,\delta })$
to construct an invariant of the log-Enriques surface Y.
$(\widetilde {X}, \gamma _{\epsilon ,\delta })$
to construct an invariant of the log-Enriques surface Y.
4.1 Eguchi-Hanson instanton
For
![]() $\epsilon \geq 0$
, let
$\epsilon \geq 0$
, let
![]() $F_{\epsilon }(z)$
be the function on
$F_{\epsilon }(z)$
be the function on
![]() $\mathbf {C}^{2}\setminus \{0\}$
defined by
$\mathbf {C}^{2}\setminus \{0\}$
defined by
 $$ \begin{align*}F_{\epsilon}(z) := \sqrt{\|z\|^{4}+\epsilon^{2}}+\epsilon\,\log\left(\frac{\|z\|^{2}}{\sqrt{\|z\|^{4}+\epsilon^{2}}+\epsilon}\right). \end{align*} $$
$$ \begin{align*}F_{\epsilon}(z) := \sqrt{\|z\|^{4}+\epsilon^{2}}+\epsilon\,\log\left(\frac{\|z\|^{2}}{\sqrt{\|z\|^{4}+\epsilon^{2}}+\epsilon}\right). \end{align*} $$
On every compact subset of
![]() $\mathbf {C}^{2}\setminus \{0\}$
, we have
$\mathbf {C}^{2}\setminus \{0\}$
, we have
![]() $\lim _{\epsilon \to 0}F_{\epsilon }(z)=\|z\|^{2}$
. For all
$\lim _{\epsilon \to 0}F_{\epsilon }(z)=\|z\|^{2}$
. For all
![]() $\epsilon \geq 0$
and
$\epsilon \geq 0$
and
![]() $\delta>0$
,
$\delta>0$
,
Let
![]() $T^{*}\mathbf {P}^{1}$
be the holomorphic cotangent bundle of the projective line, and let
$T^{*}\mathbf {P}^{1}$
be the holomorphic cotangent bundle of the projective line, and let
![]() $E\subset T^{*}\mathbf {P}^{1}$
be its zero section. Let
$E\subset T^{*}\mathbf {P}^{1}$
be its zero section. Let
be the blowing-down of the zero section. Since
 $$ \begin{align*}i\partial\bar{\partial}F_{\epsilon}(z) = i \left( \frac{\epsilon\,\partial\|z\|^{2}\wedge\bar{\partial}\|z\|^{2}}{\sqrt{\|z\|^{4}+\epsilon^{2}}+\epsilon)\sqrt{\|z\|^{4}+\epsilon^{2}}} + \frac{\|z\|^{2}\partial\bar{\partial}\|z\|^{2}}{\sqrt{\|z\|^{4}+\epsilon^{2}}+\epsilon} + \epsilon\,\partial\bar{\partial}\log\|z\|^{2} \right) \end{align*} $$
$$ \begin{align*}i\partial\bar{\partial}F_{\epsilon}(z) = i \left( \frac{\epsilon\,\partial\|z\|^{2}\wedge\bar{\partial}\|z\|^{2}}{\sqrt{\|z\|^{4}+\epsilon^{2}}+\epsilon)\sqrt{\|z\|^{4}+\epsilon^{2}}} + \frac{\|z\|^{2}\partial\bar{\partial}\|z\|^{2}}{\sqrt{\|z\|^{4}+\epsilon^{2}}+\epsilon} + \epsilon\,\partial\bar{\partial}\log\|z\|^{2} \right) \end{align*} $$
is a positive
![]() $(1,1)$
-form on
$(1,1)$
-form on
![]() $(\mathbf {C}^{2}\setminus \{0\})/\pm 1$
satisfying
$(\mathbf {C}^{2}\setminus \{0\})/\pm 1$
satisfying
its pull-back to
![]() $T^{*}\mathbf {P}^{1}$
$T^{*}\mathbf {P}^{1}$
extends to a Ricci-flat Kähler form on
![]() $T^{*}\mathbf {P}^{1}$
for
$T^{*}\mathbf {P}^{1}$
for
![]() $\epsilon>0$
, called the Eguchi-Hanson instanton. We write
$\epsilon>0$
, called the Eguchi-Hanson instanton. We write
![]() $\gamma ^{\mathrm {EH}}$
for
$\gamma ^{\mathrm {EH}}$
for
![]() $\gamma ^{\mathrm {EH}}_{1}$
. The coordinate change
$\gamma ^{\mathrm {EH}}_{1}$
. The coordinate change
![]() $z\mapsto \sqrt {\epsilon }z$
on
$z\mapsto \sqrt {\epsilon }z$
on
![]() $\mathbf {C}^{2}$
induces an isometry of Kähler manifolds
$\mathbf {C}^{2}$
induces an isometry of Kähler manifolds
When
![]() $\epsilon =0$
,
$\epsilon =0$
,
is the Euclidean Kähler form on
![]() $\mathbf {C}^{2}/\{\pm 1\}$
and
$\mathbf {C}^{2}/\{\pm 1\}$
and
![]() $\gamma ^{\mathrm {EH}}_{0}=\varPi ^{*}\{i\partial \bar {\partial }F_{0}(z)\}=\varPi ^{*}(i\partial \bar {\partial }\|z\|^{2})$
is a degenerate Kähler form on
$\gamma ^{\mathrm {EH}}_{0}=\varPi ^{*}\{i\partial \bar {\partial }F_{0}(z)\}=\varPi ^{*}(i\partial \bar {\partial }\|z\|^{2})$
is a degenerate Kähler form on
![]() $T^{*}\mathbf {P}^{1}$
.
$T^{*}\mathbf {P}^{1}$
.
Let
![]() $\omega _{\mathrm {FS}}$
be the Fubini-Study form on
$\omega _{\mathrm {FS}}$
be the Fubini-Study form on
![]() $\mathbf {P}^{1}$
such that
$\mathbf {P}^{1}$
such that
By the definition of
![]() $F_{\epsilon }$
, we get
$F_{\epsilon }$
, we get
4.2 Glueing of the Eguchi-Hanson instanton
4.2.1 A modification of the Eguchi-Hanson instanton
Let
![]() $B(r)\subset \mathbf {C}^{2}$
be the ball of radius
$B(r)\subset \mathbf {C}^{2}$
be the ball of radius
![]() $r>0$
centred at
$r>0$
centred at
![]() $0\in \mathbf {C}^{2}$
, and set
$0\in \mathbf {C}^{2}$
, and set
Let
![]() $\varPi \colon (\widetilde {V}(r),E)\to (V(r),0)$
be the blowing-up at the origin. Then
$\varPi \colon (\widetilde {V}(r),E)\to (V(r),0)$
be the blowing-up at the origin. Then
![]() $V(\infty )=\mathbf {C}^{2}/\pm 1$
and
$V(\infty )=\mathbf {C}^{2}/\pm 1$
and
![]() $\widetilde {V}(\infty )=T^{*}\mathbf {P}^{1}$
. For
$\widetilde {V}(\infty )=T^{*}\mathbf {P}^{1}$
. For
![]() $z\in (\mathbf {C}^{2}\setminus \{0\})/\pm 1$
and
$z\in (\mathbf {C}^{2}\setminus \{0\})/\pm 1$
and
![]() $\epsilon \geq 0$
, we define
$\epsilon \geq 0$
, we define
Since the error term
![]() $E(z,\epsilon )$
is a
$E(z,\epsilon )$
is a
![]() $C^{\omega }$
function on
$C^{\omega }$
function on
![]() $(V(4)\setminus V(1))\times [0,1]$
with
$(V(4)\setminus V(1))\times [0,1]$
with
![]() $E(z,0)=0$
, there is a constant
$E(z,0)=0$
, there is a constant
![]() $C_{k}$
for all
$C_{k}$
for all
![]() $k\geq 0$
with
$k\geq 0$
with
Let
![]() $\rho (t)$
be a
$\rho (t)$
be a
![]() $C^{\infty }$
function on
$C^{\infty }$
function on
![]() $\mathbf {R}$
such that
$\mathbf {R}$
such that
![]() $0\leq \rho (t)\leq 1$
on
$0\leq \rho (t)\leq 1$
on
![]() $\mathbf {R}$
,
$\mathbf {R}$
,
![]() $\rho (t)=1$
for
$\rho (t)=1$
for
![]() $t\leq 1$
and
$t\leq 1$
and
![]() $\rho (t)=0$
for
$\rho (t)=0$
for
![]() $t\geq 2$
. We set
$t\geq 2$
. We set
and we define a
![]() $(1,1)$
form on
$(1,1)$
form on
![]() $V(\infty )\setminus \{0\}$
by
$V(\infty )\setminus \{0\}$
by
Since
![]() $\phi _{\epsilon }(z)=F_{\epsilon }(z)$
on
$\phi _{\epsilon }(z)=F_{\epsilon }(z)$
on
![]() $V(1)$
,
$V(1)$
,
![]() $\kappa _{\epsilon }$
extends to a real
$\kappa _{\epsilon }$
extends to a real
![]() $(1,1)$
-form on
$(1,1)$
-form on
![]() $T^{*}\mathbf {P}^{1}$
, which is positive on
$T^{*}\mathbf {P}^{1}$
, which is positive on
![]() $\widetilde {V}(1)$
. Since
$\widetilde {V}(1)$
. Since
![]() $\phi _{\epsilon }(z)=\|z\|^{2}+\rho (\|z\|)\,E(z,\epsilon )$
on
$\phi _{\epsilon }(z)=\|z\|^{2}+\rho (\|z\|)\,E(z,\epsilon )$
on
![]() $V(2)\setminus V(1)$
, there exists by equation (4.2) a constant
$V(2)\setminus V(1)$
, there exists by equation (4.2) a constant
![]() $\epsilon (\rho )\in (0,1)$
depending only on the choice of the cut-off function
$\epsilon (\rho )\in (0,1)$
depending only on the choice of the cut-off function
![]() $\rho $
such that
$\rho $
such that
![]() $\kappa _{\epsilon }$
is a positive
$\kappa _{\epsilon }$
is a positive
![]() $(1,1)$
-form on
$(1,1)$
-form on
![]() $V(2)\setminus V(1)$
for
$V(2)\setminus V(1)$
for
![]() $0<\epsilon \leq \epsilon (\rho )$
. As a result,
$0<\epsilon \leq \epsilon (\rho )$
. As a result,
![]() $\{\kappa _{\epsilon }\}_{0<\epsilon \leq \epsilon (\rho )}$
is a family of Kähler forms on
$\{\kappa _{\epsilon }\}_{0<\epsilon \leq \epsilon (\rho )}$
is a family of Kähler forms on
![]() $T^{*}\mathbf {P}^{1}$
such that
$T^{*}\mathbf {P}^{1}$
such that
![]() $\kappa _{\epsilon }=i\partial \bar {\partial }\|z\|^{2}$
on
$\kappa _{\epsilon }=i\partial \bar {\partial }\|z\|^{2}$
on
![]() $T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2)$
.
$T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2)$
.
We have the following slightly refined estimate for the error term
![]() $E(z, \epsilon )$
. Set
$E(z, \epsilon )$
. Set
where
 $$ \begin{align*}E_{1}(z)=\sqrt{\|z\|^{4}+1}-\|z\|^{2}=\frac{1}{\sqrt{\|z\|^{4}+1}+\|z\|^{2}}, \quad E_{2}(z)=\log\frac{\|z\|^{2}}{\sqrt{\|z\|^{4}+1}+1}.\\[-15pt] \end{align*} $$
$$ \begin{align*}E_{1}(z)=\sqrt{\|z\|^{4}+1}-\|z\|^{2}=\frac{1}{\sqrt{\|z\|^{4}+1}+\|z\|^{2}}, \quad E_{2}(z)=\log\frac{\|z\|^{2}}{\sqrt{\|z\|^{4}+1}+1}.\\[-15pt] \end{align*} $$
Then for any nonnegative integer k, there exists a constant
![]() $C_{k}>0$
such that
$C_{k}>0$
such that
-
(i)
 $|\partial _{z}^{k}E_{1}(z)|\leq C_{k}(1+\|z\|)^{-(2+k)}$
for all
$|\partial _{z}^{k}E_{1}(z)|\leq C_{k}(1+\|z\|)^{-(2+k)}$
for all
 $z\in V(\infty )\setminus \{0\}$
;
$z\in V(\infty )\setminus \{0\}$
; -
(ii)
 $|\partial _{z}^{k}E_{2}(z)|\leq C_{k}(1+\|z\|)^{-(2+k)}$
for all
$|\partial _{z}^{k}E_{2}(z)|\leq C_{k}(1+\|z\|)^{-(2+k)}$
for all
 $z\in V(\infty )\setminus V(2)$
;
$z\in V(\infty )\setminus V(2)$
; -
(iii)
 $|\partial _{z}^{k}E_{2}(z)|\leq C_{k}\|z\|^{-k}$
for all
$|\partial _{z}^{k}E_{2}(z)|\leq C_{k}\|z\|^{-k}$
for all
 $z\in V(2)\setminus \{0\} k\geq 1$
;
$z\in V(2)\setminus \{0\} k\geq 1$
;
 $C_0 \log \|z\|^2$
for
$C_0 \log \|z\|^2$
for
 $k=0$
.
$k=0$
.
From these inequalities, we get
 $$ \begin{align} |\partial_{z}^{k}E(z)| \leq \begin{cases} \begin{array}{ll} C_{k}\|z\|^{-k} \ (k\geq 1; C_0 \log \|z\|^2, k=0) & (\forall\,z\in V(2)\setminus\{0\}), \\ C_{k}(1+\|z\|)^{-(2+k)} & (\forall\,z\in V(\infty)\setminus V(2)). \end{array} \end{cases} \\[-15pt]\nonumber\end{align} $$
$$ \begin{align} |\partial_{z}^{k}E(z)| \leq \begin{cases} \begin{array}{ll} C_{k}\|z\|^{-k} \ (k\geq 1; C_0 \log \|z\|^2, k=0) & (\forall\,z\in V(2)\setminus\{0\}), \\ C_{k}(1+\|z\|)^{-(2+k)} & (\forall\,z\in V(\infty)\setminus V(2)). \end{array} \end{cases} \\[-15pt]\nonumber\end{align} $$
Since
![]() $E(z,\epsilon )=\epsilon \,E(\frac {z}{\sqrt {\epsilon }},1)=\epsilon \,E(\frac {z}{\sqrt {\epsilon }})$
and hence
$E(z,\epsilon )=\epsilon \,E(\frac {z}{\sqrt {\epsilon }},1)=\epsilon \,E(\frac {z}{\sqrt {\epsilon }})$
and hence
![]() $\partial _{z}^{k}E(z,\epsilon )=\epsilon ^{1-\frac {k}{2}}(\partial _{z}^{k}E)(\frac {z}{\sqrt {\epsilon }})$
, we get by equation (4.3)
$\partial _{z}^{k}E(z,\epsilon )=\epsilon ^{1-\frac {k}{2}}(\partial _{z}^{k}E)(\frac {z}{\sqrt {\epsilon }})$
, we get by equation (4.3)
 $$ \begin{align} |\partial_{z}^{k}E(z,\epsilon)| \leq \begin{cases} \begin{array}{ll} C_{k}\epsilon\|z\|^{-k} \ (k\geq 1; C_0 \epsilon (\log \|z\|^2 + \log \epsilon), k=0) & (\forall\,z\in V(2)\setminus\{0\}), \\ C_{k}\epsilon^{2}(\sqrt{\epsilon}+\|z\|)^{-(2+k)} & (\forall\,z\in V(\infty)\setminus V(2)). \end{array} \end{cases}\\[-15pt]\nonumber \end{align} $$
$$ \begin{align} |\partial_{z}^{k}E(z,\epsilon)| \leq \begin{cases} \begin{array}{ll} C_{k}\epsilon\|z\|^{-k} \ (k\geq 1; C_0 \epsilon (\log \|z\|^2 + \log \epsilon), k=0) & (\forall\,z\in V(2)\setminus\{0\}), \\ C_{k}\epsilon^{2}(\sqrt{\epsilon}+\|z\|)^{-(2+k)} & (\forall\,z\in V(\infty)\setminus V(2)). \end{array} \end{cases}\\[-15pt]\nonumber \end{align} $$
Here, to get the estimate on
![]() $V(2)\setminus \{0\}$
, we used the fact
$V(2)\setminus \{0\}$
, we used the fact
![]() $\epsilon ^{2}(\sqrt {\epsilon } + \|z\|)^{-(2+k)} < \epsilon \|z\|^{-k}$
on
$\epsilon ^{2}(\sqrt {\epsilon } + \|z\|)^{-(2+k)} < \epsilon \|z\|^{-k}$
on
![]() $V(2) \setminus V(2\sqrt {\epsilon })$
. Replacing
$V(2) \setminus V(2\sqrt {\epsilon })$
. Replacing
![]() $\epsilon (\rho )$
by a smaller constant if necessary, we may assume by equation (4.4) the following inequality of Hermitian matrices for all
$\epsilon (\rho )$
by a smaller constant if necessary, we may assume by equation (4.4) the following inequality of Hermitian matrices for all
![]() $0<\epsilon \leq \epsilon (\rho )$
and
$0<\epsilon \leq \epsilon (\rho )$
and
![]() $z\in V(\infty )\setminus V(2)$
:
$z\in V(\infty )\setminus V(2)$
:
 $$ \begin{align} \frac{1}{2}(\delta_{ij}) \leq (\delta_{ij}+\frac{\partial^{2}E(z,\epsilon)}{\partial z_{i}\partial\bar{z}_{j}}) \leq 2(\delta_{ij}).\\[-15pt]\nonumber \end{align} $$
$$ \begin{align} \frac{1}{2}(\delta_{ij}) \leq (\delta_{ij}+\frac{\partial^{2}E(z,\epsilon)}{\partial z_{i}\partial\bar{z}_{j}}) \leq 2(\delta_{ij}).\\[-15pt]\nonumber \end{align} $$
Moreover, for
![]() $\|z\| \leq 2$
,
$\|z\| \leq 2$
,
Lemma 4.1. There exist constants
![]() $C_{1},C_{2}>0$
such that the following inequality of
$C_{1},C_{2}>0$
such that the following inequality of
![]() $(1,1)$
-forms on
$(1,1)$
-forms on
![]() $T^{*}\mathbf {P}^{1}$
hold for all
$T^{*}\mathbf {P}^{1}$
hold for all
![]() $0<\epsilon \leq \epsilon (\rho )$
:
$0<\epsilon \leq \epsilon (\rho )$
:
Proof.
(Step 1) On
![]() $\widetilde {V}(1)$
, we have
$\widetilde {V}(1)$
, we have
![]() $\kappa _{\epsilon }=\gamma _{\epsilon }^{\mathrm {EH}}$
. On
$\kappa _{\epsilon }=\gamma _{\epsilon }^{\mathrm {EH}}$
. On
![]() $\widetilde {V}(2)\setminus \widetilde {V}(1)$
, it follows from equation (4.2) that there exist constants
$\widetilde {V}(2)\setminus \widetilde {V}(1)$
, it follows from equation (4.2) that there exist constants
![]() $C_{1},C_{2}>0$
independent of
$C_{1},C_{2}>0$
independent of
![]() $\epsilon \in (0,\epsilon (\rho )]$
with
$\epsilon \in (0,\epsilon (\rho )]$
with
![]() $C_{1}\gamma _{\epsilon }^{\mathrm {EH}}\leq \kappa _{\epsilon }\leq C_{2}\gamma _{\epsilon }^{\mathrm {EH}}$
. Combining these two estimates, we get
$C_{1}\gamma _{\epsilon }^{\mathrm {EH}}\leq \kappa _{\epsilon }\leq C_{2}\gamma _{\epsilon }^{\mathrm {EH}}$
. Combining these two estimates, we get
![]() $C_{1}\gamma _{\epsilon }^{\mathrm {EH}}\leq \kappa _{\epsilon }\leq C_{2}\gamma _{\epsilon }^{\mathrm {EH}}$
on
$C_{1}\gamma _{\epsilon }^{\mathrm {EH}}\leq \kappa _{\epsilon }\leq C_{2}\gamma _{\epsilon }^{\mathrm {EH}}$
on
![]() $\widetilde {V}(2)$
.
$\widetilde {V}(2)$
.
(Step 2) We compare
![]() $\kappa _{\epsilon }$
and
$\kappa _{\epsilon }$
and
![]() $\gamma _{\epsilon }^{\mathrm {EH}}$
on
$\gamma _{\epsilon }^{\mathrm {EH}}$
on
![]() $T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2)$
. On
$T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2)$
. On
![]() $T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2)$
, we have
$T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2)$
, we have
![]() $\kappa _{\epsilon }=\gamma ^{\mathrm {EH}}_{0}$
. By equation (4.5), we have
$\kappa _{\epsilon }=\gamma ^{\mathrm {EH}}_{0}$
. By equation (4.5), we have
![]() $\frac {1}{2}\gamma ^{\mathrm {EH}}_{\epsilon }\leq \gamma ^{\mathrm {EH}}_{0}\leq 2\gamma ^{\mathrm {EH}}_{\epsilon }$
on
$\frac {1}{2}\gamma ^{\mathrm {EH}}_{\epsilon }\leq \gamma ^{\mathrm {EH}}_{0}\leq 2\gamma ^{\mathrm {EH}}_{\epsilon }$
on
![]() $T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2)$
. Since
$T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2)$
. Since
![]() $\kappa _{\epsilon }=\gamma ^{\mathrm {EH}}_{0}$
on
$\kappa _{\epsilon }=\gamma ^{\mathrm {EH}}_{0}$
on
![]() $T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2)$
, We get the desired estimate on
$T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2)$
, We get the desired estimate on
![]() $T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2)$
. This completes the proof.
$T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2)$
. This completes the proof.
4.2.2 A family of Kähler metrics on
 $\widetilde {X}$
$\widetilde {X}$
Since
![]() $E_{\frak p}$
is a
$E_{\frak p}$
is a
![]() $(-2)$
-curve on
$(-2)$
-curve on
![]() $\widetilde {X}$
, there exist a neighbourhood
$\widetilde {X}$
, there exist a neighbourhood
![]() $U_{\frak p}$
of
$U_{\frak p}$
of
![]() $E_{\frak p}$
in
$E_{\frak p}$
in
![]() $\widetilde {X}$
and an isomorphism of pairs
$\widetilde {X}$
and an isomorphism of pairs
We may and will assume that
![]() $\psi _{\frak p}$
extends to an isomorphism between an open subset of
$\psi _{\frak p}$
extends to an isomorphism between an open subset of
![]() $\widetilde {X}$
containing
$\widetilde {X}$
containing
![]() $U_{\frak p}$
and
$U_{\frak p}$
and
![]() $\widetilde {V}(4)$
. We write
$\widetilde {V}(4)$
. We write
![]() $V(r)_{{\frak p}}$
for
$V(r)_{{\frak p}}$
for
![]() $V(r)$
viewed as a neighbourhood of
$V(r)$
viewed as a neighbourhood of
![]() ${\frak p}\in \mathrm {Sing}(X)$
. In what follows, we identify
${\frak p}\in \mathrm {Sing}(X)$
. In what follows, we identify
![]() $\widetilde {V}(r)_{{\frak p}}$
with
$\widetilde {V}(r)_{{\frak p}}$
with
![]() $\psi _{\frak p}^{-1}(\widetilde {V}(r)_{{\frak p}})$
.
$\psi _{\frak p}^{-1}(\widetilde {V}(r)_{{\frak p}})$
.
Let
![]() $\gamma $
be a
$\gamma $
be a
![]() $\theta $
-invariant Kähler form on X in the sense of orbifolds, which has a potential function on every
$\theta $
-invariant Kähler form on X in the sense of orbifolds, which has a potential function on every
![]() $V(4)_{\frak p}$
. By modifying the potential of
$V(4)_{\frak p}$
. By modifying the potential of
![]() $\gamma $
on each
$\gamma $
on each
![]() $V(4)_{\frak p}$
(compare [Reference Yoshikawa43, Proof of Lemma 6.2]), there exists a Kähler form
$V(4)_{\frak p}$
(compare [Reference Yoshikawa43, Proof of Lemma 6.2]), there exists a Kähler form
![]() $\gamma _{0}$
on X in the sense of orbifolds such that
$\gamma _{0}$
on X in the sense of orbifolds such that
In particular,
![]() $\|z\|^{2}\in C^{\omega }(V(2)_{\frak p})$
is a potential function of
$\|z\|^{2}\in C^{\omega }(V(2)_{\frak p})$
is a potential function of
![]() $\gamma _{0}$
on every
$\gamma _{0}$
on every
![]() $V(2)_{\frak p}$
. Since
$V(2)_{\frak p}$
. Since
![]() $\phi _{\epsilon }(z)=\|z\|^{2}$
near
$\phi _{\epsilon }(z)=\|z\|^{2}$
near
![]() $\partial V(2)_{\frak p}$
, we can glue the Kähler form
$\partial V(2)_{\frak p}$
, we can glue the Kähler form
![]() $\kappa _{\epsilon }$
on
$\kappa _{\epsilon }$
on
![]() $\bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(2)_{\frak p}$
and the Kähler form
$\bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(2)_{\frak p}$
and the Kähler form
![]() $\gamma _{0}$
on
$\gamma _{0}$
on
![]() $X\setminus \bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(2)_{\frak p}$
by setting
$X\setminus \bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(2)_{\frak p}$
by setting
 $$ \begin{align} \gamma_{\epsilon} := \begin{cases} \begin{array}{ll} \kappa_{\epsilon}&\mbox{on }\bigcup_{{\frak p}\in\mathrm{Sing}(X)}\widetilde{V}(2)_{\frak p}, \\ \gamma_{0}&\mbox{on }X\setminus\bigcup_{{\frak p}\in\mathrm{Sing}(X)}\widetilde{V}(2)_{\frak p}. \end{array} \end{cases}\\[-17pt]\nonumber \end{align} $$
$$ \begin{align} \gamma_{\epsilon} := \begin{cases} \begin{array}{ll} \kappa_{\epsilon}&\mbox{on }\bigcup_{{\frak p}\in\mathrm{Sing}(X)}\widetilde{V}(2)_{\frak p}, \\ \gamma_{0}&\mbox{on }X\setminus\bigcup_{{\frak p}\in\mathrm{Sing}(X)}\widetilde{V}(2)_{\frak p}. \end{array} \end{cases}\\[-17pt]\nonumber \end{align} $$
By construction,
![]() $\{\gamma _{\epsilon }\}_{0<\epsilon \leq \epsilon (\rho )}$
is a family of
$\{\gamma _{\epsilon }\}_{0<\epsilon \leq \epsilon (\rho )}$
is a family of
![]() $\theta $
-invariant Kähler forms on
$\theta $
-invariant Kähler forms on
![]() $\widetilde {X}$
.
$\widetilde {X}$
.
Lemma 4.2. The family of Kähler forms
![]() $\{\gamma _{\epsilon }\}_{0<\epsilon \leq \epsilon (\rho )}$
on
$\{\gamma _{\epsilon }\}_{0<\epsilon \leq \epsilon (\rho )}$
on
![]() $\widetilde {X}$
satisfies the following:
$\widetilde {X}$
satisfies the following:
-
(1) For all
 ${\frak p}\in \mathrm {Sing}(X)$
,
${\frak p}\in \mathrm {Sing}(X)$
,
 $\gamma _{0}|_{V(2)_{\frak p}}=i\partial \bar {\partial }\|z\|^{2}$
.
$\gamma _{0}|_{V(2)_{\frak p}}=i\partial \bar {\partial }\|z\|^{2}$
. -
(2) For all
 ${\frak p}\in \mathrm {Sing}(X)$
,
${\frak p}\in \mathrm {Sing}(X)$
,
 $\gamma _{\epsilon }|_{\widetilde {V}(1)_{\frak p}}=\psi _{\frak p}^{*}\gamma ^{\mathrm {EH}}_{\epsilon }$
.
$\gamma _{\epsilon }|_{\widetilde {V}(1)_{\frak p}}=\psi _{\frak p}^{*}\gamma ^{\mathrm {EH}}_{\epsilon }$
. -
(3) On
 $\widetilde {X}$
,
$\widetilde {X}$
,
 $\gamma _{\epsilon }$
converges to
$\gamma _{\epsilon }$
converges to
 $\pi ^{*}\gamma _{0}$
in the
$\pi ^{*}\gamma _{0}$
in the
 $C^{\infty }$
-topology.
$C^{\infty }$
-topology. -
(4) There exist constants
 $C, C'>0$
independent of
$C, C'>0$
independent of
 $\epsilon $
(but depending on
$\epsilon $
(but depending on
 $\rho $
) such that
$\rho $
) such that
 $|\mathrm {Ric}(\gamma _{\epsilon })|_{\gamma _{\epsilon }}\leq C\cdot \epsilon $
on
$|\mathrm {Ric}(\gamma _{\epsilon })|_{\gamma _{\epsilon }}\leq C\cdot \epsilon $
on
 $\bigcup _{{\frak p}\in \mathrm {Sing}\,X}\widetilde {V}(2)_{\frak p}$
and
$\bigcup _{{\frak p}\in \mathrm {Sing}\,X}\widetilde {V}(2)_{\frak p}$
and
 $|\mathrm {Ric}(\gamma _{\epsilon })|_{\gamma _{\epsilon }}\leq C'$
on
$|\mathrm {Ric}(\gamma _{\epsilon })|_{\gamma _{\epsilon }}\leq C'$
on
 $\widetilde {X}$
.
$\widetilde {X}$
.
Proof. By construction, (1), (2), (3) are obvious. Let us see (4). Since
![]() $\gamma _{\epsilon }^{\mathrm {EH}}$
is Ricci-flat and since
$\gamma _{\epsilon }^{\mathrm {EH}}$
is Ricci-flat and since
![]() $\kappa _{\epsilon }=\gamma _{\epsilon }^{\mathrm {EH}}$
on
$\kappa _{\epsilon }=\gamma _{\epsilon }^{\mathrm {EH}}$
on
![]() $\widetilde {V}(1)_{\frak p}$
, we get
$\widetilde {V}(1)_{\frak p}$
, we get
![]() $\mathrm {Ric}(\kappa _{\epsilon })=\mathrm {Ric}(\gamma _{\epsilon }^{\mathrm {EH}})=0$
on
$\mathrm {Ric}(\kappa _{\epsilon })=\mathrm {Ric}(\gamma _{\epsilon }^{\mathrm {EH}})=0$
on
![]() $\widetilde {V}(1)_{\frak p}$
. On
$\widetilde {V}(1)_{\frak p}$
. On
![]() $\widetilde {V}(2)_{\frak p}\setminus \widetilde {V}(1)_{\frak p}$
, we get
$\widetilde {V}(2)_{\frak p}\setminus \widetilde {V}(1)_{\frak p}$
, we get
![]() $|\mathrm {Ric}(\gamma _{\epsilon })|_{\gamma _{\epsilon }}=|\mathrm {Ric}(\kappa _{\epsilon })|_{\kappa _{\epsilon }}\leq C\cdot \epsilon $
by equation (4.2). This proves the first estimate. Since
$|\mathrm {Ric}(\gamma _{\epsilon })|_{\gamma _{\epsilon }}=|\mathrm {Ric}(\kappa _{\epsilon })|_{\kappa _{\epsilon }}\leq C\cdot \epsilon $
by equation (4.2). This proves the first estimate. Since
![]() $\gamma _{\epsilon }=\gamma _{0}$
on
$\gamma _{\epsilon }=\gamma _{0}$
on
![]() $X\setminus \bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(2)_{\frak p}$
, we get the second estimate.
$X\setminus \bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(2)_{\frak p}$
, we get the second estimate.
4.2.3 A two-parameter family of Kähler metrics on
 $T^{*}\mathbf {P}^{1}$
$T^{*}\mathbf {P}^{1}$
For later use, we introduce another small parameter
![]() $\delta>0$
. Instead of glueing in the Eguchi-Hanson instanton in the region
$\delta>0$
. Instead of glueing in the Eguchi-Hanson instanton in the region
![]() $\widetilde {V}(2)-\widetilde {V}(1)$
, we now do it in the region
$\widetilde {V}(2)-\widetilde {V}(1)$
, we now do it in the region
![]() $\widetilde {V}(2\delta )-\widetilde {V}(\delta )$
. This is effected by replacing the cut-off function
$\widetilde {V}(2\delta )-\widetilde {V}(\delta )$
. This is effected by replacing the cut-off function
![]() $\rho (t)$
by
$\rho (t)$
by
![]() $\rho _{\delta }(t)=\rho (\frac {t}{\delta })$
in defining the Kähler potential
$\rho _{\delta }(t)=\rho (\frac {t}{\delta })$
in defining the Kähler potential
![]() $\phi _{\epsilon }$
for the Kähler metric
$\phi _{\epsilon }$
for the Kähler metric
![]() $\gamma _{\epsilon }$
such that
$\gamma _{\epsilon }$
such that
![]() $\rho _{\delta }(t)=1$
for
$\rho _{\delta }(t)=1$
for
![]() $t\leq \delta $
and
$t\leq \delta $
and
![]() $\rho _{\delta }(t)=0$
for
$\rho _{\delta }(t)=0$
for
![]() $t\geq 2\delta $
. This gives us the family of real
$t\geq 2\delta $
. This gives us the family of real
![]() $(1,1)$
-forms on
$(1,1)$
-forms on
![]() $T^{*}\mathbf {P}^{1}$
$T^{*}\mathbf {P}^{1}$
where
To verify the positivity of
![]() $\kappa _{\epsilon , \delta }$
, we see the relation between
$\kappa _{\epsilon , \delta }$
, we see the relation between
![]() $\phi _{\epsilon }$
and
$\phi _{\epsilon }$
and
![]() $\phi _{\epsilon , \delta }$
. Since
$\phi _{\epsilon , \delta }$
. Since
![]() $F_{\epsilon }(\delta z)=\delta ^{2}F_{\epsilon /\delta ^{2}}(z)$
, we get
$F_{\epsilon }(\delta z)=\delta ^{2}F_{\epsilon /\delta ^{2}}(z)$
, we get
![]() $E(\delta z,\epsilon )=\delta ^{2}E(z,\epsilon /\delta ^{2})$
. Since
$E(\delta z,\epsilon )=\delta ^{2}E(z,\epsilon /\delta ^{2})$
. Since
![]() $\phi _{\epsilon ,1}(z)=\phi _{\epsilon }(z)$
and
$\phi _{\epsilon ,1}(z)=\phi _{\epsilon }(z)$
and
![]() $\phi _{\epsilon , \delta }(z)=\|\delta \cdot \frac {z}{\delta }\|^{2}+\rho (\frac {\|z\|}{\delta })E(\delta \cdot \frac {z}{\delta },\epsilon )$
, this implies that
$\phi _{\epsilon , \delta }(z)=\|\delta \cdot \frac {z}{\delta }\|^{2}+\rho (\frac {\|z\|}{\delta })E(\delta \cdot \frac {z}{\delta },\epsilon )$
, this implies that
Hence if
![]() $0<\epsilon /\delta ^{2}\leq \epsilon (\rho )$
, then
$0<\epsilon /\delta ^{2}\leq \epsilon (\rho )$
, then
![]() $\kappa _{\epsilon , \delta }=i\partial \bar {\partial }\phi _{\epsilon , \delta }$
is a positive
$\kappa _{\epsilon , \delta }=i\partial \bar {\partial }\phi _{\epsilon , \delta }$
is a positive
![]() $(1,1)$
-form on
$(1,1)$
-form on
![]() $T^{*}\mathbf {P}^{1}$
. In what follows, we define
$T^{*}\mathbf {P}^{1}$
. In what follows, we define
![]() $\phi _{\epsilon , \delta }$
for
$\phi _{\epsilon , \delta }$
for
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $0<\epsilon /\delta ^{2}\leq \epsilon (\rho )$
. Then
$0<\epsilon /\delta ^{2}\leq \epsilon (\rho )$
. Then
![]() $\{\kappa _{\epsilon ,\delta }\}_{0<\epsilon /\delta ^{2}\leq \epsilon (\rho ),\,\epsilon ,\delta \in (0,1]}$
is a family of Kähler forms on
$\{\kappa _{\epsilon ,\delta }\}_{0<\epsilon /\delta ^{2}\leq \epsilon (\rho ),\,\epsilon ,\delta \in (0,1]}$
is a family of Kähler forms on
![]() $T^{*}\mathbf {P}^{1}$
. Moreover, the relation
$T^{*}\mathbf {P}^{1}$
. Moreover, the relation
![]() $\phi _{\epsilon , \delta }(z)=\delta ^{2}\phi _{\epsilon /\delta ^{2}}(z/\delta )$
implies that the automorphism of
$\phi _{\epsilon , \delta }(z)=\delta ^{2}\phi _{\epsilon /\delta ^{2}}(z/\delta )$
implies that the automorphism of
![]() $T^{*}\mathbf {P}^{1}$
induced from the one
$T^{*}\mathbf {P}^{1}$
induced from the one
![]() $z\mapsto z/\delta $
on
$z\mapsto z/\delta $
on
![]() $V(\infty )$
yields an isometry of Kähler manifolds
$V(\infty )$
yields an isometry of Kähler manifolds
![]() $(T^{*}\mathbf {P}^{1},\kappa _{\epsilon , \delta })\cong (T^{*}\mathbf {P}^{1},\delta ^{2}\kappa _{\epsilon /\delta ^{2}})$
such that
$(T^{*}\mathbf {P}^{1},\kappa _{\epsilon , \delta })\cong (T^{*}\mathbf {P}^{1},\delta ^{2}\kappa _{\epsilon /\delta ^{2}})$
such that
Lemma 4.3. There exist constants
![]() $C_{1},C_{2}>0$
such that the following inequality of
$C_{1},C_{2}>0$
such that the following inequality of
![]() $(1,1)$
-forms on
$(1,1)$
-forms on
![]() $T^{*}\mathbf {P}^{1}$
holds for all
$T^{*}\mathbf {P}^{1}$
holds for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $0<\epsilon /\delta ^{2}\leq \epsilon (\rho )$
:
$0<\epsilon /\delta ^{2}\leq \epsilon (\rho )$
:
Proof.
(Step 1) By Lemma 4.3 (Step 1), we get
![]() $C_{1}\gamma _{\epsilon }^{\mathrm {EH}}\leq \kappa _{\epsilon }\leq C_{2}\gamma _{\epsilon }^{\mathrm {EH}}$
on
$C_{1}\gamma _{\epsilon }^{\mathrm {EH}}\leq \kappa _{\epsilon }\leq C_{2}\gamma _{\epsilon }^{\mathrm {EH}}$
on
![]() $\widetilde {V}(2)$
. By equation (4.9) and the relation
$\widetilde {V}(2)$
. By equation (4.9) and the relation
![]() $\delta ^{2}\gamma _{\epsilon /\delta ^{2}}^{\mathrm {EH}}=\gamma _{\epsilon }^{\mathrm {EH}}$
, this implies the inequality
$\delta ^{2}\gamma _{\epsilon /\delta ^{2}}^{\mathrm {EH}}=\gamma _{\epsilon }^{\mathrm {EH}}$
, this implies the inequality
![]() $C_{1}\gamma _{\epsilon }^{\mathrm {EH}}\leq \kappa _{\epsilon ,\delta }\leq C_{2}\gamma _{\epsilon }^{\mathrm {EH}}$
on
$C_{1}\gamma _{\epsilon }^{\mathrm {EH}}\leq \kappa _{\epsilon ,\delta }\leq C_{2}\gamma _{\epsilon }^{\mathrm {EH}}$
on
![]() $\widetilde {V}(2\delta )$
. Hence we get
$\widetilde {V}(2\delta )$
. Hence we get
![]() $C_{1}C_{2}^{-1}\kappa _{\epsilon }\leq \kappa _{\epsilon ,\delta }\leq C_{2}C_{1}^{-1}\kappa _{\epsilon }$
on
$C_{1}C_{2}^{-1}\kappa _{\epsilon }\leq \kappa _{\epsilon ,\delta }\leq C_{2}C_{1}^{-1}\kappa _{\epsilon }$
on
![]() $\widetilde {V}(2\delta )$
.
$\widetilde {V}(2\delta )$
.
(Step 2) Next we compare
![]() $\kappa _{\epsilon ,\delta }$
and
$\kappa _{\epsilon ,\delta }$
and
![]() $\kappa _{\epsilon }$
on
$\kappa _{\epsilon }$
on
![]() $T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2\delta )$
. By definition, we have
$T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2\delta )$
. By definition, we have
![]() $\kappa _{\epsilon ,\delta }=\gamma _{0}^{\mathrm {EH}}$
on
$\kappa _{\epsilon ,\delta }=\gamma _{0}^{\mathrm {EH}}$
on
![]() $T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2\delta )$
. Let
$T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2\delta )$
. Let
![]() $H_{\epsilon }$
be the automorphism of
$H_{\epsilon }$
be the automorphism of
![]() $T^{*}\mathbf {P}^{1}$
induced from the automorphism
$T^{*}\mathbf {P}^{1}$
induced from the automorphism
![]() $z\mapsto \sqrt {\epsilon }z$
of
$z\mapsto \sqrt {\epsilon }z$
of
![]() $V(\infty )=\mathbf {C}^{2}/\pm 1$
. Then
$V(\infty )=\mathbf {C}^{2}/\pm 1$
. Then
![]() $H_{\epsilon }$
is an isomorphism from
$H_{\epsilon }$
is an isomorphism from
![]() $T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2\delta /\sqrt {\epsilon })$
to
$T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2\delta /\sqrt {\epsilon })$
to
![]() $T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2\delta )$
inducing the isometries
$T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2\delta )$
inducing the isometries
Since
![]() $\epsilon /\delta ^{2}\leq \epsilon (\rho )$
and hence
$\epsilon /\delta ^{2}\leq \epsilon (\rho )$
and hence
![]() $\delta /\sqrt {\epsilon }>1/\sqrt {\epsilon (\rho )}$
, we have the inclusion
$\delta /\sqrt {\epsilon }>1/\sqrt {\epsilon (\rho )}$
, we have the inclusion
![]() $T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(\frac {2\delta }{\sqrt {\epsilon }}) \subset T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2/\sqrt {\epsilon (\rho )})$
. By equation (4.4), there exist constants
$T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(\frac {2\delta }{\sqrt {\epsilon }}) \subset T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2/\sqrt {\epsilon (\rho )})$
. By equation (4.4), there exist constants
![]() $C^{\prime }_{1},C^{\prime }_{2}>0$
such that
$C^{\prime }_{1},C^{\prime }_{2}>0$
such that
![]() $C^{\prime }_{1}\gamma ^{\mathrm {EH}}\leq \gamma ^{\mathrm {EH}}_{0}\leq C^{\prime }_{2}\gamma ^{\mathrm {EH}}$
on
$C^{\prime }_{1}\gamma ^{\mathrm {EH}}\leq \gamma ^{\mathrm {EH}}_{0}\leq C^{\prime }_{2}\gamma ^{\mathrm {EH}}$
on
![]() $T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2/\sqrt {\epsilon (\rho )})$
. This, together with equations (4.10) and (4.11), yields the inequality
$T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2/\sqrt {\epsilon (\rho )})$
. This, together with equations (4.10) and (4.11), yields the inequality
![]() $C^{\prime }_{1}\gamma ^{\mathrm {EH}}_{\epsilon }\leq \gamma ^{\mathrm {EH}}_{0}\leq C^{\prime }_{2}\gamma ^{\mathrm {EH}}_{\epsilon }$
on
$C^{\prime }_{1}\gamma ^{\mathrm {EH}}_{\epsilon }\leq \gamma ^{\mathrm {EH}}_{0}\leq C^{\prime }_{2}\gamma ^{\mathrm {EH}}_{\epsilon }$
on
![]() $T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2\delta )$
for all
$T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2\delta )$
for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $0<\epsilon /\delta ^{2}\leq \epsilon (\rho )$
. Since
$0<\epsilon /\delta ^{2}\leq \epsilon (\rho )$
. Since
![]() $\kappa _{\epsilon ,\delta }=\gamma _{0}^{\mathrm {EH}}$
on
$\kappa _{\epsilon ,\delta }=\gamma _{0}^{\mathrm {EH}}$
on
![]() $T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2\delta )$
, we get
$T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2\delta )$
, we get
![]() $C^{\prime }_{1}\gamma ^{\mathrm {EH}}_{\epsilon }\leq \kappa _{\epsilon ,\delta }\leq C^{\prime }_{2}\gamma ^{\mathrm {EH}}_{\epsilon }$
on
$C^{\prime }_{1}\gamma ^{\mathrm {EH}}_{\epsilon }\leq \kappa _{\epsilon ,\delta }\leq C^{\prime }_{2}\gamma ^{\mathrm {EH}}_{\epsilon }$
on
![]() $T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2\delta )$
. By Lemma 4.1, this implies the inequality
$T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2\delta )$
. By Lemma 4.1, this implies the inequality
![]() $C^{\prime \prime }_{1}\kappa _{\epsilon }\leq \kappa _{\epsilon ,\delta }\leq C^{\prime \prime }_{2}\kappa _{\epsilon }$
on
$C^{\prime \prime }_{1}\kappa _{\epsilon }\leq \kappa _{\epsilon ,\delta }\leq C^{\prime \prime }_{2}\kappa _{\epsilon }$
on
![]() $T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2\delta )$
, where
$T^{*}\mathbf {P}^{1}\setminus \widetilde {V}(2\delta )$
, where
![]() $C^{\prime \prime }_{1},C^{\prime \prime }_{2}>0$
are constants independent of
$C^{\prime \prime }_{1},C^{\prime \prime }_{2}>0$
are constants independent of
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $0<\epsilon /\delta ^{2}\leq \epsilon (\rho )$
. This completes the proof.
$0<\epsilon /\delta ^{2}\leq \epsilon (\rho )$
. This completes the proof.
4.2.4 A two-parameter family of Kähler metrics on
 $\widetilde {X}$
$\widetilde {X}$
Modifying the construction in equation (4.8), we introduce a two-parameter family of
![]() $\theta $
-invariant Kähler forms on
$\theta $
-invariant Kähler forms on
![]() $\widetilde {X}$
by
$\widetilde {X}$
by
 $$ \begin{align} \gamma_{\epsilon,\delta} := \begin{cases} \begin{array}{ll} \kappa_{\epsilon,\delta}&\mbox{on }\bigcup_{{\frak p}\in\mathrm{Sing}(X)}\widetilde{V}(2)_{\frak p}, \\ \gamma_{0}&\mbox{on }X\setminus\bigcup_{{\frak p}\in\mathrm{Sing}(X)}\widetilde{V}(2)_{\frak p} \end{array} \end{cases}\\[-15pt]\nonumber \end{align} $$
$$ \begin{align} \gamma_{\epsilon,\delta} := \begin{cases} \begin{array}{ll} \kappa_{\epsilon,\delta}&\mbox{on }\bigcup_{{\frak p}\in\mathrm{Sing}(X)}\widetilde{V}(2)_{\frak p}, \\ \gamma_{0}&\mbox{on }X\setminus\bigcup_{{\frak p}\in\mathrm{Sing}(X)}\widetilde{V}(2)_{\frak p} \end{array} \end{cases}\\[-15pt]\nonumber \end{align} $$
for
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $0<\epsilon /\delta ^{2}\leq \epsilon (\rho )$
.
$0<\epsilon /\delta ^{2}\leq \epsilon (\rho )$
.
Lemma 4.4. There exist constants
![]() $C_{1},C_{2}>0$
such that the following inequality of
$C_{1},C_{2}>0$
such that the following inequality of
![]() $(1,1)$
-forms on
$(1,1)$
-forms on
![]() $\widetilde {X}$
hold for all
$\widetilde {X}$
hold for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $0<\epsilon /\delta ^{2}\leq \epsilon (\rho )$
:
$0<\epsilon /\delta ^{2}\leq \epsilon (\rho )$
:
Proof. On
![]() $\bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(2)_{\frak p}$
, the result follows from Lemma 4.3. On
$\bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(2)_{\frak p}$
, the result follows from Lemma 4.3. On
![]() $X\setminus \bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(2)_{\frak p}$
, the result is obvious since
$X\setminus \bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(2)_{\frak p}$
, the result is obvious since
![]() $\gamma _{\epsilon ,\delta }=\gamma _{\epsilon }=\gamma _{0}$
is independent of
$\gamma _{\epsilon ,\delta }=\gamma _{\epsilon }=\gamma _{0}$
is independent of
![]() $\epsilon ,\delta $
there.
$\epsilon ,\delta $
there.
Lemma 4.5. There exists a constant
![]() $C_{3}>0$
such that the following estimate holds for all
$C_{3}>0$
such that the following estimate holds for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $0<\epsilon /\delta ^{2}\leq \epsilon (\rho )$
:
$0<\epsilon /\delta ^{2}\leq \epsilon (\rho )$
:
Proof. Since
![]() $\gamma _{\epsilon ,\delta }=\gamma _{0}$
on
$\gamma _{\epsilon ,\delta }=\gamma _{0}$
on
![]() $X\setminus \bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(2)_{\frak p}$
, it suffices to prove the estimate on
$X\setminus \bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(2)_{\frak p}$
, it suffices to prove the estimate on
![]() $\bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(2)_{\frak p}$
. Since
$\bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(2)_{\frak p}$
. Since
![]() $\gamma _{\epsilon ,\delta }=i\partial \bar {\partial }\|z\|^{2}$
is a flat metric on
$\gamma _{\epsilon ,\delta }=i\partial \bar {\partial }\|z\|^{2}$
is a flat metric on
![]() $\bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(2)_{\frak p}\setminus \widetilde {V}(2\delta )_{\frak p}$
, it suffices to prove the estimate on
$\bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(2)_{\frak p}\setminus \widetilde {V}(2\delta )_{\frak p}$
, it suffices to prove the estimate on
![]() $\bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(2\delta )_{\frak p}$
. By equation (4.9), we get on each
$\bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(2\delta )_{\frak p}$
. By equation (4.9), we get on each
![]() $\widetilde {V}(2\delta )_{\frak p}$
$\widetilde {V}(2\delta )_{\frak p}$
where we used Lemma 4.2 (4) to get the inequality
![]() $|\mathrm {Ric}(\gamma _{\epsilon /\delta ^{2}})|_{\gamma _{\epsilon /\delta ^{2}}}\leq C(\epsilon /\delta ^{2})$
on
$|\mathrm {Ric}(\gamma _{\epsilon /\delta ^{2}})|_{\gamma _{\epsilon /\delta ^{2}}}\leq C(\epsilon /\delta ^{2})$
on
![]() $\widetilde {V}(2\delta )_{\frak p}$
. This completes the proof.
$\widetilde {V}(2\delta )_{\frak p}$
. This completes the proof.
Fix a nowhere vanishing holomorphic
![]() $2$
-form
$2$
-form
Since
![]() $(\varPi ^{-1})^{*}(\eta |_{V(1)_{\frak p}})$
is a nowhere vanishing holomorphic
$(\varPi ^{-1})^{*}(\eta |_{V(1)_{\frak p}})$
is a nowhere vanishing holomorphic
![]() $2$
-form on
$2$
-form on
![]() $V(1)_{\frak p}\setminus \{0\}$
, there exists by the Hartogs extension theorem a nowhere vanishing holomorphic function
$V(1)_{\frak p}\setminus \{0\}$
, there exists by the Hartogs extension theorem a nowhere vanishing holomorphic function
![]() $f_{\frak p}(z)$
on
$f_{\frak p}(z)$
on
![]() $B(1)$
such that
$B(1)$
such that
and
![]() $f_{\frak p}(-z)=f_{\frak p}(z)$
. Since
$f_{\frak p}(-z)=f_{\frak p}(z)$
. Since
![]() $\gamma _{\epsilon ,\delta }=\gamma _{\epsilon }$
on
$\gamma _{\epsilon ,\delta }=\gamma _{\epsilon }$
on
![]() $\widetilde {V}(\delta )_{\frak p}$
and hence
$\widetilde {V}(\delta )_{\frak p}$
and hence
we get the equality of functions on
![]() $\widetilde {V}(\delta )_{\frak p}$
$\widetilde {V}(\delta )_{\frak p}$
 $$ \begin{align} \left.\frac{\eta\wedge\overline{\eta}}{\gamma_{\epsilon,\delta}^{2}/2!}\right|_{\widetilde{V}(\delta)_{\frak p}} = \pi^{*}|f_{\frak p}(z)|^{2}. \end{align} $$
$$ \begin{align} \left.\frac{\eta\wedge\overline{\eta}}{\gamma_{\epsilon,\delta}^{2}/2!}\right|_{\widetilde{V}(\delta)_{\frak p}} = \pi^{*}|f_{\frak p}(z)|^{2}. \end{align} $$
In particular, we have the following:
-
(i) On each
 $\widetilde {V}(\epsilon )_{\frak p}$
, the volume form of
$\widetilde {V}(\epsilon )_{\frak p}$
, the volume form of
 $\gamma _{\epsilon ,\delta }$
is independent of
$\gamma _{\epsilon ,\delta }$
is independent of
 $\epsilon \in (0,\epsilon (\rho )]$
.
$\epsilon \in (0,\epsilon (\rho )]$
. -
(ii)
 $f_{\frak p}(0)$
is independent of
$f_{\frak p}(0)$
is independent of
 $\delta \in (0,1]$
and the choice of the cut-off function
$\delta \in (0,1]$
and the choice of the cut-off function
 $\rho $
.
$\rho $
.
Since
![]() $\gamma _{\epsilon ,\delta }$
converges to
$\gamma _{\epsilon ,\delta }$
converges to
![]() $\gamma _{0}$
outside
$\gamma _{0}$
outside
![]() $\bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(\delta )_{\frak p}$
, we get the continuity
$\bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(\delta )_{\frak p}$
, we get the continuity
4.3 Ricci-flat Kähler form on the blowing-down of
 $\widetilde {X}^{\theta }$
$\widetilde {X}^{\theta }$
Recall that
is the blowing-down of the disjoint union of
![]() $(-2)$
-curves
$(-2)$
-curves
![]() $\widetilde {X}^{\theta } = \amalg _{{\mathfrak p} \in \mathrm {Sing}\,X} E_{\mathfrak p}$
. Then
$\widetilde {X}^{\theta } = \amalg _{{\mathfrak p} \in \mathrm {Sing}\,X} E_{\mathfrak p}$
. Then
![]() ${\frak p}=\pi (E_{\frak p})$
. Under the identification
${\frak p}=\pi (E_{\frak p})$
. Under the identification
![]() $\psi _{\frak p}\colon (U_{\frak p},E_{\frak p})\cong (\widetilde {V}(1)_{\frak p},E)$
,
$\psi _{\frak p}\colon (U_{\frak p},E_{\frak p})\cong (\widetilde {V}(1)_{\frak p},E)$
,
![]() $\pi \colon \widetilde {X}\to X$
is identified with the blowing-down
$\pi \colon \widetilde {X}\to X$
is identified with the blowing-down
![]() $\varPi \colon T^{*}\mathbf {P}^{1}\to \mathbf {C}^{2}/\{\pm 1\}$
on each
$\varPi \colon T^{*}\mathbf {P}^{1}\to \mathbf {C}^{2}/\{\pm 1\}$
on each
![]() $V(1)_{\frak p}$
.
$V(1)_{\frak p}$
.
By [Reference Kobayash and Todorov26], there exists a Ricci-flat orbifold Kähler form
![]() $\omega _{\eta }$
on X such that
$\omega _{\eta }$
on X such that
By equation (4.13), we have
Since the right-hand side is independent of
![]() $\epsilon \in (0,1)$
, we get by putting
$\epsilon \in (0,1)$
, we get by putting
![]() $\epsilon \to 0$
$\epsilon \to 0$
Hence we get the following relation by regarding
![]() $\eta $
as a nowhere vanishing holomorphic
$\eta $
as a nowhere vanishing holomorphic
![]() $2$
-form on both
$2$
-form on both
![]() $\widetilde {X}$
and X
$\widetilde {X}$
and X
 $$ \begin{align*}\left.\frac{\eta\wedge\overline{\eta}}{\gamma_{\epsilon,\delta}^{2}/2!}\right|_{E_{\frak p}} = |f_{\frak p}(0)|^{2} = \frac{\eta\wedge\overline{\eta}}{\gamma_{0}^{2}}({\frak p}). \end{align*} $$
$$ \begin{align*}\left.\frac{\eta\wedge\overline{\eta}}{\gamma_{\epsilon,\delta}^{2}/2!}\right|_{E_{\frak p}} = |f_{\frak p}(0)|^{2} = \frac{\eta\wedge\overline{\eta}}{\gamma_{0}^{2}}({\frak p}). \end{align*} $$
5 Behaviour of some geometric quantities under the degeneration
In this section, we study the behaviour of the second Chern form, the Bott-Chern term, and the analytic torsion of the fixed curves when
![]() $\gamma _{\epsilon ,\delta }$
converges to the orbifold metric
$\gamma _{\epsilon ,\delta }$
converges to the orbifold metric
![]() $\gamma _{0}$
.
$\gamma _{0}$
.
5.1 Behaviour of the second Chern form as
 $\epsilon \to 0$
$\epsilon \to 0$
Proposition 5.1. For any
![]() $\delta \in (0,1]$
, one has
$\delta \in (0,1]$
, one has
 $$ \begin{align*}\lim_{\epsilon\to0}\pi_{*}c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) = c_{2}(X,\gamma_{0})+\frac{3}{2}\sum_{{\frak p}\in\mathrm{Sing}(X)}\delta_{\frak p} \end{align*} $$
$$ \begin{align*}\lim_{\epsilon\to0}\pi_{*}c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) = c_{2}(X,\gamma_{0})+\frac{3}{2}\sum_{{\frak p}\in\mathrm{Sing}(X)}\delta_{\frak p} \end{align*} $$
as currents on X, where
![]() $\delta _{\frak p}$
is the Dirac
$\delta _{\frak p}$
is the Dirac
![]() $\delta $
-current supported at
$\delta $
-current supported at
![]() ${\frak p}$
. In particular,
${\frak p}$
. In particular,
Proof. Let
![]() $h\in C^{\infty }(X)$
. By the definition of the Kähler form
$h\in C^{\infty }(X)$
. By the definition of the Kähler form
![]() $\gamma _{\epsilon ,\delta }$
, we have
$\gamma _{\epsilon ,\delta }$
, we have
 $$ \begin{align} \begin{aligned} \int_{\widetilde{X}}\pi^{*}h\cdot c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) &= \int_{X\setminus\bigcup_{{\frak p}\in\mathrm{Sing}(X)}\widetilde{V}(\delta)_{\frak p}}h\cdot c_{2}(X,\gamma_{\epsilon,\delta}) + \sum_{{\frak p}\in\mathrm{Sing}(X)}h({\frak p})\int_{\widetilde{V}(\delta)_{\frak p}}c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) \\ &\quad+ \sum_{{\frak p}\in\mathrm{Sing}(X)}\int_{\widetilde{V}(\delta)_{\frak p}}\pi^{*}\{h-h({\frak p})\}\cdot c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}). \end{aligned} \end{align} $$
$$ \begin{align} \begin{aligned} \int_{\widetilde{X}}\pi^{*}h\cdot c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) &= \int_{X\setminus\bigcup_{{\frak p}\in\mathrm{Sing}(X)}\widetilde{V}(\delta)_{\frak p}}h\cdot c_{2}(X,\gamma_{\epsilon,\delta}) + \sum_{{\frak p}\in\mathrm{Sing}(X)}h({\frak p})\int_{\widetilde{V}(\delta)_{\frak p}}c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) \\ &\quad+ \sum_{{\frak p}\in\mathrm{Sing}(X)}\int_{\widetilde{V}(\delta)_{\frak p}}\pi^{*}\{h-h({\frak p})\}\cdot c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}). \end{aligned} \end{align} $$
For
![]() $a>0$
, let
$a>0$
, let
![]() $T_{a}(z):=az$
be the homothety of
$T_{a}(z):=az$
be the homothety of
![]() $\mathbf {C}^{2}$
and let
$\mathbf {C}^{2}$
and let
![]() $\widetilde {T}_{a}\colon T^{*}\mathbf {P}^{1}\to T^{*}\mathbf {P}^{1}$
be the biholomorphic map induced by
$\widetilde {T}_{a}\colon T^{*}\mathbf {P}^{1}\to T^{*}\mathbf {P}^{1}$
be the biholomorphic map induced by
![]() $T_{a}$
. Then
$T_{a}$
. Then
![]() $\widetilde {T}_{\epsilon }$
induces an isometry of Kähler manifolds
$\widetilde {T}_{\epsilon }$
induces an isometry of Kähler manifolds
Under the identification
![]() $T^{*}\mathbf {P}^{1}\setminus E\cong V(\infty )\setminus \{0\}$
, we have the following estimates
$T^{*}\mathbf {P}^{1}\setminus E\cong V(\infty )\setminus \{0\}$
, we have the following estimates
for
![]() $\|z\|\gg 1$
by equation (4.3), where
$\|z\|\gg 1$
by equation (4.3), where
![]() $C>0$
is a constant and the norm is with respect to
$C>0$
is a constant and the norm is with respect to
![]() $\gamma ^{\mathrm {EH}}$
.
$\gamma ^{\mathrm {EH}}$
.
Since there is a constant
![]() $C'>0$
with
$C'>0$
with
![]() $\left |h|_{V(\delta )_{\frak p}}(z)-h({\frak p})\right |\leq C'\|z\|/(1+\|z\|)$
on
$\left |h|_{V(\delta )_{\frak p}}(z)-h({\frak p})\right |\leq C'\|z\|/(1+\|z\|)$
on
![]() $V(\delta )_{\frak p}$
, we get
$V(\delta )_{\frak p}$
, we get
 $$ \begin{align} \begin{aligned} \,& \left| \int_{\widetilde{V}(\delta)_{\frak p}}\{\pi^{*}h-h({\frak p})\}\cdot c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) \right| = \left| \int_{\widetilde{V}(\delta)_{\frak p}} \pi^{*}\{h|_{V(\delta)_{\frak p}}-h({\frak p})\}\cdot c_{2}(T^{*}\mathbf{P}^{1},\gamma^{\mathrm{EH}}_{\epsilon}) \right| \\ &= \left| \int_{\widetilde{V}(\delta\sqrt{\epsilon}^{-1})}\widetilde{T}_{\epsilon}^{*} \pi^{*}\{h|_{V(\delta)_{\frak p}}-h({\frak p})\}\cdot c_{2}(T^{*}\mathbf{P}^{1},\gamma^{\mathrm{EH}}) \right| \\ &\leq \int_{V(\delta\sqrt{\epsilon}^{-1})}C'\frac{\sqrt{\epsilon}\|z\|}{1+\sqrt{\epsilon}\|z\|}\cdot\frac{C}{1+\|z\|^{6}}\,\frac{(\gamma^{\mathrm{EH}})^{2}}{2!} \leq C"\sqrt{\epsilon}\to0\quad(\epsilon\to0), \end{aligned} \end{align} $$
$$ \begin{align} \begin{aligned} \,& \left| \int_{\widetilde{V}(\delta)_{\frak p}}\{\pi^{*}h-h({\frak p})\}\cdot c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) \right| = \left| \int_{\widetilde{V}(\delta)_{\frak p}} \pi^{*}\{h|_{V(\delta)_{\frak p}}-h({\frak p})\}\cdot c_{2}(T^{*}\mathbf{P}^{1},\gamma^{\mathrm{EH}}_{\epsilon}) \right| \\ &= \left| \int_{\widetilde{V}(\delta\sqrt{\epsilon}^{-1})}\widetilde{T}_{\epsilon}^{*} \pi^{*}\{h|_{V(\delta)_{\frak p}}-h({\frak p})\}\cdot c_{2}(T^{*}\mathbf{P}^{1},\gamma^{\mathrm{EH}}) \right| \\ &\leq \int_{V(\delta\sqrt{\epsilon}^{-1})}C'\frac{\sqrt{\epsilon}\|z\|}{1+\sqrt{\epsilon}\|z\|}\cdot\frac{C}{1+\|z\|^{6}}\,\frac{(\gamma^{\mathrm{EH}})^{2}}{2!} \leq C"\sqrt{\epsilon}\to0\quad(\epsilon\to0), \end{aligned} \end{align} $$
where
![]() $C^{\prime \prime }>0$
is a constant. By equations (5.1) and (5.2), we get
$C^{\prime \prime }>0$
is a constant. By equations (5.1) and (5.2), we get
 $$ \begin{align} \begin{aligned} \lim_{\epsilon\to0}\int_{\widetilde{X}}\pi^{*}h\cdot c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) &= \int_{X\setminus\bigcup_{{\frak p}\in\mathrm{Sing}(X)}V(\delta)_{\frak p}}h\cdot c_{2}(X,\gamma_{0}) \\ &\quad + \sum_{{\frak p}\in\mathrm{Sing}(X)}h({\frak p})\int_{T^{*}\mathbf{P}^{1}}c_{2}(T^{*}\mathbf{P}^{1},\gamma^{\mathrm{EH}}) \\ &= \int_{X}h\cdot c_{2}(X,\gamma_{0}) + \sum_{{\frak p}\in\mathrm{Sing}(X)}h({\frak p})\int_{T^{*}\mathbf{P}^{1}}c_{2}(T^{*}\mathbf{P}^{1},\gamma^{\mathrm{EH}}), \end{aligned} \end{align} $$
$$ \begin{align} \begin{aligned} \lim_{\epsilon\to0}\int_{\widetilde{X}}\pi^{*}h\cdot c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) &= \int_{X\setminus\bigcup_{{\frak p}\in\mathrm{Sing}(X)}V(\delta)_{\frak p}}h\cdot c_{2}(X,\gamma_{0}) \\ &\quad + \sum_{{\frak p}\in\mathrm{Sing}(X)}h({\frak p})\int_{T^{*}\mathbf{P}^{1}}c_{2}(T^{*}\mathbf{P}^{1},\gamma^{\mathrm{EH}}) \\ &= \int_{X}h\cdot c_{2}(X,\gamma_{0}) + \sum_{{\frak p}\in\mathrm{Sing}(X)}h({\frak p})\int_{T^{*}\mathbf{P}^{1}}c_{2}(T^{*}\mathbf{P}^{1},\gamma^{\mathrm{EH}}), \end{aligned} \end{align} $$
where we used the vanishing of
![]() $c_{2}(X,\gamma _{0})$
on
$c_{2}(X,\gamma _{0})$
on
![]() $V(\delta )_{\frak p}$
to get the second equality. Setting
$V(\delta )_{\frak p}$
to get the second equality. Setting
![]() $h=1$
in equation (5.3) and comparing it with the formula [Reference Kobayash27, p.396 l.5], we get
$h=1$
in equation (5.3) and comparing it with the formula [Reference Kobayash27, p.396 l.5], we get
The first assertion follows from equations (5.3) and (5.4).
Since
![]() $\#\mathrm {Sing}(Y)=k$
, we get by the first assertion
$\#\mathrm {Sing}(Y)=k$
, we get by the first assertion
 $$ \begin{align*}2\int_{Y}c_{2}(Y,\gamma_{0}) = \int_{X}c_{2}(X,\gamma_{0}) = \int_{\widetilde{X}}c_{2}(\widetilde{X},\gamma_{\epsilon,\delta})-\frac{3}{2}\sum_{{\frak p}\in\mathrm{Sing}(X)}\int_{X}\delta_{\frak p} = 24-\frac{3}{2}k. \end{align*} $$
$$ \begin{align*}2\int_{Y}c_{2}(Y,\gamma_{0}) = \int_{X}c_{2}(X,\gamma_{0}) = \int_{\widetilde{X}}c_{2}(\widetilde{X},\gamma_{\epsilon,\delta})-\frac{3}{2}\sum_{{\frak p}\in\mathrm{Sing}(X)}\int_{X}\delta_{\frak p} = 24-\frac{3}{2}k. \end{align*} $$
This proves the second assertion.
5.2 Behaviour of the Bott-Chern terms as
 $\epsilon \to 0$
$\epsilon \to 0$
Proposition 5.2. For any
![]() $\delta \in (0,1]$
, one has
$\delta \in (0,1]$
, one has
 $$ \begin{align*}\begin{aligned} \,& \lim_{\epsilon\to0} \int_{\widetilde{X}} \log \left\{ \frac{\eta\wedge\overline{\eta}}{\gamma_{\epsilon,\delta}^{2}/2!}\cdot\frac{\mathrm{Vol}(\widetilde{X},\gamma_{\epsilon,\delta})}{\|\eta\|_{L^{2}}^{2}} \right\}\, c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) \\ &= \int_{X} \log\left\{\frac{\eta\wedge\overline{\eta}}{\gamma_{0}^{2}/2!}\cdot\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}}^{2}}\right\}\,c_{2}(X,\gamma_{0}) + \frac{3}{2}\sum_{{\frak p}\in\mathrm{Sing}(X)}\log\left\{|f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}}^{2}}\right\}. \end{aligned} \end{align*} $$
$$ \begin{align*}\begin{aligned} \,& \lim_{\epsilon\to0} \int_{\widetilde{X}} \log \left\{ \frac{\eta\wedge\overline{\eta}}{\gamma_{\epsilon,\delta}^{2}/2!}\cdot\frac{\mathrm{Vol}(\widetilde{X},\gamma_{\epsilon,\delta})}{\|\eta\|_{L^{2}}^{2}} \right\}\, c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) \\ &= \int_{X} \log\left\{\frac{\eta\wedge\overline{\eta}}{\gamma_{0}^{2}/2!}\cdot\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}}^{2}}\right\}\,c_{2}(X,\gamma_{0}) + \frac{3}{2}\sum_{{\frak p}\in\mathrm{Sing}(X)}\log\left\{|f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}}^{2}}\right\}. \end{aligned} \end{align*} $$
Proof. Since
![]() $\gamma _{\epsilon ,\delta }$
converges to
$\gamma _{\epsilon ,\delta }$
converges to
![]() $\gamma _{0}$
outside
$\gamma _{0}$
outside
![]() $\bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(\delta )_{\frak p}$
, and since
$\bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(\delta )_{\frak p}$
, and since
![]() $\mathrm {Vol}(\widetilde {X},\gamma _{\epsilon ,\delta })$
converges to
$\mathrm {Vol}(\widetilde {X},\gamma _{\epsilon ,\delta })$
converges to
![]() $\mathrm {Vol}(X,\gamma _{0})$
as
$\mathrm {Vol}(X,\gamma _{0})$
as
![]() $\epsilon \to 0$
, we get the convergence
$\epsilon \to 0$
, we get the convergence
 $$ \begin{align*}\begin{aligned} \,& \left( \int_{\widetilde{X}\setminus\bigcup_{{\frak p}\in\mathrm{Sing}(X)}\widetilde{V}(\delta)_{\frak p}} + \sum_{{\frak p}\in\mathrm{Sing}(X)}\int_{\widetilde{V}(\delta)_{\frak p}} \right) \log \left\{ \frac{\eta\wedge\overline{\eta}}{\gamma_{\epsilon,\delta}^{2}/2!}\cdot\frac{\mathrm{Vol}(\widetilde{X},\gamma_{\epsilon,\delta})}{\|\eta\|_{L^{2}}^{2}} \right\} c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) \\ &\to \int_{\widetilde{X}\setminus\bigcup_{{\frak p}\in\mathrm{Sing}(X)}\widetilde{V}(\delta)_{\frak p}} \log \left\{ \frac{\eta\wedge\overline{\eta}}{\gamma_{0}^{2}/2!}\cdot\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}}^{2}} \right\} c_{2}(\widetilde{X},\gamma_{0}) \\ &\quad+ \lim_{\epsilon\to0} \sum_{{\frak p}\in\mathrm{Sing}(X)}\int_{\widetilde{V}(\delta)_{\frak p}} \left\{ \log\pi^{*}|f_{\frak p}(z)|^{2}c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) + \log\frac{\mathrm{Vol}(\widetilde{X},\gamma_{\epsilon,\delta})}{\|\eta\|_{L^{2}}^{2}}c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) \right\} \\ &= \int_{X\setminus\bigcup_{{\frak p}\in\mathrm{Sing}(X)}V(\delta)_{\frak p}} \log \left\{ \frac{\eta\wedge\overline{\eta}}{\gamma_{0}^{2}/2!}\cdot\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}}^{2}} \right\} c_{2}(X,\gamma_{0}) \\ &\quad+ \frac{3}{2}\sum_{{\frak p}\in\mathrm{Sing}(X)}\log \left(|f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}}^{2}}\right) \end{aligned} \end{align*} $$
$$ \begin{align*}\begin{aligned} \,& \left( \int_{\widetilde{X}\setminus\bigcup_{{\frak p}\in\mathrm{Sing}(X)}\widetilde{V}(\delta)_{\frak p}} + \sum_{{\frak p}\in\mathrm{Sing}(X)}\int_{\widetilde{V}(\delta)_{\frak p}} \right) \log \left\{ \frac{\eta\wedge\overline{\eta}}{\gamma_{\epsilon,\delta}^{2}/2!}\cdot\frac{\mathrm{Vol}(\widetilde{X},\gamma_{\epsilon,\delta})}{\|\eta\|_{L^{2}}^{2}} \right\} c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) \\ &\to \int_{\widetilde{X}\setminus\bigcup_{{\frak p}\in\mathrm{Sing}(X)}\widetilde{V}(\delta)_{\frak p}} \log \left\{ \frac{\eta\wedge\overline{\eta}}{\gamma_{0}^{2}/2!}\cdot\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}}^{2}} \right\} c_{2}(\widetilde{X},\gamma_{0}) \\ &\quad+ \lim_{\epsilon\to0} \sum_{{\frak p}\in\mathrm{Sing}(X)}\int_{\widetilde{V}(\delta)_{\frak p}} \left\{ \log\pi^{*}|f_{\frak p}(z)|^{2}c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) + \log\frac{\mathrm{Vol}(\widetilde{X},\gamma_{\epsilon,\delta})}{\|\eta\|_{L^{2}}^{2}}c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) \right\} \\ &= \int_{X\setminus\bigcup_{{\frak p}\in\mathrm{Sing}(X)}V(\delta)_{\frak p}} \log \left\{ \frac{\eta\wedge\overline{\eta}}{\gamma_{0}^{2}/2!}\cdot\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}}^{2}} \right\} c_{2}(X,\gamma_{0}) \\ &\quad+ \frac{3}{2}\sum_{{\frak p}\in\mathrm{Sing}(X)}\log \left(|f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}}^{2}}\right) \end{aligned} \end{align*} $$
as
![]() $\epsilon \to 0$
, where the last equality follows from Proposition 5.1. Since
$\epsilon \to 0$
, where the last equality follows from Proposition 5.1. Since
![]() $c_{2}(X,\gamma _{0})=0$
on
$c_{2}(X,\gamma _{0})=0$
on
![]() $\bigcup _{{\frak p}\in \mathrm {Sing}(X)}V(\delta )_{\frak p}$
, we get the result.
$\bigcup _{{\frak p}\in \mathrm {Sing}(X)}V(\delta )_{\frak p}$
, we get the result.
Corollary 5.3. For any
![]() $\delta \in (0,1]$
, one has
$\delta \in (0,1]$
, one has
 $$ \begin{align*}\begin{aligned} \lim_{\delta\to0}\lim_{\epsilon\to0}\tau(\widetilde{X},\gamma_{\epsilon,\delta}) &= \prod_{{\frak p}\in\mathrm{Sing}(X)}\left\{|f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}}^{2}}\right\}^{-\frac{1}{16}} \\ &\quad\times \exp \left( -\frac{1}{24}\int_{X} \log\left\{\frac{\eta\wedge\overline{\eta}}{\gamma_{0}^{2}/2!}\cdot\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}}^{2}}\right\}\, c_{2}(X,\gamma_{0}) \right). \end{aligned} \end{align*} $$
$$ \begin{align*}\begin{aligned} \lim_{\delta\to0}\lim_{\epsilon\to0}\tau(\widetilde{X},\gamma_{\epsilon,\delta}) &= \prod_{{\frak p}\in\mathrm{Sing}(X)}\left\{|f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}}^{2}}\right\}^{-\frac{1}{16}} \\ &\quad\times \exp \left( -\frac{1}{24}\int_{X} \log\left\{\frac{\eta\wedge\overline{\eta}}{\gamma_{0}^{2}/2!}\cdot\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}}^{2}}\right\}\, c_{2}(X,\gamma_{0}) \right). \end{aligned} \end{align*} $$
In particular, the limit
![]() $\lim _{\delta \to 0}\lim _{\epsilon \to 0}\tau (\widetilde {X},\gamma _{\epsilon ,\delta })$
is independent of the choice of
$\lim _{\delta \to 0}\lim _{\epsilon \to 0}\tau (\widetilde {X},\gamma _{\epsilon ,\delta })$
is independent of the choice of
![]() $\rho $
.
$\rho $
.
Proof. By Theorem 3.2 and Proposition 5.2, we get the desired equality. The independence of the double limit
![]() $\lim _{\delta \to 0}\lim _{\epsilon \to 0}\tau (\widetilde {X},\gamma _{\epsilon ,\delta })$
from
$\lim _{\delta \to 0}\lim _{\epsilon \to 0}\tau (\widetilde {X},\gamma _{\epsilon ,\delta })$
from
![]() $\rho $
is obvious because the right-hand side is independent of the choice of
$\rho $
is obvious because the right-hand side is independent of the choice of
![]() $\rho $
.
$\rho $
.
Define the Fubini-Study form on
![]() $E_{\frak p}$
by
$E_{\frak p}$
by
 $$ \begin{align*}\omega_{\mathrm{FS}}(E_{\frak p}):=\left.\varPi^{*}\left(\frac{i}{2\pi}\partial\bar{\partial}\log\|z\|^{2}\right)\right|_{E_{\frak p}}. \end{align*} $$
$$ \begin{align*}\omega_{\mathrm{FS}}(E_{\frak p}):=\left.\varPi^{*}\left(\frac{i}{2\pi}\partial\bar{\partial}\log\|z\|^{2}\right)\right|_{E_{\frak p}}. \end{align*} $$
Then for
![]() ${\frak p}\in \mathrm {Sing}(X)$
, we have
${\frak p}\in \mathrm {Sing}(X)$
, we have
and an isomorphism of Kähler manifolds
![]() $(E_{\frak p},\omega _{\mathrm {FS}}(E_{\frak p}))\cong (\mathbf {P}^{1},\omega _{\mathrm {FS}})$
.
$(E_{\frak p},\omega _{\mathrm {FS}}(E_{\frak p}))\cong (\mathbf {P}^{1},\omega _{\mathrm {FS}})$
.
Proposition 5.4. For any
![]() $\delta \in (0,1]$
, one has
$\delta \in (0,1]$
, one has
 $$ \begin{align*}\lim_{\epsilon\to0}A_{M}(\widetilde{X},\theta,\gamma_{\epsilon,\delta}) = \prod_{{\frak p}\in\mathrm{Sing}(X)}\left\{|f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}}^{2}}\right\}^{\frac{1}{4}}. \end{align*} $$
$$ \begin{align*}\lim_{\epsilon\to0}A_{M}(\widetilde{X},\theta,\gamma_{\epsilon,\delta}) = \prod_{{\frak p}\in\mathrm{Sing}(X)}\left\{|f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}}^{2}}\right\}^{\frac{1}{4}}. \end{align*} $$
Proof. Since
![]() $\gamma _{\epsilon ,\delta }|_{E_{\frak p}}=\epsilon \,\omega _{\mathrm {FS}}(E_{\frak p})$
, and since
$\gamma _{\epsilon ,\delta }|_{E_{\frak p}}=\epsilon \,\omega _{\mathrm {FS}}(E_{\frak p})$
, and since
![]() $\omega _{\mathrm {FS}}(E_{\frak p})$
is Kähler-Einstein, we get
$\omega _{\mathrm {FS}}(E_{\frak p})$
is Kähler-Einstein, we get
Since
![]() $(\eta \wedge \overline {\eta })/(\gamma _{\epsilon ,\delta }^{2}/2!)|_{E_{\frak p}}=|f_{\frak p}(0)|^{2}$
by equation (4.13), we get
$(\eta \wedge \overline {\eta })/(\gamma _{\epsilon ,\delta }^{2}/2!)|_{E_{\frak p}}=|f_{\frak p}(0)|^{2}$
by equation (4.13), we get
 $$ \begin{align*}\begin{aligned} \,& A_{M}(\widetilde{X},\theta,\gamma_{\epsilon,\delta}) = \exp \left[ \frac{1}{8}\int_{\widetilde{X}^{\theta}} \left.\log\left\{\frac{\eta\wedge\overline{\eta}}{\gamma_{\epsilon,\delta}^{2}/2!} \cdot \frac{\mathrm{Vol}(\widetilde{X},\gamma_{\epsilon,\delta})}{\|\eta\|_{L^{2}}^{2}}\right\}\right|_{\widetilde{X}^{\theta}}\, c_{1}(\widetilde{X}^{\theta},\gamma_{\epsilon,\delta}|_{\widetilde{X}^{\theta}}) \right] \\ &= \exp \left[ \frac{1}{4}\sum_{{\frak p}\in\mathrm{Sing}(X)}\int_{E_{\frak p}} \left.\log\left\{ |f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(\widetilde{X},\gamma_{\epsilon,\delta})}{\|\eta\|_{L^{2}}^{2}}\right\}\right|_{E_{\frak p}}\, \omega_{\mathrm{FS}}(E_{\frak p}) \right] \\ &= \exp \left[ \frac{1}{4}\sum_{{\frak p}\in\mathrm{Sing}(X)} \log\left\{ |f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(\widetilde{X},\gamma_{\epsilon,\delta})}{\|\eta\|_{L^{2}}^{2}}\right\} \right] \to \prod_{{\frak p}\in\mathrm{Sing}(X)}\left\{|f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}}^{2}}\right\}^{\frac{1}{4}} \end{aligned} \end{align*} $$
$$ \begin{align*}\begin{aligned} \,& A_{M}(\widetilde{X},\theta,\gamma_{\epsilon,\delta}) = \exp \left[ \frac{1}{8}\int_{\widetilde{X}^{\theta}} \left.\log\left\{\frac{\eta\wedge\overline{\eta}}{\gamma_{\epsilon,\delta}^{2}/2!} \cdot \frac{\mathrm{Vol}(\widetilde{X},\gamma_{\epsilon,\delta})}{\|\eta\|_{L^{2}}^{2}}\right\}\right|_{\widetilde{X}^{\theta}}\, c_{1}(\widetilde{X}^{\theta},\gamma_{\epsilon,\delta}|_{\widetilde{X}^{\theta}}) \right] \\ &= \exp \left[ \frac{1}{4}\sum_{{\frak p}\in\mathrm{Sing}(X)}\int_{E_{\frak p}} \left.\log\left\{ |f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(\widetilde{X},\gamma_{\epsilon,\delta})}{\|\eta\|_{L^{2}}^{2}}\right\}\right|_{E_{\frak p}}\, \omega_{\mathrm{FS}}(E_{\frak p}) \right] \\ &= \exp \left[ \frac{1}{4}\sum_{{\frak p}\in\mathrm{Sing}(X)} \log\left\{ |f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(\widetilde{X},\gamma_{\epsilon,\delta})}{\|\eta\|_{L^{2}}^{2}}\right\} \right] \to \prod_{{\frak p}\in\mathrm{Sing}(X)}\left\{|f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}}^{2}}\right\}^{\frac{1}{4}} \end{aligned} \end{align*} $$
as
![]() $\epsilon \to 0$
, where we used equation (4.14) to get the last limit. This completes the proof.
$\epsilon \to 0$
, where we used equation (4.14) to get the last limit. This completes the proof.
5.3 Behaviour of the analytic torsion of the exceptional divisors
Proposition 5.5. For any
![]() $\delta \in (0,1]$
and
$\delta \in (0,1]$
and
![]() ${\frak p}\in \mathrm {Sing}(X)$
, the following equality holds for all
${\frak p}\in \mathrm {Sing}(X)$
, the following equality holds for all
![]() $\epsilon \in (0,\delta ^{2}\epsilon (\rho )]$
:
$\epsilon \in (0,\delta ^{2}\epsilon (\rho )]$
:
 $$ \begin{align*}\frac{\mathrm{Vol}(E_{\frak p},\gamma_{\epsilon,\delta}|_{E_{\frak p}})\tau(E_{\frak p},\gamma_{\epsilon,\delta}|_{E_{\frak p}})} {\mathrm{Vol}(\mathbf{P}^{1},\omega_{\mathrm{FS}})\tau(\mathbf{P}^{1},\omega_{\mathrm{FS}})} = \epsilon^{1/3}. \end{align*} $$
$$ \begin{align*}\frac{\mathrm{Vol}(E_{\frak p},\gamma_{\epsilon,\delta}|_{E_{\frak p}})\tau(E_{\frak p},\gamma_{\epsilon,\delta}|_{E_{\frak p}})} {\mathrm{Vol}(\mathbf{P}^{1},\omega_{\mathrm{FS}})\tau(\mathbf{P}^{1},\omega_{\mathrm{FS}})} = \epsilon^{1/3}. \end{align*} $$
Proof. We recall a formula of Bost [Reference Bost9, Prop. 4.4]. Let
![]() $(Z,g)$
be a compact Kähler manifold of dimension d, and let
$(Z,g)$
be a compact Kähler manifold of dimension d, and let
![]() $\lambda>0$
be a constant. By [Reference Bost9, (4.2.4)], we get
$\lambda>0$
be a constant. By [Reference Bost9, (4.2.4)], we get
 $$ \begin{align} \log\left(\frac{\tau(Z,\lambda g)}{\tau(Z,g)}\right) = \left( -\sum_{i=0}^{d}(-1)^{i}(d-i)\,h^{0,i}(Z)+\int_{Z}\mathrm{Td}'(TZ) \right) \log\lambda, \end{align} $$
$$ \begin{align} \log\left(\frac{\tau(Z,\lambda g)}{\tau(Z,g)}\right) = \left( -\sum_{i=0}^{d}(-1)^{i}(d-i)\,h^{0,i}(Z)+\int_{Z}\mathrm{Td}'(TZ) \right) \log\lambda, \end{align} $$
where the characteristic class
![]() $\mathrm {Td}'(E)$
is defined as follows (compare [Reference Bost9, Prop. 4.4]). If
$\mathrm {Td}'(E)$
is defined as follows (compare [Reference Bost9, Prop. 4.4]). If
![]() $\xi _{i} (i=1,\ldots ,r=\mathrm {rk}(E))$
are the Chern roots of a vector bundle E, then
$\xi _{i} (i=1,\ldots ,r=\mathrm {rk}(E))$
are the Chern roots of a vector bundle E, then
 $$ \begin{align*}\mathrm{Td}'(E) := \mathrm{Td}(E)\cdot\sum_{i=1}^{r}\left(\frac{1}{\xi_{i}}-\frac{e^{-\xi_{i}}}{1-e^{-\xi_{i}}}\right). \end{align*} $$
$$ \begin{align*}\mathrm{Td}'(E) := \mathrm{Td}(E)\cdot\sum_{i=1}^{r}\left(\frac{1}{\xi_{i}}-\frac{e^{-\xi_{i}}}{1-e^{-\xi_{i}}}\right). \end{align*} $$
Since
 $$ \begin{align*}\mathrm{Td}'(x) = \frac{x}{1-e^{-x}}\left(\frac{1}{x}-\frac{e^{-x}}{1-e^{-x}}\right) = \frac{1}{2}+\frac{1}{6}x+O(x^{2}) \end{align*} $$
$$ \begin{align*}\mathrm{Td}'(x) = \frac{x}{1-e^{-x}}\left(\frac{1}{x}-\frac{e^{-x}}{1-e^{-x}}\right) = \frac{1}{2}+\frac{1}{6}x+O(x^{2}) \end{align*} $$
and hence
![]() $\int _{\mathbf {P}^{1}}\mathrm {Td}'(T\mathbf {P}^{1})=1/3$
, we get by equation (5.5) applied to
$\int _{\mathbf {P}^{1}}\mathrm {Td}'(T\mathbf {P}^{1})=1/3$
, we get by equation (5.5) applied to
![]() $(Z,g)=(\mathbf {P}^{1},\omega _{\mathrm {FS}})$
$(Z,g)=(\mathbf {P}^{1},\omega _{\mathrm {FS}})$
Since
6 Spectrum and heat kernels under the degeneration
In this section, we prove a uniform lower bound of the kth eigenvalue of the Laplacian and also a certain uniform exponential decay of the heat kernel for the degenerating family of metrics
![]() $\gamma _{\epsilon ,\delta }$
.
$\gamma _{\epsilon ,\delta }$
.
6.1 Uniformity of Sobolev inequality
In order to study the limit of the analytic torsions
![]() $\tau (\widetilde {X}, \gamma _{\epsilon ,\delta })$
and
$\tau (\widetilde {X}, \gamma _{\epsilon ,\delta })$
and
![]() $\tau _{{\mathbf Z}_{2}}(\widetilde {X}, \gamma _{\epsilon ,\delta })(\theta )$
in the next section, we need to establish a uniform Sobolev inequality. First, we consider our model space
$\tau _{{\mathbf Z}_{2}}(\widetilde {X}, \gamma _{\epsilon ,\delta })(\theta )$
in the next section, we need to establish a uniform Sobolev inequality. First, we consider our model space
![]() $(T^{*}\mathbf {P}^{1}, \gamma ^{\mathrm {EH}}_{\epsilon })$
, the Eguchi-Hanson instanton. Here
$(T^{*}\mathbf {P}^{1}, \gamma ^{\mathrm {EH}}_{\epsilon })$
, the Eguchi-Hanson instanton. Here
![]() $\gamma ^{\mathrm {EH}}_{\epsilon }$
is the Ricci-flat Kähler metric constructed in Section 5.1 on
$\gamma ^{\mathrm {EH}}_{\epsilon }$
is the Ricci-flat Kähler metric constructed in Section 5.1 on
![]() $\widetilde {V}(\infty )=T^{*}\mathbf {P}^{1}$
. Note that for
$\widetilde {V}(\infty )=T^{*}\mathbf {P}^{1}$
. Note that for
![]() $0<\epsilon \leq 1$
, under the identification
$0<\epsilon \leq 1$
, under the identification
![]() $\Phi :\ ({\mathbf R}^4\!-\! B(\rho ))/\{\pm 1\} \simeq \widetilde {V}(\infty )\! -\! K$
outside a compact neighbourhood
$\Phi :\ ({\mathbf R}^4\!-\! B(\rho ))/\{\pm 1\} \simeq \widetilde {V}(\infty )\! -\! K$
outside a compact neighbourhood
![]() $K = \widetilde {V}(\rho ) \subset \widetilde {V}(\infty )$
of the zero section of
$K = \widetilde {V}(\rho ) \subset \widetilde {V}(\infty )$
of the zero section of
![]() $T^{*}\mathbf {P}^{1}$
induced by the identification
$T^{*}\mathbf {P}^{1}$
induced by the identification
![]() $({\mathbf C}^2\!-\! B(\rho ))/\{\pm 1\} = V(\infty ) \!-\! V(\rho ) = \widetilde {V}(\infty ) \!-\! \widetilde {V}(\rho )$
, one has
$({\mathbf C}^2\!-\! B(\rho ))/\{\pm 1\} = V(\infty ) \!-\! V(\rho ) = \widetilde {V}(\infty ) \!-\! \widetilde {V}(\rho )$
, one has
uniformly in
![]() $\epsilon $
by equation (4.4).
$\epsilon $
by equation (4.4).
Lemma 6.1. There is a constant C such that for all
![]() $0<\epsilon \leq 1$
, the following hold:
$0<\epsilon \leq 1$
, the following hold:
-
(1) For all
 $f\in C_0^{\infty }(\widetilde {V}(\infty ))$
,
$f\in C_0^{\infty }(\widetilde {V}(\infty ))$
,  $$ \begin{align*}\| f \|_{L^4(\widetilde{V}(\infty),\gamma^{\mathrm{EH}}_{\epsilon})} \leq C \| df \|_{L^2(\widetilde{V}(\infty),\gamma^{\mathrm{EH}}_{\epsilon})}.\end{align*} $$
$$ \begin{align*}\| f \|_{L^4(\widetilde{V}(\infty),\gamma^{\mathrm{EH}}_{\epsilon})} \leq C \| df \|_{L^2(\widetilde{V}(\infty),\gamma^{\mathrm{EH}}_{\epsilon})}.\end{align*} $$
-
(2) Similarly, for all
 $\alpha \in A^{0,2}_0(\widetilde {V}(\infty ))$
,
$\alpha \in A^{0,2}_0(\widetilde {V}(\infty ))$
,  $$ \begin{align*}\| \alpha \|_{L^4(\widetilde{V}(\infty),\gamma^{\mathrm{EH}}_{\epsilon})} \leq C \| d\alpha \|_{L^2(\widetilde{V}(\infty),\gamma^{\mathrm{EH}}_{\epsilon})}.\end{align*} $$
$$ \begin{align*}\| \alpha \|_{L^4(\widetilde{V}(\infty),\gamma^{\mathrm{EH}}_{\epsilon})} \leq C \| d\alpha \|_{L^2(\widetilde{V}(\infty),\gamma^{\mathrm{EH}}_{\epsilon})}.\end{align*} $$
-
(3) For all
 $\alpha \in A^{0,1}_0(\widetilde {V}(\infty ))$
,
$\alpha \in A^{0,1}_0(\widetilde {V}(\infty ))$
,  $$ \begin{align*}\| \alpha \|^2_{L^4(\widetilde{V}(\infty),\gamma^{\mathrm{EH}}_{\epsilon})} \leq C^2 \left(\| \overline{\partial}\alpha \|^2_{L^2(\widetilde{V}(\infty),\gamma^{\mathrm{EH}}_{\epsilon})} + \| \overline{\partial}^*\alpha \|^2_{L^2(\widetilde{V}(\infty),\gamma^{\mathrm{EH}}_{\epsilon})}\right). \end{align*} $$
$$ \begin{align*}\| \alpha \|^2_{L^4(\widetilde{V}(\infty),\gamma^{\mathrm{EH}}_{\epsilon})} \leq C^2 \left(\| \overline{\partial}\alpha \|^2_{L^2(\widetilde{V}(\infty),\gamma^{\mathrm{EH}}_{\epsilon})} + \| \overline{\partial}^*\alpha \|^2_{L^2(\widetilde{V}(\infty),\gamma^{\mathrm{EH}}_{\epsilon})}\right). \end{align*} $$
Here all norms are defined with respect to the metric
![]() $\gamma ^{\mathrm {EH}}_{\epsilon }$
.
$\gamma ^{\mathrm {EH}}_{\epsilon }$
.
Proof. Since
![]() $(\widetilde {V}(\infty ),\gamma ^{\mathrm {EH}}_{\epsilon })\cong (\widetilde {V}(\infty ),\epsilon \gamma ^{\mathrm {EH}})$
by equation (4.1), and since the inequalities (1), (2), (3) above are invariant under the scaling of metrics
$(\widetilde {V}(\infty ),\gamma ^{\mathrm {EH}}_{\epsilon })\cong (\widetilde {V}(\infty ),\epsilon \gamma ^{\mathrm {EH}})$
by equation (4.1), and since the inequalities (1), (2), (3) above are invariant under the scaling of metrics
![]() $\gamma ^{\mathrm {EH}}\mapsto \epsilon \gamma ^{\mathrm {EH}}$
, it suffices to prove (1), (2), (3) for
$\gamma ^{\mathrm {EH}}\mapsto \epsilon \gamma ^{\mathrm {EH}}$
, it suffices to prove (1), (2), (3) for
![]() $\gamma ^{\mathrm {EH}}$
. In the rest of the proof, all norms are defined with respect to
$\gamma ^{\mathrm {EH}}$
. In the rest of the proof, all norms are defined with respect to
![]() $\gamma ^{\mathrm {EH}}$
. Identifying a function in
$\gamma ^{\mathrm {EH}}$
. Identifying a function in
![]() $C_0^{\infty }(\widetilde {V}(\infty )\! -\! K)$
with the corresponding
$C_0^{\infty }(\widetilde {V}(\infty )\! -\! K)$
with the corresponding
![]() $\pm 1$
-invariant function on
$\pm 1$
-invariant function on
![]() $\mathbf R^4$
with compact support via
$\mathbf R^4$
with compact support via
![]() $\Phi $
, we deduce from the Sobolev inequality for
$\Phi $
, we deduce from the Sobolev inequality for
![]() $\mathbf R^4$
that
$\mathbf R^4$
that
where C is the Sobolev constant for
![]() $\mathbf R^4$
. By an argument using partition of unity, there is a constant
$\mathbf R^4$
. By an argument using partition of unity, there is a constant
![]() $C_K> 0$
such that
$C_K> 0$
such that
Assume that there is no constant
![]() $D> 0$
such that
$D> 0$
such that
Then for any
![]() $n \in \mathbf N$
, there is a function
$n \in \mathbf N$
, there is a function
![]() $f_n \in C_0^{\infty }(\widetilde {V}(\infty ))$
such that
$f_n \in C_0^{\infty }(\widetilde {V}(\infty ))$
such that
Therefore, we have
Passing to a subsequence if necessary, it follows that the sequence
![]() $f_n$
has a weak limit
$f_n$
has a weak limit
![]() $f_{\infty } \in L^4(\widetilde {V}(\infty ))$
with
$f_{\infty } \in L^4(\widetilde {V}(\infty ))$
with
![]() $df_{\infty }= 0$
as currents on
$df_{\infty }= 0$
as currents on
![]() $\widetilde {V}(\infty )$
. This implies that in
$\widetilde {V}(\infty )$
. This implies that in
![]() $L^4(\widetilde {V}(\infty ))$
,
$L^4(\widetilde {V}(\infty ))$
,
![]() $f_{\infty } = 0$
. On the other hand, let
$f_{\infty } = 0$
. On the other hand, let
![]() $K'$
be a sufficiently big compact subset of
$K'$
be a sufficiently big compact subset of
![]() $\widetilde {V}(\infty )$
, whose open subset contains K. Now, for any compact subset
$\widetilde {V}(\infty )$
, whose open subset contains K. Now, for any compact subset
![]() $K' \subset \widetilde {V}(\infty )$
, there is a constant
$K' \subset \widetilde {V}(\infty )$
, there is a constant
![]() $C_{K'}> 0$
such that
$C_{K'}> 0$
such that
Hence, by the Rellich lemma, we may assume (by again passing to a subsequence if necessary) that
![]() $f_n$
converges to
$f_n$
converges to
![]() $f_{\infty }$
strongly in
$f_{\infty }$
strongly in
![]() $L^2(K')$
. Since
$L^2(K')$
. Since
![]() $K \subset K'$
and hence the convergence
$K \subset K'$
and hence the convergence
![]() $f_n \rightarrow f_{\infty }$
in
$f_n \rightarrow f_{\infty }$
in
![]() $L^2(K)$
is strong, we see that
$L^2(K)$
is strong, we see that
![]() $ \|f_{\infty }\|_{L^2(K)} = \lim _{n \rightarrow \infty } \|f_n\|_{L^2(K)} = 1$
. This is a contradiction. Hence there exists a constant D such that
$ \|f_{\infty }\|_{L^2(K)} = \lim _{n \rightarrow \infty } \|f_n\|_{L^2(K)} = 1$
. This is a contradiction. Hence there exists a constant D such that
![]() $ \|f\|_{L^2(K)}\leq D\|df\|_{L^2(\widetilde {V}(\infty ))}$
. By setting
$ \|f\|_{L^2(K)}\leq D\|df\|_{L^2(\widetilde {V}(\infty ))}$
. By setting
![]() $C = CK(1 + D$
), we have
$C = CK(1 + D$
), we have
This proves (1).
(2) is an immediate consequence of (1), and the isomorphism
![]() $C_0^{\infty }(\widetilde {V}(\infty ))\ni f\mapsto f\overline {\eta }\in A^{0,2}_{0}(\widetilde {V}(\infty ))$
, which commutes with the operations involved. To see (3), let
$C_0^{\infty }(\widetilde {V}(\infty ))\ni f\mapsto f\overline {\eta }\in A^{0,2}_{0}(\widetilde {V}(\infty ))$
, which commutes with the operations involved. To see (3), let
![]() $\alpha \in A^{0,1}_0(\widetilde {V}(\infty ))$
. Then by (1),
$\alpha \in A^{0,1}_0(\widetilde {V}(\infty ))$
. Then by (1),
 $$ \begin{align*}\| \alpha \|^2_{L^4(\widetilde{V}(\infty))} = \left( \int_{\widetilde{V}(\infty)} |\alpha|^4 dx\right)^{1/2} \leq C^2 \int_{\widetilde{V}(\infty)} |\, d|\alpha|\, |^2dx. \end{align*} $$
$$ \begin{align*}\| \alpha \|^2_{L^4(\widetilde{V}(\infty))} = \left( \int_{\widetilde{V}(\infty)} |\alpha|^4 dx\right)^{1/2} \leq C^2 \int_{\widetilde{V}(\infty)} |\, d|\alpha|\, |^2dx. \end{align*} $$
Using Kato’s inequality, we have
Now the Bochner formula [Reference Ma and Marinescu34, (1.4.63)] gives
![]() $(\overline {\partial }\overline {\partial }^* + \overline {\partial }^*\overline {\partial })\alpha = \nabla ^*\nabla \alpha $
since
$(\overline {\partial }\overline {\partial }^* + \overline {\partial }^*\overline {\partial })\alpha = \nabla ^*\nabla \alpha $
since
![]() $(\widetilde {V}(\infty ),\gamma ^{\mathrm {EH}}_{\epsilon })$
is Ricci flat. Our result follows.
$(\widetilde {V}(\infty ),\gamma ^{\mathrm {EH}}_{\epsilon })$
is Ricci flat. Our result follows.
Lemma 6.2. There is a constant C such that for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $\epsilon \delta ^{-2}\leq \epsilon (\rho )$
, and all
$\epsilon \delta ^{-2}\leq \epsilon (\rho )$
, and all
![]() $\alpha \in A^{0,q}_0(\widetilde {V}(\infty ))$
,
$\alpha \in A^{0,q}_0(\widetilde {V}(\infty ))$
,
![]() $0\leq q\leq 2$
,
$0\leq q\leq 2$
,
where the norms and
![]() $\bar {\partial }^{*}$
are defined with respect to the metric
$\bar {\partial }^{*}$
are defined with respect to the metric
![]() $\kappa _{\epsilon ,\delta }$
.
$\kappa _{\epsilon ,\delta }$
.
Proof. By Lemmas 4.1 and 4.3, there exist constants
![]() $C_{1},C_{2}>0$
such that
$C_{1},C_{2}>0$
such that
for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $\epsilon \delta ^{-2}\leq \epsilon (\rho )$
. Here
$\epsilon \delta ^{-2}\leq \epsilon (\rho )$
. Here
![]() $\epsilon (\rho )$
is defined in Section 4.2.1, in the discussion following equation (4.2). Hence there is a constant
$\epsilon (\rho )$
is defined in Section 4.2.1, in the discussion following equation (4.2). Hence there is a constant
![]() $C_{3}>0$
such that
$C_{3}>0$
such that
for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $\epsilon \delta ^{-2}\leq \epsilon (\rho )$
and
$\epsilon \delta ^{-2}\leq \epsilon (\rho )$
and
![]() $\alpha \in A^{0,q}_{0}(\widetilde {V}(\infty ))$
.
$\alpha \in A^{0,q}_{0}(\widetilde {V}(\infty ))$
.
Let
![]() $\Lambda _{\epsilon .\delta }$
(respectively,
$\Lambda _{\epsilon .\delta }$
(respectively,
![]() $\Lambda _{\epsilon }$
) be the Lefschetz operator defined as the adjoint of the multiplication by
$\Lambda _{\epsilon }$
) be the Lefschetz operator defined as the adjoint of the multiplication by
![]() $\kappa _{\epsilon ,\delta }$
(respectively,
$\kappa _{\epsilon ,\delta }$
(respectively,
![]() $\gamma ^{\mathrm {EH}}_{\epsilon }$
). Since
$\gamma ^{\mathrm {EH}}_{\epsilon }$
). Since
![]() $\overline {\partial }^{*}=\pm i\Lambda _{\epsilon ,\delta }\partial $
for
$\overline {\partial }^{*}=\pm i\Lambda _{\epsilon ,\delta }\partial $
for
![]() $(0,q)$
-forms by the Kähler identity, there exists by equation (6.1) a constant
$(0,q)$
-forms by the Kähler identity, there exists by equation (6.1) a constant
![]() $C_{4}>0$
such that
$C_{4}>0$
such that
By Lemma 6.1 (3) and equations (6.2), (6.3) and (6.4), we get the result.
For the minimal resolution
![]() $\widetilde {X}$
and the family of Kähler metrics
$\widetilde {X}$
and the family of Kähler metrics
![]() $\gamma _{\epsilon ,\delta }$
constructed in Section 5.2 using the Eguchi-Hanson instanton, we have
$\gamma _{\epsilon ,\delta }$
constructed in Section 5.2 using the Eguchi-Hanson instanton, we have
Proposition 6.3. There is a constant C such that for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $\epsilon \delta ^{-2}\leq \epsilon (\rho )$
, and all
$\epsilon \delta ^{-2}\leq \epsilon (\rho )$
, and all
![]() $\alpha \in A^{0,q}(\widetilde {X})$
,
$\alpha \in A^{0,q}(\widetilde {X})$
,
![]() $0\leq q\leq 2$
,
$0\leq q\leq 2$
,
where the norms are defined with respect to the metric
![]() $\gamma _{\epsilon ,\delta }$
.
$\gamma _{\epsilon ,\delta }$
.
Proof. Since
![]() $\gamma _{\epsilon ,\delta }=\kappa _{\epsilon ,\delta }$
on
$\gamma _{\epsilon ,\delta }=\kappa _{\epsilon ,\delta }$
on
![]() $\bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(\delta )_{\frak p}$
, the result follows from Lemma 6.2 and an easy partition of unity argument.
$\bigcup _{{\frak p}\in \mathrm {Sing}(X)}\widetilde {V}(\delta )_{\frak p}$
, the result follows from Lemma 6.2 and an easy partition of unity argument.
6.2 A uniform lower bound of spectrum
Let
![]() $\square _{\epsilon ,\delta }^{q}=(\bar {\partial }+\bar {\partial }^{*})^{2}$
(respectively,
$\square _{\epsilon ,\delta }^{q}=(\bar {\partial }+\bar {\partial }^{*})^{2}$
(respectively,
![]() $\square _{0}^{q}$
) be the Hodge-Kodaira Laplacian of
$\square _{0}^{q}$
) be the Hodge-Kodaira Laplacian of
![]() $(\widetilde {X},\gamma _{\epsilon ,\delta })$
(respectively,
$(\widetilde {X},\gamma _{\epsilon ,\delta })$
(respectively,
![]() $(X,\gamma _{0})$
) acting on
$(X,\gamma _{0})$
) acting on
![]() $(0,q)$
-forms. Let
$(0,q)$
-forms. Let
![]() $\lambda _{\epsilon ,\delta }^{q}(k)$
(respectively,
$\lambda _{\epsilon ,\delta }^{q}(k)$
(respectively,
![]() $\lambda _{0}^{q}(k)$
) be the kth nonzero eigenvalue of the Laplacian
$\lambda _{0}^{q}(k)$
) be the kth nonzero eigenvalue of the Laplacian
![]() $\square _{\epsilon ,\delta }^{q}$
(respectively,
$\square _{\epsilon ,\delta }^{q}$
(respectively,
![]() $\square _{0}^{q}$
). Then the nonzero eigenvalues of
$\square _{0}^{q}$
). Then the nonzero eigenvalues of
![]() $\square _{\epsilon ,\delta }^{q}$
are given by
$\square _{\epsilon ,\delta }^{q}$
are given by
and the set of corresponding eigenforms
![]() $\{\varphi _{k,\epsilon ,\delta }^{q}\}_{k\in \mathbf {N}}$
. We set
$\{\varphi _{k,\epsilon ,\delta }^{q}\}_{k\in \mathbf {N}}$
. We set
![]() $\lambda _{\epsilon ,\delta }^{q}(0)=0$
and list the corresponding eigenforms
$\lambda _{\epsilon ,\delta }^{q}(0)=0$
and list the corresponding eigenforms
![]() $\varphi _{0,\epsilon ,\delta }^{q}$
(here we abuse the notation as there would be
$\varphi _{0,\epsilon ,\delta }^{q}$
(here we abuse the notation as there would be
![]() $\dim H^0(\tilde X, \Omega ^q_{\tilde X})$
many of them) so that
$\dim H^0(\tilde X, \Omega ^q_{\tilde X})$
many of them) so that
![]() $\{\varphi _{k,\epsilon ,\delta }^{q}\}_{k=0}^{\infty }$
forms a complete orthonormal basis of
$\{\varphi _{k,\epsilon ,\delta }^{q}\}_{k=0}^{\infty }$
forms a complete orthonormal basis of
![]() $L^{0,q}_{\epsilon ,\delta }(\widetilde {X})$
, the
$L^{0,q}_{\epsilon ,\delta }(\widetilde {X})$
, the
![]() $L^{2}$
-completion of
$L^{2}$
-completion of
![]() $A^{0,q}(\widetilde {X})$
with respect to the norm associated to
$A^{0,q}(\widetilde {X})$
with respect to the norm associated to
![]() $\gamma _{\epsilon ,\delta }$
. Since
$\gamma _{\epsilon ,\delta }$
. Since
 $$ \begin{align*}K_{\epsilon,\delta}^{q}(t,x,y)=\sum_{k=0}^{\infty}e^{-t\lambda_{\epsilon,\delta}^{q}(k)}\varphi_{k,\epsilon,\delta}^{q}(x)\otimes\varphi_{k,\epsilon,\delta}^{q}(y)^{*}, \end{align*} $$
$$ \begin{align*}K_{\epsilon,\delta}^{q}(t,x,y)=\sum_{k=0}^{\infty}e^{-t\lambda_{\epsilon,\delta}^{q}(k)}\varphi_{k,\epsilon,\delta}^{q}(x)\otimes\varphi_{k,\epsilon,\delta}^{q}(y)^{*}, \end{align*} $$
we get
 $$ \begin{align} \begin{aligned} \left| K_{\epsilon,\delta}^{q}(t,x,y) \right| &\leq \sum_{k=0}^{\infty}e^{-t\lambda_{\epsilon,\delta}^{q}(k)}|\varphi_{k,\epsilon,\delta}^{q}(x)|\cdot|\varphi_{k,\epsilon,\delta}^{q}(y)| \\ &\leq \{\sum_{k=0}^{\infty}e^{-t\lambda_{\epsilon,\delta}^{q}(k)}|\varphi_{k,\epsilon,\delta}^{q}(x)|^{2}\}^{1/2} \{\sum_{k=0}^{\infty}e^{-t\lambda_{\epsilon,\delta}^{q}(k)}|\varphi_{k,\epsilon,\delta}^{q}(y)|^{2}\}^{1/2} \\ &= \sqrt{\mathrm{tr}\,K_{\epsilon,\delta}^{q}(t,x,x)}\sqrt{\mathrm{tr}\,K_{\epsilon,\delta}^{q}(t,y,y)}. \end{aligned} \end{align} $$
$$ \begin{align} \begin{aligned} \left| K_{\epsilon,\delta}^{q}(t,x,y) \right| &\leq \sum_{k=0}^{\infty}e^{-t\lambda_{\epsilon,\delta}^{q}(k)}|\varphi_{k,\epsilon,\delta}^{q}(x)|\cdot|\varphi_{k,\epsilon,\delta}^{q}(y)| \\ &\leq \{\sum_{k=0}^{\infty}e^{-t\lambda_{\epsilon,\delta}^{q}(k)}|\varphi_{k,\epsilon,\delta}^{q}(x)|^{2}\}^{1/2} \{\sum_{k=0}^{\infty}e^{-t\lambda_{\epsilon,\delta}^{q}(k)}|\varphi_{k,\epsilon,\delta}^{q}(y)|^{2}\}^{1/2} \\ &= \sqrt{\mathrm{tr}\,K_{\epsilon,\delta}^{q}(t,x,x)}\sqrt{\mathrm{tr}\,K_{\epsilon,\delta}^{q}(t,y,y)}. \end{aligned} \end{align} $$
Proposition 6.4. If
![]() $q=0,2$
, then there are constants
$q=0,2$
, then there are constants
![]() $A, C>0$
such that for all
$A, C>0$
such that for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $\epsilon \delta ^{-2}\leq \epsilon (\rho )$
, and
$\epsilon \delta ^{-2}\leq \epsilon (\rho )$
, and
![]() $x,y\in \widetilde {X}$
,
$x,y\in \widetilde {X}$
,
![]() $t>0$
, the following inequality holds:
$t>0$
, the following inequality holds:
Moreover, for all
![]() $(\epsilon ,\delta )\in (0,1]$
with
$(\epsilon ,\delta )\in (0,1]$
with
![]() $\epsilon \delta ^{-2}\leq \epsilon (\rho )$
, and for all
$\epsilon \delta ^{-2}\leq \epsilon (\rho )$
, and for all
![]() $t>0$
,
$t>0$
,
![]() $q\geq 0$
, the following inequality holds:
$q\geq 0$
, the following inequality holds:
Proof.
(Case 1) Let
![]() $q=0$
. By Proposition 6.3, the Sobolev constant is uniform for
$q=0$
. By Proposition 6.3, the Sobolev constant is uniform for
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $\epsilon \delta ^{-2}\leq \epsilon (\rho )$
. By [Reference Carlen, Kusuoka and Stroock10, Thms. 2.1 and 2.16], there are constants
$\epsilon \delta ^{-2}\leq \epsilon (\rho )$
. By [Reference Carlen, Kusuoka and Stroock10, Thms. 2.1 and 2.16], there are constants
![]() $A>0$
,
$A>0$
,
![]() $B\geq 0$
such that for all
$B\geq 0$
such that for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $\epsilon \delta ^{-2}\leq \epsilon (\rho )$
, and
$\epsilon \delta ^{-2}\leq \epsilon (\rho )$
, and
![]() $x,y\in \widetilde {X}$
,
$x,y\in \widetilde {X}$
,
![]() $t>0$
,
$t>0$
,
Let
![]() $q=2$
. By Lemma 4.5, the Lichnerowicz formula and [Reference Hess, Schrader and Uhlenbrock24, p.32 l.4-l.5], we have
$q=2$
. By Lemma 4.5, the Lichnerowicz formula and [Reference Hess, Schrader and Uhlenbrock24, p.32 l.4-l.5], we have
For
![]() $t\leq 1$
, we get equation (6.6) by equations (6.8) and (6.9). For
$t\leq 1$
, we get equation (6.6) by equations (6.8) and (6.9). For
![]() $t\geq 1$
, since
$t\geq 1$
, since
![]() $\mathrm {tr}\,K_{\epsilon ,\delta }^{q}(t,x,x)$
is a decreasing function in t, we deduce equation (6.6) from equations (6.5), (6.8) and (6.9) and the inequality
$\mathrm {tr}\,K_{\epsilon ,\delta }^{q}(t,x,x)$
is a decreasing function in t, we deduce equation (6.6) from equations (6.5), (6.8) and (6.9) and the inequality
Since
![]() $\mathrm {Tr}\,e^{-t\,\square _{\epsilon ,\delta }^{q}}=\int _{\widetilde {X}}\mathrm {tr}\,K_{\epsilon ,\delta }^{q}(t,x,x)\,dx$
, we get equation (6.7) from equation (6.6).
$\mathrm {Tr}\,e^{-t\,\square _{\epsilon ,\delta }^{q}}=\int _{\widetilde {X}}\mathrm {tr}\,K_{\epsilon ,\delta }^{q}(t,x,x)\,dx$
, we get equation (6.7) from equation (6.6).
(Case 2) Let
![]() $q=1$
. Since
$q=1$
. Since
![]() $\sum _{q}(-1)^{q}\mathrm {Tr}\,e^{-t\,\square _{\epsilon ,\delta }^{q}}=0$
for all
$\sum _{q}(-1)^{q}\mathrm {Tr}\,e^{-t\,\square _{\epsilon ,\delta }^{q}}=0$
for all
![]() $t>0$
, equation (6.7) for
$t>0$
, equation (6.7) for
![]() $q=1$
follows from equation (6.7) for
$q=1$
follows from equation (6.7) for
![]() $q=0,2$
. This completes the proof.
$q=0,2$
. This completes the proof.
Write
![]() $\lambda _{\epsilon }^{q}(k)$
for
$\lambda _{\epsilon }^{q}(k)$
for
![]() $\lambda _{\epsilon ,1}^{q}(k)$
.
$\lambda _{\epsilon ,1}^{q}(k)$
.
Lemma 6.5. There is a constant
![]() $\lambda>0$
such that for all
$\lambda>0$
such that for all
![]() $\epsilon \in (0,\epsilon (\rho )]$
and
$\epsilon \in (0,\epsilon (\rho )]$
and
![]() $q\geq 0$
,
$q\geq 0$
,
Proof. Since
![]() $\dim \widetilde {X}=2$
and hence
$\dim \widetilde {X}=2$
and hence
![]() $\lambda _{\epsilon }^{1}(1)=\lambda _{\epsilon }^{0}(1)$
or
$\lambda _{\epsilon }^{1}(1)=\lambda _{\epsilon }^{0}(1)$
or
![]() $\lambda _{\epsilon }^{1}(1)=\lambda _{\epsilon }^{2}(1)$
, it suffices to prove equation (6.10) for
$\lambda _{\epsilon }^{1}(1)=\lambda _{\epsilon }^{2}(1)$
, it suffices to prove equation (6.10) for
![]() $q=0,2$
. Assume that there is a sequence
$q=0,2$
. Assume that there is a sequence
![]() $\{\epsilon _{n}\}$
such that
$\{\epsilon _{n}\}$
such that
![]() $\epsilon _{n}\to 0$
and
$\epsilon _{n}\to 0$
and
![]() $\lambda _{\epsilon _{n}}^{q}(1)\to 0$
as
$\lambda _{\epsilon _{n}}^{q}(1)\to 0$
as
![]() $n\to \infty $
for
$n\to \infty $
for
![]() $q=0$
or
$q=0$
or
![]() $2$
. By the same argument as in [Reference Yoshikawa42, p.434–p.436] using the uniformity of the Sobolev constant (compare Proposition 6.3), there is a holomorphic q-form
$2$
. By the same argument as in [Reference Yoshikawa42, p.434–p.436] using the uniformity of the Sobolev constant (compare Proposition 6.3), there is a holomorphic q-form
![]() $\psi $
on
$\psi $
on
![]() $X \setminus \mathrm {Sing}\,X$
, which is possibly meromorphic on
$X \setminus \mathrm {Sing}\,X$
, which is possibly meromorphic on
![]() $\widetilde {X}$
, with the following properties:
$\widetilde {X}$
, with the following properties:
-
(i) The complex conjugation
 $\overline {\varphi _{1,\epsilon _{n}}^{q}}$
converges to
$\overline {\varphi _{1,\epsilon _{n}}^{q}}$
converges to
 $\psi $
on every compact subset of
$\psi $
on every compact subset of
 $X\setminus \mathrm {Sing}(X)$
as
$X\setminus \mathrm {Sing}(X)$
as
 $n\to \infty $
.
$n\to \infty $
. -
(ii)
 $\|\psi \|_{L^{2}}=1$
and
$\|\psi \|_{L^{2}}=1$
and
 $\pi ^{*}\psi \perp H^{0}(\widetilde {X},\Omega ^{q}_{\widetilde {X}})$
with respect to the degenerate Kähler metric
$\pi ^{*}\psi \perp H^{0}(\widetilde {X},\Omega ^{q}_{\widetilde {X}})$
with respect to the degenerate Kähler metric
 $\pi ^{*}\gamma _{0}$
on
$\pi ^{*}\gamma _{0}$
on
 $\widetilde {X}$
.
$\widetilde {X}$
.
Since
![]() $\mathrm {Sing}\,X$
consists of isolated orbifold points, it follows from the Riemann extension theorem that
$\mathrm {Sing}\,X$
consists of isolated orbifold points, it follows from the Riemann extension theorem that
![]() $\psi $
extends to a holomorphic q-form on X in the sense of orbifolds. When
$\psi $
extends to a holomorphic q-form on X in the sense of orbifolds. When
![]() $q=0$
,
$q=0$
,
![]() $\psi $
is a constant. When
$\psi $
is a constant. When
![]() $q=2$
, since X has canonical singularities,
$q=2$
, since X has canonical singularities,
![]() $\pi ^{*}\psi $
is a holomorphic
$\pi ^{*}\psi $
is a holomorphic
![]() $2$
-form on
$2$
-form on
![]() $\widetilde {X}$
. In both cases, the condition
$\widetilde {X}$
. In both cases, the condition
![]() $\pi ^{*}\psi \perp H^{0}(\widetilde {X},\Omega ^{q}_{\widetilde {X}})$
implies
$\pi ^{*}\psi \perp H^{0}(\widetilde {X},\Omega ^{q}_{\widetilde {X}})$
implies
![]() $\psi =0$
, which contradicts the other condition
$\psi =0$
, which contradicts the other condition
![]() $\|\psi \|_{L^{2}}=1$
. This proves the result.
$\|\psi \|_{L^{2}}=1$
. This proves the result.
Lemma 6.6. There is a constant
![]() $\lambda '>0$
such that for all
$\lambda '>0$
such that for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $\epsilon \delta ^{-2}\leq \epsilon (\rho )]$
and
$\epsilon \delta ^{-2}\leq \epsilon (\rho )]$
and
![]() $q\geq 0$
,
$q\geq 0$
,
Proof. Firstly, we prove the inequality when
![]() $q=1$
. Since
$q=1$
. Since
![]() $\widetilde {X}$
is a
$\widetilde {X}$
is a
![]() $K3$
surface and hence
$K3$
surface and hence
![]() $\ker \square _{\epsilon ,\delta }^{1}=0$
, we get by equation (6.10)
$\ker \square _{\epsilon ,\delta }^{1}=0$
, we get by equation (6.10)
for all
![]() $\alpha \in A^{0,1}(\widetilde {X})$
, where we used the coincidence of the
$\alpha \in A^{0,1}(\widetilde {X})$
, where we used the coincidence of the
![]() $\bar {\partial }$
-Laplacian and the
$\bar {\partial }$
-Laplacian and the
![]() $\partial $
-Laplacian for Kähler manifolds to get the equality in equation (6.11). By Lemma 4.4, there exist constants
$\partial $
-Laplacian for Kähler manifolds to get the equality in equation (6.11). By Lemma 4.4, there exist constants
![]() $C_{1}>0$
such that for all
$C_{1}>0$
such that for all
![]() $\alpha \in A^{0,1}(\widetilde {X})$
,
$\alpha \in A^{0,1}(\widetilde {X})$
,
Combining these inequalities and equation (6.11), we get for all
![]() $\alpha \in A^{0,1}(\widetilde {X})$
$\alpha \in A^{0,1}(\widetilde {X})$
The result for
![]() $q=1$
follows from equation (6.12). Since
$q=1$
follows from equation (6.12). Since
![]() $\bar {\partial }\varphi _{\epsilon ,\delta }^{0}(1)$
and
$\bar {\partial }\varphi _{\epsilon ,\delta }^{0}(1)$
and
![]() $\bar {\partial }^{*}\varphi _{\epsilon ,\delta }^{2}(1)$
are nonzero eigenforms of
$\bar {\partial }^{*}\varphi _{\epsilon ,\delta }^{2}(1)$
are nonzero eigenforms of
![]() $\square _{\epsilon ,\delta }^{1}$
, we get
$\square _{\epsilon ,\delta }^{1}$
, we get
![]() $\lambda '\leq \lambda _{\epsilon ,\delta }^{1}(1)\leq \lambda _{\epsilon ,\delta }^{0}(1)$
and
$\lambda '\leq \lambda _{\epsilon ,\delta }^{1}(1)\leq \lambda _{\epsilon ,\delta }^{0}(1)$
and
![]() $\lambda '\leq \lambda _{\epsilon ,\delta }^{1}(1)\leq \lambda _{\epsilon ,\delta }^{2}(1)$
.
$\lambda '\leq \lambda _{\epsilon ,\delta }^{1}(1)\leq \lambda _{\epsilon ,\delta }^{2}(1)$
.
Theorem 6.7. There are constants
![]() $\Lambda , C>0$
such that for all
$\Lambda , C>0$
such that for all
![]() $k\in \mathbf {N}$
,
$k\in \mathbf {N}$
,
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $\epsilon \delta ^{-2}\leq \epsilon (\rho )$
and
$\epsilon \delta ^{-2}\leq \epsilon (\rho )$
and
![]() $q\geq 0$
,
$q\geq 0$
,
Proof. By Proposition 6.4, we get for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $\epsilon \delta ^{-2}\leq \epsilon (\rho )$
and
$\epsilon \delta ^{-2}\leq \epsilon (\rho )$
and
![]() $t\in (0,1]$
$t\in (0,1]$
 $$ \begin{align*}\sum_{i=1}^{k}e^{-t\lambda_{\epsilon,\delta}^{q}(i)} \leq h^{0,q}(\widetilde{X})+\sum_{i=1}^{\infty}e^{-t\lambda_{\epsilon,\delta}^{q}(i)} = \mathrm{Tr}\,e^{-t\square_{\epsilon,\delta}^{q}} \leq A'e^{C(\epsilon\delta^{-4}+1)} \,t^{-2}, \end{align*} $$
$$ \begin{align*}\sum_{i=1}^{k}e^{-t\lambda_{\epsilon,\delta}^{q}(i)} \leq h^{0,q}(\widetilde{X})+\sum_{i=1}^{\infty}e^{-t\lambda_{\epsilon,\delta}^{q}(i)} = \mathrm{Tr}\,e^{-t\square_{\epsilon,\delta}^{q}} \leq A'e^{C(\epsilon\delta^{-4}+1)} \,t^{-2}, \end{align*} $$
where
![]() $A'$
is a constant such that
$A'$
is a constant such that
![]() $A\mathrm {Vol}(\widetilde {X},\gamma _{\epsilon ,\delta }) \leq A'$
. Since
$A\mathrm {Vol}(\widetilde {X},\gamma _{\epsilon ,\delta }) \leq A'$
. Since
![]() $\lambda '/\lambda _{\epsilon ,\delta }^{q}(k)\leq 1$
by Lemma 6.6, substituting
$\lambda '/\lambda _{\epsilon ,\delta }^{q}(k)\leq 1$
by Lemma 6.6, substituting
![]() $t:=\lambda '/\lambda _{\epsilon ,\delta }^{q}(k)$
in this inequality and using
$t:=\lambda '/\lambda _{\epsilon ,\delta }^{q}(k)$
in this inequality and using
![]() $\lambda _{\epsilon ,\delta }^{q}(i)/\lambda _{\epsilon ,\delta }^{q}(k)\leq 1$
for
$\lambda _{\epsilon ,\delta }^{q}(i)/\lambda _{\epsilon ,\delta }^{q}(k)\leq 1$
for
![]() $i\leq k$
, we get
$i\leq k$
, we get
 $$ \begin{align*}k\,e^{-\lambda'} \leq \sum_{i=1}^{k}e^{-\frac{\lambda'\lambda_{\epsilon,\delta}^{q}(i)}{\lambda_{\epsilon,\delta}^{q}(k)}} \leq A'e^{C(\epsilon\delta^{-4}+1)}\,\left(\frac{\lambda'}{\lambda_{\epsilon,\delta}^{q}(k)}\right)^{-2}. \end{align*} $$
$$ \begin{align*}k\,e^{-\lambda'} \leq \sum_{i=1}^{k}e^{-\frac{\lambda'\lambda_{\epsilon,\delta}^{q}(i)}{\lambda_{\epsilon,\delta}^{q}(k)}} \leq A'e^{C(\epsilon\delta^{-4}+1)}\,\left(\frac{\lambda'}{\lambda_{\epsilon,\delta}^{q}(k)}\right)^{-2}. \end{align*} $$
We get the result by setting
![]() $\Lambda :=(A')^{-1/2}\lambda ' e^{-\lambda '/2}$
.
$\Lambda :=(A')^{-1/2}\lambda ' e^{-\lambda '/2}$
.
Corollary 6.8. Let C and
![]() $\Lambda $
be the same constants as in Theorem 6.7, and set
$\Lambda $
be the same constants as in Theorem 6.7, and set
![]() $\Lambda (R):=\Lambda e^{-\frac {1}{2}CR}$
and
$\Lambda (R):=\Lambda e^{-\frac {1}{2}CR}$
and
![]() $\Psi (R):=\sum _{k=1}^{\infty }e^{-\frac {1}{2}\Lambda (R) k^{1/2}}$
. Then for all
$\Psi (R):=\sum _{k=1}^{\infty }e^{-\frac {1}{2}\Lambda (R) k^{1/2}}$
. Then for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $\epsilon \delta ^{-2}\leq \epsilon (\rho )$
and
$\epsilon \delta ^{-2}\leq \epsilon (\rho )$
and
![]() $t\geq 1$
, the following inequality holds:
$t\geq 1$
, the following inequality holds:
Proof. Since
![]() $\lambda _{\epsilon ,\delta }^{q}(k)\geq \frac {\Lambda (\epsilon \delta ^{-4}+1)}{2}(k^{1/2}+1)$
by Theorem 6.7, we get
$\lambda _{\epsilon ,\delta }^{q}(k)\geq \frac {\Lambda (\epsilon \delta ^{-4}+1)}{2}(k^{1/2}+1)$
by Theorem 6.7, we get
![]() $\sum _{k=1}^{\infty }e^{-t\lambda _{\epsilon ,\delta }^{q}(k)} \leq e^{-t\Lambda (\epsilon \delta ^{-4}+1)/2}\sum _{k=1}^{\infty }e^{-\frac {1}{2}t\Lambda (\epsilon \delta ^{-4}+1) k^{1/2}} \leq \Psi (\epsilon \delta ^{-4}+1)\,e^{-t\Lambda (\epsilon \delta ^{-4}+1)/2}$
for
$\sum _{k=1}^{\infty }e^{-t\lambda _{\epsilon ,\delta }^{q}(k)} \leq e^{-t\Lambda (\epsilon \delta ^{-4}+1)/2}\sum _{k=1}^{\infty }e^{-\frac {1}{2}t\Lambda (\epsilon \delta ^{-4}+1) k^{1/2}} \leq \Psi (\epsilon \delta ^{-4}+1)\,e^{-t\Lambda (\epsilon \delta ^{-4}+1)/2}$
for
![]() $t\geq 1$
.
$t\geq 1$
.
We also need an estimate for the heat kernel
![]() $K_{\epsilon ,\delta ,\infty }^{q}(t,x,y)$
of
$K_{\epsilon ,\delta ,\infty }^{q}(t,x,y)$
of
![]() $(\widetilde {V}(\infty ),\kappa _{\epsilon ,\delta })$
.
$(\widetilde {V}(\infty ),\kappa _{\epsilon ,\delta })$
.
Proposition 6.9. There are constants
![]() $A', C'>0$
such that for all
$A', C'>0$
such that for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $\epsilon \delta ^{-2}\leq \epsilon (\rho )$
,
$\epsilon \delta ^{-2}\leq \epsilon (\rho )$
,
![]() $x\in \widetilde {V}(\infty )$
,
$x\in \widetilde {V}(\infty )$
,
![]() $t>0$
and
$t>0$
and
![]() $q\geq 0$
, the following inequality holds:
$q\geq 0$
, the following inequality holds:
Proof. When
![]() $q=0$
, the result follows from Lemma 6.2 and [Reference Carlen, Kusuoka and Stroock10, Thms. 2.1 and 2.16]. Let
$q=0$
, the result follows from Lemma 6.2 and [Reference Carlen, Kusuoka and Stroock10, Thms. 2.1 and 2.16]. Let
![]() $q>0$
. Since
$q>0$
. Since
![]() $|\mathrm {Ric}(\gamma _{\epsilon ,\delta })|\leq C(\epsilon \delta ^{-4}+1)$
by Lemma 4.5, we deduce from [Reference Hess, Schrader and Uhlenbrock24, p.32 l.4-l.5] and the Lichnerowicz formula for
$|\mathrm {Ric}(\gamma _{\epsilon ,\delta })|\leq C(\epsilon \delta ^{-4}+1)$
by Lemma 4.5, we deduce from [Reference Hess, Schrader and Uhlenbrock24, p.32 l.4-l.5] and the Lichnerowicz formula for
![]() $\square _{\epsilon ,\delta }^{q}$
that
$\square _{\epsilon ,\delta }^{q}$
that
This proves the result for
![]() $t\leq 1$
. Since equation (6.5) remains valid for
$t\leq 1$
. Since equation (6.5) remains valid for
![]() $K_{\epsilon ,\delta ,\infty }^{q}(t,x,y)$
by the fact that
$K_{\epsilon ,\delta ,\infty }^{q}(t,x,y)$
by the fact that
![]() $K_{\epsilon ,\delta ,\infty }^{q}(t,x,y)$
is obtained as the limit
$K_{\epsilon ,\delta ,\infty }^{q}(t,x,y)$
is obtained as the limit
![]() $R\to \infty $
of the Dirichlet heat kernel of
$R\to \infty $
of the Dirichlet heat kernel of
![]() $\widetilde {V}(R)$
, the result for
$\widetilde {V}(R)$
, the result for
![]() $t\geq 1$
also follows. This completes the proof.
$t\geq 1$
also follows. This completes the proof.
7 Behaviour of (equivariant) analytic torsion
In the previous sections, the additional parameter
![]() $\delta $
is pretty harmless, and the results still hold in its presence. This parameter will play a more essential role in this section. Indeed, we shall prove the following:
$\delta $
is pretty harmless, and the results still hold in its presence. This parameter will play a more essential role in this section. Indeed, we shall prove the following:
Theorem 7.1. There exist constants
![]() $C_{0}(k),C_{1}(k)>0$
depending only on
$C_{0}(k),C_{1}(k)>0$
depending only on
![]() $k=\#\mathrm {Sing}(Y)$
such that
$k=\#\mathrm {Sing}(Y)$
such that
7.1 Existence of limits
By Corollary 5.3, the first limit exists and is independent of the choice of a cut-off function
![]() $\rho $
. For the second limit, we have
$\rho $
. For the second limit, we have
Proposition 7.2. For any
![]() $\delta \in (0,1]$
, the number
$\delta \in (0,1]$
, the number
is independent of
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $0<\epsilon \delta ^{-2}\leq \epsilon (\rho )$
. In particular, for any
$0<\epsilon \delta ^{-2}\leq \epsilon (\rho )$
. In particular, for any
![]() $\delta \in (0,1]$
, the following limit exists as
$\delta \in (0,1]$
, the following limit exists as
![]() $\epsilon \to 0$
$\epsilon \to 0$
and the limit is independent of
![]() $\delta \in (0,1]$
and the choice of a cut-off function
$\delta \in (0,1]$
and the choice of a cut-off function
![]() $\rho $
.
$\rho $
.
Proof.
(Step 1) Let
![]() $g_{0},g_{1}$
be
$g_{0},g_{1}$
be
![]() $\theta $
-invariant Kähler metrics on
$\theta $
-invariant Kähler metrics on
![]() $\widetilde {X}$
. Let
$\widetilde {X}$
. Let
![]() $\widetilde {\mathrm {Td}}_{\theta }(T\widetilde {X};g_{0},g_{1})^{(1,1)}$
be the Bott-Chern class such that
$\widetilde {\mathrm {Td}}_{\theta }(T\widetilde {X};g_{0},g_{1})^{(1,1)}$
be the Bott-Chern class such that
By Bismut [Reference Bismut4, Th. 2.5],
 $$ \begin{align} \log \left( \frac{\tau_{\mathbf{Z}_{2}}(\widetilde{X},g_{0})(\theta)\mathrm{Vol}(\widetilde{X},g_{0})}{\tau_{\mathbf{Z}_{2}}(\widetilde{X},g_{1})(\theta)\mathrm{ Vol}(\widetilde{X},g_{1})} \right) = \int_{\widetilde{X}^{\theta}} \widetilde{\mathrm{Td}}_{\theta}(T\widetilde{X};g_{0},g_{1}). \end{align} $$
$$ \begin{align} \log \left( \frac{\tau_{\mathbf{Z}_{2}}(\widetilde{X},g_{0})(\theta)\mathrm{Vol}(\widetilde{X},g_{0})}{\tau_{\mathbf{Z}_{2}}(\widetilde{X},g_{1})(\theta)\mathrm{ Vol}(\widetilde{X},g_{1})} \right) = \int_{\widetilde{X}^{\theta}} \widetilde{\mathrm{Td}}_{\theta}(T\widetilde{X};g_{0},g_{1}). \end{align} $$
Since
by [Reference Yoshikawa43, Prop. 5.3], we have the following equality of Bott-Chern classes:
 $$ \begin{align*}\begin{aligned} \widetilde{\mathrm{Td}}_{\theta}(T\widetilde{X};g_{0},g_{1})^{(1,1)} &= \frac{1}{8} \widetilde{c_{1}(T\widetilde{X})|_{\widetilde{X}^{\theta}}c_{1}(T\widetilde{X}^{\theta})}(g_{0},g_{1}) - \frac{1}{12} \widetilde{c_{1}(T\widetilde{X}^{\theta})^{2}}(g_{0},g_{1}) \\ &= \frac{1}{8} \widetilde{c_{1}}(T\widetilde{X};g_{0},g_{1})|_{\widetilde{X}^{\theta}}c_{1}(T\widetilde{X}^{\theta},g_{1}) + \frac{1}{8} c_{1}(T\widetilde{X},g_{0})|_{\widetilde{X}^{\theta}}\widetilde{c_{1}}(T\widetilde{X}^{\theta};g_{0},g_{1}) \\ &\quad - \frac{1}{12} \widetilde{c_{1}}(T\widetilde{X}^{\theta};g_{0},g_{1})\{c_{1}(T\widetilde{X}^{\theta},g_{0})+c_{1}(T\widetilde{X}^{\theta},g_{1})\}, \end{aligned} \end{align*} $$
$$ \begin{align*}\begin{aligned} \widetilde{\mathrm{Td}}_{\theta}(T\widetilde{X};g_{0},g_{1})^{(1,1)} &= \frac{1}{8} \widetilde{c_{1}(T\widetilde{X})|_{\widetilde{X}^{\theta}}c_{1}(T\widetilde{X}^{\theta})}(g_{0},g_{1}) - \frac{1}{12} \widetilde{c_{1}(T\widetilde{X}^{\theta})^{2}}(g_{0},g_{1}) \\ &= \frac{1}{8} \widetilde{c_{1}}(T\widetilde{X};g_{0},g_{1})|_{\widetilde{X}^{\theta}}c_{1}(T\widetilde{X}^{\theta},g_{1}) + \frac{1}{8} c_{1}(T\widetilde{X},g_{0})|_{\widetilde{X}^{\theta}}\widetilde{c_{1}}(T\widetilde{X}^{\theta};g_{0},g_{1}) \\ &\quad - \frac{1}{12} \widetilde{c_{1}}(T\widetilde{X}^{\theta};g_{0},g_{1})\{c_{1}(T\widetilde{X}^{\theta},g_{0})+c_{1}(T\widetilde{X}^{\theta},g_{1})\}, \end{aligned} \end{align*} $$
where [Reference Gillet and Soulé21, Eq. (1.3.1.2)] is used to get the second equality. For a holomorphic line bundle L and Hermitian metrics
![]() $h_{0},h_{1}$
on L, we have
$h_{0},h_{1}$
on L, we have
by [Reference Gillet and Soulé21, Eq. (1.2.5.1)]. (Our sign convention is different from the one in Gillet-Soulé [Reference Gillet and Soulé21]. Our
![]() $\widetilde {c_{1}}(L;h_{0},h_{1})$
is
$\widetilde {c_{1}}(L;h_{0},h_{1})$
is
![]() $-\widetilde {c_{1}}(L;h_{0},h_{1})$
in [Reference Gillet and Soulé21].) Hence
$-\widetilde {c_{1}}(L;h_{0},h_{1})$
in [Reference Gillet and Soulé21].) Hence
 $$ \begin{align} \begin{aligned} \widetilde{\mathrm{Td}}_{\theta}(T\widetilde{X};g_{0},g_{1})^{(1,1)} &\equiv \frac{1}{8} \left. \log\left(\frac{\det g_{0}}{\det g_{1}}\right) \right|_{\widetilde{X}^{\theta}} c_{1}(\widetilde{X}^{\theta},g_{1}) + \frac{1}{8} \left. c_{1}(\widetilde{X},g_{0}) \log\left(\frac{g_{0}}{g_{1}} \right|_{\widetilde{X}^{\theta}} \right) \\ &\quad - \frac{1}{12} \left. \log\left(\frac{g_{0}}{g_{1}} \right|_{\widetilde{X}^{\theta}} \right) \{c_{1}(\widetilde{X}^{\theta},g_{0})+c_{1}(\widetilde{X}^{\theta},g_{1})\} \\ &\qquad \mod \mathrm{Im}\,\partial+\mathrm{Im}\,\bar{\partial}. \end{aligned} \end{align} $$
$$ \begin{align} \begin{aligned} \widetilde{\mathrm{Td}}_{\theta}(T\widetilde{X};g_{0},g_{1})^{(1,1)} &\equiv \frac{1}{8} \left. \log\left(\frac{\det g_{0}}{\det g_{1}}\right) \right|_{\widetilde{X}^{\theta}} c_{1}(\widetilde{X}^{\theta},g_{1}) + \frac{1}{8} \left. c_{1}(\widetilde{X},g_{0}) \log\left(\frac{g_{0}}{g_{1}} \right|_{\widetilde{X}^{\theta}} \right) \\ &\quad - \frac{1}{12} \left. \log\left(\frac{g_{0}}{g_{1}} \right|_{\widetilde{X}^{\theta}} \right) \{c_{1}(\widetilde{X}^{\theta},g_{0})+c_{1}(\widetilde{X}^{\theta},g_{1})\} \\ &\qquad \mod \mathrm{Im}\,\partial+\mathrm{Im}\,\bar{\partial}. \end{aligned} \end{align} $$
(Step 2) We set
![]() $g_{0}=\gamma _{\epsilon ,\delta }$
and
$g_{0}=\gamma _{\epsilon ,\delta }$
and
![]() $g_{1}=\gamma _{\epsilon (\rho )}$
in Step 1. Since
$g_{1}=\gamma _{\epsilon (\rho )}$
in Step 1. Since
![]() $g_{0}=\gamma _{\epsilon ,\delta }$
is Ricci-flat on a neighbourhood of
$g_{0}=\gamma _{\epsilon ,\delta }$
is Ricci-flat on a neighbourhood of
![]() $\widetilde {X}^{\theta }$
, we have
$\widetilde {X}^{\theta }$
, we have
Since the volume form of EH instanton
![]() $i\partial \bar {\partial }F_{\epsilon }$
is the standard Euclidean volume form
$i\partial \bar {\partial }F_{\epsilon }$
is the standard Euclidean volume form
and since
![]() $\gamma _{\epsilon ,\delta }=i\partial \bar {\partial }F_{\epsilon }$
on
$\gamma _{\epsilon ,\delta }=i\partial \bar {\partial }F_{\epsilon }$
on
![]() $\widetilde {V}(\delta )_{\frak p}$
, we get
$\widetilde {V}(\delta )_{\frak p}$
, we get
 $$ \begin{align*}\left. \left( \frac{\det\gamma_{\epsilon,\delta}}{\det\gamma_{\epsilon(\rho)}}\right) \right|_{\widetilde{X}^{\theta}} = \left. \left( \frac{\gamma_{\epsilon,\delta}^{2}/2!}{\gamma_{\epsilon(\rho)}^{2}/2!}\right) \right|_{\widetilde{X}^{\theta}} = 1. \end{align*} $$
$$ \begin{align*}\left. \left( \frac{\det\gamma_{\epsilon,\delta}}{\det\gamma_{\epsilon(\rho)}}\right) \right|_{\widetilde{X}^{\theta}} = \left. \left( \frac{\gamma_{\epsilon,\delta}^{2}/2!}{\gamma_{\epsilon(\rho)}^{2}/2!}\right) \right|_{\widetilde{X}^{\theta}} = 1. \end{align*} $$
If
![]() $E_{i}\cong \mathbf {P}^{1}$
is a component of
$E_{i}\cong \mathbf {P}^{1}$
is a component of
![]() $\widetilde {X}^{\theta }$
, then
$\widetilde {X}^{\theta }$
, then
![]() $(E_{i},\gamma _{\epsilon ,\delta }|_{E_{i}})\cong (\mathbf {P}^{1},\epsilon \,\omega _{\mathrm {FS}})$
and
$(E_{i},\gamma _{\epsilon ,\delta }|_{E_{i}})\cong (\mathbf {P}^{1},\epsilon \,\omega _{\mathrm {FS}})$
and
![]() $(E_{i},\gamma _{\epsilon (\rho )}|_{E_{i}})\cong (\mathbf {P}^{1},\epsilon (\rho )\omega _{\mathrm {FS}})$
. Hence
$(E_{i},\gamma _{\epsilon (\rho )}|_{E_{i}})\cong (\mathbf {P}^{1},\epsilon (\rho )\omega _{\mathrm {FS}})$
. Hence
 $$ \begin{align*}\left.\frac{\gamma_{\epsilon,\delta}}{\gamma_{\epsilon(\rho)}}\right|_{\widetilde{X}^{\theta}}=\frac{\epsilon}{\epsilon(\rho)}. \end{align*} $$
$$ \begin{align*}\left.\frac{\gamma_{\epsilon,\delta}}{\gamma_{\epsilon(\rho)}}\right|_{\widetilde{X}^{\theta}}=\frac{\epsilon}{\epsilon(\rho)}. \end{align*} $$
Altogether, we get
 $$ \begin{align} \begin{aligned} \,& \int_{\widetilde{X}^{\theta}} \widetilde{\mathrm{Td}}_{\theta}(T\widetilde{X};\gamma_{\epsilon,\delta},\gamma_{\epsilon(\rho)})^{(1,1)} = -\int_{\widetilde{X}^{\theta}} \frac{1}{12} \left. \log\left(\frac{\gamma_{\epsilon,\delta}}{\gamma_{\epsilon(\rho)}} \right|{}_{\widetilde{X}^{\theta}} \right) \{c_{1}(\widetilde{X}^{\theta},\gamma_{\epsilon,\delta})+c_{1}(\widetilde{X}^{\theta},\gamma_{\epsilon(\rho)})\} \\ &= -\frac{\log(\epsilon/\epsilon(\rho))}{6} \int_{\widetilde{X}^{\theta}}c_{1}(\widetilde{X}^{\theta}) = -\frac{\log(\epsilon/\epsilon(\rho))}{6}\chi(\widetilde{X}^{\theta}) = -\frac{k}{3}\,\log\frac{\epsilon}{\epsilon(\rho)}, \end{aligned} \end{align} $$
$$ \begin{align} \begin{aligned} \,& \int_{\widetilde{X}^{\theta}} \widetilde{\mathrm{Td}}_{\theta}(T\widetilde{X};\gamma_{\epsilon,\delta},\gamma_{\epsilon(\rho)})^{(1,1)} = -\int_{\widetilde{X}^{\theta}} \frac{1}{12} \left. \log\left(\frac{\gamma_{\epsilon,\delta}}{\gamma_{\epsilon(\rho)}} \right|{}_{\widetilde{X}^{\theta}} \right) \{c_{1}(\widetilde{X}^{\theta},\gamma_{\epsilon,\delta})+c_{1}(\widetilde{X}^{\theta},\gamma_{\epsilon(\rho)})\} \\ &= -\frac{\log(\epsilon/\epsilon(\rho))}{6} \int_{\widetilde{X}^{\theta}}c_{1}(\widetilde{X}^{\theta}) = -\frac{\log(\epsilon/\epsilon(\rho))}{6}\chi(\widetilde{X}^{\theta}) = -\frac{k}{3}\,\log\frac{\epsilon}{\epsilon(\rho)}, \end{aligned} \end{align} $$
where we used the fact
![]() $\widetilde {X}^{\theta }=E_{1}\amalg \cdots \amalg E_{k}$
,
$\widetilde {X}^{\theta }=E_{1}\amalg \cdots \amalg E_{k}$
,
![]() $k=\#\mathrm {Sing}\,X$
,
$k=\#\mathrm {Sing}\,X$
,
![]() $E_{i}\cong \mathbf {P}^{1}$
. This, together with equation (7.1), yields that
$E_{i}\cong \mathbf {P}^{1}$
. This, together with equation (7.1), yields that
is independent of
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $0<\epsilon \delta ^{-2}\leq \epsilon (\rho )$
.
$0<\epsilon \delta ^{-2}\leq \epsilon (\rho )$
.
(Step 3) Let
![]() $\chi $
be another cut-off function to glue Eguchi-Hanson instanton to the initial Kähler form
$\chi $
be another cut-off function to glue Eguchi-Hanson instanton to the initial Kähler form
![]() $\gamma _{0}$
on X (compare Sections 5.2.1 and 5.2.3). Then there exists
$\gamma _{0}$
on X (compare Sections 5.2.1 and 5.2.3). Then there exists
![]() $\epsilon (\chi )\in (0,1)$
such that the function
$\epsilon (\chi )\in (0,1)$
such that the function
![]() $\phi ^{\prime }_{\epsilon ,\delta }(z):=\|z\|^{2}+\chi _{\delta }(\|z\|)E(z,\epsilon )$
on
$\phi ^{\prime }_{\epsilon ,\delta }(z):=\|z\|^{2}+\chi _{\delta }(\|z\|)E(z,\epsilon )$
on
![]() $V(\infty )\setminus \{0\}$
is a potential of a Kähler form on
$V(\infty )\setminus \{0\}$
is a potential of a Kähler form on
![]() $T^{*}\mathbf {P}^{1}=\widetilde {V}(\infty )$
for any
$T^{*}\mathbf {P}^{1}=\widetilde {V}(\infty )$
for any
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $0<\epsilon \delta ^{-2}\leq \epsilon (\chi )$
. Let
$0<\epsilon \delta ^{-2}\leq \epsilon (\chi )$
. Let
![]() $\gamma ^{\prime }_{\epsilon ,\delta }$
be the families of Kähler forms on
$\gamma ^{\prime }_{\epsilon ,\delta }$
be the families of Kähler forms on
![]() $\widetilde {X}$
constructed in the same way as in equation (4.12), using
$\widetilde {X}$
constructed in the same way as in equation (4.12), using
![]() $\kappa ^{\prime }_{\epsilon ,\delta }:=i\partial \bar {\partial }\phi ^{\prime }_{\epsilon ,\delta }$
instead of
$\kappa ^{\prime }_{\epsilon ,\delta }:=i\partial \bar {\partial }\phi ^{\prime }_{\epsilon ,\delta }$
instead of
![]() $\kappa _{\epsilon ,\delta }$
. By Step 2, we get
$\kappa _{\epsilon ,\delta }$
. By Step 2, we get
for any
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $0<\epsilon \delta ^{-2}\leq \epsilon (\chi )$
. To prove the independence of the limit
$0<\epsilon \delta ^{-2}\leq \epsilon (\chi )$
. To prove the independence of the limit
![]() $\lim _{\epsilon \to 0}\epsilon ^{k/3}\tau _{\mathbf {Z}_{2}}(\widetilde {X},\gamma _{\epsilon ,\delta })(\theta )\mathrm {Vol}(\widetilde {X},\gamma _{\epsilon ,\delta })$
from the choice of
$\lim _{\epsilon \to 0}\epsilon ^{k/3}\tau _{\mathbf {Z}_{2}}(\widetilde {X},\gamma _{\epsilon ,\delta })(\theta )\mathrm {Vol}(\widetilde {X},\gamma _{\epsilon ,\delta })$
from the choice of
![]() $\rho $
, we must prove
$\rho $
, we must prove
We set
![]() $g_{0}=\gamma _{\epsilon (\rho )}$
and
$g_{0}=\gamma _{\epsilon (\rho )}$
and
![]() $g_{1}=\gamma ^{\prime }_{\epsilon (\chi )}$
in equation (7.2). By the same computation as in equation (7.3), we get
$g_{1}=\gamma ^{\prime }_{\epsilon (\chi )}$
in equation (7.2). By the same computation as in equation (7.3), we get
This, together with equation (7.1), yields equation (7.4). This completes the proof.
7.2 A comparison of heat kernels
Recall that
![]() $K_{\epsilon ,\delta }^{q}(t, x, y)$
denote the heat kernel of the Hodge-Kodaira Laplacian
$K_{\epsilon ,\delta }^{q}(t, x, y)$
denote the heat kernel of the Hodge-Kodaira Laplacian
![]() $\square _{q}^{\epsilon ,\delta }$
for the Kähler metric
$\square _{q}^{\epsilon ,\delta }$
for the Kähler metric
![]() $\gamma _{\epsilon ,\delta }$
on
$\gamma _{\epsilon ,\delta }$
on
![]() $\widetilde {X}$
, and
$\widetilde {X}$
, and
![]() $K_{0}^q(t, x, y)$
the heat kernel of the Hodge-Kodaira Laplacian
$K_{0}^q(t, x, y)$
the heat kernel of the Hodge-Kodaira Laplacian
![]() $\square _{q}^{0}$
for the Kähler metric
$\square _{q}^{0}$
for the Kähler metric
![]() $\gamma _{0}$
on X. For
$\gamma _{0}$
on X. For
![]() $0<r\leq 4$
, let
$0<r\leq 4$
, let
 $$ \begin{align*}\widetilde{V}_r:=\bigcup_{{\frak p}\in\mathrm{Sing}(X)} \widetilde{V}(r)_{\frak p}, \qquad \widetilde{X}_r:=\widetilde{X}-\widetilde{V}_r. \end{align*} $$
$$ \begin{align*}\widetilde{V}_r:=\bigcup_{{\frak p}\in\mathrm{Sing}(X)} \widetilde{V}(r)_{\frak p}, \qquad \widetilde{X}_r:=\widetilde{X}-\widetilde{V}_r. \end{align*} $$
Define
![]() $\widetilde {V}_{\infty }$
to be
$\widetilde {V}_{\infty }$
to be
![]() $\widetilde {V}_4$
extended by k copies of the infinite cone
$\widetilde {V}_4$
extended by k copies of the infinite cone
![]() $(\mathbf C^2 - B(4))/\{\pm 1\}$
. The metric
$(\mathbf C^2 - B(4))/\{\pm 1\}$
. The metric
![]() $\gamma _{\epsilon , \delta }|_{\widetilde {V}_4}$
similarly extends to a Kähler metric
$\gamma _{\epsilon , \delta }|_{\widetilde {V}_4}$
similarly extends to a Kähler metric
![]() $\gamma _{\epsilon , \delta }^{\infty }$
on
$\gamma _{\epsilon , \delta }^{\infty }$
on
![]() $\widetilde {V}_{\infty }$
. We denote by
$\widetilde {V}_{\infty }$
. We denote by
![]() $K_{\epsilon , \delta , \infty }^q(t, x, y)$
the corresponding heat kernel on
$K_{\epsilon , \delta , \infty }^q(t, x, y)$
the corresponding heat kernel on
![]() $\widetilde {V}_{\infty }$
. Similarly, we have the corresponding
$\widetilde {V}_{\infty }$
. Similarly, we have the corresponding
![]() $X_r, V_r, V_{\infty }$
on X, with
$X_r, V_r, V_{\infty }$
on X, with
![]() $X_r$
identified with
$X_r$
identified with
![]() $\widetilde {X}_r$
. Note that
$\widetilde {X}_r$
. Note that
![]() $V_{\infty }$
is just k copies of the infinite cone.
$V_{\infty }$
is just k copies of the infinite cone.
We first established some uniform estimates on the heat kernel
![]() $K_{\epsilon ,\delta }^{q}(t, x, y)$
, improving on Proposition 6.4 when the points are in specific regions.
$K_{\epsilon ,\delta }^{q}(t, x, y)$
, improving on Proposition 6.4 when the points are in specific regions.
Theorem 7.3. There are constants
![]() $A, C$
depending only on the Sobolev constant and dimension such that, for all
$A, C$
depending only on the Sobolev constant and dimension such that, for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $\epsilon \delta ^{-2}\leq \epsilon (\rho )$
and
$\epsilon \delta ^{-2}\leq \epsilon (\rho )$
and
![]() $0\leq q\leq 2$
, we have
$0\leq q\leq 2$
, we have
Similarly, we have
![]() $\forall x \in \widetilde {X}_{3\delta }, \ z\in \widetilde {V}_{2\delta }, \ t>0$
,
$\forall x \in \widetilde {X}_{3\delta }, \ z\in \widetilde {V}_{2\delta }, \ t>0$
,
Here
![]() $d, \ d^*_{\epsilon , \delta }$
could act on either the x or z variable. Finally, for
$d, \ d^*_{\epsilon , \delta }$
could act on either the x or z variable. Finally, for
![]() $0<r<2\delta $
,
$0<r<2\delta $
,
![]() $x \in \widetilde {X}_{3\delta }, \ \ z\in \widetilde {V}_{2\delta , r} = \widetilde {V}_{2\delta }-\widetilde {V}_{r}$
, and
$x \in \widetilde {X}_{3\delta }, \ \ z\in \widetilde {V}_{2\delta , r} = \widetilde {V}_{2\delta }-\widetilde {V}_{r}$
, and
![]() $i\in \mathbf N$
,
$i\in \mathbf N$
,
for a constant
![]() $C(i, \delta , r)$
, depending on
$C(i, \delta , r)$
, depending on
![]() $i, \delta , r$
. Here
$i, \delta , r$
. Here
![]() $\nabla ^i$
denotes the ith covariant derivative with respect to the metric
$\nabla ^i$
denotes the ith covariant derivative with respect to the metric
![]() $\gamma _{\epsilon ,\delta }$
acting on either variable.
$\gamma _{\epsilon ,\delta }$
acting on either variable.
Proof. Throughout the proof, we fix
![]() $ x \in \widetilde {X}_{3\delta }, \ \ z\in \widetilde {V}_{2\delta }, \ \ t>0.$
Since the Ricci curvature of
$ x \in \widetilde {X}_{3\delta }, \ \ z\in \widetilde {V}_{2\delta }, \ \ t>0.$
Since the Ricci curvature of
![]() $\gamma _{\epsilon , \delta }$
is bounded by Lemma 4.5, the Sobolev estimate, together with the Moser iteration technique combined with the finite propagation speed argument as in Cheeger-Gromov-Taylor [Reference Cheeger, Gromov and Taylor13], gives the uniform estimate
$\gamma _{\epsilon , \delta }$
is bounded by Lemma 4.5, the Sobolev estimate, together with the Moser iteration technique combined with the finite propagation speed argument as in Cheeger-Gromov-Taylor [Reference Cheeger, Gromov and Taylor13], gives the uniform estimate
Indeed, the finite propagation speed technique gives us the
![]() $L^2$
estimate
$L^2$
estimate
for some uniform constant c. Now Moser iteration as in [Reference Cheeger, Gromov and Taylor13][pages 16–26], together with semi-group domination [Reference Hess, Schrader and Uhlenbrock24], yields the desired estimate.
For the estimate on
![]() $ d K_{\epsilon }^q(t, x, z), \ d^*_{\epsilon , \delta } K_{\epsilon }^q(t, x, z)$
, let
$ d K_{\epsilon }^q(t, x, z), \ d^*_{\epsilon , \delta } K_{\epsilon }^q(t, x, z)$
, let
![]() $\eta (r)$
be a smooth cut-off function that is identically
$\eta (r)$
be a smooth cut-off function that is identically
![]() $1$
for
$1$
for
![]() $|r|\leq \delta /8$
and identically
$|r|\leq \delta /8$
and identically
![]() $0$
for
$0$
for
![]() $|r|\geq \delta /4$
and
$|r|\geq \delta /4$
and
![]() $|\eta '| \leq \frac {16}{\delta }$
. We will continue to denote by
$|\eta '| \leq \frac {16}{\delta }$
. We will continue to denote by
![]() $\eta $
its composition with a distance function (either
$\eta $
its composition with a distance function (either
![]() $d(x, \cdot )$
or
$d(x, \cdot )$
or
![]() $d(z, \cdot )$
). Note then
$d(z, \cdot )$
). Note then
 $$ \begin{align*} \|(d+d^*_{\epsilon, \delta} )_z[\eta K_{\epsilon}^q(t, \cdot, \cdot)] \|^2_{L^2(B_{\delta/4}(x)\times B_{\delta/4}(z) )}& = \|(d)_z[\eta K_{\epsilon}^q(t, \cdot, \cdot)] \|^2_{L^2(B_{\delta/4}(x)\times B_{\delta/4}(z) )} \\ &\quad + \|(d^*_{\epsilon, \delta} )_z[\eta K_{\epsilon}^q(t, \cdot, \cdot)] \|^2_{L^2(B_{\delta/4}(x)\times B_{\delta/4}(z) )}, \end{align*} $$
$$ \begin{align*} \|(d+d^*_{\epsilon, \delta} )_z[\eta K_{\epsilon}^q(t, \cdot, \cdot)] \|^2_{L^2(B_{\delta/4}(x)\times B_{\delta/4}(z) )}& = \|(d)_z[\eta K_{\epsilon}^q(t, \cdot, \cdot)] \|^2_{L^2(B_{\delta/4}(x)\times B_{\delta/4}(z) )} \\ &\quad + \|(d^*_{\epsilon, \delta} )_z[\eta K_{\epsilon}^q(t, \cdot, \cdot)] \|^2_{L^2(B_{\delta/4}(x)\times B_{\delta/4}(z) )}, \end{align*} $$
from which we deduce
 $$ \begin{align*} \|(d)_z[ K_{\epsilon}^q(t, \cdot, \cdot)] \|_{L^2(B_{\delta/8}(x)\times B_{\delta/8}(z) )} & \leq \|(d+d^*_{\epsilon, \delta} )_z[\eta K_{\epsilon}^q(t, \cdot, \cdot)] \|_{L^2(B_{\delta/4}(x)\times B_{\delta/4}(z) )} \\ &\quad + \frac{16}{\delta} \| K_{\epsilon}^q(t, \cdot, \cdot) \|_{L^2(B_{\delta/4}(x)\times B_{\delta/4}(z) )} \\ & \leq \|(d+d^*_{\epsilon, \delta} )_z[ K_{\epsilon}^q(t, \cdot, \cdot)] \|_{L^2(B_{\delta/4}(x)\times B_{\delta/4}(z) )} \\ &\quad + \frac{32}{\delta} \| K_{\epsilon}^q(t, \cdot, \cdot) \|_{L^2(B_{\delta/4}(x)\times B_{\delta/4}(z) )}. \end{align*} $$
$$ \begin{align*} \|(d)_z[ K_{\epsilon}^q(t, \cdot, \cdot)] \|_{L^2(B_{\delta/8}(x)\times B_{\delta/8}(z) )} & \leq \|(d+d^*_{\epsilon, \delta} )_z[\eta K_{\epsilon}^q(t, \cdot, \cdot)] \|_{L^2(B_{\delta/4}(x)\times B_{\delta/4}(z) )} \\ &\quad + \frac{16}{\delta} \| K_{\epsilon}^q(t, \cdot, \cdot) \|_{L^2(B_{\delta/4}(x)\times B_{\delta/4}(z) )} \\ & \leq \|(d+d^*_{\epsilon, \delta} )_z[ K_{\epsilon}^q(t, \cdot, \cdot)] \|_{L^2(B_{\delta/4}(x)\times B_{\delta/4}(z) )} \\ &\quad + \frac{32}{\delta} \| K_{\epsilon}^q(t, \cdot, \cdot) \|_{L^2(B_{\delta/4}(x)\times B_{\delta/4}(z) )}. \end{align*} $$
Now the same finite propagation speed technique gives
which in turn gives
The same method as above then yields
The others can be proven in exactly the same way.
Finally, for
![]() $0<r<2\delta $
, we note that the curvature tensor and its derivatives of
$0<r<2\delta $
, we note that the curvature tensor and its derivatives of
![]() $\gamma _{\epsilon ,\delta }$
are bounded in
$\gamma _{\epsilon ,\delta }$
are bounded in
![]() $\widetilde {V}_{2\delta , r} = \widetilde {V}_{2\delta }-\widetilde {V}_{r}$
by a constant depending on
$\widetilde {V}_{2\delta , r} = \widetilde {V}_{2\delta }-\widetilde {V}_{r}$
by a constant depending on
![]() $\delta , r$
. Moreover, the injectivity radius of
$\delta , r$
. Moreover, the injectivity radius of
![]() $\gamma _{\epsilon ,\delta }$
in
$\gamma _{\epsilon ,\delta }$
in
![]() $\widetilde {V}_{2\delta , r}$
is bounded away from zero by a constant depending on
$\widetilde {V}_{2\delta , r}$
is bounded away from zero by a constant depending on
![]() $\delta , r$
. Hence, by the elliptic estimate combined with the argument as before, we have, for
$\delta , r$
. Hence, by the elliptic estimate combined with the argument as before, we have, for
![]() $x \in \widetilde {X}_{3\delta }, \ \ z\in \widetilde {V}_{2\delta , r} = \widetilde {V}_{2\delta }-\widetilde {V}_{r}$
, and
$x \in \widetilde {X}_{3\delta }, \ \ z\in \widetilde {V}_{2\delta , r} = \widetilde {V}_{2\delta }-\widetilde {V}_{r}$
, and
![]() $i\in \mathbf N$
,
$i\in \mathbf N$
,
for a constant
![]() $C(i, \delta , r)$
depending on
$C(i, \delta , r)$
depending on
![]() $i, \delta , r$
.
$i, \delta , r$
.
Theorem 7.4. There are constants
![]() $A, C$
depending only on the Sobolev constant and dimension such that, for all
$A, C$
depending only on the Sobolev constant and dimension such that, for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $\epsilon \delta ^{-2}\leq \epsilon (\rho )$
and
$\epsilon \delta ^{-2}\leq \epsilon (\rho )$
and
![]() $0\leq q\leq 2$
, we have
$0\leq q\leq 2$
, we have
Furthermore,
![]() $\forall x, y \in \widetilde {X}_{3\delta }, \ \ t>0$
, we have the pointwise (although not necessarily uniform) convergence as
$\forall x, y \in \widetilde {X}_{3\delta }, \ \ t>0$
, we have the pointwise (although not necessarily uniform) convergence as
![]() $\epsilon \rightarrow 0$
,
$\epsilon \rightarrow 0$
,
Proof. For
![]() $0<r\leq 4$
, we apply the Duhamel principle [Reference Cheeger11, (3.9)] to
$0<r\leq 4$
, we apply the Duhamel principle [Reference Cheeger11, (3.9)] to
![]() $K_{\epsilon , \delta }^q(t, x, y)- K_{0}^q(t, x, y)$
on
$K_{\epsilon , \delta }^q(t, x, y)- K_{0}^q(t, x, y)$
on
![]() $\widetilde {X}_r$
to obtain
$\widetilde {X}_r$
to obtain
 $$ \begin{align*} K_{\epsilon, \delta}^q(t, x, y)- K_{0}^q(t, x, y) & = -\int_0^t\int_{\widetilde{X}_r} \left[ (\partial_t + \square_0^q) K_{\epsilon, \delta}^q(t-s, x, z) \right] \wedge * K_{0}^q(s, z, y) \\ &\quad + \int_0^t\int_{\partial \widetilde{X}_r} K_{\epsilon, \delta}^q(t-s, x, z) \wedge * d K_{0}^q(s, z, y)\\ &\quad + (-1)^{4q+1} \int_0^t\int_{\partial \widetilde{X}_r} * d K_{\epsilon, \delta}^q(t-s, x, z) \wedge K_{0}^q(s, z, y) \\ &\quad + (-1)^{4q+1} \int_0^t\int_{\partial \widetilde{X}_r} * K_{\epsilon, \delta}^q(t-s, x, z) \wedge d^* K_{0}^q(s, z, y) \\ &\quad + \int_0^t\int_{\partial \widetilde{X}_r} d^* K_{\epsilon, \delta}^q(t-s, x, z) \wedge * K_{0}^q(s, z, y). \end{align*} $$
$$ \begin{align*} K_{\epsilon, \delta}^q(t, x, y)- K_{0}^q(t, x, y) & = -\int_0^t\int_{\widetilde{X}_r} \left[ (\partial_t + \square_0^q) K_{\epsilon, \delta}^q(t-s, x, z) \right] \wedge * K_{0}^q(s, z, y) \\ &\quad + \int_0^t\int_{\partial \widetilde{X}_r} K_{\epsilon, \delta}^q(t-s, x, z) \wedge * d K_{0}^q(s, z, y)\\ &\quad + (-1)^{4q+1} \int_0^t\int_{\partial \widetilde{X}_r} * d K_{\epsilon, \delta}^q(t-s, x, z) \wedge K_{0}^q(s, z, y) \\ &\quad + (-1)^{4q+1} \int_0^t\int_{\partial \widetilde{X}_r} * K_{\epsilon, \delta}^q(t-s, x, z) \wedge d^* K_{0}^q(s, z, y) \\ &\quad + \int_0^t\int_{\partial \widetilde{X}_r} d^* K_{\epsilon, \delta}^q(t-s, x, z) \wedge * K_{0}^q(s, z, y). \end{align*} $$
Now fix
![]() $x, y \in \widetilde {X}_{3\delta }$
. First we let
$x, y \in \widetilde {X}_{3\delta }$
. First we let
![]() $r=2\delta $
. Then the first term on the right-hand side goes away, and we are left with only boundary terms. By Theorem 7.3, and noticing that similar estimates hold for the orbifold heat kernel
$r=2\delta $
. Then the first term on the right-hand side goes away, and we are left with only boundary terms. By Theorem 7.3, and noticing that similar estimates hold for the orbifold heat kernel
as well as its derivatives, we deduce then that
To prove the pointwise convergence, we let
![]() $r<2\delta $
and denote
$r<2\delta $
and denote
![]() $\widetilde {V}_{2\delta , r}=\widetilde {V}_{2\delta }-\widetilde {V}_r$
. Then the Duhamel principle becomes
$\widetilde {V}_{2\delta , r}=\widetilde {V}_{2\delta }-\widetilde {V}_r$
. Then the Duhamel principle becomes
 $$ \begin{align*} K_{\epsilon, \delta}^q(t, x, y)- K_{0}^q(t, x, y) & = -\int_0^t\int_{\widetilde{V}_{2\delta}, r} \left[ (\partial_t + \square_0^q) K_{\epsilon, \delta}^q(t-s, x, z) \right] \wedge * K_{0}^q(s, z, y) \\ &\quad + \int_0^t\int_{\partial \widetilde{X}_r} K_{\epsilon, \delta}^q(t-s, x, z) \wedge * d K_{0}^q(s, z, y)\\ &\quad + (-1)^{4q+1} \int_0^t\int_{\partial \widetilde{X}_r} * d K_{\epsilon, \delta}^q(t-s, x, z) \wedge K_{0}^q(s, z, y)\\ &\quad + (-1)^{4q+1} \int_0^t\int_{\partial \widetilde{X}_r} * K_{\epsilon, \delta}^q(t-s, x, z) \wedge d^* K_{0}^q(s, z, y) \\ &\quad + \int_0^t\int_{\partial \widetilde{X}_r} d^* K_{\epsilon, \delta}^q(t-s, x, z) \wedge * K_{0}^q(s, z, y). \end{align*} $$
$$ \begin{align*} K_{\epsilon, \delta}^q(t, x, y)- K_{0}^q(t, x, y) & = -\int_0^t\int_{\widetilde{V}_{2\delta}, r} \left[ (\partial_t + \square_0^q) K_{\epsilon, \delta}^q(t-s, x, z) \right] \wedge * K_{0}^q(s, z, y) \\ &\quad + \int_0^t\int_{\partial \widetilde{X}_r} K_{\epsilon, \delta}^q(t-s, x, z) \wedge * d K_{0}^q(s, z, y)\\ &\quad + (-1)^{4q+1} \int_0^t\int_{\partial \widetilde{X}_r} * d K_{\epsilon, \delta}^q(t-s, x, z) \wedge K_{0}^q(s, z, y)\\ &\quad + (-1)^{4q+1} \int_0^t\int_{\partial \widetilde{X}_r} * K_{\epsilon, \delta}^q(t-s, x, z) \wedge d^* K_{0}^q(s, z, y) \\ &\quad + \int_0^t\int_{\partial \widetilde{X}_r} d^* K_{\epsilon, \delta}^q(t-s, x, z) \wedge * K_{0}^q(s, z, y). \end{align*} $$
We use the relation
![]() $ (\partial _t + \square _0^q) K_{\epsilon ,\delta }^q(t-s, x, z)= (\square _0^q-\square _{\epsilon ,\delta }^q) K_{\epsilon ,\delta }^q(t-s, x, z)$
to control the first integral. Since
$ (\partial _t + \square _0^q) K_{\epsilon ,\delta }^q(t-s, x, z)= (\square _0^q-\square _{\epsilon ,\delta }^q) K_{\epsilon ,\delta }^q(t-s, x, z)$
to control the first integral. Since
![]() $\gamma _{\epsilon , \delta }= i \partial \overline {\partial } \phi _{\epsilon , \delta }$
,
$\gamma _{\epsilon , \delta }= i \partial \overline {\partial } \phi _{\epsilon , \delta }$
,
![]() $\phi _{\epsilon , \delta }(z)=\|z\|^2 + \rho _{\delta }(\| z \|)E(z, \epsilon )$
,
$\phi _{\epsilon , \delta }(z)=\|z\|^2 + \rho _{\delta }(\| z \|)E(z, \epsilon )$
,
![]() $\rho _{\delta }(t)=\rho (t/\delta )$
,
$\rho _{\delta }(t)=\rho (t/\delta )$
,
![]() $\rho (\cdot )\in C^{\infty }({\mathbf R})$
on
$\rho (\cdot )\in C^{\infty }({\mathbf R})$
on
![]() $\widetilde {V}_{2\delta , r}$
and since
$\widetilde {V}_{2\delta , r}$
and since
![]() $E(z,\epsilon )$
is a real analytic function on
$E(z,\epsilon )$
is a real analytic function on
![]() $\widetilde {V}_{3\delta ,r/2}\times [0,1)$
with
$\widetilde {V}_{3\delta ,r/2}\times [0,1)$
with
![]() $E(z,0)=0$
,
$E(z,0)=0$
,
![]() $\frac {1}{\epsilon }(\square _{0}^{q} - \square _{\epsilon ,\delta }^{q})$
is a second order differential operator with coefficients in
$\frac {1}{\epsilon }(\square _{0}^{q} - \square _{\epsilon ,\delta }^{q})$
is a second order differential operator with coefficients in
![]() $C^{\infty }(\widetilde {V}_{3\delta ,r/2}\times [0,\epsilon (\rho )\delta ^{2}))$
. Hence there is a constant
$C^{\infty }(\widetilde {V}_{3\delta ,r/2}\times [0,\epsilon (\rho )\delta ^{2}))$
. Hence there is a constant
![]() $C_{0}(\delta ,r)>0$
depending on
$C_{0}(\delta ,r)>0$
depending on
![]() $\delta $
, r but not on
$\delta $
, r but not on
![]() $\epsilon $
such that for all
$\epsilon $
such that for all
![]() $(z,\epsilon ) \in \widetilde {V}_{3\delta ,r/2}\times [0,\epsilon (\rho )\delta ^{2})$
,
$(z,\epsilon ) \in \widetilde {V}_{3\delta ,r/2}\times [0,\epsilon (\rho )\delta ^{2})$
,
(This can also be deduced from equations (4.2), (4.4) and (4.6).)
By the second statement of Theorem 7.3 applied to the right-hand side, we have, for
![]() $x \in \widetilde {X}_{3\delta }, \ \ z\in \widetilde {V}_{2\delta , r}$
,
$x \in \widetilde {X}_{3\delta }, \ \ z\in \widetilde {V}_{2\delta , r}$
,
for a constant
![]() $C(\delta , r)$
depending on
$C(\delta , r)$
depending on
![]() $\delta , r$
but not on
$\delta , r$
but not on
![]() $\epsilon $
.
$\epsilon $
.
Combining with the uniform estimates in Theorem 7.4, we obtain, for
![]() $x, y \in \widetilde {X}_{3\delta }$
,
$x, y \in \widetilde {X}_{3\delta }$
,
Now for any
![]() $\eta>0$
, we take r sufficiently small so that
$\eta>0$
, we take r sufficiently small so that
![]() $ C^{\prime \prime }(\delta )t e^{-\frac {\delta ^2}{16t}} \mathrm {vol}(\partial \widetilde {X}_r)< \frac {\eta }{2}$
. Then we take
$ C^{\prime \prime }(\delta )t e^{-\frac {\delta ^2}{16t}} \mathrm {vol}(\partial \widetilde {X}_r)< \frac {\eta }{2}$
. Then we take
![]() $\epsilon $
sufficiently small such that
$\epsilon $
sufficiently small such that
![]() $\epsilon t C'(\delta , r) e^{-\frac {\delta ^2}{16t}}< \frac {\eta }{2}$
. Hence
$\epsilon t C'(\delta , r) e^{-\frac {\delta ^2}{16t}}< \frac {\eta }{2}$
. Hence
This proves the pointwise convergence.
Remark 7.5. Since we have the Ricci curvature lower bound, the pointwise convergence of the heat kernels should also be a consequence of some general spectral convergence results due to Cheeger-Colding [Reference Cheeger and Colding12] for the case
![]() $q=0$
, Honda [Reference Honda25] for the case
$q=0$
, Honda [Reference Honda25] for the case
![]() $q=1$
and Bei [Reference Bei2] for
$q=1$
and Bei [Reference Bei2] for
![]() $q=n=2$
. See also [Reference Ding16].
$q=n=2$
. See also [Reference Ding16].
Theorem 7.6. There is a constant C depending only on the Sobolev constant and dimension such that, for
![]() $\delta \leq 1$
,
$\delta \leq 1$
,
Proof. The Duhamel principle [Reference Cheeger11, (3.9)] applied to
![]() $K_{0}^q(t, x, y)- K_{0, \infty }^q(t, x, y)$
on
$K_{0}^q(t, x, y)- K_{0, \infty }^q(t, x, y)$
on
![]() $V_4$
gives us
$V_4$
gives us
 $$ \begin{align*} K_{0}^q(t, x, y)- K_{0, \infty}^q(t, x, y) & = \int_0^t\int_{\partial V_4} K_{0}^q(s, x, z) \wedge * d K_{0, \infty}^q(t-s, z, y) \\ &\quad + (-1)^{4q+1} \int_0^t\int_{\partial V_4} * d K_{0}^q(s, x, z) \wedge K_{0, \infty}^q(t-s, z, y) \\ &\quad + (-1)^{4q+1} \int_0^t\int_{\partial V_4} * K_{0}^q(s, x, z) \wedge d^* K_{0, \infty}^q(t-s, z, y) \\ &\quad + \int_0^t\int_{\partial V_4} d^* K_{0}^q(s, x, z) \wedge * K_{0, \infty}^q(t-s, z, y). \end{align*} $$
$$ \begin{align*} K_{0}^q(t, x, y)- K_{0, \infty}^q(t, x, y) & = \int_0^t\int_{\partial V_4} K_{0}^q(s, x, z) \wedge * d K_{0, \infty}^q(t-s, z, y) \\ &\quad + (-1)^{4q+1} \int_0^t\int_{\partial V_4} * d K_{0}^q(s, x, z) \wedge K_{0, \infty}^q(t-s, z, y) \\ &\quad + (-1)^{4q+1} \int_0^t\int_{\partial V_4} * K_{0}^q(s, x, z) \wedge d^* K_{0, \infty}^q(t-s, z, y) \\ &\quad + \int_0^t\int_{\partial V_4} d^* K_{0}^q(s, x, z) \wedge * K_{0, \infty}^q(t-s, z, y). \end{align*} $$
Thus we obtain, for
![]() $x, y\in V_{3\delta }, \delta \leq 1$
, using the estimate 7.5, except with the
$x, y\in V_{3\delta }, \delta \leq 1$
, using the estimate 7.5, except with the
![]() $\delta $
there replaced by a fixed constant, say
$\delta $
there replaced by a fixed constant, say
![]() $1/4$
, as well as a similar estimate for
$1/4$
, as well as a similar estimate for
![]() $K_{0, \infty }^q(t, x, y) $
,
$K_{0, \infty }^q(t, x, y) $
,
Our final task here is to compare the heat kernel
![]() $K_{\epsilon ,\delta }^{q}(t, x, y)$
with
$K_{\epsilon ,\delta }^{q}(t, x, y)$
with
![]() $K_{\epsilon , \delta , \infty }^q(t, x, y)$
, the heat kernel on
$K_{\epsilon , \delta , \infty }^q(t, x, y)$
, the heat kernel on
![]() $\widetilde {V}_{\infty }$
, when
$\widetilde {V}_{\infty }$
, when
![]() $x, y \in \widetilde {V}_{3\delta }$
.
$x, y \in \widetilde {V}_{3\delta }$
.
Theorem 7.7. There are constants
![]() $A, C$
depending only on the Sobolev constant and dimension such that, for all
$A, C$
depending only on the Sobolev constant and dimension such that, for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $\epsilon \delta ^{-2}\leq \epsilon (\rho )$
and
$\epsilon \delta ^{-2}\leq \epsilon (\rho )$
and
![]() $0\leq q\leq 2$
, we have
$0\leq q\leq 2$
, we have
Proof. The proof follows the same line as above. We apply the Duhamel principle to
![]() $K_{\epsilon ,\delta }^{q}(t, x, y)- K_{\epsilon ,\delta , \infty }^{q}(t, x, y)$
on
$K_{\epsilon ,\delta }^{q}(t, x, y)- K_{\epsilon ,\delta , \infty }^{q}(t, x, y)$
on
![]() $\widetilde {V}_{4}$
and use the heat kernel estimate in Theorem 7.3 as well as the analogous estimate for
$\widetilde {V}_{4}$
and use the heat kernel estimate in Theorem 7.3 as well as the analogous estimate for
![]() $K_{\epsilon ,\delta , \infty }^{q}(t, x, y)$
to obtain the desired estimate.
$K_{\epsilon ,\delta , \infty }^{q}(t, x, y)$
to obtain the desired estimate.
7.3 Partial analytic torsion
Recall that in Section 4.1, for a compact Kähler orbifold
![]() $(Z, \gamma )$
of dimension n,
$(Z, \gamma )$
of dimension n,
 $$ \begin{align*}\zeta_{q}(s)=\sum_{\lambda\in\sigma(\square_{q})\setminus\{0\}}\lambda^{-s}\,\dim E(\lambda;\square_{q}) = \frac{1}{\Gamma(s)} \int_0^{\infty} t^{s-1} \mathrm{Tr}(e^{-t\square_q }P^{\perp}_q) \, dt \end{align*} $$
$$ \begin{align*}\zeta_{q}(s)=\sum_{\lambda\in\sigma(\square_{q})\setminus\{0\}}\lambda^{-s}\,\dim E(\lambda;\square_{q}) = \frac{1}{\Gamma(s)} \int_0^{\infty} t^{s-1} \mathrm{Tr}(e^{-t\square_q }P^{\perp}_q) \, dt \end{align*} $$
with
![]() $P^{\perp }_q$
the orthogonal projection onto the orthogonal complement of
$P^{\perp }_q$
the orthogonal projection onto the orthogonal complement of
![]() $\mathrm {ker}\, \square _q$
and (the logarithm of) the analytic torsion
$\mathrm {ker}\, \square _q$
and (the logarithm of) the analytic torsion
 $$ \begin{align*}\ln \tau(Z,\gamma)=-\sum_{q=0}^{n}(-1)^{q}q\,\zeta^{\prime}_{q}(0)= -\zeta^{\prime}_{T}(0), \end{align*} $$
$$ \begin{align*}\ln \tau(Z,\gamma)=-\sum_{q=0}^{n}(-1)^{q}q\,\zeta^{\prime}_{q}(0)= -\zeta^{\prime}_{T}(0), \end{align*} $$
where
Here
![]() $\square $
denotes the Hodge-Kodaira Laplacian on
$\square $
denotes the Hodge-Kodaira Laplacian on
![]() $A^{0, *}(Z)$
,
$A^{0, *}(Z)$
,
![]() $P^{\perp }$
the orthogonal projection onto the orthogonal compliment of
$P^{\perp }$
the orthogonal projection onto the orthogonal compliment of
![]() $\mathrm {ker}\, \square $
and
$\mathrm {ker}\, \square $
and
![]() $\mathrm {Tr}_s$
the supertrace on
$\mathrm {Tr}_s$
the supertrace on
![]() $A^{0, *}(Z)$
: that is, the alternating sum of the traces on each degree and N the so-called number operator, which simply multiplies a differential form by its degree.
$A^{0, *}(Z)$
: that is, the alternating sum of the traces on each degree and N the so-called number operator, which simply multiplies a differential form by its degree.
By the Lidskii theorem,
 $$ \begin{align*} \mathrm{Tr}_s(Ne^{-t\square}P^{\perp}) & = \int_Z \mathrm{tr}_s(N K(t, x, x)P^{\perp}(x, x)) \, dx \\ & = \sum_{q=0}^{n}(-1)^{q}q\,\int_Z \mathrm{tr}(K_q(t, x, x)P_q^{\perp}(x, x)) \, dx, \end{align*} $$
$$ \begin{align*} \mathrm{Tr}_s(Ne^{-t\square}P^{\perp}) & = \int_Z \mathrm{tr}_s(N K(t, x, x)P^{\perp}(x, x)) \, dx \\ & = \sum_{q=0}^{n}(-1)^{q}q\,\int_Z \mathrm{tr}(K_q(t, x, x)P_q^{\perp}(x, x)) \, dx, \end{align*} $$
where
![]() $K(t, x, y)$
,
$K(t, x, y)$
,
![]() $K_q(t, x, y)$
denotes the heat kernel of
$K_q(t, x, y)$
denotes the heat kernel of
![]() $\square $
,
$\square $
,
![]() $\square _q$
, respectively,
$\square _q$
, respectively,
![]() $P^{\perp }(x, x)$
the Schwartz kernel of
$P^{\perp }(x, x)$
the Schwartz kernel of
![]() $P^{\perp }$
and
$P^{\perp }$
and
![]() $\mathrm {tr}_s$
(abusing notation) also the pointwise supertrace.
$\mathrm {tr}_s$
(abusing notation) also the pointwise supertrace.
At this point, it is convenient to introduce what is called ‘partial analytic torsion’ in [Reference Dai14]. For a domain
![]() $D\subset Z$
, we define
$D\subset Z$
, we define
and
Clearly
Similarly, we can define the equivariant version
![]() $\tau _{\mathbf {Z}_{2}}(D, Z, \gamma )(\theta )$
for
$\tau _{\mathbf {Z}_{2}}(D, Z, \gamma )(\theta )$
for
![]() $\theta $
-invariant domain
$\theta $
-invariant domain
![]() $D\subset Z$
. That is, we define
$D\subset Z$
. That is, we define
and
Then the discussion applies to the equivariant version as well.
7.4 Limit of partial analytic torsion I
Theorem 7.8. For
![]() $0<\delta \leq 1$
, we have
$0<\delta \leq 1$
, we have
and
Proof. (Step 1) Let
 $$ \begin{align*}\mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x)) \sim \sum_{i=0}^{\infty} a_i^{\epsilon, \delta}(x)\, t^{i-2} \end{align*} $$
$$ \begin{align*}\mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x)) \sim \sum_{i=0}^{\infty} a_i^{\epsilon, \delta}(x)\, t^{i-2} \end{align*} $$
be the pointwise small time asymptotic expansion, and write
 $$ \begin{align*} \zeta_{T}^{\widetilde{X}_{3\delta}, \widetilde{X}}(s) & = \frac{1}{\Gamma(s)} \left[ \int_1^{\infty} t^{s-1} \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x)) \, dx \, dt \right. \\ &\quad + \int_0^{1} t^{s-1} \int_{\widetilde{X}_{3\delta}} [ \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x))- \sum_{i=0}^{2} a_i^{\epsilon, \delta}(x)\, t^{i-2} ] \, dx \, dt \\ &\quad + \left. \sum_{i=0}^{1} \int_{\widetilde{X}_{3\delta}} \frac{a_i^{\epsilon, \delta}(x)}{s+ i-2} \, dx + \frac{1}{s} \int_{\widetilde{X}_{3\delta}}[ a_2^{\epsilon, \delta}(x) - \mathrm{tr}_s(N P_{\epsilon, \delta}(x, x))] \, dx \right] , \end{align*} $$
$$ \begin{align*} \zeta_{T}^{\widetilde{X}_{3\delta}, \widetilde{X}}(s) & = \frac{1}{\Gamma(s)} \left[ \int_1^{\infty} t^{s-1} \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x)) \, dx \, dt \right. \\ &\quad + \int_0^{1} t^{s-1} \int_{\widetilde{X}_{3\delta}} [ \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x))- \sum_{i=0}^{2} a_i^{\epsilon, \delta}(x)\, t^{i-2} ] \, dx \, dt \\ &\quad + \left. \sum_{i=0}^{1} \int_{\widetilde{X}_{3\delta}} \frac{a_i^{\epsilon, \delta}(x)}{s+ i-2} \, dx + \frac{1}{s} \int_{\widetilde{X}_{3\delta}}[ a_2^{\epsilon, \delta}(x) - \mathrm{tr}_s(N P_{\epsilon, \delta}(x, x))] \, dx \right] , \end{align*} $$
where
![]() $P_{\epsilon , \delta }(x, x)$
is the Schwartz kernel of
$P_{\epsilon , \delta }(x, x)$
is the Schwartz kernel of
![]() $P_{\epsilon , \delta }$
, the orthogonal projection onto
$P_{\epsilon , \delta }$
, the orthogonal projection onto
![]() $\mathrm {ker}\, \square _{\epsilon , \delta }$
. We obtain
$\mathrm {ker}\, \square _{\epsilon , \delta }$
. We obtain
 $$ \begin{align*} \ln \tau(\widetilde{X}_{3\delta}, \widetilde{X}, \gamma_{\epsilon, \delta}) & = - \int_1^{\infty} t^{-1} \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x)) \, dx \, dt \\ &\quad - \int_0^{1} t^{-1} \int_{\widetilde{X}_{3\delta}}\left[ \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x))- \sum_{i=0}^{2} a_i^{\epsilon, \delta}(x)\, t^{i-2}\right] \, dx \, dt \\ &\quad - \sum_{i=0}^{1} \int_{\widetilde{X}_{3\delta}} \frac{a_i^{\epsilon, \delta}(x)}{ i-2} \, dx + \Gamma'(1) \int_{\widetilde{X}_{3\delta}}\left[ a_2^{\epsilon, \delta}(x) - \mathrm{tr}_s(N P_{\epsilon, \delta}(x, x))\right] \, dx \end{align*} $$
$$ \begin{align*} \ln \tau(\widetilde{X}_{3\delta}, \widetilde{X}, \gamma_{\epsilon, \delta}) & = - \int_1^{\infty} t^{-1} \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x)) \, dx \, dt \\ &\quad - \int_0^{1} t^{-1} \int_{\widetilde{X}_{3\delta}}\left[ \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x))- \sum_{i=0}^{2} a_i^{\epsilon, \delta}(x)\, t^{i-2}\right] \, dx \, dt \\ &\quad - \sum_{i=0}^{1} \int_{\widetilde{X}_{3\delta}} \frac{a_i^{\epsilon, \delta}(x)}{ i-2} \, dx + \Gamma'(1) \int_{\widetilde{X}_{3\delta}}\left[ a_2^{\epsilon, \delta}(x) - \mathrm{tr}_s(N P_{\epsilon, \delta}(x, x))\right] \, dx \end{align*} $$
and similarly for
![]() $\ln \tau (X_{3\delta }, X, \gamma _{0})$
. Since the asymptotic expansion depends only on the local data, we have
$\ln \tau (X_{3\delta }, X, \gamma _{0})$
. Since the asymptotic expansion depends only on the local data, we have
![]() $a_i^{\epsilon , \delta }(x)=a_i^{0}(x)$
on
$a_i^{\epsilon , \delta }(x)=a_i^{0}(x)$
on
![]() $\widetilde {X}_{3\delta }$
. Hence
$\widetilde {X}_{3\delta }$
. Hence
 $$ \begin{align} \begin{aligned} \,& \ln \tau(\widetilde{X}_{3\delta}, \widetilde{X}, \gamma_{\epsilon, \delta}) - \ln \tau(X_{3\delta}, X, \gamma_{0}) \\ &= - \int_1^{\infty} t^{-1} \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s \left[ N K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x) - N K_{0}(t, x, x)P^{\perp}_{0}(x, x) \right] \, dx \, dt \\ &\quad - \int_0^{1} t^{-1} \int_{\widetilde{X}_{3\delta}}\left[ \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x) -N K_{0}(t, x, x)) \right] \, dx \, dt \\ &\quad - \Gamma'(1) \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s(N P_{\epsilon, \delta}(x, x) - N P_{0}(x, x))\, dx. \end{aligned} \end{align} $$
$$ \begin{align} \begin{aligned} \,& \ln \tau(\widetilde{X}_{3\delta}, \widetilde{X}, \gamma_{\epsilon, \delta}) - \ln \tau(X_{3\delta}, X, \gamma_{0}) \\ &= - \int_1^{\infty} t^{-1} \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s \left[ N K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x) - N K_{0}(t, x, x)P^{\perp}_{0}(x, x) \right] \, dx \, dt \\ &\quad - \int_0^{1} t^{-1} \int_{\widetilde{X}_{3\delta}}\left[ \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x) -N K_{0}(t, x, x)) \right] \, dx \, dt \\ &\quad - \Gamma'(1) \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s(N P_{\epsilon, \delta}(x, x) - N P_{0}(x, x))\, dx. \end{aligned} \end{align} $$
We estimate each term on the right-hand side.
(Step 2) Let
![]() $\Psi (\cdot )> 0$
and
$\Psi (\cdot )> 0$
and
![]() $\Lambda (\cdot )>0 $
be as defined in Corollary 6.8. By Corollary 6.8,
$\Lambda (\cdot )>0 $
be as defined in Corollary 6.8. By Corollary 6.8,
 $$ \begin{align*}\begin{aligned} \int_{\widetilde{X}_{3\delta}}\left|\mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x))\right|\,dx &\leq \sum_{q\geq0}q\,(\mathrm{Tr}\,e^{-t\square_{\epsilon,\delta}}-h^{0,q}(\widetilde{X})) \\ &\leq \Psi(\epsilon\delta^{-4}+1)\,\exp[-\frac{1}{2}t\Lambda(\epsilon\delta^{-4}+1)] \end{aligned} \end{align*} $$
$$ \begin{align*}\begin{aligned} \int_{\widetilde{X}_{3\delta}}\left|\mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x))\right|\,dx &\leq \sum_{q\geq0}q\,(\mathrm{Tr}\,e^{-t\square_{\epsilon,\delta}}-h^{0,q}(\widetilde{X})) \\ &\leq \Psi(\epsilon\delta^{-4}+1)\,\exp[-\frac{1}{2}t\Lambda(\epsilon\delta^{-4}+1)] \end{aligned} \end{align*} $$
for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $\epsilon \delta ^{-2}\leq \epsilon (\rho )$
and
$\epsilon \delta ^{-2}\leq \epsilon (\rho )$
and
![]() $t\geq 1$
. Hence for any
$t\geq 1$
. Hence for any
![]() $\nu>0$
, there is
$\nu>0$
, there is
![]() $T'=T'(\nu )>0$
depending only on
$T'=T'(\nu )>0$
depending only on
![]() $\nu $
such that for all
$\nu $
such that for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $\epsilon <\min \{\epsilon (\rho )\delta ^{2},\delta ^{4}\}$
, and
$\epsilon <\min \{\epsilon (\rho )\delta ^{2},\delta ^{4}\}$
, and
![]() $T>T'$
,
$T>T'$
,
 $$ \begin{align} \int_T^{\infty} t^{-1} \int_{\widetilde{X}_{3\delta}} \left|\mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x)) \right|\, dx \, dt \leq \Psi(2)\int_{T}^{\infty}e^{-\Lambda(2) t/2}\frac{dt}{t} < \nu \end{align} $$
$$ \begin{align} \int_T^{\infty} t^{-1} \int_{\widetilde{X}_{3\delta}} \left|\mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x)) \right|\, dx \, dt \leq \Psi(2)\int_{T}^{\infty}e^{-\Lambda(2) t/2}\frac{dt}{t} < \nu \end{align} $$
and similarly for the same term involving
![]() $K_0$
. By Theorem 7.4 and Lebesgue dominated convergence theorem, there exists
$K_0$
. By Theorem 7.4 and Lebesgue dominated convergence theorem, there exists
![]() $\epsilon _0>0$
such that
$\epsilon _0>0$
such that
 $$ \begin{align} \left| \int_1^{T} t^{-1} \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s[N( K_{\epsilon, \delta}(t, x, x)) - K_{0}(t, x, x))] \, dx \, dt \right| < \nu \end{align} $$
$$ \begin{align} \left| \int_1^{T} t^{-1} \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s[N( K_{\epsilon, \delta}(t, x, x)) - K_{0}(t, x, x))] \, dx \, dt \right| < \nu \end{align} $$
whenever
![]() $\epsilon < \epsilon _0$
. Similarly,
$\epsilon < \epsilon _0$
. Similarly,
 $$ \begin{align} \left| \int_0^{1} t^{-1} \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s[N( K_{\epsilon, \delta}(t, x, x)) - K_{0}(t, x, x))] \, dx \, dt \right| < \nu \end{align} $$
$$ \begin{align} \left| \int_0^{1} t^{-1} \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s[N( K_{\epsilon, \delta}(t, x, x)) - K_{0}(t, x, x))] \, dx \, dt \right| < \nu \end{align} $$
whenever
![]() $\epsilon < \epsilon _0$
.
$\epsilon < \epsilon _0$
.
On the other hand,
and similarly for
![]() $K_0$
. Recall
$K_0$
. Recall
![]() $\ker \square _{0}=\ker \square _{\epsilon ,\delta }=\mathbf {C}\cdot 1\oplus \mathbf {C}\cdot \bar {\eta }$
. For
$\ker \square _{0}=\ker \square _{\epsilon ,\delta }=\mathbf {C}\cdot 1\oplus \mathbf {C}\cdot \bar {\eta }$
. For
![]() $x\in \widetilde {X}_{3\delta }$
, we get
$x\in \widetilde {X}_{3\delta }$
, we get
 $$ \begin{align} \mathrm{tr}_{s}[N(P_{\epsilon,\delta}(x,x)-P_{0}(x,x))] = \frac{2}{\|\eta\|_{L^{2}}^{2}} \left( \frac{\eta\wedge\bar{\eta}}{\gamma_{\epsilon,\delta}^{2}/2!}(x)-\frac{\eta\wedge\bar{\eta}}{\gamma_{0}^{2}/2!}(x) \right) =0, \end{align} $$
$$ \begin{align} \mathrm{tr}_{s}[N(P_{\epsilon,\delta}(x,x)-P_{0}(x,x))] = \frac{2}{\|\eta\|_{L^{2}}^{2}} \left( \frac{\eta\wedge\bar{\eta}}{\gamma_{\epsilon,\delta}^{2}/2!}(x)-\frac{\eta\wedge\bar{\eta}}{\gamma_{0}^{2}/2!}(x) \right) =0, \end{align} $$
because
![]() $\gamma _{\epsilon ,\delta }=\gamma _{0}$
on
$\gamma _{\epsilon ,\delta }=\gamma _{0}$
on
![]() $\widetilde {X}_{3\delta }$
. It follows from equations (7.11), (7.13) and (7.14) that
$\widetilde {X}_{3\delta }$
. It follows from equations (7.11), (7.13) and (7.14) that
 $$ \begin{align} \left| \int_1^{T} t^{-1} \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s(N[ K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x)-K_0(t, x, x)P^{\perp}_0(x, x)]) \, dx \, dt \right| < \nu. \end{align} $$
$$ \begin{align} \left| \int_1^{T} t^{-1} \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s(N[ K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x)-K_0(t, x, x)P^{\perp}_0(x, x)]) \, dx \, dt \right| < \nu. \end{align} $$
Substituting equations (7.10), (7.11), (7.12), (7.14) and (7.15) into equation (7.9), we get
whenever
![]() $\epsilon < \epsilon _0$
. Since
$\epsilon < \epsilon _0$
. Since
![]() $\nu>0$
can be chosen arbitrarily small, this finishes the proof of the first formula.
$\nu>0$
can be chosen arbitrarily small, this finishes the proof of the first formula.
To prove the result about the equivariant torsion, we follow the same line of argument, except with a simplification, since
![]() $\theta $
has no fixed points in
$\theta $
has no fixed points in
![]() $\widetilde {X}_{3\delta }$
. Indeed,
$\widetilde {X}_{3\delta }$
. Indeed,
 $$ \begin{align*} \ln \tau_{\mathbf{Z}_{2}}(\widetilde{X}_{3\delta}, \widetilde{X}, \gamma_{\epsilon, \delta})(\theta) & = - \int_1^{\infty} t^{-1} \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, \theta x)P^{\perp}_{\epsilon, \delta}(x, \theta x)) \, dx \, dt \\ &\quad - \int_0^{1} t^{-1} \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, \theta x))\, dx \, dt \\ &\quad - \Gamma'(1) \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s(N P_{\epsilon, \delta}(x, \theta x)\, dx \end{align*} $$
$$ \begin{align*} \ln \tau_{\mathbf{Z}_{2}}(\widetilde{X}_{3\delta}, \widetilde{X}, \gamma_{\epsilon, \delta})(\theta) & = - \int_1^{\infty} t^{-1} \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, \theta x)P^{\perp}_{\epsilon, \delta}(x, \theta x)) \, dx \, dt \\ &\quad - \int_0^{1} t^{-1} \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, \theta x))\, dx \, dt \\ &\quad - \Gamma'(1) \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s(N P_{\epsilon, \delta}(x, \theta x)\, dx \end{align*} $$
and
 $$ \begin{align} \begin{aligned} \,& \ln \tau_{\mathbf{Z}_{2}}(\widetilde{X}_{3\delta}, \widetilde{X}, \gamma_{\epsilon, \delta})(\theta) -\ln \tau_{\mathbf{Z}_{2}}(X_{3\delta}, X, \gamma_{0})(\iota) \\ &= - \int_1^{\infty} t^{-1} \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s \left[ N K_{\epsilon, \delta}(t, x, \theta x)P^{\perp}_{\epsilon, \delta}(x, \theta x) - N K_{0}(t, x, \theta x)P^{\perp}_{0}(x,\theta x) \right] \, dx \, dt \\ &\quad - \int_0^{1} t^{-1} \int_{\widetilde{X}_{3\delta}}\left[ \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x,\theta x) -N K_{0}(t, x,\theta x)) \right] \, dx \, dt \\ &\quad - \Gamma'(1) \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s(N P_{\epsilon, \delta}(x, \theta x) - N P_{0}(x,\theta x))\, dx. \end{aligned} \end{align} $$
$$ \begin{align} \begin{aligned} \,& \ln \tau_{\mathbf{Z}_{2}}(\widetilde{X}_{3\delta}, \widetilde{X}, \gamma_{\epsilon, \delta})(\theta) -\ln \tau_{\mathbf{Z}_{2}}(X_{3\delta}, X, \gamma_{0})(\iota) \\ &= - \int_1^{\infty} t^{-1} \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s \left[ N K_{\epsilon, \delta}(t, x, \theta x)P^{\perp}_{\epsilon, \delta}(x, \theta x) - N K_{0}(t, x, \theta x)P^{\perp}_{0}(x,\theta x) \right] \, dx \, dt \\ &\quad - \int_0^{1} t^{-1} \int_{\widetilde{X}_{3\delta}}\left[ \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x,\theta x) -N K_{0}(t, x,\theta x)) \right] \, dx \, dt \\ &\quad - \Gamma'(1) \int_{\widetilde{X}_{3\delta}} \mathrm{tr}_s(N P_{\epsilon, \delta}(x, \theta x) - N P_{0}(x,\theta x))\, dx. \end{aligned} \end{align} $$
Now we proceed as before.
7.5 Limit of partial analytic torsion II
To relate
![]() $\ln \tau (X_{3\delta }, X, \gamma _{0})$
to
$\ln \tau (X_{3\delta }, X, \gamma _{0})$
to
![]() $\ln \tau (X, \gamma _{0})$
, by (7.7), it suffices to show
$\ln \tau (X, \gamma _{0})$
, by (7.7), it suffices to show
Theorem 7.9. We have
Remark 7.10. This is closely related to [Reference Dai and Yu15], where analytic torsions on orbifolds defined from conical singularity pointview are shown to be the same as the ones defined from orbifold singularity pointview.
Proof. Again, the proofs for both formulas work the same, so we only present the first one. Moreover, the argument works for any orbifold singularity, but we will work with the cyclic quotient singularity of type
![]() $\frac {1}{4}(1,1)$
in our situation. First of all, by the same kind of argument as above, using Theorem 7.6 and
$\frac {1}{4}(1,1)$
in our situation. First of all, by the same kind of argument as above, using Theorem 7.6 and
![]() $\mathrm {vol}(V_{3\delta }) \rightarrow 0$
as
$\mathrm {vol}(V_{3\delta }) \rightarrow 0$
as
![]() $\delta \rightarrow 0$
, one has
$\delta \rightarrow 0$
, one has
Now the right-hand side can be explicitly computed since the heat kernel is explicitly known. Indeed, as
![]() $V_{\infty }$
is just k copies of
$V_{\infty }$
is just k copies of
![]() $\mathbf C^2/\mathbf Z_2$
, the (orbifold) heat kernel of
$\mathbf C^2/\mathbf Z_2$
, the (orbifold) heat kernel of
![]() $( V_{\infty }, \kappa _0)$
on the
$( V_{\infty }, \kappa _0)$
on the
![]() $(0,q)$
forms is
$(0,q)$
forms is
![]() $k\binom {n}{q}$
(
$k\binom {n}{q}$
(
![]() $n=2$
in our case) copies of
$n=2$
in our case) copies of
 $$ \begin{align*}K_0(t, x,x')= \frac{1}{(4\pi t)^{n/2}}\left( e^{-\frac{|x-x'|^2}{4t}} + e^{-\frac{|x+x'|^2}{4t}} \right). \end{align*} $$
$$ \begin{align*}K_0(t, x,x')= \frac{1}{(4\pi t)^{n/2}}\left( e^{-\frac{|x-x'|^2}{4t}} + e^{-\frac{|x+x'|^2}{4t}} \right). \end{align*} $$
In terms of the polar coordinates
![]() $x=(r, y), y\in S^{2n-1}$
,
$x=(r, y), y\in S^{2n-1}$
,
Thus
 $$ \begin{align*}\int_{V_{3\delta}} K_0(t, x, x) dx = c_n \delta^n t^{-n/2} + d_n \int_0^{\frac{3\delta}{t^{1/2}}} \xi^{n-1}e^{-\xi^2} d\xi, \end{align*} $$
$$ \begin{align*}\int_{V_{3\delta}} K_0(t, x, x) dx = c_n \delta^n t^{-n/2} + d_n \int_0^{\frac{3\delta}{t^{1/2}}} \xi^{n-1}e^{-\xi^2} d\xi, \end{align*} $$
where
![]() $c_n=\frac {3^n\omega _n}{n(4\pi )^{n/2}}$
,
$c_n=\frac {3^n\omega _n}{n(4\pi )^{n/2}}$
,
![]() $d_n=\frac {\omega _n}{(4\pi )^{n/2}}$
,
$d_n=\frac {\omega _n}{(4\pi )^{n/2}}$
,
![]() $\omega _n=\mathrm {vol}(S^{n-1})$
.
$\omega _n=\mathrm {vol}(S^{n-1})$
.
The second term has different asymptotic behaviours for
![]() $t\rightarrow 0$
and
$t\rightarrow 0$
and
![]() $t\rightarrow \infty $
. Since
$t\rightarrow \infty $
. Since
 $$ \begin{align*}\int_0^{\frac{3\delta}{t^{1/2}}} \xi^{n-1}e^{-\xi^2} d\xi= \int_0^{\infty} \xi^{n-1}e^{-\xi^2} d\xi -\int^\infty_{\frac{3\delta}{t^{1/2}}} \xi^{n-1}e^{-\xi^2} d\xi, \end{align*} $$
$$ \begin{align*}\int_0^{\frac{3\delta}{t^{1/2}}} \xi^{n-1}e^{-\xi^2} d\xi= \int_0^{\infty} \xi^{n-1}e^{-\xi^2} d\xi -\int^\infty_{\frac{3\delta}{t^{1/2}}} \xi^{n-1}e^{-\xi^2} d\xi, \end{align*} $$
by some elementary inequality, it is a constant
![]() $d_n'=d_n \int _0^{\infty } \xi ^{n-1}e^{-\xi ^2} d\xi $
plus an exponentially decaying term as
$d_n'=d_n \int _0^{\infty } \xi ^{n-1}e^{-\xi ^2} d\xi $
plus an exponentially decaying term as
![]() $t\rightarrow 0$
(or one could just invoke the known asymptotic for the complementary error function for a large argument). On the other hand, it is also straightforward to see that as
$t\rightarrow 0$
(or one could just invoke the known asymptotic for the complementary error function for a large argument). On the other hand, it is also straightforward to see that as
![]() $t \rightarrow \infty $
, the second term is
$t \rightarrow \infty $
, the second term is
![]() $O(t^{-\frac {n-1}{2}})$
.
$O(t^{-\frac {n-1}{2}})$
.
Set
 $$ \begin{align*} \zeta_\delta(s) & = \frac{1}{\Gamma(s)}\int_0^\infty t^{s-1} \left(\int_{V_{3\delta}} K_0(t, x, x) dx\right) dt \\ & = \frac{1}{\Gamma(s)}\left[ \int_0^1 t^{s-1} \left(\int_{V_{3\delta}} K_0(t, x, x) dx\right) dt + \int_1^\infty t^{s-1} \left(\int_{V_{3\delta}} K_0(t, x, x) dx\right) dt \right], \end{align*} $$
$$ \begin{align*} \zeta_\delta(s) & = \frac{1}{\Gamma(s)}\int_0^\infty t^{s-1} \left(\int_{V_{3\delta}} K_0(t, x, x) dx\right) dt \\ & = \frac{1}{\Gamma(s)}\left[ \int_0^1 t^{s-1} \left(\int_{V_{3\delta}} K_0(t, x, x) dx\right) dt + \int_1^\infty t^{s-1} \left(\int_{V_{3\delta}} K_0(t, x, x) dx\right) dt \right], \end{align*} $$
where the first term is defined through analytic continuation from a region where the real part of s is sufficiently large, whereas the second term is defined through analytic continuation from a region where the real part of s is sufficiently negative. Therefore
 $$ \begin{align*} \frac{1}{\Gamma(s)}\int_0^1 t^{s-1} \left(\int_{V_{3\delta}} K_0(t, x, x) dx\right) dt & = \frac{1}{\Gamma(s)} \frac{c_n\delta^n}{s-n/2} + \frac{d_n'}{\Gamma(s+1)} \\ &\quad - \frac{d_n}{\Gamma(s)}\int_0^1 t^{s-1}\int^\infty_{\frac{3\delta}{t^{1/2}}} \xi^{n-1}e^{-\xi^2} d\xi dt , \end{align*} $$
$$ \begin{align*} \frac{1}{\Gamma(s)}\int_0^1 t^{s-1} \left(\int_{V_{3\delta}} K_0(t, x, x) dx\right) dt & = \frac{1}{\Gamma(s)} \frac{c_n\delta^n}{s-n/2} + \frac{d_n'}{\Gamma(s+1)} \\ &\quad - \frac{d_n}{\Gamma(s)}\int_0^1 t^{s-1}\int^\infty_{\frac{3\delta}{t^{1/2}}} \xi^{n-1}e^{-\xi^2} d\xi dt , \end{align*} $$
and
 $$ \begin{align*} \frac{1}{\Gamma(s)}\int_1^\infty t^{s-1} \left(\int_{V_{3\delta}} K_0(t, x, x) dx\right) dt & = - \frac{1}{\Gamma(s)} \frac{c_n\delta^n}{s-n/2} \\ &\quad + \frac{d_n}{\Gamma(s)}\int_0^1 t^{s-1}\int_0^{\frac{3\delta}{t^{1/2}}} \xi^{n-1}e^{-\xi^2} d\xi dt. \end{align*} $$
$$ \begin{align*} \frac{1}{\Gamma(s)}\int_1^\infty t^{s-1} \left(\int_{V_{3\delta}} K_0(t, x, x) dx\right) dt & = - \frac{1}{\Gamma(s)} \frac{c_n\delta^n}{s-n/2} \\ &\quad + \frac{d_n}{\Gamma(s)}\int_0^1 t^{s-1}\int_0^{\frac{3\delta}{t^{1/2}}} \xi^{n-1}e^{-\xi^2} d\xi dt. \end{align*} $$
Thus,
 $$ \begin{align*}\zeta_\delta'(0) = -d_n'\Gamma'(1) -d_n \int_0^1 t^{-1}\int^\infty_{\frac{3\delta}{t^{1/2}}}\xi^{n-1}e^{-\xi^2} d\xi dt + d_n \int_0^1 t^{-1}\int^{\frac{3\delta}{t^{1/2}}}_0 \xi^{n-1}e^{-\xi^2} d\xi dt. \end{align*} $$
$$ \begin{align*}\zeta_\delta'(0) = -d_n'\Gamma'(1) -d_n \int_0^1 t^{-1}\int^\infty_{\frac{3\delta}{t^{1/2}}}\xi^{n-1}e^{-\xi^2} d\xi dt + d_n \int_0^1 t^{-1}\int^{\frac{3\delta}{t^{1/2}}}_0 \xi^{n-1}e^{-\xi^2} d\xi dt. \end{align*} $$
By a simple change of integration, we arrive at
 $$ \begin{align*}\zeta_\delta'(0)= -d_n'\Gamma'(1) -d_n \int_{3\delta}^\infty 2\ln \frac{3\delta}{\xi} \xi^{n-1}e^{-\xi^2} d\xi + d_n \int_0^{3\delta} 2\ln \frac{3\delta}{\xi} \xi^{n-1}e^{-\xi^2} d\xi. \end{align*} $$
$$ \begin{align*}\zeta_\delta'(0)= -d_n'\Gamma'(1) -d_n \int_{3\delta}^\infty 2\ln \frac{3\delta}{\xi} \xi^{n-1}e^{-\xi^2} d\xi + d_n \int_0^{3\delta} 2\ln \frac{3\delta}{\xi} \xi^{n-1}e^{-\xi^2} d\xi. \end{align*} $$
This has a logarithmic divergence (
![]() $2d_n' \ln 3\delta $
) as
$2d_n' \ln 3\delta $
) as
![]() $\delta \rightarrow 0$
, but
$\delta \rightarrow 0$
, but
 $$ \begin{align*}\ln \tau(V_{3\delta}, V_{\infty}, \gamma_0)= - k\zeta_\delta'(0)\sum_{q=0}^n (-1)^q q \binom{n}{q} =0 \end{align*} $$
$$ \begin{align*}\ln \tau(V_{3\delta}, V_{\infty}, \gamma_0)= - k\zeta_\delta'(0)\sum_{q=0}^n (-1)^q q \binom{n}{q} =0 \end{align*} $$
by combinatorial formula since
![]() $n\geq 2$
(in fact equal to
$n\geq 2$
(in fact equal to
![]() $2$
in this case). The proof for the partial equivariant torsion is almost the same. We just need to insert the action of the involution
$2$
in this case). The proof for the partial equivariant torsion is almost the same. We just need to insert the action of the involution
![]() $\theta $
into the heat kernel, which will result in only the
$\theta $
into the heat kernel, which will result in only the
![]() $d_n$
terms similar to the above formulas.
$d_n$
terms similar to the above formulas.
Corollary 7.11. We have
Proof. Since
![]() $\ln \tau (X, \gamma _0)=\ln \tau (X_{3\delta },X, \gamma _0)+\ln \tau (V_{3\delta },X,\gamma _0)$
and
$\ln \tau (X, \gamma _0)=\ln \tau (X_{3\delta },X, \gamma _0)+\ln \tau (V_{3\delta },X,\gamma _0)$
and
![]() $\ln \tau _{\mathbf {Z}_{2}}(X, \gamma _0)(\iota )=\ln \tau _{\mathbf {Z}_{2}}(X_{3\delta },X, \gamma _0)(\iota )+\ln \tau _{\mathbf {Z}_{2}}(V_{3\delta },X,\gamma _0)(\iota )$
by equation (7.7), we get by Theorem 7.9
$\ln \tau _{\mathbf {Z}_{2}}(X, \gamma _0)(\iota )=\ln \tau _{\mathbf {Z}_{2}}(X_{3\delta },X, \gamma _0)(\iota )+\ln \tau _{\mathbf {Z}_{2}}(V_{3\delta },X,\gamma _0)(\iota )$
by equation (7.7), we get by Theorem 7.9
which, together with Theorem 7.8, yields the result.
7.6 Limit of partial analytic torsion III
On the other hand, we have
Theorem 7.12. The following equalities hold:
where the constants
![]() $C_0^{\mathrm {EH}}(\rho )$
,
$C_0^{\mathrm {EH}}(\rho )$
,
![]() $C_1^{\mathrm {EH}}(\rho )$
depend only on the cut-off function
$C_1^{\mathrm {EH}}(\rho )$
depend only on the cut-off function
![]() $\rho $
.
$\rho $
.
At this stage, the constants
![]() $C_0^{\mathrm {EH}}(\rho )$
,
$C_0^{\mathrm {EH}}(\rho )$
,
![]() $C_1^{\mathrm {EH}}(\rho )$
may depend on
$C_1^{\mathrm {EH}}(\rho )$
may depend on
![]() $\rho $
. The fact that they are independent of
$\rho $
. The fact that they are independent of
![]() $\rho $
will be postponed to the next subsection.
$\rho $
will be postponed to the next subsection.
7.6.1 An integral expression of
 $\tau (\widetilde {V}_{3\delta }, \widetilde {X}, \gamma _{\epsilon , \delta })$
and
$\tau (\widetilde {V}_{3\delta }, \widetilde {X}, \gamma _{\epsilon , \delta })$
and
 $\tau _{\mathbf {Z}_{2}}(\widetilde {V}_{3\delta }, \widetilde {X}, \gamma _{\epsilon , \delta })(\theta )$
$\tau _{\mathbf {Z}_{2}}(\widetilde {V}_{3\delta }, \widetilde {X}, \gamma _{\epsilon , \delta })(\theta )$
For the proof of Theorem 7.12, as before, we compute
 $$ \begin{align*} \ln \tau(\widetilde{V}_{3\delta}, \widetilde{X}, \gamma_{\epsilon, \delta}) & = - \int_1^{\infty} t^{-1} \int_{\widetilde{V}_{3\delta}} \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x)) \, dx \, dt \\ &\quad - \int_0^{1} t^{-1} \int_{\widetilde{V}_{3\delta}}\left[ \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x))- \sum_{i=0}^{2} a_i^{\epsilon, \delta}(x)\, t^{i-2}\right] \, dx \, dt \\ &\quad - \sum_{i=0}^{1} \int_{\widetilde{V}_{3\delta}} \frac{a_i^{\epsilon, \delta}(x)}{ i-2} dx + \Gamma'(1) \int_{\widetilde{V}_{3\delta}}\left[ a_2^{\epsilon, \delta}(x) - \mathrm{tr}_s(N P_{\epsilon, \delta}(x, x))\right] dx, \end{align*} $$
$$ \begin{align*} \ln \tau(\widetilde{V}_{3\delta}, \widetilde{X}, \gamma_{\epsilon, \delta}) & = - \int_1^{\infty} t^{-1} \int_{\widetilde{V}_{3\delta}} \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x)) \, dx \, dt \\ &\quad - \int_0^{1} t^{-1} \int_{\widetilde{V}_{3\delta}}\left[ \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x))- \sum_{i=0}^{2} a_i^{\epsilon, \delta}(x)\, t^{i-2}\right] \, dx \, dt \\ &\quad - \sum_{i=0}^{1} \int_{\widetilde{V}_{3\delta}} \frac{a_i^{\epsilon, \delta}(x)}{ i-2} dx + \Gamma'(1) \int_{\widetilde{V}_{3\delta}}\left[ a_2^{\epsilon, \delta}(x) - \mathrm{tr}_s(N P_{\epsilon, \delta}(x, x))\right] dx, \end{align*} $$
where
![]() $a_i^{\epsilon , \delta }(x)$
are the coefficients of the pointwise small time asymptotic expansion for
$a_i^{\epsilon , \delta }(x)$
are the coefficients of the pointwise small time asymptotic expansion for
![]() $\mathrm {tr}_s(N K_{\epsilon , \delta }(t, x, x))$
described in the proof of Theorem 7.8. Similarly,
$\mathrm {tr}_s(N K_{\epsilon , \delta }(t, x, x))$
described in the proof of Theorem 7.8. Similarly,
 $$ \begin{align*} \ln \tau_{\mathbf{Z}_{2}}(\widetilde{V}_{3\delta}, \widetilde{X}, \gamma_{\epsilon, \delta}) & = - \int_1^{\infty} t^{-1} \int_{\widetilde{V}_{3\delta}} \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, \theta(x))P^{\perp}_{\epsilon, \delta}(x, \theta(x))) \, dx \, dt \\ &\quad - \int_0^{1} \frac{dt}{t} \left[ \int_{\widetilde{V}_{3\delta}}\mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, \theta(x)))\,dx - \sum_{i=0}^{1}t^{i-1} \int_{E}b_i^{\epsilon, \delta}(z)\, dz\right] \\ &\quad + \int_{E} b_0^{\epsilon, \delta}(z) \, dz + \Gamma'(1) \left[ \int_{E}b_1^{\epsilon, \delta}(z)\,dz - \int_{\widetilde{V}_{3\delta}} \mathrm{tr}_s(N P_{\epsilon, \delta}(x, \theta(x)))\, dx\right] \end{align*} $$
$$ \begin{align*} \ln \tau_{\mathbf{Z}_{2}}(\widetilde{V}_{3\delta}, \widetilde{X}, \gamma_{\epsilon, \delta}) & = - \int_1^{\infty} t^{-1} \int_{\widetilde{V}_{3\delta}} \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, \theta(x))P^{\perp}_{\epsilon, \delta}(x, \theta(x))) \, dx \, dt \\ &\quad - \int_0^{1} \frac{dt}{t} \left[ \int_{\widetilde{V}_{3\delta}}\mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, \theta(x)))\,dx - \sum_{i=0}^{1}t^{i-1} \int_{E}b_i^{\epsilon, \delta}(z)\, dz\right] \\ &\quad + \int_{E} b_0^{\epsilon, \delta}(z) \, dz + \Gamma'(1) \left[ \int_{E}b_1^{\epsilon, \delta}(z)\,dz - \int_{\widetilde{V}_{3\delta}} \mathrm{tr}_s(N P_{\epsilon, \delta}(x, \theta(x)))\, dx\right] \end{align*} $$
with
![]() $b_i^{\epsilon , \delta }(x)$
the coefficients of the pointwise small time asymptotic expansion for
$b_i^{\epsilon , \delta }(x)$
the coefficients of the pointwise small time asymptotic expansion for
![]() $\mathrm {tr}_s(N K_{\epsilon , \delta }(t, x, \theta (x)))$
. We study the behaviour of each term on the right-hand side as
$\mathrm {tr}_s(N K_{\epsilon , \delta }(t, x, \theta (x)))$
. We study the behaviour of each term on the right-hand side as
![]() $\epsilon \to 0$
and
$\epsilon \to 0$
and
![]() $\delta \to 0$
. For this, we set
$\delta \to 0$
. For this, we set
 $$ \begin{align*}\begin{aligned} I(\epsilon,\delta;\rho) &:= - \int_{0}^{1}\frac{dt}{t}\int_{\widetilde{V}(3\delta)}\left[ \mathrm{tr}_{s}(N K_{\epsilon, \delta,\infty}(t, x, x))- \sum_{i=0}^{2} a_{i}^{\epsilon, \delta}(x)\, t^{i-2}\right] \, dx \\ &\qquad - \sum_{i=0}^{1} \int_{\widetilde{V}(3\delta)} \frac{a_{i}^{\epsilon, \delta}(x)}{ i-2} \, dx + \Gamma'(1) \int_{\widetilde{V}(3\delta)}a_{2}^{\epsilon, \delta}(x) \, dx, \end{aligned} \end{align*} $$
$$ \begin{align*}\begin{aligned} I(\epsilon,\delta;\rho) &:= - \int_{0}^{1}\frac{dt}{t}\int_{\widetilde{V}(3\delta)}\left[ \mathrm{tr}_{s}(N K_{\epsilon, \delta,\infty}(t, x, x))- \sum_{i=0}^{2} a_{i}^{\epsilon, \delta}(x)\, t^{i-2}\right] \, dx \\ &\qquad - \sum_{i=0}^{1} \int_{\widetilde{V}(3\delta)} \frac{a_{i}^{\epsilon, \delta}(x)}{ i-2} \, dx + \Gamma'(1) \int_{\widetilde{V}(3\delta)}a_{2}^{\epsilon, \delta}(x) \, dx, \end{aligned} \end{align*} $$
 $$ \begin{align*}\begin{aligned} J(\epsilon,\delta;\rho) &:= - \int_{0}^{1}\frac{dt}{t} \left[ \int_{\widetilde{V}(3\delta)}\mathrm{tr}_{s}(N K_{\epsilon, \delta,\infty}(t, x, \theta(x)))\, dx - \sum_{i=0}^{1}t^{i-1}\int_{E}b_{i}^{\epsilon, \delta}(z)\,dz \right] \\ &\qquad + \int_{E} b_{0}^{\epsilon, \delta}(z)\, dz + \Gamma'(1) \int_{E}b_{1}^{\epsilon, \delta}(z) \, dz. \end{aligned} \end{align*} $$
$$ \begin{align*}\begin{aligned} J(\epsilon,\delta;\rho) &:= - \int_{0}^{1}\frac{dt}{t} \left[ \int_{\widetilde{V}(3\delta)}\mathrm{tr}_{s}(N K_{\epsilon, \delta,\infty}(t, x, \theta(x)))\, dx - \sum_{i=0}^{1}t^{i-1}\int_{E}b_{i}^{\epsilon, \delta}(z)\,dz \right] \\ &\qquad + \int_{E} b_{0}^{\epsilon, \delta}(z)\, dz + \Gamma'(1) \int_{E}b_{1}^{\epsilon, \delta}(z) \, dz. \end{aligned} \end{align*} $$
Since
![]() $K_{\epsilon , \delta ,\infty }(t, x, y)=\oplus _{q}K_{\epsilon , \delta ,\infty }^{q}(t, x, y)$
is
$K_{\epsilon , \delta ,\infty }(t, x, y)=\oplus _{q}K_{\epsilon , \delta ,\infty }^{q}(t, x, y)$
is
![]() $k$
-copies of the heat kernel of
$k$
-copies of the heat kernel of
![]() $(T^{*}\mathbf {P}^{1},\kappa _{\epsilon ,\delta })$
,
$(T^{*}\mathbf {P}^{1},\kappa _{\epsilon ,\delta })$
,
![]() $I(\epsilon ,\delta ;\rho )$
and
$I(\epsilon ,\delta ;\rho )$
and
![]() $J(\epsilon ,\delta ;\rho )$
depend only on
$J(\epsilon ,\delta ;\rho )$
depend only on
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $\epsilon \delta ^{-2}\leq \epsilon (\rho )$
and the cut-off function
$\epsilon \delta ^{-2}\leq \epsilon (\rho )$
and the cut-off function
![]() $\rho $
. Since
$\rho $
. Since
![]() $\widetilde {V}_{3\delta }$
is k-copies of
$\widetilde {V}_{3\delta }$
is k-copies of
![]() $\widetilde {V}(3\delta )$
, we have
$\widetilde {V}(3\delta )$
, we have
 $$ \begin{align*}\begin{aligned} \ln \tau(\widetilde{V}_{3\delta}, \widetilde{X}, \gamma_{\epsilon, \delta}) &= -\int_1^{\infty} \frac{dt}{t} \int_{\widetilde{V}_{3\delta}} \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x)) \, dx \\ &\quad -\int_{0}^{1}\frac{dt}{t}\int_{\widetilde{V}_{3\delta}}\mathrm{tr}_{s}\{N K_{\epsilon,\delta}(t,x,x)-N K_{\epsilon,\delta,\infty}(t,x,x)\}dx \\ &\quad -\Gamma'(1)\int_{\widetilde{V}_{3\delta}}\mathrm{tr}_{s}(N P_{\epsilon,\delta}(x,x))\,dx+k\cdot I(\epsilon,\delta;\rho) \end{aligned} \end{align*} $$
$$ \begin{align*}\begin{aligned} \ln \tau(\widetilde{V}_{3\delta}, \widetilde{X}, \gamma_{\epsilon, \delta}) &= -\int_1^{\infty} \frac{dt}{t} \int_{\widetilde{V}_{3\delta}} \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x)) \, dx \\ &\quad -\int_{0}^{1}\frac{dt}{t}\int_{\widetilde{V}_{3\delta}}\mathrm{tr}_{s}\{N K_{\epsilon,\delta}(t,x,x)-N K_{\epsilon,\delta,\infty}(t,x,x)\}dx \\ &\quad -\Gamma'(1)\int_{\widetilde{V}_{3\delta}}\mathrm{tr}_{s}(N P_{\epsilon,\delta}(x,x))\,dx+k\cdot I(\epsilon,\delta;\rho) \end{aligned} \end{align*} $$
and similarly
 $$ \begin{align*}\begin{aligned} \ln \tau_{\mathbf{Z}_{2}}(\widetilde{V}_{3\delta}, \widetilde{X}, \gamma_{\epsilon, \delta}) &= -\int_1^{\infty} \frac{dt}{t} \int_{\widetilde{V}_{3\delta}} \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, \theta(x))P^{\perp}_{\epsilon, \delta}(x, \theta(x))) \, dx \\ &\quad -\int_{0}^{1}\frac{dt}{t}\int_{\widetilde{V}_{3\delta}}\mathrm{tr}_{s}\{N K_{\epsilon,\delta}(t,x,\theta(x))-N K_{\epsilon,\delta,\infty}(t,x,\theta(x))\}dx \\ &\quad -\Gamma'(1)\int_{\widetilde{V}_{3\delta}}\mathrm{tr}_{s}\{N P_{\epsilon,\delta}(x,\theta(x))\}\,dx+k\cdot J(\epsilon,\delta;\rho). \end{aligned} \end{align*} $$
$$ \begin{align*}\begin{aligned} \ln \tau_{\mathbf{Z}_{2}}(\widetilde{V}_{3\delta}, \widetilde{X}, \gamma_{\epsilon, \delta}) &= -\int_1^{\infty} \frac{dt}{t} \int_{\widetilde{V}_{3\delta}} \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, \theta(x))P^{\perp}_{\epsilon, \delta}(x, \theta(x))) \, dx \\ &\quad -\int_{0}^{1}\frac{dt}{t}\int_{\widetilde{V}_{3\delta}}\mathrm{tr}_{s}\{N K_{\epsilon,\delta}(t,x,\theta(x))-N K_{\epsilon,\delta,\infty}(t,x,\theta(x))\}dx \\ &\quad -\Gamma'(1)\int_{\widetilde{V}_{3\delta}}\mathrm{tr}_{s}\{N P_{\epsilon,\delta}(x,\theta(x))\}\,dx+k\cdot J(\epsilon,\delta;\rho). \end{aligned} \end{align*} $$
7.6.2 Limit of the first integral
Proposition 7.13. The following equality holds:
 $$ \begin{align*}\lim_{\delta\to0}\lim_{\epsilon\to0} \int_1^{\infty} \frac{dt}{t} \int_{\widetilde{V}_{3\delta}} \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x)) \, dx=0. \end{align*} $$
$$ \begin{align*}\lim_{\delta\to0}\lim_{\epsilon\to0} \int_1^{\infty} \frac{dt}{t} \int_{\widetilde{V}_{3\delta}} \mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x)) \, dx=0. \end{align*} $$
The same is true for the first integral in the expression of
![]() $\ln \tau _{\mathbf {Z}_{2}}(\widetilde {V}_{3\delta }, \widetilde {X}, \gamma _{\epsilon , \delta })$
.
$\ln \tau _{\mathbf {Z}_{2}}(\widetilde {V}_{3\delta }, \widetilde {X}, \gamma _{\epsilon , \delta })$
.
Proof. Let
![]() $\nu>0$
be arbitrary. As in the proof of Theorem 7.8 Step 2, there is
$\nu>0$
be arbitrary. As in the proof of Theorem 7.8 Step 2, there is
![]() $T=T(\nu )>0$
depending only on
$T=T(\nu )>0$
depending only on
![]() $\nu $
such that
$\nu $
such that
 $$ \begin{align} \int_T^{\infty} t^{-1} \int_{\widetilde{V}_{3\delta}} \left|\mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x)) \right|\, dx \, dt < \nu \end{align} $$
$$ \begin{align} \int_T^{\infty} t^{-1} \int_{\widetilde{V}_{3\delta}} \left|\mathrm{tr}_s(N K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x)) \right|\, dx \, dt < \nu \end{align} $$
for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $\epsilon \leq \min \{\epsilon (\rho )\delta ^{2}, \delta ^{4}\}$
, which will be assumed throughout the proof. By Theorem 7.7,
$\epsilon \leq \min \{\epsilon (\rho )\delta ^{2}, \delta ^{4}\}$
, which will be assumed throughout the proof. By Theorem 7.7,
 $$ \begin{align} \left| \int_1^{T} t^{-1} \int_{\widetilde{V}_{3\delta}} \mathrm{tr}_s[N\{ K_{\epsilon, \delta}(t, x, x)) - K_{\epsilon, \delta,\infty}(t, x, x)\}] \, dx \, dt \right| \leq C(T)\, \mathrm{vol}(\widetilde{V}_{3\delta}), \end{align} $$
$$ \begin{align} \left| \int_1^{T} t^{-1} \int_{\widetilde{V}_{3\delta}} \mathrm{tr}_s[N\{ K_{\epsilon, \delta}(t, x, x)) - K_{\epsilon, \delta,\infty}(t, x, x)\}] \, dx \, dt \right| \leq C(T)\, \mathrm{vol}(\widetilde{V}_{3\delta}), \end{align} $$
where
![]() $C(T)$
is a constant depending only on T. By equation (7.14), we get
$C(T)$
is a constant depending only on T. By equation (7.14), we get
 $$ \begin{align} \int_{\widetilde{V}_{3\delta}}\mathrm{tr}_{s}[N(P_{\epsilon,\delta}(x,x)]\,dx = \int_{\widetilde{V}_{3\delta}}\frac{2\eta\wedge\bar{\eta}}{\|\eta\|_{L^{2}}^{2}} \leq 2\frac{\|\eta\wedge\bar{\eta}/\gamma_{0}^{2}\|_{L^{\infty}}}{\|\eta\|_{L^{2}}}\mathrm{Vol}(\widetilde{V}_{3\delta}). \end{align} $$
$$ \begin{align} \int_{\widetilde{V}_{3\delta}}\mathrm{tr}_{s}[N(P_{\epsilon,\delta}(x,x)]\,dx = \int_{\widetilde{V}_{3\delta}}\frac{2\eta\wedge\bar{\eta}}{\|\eta\|_{L^{2}}^{2}} \leq 2\frac{\|\eta\wedge\bar{\eta}/\gamma_{0}^{2}\|_{L^{\infty}}}{\|\eta\|_{L^{2}}}\mathrm{Vol}(\widetilde{V}_{3\delta}). \end{align} $$
By equations (7.13), (7.18) and (7.19), we get
 $$ \begin{align} \begin{aligned} \,& \left| \int_1^{T} t^{-1} \int_{\widetilde{V}_{3\delta}} \mathrm{tr}_s[N\{ K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x)-K_{\epsilon, \delta,\infty}(t, x,x)\}] \, dx \, dt \right| \\ & \leq \{C(T)+2\frac{\|\eta\wedge\bar{\eta}/\gamma_{0}^{2}\|_{L^{\infty}}}{\|\eta\|_{L^{2}}}\log T\}\, \mathrm{vol}(\widetilde{V}_{3\delta}). \end{aligned} \end{align} $$
$$ \begin{align} \begin{aligned} \,& \left| \int_1^{T} t^{-1} \int_{\widetilde{V}_{3\delta}} \mathrm{tr}_s[N\{ K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x)-K_{\epsilon, \delta,\infty}(t, x,x)\}] \, dx \, dt \right| \\ & \leq \{C(T)+2\frac{\|\eta\wedge\bar{\eta}/\gamma_{0}^{2}\|_{L^{\infty}}}{\|\eta\|_{L^{2}}}\log T\}\, \mathrm{vol}(\widetilde{V}_{3\delta}). \end{aligned} \end{align} $$
By Proposition 6.9, there is a constant
![]() $A>0$
such that
$A>0$
such that
 $$ \begin{align} \int_1^{T} t^{-1} \int_{\widetilde{V}_{3\delta}} \left| K_{\epsilon, \delta,\infty}(t, x,x) \right|\, dx \, dt \leq Ae^{C(\epsilon\delta^{-4}+1)T}\,\log T\cdot\mathrm{vol}(\widetilde{V}_{3\delta}) \end{align} $$
$$ \begin{align} \int_1^{T} t^{-1} \int_{\widetilde{V}_{3\delta}} \left| K_{\epsilon, \delta,\infty}(t, x,x) \right|\, dx \, dt \leq Ae^{C(\epsilon\delta^{-4}+1)T}\,\log T\cdot\mathrm{vol}(\widetilde{V}_{3\delta}) \end{align} $$
for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $\epsilon\, \delta ^{-2}\leq \epsilon (\rho )$
. By equations (7.20) and (7.21), we get
$\epsilon\, \delta ^{-2}\leq \epsilon (\rho )$
. By equations (7.20) and (7.21), we get
 $$ \begin{align} \left| \int_1^{T} t^{-1} \int_{\widetilde{V}_{3\delta}} \mathrm{tr}_s[N K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x)] \, dx \, dt \right| \leq \widetilde{C}(T)\, \mathrm{vol}(\widetilde{V}_{3\delta}), \end{align} $$
$$ \begin{align} \left| \int_1^{T} t^{-1} \int_{\widetilde{V}_{3\delta}} \mathrm{tr}_s[N K_{\epsilon, \delta}(t, x, x)P^{\perp}_{\epsilon, \delta}(x, x)] \, dx \, dt \right| \leq \widetilde{C}(T)\, \mathrm{vol}(\widetilde{V}_{3\delta}), \end{align} $$
where
![]() $\widetilde {C}(T)=C(T)+ (2\frac {\|\eta \wedge \bar {\eta }/\gamma _{0}^{2}\|_{L^{\infty }}}{\|\eta \|_{L^{2}}}+Ae^{2CT})\log T$
. Since
$\widetilde {C}(T)=C(T)+ (2\frac {\|\eta \wedge \bar {\eta }/\gamma _{0}^{2}\|_{L^{\infty }}}{\|\eta \|_{L^{2}}}+Ae^{2CT})\log T$
. Since
![]() $\nu>0$
can be chosen arbitrarily small, by taking into account that
$\nu>0$
can be chosen arbitrarily small, by taking into account that
![]() $\mathrm {vol}(\widetilde {V}_{3\delta }) $
goes to zero as
$\mathrm {vol}(\widetilde {V}_{3\delta }) $
goes to zero as
![]() $\delta \rightarrow 0$
, the result follows from equations (7.17) and (7.22).
$\delta \rightarrow 0$
, the result follows from equations (7.17) and (7.22).
7.6.3 Limit of the second integral
Proposition 7.14. The following equality holds:
 $$ \begin{align*}\lim_{\delta\to0}\lim_{\epsilon\to0} \int_{0}^{1}\frac{dt}{t}\int_{\widetilde{V}_{3\delta}}\mathrm{tr}_{s}\{N K_{\epsilon,\delta}(t,x,x)-N K_{\epsilon,\delta,\infty}(t,x,x)\}dx=0. \end{align*} $$
$$ \begin{align*}\lim_{\delta\to0}\lim_{\epsilon\to0} \int_{0}^{1}\frac{dt}{t}\int_{\widetilde{V}_{3\delta}}\mathrm{tr}_{s}\{N K_{\epsilon,\delta}(t,x,x)-N K_{\epsilon,\delta,\infty}(t,x,x)\}dx=0. \end{align*} $$
The same is true for the second integral in the expression of
![]() $\ln \tau _{\mathbf {Z}_{2}}(\widetilde {V}_{3\delta }, \widetilde {X}, \gamma _{\epsilon , \delta })$
.
$\ln \tau _{\mathbf {Z}_{2}}(\widetilde {V}_{3\delta }, \widetilde {X}, \gamma _{\epsilon , \delta })$
.
Proof. The proof is the same as above, using the estimate of Theorem 7.7. Indeed, we have, for all
![]() $\epsilon ,\delta \in (0,1]$
with
$\epsilon ,\delta \in (0,1]$
with
![]() $\epsilon \leq \min \{\epsilon (\rho )\delta ^{2}, \delta ^{4}\}$
, that there is a constant
$\epsilon \leq \min \{\epsilon (\rho )\delta ^{2}, \delta ^{4}\}$
, that there is a constant
![]() $C>0$
such that
$C>0$
such that
for all
![]() $(x,t)\in \widetilde {V}_{3\delta }\times (0,1]$
. Hence
$(x,t)\in \widetilde {V}_{3\delta }\times (0,1]$
. Hence
 $$ \begin{align} \left| \int_{0}^{1}\frac{dt}{t}\int_{\widetilde{V}_{3\delta}}\mathrm{tr}_{s}\{N K_{\epsilon,\delta}(t,x,x)-N K_{\epsilon,\delta,\infty}(t,x,x)\}dx \right| \leq C\,\mathrm{Vol}(\widetilde{V}_{3\delta},\gamma_{\epsilon, \delta}). \end{align} $$
$$ \begin{align} \left| \int_{0}^{1}\frac{dt}{t}\int_{\widetilde{V}_{3\delta}}\mathrm{tr}_{s}\{N K_{\epsilon,\delta}(t,x,x)-N K_{\epsilon,\delta,\infty}(t,x,x)\}dx \right| \leq C\,\mathrm{Vol}(\widetilde{V}_{3\delta},\gamma_{\epsilon, \delta}). \end{align} $$
By the fact that
we get the result.
7.6.4 Proof of Theorem 7.12
By equation (7.14), we get
 $$ \begin{align*}\lim_{\delta\to0}\lim_{\epsilon\to0}\int_{\widetilde{V}_{3\delta}}\mathrm{tr}_{s}(N P_{\epsilon,\delta}(x,x))\,dx = \lim_{\delta\to0}\lim_{\epsilon\to0}\int_{\widetilde{V}_{3\delta}}\mathrm{tr}_{s}(N P_{\epsilon,\delta}(x,\theta(x)))\,dx =0. \end{align*} $$
$$ \begin{align*}\lim_{\delta\to0}\lim_{\epsilon\to0}\int_{\widetilde{V}_{3\delta}}\mathrm{tr}_{s}(N P_{\epsilon,\delta}(x,x))\,dx = \lim_{\delta\to0}\lim_{\epsilon\to0}\int_{\widetilde{V}_{3\delta}}\mathrm{tr}_{s}(N P_{\epsilon,\delta}(x,\theta(x)))\,dx =0. \end{align*} $$
From Propositions 7.13 and 7.14, it follows that
 $$ \begin{align*}\lim_{\delta\to0}\lim_{\epsilon\to0} \ln\left[\epsilon^{k/3} \tau_{\mathbf{Z}_{2}}(\widetilde{V}_{3\delta}, \widetilde{X}, \gamma_{\epsilon, \delta})\right] = k\,\lim_{\delta\to0}\lim_{\epsilon\to0}\left[J(\epsilon,\delta;\rho)+\frac{1}{3}\ln\epsilon\right]. \end{align*} $$
$$ \begin{align*}\lim_{\delta\to0}\lim_{\epsilon\to0} \ln\left[\epsilon^{k/3} \tau_{\mathbf{Z}_{2}}(\widetilde{V}_{3\delta}, \widetilde{X}, \gamma_{\epsilon, \delta})\right] = k\,\lim_{\delta\to0}\lim_{\epsilon\to0}\left[J(\epsilon,\delta;\rho)+\frac{1}{3}\ln\epsilon\right]. \end{align*} $$
Since the right-hand side depends only on the choice of
![]() $\rho $
, we get the result by setting
$\rho $
, we get the result by setting
 $$ \begin{align*}\ln C_{0}^{\mathrm{EH}}(\rho):=\lim_{\delta\to0}\lim_{\epsilon\to0}I(\epsilon,\delta;\rho), \qquad \ln C_{1}^{\mathrm{EH}}(\rho):=\lim_{\delta\to0}\lim_{\epsilon\to0}\left[J(\epsilon,\delta;\rho)+\frac{1}{3}\ln\epsilon\right]. \end{align*} $$
$$ \begin{align*}\ln C_{0}^{\mathrm{EH}}(\rho):=\lim_{\delta\to0}\lim_{\epsilon\to0}I(\epsilon,\delta;\rho), \qquad \ln C_{1}^{\mathrm{EH}}(\rho):=\lim_{\delta\to0}\lim_{\epsilon\to0}\left[J(\epsilon,\delta;\rho)+\frac{1}{3}\ln\epsilon\right]. \end{align*} $$
This completes the proof, provided that these double limits exist. This will be addressed in what follows.
Remark 7.15.
![]() $C_0^{\mathrm {EH}}(\rho )$
, respectively
$C_0^{\mathrm {EH}}(\rho )$
, respectively
![]() $C_1^{\mathrm {EH}}(\rho )$
, is renormalised (respectively, equivariant) analytic torsion for the asymptotically conical space
$C_1^{\mathrm {EH}}(\rho )$
, is renormalised (respectively, equivariant) analytic torsion for the asymptotically conical space
![]() $\widetilde {V}(\infty )=(T^{*}\mathbf {P}^{1},\gamma ^{\mathrm {EH}})$
.
$\widetilde {V}(\infty )=(T^{*}\mathbf {P}^{1},\gamma ^{\mathrm {EH}})$
.
7.7 Proof of Theorem 7.1
Since
and
by the definition of partial (equivariant) analytic torsion, we get by Corollary 7.11 and Theorem 7.12
As the double limits on the left-hand side of equations (7.24) and (7.25) exist by virtue of Corollary 5.3 and Proposition 7.2, so do the double limits in defining
![]() $\ln C_{0}^{\mathrm {EH}}(\rho )$
and
$\ln C_{0}^{\mathrm {EH}}(\rho )$
and
![]() $\ln C_{1}^{\mathrm {EH}}(\rho )$
.
$\ln C_{1}^{\mathrm {EH}}(\rho )$
.
On the other hand, again by Corollary 5.3 and Proposition 7.2, the double limits
![]() $\lim _{\delta \to 0}\lim _{\epsilon \to 0}\ln \tau (\widetilde {X}, \gamma _{\epsilon , \delta })$
and
$\lim _{\delta \to 0}\lim _{\epsilon \to 0}\ln \tau (\widetilde {X}, \gamma _{\epsilon , \delta })$
and
![]() $\lim _{\delta \to 0}\lim _{\epsilon \to 0}\ln \left [\epsilon ^{k/3} \tau _{\mathbf {Z}_{2}}(\widetilde {X}, \gamma _{\epsilon , \delta })\right ]$
are independent of the choice of
$\lim _{\delta \to 0}\lim _{\epsilon \to 0}\ln \left [\epsilon ^{k/3} \tau _{\mathbf {Z}_{2}}(\widetilde {X}, \gamma _{\epsilon , \delta })\right ]$
are independent of the choice of
![]() $\rho $
. Hence
$\rho $
. Hence
![]() $C_{0}^{\mathrm {EH}}(\rho )$
and
$C_{0}^{\mathrm {EH}}(\rho )$
and
![]() $C_{1}^{\mathrm {EH}}(\rho )$
in equations (7.24) and (7.25) are in fact independent of
$C_{1}^{\mathrm {EH}}(\rho )$
in equations (7.24) and (7.25) are in fact independent of
![]() $\rho $
. This completes the proof of Theorem 7.1.
$\rho $
. This completes the proof of Theorem 7.1.
8 A holomorphic torsion invariant of log-Enriques surfaces
In this section, we introduce a holomorphic torsion invariant of log-Enriques surfaces and give its explicit formula as a function on the moduli space.
8.1 A construction of invariant
Theorem 8.1. There is a constant
![]() $C(k)$
depending only on
$C(k)$
depending only on
![]() $k=\#\mathrm {Sing}(Y)$
with
$k=\#\mathrm {Sing}(Y)$
with
 $$ \begin{align*}\begin{aligned} \tau_{M}(\widetilde{X},\theta) &= C(k)\mathrm{Vol}(Y,\gamma_{0})^{\frac{4-k}{4}}\,\tau(Y,\gamma_{0})^{2} \times \prod_{{\frak p}\in\mathrm{Sing}(X)}\left\{|f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(Y,\gamma_{0})}{\|\eta\|_{L^{2}(Y)}^{2}}\right\}^{\frac{5}{16}} \\ &\quad\times \exp \left( \frac{1}{12}\int_{Y} \log\left\{\frac{\eta\wedge\bar{\eta}}{\gamma_{0}^{2}/2!}\cdot\frac{\mathrm{Vol}(Y,\gamma_{0})}{\|\eta\|_{L^{2}(Y)}^{2}}\right\}\, c_{2}(Y,\gamma_{0}) \right). \end{aligned} \end{align*} $$
$$ \begin{align*}\begin{aligned} \tau_{M}(\widetilde{X},\theta) &= C(k)\mathrm{Vol}(Y,\gamma_{0})^{\frac{4-k}{4}}\,\tau(Y,\gamma_{0})^{2} \times \prod_{{\frak p}\in\mathrm{Sing}(X)}\left\{|f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(Y,\gamma_{0})}{\|\eta\|_{L^{2}(Y)}^{2}}\right\}^{\frac{5}{16}} \\ &\quad\times \exp \left( \frac{1}{12}\int_{Y} \log\left\{\frac{\eta\wedge\bar{\eta}}{\gamma_{0}^{2}/2!}\cdot\frac{\mathrm{Vol}(Y,\gamma_{0})}{\|\eta\|_{L^{2}(Y)}^{2}}\right\}\, c_{2}(Y,\gamma_{0}) \right). \end{aligned} \end{align*} $$
Here
![]() $f_{\frak p}$
is defined in the discussion immediately preceding equation (4.13).
$f_{\frak p}$
is defined in the discussion immediately preceding equation (4.13).
Proof. Since
![]() $M_{k}^{\perp }\cong \Lambda _{k}(2)$
, we have
$M_{k}^{\perp }\cong \Lambda _{k}(2)$
, we have
![]() $\frac {14-r(H^{2}(\widetilde {X},\mathbf {Z})^{+})}{4}=\frac {4-k}{4}$
. By its independence of the choice of
$\frac {14-r(H^{2}(\widetilde {X},\mathbf {Z})^{+})}{4}=\frac {4-k}{4}$
. By its independence of the choice of
![]() $\theta $
-invariant Kähler metric on
$\theta $
-invariant Kähler metric on
![]() $\widetilde {X}$
,
$\widetilde {X}$
,
![]() $\tau _{M}(\widetilde {X},\theta )$
is given by
$\tau _{M}(\widetilde {X},\theta )$
is given by
 $$ \begin{align*}& \lim_{\delta\to0}\lim_{\epsilon\to0} \tau(\widetilde{X},\gamma_{\epsilon,\delta})\tau_{\mathbf{Z}_{2}}(\widetilde{X},\gamma_{\epsilon,\delta})(\theta)\, \mathrm{Vol}(\widetilde{X},\gamma_{\epsilon,\delta})^{\frac{4-k}{4}} \mathrm{Vol}(\widetilde{X}^{\theta},\gamma_{\epsilon,\delta}|_{\widetilde{X}^{\theta}}) \tau(\widetilde{X}^{\theta},\gamma_{\epsilon,\delta}|_{\widetilde{X}^{\theta}}) \\ &\quad\times A_{M}(\widetilde{X},\theta,\gamma_{\epsilon,\delta})\, \exp \left( \frac{1}{24}\int_{\widetilde{X}} \log\left\{ \frac{\eta\wedge\bar{\eta}}{\gamma_{\epsilon,\delta}^{2}/2!} \cdot \frac{\mathrm{Vol}(\widetilde{X},\gamma_{\epsilon,\delta})}{\|\eta\|_{L^{2}(\widetilde{X})}^{2}} \right\}\, c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) \right) \\ &= \lim_{\delta\to0}\lim_{\epsilon\to0} \{\epsilon^{\frac{k}{3}}\tau(\widetilde{X},\gamma_{\epsilon,\delta})\tau_{\mathbf{Z}_{2}}(\widetilde{X},\gamma_{\epsilon,\delta})(\theta) \mathrm{Vol}(\widetilde{X},\gamma_{\epsilon,\delta})^{\frac{4-k}{4}} \} \\ &\quad\times \lim_{\delta\to0}\lim_{\epsilon\to0} \prod_{{\frak p}\in\mathrm{Sing}(X)}\epsilon^{-\frac{1}{3}}\mathrm{Vol}(E_{\frak p},\gamma_{\epsilon,\delta}|_{E_{\frak p}}) \tau(E_{\frak p},\gamma_{\epsilon,\delta}|_{E_{\frak p}}) \times \lim_{\delta\to0}\lim_{\epsilon\to0}A_{M}(\widetilde{X},\theta,\gamma_{\epsilon,\delta}) \\ &\quad\times \lim_{\delta\to0}\lim_{\epsilon\to0} \exp \left( \frac{1}{24}\int_{\widetilde{X}} \log \left\{ \frac{\eta\wedge\bar{\eta}}{\gamma_{\epsilon,\delta}^{2}/2!} \cdot \frac{\mathrm{Vol}(\widetilde{X},\gamma_{\epsilon,\delta})}{\|\eta\|_{L^{2}(\widetilde{X})}^{2}} \right\}\, c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) \right). \end{align*} $$
$$ \begin{align*}& \lim_{\delta\to0}\lim_{\epsilon\to0} \tau(\widetilde{X},\gamma_{\epsilon,\delta})\tau_{\mathbf{Z}_{2}}(\widetilde{X},\gamma_{\epsilon,\delta})(\theta)\, \mathrm{Vol}(\widetilde{X},\gamma_{\epsilon,\delta})^{\frac{4-k}{4}} \mathrm{Vol}(\widetilde{X}^{\theta},\gamma_{\epsilon,\delta}|_{\widetilde{X}^{\theta}}) \tau(\widetilde{X}^{\theta},\gamma_{\epsilon,\delta}|_{\widetilde{X}^{\theta}}) \\ &\quad\times A_{M}(\widetilde{X},\theta,\gamma_{\epsilon,\delta})\, \exp \left( \frac{1}{24}\int_{\widetilde{X}} \log\left\{ \frac{\eta\wedge\bar{\eta}}{\gamma_{\epsilon,\delta}^{2}/2!} \cdot \frac{\mathrm{Vol}(\widetilde{X},\gamma_{\epsilon,\delta})}{\|\eta\|_{L^{2}(\widetilde{X})}^{2}} \right\}\, c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) \right) \\ &= \lim_{\delta\to0}\lim_{\epsilon\to0} \{\epsilon^{\frac{k}{3}}\tau(\widetilde{X},\gamma_{\epsilon,\delta})\tau_{\mathbf{Z}_{2}}(\widetilde{X},\gamma_{\epsilon,\delta})(\theta) \mathrm{Vol}(\widetilde{X},\gamma_{\epsilon,\delta})^{\frac{4-k}{4}} \} \\ &\quad\times \lim_{\delta\to0}\lim_{\epsilon\to0} \prod_{{\frak p}\in\mathrm{Sing}(X)}\epsilon^{-\frac{1}{3}}\mathrm{Vol}(E_{\frak p},\gamma_{\epsilon,\delta}|_{E_{\frak p}}) \tau(E_{\frak p},\gamma_{\epsilon,\delta}|_{E_{\frak p}}) \times \lim_{\delta\to0}\lim_{\epsilon\to0}A_{M}(\widetilde{X},\theta,\gamma_{\epsilon,\delta}) \\ &\quad\times \lim_{\delta\to0}\lim_{\epsilon\to0} \exp \left( \frac{1}{24}\int_{\widetilde{X}} \log \left\{ \frac{\eta\wedge\bar{\eta}}{\gamma_{\epsilon,\delta}^{2}/2!} \cdot \frac{\mathrm{Vol}(\widetilde{X},\gamma_{\epsilon,\delta})}{\|\eta\|_{L^{2}(\widetilde{X})}^{2}} \right\}\, c_{2}(\widetilde{X},\gamma_{\epsilon,\delta}) \right). \end{align*} $$
By equation (4.14), Propositions 5.2, 5.4, 5.5, Corollary 5.3 and Theorem 7.1, we get
 $$ \begin{align*}\begin{aligned} \tau_{M}(\widetilde{X},\theta) &= (C_{0}^{\mathrm{EH}}C_{1}^{\mathrm{EH}})^{k}\,\tau(X,\gamma_{0})\tau_{\mathbf{Z}_{2}}(X,\gamma_{0})(\iota)\, \{2\mathrm{Vol}(Y,\gamma_{0})\}^{\frac{4-k}{4}} \\ &\quad\times \{\mathrm{Vol}(\mathbf{P}^{1},\omega_{\mathrm{FS}})\tau(\mathbf{P}^{1},\omega_{\mathrm{FS}})\}^{k} \times \prod_{{\frak p}\in\mathrm{Sing}(X)}\left\{|f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}(X)}^{2}}\right\}^{\frac{1}{4}+\frac{1}{16}} \\ &\quad\times \exp \left[ \frac{1}{24}\int_{X} \log\left\{\frac{\eta\wedge\bar{\eta}}{\gamma_{0}^{2}/2!}\cdot\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}(X)}^{2}}\right\}\, c_{2}(X,\gamma_{0}) \right]. \end{aligned} \end{align*} $$
$$ \begin{align*}\begin{aligned} \tau_{M}(\widetilde{X},\theta) &= (C_{0}^{\mathrm{EH}}C_{1}^{\mathrm{EH}})^{k}\,\tau(X,\gamma_{0})\tau_{\mathbf{Z}_{2}}(X,\gamma_{0})(\iota)\, \{2\mathrm{Vol}(Y,\gamma_{0})\}^{\frac{4-k}{4}} \\ &\quad\times \{\mathrm{Vol}(\mathbf{P}^{1},\omega_{\mathrm{FS}})\tau(\mathbf{P}^{1},\omega_{\mathrm{FS}})\}^{k} \times \prod_{{\frak p}\in\mathrm{Sing}(X)}\left\{|f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}(X)}^{2}}\right\}^{\frac{1}{4}+\frac{1}{16}} \\ &\quad\times \exp \left[ \frac{1}{24}\int_{X} \log\left\{\frac{\eta\wedge\bar{\eta}}{\gamma_{0}^{2}/2!}\cdot\frac{\mathrm{Vol}(X,\gamma_{0})}{\|\eta\|_{L^{2}(X)}^{2}}\right\}\, c_{2}(X,\gamma_{0}) \right]. \end{aligned} \end{align*} $$
Since
and since X is a double covering of Y, we get the result by setting
This completes the proof.
Theorem 8.2. Let
![]() $\gamma $
be a Kähler form on Y in the sense of orbifolds. Then the following equality holds:
$\gamma $
be a Kähler form on Y in the sense of orbifolds. Then the following equality holds:
 $$ \begin{align*}\frac{\tau(Y,\gamma)\mathrm{Vol}(Y,\gamma)}{\tau(Y,\omega_{\eta})\mathrm{Vol}(Y,\omega_{\eta})} = \left\{ \prod_{{\frak p}\in\mathrm{Sing}(Y)}\left(\frac{\omega_{\eta}^{2}}{\gamma^{2}}\right)({\frak p}) \right\}^{-\frac{5}{32}} \exp \left\{ -\frac{1}{24}\int_{Y} \log\left(\frac{\omega_{\eta}^{2}}{\gamma^{2}}\right)\,c_{2}(Y,\gamma) \right\}. \end{align*} $$
$$ \begin{align*}\frac{\tau(Y,\gamma)\mathrm{Vol}(Y,\gamma)}{\tau(Y,\omega_{\eta})\mathrm{Vol}(Y,\omega_{\eta})} = \left\{ \prod_{{\frak p}\in\mathrm{Sing}(Y)}\left(\frac{\omega_{\eta}^{2}}{\gamma^{2}}\right)({\frak p}) \right\}^{-\frac{5}{32}} \exp \left\{ -\frac{1}{24}\int_{Y} \log\left(\frac{\omega_{\eta}^{2}}{\gamma^{2}}\right)\,c_{2}(Y,\gamma) \right\}. \end{align*} $$
Proof. Let
![]() ${\frak p}\in \mathrm {Sing}(Y)$
, and let
${\frak p}\in \mathrm {Sing}(Y)$
, and let
![]() $({\mathcal U}_{\frak p},0)\subset (\mathbf {C}^{2},0)$
be an open subset that uniformises the germ
$({\mathcal U}_{\frak p},0)\subset (\mathbf {C}^{2},0)$
be an open subset that uniformises the germ
![]() $(Y,{\frak p})$
. We have an isomorphism
$(Y,{\frak p})$
. We have an isomorphism
![]() $(Y,{\frak p})\cong (\mathbf {C}^{2}/\Gamma _{\frak p},0)$
of germs, where
$(Y,{\frak p})\cong (\mathbf {C}^{2}/\Gamma _{\frak p},0)$
of germs, where
![]() $\Gamma _{\frak p}=\mathbf {Z}/4\mathbf {Z}=\langle i\rangle $
, such that
$\Gamma _{\frak p}=\mathbf {Z}/4\mathbf {Z}=\langle i\rangle $
, such that
![]() $\omega _{\eta }$
and
$\omega _{\eta }$
and
![]() $\gamma $
lift to Kähler metrics on
$\gamma $
lift to Kähler metrics on
![]() ${\mathcal U}_{\frak p}$
. Following Ma [Reference Ma32], we define
${\mathcal U}_{\frak p}$
. Following Ma [Reference Ma32], we define
![]() $Y^{\Sigma }$
as the union
$Y^{\Sigma }$
as the union
![]() $Y^{\Sigma }:=Y^{i}\amalg Y^{i^{2}}\amalg Y^{i^{3}}$
, where
$Y^{\Sigma }:=Y^{i}\amalg Y^{i^{2}}\amalg Y^{i^{3}}$
, where
![]() $Y^{i^{\nu }}=\{{\frak p}^{i^{\nu }}\}_{{\frak p}\in \mathrm {Sing}(Y)}$
and the germ
$Y^{i^{\nu }}=\{{\frak p}^{i^{\nu }}\}_{{\frak p}\in \mathrm {Sing}(Y)}$
and the germ
![]() $(Y^{i^{\nu }},{\frak p}^{i^{\nu }})$
is equipped with orbifold structure
$(Y^{i^{\nu }},{\frak p}^{i^{\nu }})$
is equipped with orbifold structure
![]() $(Y^{i^{\nu }},{\frak p}^{i^{\nu }})\cong (\mathbf {C}^{2}/\langle i^{\nu }\rangle ,0)$
.
$(Y^{i^{\nu }},{\frak p}^{i^{\nu }})\cong (\mathbf {C}^{2}/\langle i^{\nu }\rangle ,0)$
.
Recall that the characteristic class
![]() $\mathrm {Td}^{\Sigma }(TY)$
supported on the singular locus of Y appears in the Riemann-Roch theorem for orbifolds, for which we refer the reader to, for example, [Reference Ma32]. By the anomaly formula for Quillen metrics for orbifolds [Reference Ma32], we get
$\mathrm {Td}^{\Sigma }(TY)$
supported on the singular locus of Y appears in the Riemann-Roch theorem for orbifolds, for which we refer the reader to, for example, [Reference Ma32]. By the anomaly formula for Quillen metrics for orbifolds [Reference Ma32], we get
 $$ \begin{align} \begin{aligned} \,& \log \left( \frac{\tau(Y,\gamma)\mathrm{Vol}(Y,\gamma)}{\tau(Y,\omega_{\eta})\mathrm{Vol}(Y,\omega_{\eta})} \right) = \frac{1}{4}\int_{Y^{\Sigma}}\widetilde{\mathrm{Td}}^{\Sigma}(TY;\gamma,\omega_{\eta}) + \frac{1}{24}\int_{Y}\widetilde{c_{1}c_{2}}(TY;\gamma,\omega_{\eta}) \\ &= \frac{1}{4}\sum_{{\frak p}\in\mathrm{Sing}(Y)}\sum_{\nu=1}^{3} \left[ \left(\widetilde{\frac{\mathrm{Td}}{\mathrm{e}}}\right)_{\nu/2}(TU_{\frak p};\gamma,\omega_{\eta}) \right]^{(0,0)}({\frak p}) + \frac{1}{24}\int_{Y}\widetilde{c_{1}c_{2}}(TY;\gamma,\omega_{\eta})^{(2,2)}. \end{aligned} \end{align} $$
$$ \begin{align} \begin{aligned} \,& \log \left( \frac{\tau(Y,\gamma)\mathrm{Vol}(Y,\gamma)}{\tau(Y,\omega_{\eta})\mathrm{Vol}(Y,\omega_{\eta})} \right) = \frac{1}{4}\int_{Y^{\Sigma}}\widetilde{\mathrm{Td}}^{\Sigma}(TY;\gamma,\omega_{\eta}) + \frac{1}{24}\int_{Y}\widetilde{c_{1}c_{2}}(TY;\gamma,\omega_{\eta}) \\ &= \frac{1}{4}\sum_{{\frak p}\in\mathrm{Sing}(Y)}\sum_{\nu=1}^{3} \left[ \left(\widetilde{\frac{\mathrm{Td}}{\mathrm{e}}}\right)_{\nu/2}(TU_{\frak p};\gamma,\omega_{\eta}) \right]^{(0,0)}({\frak p}) + \frac{1}{24}\int_{Y}\widetilde{c_{1}c_{2}}(TY;\gamma,\omega_{\eta})^{(2,2)}. \end{aligned} \end{align} $$
Here, for
![]() $\theta \in \mathbf {R}$
and a square matrix A, we define
$\theta \in \mathbf {R}$
and a square matrix A, we define
![]() $\left ({\frac {\mathrm {Td}}{\mathrm {e}}}\right )_{\theta }(A):=\det \left (\frac {I}{I-e^{-(\pi i\theta +A)}}\right )$
, and
$\left ({\frac {\mathrm {Td}}{\mathrm {e}}}\right )_{\theta }(A):=\det \left (\frac {I}{I-e^{-(\pi i\theta +A)}}\right )$
, and
![]() $(\widetilde {\mathrm {Td}/\mathrm {e}})_{\theta }$
is the Bott-Chern secondary class associated to
$(\widetilde {\mathrm {Td}/\mathrm {e}})_{\theta }$
is the Bott-Chern secondary class associated to
![]() $\left (\mathrm {Td}/\mathrm {e}\right )_{\theta }(A)$
such that for any holomorphic vector bundle E and Hermitian metrics h,
$\left (\mathrm {Td}/\mathrm {e}\right )_{\theta }(A)$
such that for any holomorphic vector bundle E and Hermitian metrics h,
![]() $h'$
on E
$h'$
on E
 $$ \begin{align*}-dd^{c}\left(\widetilde{\frac{\mathrm{Td}}{\mathrm{e}}}\right)_{\theta}(E;h,h') = \left(\frac{\mathrm{Td}}{\mathrm{e}}\right)_{\theta}\left(-\frac{1}{2\pi i}R(E,h)\right) - \left(\frac{\mathrm{Td}}{\mathrm{e}}\right)_{\theta}\left(-\frac{1}{2\pi i}R(E,h')\right). \end{align*} $$
$$ \begin{align*}-dd^{c}\left(\widetilde{\frac{\mathrm{Td}}{\mathrm{e}}}\right)_{\theta}(E;h,h') = \left(\frac{\mathrm{Td}}{\mathrm{e}}\right)_{\theta}\left(-\frac{1}{2\pi i}R(E,h)\right) - \left(\frac{\mathrm{Td}}{\mathrm{e}}\right)_{\theta}\left(-\frac{1}{2\pi i}R(E,h')\right). \end{align*} $$
Similarly,
![]() $\widetilde {c_{1}c_{2}}$
is the Bott-Chern secondary class associated to the invariant polynomial
$\widetilde {c_{1}c_{2}}$
is the Bott-Chern secondary class associated to the invariant polynomial
![]() $c_{1}(A)c_{2}(A)$
such that for any holomorphic vector bundle E and Hermitian metrics h,
$c_{1}(A)c_{2}(A)$
such that for any holomorphic vector bundle E and Hermitian metrics h,
![]() $h'$
on E
$h'$
on E
For
![]() $A=\mathrm {diag}(\lambda _{1},\lambda _{2})$
, we have
$A=\mathrm {diag}(\lambda _{1},\lambda _{2})$
, we have
 $$ \begin{align*}\left(\frac{\mathrm{Td}}{\mathrm{e}}\right)_{\frac{\nu}{2}}(A)=\frac{1}{(1-i^{-\nu})^{2}}\{1-\frac{i^{-\nu}}{1-i^{-\nu}}c_{1}(A)+O(2)\}. \end{align*} $$
$$ \begin{align*}\left(\frac{\mathrm{Td}}{\mathrm{e}}\right)_{\frac{\nu}{2}}(A)=\frac{1}{(1-i^{-\nu})^{2}}\{1-\frac{i^{-\nu}}{1-i^{-\nu}}c_{1}(A)+O(2)\}. \end{align*} $$
Thus we get
 $$ \begin{align} \begin{aligned} \sum_{\nu=1}^{3} \left[ \left(\widetilde{\frac{\mathrm{Td}}{\mathrm{e}}}\right)_{\frac{\nu}{2}}(TU_{\frak p};\gamma,\omega_{\eta}) \right]^{(0,0)} ({\frak p}) &= -\sum_{\nu=1}^{3}\frac{i^{-\nu}}{(1-i^{-\nu})^{3}}\,\widetilde{c_{1}}(TU_{\frak p};\gamma,\omega_{\eta})({\frak p}) \\ &= \frac{5}{8}\widetilde{c_{1}}(TU_{\frak p};\gamma,\omega_{\eta})({\frak p}) = -\frac{5}{8}\log\left(\omega_{\eta}^{2}/\gamma^{2}\right)({\frak p}). \end{aligned} \end{align} $$
$$ \begin{align} \begin{aligned} \sum_{\nu=1}^{3} \left[ \left(\widetilde{\frac{\mathrm{Td}}{\mathrm{e}}}\right)_{\frac{\nu}{2}}(TU_{\frak p};\gamma,\omega_{\eta}) \right]^{(0,0)} ({\frak p}) &= -\sum_{\nu=1}^{3}\frac{i^{-\nu}}{(1-i^{-\nu})^{3}}\,\widetilde{c_{1}}(TU_{\frak p};\gamma,\omega_{\eta})({\frak p}) \\ &= \frac{5}{8}\widetilde{c_{1}}(TU_{\frak p};\gamma,\omega_{\eta})({\frak p}) = -\frac{5}{8}\log\left(\omega_{\eta}^{2}/\gamma^{2}\right)({\frak p}). \end{aligned} \end{align} $$
On the other hand, by the same computations as in equation (3.3), we get
Substituting equation (8.3) and equation (8.4) into equation (8.2), we get the result.
Theorem 8.3. For every Ricci-flat log-Enriques surface
![]() $(Y,\omega )$
, one has
$(Y,\omega )$
, one has
where
![]() $C(k)$
is the same constant as in Theorem 8.1.
$C(k)$
is the same constant as in Theorem 8.1.
Proof. We put
![]() $\gamma =\gamma _{0}$
in Theorem 8.2. Then we get by Theorem 8.1
$\gamma =\gamma _{0}$
in Theorem 8.2. Then we get by Theorem 8.1
 $$ \begin{align*}\begin{aligned} \,& \tau(Y,\omega_{\eta})\mathrm{Vol}(Y,\omega_{\eta}) = \tau(Y,\gamma_{0})\mathrm{Vol}(Y,\gamma_{0})^{\frac{4-k}{8}}\mathrm{Vol}(Y,\gamma_{0})^{\frac{4+k}{8}} \\ &\qquad\qquad\qquad\qquad\quad\times \{ \prod_{{\frak p}\in\mathrm{Sing}(X)}\left(\frac{\omega_{\eta}^{2}}{\gamma_{0}^{2}}\right)({\frak p}) \}^{\frac{5}{32}} \exp \left[ \frac{1}{24}\int_{Y} \log\left(\frac{\omega_{\eta}^{2}}{\gamma_{0}^{2}}\right)\,c_{2}(Y,\gamma_{0}) \right] \\ &= C(k)^{-1}\tau_{M}(\widetilde{X},\theta)^{\frac{1}{2}}\mathrm{Vol}(Y,\gamma_{0})^{\frac{4+k}{8}} \times \prod_{{\frak p}\in\mathrm{Sing}(X)}\left\{|f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(Y,\gamma_{0})}{\|\eta\|_{L^{2}(Y)}^{2}}\right\}^{-\frac{5}{32}} \\ &\quad\times \exp \left[ -\frac{1}{24}\int_{Y} \log\left\{\frac{\eta\wedge\bar{\eta}}{\gamma_{0}^{2}/2!}\cdot\frac{\mathrm{Vol}(Y,\gamma_{0})}{\|\eta\|_{L^{2}(Y)}^{2}}\right\}\, c_{2}(Y,\gamma_{0}) \right] \\ &\quad\times \{ \prod_{{\frak p}\in\mathrm{Sing}(X)}\left(\frac{\omega_{\eta}^{2}}{\gamma_{0}^{2}}\right)({\frak p}) \}^{\frac{5}{32}} \exp \left[ \frac{1}{24}\int_{Y} \log\left(\frac{\eta\wedge\bar{\eta}}{\gamma_{0}^{2}/2!}\right)\,c_{2}(Y,\gamma_{0}) \right]. \end{aligned} \end{align*} $$
$$ \begin{align*}\begin{aligned} \,& \tau(Y,\omega_{\eta})\mathrm{Vol}(Y,\omega_{\eta}) = \tau(Y,\gamma_{0})\mathrm{Vol}(Y,\gamma_{0})^{\frac{4-k}{8}}\mathrm{Vol}(Y,\gamma_{0})^{\frac{4+k}{8}} \\ &\qquad\qquad\qquad\qquad\quad\times \{ \prod_{{\frak p}\in\mathrm{Sing}(X)}\left(\frac{\omega_{\eta}^{2}}{\gamma_{0}^{2}}\right)({\frak p}) \}^{\frac{5}{32}} \exp \left[ \frac{1}{24}\int_{Y} \log\left(\frac{\omega_{\eta}^{2}}{\gamma_{0}^{2}}\right)\,c_{2}(Y,\gamma_{0}) \right] \\ &= C(k)^{-1}\tau_{M}(\widetilde{X},\theta)^{\frac{1}{2}}\mathrm{Vol}(Y,\gamma_{0})^{\frac{4+k}{8}} \times \prod_{{\frak p}\in\mathrm{Sing}(X)}\left\{|f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(Y,\gamma_{0})}{\|\eta\|_{L^{2}(Y)}^{2}}\right\}^{-\frac{5}{32}} \\ &\quad\times \exp \left[ -\frac{1}{24}\int_{Y} \log\left\{\frac{\eta\wedge\bar{\eta}}{\gamma_{0}^{2}/2!}\cdot\frac{\mathrm{Vol}(Y,\gamma_{0})}{\|\eta\|_{L^{2}(Y)}^{2}}\right\}\, c_{2}(Y,\gamma_{0}) \right] \\ &\quad\times \{ \prod_{{\frak p}\in\mathrm{Sing}(X)}\left(\frac{\omega_{\eta}^{2}}{\gamma_{0}^{2}}\right)({\frak p}) \}^{\frac{5}{32}} \exp \left[ \frac{1}{24}\int_{Y} \log\left(\frac{\eta\wedge\bar{\eta}}{\gamma_{0}^{2}/2!}\right)\,c_{2}(Y,\gamma_{0}) \right]. \end{aligned} \end{align*} $$
Since
![]() $|f_{\frak p}(0)|^{2}=[\eta \wedge \bar {\eta }/(\gamma _{0}^{2}/2!)]({\frak p})=[\omega _{\eta }^{2}/\gamma _{0}^{2}]({\frak p})$
, we get
$|f_{\frak p}(0)|^{2}=[\eta \wedge \bar {\eta }/(\gamma _{0}^{2}/2!)]({\frak p})=[\omega _{\eta }^{2}/\gamma _{0}^{2}]({\frak p})$
, we get
 $$ \begin{align*}\begin{aligned} \,& \tau(Y,\omega_{\eta})\mathrm{Vol}(Y,\omega_{\eta}) \\ &= C(k)^{-1}\tau_{M}(\widetilde{X},\theta)^{\frac{1}{2}}\mathrm{Vol}(Y,\gamma_{0})^{\frac{4+k}{8}} \times \prod_{{\frak p}\in\mathrm{Sing}(X)}\left(|f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(Y,\gamma_{0})}{\mathrm{Vol}(Y,\omega_{\eta})}\right)^{-\frac{5}{32}} \\ &\quad\times \{ \prod_{{\frak p}\in\mathrm{Sing}(X)}|f_{\frak p}(0)|^{2} \}^{\frac{5}{32}} \exp \left[ -\frac{1}{24}\int_{Y} \log\left\{\frac{\mathrm{Vol}(Y,\gamma_{0})}{\mathrm{Vol}(Y,\omega_{\eta})}\right\}\, c_{2}(Y,\gamma_{0}) \right] \\ &= C(k)^{-1}\tau_{M}(\widetilde{X},\theta)^{\frac{1}{2}}\mathrm{Vol}(Y,\gamma_{0})^{\frac{4+k}{8}} \left(\frac{\mathrm{Vol}(Y,\gamma_{0})}{\mathrm{Vol}(Y,\omega_{\eta})}\right)^{-\frac{5}{32}k} \exp\left[ -\frac{16-k}{32}\log\left(\frac{\mathrm{Vol}(Y,\gamma_{0})}{\mathrm{Vol}(Y,\omega_{\eta})}\right) \right] \\ &= C(k)^{-1}\tau_{M}(\widetilde{X},\theta)^{\frac{1}{2}}\mathrm{Vol}(Y,\omega_{\eta})^{\frac{4+k}{8}}, \end{aligned} \end{align*} $$
$$ \begin{align*}\begin{aligned} \,& \tau(Y,\omega_{\eta})\mathrm{Vol}(Y,\omega_{\eta}) \\ &= C(k)^{-1}\tau_{M}(\widetilde{X},\theta)^{\frac{1}{2}}\mathrm{Vol}(Y,\gamma_{0})^{\frac{4+k}{8}} \times \prod_{{\frak p}\in\mathrm{Sing}(X)}\left(|f_{\frak p}(0)|^{2}\frac{\mathrm{Vol}(Y,\gamma_{0})}{\mathrm{Vol}(Y,\omega_{\eta})}\right)^{-\frac{5}{32}} \\ &\quad\times \{ \prod_{{\frak p}\in\mathrm{Sing}(X)}|f_{\frak p}(0)|^{2} \}^{\frac{5}{32}} \exp \left[ -\frac{1}{24}\int_{Y} \log\left\{\frac{\mathrm{Vol}(Y,\gamma_{0})}{\mathrm{Vol}(Y,\omega_{\eta})}\right\}\, c_{2}(Y,\gamma_{0}) \right] \\ &= C(k)^{-1}\tau_{M}(\widetilde{X},\theta)^{\frac{1}{2}}\mathrm{Vol}(Y,\gamma_{0})^{\frac{4+k}{8}} \left(\frac{\mathrm{Vol}(Y,\gamma_{0})}{\mathrm{Vol}(Y,\omega_{\eta})}\right)^{-\frac{5}{32}k} \exp\left[ -\frac{16-k}{32}\log\left(\frac{\mathrm{Vol}(Y,\gamma_{0})}{\mathrm{Vol}(Y,\omega_{\eta})}\right) \right] \\ &= C(k)^{-1}\tau_{M}(\widetilde{X},\theta)^{\frac{1}{2}}\mathrm{Vol}(Y,\omega_{\eta})^{\frac{4+k}{8}}, \end{aligned} \end{align*} $$
where we used the second assertion of Proposition 5.1 to get the second equality. This proves the result.
Theorem 8.4. Let
![]() $\gamma $
be a Kähler form on Y in the sense of orbifolds, and let
$\gamma $
be a Kähler form on Y in the sense of orbifolds, and let
![]() $\varXi \in H^{0}(Y,K_{Y}^{\otimes 2})\setminus \{0\}$
be a nowhere vanishing bicanonical form on Y. Then
$\varXi \in H^{0}(Y,K_{Y}^{\otimes 2})\setminus \{0\}$
be a nowhere vanishing bicanonical form on Y. Then
 $$ \begin{align*}\begin{aligned} \tau_{k}(Y) &:= \tau(Y,\gamma)\mathrm{Vol}(Y,\gamma)\,\|\varXi\|_{L^{1}(Y)}^{-\frac{4+k}{8}} \left\{ \prod_{{\frak p}\in\mathrm{Sing}(Y)}\left(\frac{\gamma^{2}/2!}{|\varXi|}\right)({\frak p}) \right\}^{\frac{5}{32}} \\ &\quad\times \exp \left[ \frac{1}{24}\int_{Y} \log\left(\frac{|\varXi|}{\gamma^{2}/2!}\right)\,c_{2}(Y,\gamma) \right] \end{aligned} \end{align*} $$
$$ \begin{align*}\begin{aligned} \tau_{k}(Y) &:= \tau(Y,\gamma)\mathrm{Vol}(Y,\gamma)\,\|\varXi\|_{L^{1}(Y)}^{-\frac{4+k}{8}} \left\{ \prod_{{\frak p}\in\mathrm{Sing}(Y)}\left(\frac{\gamma^{2}/2!}{|\varXi|}\right)({\frak p}) \right\}^{\frac{5}{32}} \\ &\quad\times \exp \left[ \frac{1}{24}\int_{Y} \log\left(\frac{|\varXi|}{\gamma^{2}/2!}\right)\,c_{2}(Y,\gamma) \right] \end{aligned} \end{align*} $$
is independent of the choices of
![]() $\gamma $
and
$\gamma $
and
![]() $\varXi $
, where
$\varXi $
, where
![]() $|\varXi |:=\sqrt {\varXi \otimes \overline {\varXi }}$
is the Ricci-flat volume form on Y induced by
$|\varXi |:=\sqrt {\varXi \otimes \overline {\varXi }}$
is the Ricci-flat volume form on Y induced by
![]() $\varXi $
. In fact,
$\varXi $
. In fact,
Proof. Let
![]() $\omega $
be a Ricci-flat Kähler form on Y in the sense of orbifolds such that
$\omega $
be a Ricci-flat Kähler form on Y in the sense of orbifolds such that
![]() $\omega ^{2}/2!=|\varXi |$
. Since
$\omega ^{2}/2!=|\varXi |$
. Since
![]() $\mathrm {Vol}(Y,\omega )=\|\varXi \|_{L^{1}(Y)}$
, we get by Theorem 8.3
$\mathrm {Vol}(Y,\omega )=\|\varXi \|_{L^{1}(Y)}$
, we get by Theorem 8.3
Let
![]() $\xi \in H^{0}(\widetilde {X},K_{\widetilde {X}})$
be a nowhere vanishing holomorphic
$\xi \in H^{0}(\widetilde {X},K_{\widetilde {X}})$
be a nowhere vanishing holomorphic
![]() $2$
-form on
$2$
-form on
![]() $\widetilde {X}$
such that
$\widetilde {X}$
such that
![]() $(p\circ \pi )^{*}\varXi =\xi ^{\otimes 2}$
. Since
$(p\circ \pi )^{*}\varXi =\xi ^{\otimes 2}$
. Since
![]() $\omega =\omega _{\xi }$
: that is,
$\omega =\omega _{\xi }$
: that is,
![]() $\omega ^{2}/2!=\xi \wedge \overline {\xi }=|\varXi |$
, we get by Theorem 8.2
$\omega ^{2}/2!=\xi \wedge \overline {\xi }=|\varXi |$
, we get by Theorem 8.2
 $$ \begin{align} \frac{\tau(Y,\gamma)\mathrm{Vol}(Y,\gamma)}{\tau(Y,\omega)\mathrm{Vol}(Y,\omega)} = \left\{ \prod_{{\frak p}\in\mathrm{Sing}(Y)}\left(\frac{|\varXi|}{\gamma^{2}/2!}\right)({\frak p}) \right\}^{-\frac{5}{32}} \exp \left[ -\frac{1}{24}\int_{Y} \log\left(\frac{|\varXi|}{\gamma^{2}/2!}\right)\,c_{2}(Y,\gamma) \right]. \end{align} $$
$$ \begin{align} \frac{\tau(Y,\gamma)\mathrm{Vol}(Y,\gamma)}{\tau(Y,\omega)\mathrm{Vol}(Y,\omega)} = \left\{ \prod_{{\frak p}\in\mathrm{Sing}(Y)}\left(\frac{|\varXi|}{\gamma^{2}/2!}\right)({\frak p}) \right\}^{-\frac{5}{32}} \exp \left[ -\frac{1}{24}\int_{Y} \log\left(\frac{|\varXi|}{\gamma^{2}/2!}\right)\,c_{2}(Y,\gamma) \right]. \end{align} $$
Comparing equation (8.5) and equation (8.6), we get
 $$ \begin{align} \begin{aligned} \tau(Y,\gamma)\mathrm{Vol}(Y,\gamma) &= C(k)^{-1}\tau_{M}(\widetilde{X},\theta)^{\frac{1}{2}}\,\|\varXi\|_{L^{1}(Y)}^{\frac{4+k}{8}} \left\{ \prod_{{\frak p}\in\mathrm{Sing}(Y)}\left(\frac{|\varXi|}{\gamma^{2}/2!}\right)({\frak p}) \right\}^{-\frac{5}{32}} \\ &\quad\times \exp \left[ -\frac{1}{24}\int_{Y} \log\left(\frac{|\varXi|}{\gamma^{2}/2!}\right)\,c_{2}(Y,\gamma) \right]. \end{aligned} \end{align} $$
$$ \begin{align} \begin{aligned} \tau(Y,\gamma)\mathrm{Vol}(Y,\gamma) &= C(k)^{-1}\tau_{M}(\widetilde{X},\theta)^{\frac{1}{2}}\,\|\varXi\|_{L^{1}(Y)}^{\frac{4+k}{8}} \left\{ \prod_{{\frak p}\in\mathrm{Sing}(Y)}\left(\frac{|\varXi|}{\gamma^{2}/2!}\right)({\frak p}) \right\}^{-\frac{5}{32}} \\ &\quad\times \exp \left[ -\frac{1}{24}\int_{Y} \log\left(\frac{|\varXi|}{\gamma^{2}/2!}\right)\,c_{2}(Y,\gamma) \right]. \end{aligned} \end{align} $$
From equation (8.7), we get
![]() $\tau _{k}(Y)=C(k)^{-1}\tau _{M}(\widetilde {X},\theta )^{1/2}$
. Since the right-hand side is independent of the choices of
$\tau _{k}(Y)=C(k)^{-1}\tau _{M}(\widetilde {X},\theta )^{1/2}$
. Since the right-hand side is independent of the choices of
![]() $\gamma $
and
$\gamma $
and
![]() $\varXi $
, so is
$\varXi $
, so is
![]() $\tau _{k}(Y)$
. This completes the proof.
$\tau _{k}(Y)$
. This completes the proof.
8.2 Del Pezzo surfaces and an explicit formula for the invariant
 $\tau _{k}$
$\tau _{k}$
In this subsection, we give an explicit formula for
![]() $\tau _{k}$
as an automorphic function on the Kähler moduli of Del Pezzo surfaces. Let
$\tau _{k}$
as an automorphic function on the Kähler moduli of Del Pezzo surfaces. Let
![]() $1\leq k\leq 9$
. We define the unimodular Lorentzian lattices
$1\leq k\leq 9$
. We define the unimodular Lorentzian lattices
![]() $L_{k}$
and
$L_{k}$
and
![]() ${\Bbb U}(-1)$
as
${\Bbb U}(-1)$
as
 $$ \begin{align*}L_{k}:=\begin{pmatrix}1&0\\0&-I_{9-k}\end{pmatrix} \quad(k\not=8), \qquad L_{8} := \begin{pmatrix}1&0\\0&-1\end{pmatrix}\mbox{ or }\begin{pmatrix}0&1\\1&0\end{pmatrix}, \end{align*} $$
$$ \begin{align*}L_{k}:=\begin{pmatrix}1&0\\0&-I_{9-k}\end{pmatrix} \quad(k\not=8), \qquad L_{8} := \begin{pmatrix}1&0\\0&-1\end{pmatrix}\mbox{ or }\begin{pmatrix}0&1\\1&0\end{pmatrix}, \end{align*} $$
 $$ \begin{align*}{\Bbb U}(-1):=\begin{pmatrix} 0&-1 \\ -1&0 \end{pmatrix}. \end{align*} $$
$$ \begin{align*}{\Bbb U}(-1):=\begin{pmatrix} 0&-1 \\ -1&0 \end{pmatrix}. \end{align*} $$
We fix an isometry of lattices
![]() $\Lambda _{k}\cong {\Bbb U}(-1)\oplus L_{k}$
and identify
$\Lambda _{k}\cong {\Bbb U}(-1)\oplus L_{k}$
and identify
![]() $\Lambda _{k}$
with
$\Lambda _{k}$
with
![]() ${\Bbb U}(-1)\oplus L_{k}$
.
${\Bbb U}(-1)\oplus L_{k}$
.
Let V be a Del Pezzo surface of degree k: that is,
Then
![]() $V = \mathrm {Bl}_{9-k}( {\mathbf P}^{2} )$
is the blowing-up of
$V = \mathrm {Bl}_{9-k}( {\mathbf P}^{2} )$
is the blowing-up of
![]() ${\mathbf P}^{2}$
at
${\mathbf P}^{2}$
at
![]() $9-k$
points in general position when
$9-k$
points in general position when
![]() $k\not =8$
. When
$k\not =8$
. When
![]() $k=8$
,
$k=8$
,
![]() $V\cong \Sigma _{0}$
or
$V\cong \Sigma _{0}$
or
![]() $\Sigma _{1}$
, where
$\Sigma _{1}$
, where
![]() $\Sigma _{n} = {\mathbf P}( {\mathcal O}_{{\mathbf P}^{1}} \oplus {\mathcal O}_{{\mathbf P}^{1}}(n) )$
is the Hirzebruch surface. Notice that
$\Sigma _{n} = {\mathbf P}( {\mathcal O}_{{\mathbf P}^{1}} \oplus {\mathcal O}_{{\mathbf P}^{1}}(n) )$
is the Hirzebruch surface. Notice that
![]() $\Sigma _{0}={\mathbf P}^{1} \times {\mathbf P}^{1}$
and
$\Sigma _{0}={\mathbf P}^{1} \times {\mathbf P}^{1}$
and
![]() $\Sigma _{1}=\mathrm {Bl}_{1}( {\mathbf P}^{2} )$
. When
$\Sigma _{1}=\mathrm {Bl}_{1}( {\mathbf P}^{2} )$
. When
![]() $V\not \cong \Sigma _{0}$
,
$V\not \cong \Sigma _{0}$
,
![]() $H^{2}(V, {\mathbf Z})$
endowed with the cup product pairing is isometric to
$H^{2}(V, {\mathbf Z})$
endowed with the cup product pairing is isometric to
![]() $L_{k}$
by identifying
$L_{k}$
by identifying
![]() $H, E_{1},\ldots ,E_{9-k}$
with the standard basis of
$H, E_{1},\ldots ,E_{9-k}$
with the standard basis of
![]() $L_{k}$
, where
$L_{k}$
, where
![]() $H \in H^{2}(V, {\mathbf Z})$
is the class obtained from the hyperplane class of
$H \in H^{2}(V, {\mathbf Z})$
is the class obtained from the hyperplane class of
![]() $H^{2}({\mathbf P}^{2}, {\mathbf Z})$
and
$H^{2}({\mathbf P}^{2}, {\mathbf Z})$
and
![]() $E_{i} (i=1,\ldots ,9-k)$
are the classes of exceptional divisors. Similarly,
$E_{i} (i=1,\ldots ,9-k)$
are the classes of exceptional divisors. Similarly,
![]() $H(V, {\mathbf Z})$
endowed with the Mukai pairing is isometric to
$H(V, {\mathbf Z})$
endowed with the Mukai pairing is isometric to
![]() $\Lambda _{k}$
. In what follows, we identify
$\Lambda _{k}$
. In what follows, we identify
![]() $L_{k}$
(respectively,
$L_{k}$
(respectively,
![]() $\Lambda _{k}$
) with
$\Lambda _{k}$
) with
![]() $H^{2}(V, {\mathbf Z})$
(respectively,
$H^{2}(V, {\mathbf Z})$
(respectively,
![]() $H(V, {\mathbf Z})$
) in this way.
$H(V, {\mathbf Z})$
) in this way.
Recall that the type IV domain
![]() $\Omega _{k}$
associated with
$\Omega _{k}$
associated with
![]() $\Lambda _{k}$
was defined in Section 2.4. We identify
$\Lambda _{k}$
was defined in Section 2.4. We identify
![]() $\Omega _{H(V, {\mathbf Z})}$
with the tube domain
$\Omega _{H(V, {\mathbf Z})}$
with the tube domain
![]() $H^{2}(V, {\mathbf Z})\otimes {\mathbf R}+i\,{\mathcal C}_{H^{2}(V, {\mathbf Z})} \subset H^{2}(V, {\mathbf C})$
via the map
$H^{2}(V, {\mathbf Z})\otimes {\mathbf R}+i\,{\mathcal C}_{H^{2}(V, {\mathbf Z})} \subset H^{2}(V, {\mathbf C})$
via the map
where
![]() ${\mathcal C}_{H^{2}(V, {\mathbf Z})}:=\{v\in H^{2}(V, {\mathbf R}); \, v^{2}>0 \}$
is the positive cone of
${\mathcal C}_{H^{2}(V, {\mathbf Z})}:=\{v\in H^{2}(V, {\mathbf R}); \, v^{2}>0 \}$
is the positive cone of
![]() $H^{2}(V, {\mathbf R})$
. Through the isomorphism given by equation (8.8),
$H^{2}(V, {\mathbf R})$
. Through the isomorphism given by equation (8.8),
![]() $O(H(V,{\mathbf Z}))$
acts on
$O(H(V,{\mathbf Z}))$
acts on
![]() $H^{2}(V, {\mathbf R})+i\,{\mathcal C}_{H^{2}(V, {\mathbf Z})}$
.
$H^{2}(V, {\mathbf R})+i\,{\mathcal C}_{H^{2}(V, {\mathbf Z})}$
.
Let
![]() ${\mathcal K}_{V} \subset {\mathcal C}_{H^{2}(V, {\mathbf Z})}$
be the Kähler cone of V: that is, the cone of
${\mathcal K}_{V} \subset {\mathcal C}_{H^{2}(V, {\mathbf Z})}$
be the Kähler cone of V: that is, the cone of
![]() $H^{2}(V, {\mathbf R})$
consisting of Kähler classes on V. Let
$H^{2}(V, {\mathbf R})$
consisting of Kähler classes on V. Let
![]() $\mathrm {Eff}(V) \subset H^{2}(V, {\mathbf R})$
be the effective cone of V: that is, the dual cone of the Kähler cone
$\mathrm {Eff}(V) \subset H^{2}(V, {\mathbf R})$
be the effective cone of V: that is, the dual cone of the Kähler cone
![]() ${\mathcal K}_{V}$
.
${\mathcal K}_{V}$
.
Definition 8.5. Define the infinite product
![]() $\Phi _{V}(z)$
on
$\Phi _{V}(z)$
on
![]() $H^{2}(V, {\mathbf Z})\otimes \mathbf {R}+i\,{\mathcal K}_{V}$
by
$H^{2}(V, {\mathbf Z})\otimes \mathbf {R}+i\,{\mathcal K}_{V}$
by
 $$ \begin{align*}\begin{aligned} \Phi_{V}(z) &:= e^{\pi i\langle c_{1}(V), z\rangle} \prod_{\alpha\in \mathrm{Eff}(V)} (1-e^{2\pi i\langle\alpha,z\rangle})^{c_{k}^{(0)}(\alpha^{2})} \\ &\qquad\times \prod_{\beta\in \mathrm{Eff}(V),\,\, \beta/2\equiv c_{1}(V)/2\mod H^{2}(V, {\mathbf Z})} (1-e^{\pi i\langle\beta,z\rangle})^{c_{k}^{(1)}(\beta^{2}/4)}, \end{aligned} \end{align*} $$
$$ \begin{align*}\begin{aligned} \Phi_{V}(z) &:= e^{\pi i\langle c_{1}(V), z\rangle} \prod_{\alpha\in \mathrm{Eff}(V)} (1-e^{2\pi i\langle\alpha,z\rangle})^{c_{k}^{(0)}(\alpha^{2})} \\ &\qquad\times \prod_{\beta\in \mathrm{Eff}(V),\,\, \beta/2\equiv c_{1}(V)/2\mod H^{2}(V, {\mathbf Z})} (1-e^{\pi i\langle\beta,z\rangle})^{c_{k}^{(1)}(\beta^{2}/4)}, \end{aligned} \end{align*} $$
where
![]() $\{c_{k}^{(0)}(l)\}_{l\in \mathbf {Z}}$
,
$\{c_{k}^{(0)}(l)\}_{l\in \mathbf {Z}}$
,
![]() $\{c_{k}^{(1)}(l)\}_{l\in \mathbf {Z}+k/4}$
are defined by the generating functions
$\{c_{k}^{(1)}(l)\}_{l\in \mathbf {Z}+k/4}$
are defined by the generating functions
 $$ \begin{align*}\sum_{l\in\mathbf{Z}}c_{k}^{(0)}(l)\,q^{l} = \frac{\eta(2\tau)^{8}\theta_{{\Bbb A}_{1}}(\tau)^{k}}{\eta(\tau)^{8}\eta(4\tau)^{8}}, \qquad \sum_{l\in\frac{k}{4}+\mathbf{Z}}c_{k}^{(1)}(l)\,q^{l} = -8\,\frac{\eta(4\tau)^{8}\theta_{{\Bbb A}_{1}+1/2}(\tau)^{k}}{\eta(2\tau)^{16}}. \end{align*} $$
$$ \begin{align*}\sum_{l\in\mathbf{Z}}c_{k}^{(0)}(l)\,q^{l} = \frac{\eta(2\tau)^{8}\theta_{{\Bbb A}_{1}}(\tau)^{k}}{\eta(\tau)^{8}\eta(4\tau)^{8}}, \qquad \sum_{l\in\frac{k}{4}+\mathbf{Z}}c_{k}^{(1)}(l)\,q^{l} = -8\,\frac{\eta(4\tau)^{8}\theta_{{\Bbb A}_{1}+1/2}(\tau)^{k}}{\eta(2\tau)^{16}}. \end{align*} $$
Here
![]() $\theta _{{\Bbb A}_{1}+\epsilon /2}(\tau ):=\sum _{n\in \mathbf {Z}}q^{(n+\epsilon /2)^{2}}$
and
$\theta _{{\Bbb A}_{1}+\epsilon /2}(\tau ):=\sum _{n\in \mathbf {Z}}q^{(n+\epsilon /2)^{2}}$
and
![]() $\eta (\tau ):=q^{1/24}\prod _{n>0}(1-q^{n})$
.
$\eta (\tau ):=q^{1/24}\prod _{n>0}(1-q^{n})$
.
Let
![]() ${\mathcal C}_{H^{2}(V, {\mathbf Z})}^{+}$
be the connected component of
${\mathcal C}_{H^{2}(V, {\mathbf Z})}^{+}$
be the connected component of
![]() ${\mathcal C}_{H^{2}(V, {\mathbf Z})}$
that contains
${\mathcal C}_{H^{2}(V, {\mathbf Z})}$
that contains
![]() ${\mathcal K}_{V}$
, and let
${\mathcal K}_{V}$
, and let
![]() $\Omega _{H(V, {\mathbf Z})}^{+}$
be the component of
$\Omega _{H(V, {\mathbf Z})}^{+}$
be the component of
![]() $\Omega _{H(V, {\mathbf Z})}$
corresponding to
$\Omega _{H(V, {\mathbf Z})}$
corresponding to
![]() $H^{2}(V, {\mathbf R}) + i\,{\mathcal C}_{H^{2}(V, {\mathbf Z})}^{+}$
via the isomorphism given by equation (8.8). By Borcherds [Reference Borcherds8, Th. 13.3] (compare [Reference Yoshikawa44]),
$H^{2}(V, {\mathbf R}) + i\,{\mathcal C}_{H^{2}(V, {\mathbf Z})}^{+}$
via the isomorphism given by equation (8.8). By Borcherds [Reference Borcherds8, Th. 13.3] (compare [Reference Yoshikawa44]),
![]() $\Phi _{V}(z)$
converges absolutely for those
$\Phi _{V}(z)$
converges absolutely for those
![]() $z\in H^{2}(V, {\mathbf R}) + i\,{\mathcal K}_{V}$
with
$z\in H^{2}(V, {\mathbf R}) + i\,{\mathcal K}_{V}$
with
![]() $\Im z\gg 0$
and extends to an automorphic form on
$\Im z\gg 0$
and extends to an automorphic form on
![]() $\Omega _{H(V, {\mathbf Z})}^{+}$
for
$\Omega _{H(V, {\mathbf Z})}^{+}$
for
![]() $O^{+}(H(V, {\mathbf Z}))$
of weight
$O^{+}(H(V, {\mathbf Z}))$
of weight
![]() $\deg V+4$
with zero divisor
$\deg V+4$
with zero divisor
![]() $\mathrm {div}(\Phi _{V})=\sum _{d\in H(V, {\mathbf Z}),\,d^{2}=-1}d^{\perp }$
under the identification
$\mathrm {div}(\Phi _{V})=\sum _{d\in H(V, {\mathbf Z}),\,d^{2}=-1}d^{\perp }$
under the identification
![]() $H^{2}(V, {\mathbf R}) + i\,{\mathcal C}_{H^{2}(V, {\mathbf Z})}^{+} \cong \Omega _{H(V, {\mathbf Z})}^{+}$
.
$H^{2}(V, {\mathbf R}) + i\,{\mathcal C}_{H^{2}(V, {\mathbf Z})}^{+} \cong \Omega _{H(V, {\mathbf Z})}^{+}$
.
Recently, an explicit Fourier series expansion of
![]() $\Phi _{V}(z)$
is discovered by Gritsenko [Reference Gritsenko22, Cor. 5.1]. It is also remarkable that
$\Phi _{V}(z)$
is discovered by Gritsenko [Reference Gritsenko22, Cor. 5.1]. It is also remarkable that
![]() $\Phi _{V}$
is the denominator function of a generalised Kac-Moody algebra, whose real and imaginary simple roots are explicitly given by the Fourier series expansion of
$\Phi _{V}$
is the denominator function of a generalised Kac-Moody algebra, whose real and imaginary simple roots are explicitly given by the Fourier series expansion of
![]() $\Phi _{V}$
[Reference Gritsenko and Nikulin23, §6.2, Th. 6.1 Eq. (6.1), (6.10)]. In this sense, the series of Borcherds products
$\Phi _{V}$
[Reference Gritsenko and Nikulin23, §6.2, Th. 6.1 Eq. (6.1), (6.10)]. In this sense, the series of Borcherds products
![]() $\Phi _{V}$
associated to Del Pezzo surfaces is quite analogous to the Borcherds
$\Phi _{V}$
associated to Del Pezzo surfaces is quite analogous to the Borcherds
![]() $\Phi $
-function of rank
$\Phi $
-function of rank
![]() $10$
.
$10$
.
We define the Petersson norm of
![]() $\Phi _{V}(z)$
by
$\Phi _{V}(z)$
by
where
![]() $z\in H^{2}(V, {\mathbf R}) + i\,{\mathcal C}_{H^{2}(V, {\mathbf Z})}^{+}$
. Then
$z\in H^{2}(V, {\mathbf R}) + i\,{\mathcal C}_{H^{2}(V, {\mathbf Z})}^{+}$
. Then
![]() $\|\Phi _{V}\|^{2}$
is an
$\|\Phi _{V}\|^{2}$
is an
![]() $O^{+}(H(V, {\mathbf Z}))$
-invariant
$O^{+}(H(V, {\mathbf Z}))$
-invariant
![]() $C^{\infty }$
function on
$C^{\infty }$
function on
![]() $\Omega _{H(V, {\mathbf Z})}^{+}$
. Hence
$\Omega _{H(V, {\mathbf Z})}^{+}$
. Hence
![]() $\|\Phi _{V}\|^{2}$
is identified with a
$\|\Phi _{V}\|^{2}$
is identified with a
![]() $C^{\infty }$
function on
$C^{\infty }$
function on
![]() ${\mathcal M}_{\deg V}$
in the sense of orbifolds.
${\mathcal M}_{\deg V}$
in the sense of orbifolds.
Theorem 8.6. Let
![]() $1\leq k\leq 9$
. There exists a constant
$1\leq k\leq 9$
. There exists a constant
![]() $\widetilde {C}(k)>0$
depending only on k such that for every
$\widetilde {C}(k)>0$
depending only on k such that for every
![]() $2$
-elementary
$2$
-elementary
![]() $K3$
surface
$K3$
surface
![]() $(\widetilde {X},\theta )$
of type
$(\widetilde {X},\theta )$
of type
![]() $M_{k}:=\Lambda _{k}(2)^{\perp }$
,
$M_{k}:=\Lambda _{k}(2)^{\perp }$
,
where
![]() $k = \deg V$
.
$k = \deg V$
.
Proof. See [Reference Yoshikawa44, Th. 4.2 (1)] and [Reference Yoshikawa45, Th. 0.1].
Theorem 8.7. Let
![]() $1\leq k\leq 9$
. Then there exists a constant
$1\leq k\leq 9$
. Then there exists a constant
![]() $C_{k}>0$
depending only on k such that for every good log-Enriques surface Y with
$C_{k}>0$
depending only on k such that for every good log-Enriques surface Y with
![]() $\#\mathrm {Sing}(Y) = \deg V$
,
$\#\mathrm {Sing}(Y) = \deg V$
,
Proof. We set
![]() $k = \deg V$
. When
$k = \deg V$
. When
![]() $k=2$
, we define
$k=2$
, we define
![]() $V=\Sigma _{0}$
when Y is of even type and
$V=\Sigma _{0}$
when Y is of even type and
![]() $V=\Sigma _{1}$
when Y is of odd type. Let
$V=\Sigma _{1}$
when Y is of odd type. Let
![]() $(\widetilde {X},\theta )$
be the
$(\widetilde {X},\theta )$
be the
![]() $2$
-elementary
$2$
-elementary
![]() $K3$
surface of type
$K3$
surface of type
![]() $M_{k}$
associated to Y. By the definition of the period of Y, we have
$M_{k}$
associated to Y. By the definition of the period of Y, we have
![]() $\overline {\varpi }(Y)=\overline {\varpi }(\widetilde {X},\theta )$
. Hence
$\overline {\varpi }(Y)=\overline {\varpi }(\widetilde {X},\theta )$
. Hence
By Theorems 8.4, 8.6 and equation (8.9), we get
 $$ \begin{align} \begin{aligned} \tau_{k}(Y) &= C(k)^{-1}\tau_{M_{k}}(\widetilde{X},\theta)^{1/2} = C(k)^{-1}\widetilde{C}(k)\,\|\Phi_{V}(\overline{\varpi}(\widetilde{X},\theta))\|^{-1/4} \\ &= C(k)^{-1}\widetilde{C}(k)\,\left\|\Phi_{V}\left(\overline{\varpi}(Y)\right)\right\|^{-1/4}. \end{aligned} \end{align} $$
$$ \begin{align} \begin{aligned} \tau_{k}(Y) &= C(k)^{-1}\tau_{M_{k}}(\widetilde{X},\theta)^{1/2} = C(k)^{-1}\widetilde{C}(k)\,\|\Phi_{V}(\overline{\varpi}(\widetilde{X},\theta))\|^{-1/4} \\ &= C(k)^{-1}\widetilde{C}(k)\,\left\|\Phi_{V}\left(\overline{\varpi}(Y)\right)\right\|^{-1/4}. \end{aligned} \end{align} $$
Setting
![]() $C_{k}:=C(k)^{-1}\widetilde {C}(k)$
in equation (8.10), we get the result.
$C_{k}:=C(k)^{-1}\widetilde {C}(k)$
in equation (8.10), we get the result.
8.3 The quasi-pullback of
 $\Phi _{V}$
$\Phi _{V}$
We define the Kähler moduli of V by
Since
![]() $H(V,{\mathbf Z}) \cong \Lambda _{\deg V}$
, we have
$H(V,{\mathbf Z}) \cong \Lambda _{\deg V}$
, we have
![]() $\mathcal {KM}(V)={\mathcal M}_{\deg V}$
, where the orthogonal modular variety
$\mathcal {KM}(V)={\mathcal M}_{\deg V}$
, where the orthogonal modular variety
![]() ${\mathcal M}_{k}$
was defined in Section 2.4. Let
${\mathcal M}_{k}$
was defined in Section 2.4. Let
![]() $\pi \colon \widetilde {V}:=\mathrm {Bl}_{p}(V)\to V$
be the blow-up of V at p, and let
$\pi \colon \widetilde {V}:=\mathrm {Bl}_{p}(V)\to V$
be the blow-up of V at p, and let
![]() $E:=\pi ^{-1}(p)$
be the exceptional curve of
$E:=\pi ^{-1}(p)$
be the exceptional curve of
![]() $\pi $
. Then we have a map of cohomologies
$\pi $
. Then we have a map of cohomologies
![]() $\pi ^{*}\colon H(V,{\mathbf Z})\to H(\widetilde {V},{\mathbf Z})$
, which induces the canonical identification
$\pi ^{*}\colon H(V,{\mathbf Z})\to H(\widetilde {V},{\mathbf Z})$
, which induces the canonical identification
Since
![]() $[E]$
is a norm
$[E]$
is a norm
![]() $(-1)$
-vector of
$(-1)$
-vector of
![]() $H^{2}(\widetilde {V}, {\mathbf Z})$
, this implies that
$H^{2}(\widetilde {V}, {\mathbf Z})$
, this implies that
![]() $\mathcal {KM}(V)$
is identified with a component of the Heegner divisor of norm
$\mathcal {KM}(V)$
is identified with a component of the Heegner divisor of norm
![]() $(-1)$
-vectors of
$(-1)$
-vectors of
![]() $\mathcal {KM}(\widetilde {V})$
. Since
$\mathcal {KM}(\widetilde {V})$
. Since
![]() $O(H(\widetilde {V}, {\mathbf Z}))$
acts transitively on the norm
$O(H(\widetilde {V}, {\mathbf Z}))$
acts transitively on the norm
![]() $(-1)$
-vectors of
$(-1)$
-vectors of
![]() $H(\widetilde {V}, {\mathbf Z})$
except the case
$H(\widetilde {V}, {\mathbf Z})$
except the case
![]() $\deg \widetilde {V} = 7$
– that is,
$\deg \widetilde {V} = 7$
– that is,
![]() $H(\widetilde {V}, {\mathbf Z}) \cong {\mathbb U}^{\oplus 2} \oplus \langle -1 \rangle $
–
$H(\widetilde {V}, {\mathbf Z}) \cong {\mathbb U}^{\oplus 2} \oplus \langle -1 \rangle $
–
![]() $\mathcal {KM}(V)$
coincides with the Heegner divisor of norm
$\mathcal {KM}(V)$
coincides with the Heegner divisor of norm
![]() $(-1)$
-vectors of
$(-1)$
-vectors of
![]() $\mathcal {KM}(\widetilde {V})$
when
$\mathcal {KM}(\widetilde {V})$
when
![]() $\deg V \not =7$
. When
$\deg V \not =7$
. When
![]() $\deg V=7$
, the Heegner divisor of norm
$\deg V=7$
, the Heegner divisor of norm
![]() $(-1)$
-vectors of
$(-1)$
-vectors of
![]() $\mathcal {KM}(\widetilde {V})$
consists of two components; one is given by
$\mathcal {KM}(\widetilde {V})$
consists of two components; one is given by
![]() $\mathcal {KM}(\varSigma _{0})$
, and the other is given by
$\mathcal {KM}(\varSigma _{0})$
, and the other is given by
![]() $\mathcal {KM}(\varSigma _{1})$
, where
$\mathcal {KM}(\varSigma _{1})$
, where
![]() $\varSigma _{n} = {\mathbf P}( {\mathcal O}_{{\mathbf P}^{1}} \oplus {\mathcal O}_{{\mathbf P}^{1}}(n) )$
is the Hirzebruch surface. In the following theorem, we use the convention that a Del Pezzo surface of degree
$\varSigma _{n} = {\mathbf P}( {\mathcal O}_{{\mathbf P}^{1}} \oplus {\mathcal O}_{{\mathbf P}^{1}}(n) )$
is the Hirzebruch surface. In the following theorem, we use the convention that a Del Pezzo surface of degree
![]() $0$
is an Enriques surface.
$0$
is an Enriques surface.
Theorem 8.8.
![]() $\Phi _{V}$
is the quasi-pullback of
$\Phi _{V}$
is the quasi-pullback of
![]() $\Phi _{\widetilde {V}}$
to
$\Phi _{\widetilde {V}}$
to
![]() $\mathcal {KM}(V) = [E]^{\perp }$
, up to a constant. Namely, in the infinite product expression in Definition 8.5, the following equality holds:
$\mathcal {KM}(V) = [E]^{\perp }$
, up to a constant. Namely, in the infinite product expression in Definition 8.5, the following equality holds:
 $$ \begin{align*}\Phi_{V} = \mathrm{Const}.\, \left. \frac{\Phi_{\widetilde{V}}(\cdot)}{\langle \cdot, [E]\rangle} \right|_{[E]^{\perp}}, \end{align*} $$
$$ \begin{align*}\Phi_{V} = \mathrm{Const}.\, \left. \frac{\Phi_{\widetilde{V}}(\cdot)}{\langle \cdot, [E]\rangle} \right|_{[E]^{\perp}}, \end{align*} $$
where
![]() $\langle z, [E] \rangle $
is the linear form on
$\langle z, [E] \rangle $
is the linear form on
![]() $H^{2}(\widetilde {V}, {\mathbf C})$
defined by the norm
$H^{2}(\widetilde {V}, {\mathbf C})$
defined by the norm
![]() $(-1)$
-vector
$(-1)$
-vector
![]() $[E]$
.
$[E]$
.
Proof. The result is a special case of [Reference Ma29, Th. 1.1]. See also [Reference Ma29, Example 3.17].
This theorem can be summarised in the following diagrams:
 $$ \begin{align*}\begin{array}{lllllllllll} \mathcal{KM}(\mathrm{Enr}) &\supset &\mathcal{KM}(\mathrm{dP}_{1}) &\supset &\cdots &\supset &\mathcal{KM}(\mathrm{dP}_{7}) &\supset &\mathcal{KM}(\Sigma_{1}) &\supset &\mathcal{KM}({\mathbf P}^{2}) \\ \Phi_{\mathrm{Enr}} & \to & \Phi_{\mathrm{dP}_{1}} & \to & \cdots & \to & \Phi_{\mathrm{dP}_{7}} & \to & \Phi_{\Sigma_{1}} & \to & \Phi_{{\mathbf P}^{2}} \\ \eta_{1^{-8}2^{8}4^{-8}} & \to & \eta_{1^{-8}2^{8}4^{-8}} \theta & \to & \cdots & \to & \eta_{1^{-8}2^{8}4^{-8}} \theta^{7} & \to & \eta_{1^{-8}2^{8}4^{-8}} \theta^{8} & \to & \eta_{1^{-8}2^{8}4^{-8}} \theta^{9} \end{array} \end{align*} $$
$$ \begin{align*}\begin{array}{lllllllllll} \mathcal{KM}(\mathrm{Enr}) &\supset &\mathcal{KM}(\mathrm{dP}_{1}) &\supset &\cdots &\supset &\mathcal{KM}(\mathrm{dP}_{7}) &\supset &\mathcal{KM}(\Sigma_{1}) &\supset &\mathcal{KM}({\mathbf P}^{2}) \\ \Phi_{\mathrm{Enr}} & \to & \Phi_{\mathrm{dP}_{1}} & \to & \cdots & \to & \Phi_{\mathrm{dP}_{7}} & \to & \Phi_{\Sigma_{1}} & \to & \Phi_{{\mathbf P}^{2}} \\ \eta_{1^{-8}2^{8}4^{-8}} & \to & \eta_{1^{-8}2^{8}4^{-8}} \theta & \to & \cdots & \to & \eta_{1^{-8}2^{8}4^{-8}} \theta^{7} & \to & \eta_{1^{-8}2^{8}4^{-8}} \theta^{8} & \to & \eta_{1^{-8}2^{8}4^{-8}} \theta^{9} \end{array} \end{align*} $$
and
 $$ \begin{align*}\begin{array}{lllllllll} \mathcal{KM}(\mathrm{dP}_{7}) &\supset &\mathcal{KM}(\Sigma_{0}) \\ \Phi_{\mathrm{dP}_{7}} & \to & \Phi_{\Sigma_{0}} \\ \eta_{1^{-8}2^{8}4^{-8}} \theta^{7} & \to & \eta_{1^{-8}2^{8}4^{-8}} \theta^{8} \end{array} \end{align*} $$
$$ \begin{align*}\begin{array}{lllllllll} \mathcal{KM}(\mathrm{dP}_{7}) &\supset &\mathcal{KM}(\Sigma_{0}) \\ \Phi_{\mathrm{dP}_{7}} & \to & \Phi_{\Sigma_{0}} \\ \eta_{1^{-8}2^{8}4^{-8}} \theta^{7} & \to & \eta_{1^{-8}2^{8}4^{-8}} \theta^{8} \end{array} \end{align*} $$
where the inclusion implies the embedding as the discriminant divisor, the arrow in the second line implies the quasi-pullback (up to a constant), and the arrow in the third line describes the change of elliptic modular form for
![]() $\Gamma _{0}(4)$
corresponding to
$\Gamma _{0}(4)$
corresponding to
![]() $\Phi _{V}$
. We remark that there are no inclusions of
$\Phi _{V}$
. We remark that there are no inclusions of
![]() $\mathcal {KM}({\mathbf P}^{2})$
into
$\mathcal {KM}({\mathbf P}^{2})$
into
![]() $\mathcal {KM}(\Sigma _{0})$
.
$\mathcal {KM}(\Sigma _{0})$
.
9 The invariant
 $\tau _{k}$
and the BCOV invariant
$\tau _{k}$
and the BCOV invariant
9.1 The BCOV invariant of log-Enriques surfaces
In this subsection, we prove that the invariant
![]() $\tau _{k}$
is viewed as the BCOV invariant of good log-Enriques surfaces. Recall that for a compact connected Kähler orbifold
$\tau _{k}$
is viewed as the BCOV invariant of good log-Enriques surfaces. Recall that for a compact connected Kähler orbifold
![]() $(V, \gamma )$
, the BCOV torsion
$(V, \gamma )$
, the BCOV torsion
![]() $T_{\mathrm {BCOV}}(V,\gamma )$
is defined as
$T_{\mathrm {BCOV}}(V,\gamma )$
is defined as
where
![]() $\zeta _{p,q}(s)$
is the spectral zeta function of the Laplacian
$\zeta _{p,q}(s)$
is the spectral zeta function of the Laplacian
![]() $\square _{p,q}$
acting on
$\square _{p,q}$
acting on
![]() $(p,q)$
-forms on V in the sense of orbifolds. As before, the analytic torsion of the trivial line bundle on V is denoted by
$(p,q)$
-forms on V in the sense of orbifolds. As before, the analytic torsion of the trivial line bundle on V is denoted by
![]() $\tau (V,\gamma )$
.
$\tau (V,\gamma )$
.
Lemma 9.1. If
![]() $\dim V = 2$
, then the following equality holds:
$\dim V = 2$
, then the following equality holds:
Proof. Since
![]() $\square _{p,q}$
and
$\square _{p,q}$
and
![]() $\square _{2-q,2-p}$
are isospectral via the Hodge
$\square _{2-q,2-p}$
are isospectral via the Hodge
![]() $*$
-operator, we have
$*$
-operator, we have
![]() $\zeta _{p,q}(s) = \zeta _{2-q,2-p}(s)$
. Since
$\zeta _{p,q}(s) = \zeta _{2-q,2-p}(s)$
. Since
![]() $\square _{p,q}$
and
$\square _{p,q}$
and
![]() $\square _{q,p}$
are isospectral via the complex conjugation, we have
$\square _{q,p}$
are isospectral via the complex conjugation, we have
![]() $\zeta _{p,q}(s)=\zeta _{q,p}(s)$
. Using these relations, we have
$\zeta _{p,q}(s)=\zeta _{q,p}(s)$
. Using these relations, we have
Since
![]() $\zeta _{0,0}(s) - \zeta _{0,1}(s) + \zeta _{0,2}(s) =0$
and
$\zeta _{0,0}(s) - \zeta _{0,1}(s) + \zeta _{0,2}(s) =0$
and
![]() $\zeta _{1,0}(s) - \zeta _{1,1}(s) + \zeta _{1,2}(s) =0$
, we have
$\zeta _{1,0}(s) - \zeta _{1,1}(s) + \zeta _{1,2}(s) =0$
, we have
![]() $4\zeta ^{\prime }_{0,0}(0) - 4\zeta ^{\prime }_{0,1}(0) = -4\zeta ^{\prime }_{0,2}(0)$
and
$4\zeta ^{\prime }_{0,0}(0) - 4\zeta ^{\prime }_{0,1}(0) = -4\zeta ^{\prime }_{0,2}(0)$
and
![]() $\zeta ^{\prime }_{1,1}(0) = \zeta ^{\prime }_{1,0}(0) + \zeta ^{\prime }_{1,2}(0) = \zeta ^{\prime }_{1,0}(0) + \zeta ^{\prime }_{0,1}(0) = 2\zeta ^{\prime }_{0,1}(0)$
. Substituting these into equation (9.1), we get the result.
$\zeta ^{\prime }_{1,1}(0) = \zeta ^{\prime }_{1,0}(0) + \zeta ^{\prime }_{1,2}(0) = \zeta ^{\prime }_{1,0}(0) + \zeta ^{\prime }_{0,1}(0) = 2\zeta ^{\prime }_{0,1}(0)$
. Substituting these into equation (9.1), we get the result.
Now we have the following:
Theorem 9.2. Let Y be a good log-Enriques surface with k singular points. Let
![]() $\gamma $
be a Kähler form on Y in the sense of orbifolds, and let
$\gamma $
be a Kähler form on Y in the sense of orbifolds, and let
![]() $\varXi \in H^{0}(Y,K_{Y}^{\otimes 2})\setminus \{0\}$
be a nowhere vanishing bicanonical form on Y. Then
$\varXi \in H^{0}(Y,K_{Y}^{\otimes 2})\setminus \{0\}$
be a nowhere vanishing bicanonical form on Y. Then
 $$ \begin{align*}\begin{aligned} \tau_{\mathrm{BCOV}}(Y) &:= T_{\mathrm{BCOV}}(Y,\gamma)\mathrm{Vol}(Y,\gamma)^{-2}\,\|\varXi\|_{L^{1}(Y)}^{\frac{4+k}{4}} \left\{ \prod_{{\frak p}\in\mathrm{Sing}(Y)}\left(\frac{\gamma^{2}/2!}{|\varXi|}\right)({\frak p}) \right\}^{-\frac{5}{16}} \\ &\quad\times \exp \left[ -\frac{1}{12}\int_{Y} \log\left(\frac{|\varXi|}{\gamma^{2}/2!}\right)\,c_{2}(Y,\gamma) \right] \end{aligned} \end{align*} $$
$$ \begin{align*}\begin{aligned} \tau_{\mathrm{BCOV}}(Y) &:= T_{\mathrm{BCOV}}(Y,\gamma)\mathrm{Vol}(Y,\gamma)^{-2}\,\|\varXi\|_{L^{1}(Y)}^{\frac{4+k}{4}} \left\{ \prod_{{\frak p}\in\mathrm{Sing}(Y)}\left(\frac{\gamma^{2}/2!}{|\varXi|}\right)({\frak p}) \right\}^{-\frac{5}{16}} \\ &\quad\times \exp \left[ -\frac{1}{12}\int_{Y} \log\left(\frac{|\varXi|}{\gamma^{2}/2!}\right)\,c_{2}(Y,\gamma) \right] \end{aligned} \end{align*} $$
is independent of the choices of
![]() $\gamma $
and
$\gamma $
and
![]() $\varXi $
. In fact,
$\varXi $
. In fact,
where
![]() $C_{k}$
is the same constant as in Theorem 8.7.
$C_{k}$
is the same constant as in Theorem 8.7.
Proof. Since
![]() $\tau _{\mathrm {BCOV}}(Y)=\tau _{k}(Y)^{-2}$
by Theorem 8.4 and Lemma 9.1, we get the first claim. The second claim follows from Theorem 8.7.
$\tau _{\mathrm {BCOV}}(Y)=\tau _{k}(Y)^{-2}$
by Theorem 8.4 and Lemma 9.1, we get the first claim. The second claim follows from Theorem 8.7.
We call
![]() $\tau _{\mathrm {BCOV}}(Y)$
the BCOV invariant of Y. When
$\tau _{\mathrm {BCOV}}(Y)$
the BCOV invariant of Y. When
![]() $\gamma $
is Ricci-flat and
$\gamma $
is Ricci-flat and
![]() $|\varXi | = \gamma ^{2}/2!$
, we have the following simple expression:
$|\varXi | = \gamma ^{2}/2!$
, we have the following simple expression:
As in the case of Enriques surfaces, the BCOV invariant of good log-Enriques surfaces is expressed by the Peterssion norm of a Borcherds product. In particular, the BCOV invariant of log-Enriques surfaces is not a birational invariant, for the birational equivalence classes of log-Enriques surfaces consist of a single class.
Problem 9.3. For a good log-Enriques surface Y, there exists a log-Enriques surface
![]() $Y'$
with a unique singular point admitting a birational morphism
$Y'$
with a unique singular point admitting a birational morphism
![]() $Y\to Y'$
(compare [Reference Zhang47]). In general, the singularity of
$Y\to Y'$
(compare [Reference Zhang47]). In general, the singularity of
![]() $Y'$
is worse than those of Y. Can one construct a holomorphic torsion invariant of
$Y'$
is worse than those of Y. Can one construct a holomorphic torsion invariant of
![]() $Y'$
using some ALE instanton instead of the Eguchi-Hanson instanton? If this is the case, compare the holomorphic torsion invariants between Y and
$Y'$
using some ALE instanton instead of the Eguchi-Hanson instanton? If this is the case, compare the holomorphic torsion invariants between Y and
![]() $Y'$
.
$Y'$
.
Problem 9.4. Let Y be a good log-Enriques surface. Let
![]() $p\colon \widetilde {Y}\to Y$
be a resolution such that
$p\colon \widetilde {Y}\to Y$
be a resolution such that
![]() $p^{-1}(\mathrm {Sing}\,Y)$
is a disjoint union of smooth
$p^{-1}(\mathrm {Sing}\,Y)$
is a disjoint union of smooth
![]() $(-4)$
-curves. Compare the BCOV invariant of Y and that of the pair
$(-4)$
-curves. Compare the BCOV invariant of Y and that of the pair
![]() $(\widetilde {Y}, p^{-1}(\mathrm {Sing}\,Y))$
defined by Zhang [Reference Zhang49].
$(\widetilde {Y}, p^{-1}(\mathrm {Sing}\,Y))$
defined by Zhang [Reference Zhang49].
Problem 9.5. Can one construct a holomorphic torsion invariant of log-Enriques surfaces with index
![]() $\geq 3$
and prove its automorphy?
$\geq 3$
and prove its automorphy?
9.2 The BCOV invariant of certain Borcea-Voisin type orbifolds
Let Y be a good log-Enriques surface with k singular points, and let X be the canonical double covering of Y. Then X is a nodal
![]() $K3$
surface with k nodes endowed with an anti-symplectic involution
$K3$
surface with k nodes endowed with an anti-symplectic involution
![]() $\iota $
with a fixed point set
$\iota $
with a fixed point set
![]() $\mathrm {Sing}\,X =\{ p_{1}, \ldots , p_{k} \}$
. Let T be an elliptic curve. We define
$\mathrm {Sing}\,X =\{ p_{1}, \ldots , p_{k} \}$
. Let T be an elliptic curve. We define
Then V is a Calabi-Yau orbifold of dimension
![]() $3$
. Let
$3$
. Let
![]() $\widetilde {V}$
be the Borcea-Voisin orbifold
$\widetilde {V}$
be the Borcea-Voisin orbifold
where
![]() $\pi \colon \widetilde {X} \to X$
is the minimal resolution of X and
$\pi \colon \widetilde {X} \to X$
is the minimal resolution of X and
![]() $\theta $
is the involution on
$\theta $
is the involution on
![]() $\widetilde {X}$
induced from
$\widetilde {X}$
induced from
![]() $\iota $
. As before, we set
$\iota $
. As before, we set
![]() $E_{i}:=\pi ^{-1}(p_{i}) \cong {\mathbf P}^{1}$
. The birational morphism from
$E_{i}:=\pi ^{-1}(p_{i}) \cong {\mathbf P}^{1}$
. The birational morphism from
![]() $\widetilde {V}$
to V induced by
$\widetilde {V}$
to V induced by
![]() $\pi $
is denoted again by
$\pi $
is denoted again by
![]() $\pi $
. Then
$\pi $
. Then
![]() $\pi \colon \widetilde {V} \to V$
is a partial resolution such that the k cyclic quotient singularities of type
$\pi \colon \widetilde {V} \to V$
is a partial resolution such that the k cyclic quotient singularities of type
![]() $(\frac {1}{4},\frac {1}{4},\frac {1}{2})$
of V are replaced by the milder cyclic quotient singularities of type
$(\frac {1}{4},\frac {1}{4},\frac {1}{2})$
of V are replaced by the milder cyclic quotient singularities of type
![]() $(\frac {1}{2},\frac {1}{2},0)$
. As an application of some results in Section 8, we compare the BCOV invariants between
$(\frac {1}{2},\frac {1}{2},0)$
. As an application of some results in Section 8, we compare the BCOV invariants between
![]() $\widetilde {V}$
and V.
$\widetilde {V}$
and V.
Let
![]() $\gamma _{X}$
(respectively,
$\gamma _{X}$
(respectively,
![]() $\gamma _{\widetilde {X}}$
) be a Ricci-flat Kähler from on X (respectively,
$\gamma _{\widetilde {X}}$
) be a Ricci-flat Kähler from on X (respectively,
![]() $\widetilde {X}$
), and let
$\widetilde {X}$
), and let
![]() $\gamma _{T}$
be the flat Kähler form with
$\gamma _{T}$
be the flat Kähler form with
![]() $\mathrm {Vol}(V,\gamma _{T})=1$
. Let
$\mathrm {Vol}(V,\gamma _{T})=1$
. Let
![]() $\pi _{1} \colon V \to Y=X/\iota $
and
$\pi _{1} \colon V \to Y=X/\iota $
and
![]() $\pi _{2} \colon V \to T/(-1)_{T}$
be the projections. Similarly, let
$\pi _{2} \colon V \to T/(-1)_{T}$
be the projections. Similarly, let
![]() $\widetilde {\pi }_{1} \colon \widetilde {V} \to \widetilde {X}/\theta $
and
$\widetilde {\pi }_{1} \colon \widetilde {V} \to \widetilde {X}/\theta $
and
![]() $\widetilde {\pi }_{2} \colon \widetilde {V} \to T/(-1)_{T}$
be the projections. We define a Ricci-flat Kähler form
$\widetilde {\pi }_{2} \colon \widetilde {V} \to T/(-1)_{T}$
be the projections. We define a Ricci-flat Kähler form
![]() $\gamma $
(respectively,
$\gamma $
(respectively,
![]() $\widetilde {\gamma }$
) on V (respectively,
$\widetilde {\gamma }$
) on V (respectively,
![]() $\widetilde {V}$
) by
$\widetilde {V}$
) by
Since
![]() $\mathrm {Sing}(X\times T) = (\{ p_{1} \} \times T) \amalg \cdots \amalg (\{ p_{k} \} \times T)$
, we have
$\mathrm {Sing}(X\times T) = (\{ p_{1} \} \times T) \amalg \cdots \amalg (\{ p_{k} \} \times T)$
, we have
 $$ \begin{align*}\begin{aligned} \mathrm{Sing}\,V &= (\{ p_{1} \} \times T/(-1)_{T}) \amalg\cdots\amalg (\{ p_{k} \} \times T/(-1)_{T}) \amalg (X^{\iota} \times T[2]) \\ &= (\{ p_{1} \} \times T/(-1)_{T}) \amalg\cdots\amalg (\{ p_{k} \} \times T/(-1)_{T}) \amalg (\mathrm{Sing}\,X \times T[2]), \end{aligned} \end{align*} $$
$$ \begin{align*}\begin{aligned} \mathrm{Sing}\,V &= (\{ p_{1} \} \times T/(-1)_{T}) \amalg\cdots\amalg (\{ p_{k} \} \times T/(-1)_{T}) \amalg (X^{\iota} \times T[2]) \\ &= (\{ p_{1} \} \times T/(-1)_{T}) \amalg\cdots\amalg (\{ p_{k} \} \times T/(-1)_{T}) \amalg (\mathrm{Sing}\,X \times T[2]), \end{aligned} \end{align*} $$
where
![]() $T[2]$
denotes the points of order
$T[2]$
denotes the points of order
![]() $2$
of T. Similarly,
$2$
of T. Similarly,
Hence the
![]() $1$
-dimensional strata of
$1$
-dimensional strata of
![]() $\mathrm {Sing}\,V$
(respectively,
$\mathrm {Sing}\,V$
(respectively,
![]() $\mathrm {Sing}\,\widetilde {V}$
) consist of k-copies of the quotient
$\mathrm {Sing}\,\widetilde {V}$
) consist of k-copies of the quotient
![]() $T/(-1)_{T}$
(respectively,
$T/(-1)_{T}$
(respectively,
![]() $4$
-copies of
$4$
-copies of
![]() $E_{1},\ldots ,E_{k}$
), which are endowed with the flat orbifold Kähler form
$E_{1},\ldots ,E_{k}$
), which are endowed with the flat orbifold Kähler form
![]() $\gamma _{T}$
(respectively, Kähler form
$\gamma _{T}$
(respectively, Kähler form
![]() $\gamma _{\widetilde {X}}|_{E_{i}}$
induced from
$\gamma _{\widetilde {X}}|_{E_{i}}$
induced from
![]() $\gamma _{\widetilde {X}}$
).
$\gamma _{\widetilde {X}}$
).
Recall from [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46, (6.12)] that the orbifold BCOV invariant of V is defined by
 $$ \begin{align} \begin{aligned} \tau_{\mathrm{BCOV}}^{\mathrm{orb}}(V) &= T_{\mathrm{BCOV}}(V,\gamma)\mathrm{Vol}(V,\gamma)^{-3+\frac{\chi^{\mathrm{orb}}(V)}{12}}\mathrm{Vol}_{L^{2}}(H^{2}(V,{\mathbf Z}),\gamma)^{-1} \\ &\quad\times \prod_{i=1}^{k} \tau( \{ p_{i} \}\times (T/(-1)_{T}), \gamma_{T})^{-1}\mathrm{Vol}(T/(-1)_{T}, \gamma_{T})^{-1} \\ &= T_{\mathrm{BCOV}}(V,\gamma)\mathrm{Vol}(V,\gamma)^{-3+\frac{\chi^{\mathrm{orb}}(V)}{12}}\mathrm{Vol}_{L^{2}}(H^{2}(V,{\mathbf Z}),\gamma)^{-1} 2^{k}\tau( T, \gamma_{T})^{-\frac{k}{2}}, \end{aligned} \end{align} $$
$$ \begin{align} \begin{aligned} \tau_{\mathrm{BCOV}}^{\mathrm{orb}}(V) &= T_{\mathrm{BCOV}}(V,\gamma)\mathrm{Vol}(V,\gamma)^{-3+\frac{\chi^{\mathrm{orb}}(V)}{12}}\mathrm{Vol}_{L^{2}}(H^{2}(V,{\mathbf Z}),\gamma)^{-1} \\ &\quad\times \prod_{i=1}^{k} \tau( \{ p_{i} \}\times (T/(-1)_{T}), \gamma_{T})^{-1}\mathrm{Vol}(T/(-1)_{T}, \gamma_{T})^{-1} \\ &= T_{\mathrm{BCOV}}(V,\gamma)\mathrm{Vol}(V,\gamma)^{-3+\frac{\chi^{\mathrm{orb}}(V)}{12}}\mathrm{Vol}_{L^{2}}(H^{2}(V,{\mathbf Z}),\gamma)^{-1} 2^{k}\tau( T, \gamma_{T})^{-\frac{k}{2}}, \end{aligned} \end{align} $$
where we used the facts
![]() $\tau (T/(-1)_{T},\gamma _{T}) = \tau (T,\gamma _{T})^{1/2}$
and
$\tau (T/(-1)_{T},\gamma _{T}) = \tau (T,\gamma _{T})^{1/2}$
and
![]() $\mathrm {Vol}(T/(-1)_{T},\gamma _{T})=1/2$
to get the second equality and
$\mathrm {Vol}(T/(-1)_{T},\gamma _{T})=1/2$
to get the second equality and
![]() $\mathrm {Vol}_{L^{2}}(H^{2}(V,{\mathbf Z}),\gamma )$
is the covolume of the lattice
$\mathrm {Vol}_{L^{2}}(H^{2}(V,{\mathbf Z}),\gamma )$
is the covolume of the lattice
![]() $H^{2}(V,{\mathbf Z})_{\mathrm {fr}}:=H^{2}(V,{\mathbf Z})/\mathrm {Torsion}$
with respect to the
$H^{2}(V,{\mathbf Z})_{\mathrm {fr}}:=H^{2}(V,{\mathbf Z})/\mathrm {Torsion}$
with respect to the
![]() $L^{2}$
metric induced by
$L^{2}$
metric induced by
![]() $\gamma $
. (In what follows, for a finitely generated
$\gamma $
. (In what follows, for a finitely generated
![]() ${\mathbf Z}$
-module M, we set
${\mathbf Z}$
-module M, we set
![]() $M_{\mathrm {fr}} := M/\mathrm {Tors}(M)$
.) For the definition of
$M_{\mathrm {fr}} := M/\mathrm {Tors}(M)$
.) For the definition of
![]() $\chi ^{\mathrm {orb}}(V)$
, see [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46, (6.2)]. By [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46, Prop. 6.2],
$\chi ^{\mathrm {orb}}(V)$
, see [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46, (6.2)]. By [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46, Prop. 6.2],
![]() $\chi ^{\mathrm { orb}}(V)$
coincides with the Euler characteristic of a crepant resolution of V. Similarly, we have
$\chi ^{\mathrm { orb}}(V)$
coincides with the Euler characteristic of a crepant resolution of V. Similarly, we have
 $$ \begin{align*}\begin{aligned} \tau_{\mathrm{BCOV}}^{\mathrm{orb}}(\widetilde{V}) &= T_{\mathrm{BCOV}}(\widetilde{V},\widetilde{\gamma}) \mathrm{Vol}(\widetilde{V},\widetilde{\gamma})^{-3+\frac{\chi^{\mathrm{orb}}(\widetilde{V})}{12}} \mathrm{Vol}_{L^{2}}(H^{2}(\widetilde{V},{\mathbf Z}),\widetilde{\gamma})^{-1} \\ &\quad\times \{\prod_{i=1}^{k}\tau(E_{i},\gamma_{\widetilde{X}}|_{E_{i}})\mathrm{Vol}(E_{i}, \gamma_{\widetilde{X}}|_{E_{i}})\}^{-4}. \end{aligned} \end{align*} $$
$$ \begin{align*}\begin{aligned} \tau_{\mathrm{BCOV}}^{\mathrm{orb}}(\widetilde{V}) &= T_{\mathrm{BCOV}}(\widetilde{V},\widetilde{\gamma}) \mathrm{Vol}(\widetilde{V},\widetilde{\gamma})^{-3+\frac{\chi^{\mathrm{orb}}(\widetilde{V})}{12}} \mathrm{Vol}_{L^{2}}(H^{2}(\widetilde{V},{\mathbf Z}),\widetilde{\gamma})^{-1} \\ &\quad\times \{\prod_{i=1}^{k}\tau(E_{i},\gamma_{\widetilde{X}}|_{E_{i}})\mathrm{Vol}(E_{i}, \gamma_{\widetilde{X}}|_{E_{i}})\}^{-4}. \end{aligned} \end{align*} $$
Let
![]() $q \colon X \times T \to V$
and
$q \colon X \times T \to V$
and
![]() $\widetilde {q} \colon \widetilde {X} \times T \to \widetilde {V}$
be the quotient maps. Let
$\widetilde {q} \colon \widetilde {X} \times T \to \widetilde {V}$
be the quotient maps. Let
![]() $H^{2}(X\times T, {\mathbf Z})^{+}$
(respectively,
$H^{2}(X\times T, {\mathbf Z})^{+}$
(respectively,
![]() $H^{2}(\widetilde {X}\times T, {\mathbf Z})^{+}$
) be the invariant subspace with respect to the
$H^{2}(\widetilde {X}\times T, {\mathbf Z})^{+}$
) be the invariant subspace with respect to the
![]() $\iota \times (-1)_{T}$
(respectively,
$\iota \times (-1)_{T}$
(respectively,
![]() $\theta \times (-1)_{T}$
)-action on
$\theta \times (-1)_{T}$
)-action on
![]() $X \times T$
(respectively,
$X \times T$
(respectively,
![]() $\widetilde {X}\times T$
). We define
$\widetilde {X}\times T$
). We define
![]() $H^{2}(X, {\mathbf Z})^{+}$
and
$H^{2}(X, {\mathbf Z})^{+}$
and
![]() $H^{2}(\widetilde {X}, {\mathbf Z})^{+}$
in the same way. Let
$H^{2}(\widetilde {X}, {\mathbf Z})^{+}$
in the same way. Let
![]() $r:=\mathrm {rk}_{\mathbf Z} H^{2}(X, {\mathbf Z})^{+}$
and
$r:=\mathrm {rk}_{\mathbf Z} H^{2}(X, {\mathbf Z})^{+}$
and
![]() $\widetilde {r} := \mathrm {rk}_{\mathbf Z} H^{2}(\widetilde {X}, {\mathbf Z})^{+}$
. Then
$\widetilde {r} := \mathrm {rk}_{\mathbf Z} H^{2}(\widetilde {X}, {\mathbf Z})^{+}$
. Then
![]() $\widetilde {r}=r+k=10+k$
. The maps of cohomologies
$\widetilde {r}=r+k=10+k$
. The maps of cohomologies
have finite cokernel. Let
![]() $\mathrm {disc}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm {fr}})$
be the discriminant of the lattice
$\mathrm {disc}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm {fr}})$
be the discriminant of the lattice
![]() $H^{2}(X,{\mathbf Z})^{+}_{\mathrm {fr}}$
with respect to the intersection pairing
$H^{2}(X,{\mathbf Z})^{+}_{\mathrm {fr}}$
with respect to the intersection pairing
![]() $\langle \cdot ,\cdot \rangle $
on
$\langle \cdot ,\cdot \rangle $
on
![]() $H^{2}(X,{\mathbf Z})_{\mathrm {fr}}\subset H^{2}(X,{\mathbf Q})$
. Namely, if
$H^{2}(X,{\mathbf Z})_{\mathrm {fr}}\subset H^{2}(X,{\mathbf Q})$
. Namely, if
![]() $\{ {\mathbf e}_{1},\ldots ,{\mathbf e}_{r} \}$
is a basis of
$\{ {\mathbf e}_{1},\ldots ,{\mathbf e}_{r} \}$
is a basis of
![]() $H^{2}(X,{\mathbf Z})_{\mathrm {fr}}$
, then
$H^{2}(X,{\mathbf Z})_{\mathrm {fr}}$
, then
![]() $\mathrm {disc}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm {fr}}) := \det ( \langle {\mathbf e}_{i}, {\mathbf e}_{j} \rangle )$
. Obviously,
$\mathrm {disc}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm {fr}}) := \det ( \langle {\mathbf e}_{i}, {\mathbf e}_{j} \rangle )$
. Obviously,
![]() $|\mathrm {Coker}\, q^{*}|$
,
$|\mathrm {Coker}\, q^{*}|$
,
![]() $|\mathrm {Coker}\, \widetilde {q}^{*}|$
,
$|\mathrm {Coker}\, \widetilde {q}^{*}|$
,
![]() $\mathrm {disc}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm {fr}})$
,
$\mathrm {disc}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm {fr}})$
,
![]() $\mathrm {disc}(H^{2}(\widetilde {X},{\mathbf Z})^{+})$
depend only on k. Recall that the constant
$\mathrm {disc}(H^{2}(\widetilde {X},{\mathbf Z})^{+})$
depend only on k. Recall that the constant
![]() $C(k)$
was defined in equation (8.1), which is the kth power of the product of the normalised analytic torsion of the Eguchi-Hanson instanton and the analytic torsion of
$C(k)$
was defined in equation (8.1), which is the kth power of the product of the normalised analytic torsion of the Eguchi-Hanson instanton and the analytic torsion of
![]() ${\mathbf P}^{1}$
endowed with the Fubini-Study metric, up to a universal constant.
${\mathbf P}^{1}$
endowed with the Fubini-Study metric, up to a universal constant.
Theorem 9.6. The following equality holds:
 $$ \begin{align*}\frac{\tau_{\mathrm{BCOV}}^{\mathrm{orb}}(V)}{\tau_{\mathrm{BCOV}}^{\mathrm{orb}}(\widetilde{V})} = 2^{-k-4}C(k)^{8}\left(\frac{|\mathrm{Coker}\, q^{*}|}{|\mathrm{Coker}\, \widetilde{q}^{*}|}\right)^{-2} \left( \frac{|\mathrm{disc}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm{fr}})|}{|\mathrm{disc}(H^{2}(\widetilde{X},{\mathbf Z})^{+})|} \right)^{-1}. \end{align*} $$
$$ \begin{align*}\frac{\tau_{\mathrm{BCOV}}^{\mathrm{orb}}(V)}{\tau_{\mathrm{BCOV}}^{\mathrm{orb}}(\widetilde{V})} = 2^{-k-4}C(k)^{8}\left(\frac{|\mathrm{Coker}\, q^{*}|}{|\mathrm{Coker}\, \widetilde{q}^{*}|}\right)^{-2} \left( \frac{|\mathrm{disc}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm{fr}})|}{|\mathrm{disc}(H^{2}(\widetilde{X},{\mathbf Z})^{+})|} \right)^{-1}. \end{align*} $$
Proof. We express
![]() $T_{\mathrm {BCOV}}(V,\gamma )$
in terms of
$T_{\mathrm {BCOV}}(V,\gamma )$
in terms of
![]() $\tau _{{\mathbf Z}_{2}}(X,\gamma _{X})(\iota )$
and
$\tau _{{\mathbf Z}_{2}}(X,\gamma _{X})(\iota )$
and
![]() $\tau (T,\gamma _{T})$
. As is easily verified, Lemmas 8.3–8.7 of [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46] hold true for V without any change. Since
$\tau (T,\gamma _{T})$
. As is easily verified, Lemmas 8.3–8.7 of [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46] hold true for V without any change. Since
![]() $h^{1,1}(X)=20-k$
, the coefficient
$h^{1,1}(X)=20-k$
, the coefficient
![]() $21$
of
$21$
of
![]() $\zeta ^{T,+}(s)$
in [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46, Lemma 8.8] should be replaced by
$\zeta ^{T,+}(s)$
in [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46, Lemma 8.8] should be replaced by
![]() $21-k$
. Hence, for V, the equality corresponding to [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46, (8.28), p.357] becomes
$21-k$
. Hence, for V, the equality corresponding to [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46, (8.28), p.357] becomes
As a result, we get the following equality as in the first equality of [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46, (8.29), p.358]:
By [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46, (8.29), p.358 l.2-3], we have
Since
![]() $\widetilde {X}^{\theta }$
consists of k copies of mutually disjoint
$\widetilde {X}^{\theta }$
consists of k copies of mutually disjoint
![]() ${\mathbf P}^{1}$
, we get
${\mathbf P}^{1}$
, we get
![]() $\chi ^{\mathrm {orb}}(V) = \chi ^{\mathrm {orb}}( \widetilde {V} ) = \frac {1}{2}\chi (\widetilde {X}\times T) + \frac {3}{2}\chi ( \widetilde {X}^{\theta } \times T[2])=12k$
by [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46, Prop. 6.1 and (6.3)]. Hence
$\chi ^{\mathrm {orb}}(V) = \chi ^{\mathrm {orb}}( \widetilde {V} ) = \frac {1}{2}\chi (\widetilde {X}\times T) + \frac {3}{2}\chi ( \widetilde {X}^{\theta } \times T[2])=12k$
by [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46, Prop. 6.1 and (6.3)]. Hence
where we used the fact
![]() $\mathrm {Vol}(T,\gamma _{T})=1$
and
$\mathrm {Vol}(T,\gamma _{T})=1$
and
![]() $\mathrm {Vol}(V,\gamma )=\mathrm {Vol}(X,\gamma _{X})\mathrm {Vol}(T,\gamma _{T})/2$
. Similarly,
$\mathrm {Vol}(V,\gamma )=\mathrm {Vol}(X,\gamma _{X})\mathrm {Vol}(T,\gamma _{T})/2$
. Similarly,
Let
![]() $\{{\mathbf f}_{1},\ldots ,{\mathbf f}_{r+1}\}$
be a basis of
$\{{\mathbf f}_{1},\ldots ,{\mathbf f}_{r+1}\}$
be a basis of
![]() $H^{2}(V,{\mathbf Z})_{\mathrm {fr}}$
. By definition, we have
$H^{2}(V,{\mathbf Z})_{\mathrm {fr}}$
. By definition, we have
where
![]() $\langle \cdot ,\cdot \rangle _{L^{2}}$
denotes the
$\langle \cdot ,\cdot \rangle _{L^{2}}$
denotes the
![]() $L^{2}$
inner product on
$L^{2}$
inner product on
![]() $H^{2}(V,{\mathbf R})$
induced from
$H^{2}(V,{\mathbf R})$
induced from
![]() $\gamma $
. Since
$\gamma $
. Since
![]() $\mathrm {Vol}_{L^{2}}(H^{2}(T,{\mathbf Z}),\gamma _{T})=1$
, the same calculations as in [Reference Fang, Lu and Yoshikawa19, Lemma 13.4] yield that
$\mathrm {Vol}_{L^{2}}(H^{2}(T,{\mathbf Z}),\gamma _{T})=1$
, the same calculations as in [Reference Fang, Lu and Yoshikawa19, Lemma 13.4] yield that
 $$ \begin{align} \begin{aligned} \mathrm{Vol}_{L^{2}}(H^{2}(V,{\mathbf Z}),\gamma) &= |\mathrm{Coker}\,q^{*}|^{2}\,\mathrm{Vol}_{L^{2}}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm{fr}}\oplus H^{2}(T,{\mathbf Z}),\gamma_{X}\oplus\gamma_{T}) \\ &= |\mathrm{Coker}\,q^{*}|^{2}\,\mathrm{Vol}_{L^{2}}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm{fr}},\gamma_{X})\,\mathrm{Vol}(X,\gamma_{X})/2 \\ &= 2^{-(r+1)}|\mathrm{Coker}\,q^{*}|^{2}\,|\mathrm{disc}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm{fr}})|\,\mathrm{Vol}(X,\gamma_{X}). \end{aligned} \end{align} $$
$$ \begin{align} \begin{aligned} \mathrm{Vol}_{L^{2}}(H^{2}(V,{\mathbf Z}),\gamma) &= |\mathrm{Coker}\,q^{*}|^{2}\,\mathrm{Vol}_{L^{2}}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm{fr}}\oplus H^{2}(T,{\mathbf Z}),\gamma_{X}\oplus\gamma_{T}) \\ &= |\mathrm{Coker}\,q^{*}|^{2}\,\mathrm{Vol}_{L^{2}}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm{fr}},\gamma_{X})\,\mathrm{Vol}(X,\gamma_{X})/2 \\ &= 2^{-(r+1)}|\mathrm{Coker}\,q^{*}|^{2}\,|\mathrm{disc}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm{fr}})|\,\mathrm{Vol}(X,\gamma_{X}). \end{aligned} \end{align} $$
Similarly, we have
Substituting equations (9.4), (9.6) and (9.8) into equation (9.3) and using equation (3.7), we get
 $$ \begin{align} \begin{aligned} \tau_{\mathrm{BCOV}}^{\mathrm{orb}}(V) &= 2^{r+4}|\mathrm{Coker}\,q^{*}|^{-2}\,|\mathrm{disc}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm{fr}})|^{-1} \\ &\quad\times \tau_{{\mathbf Z}_{2}}(X,\gamma_{X})(\iota)^{-4}\mathrm{Vol}(X,\gamma_{X})^{-4+k}\tau(T,\gamma_{T})^{-12} \\ &= 2^{r+4}|\mathrm{Coker}\,q^{*}|^{-2}\,|\mathrm{disc}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm{fr}})|^{-1} \\ &\quad\times \tau(X,\gamma_{X})^{-4}\tau_{{\mathbf Z}_{2}}(X,\gamma_{X})(\iota)^{-4}\mathrm{Vol}(X,\gamma_{X})^{-4+k}\tau(T,\gamma_{T})^{-12} \\ &= 2^{r-k}|\mathrm{Coker}\,q^{*}|^{-2}\,|\mathrm{disc}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm{fr}})|^{-1} \tau(Y,\gamma_{Y})^{-8}\mathrm{Vol}(Y,\gamma_{Y})^{-4+k}\tau(T,\gamma_{T})^{-12} \\ &= 2^{r-k}|\mathrm{Coker}\,q^{*}|^{-2}\,|\mathrm{disc}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm{fr}})|^{-1}C(k)^{8} \tau_{M}(\widetilde{X},\theta)^{-4}\tau(T,\gamma_{T})^{-12}, \end{aligned} \end{align} $$
$$ \begin{align} \begin{aligned} \tau_{\mathrm{BCOV}}^{\mathrm{orb}}(V) &= 2^{r+4}|\mathrm{Coker}\,q^{*}|^{-2}\,|\mathrm{disc}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm{fr}})|^{-1} \\ &\quad\times \tau_{{\mathbf Z}_{2}}(X,\gamma_{X})(\iota)^{-4}\mathrm{Vol}(X,\gamma_{X})^{-4+k}\tau(T,\gamma_{T})^{-12} \\ &= 2^{r+4}|\mathrm{Coker}\,q^{*}|^{-2}\,|\mathrm{disc}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm{fr}})|^{-1} \\ &\quad\times \tau(X,\gamma_{X})^{-4}\tau_{{\mathbf Z}_{2}}(X,\gamma_{X})(\iota)^{-4}\mathrm{Vol}(X,\gamma_{X})^{-4+k}\tau(T,\gamma_{T})^{-12} \\ &= 2^{r-k}|\mathrm{Coker}\,q^{*}|^{-2}\,|\mathrm{disc}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm{fr}})|^{-1} \tau(Y,\gamma_{Y})^{-8}\mathrm{Vol}(Y,\gamma_{Y})^{-4+k}\tau(T,\gamma_{T})^{-12} \\ &= 2^{r-k}|\mathrm{Coker}\,q^{*}|^{-2}\,|\mathrm{disc}(H^{2}(X,{\mathbf Z})^{+}_{\mathrm{fr}})|^{-1}C(k)^{8} \tau_{M}(\widetilde{X},\theta)^{-4}\tau(T,\gamma_{T})^{-12}, \end{aligned} \end{align} $$
where we used the equality
![]() $\tau (Y,\gamma _{Y})^{2}=\tau (X,\gamma _{X})\tau _{{\mathbf Z}_{2}}(X,\gamma _{X})(\iota )$
to get the third equality and Theorem 8.3 to get the last equality. Similarly, substituting equations (9.5), (9.7) and (9.9) into [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46, (6.12)], we get
$\tau (Y,\gamma _{Y})^{2}=\tau (X,\gamma _{X})\tau _{{\mathbf Z}_{2}}(X,\gamma _{X})(\iota )$
to get the third equality and Theorem 8.3 to get the last equality. Similarly, substituting equations (9.5), (9.7) and (9.9) into [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46, (6.12)], we get
 $$ \begin{align} \begin{aligned} \tau_{\mathrm{BCOV}}^{\mathrm{orb}}(\widetilde{V}) &= \tau_{{\mathbf Z}_{2}}(\widetilde{X},\gamma_{\widetilde{X}})(\theta)^{-4}\tau(T,\gamma_{T})^{-12} \{\prod_{i=1}^{k}\tau(E_{i},\gamma_{\widetilde{X}}|_{E_{i}})\mathrm{Vol}(E_{i}, \gamma_{\widetilde{X}}|_{E_{i}})\}^{-4} \\ &\quad\times 2^{3-k}\,\mathrm{Vol}(\widetilde{X},\gamma_{\widetilde{X}})^{-3+k} 2^{\widetilde{r}+1}|\mathrm{Coker}\,\widetilde{q}^{*}|^{-2}\,|\mathrm{disc}(H^{2}(\widetilde{X},{\mathbf Z})^{+})|^{-1} \mathrm{Vol}(\widetilde{X},\gamma_{\widetilde{X}})^{-1} \\ &= 2^{\widetilde{r}+4-k}|\mathrm{Coker}\,\widetilde{q}^{*}|^{-2}\,|\mathrm{disc}(H^{2}(\widetilde{X},{\mathbf Z})^{+})|^{-1}\tau(T,\gamma_{T})^{-12} \\ &\quad\times \tau_{{\mathbf Z}_{2}}(\widetilde{X},\gamma_{\widetilde{X}})(\theta)^{-4}\mathrm{Vol}(\widetilde{X},\gamma_{\widetilde{X}})^{-4+k} \{\prod_{i=1}^{k}\tau(E_{i},\gamma_{\widetilde{X}}|_{E_{i}})\mathrm{Vol}(E_{i}, \gamma_{\widetilde{X}}|_{E_{i}})\}^{-4} \\ &= 2^{r+4}|\mathrm{Coker}\,\widetilde{q}^{*}|^{-2}\,|\mathrm{disc}(H^{2}(\widetilde{X},{\mathbf Z})^{+})|^{-1} \tau_{M}(\widetilde{X},\theta)^{-4}\tau(T,\gamma_{T})^{-12}. \end{aligned} \end{align} $$
$$ \begin{align} \begin{aligned} \tau_{\mathrm{BCOV}}^{\mathrm{orb}}(\widetilde{V}) &= \tau_{{\mathbf Z}_{2}}(\widetilde{X},\gamma_{\widetilde{X}})(\theta)^{-4}\tau(T,\gamma_{T})^{-12} \{\prod_{i=1}^{k}\tau(E_{i},\gamma_{\widetilde{X}}|_{E_{i}})\mathrm{Vol}(E_{i}, \gamma_{\widetilde{X}}|_{E_{i}})\}^{-4} \\ &\quad\times 2^{3-k}\,\mathrm{Vol}(\widetilde{X},\gamma_{\widetilde{X}})^{-3+k} 2^{\widetilde{r}+1}|\mathrm{Coker}\,\widetilde{q}^{*}|^{-2}\,|\mathrm{disc}(H^{2}(\widetilde{X},{\mathbf Z})^{+})|^{-1} \mathrm{Vol}(\widetilde{X},\gamma_{\widetilde{X}})^{-1} \\ &= 2^{\widetilde{r}+4-k}|\mathrm{Coker}\,\widetilde{q}^{*}|^{-2}\,|\mathrm{disc}(H^{2}(\widetilde{X},{\mathbf Z})^{+})|^{-1}\tau(T,\gamma_{T})^{-12} \\ &\quad\times \tau_{{\mathbf Z}_{2}}(\widetilde{X},\gamma_{\widetilde{X}})(\theta)^{-4}\mathrm{Vol}(\widetilde{X},\gamma_{\widetilde{X}})^{-4+k} \{\prod_{i=1}^{k}\tau(E_{i},\gamma_{\widetilde{X}}|_{E_{i}})\mathrm{Vol}(E_{i}, \gamma_{\widetilde{X}}|_{E_{i}})\}^{-4} \\ &= 2^{r+4}|\mathrm{Coker}\,\widetilde{q}^{*}|^{-2}\,|\mathrm{disc}(H^{2}(\widetilde{X},{\mathbf Z})^{+})|^{-1} \tau_{M}(\widetilde{X},\theta)^{-4}\tau(T,\gamma_{T})^{-12}. \end{aligned} \end{align} $$
Comparing equation (9.10) and equation (9.11), we get the result.
We define the BCOV invariant of elliptic curve T as
 $$ \begin{align*}\tau_{\mathrm{BCOV}}(T) := \mathrm{Vol}(T,\omega)^{-1}\,\tau_{\mathrm{BCOV}}(T,\omega)\, \exp\left[-\frac{1}{12}\int_{T} \log\left( \frac{i\,\xi\wedge\overline{\xi}}{\omega} \right)\,c_{1}(T,\omega)\right], \end{align*} $$
$$ \begin{align*}\tau_{\mathrm{BCOV}}(T) := \mathrm{Vol}(T,\omega)^{-1}\,\tau_{\mathrm{BCOV}}(T,\omega)\, \exp\left[-\frac{1}{12}\int_{T} \log\left( \frac{i\,\xi\wedge\overline{\xi}}{\omega} \right)\,c_{1}(T,\omega)\right], \end{align*} $$
where
![]() $\omega $
is an arbitrary Kähler from on T. By [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46, Th. 8.1],
$\omega $
is an arbitrary Kähler from on T. By [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46, Th. 8.1],
![]() $\tau _{\mathrm {BCOV}}(T)$
is independent of the choice of
$\tau _{\mathrm {BCOV}}(T)$
is independent of the choice of
![]() $\omega $
and is expressed by the Petersson norm of the Dedekind
$\omega $
and is expressed by the Petersson norm of the Dedekind
![]() $\eta $
-function. By definition, we have
$\eta $
-function. By definition, we have
![]() $\tau _{\mathrm {BCOV}}(T) = \tau (T,\gamma _{T})^{-1}$
. By equation (9.10), we have the following factorisation of the orbifold BCOV invariant of V.
$\tau _{\mathrm {BCOV}}(T) = \tau (T,\gamma _{T})^{-1}$
. By equation (9.10), we have the following factorisation of the orbifold BCOV invariant of V.
Corollary 9.7. The following equality of BCOV invariants holds:
Remark 9.8. In [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46, p.357 l.7], it seems that the equality
![]() $H^{2}(X,{\mathbf Z}) = H^{2}(S\times T,{\mathbf Z})^{+}$
does not hold in general. As the difference of these two quantities,
$H^{2}(X,{\mathbf Z}) = H^{2}(S\times T,{\mathbf Z})^{+}$
does not hold in general. As the difference of these two quantities,
![]() $|\mathrm {Coker}\,\widetilde {q}^{*}|$
should appear in the formula for
$|\mathrm {Coker}\,\widetilde {q}^{*}|$
should appear in the formula for
![]() $\tau _{\mathrm {BCOV}}^{\mathrm {orb}}(\widetilde {V})$
as in equation (9.11).
$\tau _{\mathrm {BCOV}}^{\mathrm {orb}}(\widetilde {V})$
as in equation (9.11).
Remark 9.9. In this subsection, for the sake of simplicity of notation, we adopt the definitions
![]() $\mathrm {Vol}(V,\gamma )=\int _{V}\gamma ^{3}/3!$
and
$\mathrm {Vol}(V,\gamma )=\int _{V}\gamma ^{3}/3!$
and
![]() $\langle \alpha , \beta \rangle _{L^{2}} = \int _{V} ({\mathcal H}\alpha ) \wedge \overline {*}({\mathcal H}\beta )$
, and so on, where
$\langle \alpha , \beta \rangle _{L^{2}} = \int _{V} ({\mathcal H}\alpha ) \wedge \overline {*}({\mathcal H}\beta )$
, and so on, where
![]() ${\mathcal H}(\cdot )$
denotes the harmonic projection. If we follow the tradition in Arakelov geometry, it is more natural to define the
${\mathcal H}(\cdot )$
denotes the harmonic projection. If we follow the tradition in Arakelov geometry, it is more natural to define the
![]() $L^{2}$
-inner product by
$L^{2}$
-inner product by
![]() $\mathrm {Vol}(V,\gamma )=(2\pi )^{-\dim V}\int _{V}\gamma ^{3}/3!$
and
$\mathrm {Vol}(V,\gamma )=(2\pi )^{-\dim V}\int _{V}\gamma ^{3}/3!$
and
![]() $\langle \alpha , \beta \rangle _{L^{2}} = (2\pi )^{-\dim V}\int _{V} ({\mathcal H}\alpha ) \wedge \overline {*}({\mathcal H}\beta )$
, and so on. Notice that in [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46], this latter definition is adopted.
$\langle \alpha , \beta \rangle _{L^{2}} = (2\pi )^{-\dim V}\int _{V} ({\mathcal H}\alpha ) \wedge \overline {*}({\mathcal H}\beta )$
, and so on. Notice that in [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46], this latter definition is adopted.
Problem 9.10. Is the orbifold BCOV invariant [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46] a birational invariant of Calabi-Yau orbifolds? (To our knowledge, it is still open that the BCOV invariant of KLT Calabi-Yau varieties [Reference Fu and Zhang20] coincides with the orbifold BCOV invariant [Reference Yoshikawa, Bost, Hofer, Labourie, Le Jan, Ma and Zhang46].) If the answer is affirmative, then it follows from Theorem 9.6 that the normalised analytic torsion of the Eguchi-Hanson instanton will essentially be given by the analytic torsion of
![]() ${\mathbf P}^{1}$
with respect to the Fubini-Study metric. Once a comparison formula for the BCOV invariants for birational Calabi-Yau orbifolds is obtained, then one will get a formula for the normalised analytic torsion of the Eguchi-Hanson instanton through Theorem 9.6.
${\mathbf P}^{1}$
with respect to the Fubini-Study metric. Once a comparison formula for the BCOV invariants for birational Calabi-Yau orbifolds is obtained, then one will get a formula for the normalised analytic torsion of the Eguchi-Hanson instanton through Theorem 9.6.
Acknowledgements
The first author is partially supported by NSF-DMS1611915 and the Simons Foundation. The second author is partially supported by JSPS KAKENHI Grant Numbers 16H03935, 16H06335, 21H00984, 21H04429. This research was initiated when the authors met at the conference ‘Analytic torsion and its applications’ held in the department of mathematics of Paris-Sud University in June 2012. The authors thank the organiser of this conference.
Conflicts of Interest
None.