Published online by Cambridge University Press: 24 November 2012
We analyse the buckling stability of a thin, viscous sheet when subject to simple shear, providing conditions for the onset of the dominant out-of-plane modes using two models: (i) an asymptotic theory for the dynamics of a viscous plate, and (ii) the full Stokes equations. In either case, the plate is stabilized by a combination of viscous resistance, surface tension and buoyancy relative to an underlying denser fluid. In the limit of vanishing thickness, plates buckle at a shear rate 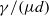 independent of buoyancy, where
independent of buoyancy, where  is the plate thickness,
is the plate thickness, 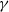 is the average surface tension between the upper and lower surfaces, and
is the average surface tension between the upper and lower surfaces, and 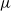 is the fluid viscosity. For thicker plates stabilized by an equal surface tension at the upper and lower surfaces, at and above onset, the most unstable mode has moderate wavelength, is stationary in the frame of the centreline, spans the width of the plate with crests and troughs aligned at approximately
is the fluid viscosity. For thicker plates stabilized by an equal surface tension at the upper and lower surfaces, at and above onset, the most unstable mode has moderate wavelength, is stationary in the frame of the centreline, spans the width of the plate with crests and troughs aligned at approximately 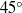 to the walls, and closely resembles elastic shear modes. The thickest plates that can buckle have an aspect ratio (thickness/width) of approximately 0.6 and are stabilized only by internal viscous resistance. We show that the viscous plate model can only accurately describe the onset of buckling for vanishingly thin plates but provides an excellent description of the most unstable mode above onset. Finally, we show that, by modifying the plate model to incorporate advection and make the model material-frame-invariant, it is possible to extend its predictive power to describe relatively short, travelling waves.
to the walls, and closely resembles elastic shear modes. The thickest plates that can buckle have an aspect ratio (thickness/width) of approximately 0.6 and are stabilized only by internal viscous resistance. We show that the viscous plate model can only accurately describe the onset of buckling for vanishingly thin plates but provides an excellent description of the most unstable mode above onset. Finally, we show that, by modifying the plate model to incorporate advection and make the model material-frame-invariant, it is possible to extend its predictive power to describe relatively short, travelling waves.