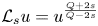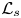Optimal decay for solutions of nonlocal semilinear equations with critical exponent in homogeneous groups

Abstract

In this paper, we establish the sharp asymptotic decay of positive solutions of the Yamabe type equation $\mathcal {L}_s u=u^{\frac {Q+2s}{Q-2s}}$ in a homogeneous Lie group, where $\mathcal {L}_s$ represents a suitable pseudodifferential operator modelled on a class of nonlocal operators arising in conformal CR geometry.

1. Introduction

The study of the CR Yamabe problem began with the celebrated works of Jerison and Lee [26–29]. The prototypical nonlinear partial differential equation in this problem is

\[ \mathscr L u = u^{\frac{Q+2}{Q-2}}, \]

where $\mathscr L$ indicates the negative sum of squares of the left-invariant vector fields generating the horizontal space in the Heisenberg group $\mathbb {H}^n$ with real dimension $2n+1$, whereas $Q = 2n+2$ denotes the so-called homogeneous dimension associated with the non-isotropic group dilations. (In this paper, we always use the group law dictated by the Baker–Campbell–Hausdorff formula. When the Lie group is $\mathbb {H}^n$, or more in general a group of Heisenberg type, this choice obviously affects the expression of the horizontal Laplacian.) In the present paper, we are interested in the following nonlocal version of the above equation:

(1.1)\begin{equation} \mathscr L_s u = u^{\frac{Q+2s}{Q-2s}}, \end{equation}

where the fractional parameter $s\in (0,\,1)$, and $\mathscr L_s$ denotes a certain pseudodifferential operator which arises in conformal CR geometry. As an application of our main result we derive sharp decay estimates of nonnegative solutions of (1.1).

The operator $\mathscr L_s$ in (1.1) was first introduced in [2] via the spectral formula:

(1.2)\begin{equation} \mathscr L_s = 2^s |T|^s \frac{\Gamma(-\frac 12\mathscr L |T|^{{-}1} + \frac{1+s}2)}{\Gamma(-\frac 12 \mathscr L |T|^{{-}1} + \frac{1-s}2)}, \end{equation}

where $\Gamma (x) = \int _0^\infty t^{x-1} {\rm e}^{-t} {\rm d}t$ denotes Euler gamma function. In (1.2) we have let $T = \partial _\sigma$, where for a point $g\in \mathbb {H}^n$ we have indicated with $g = (z,\,\sigma )$ its logarithmic coordinates. More in general, in a group of Heisenberg type $\mathbb {G}$, with logarithmic coordinates $g= (z,\,\sigma )\in \mathbb {G}$, where $z$ denotes the horizontal variable and $\sigma$ the vertical one, the pseudodifferential operator $\mathscr L_s$ is defined by the following generalization of (1.2):

(1.3)\begin{equation} \mathscr L_s = 2^s (-\Delta_\sigma)^{s/2} \frac{\Gamma(-\frac 12\mathscr L (-\Delta_\sigma)^{{-}1/2} + \frac{1+s}2)}{\Gamma(-\frac 12 \mathscr L (-\Delta_\sigma)^{{-}1/2} + \frac{1-s}2)}, \end{equation}

where $-\Delta _\sigma$ is the positive Laplacian in the centre of the group, see [37]. Formulas (1.2) and (1.3) should be seen as the counterpart of the well-known spectral representation $\widehat {(-\Delta )^s u} = (2\pi |\xi |)^{2s} \hat u$, where we have denoted by $\hat f$ the Fourier transform of a function $f$, see [38, Chap. 5]. An important fact, first proved for $\mathbb {H}^n$ in [12] using hyperbolic scattering, and subsequently generalized to any group of Heisenberg type in [37] using non-commutative harmonic analysis, is the following Dirichlet-to-Neumann characterization of $\mathscr L_s$:

\[ - \underset{y\to 0^+}{\lim} y^{1-2s} \partial_y U((z,\sigma),y) = 2^{1-2s} \frac{\Gamma(1-s)}{\Gamma(s)}\mathscr L_s u(z,\sigma), \]

where $U((z,\,\sigma ),\,y)$ is the solution to a certain extension problem from conformal CR geometry very different from that of Caffarelli–Silvestre in [5]. Yet another fundamental fact, proved in [36, Proposition 4.1] and [37, Theorem 1.2] for $0< s<1/2$, is the following remarkable Riesz type representation:

(1.4)\begin{equation} \alpha(m,k,s)\ \mathscr L_s u(g)=\int_{\mathbb{G}} \frac{u(g)-u(h)}{|h|^{Q+2s}}{\rm d}h, \end{equation}

where with $g = (z,\,\sigma )$, we have denoted by $|g| = |(z,\,\sigma )| = (|z|^4 + 16 |\sigma |^2)^{1/4}$ the non-isotropic gauge in a group of Heisenberg type $\mathbb {G}$. Using the heat equation approach in [17, 18], formula (1.4) can be extended to cover the whole range $0< s<1$. In (1.4) the number $\alpha (m,\,k,\,s)>0$ denotes an explicit constant depending on $s$ and the dimensions $m$ and $k$ of the horizontal and vertical layers of the Lie algebra of $\mathbb {G}$. While by (1.2), (1.3), and the classical formula $\Gamma (x+1) = x \Gamma (x)$, it is formally almost obvious that in the limit as $s\nearrow 1$ the operator $\mathscr L_s$ tends to the negative of the horizontal Laplacian $\mathscr L$, we emphasize that, contrarily to an unfortunate misconception, when $\mathbb {G} = \mathbb {H}^n$, or more in general it is of Heisenberg type, for no $s\in (0,\,1)$ does the standard fractional power

(1.5)\begin{equation} \mathscr L^s u(g) \overset{def}= (-\mathscr L)^s u(g) ={-} \frac{s}{\Gamma(1-s)} \int_0^\infty \frac{1}{t^{1+s}} \left(P_t u(g) - u(g)\right) {\rm d}t \end{equation}

coincide with the pseudodifferential operator defined by the left-hand side of (1.4) (in (1.5) we have denoted by $P_t = {\rm e}^{-t\mathscr L}$ the heat semigroup constructed in [10]). Unlike their classical predecessors $(-\Delta )^s$, in the purely non-Abelian setting of $\mathbb {H}^n$ the pseudodifferential operators $\mathscr L^s$ in (1.5) are not CR conformally invariant, nor they have any special geometric meaning, while the operators $\mathscr L_s$ are CR conformally invariant. For these reasons, we will refer to the operator $\mathscr L_s$ as the geometric (or conformal) fractional sub-Laplacian, even in the general setting of groups of Heisenberg type, see [12, Section 8.3] for relevant remarks in the remaining non-Abelian groups of Iwasawa type. Furthermore, it is not true that the fundamental solution $\mathscr E^{(s)}(z,\,\sigma )$ of $\mathscr L^s$ is a multiple of $|(z,\,\sigma )|^{2s-Q}$, see [17, Theor. 5.1]. What is instead true, as proven originally by Cowling and Haagerup [6], see also [36, (3.10)], and with a completely different approach based on heat equation techniques in [17, Theor. 1.2] (the reader should also see in this respect the works [18] and [19]), is that the fundamental solution of the conformal fractional sub-Laplacian $\mathscr L_s$ in (1.3) is given by

(1.6)\begin{equation} \mathscr E_{(s)}(z,\sigma) = \frac{C_{(s)}(m,k)}{|(z,\sigma)|^{Q-2s}}, \end{equation}

where

\[ C_{(s)}(m,k) = \frac{2^{\frac m2 + 2k-3s-1} \Gamma(\frac 12(\frac m2+1-s)) \Gamma(\frac 12(\frac m2 + k -s))}{\pi^{\frac{m+k+1}2} \Gamma(s)}. \]

It is worth emphasizing here that, when $s\to 1$, one recovers from (1.6) the famous formula for the fundamental solution of $-\mathscr L$, first found by Folland in [9] in $\mathbb {H}^n$, and subsequently generalized by Kaplan in [30] to groups of Heisenberg type. Before proceeding, we pause to notice that from the stochastic completeness and left-invariance of $P_t$, in any Carnot group $\mathbb {G}$ one tautologically obtains from (1.5)

(1.7)\begin{equation} \mathscr L^s u(g) = \frac{1}{2}\int_{\mathbb{G}} \frac{2u(g)-u(gh)-u(gh^{{-}1})}{||h||_{(s)}^{Q+2s}}{\rm d}h, \end{equation}

where for $g\in \mathbb {G}$ we have defined

(1.8)\begin{equation} \frac{1}{||g||_{(s)}^{Q+2s}} \overset{def}= \frac{2s}{\Gamma(1-s)} \int_0^\infty \frac{1}{t^{1+s}} p(g,t) {\rm d}t, \end{equation}

with $p(g,\,t)$ the positive heat kernel of $-\mathscr L$. While in the Abelian case $\mathbb {G} = \mathbb {R}^n$, with Euclidean norm $|\cdot |$, an elementary explicit calculation in (1.8), based on the knowledge that $p(x,\,t) = (4\pi t)^{-\frac n2} {\rm e}^{-\frac {|x|^2}{4t}}$, gives

\[ \frac{1}{||x||_{(s)}^{n+2s}} = \frac{s 2^{2s+1} \Gamma(\frac{n+2s}2)}{\pi^{\frac n2}\Gamma(1-s)} \frac{1}{|x|^{n+2s}}, \]

and one recovers from (1.5) Riesz’ classical representation, when $\mathbb {G}$ is a non-Abelian Carnot group it is not true that the right-hand side of (1.8) defines a function of the gauge $|g| = |(z,\,\sigma )| = (|z|^4 + 16 |\sigma |^2)^{1/4}$. In fact, in any (non-Abelian) group of Heisenberg type the following explicit expression of (1.8) was computed in [17, Theorem 5.1] (to obtain it, one should change $s$ into $-s$ in that result, see [17, Remark 5.2])

(1.9)\begin{align} \frac{1}{||g||_{(s)}^{Q+2s}} & = \frac{s 2^{k+ 2s}\Gamma(\frac m2 + k + s)}{\pi^{\frac{m+k}2}\Gamma(1-s)\Gamma(\frac k2)} \frac{1}{ |z|^{2(\frac m2 + k + s)}}\notag\\ & \quad \times \int_0^1 (\tanh^{{-}1} \sqrt y)^{{-}s-1} \left(1-y\right)^{\frac m4-1} y^{\frac 12(k + s-1)}\notag\\ & \quad\times F\left(\frac{1}{2}\left(\frac m2 + k + s\right),\frac 12\left(\frac m2 + k+1+s\right);\frac k2;- \frac{16|\sigma|^2}{|z|^4} y\right) \ {\rm d}y, \end{align}

where we have denoted by $F(a,\,b;c;z)$ the Gauss hypergeometric series. Formula (1.9) proves in particular that the function defined by (1.8) is not a function of the gauge $N(z,\,\sigma ) = (|z|^4 + 16 |\sigma |^2)^{1/4}$ (although it does have the expected cylindrical symmetry since it depends on $|z|^4$ and $|\sigma |^2$). If we substitute (1.9) in (1.8), and then (1.8) in (1.7), by comparing with formula (1.4), we conclude that $\mathscr L^s \not = \mathscr L_s$ for every $0< s<1$.

Formulas (1.4) and (1.6) motivated the results in the present work. As we have mentioned, we are interested in optimal decay estimates for nonnegative subsolutions of (1.1). In this respect, [36, Theorem 3.1] and [37, Theorem 3.7] gave the explicit form of a solution to the fractional Yamabe equation on group of Heisenberg type as a consequence of the intertwining properties of $\mathscr L_s$ for $0 < s < n + 1$, see also [18] for a different approach to intertwining based on the heat equation. In the notation of [18, Corollary 3.3], the result is that if $\mathbb {G}$ is of Heisenberg type, and $0< s<1$, then for every $(z,\,\sigma )\in \mathbb {G}$, and $y>0$ one has the following intertwining identity:

(1.10)\begin{align} & \mathscr L_s\left(((|z|^2+y^2)^2+16|\sigma|^2)^{-\frac{m + 2k - 2s}4}\right)=\frac{\Gamma\left(\frac{m+2+2s}{4}\right)\Gamma\left(\frac{m+2k+2s}{4}\right)}{\Gamma\left(\frac{m+2-2s}{4}\right)\Gamma\left(\frac{m+2k-2s}{4}\right)} \\ & \quad\times\ (4y)^{2s}((|z|^2+y^2)^2+16|\sigma|^2)^{-\frac{m + 2k + 2s}4}. \nonumber \end{align}

Here, it might be worth clarifying for the reader that the parameter $y$ appearing in (1.10) is precisely the ‘extension’ variable in the parabolic counterpart of the conformal version of the extension problem discovered in [12]. An immediate consequence of (1.10) is that, for any real positive number $y>0$, the function

(1.11)\begin{align} u_y(z,\sigma)= \left(\frac{\Gamma\left(\frac{m+2+2s}{4}\right)\Gamma\left(\frac{m+2k+2s}{4}\right)}{\Gamma\left(\frac{m+2-2s}{4}\right)\Gamma\left(\frac{m+2k-2s}{4}\right)}\right)^{\frac{m + 2k - 2s}{4s}}\left(\frac{16y^2}{(|z|^2+y^2)^2+16|\sigma|^2}\right)^{\frac{m + 2k - 2s}4} \end{align}

is a positive solution of the nonlinear equation (1.1). In this sense, we might say that functions (1.11) represent the counterpart of the so-called ‘bubbles’ from conformal geometry. We note that in the particular setting of the Heisenberg group $\mathbb {H}^n$ (which corresponds to the case $m = 2n$ and $k=1$) the function appearing in the left-hand side of (1.10) defines, up to group translations, the unique extremal of the Hardy–Littlewood–Sobolev inequalities obtained by Frank and Lieb in [14]. (We emphasize that letting $s\nearrow 1$ one recovers from (1.11) the functions that, in the local case $s=1$, were shown to be the unique positive solutions of the CR Yamabe equation respectively in [27], for the Heisenberg group $\mathbb {H}^n$, and [24], for the quaternionic Heisenberg group. See also the important cited work [14], and [20, Theor. 1.1] and [21] for partial results in groups of Heisenberg type.)

Whether in a group of Heisenberg type $\mathbb {G}$ all nonnegative solutions of (1.1) are, up to left-translations, given by (1.11) presently remains a challenging open question. A first step in such problem is understanding the optimal decay of nonnegative solutions to (1.1). Keeping in mind that the number $m+2k$ in (1.11) represents the homogeneous dimension $Q$ of the group $\mathbb {G}$, by setting the scaling factor $y=1$, we see that there exists a universal constant $C>0$ such that

\[ u_1(z,\sigma)\le \frac{C}{|(z,\sigma)|^{Q-2s}}. \]

It is thus natural to guess that the optimal decay of all nonnegative solutions to (1.1) should be dictated by (1.6), i.e. by the fundamental solution of $\mathscr L_s$. In theorem 1.2 we prove that this guess is correct.

To facilitate the exposition of the ideas and underline the general character of our approach, in this paper we have chosen to work in the setting of homogeneous Lie groups $\mathbb {G}$ with dilations $\{\delta _\lambda \}_{\lambda >0}$, as in the seminal monograph of Folland and Stein [11]. We emphasize that such groups encompass the stratified, nilpotent Lie groups in [39], [10], and [11] (but they are a strictly larger class). In particular, our results include Lie groups of Iwasawa type for which (1.1) becomes significant in the case of pseudo-conformal CR and quaternionic contact geometry. We shall assume throughout that $|\cdot |$ is a fixed homogeneous norm in $\mathbb {G}$, i.e. $g\mapsto |g|$ is a continuous function on $\mathbb {G}$ which is $C^\infty$ smooth on $\mathbb {G}\setminus \{e\}$, where $e$ is the group identity, $|g|=0$ if and only if $g=e$, and for all $g\in \mathbb {G}$ we have

(1.12)\begin{equation} (i) \ |g^{{-}1}|=|g|; \quad (ii)\ |\delta_\lambda g|=\lambda|g|. \end{equation}

Finally, we shall assume that the fixed norm satisfies the triangle inequality:

(1.13)\begin{equation} |g\cdot h|\leq |g|+|h|, \qquad g,h\in \mathbb{G}. \end{equation}

We stress that, according to [23], any homogeneous group allows a norm which satisfies the triangle inequality. (It is well-known that in a group of Heisenberg type the anisotropic gauge $|g| = |(z,\,\sigma )| = (|z|^4 + 16 |\sigma |^2)^{1/4}$ satisfies (i)–(iii), see [7].) We shall denote with

\[ B_R(g)\equiv B(g,R)=\{h\, \mid\, |g^{{-}1}\circ h|< R\} \]

the resulting open balls with centre $g$ and radius $R$.

For $1\le p < \infty$ and $0< s<1$ we consider the Banach space $\mathcal {D}^{s,p}(\mathbb {G})$ defined as the closure of the space of functions $u\in \mathcal {C}^\infty _0 (\mathbb {G})$ with respect to the norm:

(1.14)\begin{equation} ||u||_{\mathcal{D}^{s,p}(\mathbb{G})}= [u]_{s,p} = \left(\int_{\mathbb{G}}\int_{\mathbb{G}}\frac{|u(g)-u(h)|^p}{|g^{{-}1}\cdot h|^{Q+ps}} {\rm d}g {\rm d}h\right)^{1/p} < \infty. \end{equation}

We are particularly interested in the case $p=2$. In this case, the Euler–Lagrange equation of (1.14) involves the following left-invariant nonlocal operator, initially defined on functions $u\in C^\infty _0(\mathbb {G})$

(1.15)\begin{align} \mathcal{L}_s u(g)=\frac{1}{2}\int_{\mathbb{G}} \frac{2u(g)-u(gh)-u(gh^{{-}1})}{|h|^{Q+2s}}{\rm d}h =\lim_{\varepsilon\rightarrow 0}\int_{\mathbb{G}\setminus B(g,\varepsilon)} \frac{u(g)-u(h)}{|g^{{-}1}\cdot h|^{Q+2s}}{\rm d}h, \end{align}

see [16] for a general construction of the fractional operator $\mathcal {L}_s$ on the Dirichlet space $\mathcal {D}^{s,2}(\mathbb {G})$ and relevant Sobolev-type embedding results. In (1.15), and hereafter in this work, the number $Q>0$ represents the homogeneous dimension of $\mathbb {G}$ associated with the group dilations $\{\delta _\lambda \}_{\lambda >0}$. It is clear from (1.4) that, when $\mathbb {G}$ is of Heisenberg type, the nonlocal operator $\mathcal {L}_s$ defined using the Koranyi gauge is just a multiple of $\mathscr L_s$ in (1.3), and this provides strong enough motivation to work with (1.15). A second motivation comes from [16, Theor. 1.2], in which we prove that, if $X_1,\,\ldots,\,X_m$ are the left-invariant vector fields of homogeneity one with associated coordinates $x_j$, and the fixed homogeneous norm $|g|$ is a spherically symmetric function of the coordinates $(x_1,\,\dots,\,x_m)$, then for a function $u\in C^\infty _0(\mathbb {G})$ we have the identities:

(1.16)\begin{equation} \lim_{s\rightarrow 0^+} \frac{2s}{\sigma_Q}\mathcal{L}_s u(g)={-}u(g) \quad\text{and}\quad \lim_{s\rightarrow 1^-} \frac{4m(1-s)}{\tau_m}\mathcal{L}_s u(g)={-}\sum_{i=1}^m X_i^2u(g), \end{equation}

where $\sigma _Q,\, \tau _m>0$ are suitable universal constants. Throughout the paper, for $0< s<1$ we let

(1.17)\begin{equation} 2^*(s)\overset{def}=\frac{2Q}{{Q-2s}} \qquad \text{and}\qquad (2^*(s))'=\frac{2Q}{{Q+2s}}, \end{equation}

so that $2^*(s)$, which is the Sobolev exponent associated with the fractional Sobolev inequality [16, Theorem 1.2]:

(1.18)\begin{equation} \left ( \int_{\mathbb{G}} |u|^{2^*(s)}(g){{\rm d}g}\right)^{1/2^*(s)}\leq S \left(\int_{\mathbb{G}}\int_{\mathbb{G}} \frac{|u(g)-u(h)|^2}{| h^{{-}1}\cdot g|^{Q+2s}}\, {{\rm d}g}{\rm d}h \right )^{1/2}, \end{equation}

and $(2^*(s))'$ is its Hölder conjugate. In addition to the fractional Sobolev exponent $2^*(s)$, the following exponents will be used:

(1.19)\begin{equation} r\overset{def}=\frac{2^*(s)}{2}=\frac{Q}{Q-2s}\qquad\text{and}\qquad r'=\frac{r}{r-1} = \frac{Q}{2s}. \end{equation}

With all this being said, we are ready to state our results. The first one concerns the nonlocal Schrödinger type equation (1.20). For the notion of subsolution to such equation, see (2.6).

Theorem 1.1 Let $\mathbb {G}$ be a homogeneous group. Let $u\in \mathcal {D}^{s,2}(\mathbb {G})$ be a nonnegative subsolution to the equation:

(1.20)\begin{equation} \mathcal{L}_s u=Vu. \end{equation}

Suppose the following conditions hold true:

1. (i) for some $t_0>r'= \frac {Q}{2s}$ we have $V\in L^{r'}(\mathbb {G})\cap L^{t_0}(\mathbb {G})$;

2. (ii) there exist $\bar R_0$ and $K_0$ so that for $R>\bar R_0$ we have

   (1.21)\begin{equation} \int_{\{|g|>R\}} |V(g)|^{t_0}{{\rm d}g}\leq \frac{K_0}{R^{2st_0-Q}}. \end{equation}

Then there exists a constant $C>0$, depending on $Q$, $s$, and $K_0$, such that for all $g_0\in \mathbb {G}$ with $|g_0|=2R_0\geq 4\bar R_0$, we have for $0< R\leq R_0$

(1.22)\begin{equation} \sup_{B(g_0,R/2)} u\ \leq\ C {\unicode{x2A0F}}_{B(g_0,R)} u + {C}T(u; g_0,R/2), \end{equation}

where the ‘tail’ is given by

(1.23)\begin{equation} T(u;g_0,R)=R^{2s}\int_{\{| g_0^{{-}1}\cdot h|>R\}}\frac{u(h)}{|g_0^{{-}1}\cdot h|^{Q+2s}}{\rm d}h. \end{equation}

We note that the potential $V$ in (1.20) is not assumed to be radial (i.e. a function of the norm $|\cdot |$), or controlled by a power of $u$. Hypothesis (1.21) goes back to the work [1], see also [42] where a similar assumption was used in the case of Schrödinger type equations modelled on the equations for the extremals to Hardy–Sobolev inequalities with polyradial symmetry. For other results about the Schrödinger equation see [13]. The ‘tail’ in (1.23) appeared in [35] in the setting of the Heisenberg group $\mathbb {H}^n$.

Our second result is the following theorem in which we establish the sharp asymptotic decay of weak nonnegative subsolutions to the fractional Yamabe type equation (1.1). The result applies to weak solutions of $\mathcal {L}_s u=|u|^{2^*(s)-2} u$, since then $|u|$ is a weak subsolution of the Yamabe type equation.

Theorem 1.2 Let $\mathbb {G}$ be a homogeneous group of homogeneous dimension $Q$ and $0< s<1$. If $u\in \mathcal {D}^{s,2}(\mathbb {G})$ is a nonnegative subsolution to the nonlocal Yamabe type equation

(1.24)\begin{equation} \mathcal{L}_s u=u^{\frac{Q+2s}{Q-2s}}, \end{equation}

then $|\cdot |^{{Q-2s}}\,u\in L^\infty (\mathbb {G})$.

We mention that in [3, Theor. 1.1] the authors established, in the setting of $\mathbb {R}^n$, the sharp asymptotic behaviour of the spherically symmetric extremals for the fractional $L^p$ Sobolev inequality, i.e. for the radial nonnegative solutions in $\mathbb {R}^n$ of the equation with critical exponent

\[ (-\Delta_p)^s u =u^{\frac{n(p-1)+sp}{n-sp}}, \]

where $0< s<1$, $1< p<\frac {n}s$, see also [34]. However, both [3] and [34] use in a critical way the monotonicity and radial symmetry of the solutions in order to derive the asymptotic behaviour from the regularity of $u$ in the weak space $L^{r,\infty }$, where $r = \frac {n(p-1)}{n-sp}$. As it is well-known, in the Euclidean setting one can use radially decreasing rearrangement or the moving plane method to establish monotonicity and radial symmetry of solutions to variational problems and partial differential equations. These tools are not available in Carnot groups and proving the relevant symmetries of similar problems remains a very challenging task.

The result of theorem 1.2 does not rely on the symmetry of the solution, hence the method of proof is new even in the Euclidean setting. In order to obtain the optimal decay theorem 1.2 without relying on symmetry of the solution, we use a version of the local boundedness estimate given in theorem 1.1 and then obtain a new estimate of the tail term, which is particular for the fractional case.

In closing, we provide a brief description of the paper. In § 2 we introduce the geometric setting of the paper and the relevant definitions. We also prove proposition 2.1, a preparatory result which provides regularity in $L^p$ spaces for subsolutions of fractional Schrödinger equations. In § 3 we prove theorem 1.1. Finally, in § 4 we prove theorem 1.2.

2. Homogeneous groups and fractional operators

This section is devoted to providing the necessary background and stating a preliminary result, proposition 2.1. Let $\mathbb {G}$ be a homogeneous group as defined in [11, Chapter 1]. In particular, $(\mathbb {G},\,\circ )$ is a connected simply connected nilpotent Lie group. Furthermore, the exponential map $\exp :\mathfrak g\rightarrow \mathbb {G}$ is a diffeomorphism of the Lie algebra $\mathfrak g$ onto the group $\mathbb {G}$ and $\mathfrak g$ is endowed with a family of non-isotropic group dilations $\delta _\lambda$ for $\lambda >0$. Explicitly, there is a basis $X_j$, $j=1,\,\dots,\,n$ of the Lie algebra $\mathfrak g$ and positive real numbers $d_j$, such that,

\[ 1=d_1\leq d_2\leq\dots\leq d_n \quad \text{and}\quad \delta_\lambda X_j=\lambda^{d_j} X_j, \]

which, using the exponential map, define 1-parameter family of automorphisms of the group $\mathbb {G}$ given by $\exp \circ \delta _\lambda \circ \exp ^{-1}$. We will use the same notation $\delta _\lambda$ for the group automorphisms. As customary, we indicate with

\[ Q=d_1+\dots+d_n \]

the homogeneous dimension of $\mathbb {G}$ with respect to the nonisotropic dilations $\delta _\lambda$. We will denote with $\, {\rm d}{g}$ a fixed Haar measure given by the push forward of the Lebesgue measure on the Lie algebra via the exponential map, see [11, Proposition 1.2]. We note that this gives a bi-invariant Haar measure. Furthermore, the homogeneous dimension and the Haar measure are related by the identity $d(\delta _\lambda g)=t^Q\, {\rm d}g$.

The polar coordinates formula for the Haar measure gives the existence of a unique Radon measure ${\rm d}\sigma (g)$, such that, for $u\in L^1(\mathbb {G})$ we have the identity, [11, Prop. (1.15)]:

(2.1)\begin{equation} \int_{\mathbb{G}} u(g){\rm d}g=\int_0^\infty\int_{\{|g|=1\}} u(\delta_r g) r^{Q-1}{\rm d}\sigma(g){\rm d}r. \end{equation}

In particular, we have, see [8]:

(2.2)\begin{equation} \int_{\{r < |g| < R\}}|g|^{-\gamma}\, {\rm d}{g}=\left\{ \begin{array}{@{}ll} \displaystyle\dfrac{\sigma_Q}{Q-\gamma}\left(R^{Q-\gamma} - r^{Q-\gamma} \right), & \gamma\not=Q\\ \sigma_Q\log(R/r), & \gamma=Q, \end{array} \right. \end{equation}

where $\sigma _Q = Q \omega _Q$, and $\omega _Q = \int _{B_1} {\rm d}g>0$.

2.1. The fractional operator

For $0< s<1$ consider the quadratic form:

\[ \mathscr{Q}_s(u,\phi)\overset{def}= \int_{\mathbb{G}}\int_{\mathbb{G}} \frac{(u(g)-u(h))(\phi(g)-\phi(h))}{|g^{{-}1}\cdot h|^{Q+2s}} {{\rm d}g}{\rm d}h. \]

Following [16], we let $\mathcal {D}^{s,2}(\mathbb {G})$ be the fractional Sobolev space defined as the closure of $C^\infty _0(\mathbb {G})$ with respect to the case $p=2$ of the seminorm (1.14), i.e.

(2.3)\begin{equation} [u]_{s,2} = \mathscr{Q}_s(u,u)^{1/2} = \left(\int_{\mathbb{G}}\int_{\mathbb{G}}\frac{|u(g)-u(h)|^2}{|g^{{-}1}\cdot h|^{Q+2s}}\, {{\rm d}g}{\rm d}h\right)^{1/2}. \end{equation}

The infinitesimal generator of the quadratic form $\mathscr {Q}_s(u,\,\phi )$ is the nonlocal operator $\mathcal {L}_s$ defined in (1.15). By a weak solution of the equation $\mathcal {L}_s u =F$ we intend a function $u\in \mathcal {D}^{s,2}(\mathbb {G})$ such that for any $\phi \in C^\infty _0(\mathbb {G})$ one has:

(2.4)\begin{equation} \mathscr{Q}_s(u,\phi) = \int_{\mathbb{G}}\int_{\mathbb{G}} \frac{(u(g)-u(h))(\phi(g)-\phi(h))}{|g^{{-}1}\cdot h|^{Q+2s}}\, {{\rm d}g}{\rm d}h=\int_{\mathbb{G}}F(g)\phi(g){{\rm d}g}. \end{equation}

Weak subsolutions are defined by requiring

\[ \mathscr{Q}_s(u,\phi) \leq \int_{\mathbb{G}}F(g)\phi(g){{\rm d}g} \]

for all non-negative test functions $\phi$. As shown in [16, Theorem 1.1] this is equivalent to defining the fractional operator $\mathcal {L}_s u$ by formula (1.15). As we have underlined in § 1, besides the Euclidean case $\mathbb {G} = \mathbb {R}^n$, in a Lie group of Heisenberg type, equipped with the Koranyi norm, definition (1.15) equals, up to a multiplicative constant, the fractional powers of the conformally invariant (or geometric) horizontal Laplacian defined by (1.3), see [12, 37] and [17].

2.2. A preparatory result on Lebesgue space regularity

In the proof of theorem 1.1 we will need the following regularity in Lebesgue spaces involving the fractional operator (1.15). In its proof we adapt the arguments that in the local case were developed in [20, Lemma 10.2], [40, Theor. 4.1], and [41, Theor. 2.5], except that in the nonlocal case one has to use the Sobolev inequality (1.18), rather than the Folland–Stein embedding $\mathcal {D}^{1,2}(\mathbb {G}) \hookrightarrow L^{\frac {2Q}{Q-2}}(\mathbb {G})$. As far as part (b) of proposition 2.1 is concerned, in addition to the cited references we also mention [15, Sec. 4], where a similar result was proved for $L^2$ solutions, and [32, Lemma 2.3], for a closely related result concerning the Yamabe equation on the Heisenberg group $\mathbb {H}^n$. In the local case in $\mathbb {R}^n$, a sharp Lorentz space result was obtained for solutions to equations modelled on Yamabe type equations, or more generally for the Euler–Lagrange equation related to the $L^p$ Sobolev inequality. This type of result originated with the work [25], and was subsequently used to obtain the sharp $L^p$ regularity and the asymptotic behaviour for solutions of such equations, see [43, Lemma 2.2] and [3, Proposition 3.3]. These results were extended to Yamabe type equations in Carnot groups in [33, Theorem 11 and Proposition 3.2]. We mention that, since we work in the more general setting of a Schrödinger type equation, in proposition 2.1(b) we do not obtain a borderline $L^{r,\infty }(\mathbb {G})$ Lorentz regularity for the considered non-negative subsolutions, instead, we show that $u\in L^{q}(\mathbb {G})$ for $r=\frac {2^*(s)}{2}< q< \infty$. For the statement of the next proposition, the reader should keep in mind definition (1.19) of the exponents $r$ and $r'$.

Proposition 2.1 Let $\mathbb {G}$ be a homogeneous group and suppose that $u\in \mathcal {D}^{s,2}(\mathbb {G})$ be a nonnegative subsolution to the nonlocal equation

(2.5)\begin{equation} \mathcal{L}_s u=Vu, \end{equation}

with $V\in L^{r'}(\mathbb {G})$, i.e. for every $\phi \in C^\infty _0(\mathbb {G})$ such that $\phi \ge 0$ one has

(2.6)\begin{equation} \mathscr{Q}_s(u,\phi) = \int_{\mathbb{G}}\int_{\mathbb{G}} \frac{(u(g)-u(h))(\phi(g)-\phi(h))}{|g^{{-}1}\cdot h|^{Q+2s}}\, {{\rm d}g}{\rm d}h\leq \int_{\mathbb{G}} V(g)u(g)\phi(g){{\rm d}g}. \end{equation}

1. (a) We have $u\in L^q(\Omega )$ for every $2^*(s)\ \leq \ q < \infty.$ Furthermore, for any $2^*(s)< q<\infty$ there exist a constant $C_Q>0$, such that for all sufficiently large $M$ for which

   (2.7)\begin{equation} \left(\int_{\{|V|>M\}} V^{r'}{\rm d}g \right)^{1/r'}\leq \frac{1}{qC_Q}, \end{equation}
   one has
   \[ \lVert u\rVert_{L^q(\mathbb{G})}\leq (qC_Q M)^{1/q}\lVert u\rVert_{\mathcal{D}^{s,2}(\mathbb{G})}. \]

2. (b) In fact, it holds $u\in L^{q}(\mathbb {G})$ for $r=\frac {2^*(s)}{2}< q< \infty$.

3. (c) If, in addition, $V\in L^{t_0}(\mathbb {G})$ for some $t_0 > r'$, then $u\in L^{q}(\mathbb {G})$ for $r=\frac {2^*(s)}{2}< q\leq \infty$. In addition, the sup of $u$ is estimated as follows:

   \[ \lVert u\rVert_{L^\infty(\mathbb{G})} \ \leq\ C_Q \, \lVert V\rVert_{L^{t_0}(\mathbb{G})}^{\frac{t_0'r}{r-t_0'}} \, \lVert u\rVert_{L^{2^*(s)t_0'}(\mathbb{G})}, \]
   where $t_0'$ is the Hölder conjugate to $t_0$ and $C_Q$ is a constant depending on the homogeneous dimension.

Proof. We begin by recalling a few basic facts which are crucial for working with appropriate test functions in the weak formulation of the nonlocal equation (2.5). First, using Hölder's inequality and the definition of the homogeneous fractional Sobolev space $\mathcal {D}^{s,2}(\mathbb {G})$, we can take $\phi \in \mathcal {D}^{s,2}(\mathbb {G})$ in the weak formulation (2.6). For a globally Lipschitz function $F$ defined on $\mathbb {R}$ and a function $u\in \mathcal {D}^{s,2}(\mathbb {G})$ we have from (2.3) the inequality

\[ [F\circ u]_{s,2}\leq \lVert F'\rVert_{L^\infty(\mathbb{R})} [u]_{s,2}, \]

hence $F\circ u\in \mathcal {D}^{s,2}(\mathbb {G})$. Assuming, in addition, that $F$ is of the form $F(t)=\int _0^t G'(\tau )^2 {\rm d}\tau$, then from Jensen's inequality we have for any nonnegative numbers $a\le b$ the inequality:

\[ \left(\frac{G(b) - G(a)}{b-a}\right)^2 = \left(\frac{1}{b-a}\int_a^b G'(\tau){\rm d}\tau\right)^2\le \frac{1}{b-a} \int_a^b G'(\tau)^2 {\rm d}\tau = \frac{F(b)-F(a)}{b-a}, \]

which gives

(2.8)\begin{equation} (b-a)\left(F(b)-F(a) \right)\geq \left(G(b)-G(a) \right)^2. \end{equation}

Applying the Sobolev inequality (1.18) to the function $G\circ u$, and using (2.8), we find

(2.9)\begin{align} \lVert G\circ u\rVert_{L^{2^*(s)}(\mathbb{G})}^{2}& \leq S^2 \int_{\mathbb{G}}\int_{\mathbb{G}} \frac{|G\circ u(g)-G\circ u(h)|^2}{| h^{{-}1}\cdot g|^{Q+2s}}\, {{\rm d}g}{\rm d}h \\ & \leq S^2 \int_{\mathbb{G}}\int_{\mathbb{G}} \frac{(u(g)-u(h))(F\circ u(g)-F\circ u(h))}{|g^{{-}1}\cdot h|^{Q+2s}}\, {{\rm d}g}{\rm d}h\notag\\ & \le S^2 \int_{\mathbb{G}}V(g) u(g) (F\circ u)(g) {{\rm d}g}, \nonumber \end{align}

where in the last inequality we have used (2.6) with the choice $\phi = F\circ u$ as a test function.

For the proof of parts (a) and (c) see for example [20, Lemma 10.2] and [40, Theorem 4.1], but one has to use the fractional Sobolev inequality (1.18) rather than the Folland–Stein inequality. We give the proof of part (c) below taking into account also [3, Proposition 3.3] which dealt with the Euler–Lagrange equation of the fractional $p$-Laplacian in the Euclidean setting.

To prove (b) in proposition 2.1 we will show that for any $0<\alpha <1$ we have that $u\in L^{r(1+\alpha )}(\mathbb {G})$. From part (a) and the fact that $2^*(s)/2 < r(1+\alpha )<2r=2^*(s)$ the claim of part (b) will be proven. The details are as follows. For $\varepsilon >0$ and $0<\alpha <1$, consider the functions:

\[ F_\varepsilon(t)=\int_0^t G'_\varepsilon(\tau)^{2}{\rm d}\tau,\quad\text{ where } G_\varepsilon(t)=t(t+\varepsilon)^{(\alpha-1)/2}. \]

Notice that $F_\varepsilon$ is nondecreasing by definition. A simple calculation shows that

(2.10)\begin{equation} 0 \le G'_\varepsilon(t) = \frac{1}{(t + \varepsilon)^{(3-\alpha)/2}}\left [\frac{1+\alpha}{2} t + \varepsilon \right] \leq \frac{1}{(t + \varepsilon)^{(1-\alpha)/2}}\leq \frac{1}{ \varepsilon^{(1-\alpha)/2}}, \end{equation}

where we have used that $\alpha <1$. This shows in particular that $F'_\varepsilon (t) = G'_\varepsilon (t)^2 \le \varepsilon ^{\alpha -1}$, therefore $F_\varepsilon$ is a globally Lipschitz function. We thus find from (2.9):

(2.11)\begin{equation} \lVert G_\varepsilon\circ u\rVert_{L^{2^*(s)}(\mathbb{G})}^{2}\leq S^2\int_{\mathbb{G}}|V| uF_\varepsilon(u){\rm d}g. \end{equation}

In order to estimate the right-hand side in (2.11) we will use the following inequalities, which are valid for $u\ge 0$:

(2.12)\begin{equation} F_\varepsilon(u)\leq \frac{1}{\alpha}u^\alpha\qquad \text{and} \qquad uF_\varepsilon(u)\leq \frac{1}{\alpha} G_\varepsilon(u)^2. \end{equation}

The former is easily proved by noting that:

\[ F_\varepsilon(u)\leq \int_0^u \frac{{\rm d}t}{(t + \varepsilon)^{1-\alpha}} \le \frac{(u+\varepsilon)^{-\alpha}}{\alpha} \leq \frac{1}{\alpha}u^\alpha. \]

This estimate trivially gives $u F_\varepsilon (u) \le u \frac {(u+\varepsilon )^\alpha - \varepsilon ^\alpha }{\alpha }$, and therefore from the definition of $G_\varepsilon$ we see that the latter inequality in (2.12) does hold provided that

\[ (u+\varepsilon)^\alpha - \varepsilon^\alpha \le \frac{u}{u+\varepsilon}(u+\varepsilon)^{\alpha},\quad \text{i.e.}~ (u+\varepsilon)^{\alpha-1} - \varepsilon^{\alpha-1} \leq 0. \]

The latter inequality follows from the trivial inequality

\[ (1+x)^{1-\alpha}\geq 1 \]

valid for $x\geq 0$ and $0<\alpha <1$.

Keeping in mind definition (1.19) of the exponents $r$ and $r'$, using now in (2.11) the first inequality in (2.12) and Hölder inequality, we easily obtain for a fixed $\delta >0$:

(2.13)\begin{align} \lVert G_\varepsilon\circ u\rVert_{L^{2^*(s)}(\mathbb{G})}^{2}& \leq S^2\left( \frac{1}{\alpha}\int_{\{|V|>\delta\}}|V|u^{1+\alpha}{\rm d}g + \int_{\{|V|\leq \delta\}}|V|u F_\varepsilon(u){\rm d}g\right)\\ & \leq S^2 \left[ \frac{1}{\alpha} \left(\int_{\{|V|>\delta\}}|V|^{r'}{\rm d}g\right)^{1/r'} \left(\int_{\{|V|>\delta\}}u^{r(1+\alpha)}{\rm d}g \right)^{1/r} \right] \nonumber\\ & \qquad+ S^2\left[ \left(\int_{\{|V|\leq \delta\}}|V|^{r'}{\rm d}g\right)^{1/r'} \left(\int_{\{|V|\leq \delta\}}(uF_\varepsilon(u))^{r}{\rm d}g \right)^{1/r}\right]. \nonumber \end{align}

Next, we use the second of inequalities (2.12) to obtain the estimate:

(2.14)\begin{align} \left(\int_{\{|V|\leq \delta\}}(uF_\varepsilon(u))^{r}{\rm d}g \right)^{1/r}\leq \frac{1}{\alpha}\left(\int_{\{|V|\leq \delta\}}\left(G_\varepsilon(u)\right)^{2r} \right)^{1/r}\leq \frac{1}{\alpha}\lVert G_\varepsilon\circ u\rVert_{L^{2^*(s)}(\mathbb{G})}^{2}. \end{align}

By Lebesgue-dominated convergence one has $\int _{\{|V|\leq \delta \}}|V|^{r'}{\rm d}g\longrightarrow 0$ as $\delta \to 0^+$. Therefore, we can choose $\delta >0$ so small that

(2.15)\begin{equation} \frac{S^2}{\alpha}\left(\int_{\{|V|\leq \delta\}}|V|^{r'}{\rm d}g\right)^{1/r'} <\frac 12. \end{equation}

Combining (2.15) with (2.14) we can absorb in the left-hand side the second term in the right-hand side of (2.13), obtaining the inequality:

(2.16)\begin{equation} \lVert G_\varepsilon\circ u\rVert_{L^{2^*(s)}(\mathbb{G})}^{2}\leq \frac{2S^2}{\alpha}\left(\int_{\{|V|>\delta\}}|V|^{r'}{\rm d}g\right)^{1/r'} \left(\int_{\{|V|>\delta\}}u^{r(1+\alpha)}{\rm d}g \right)^{1/r}. \end{equation}

Notice that the hypothesis $V\in L^{r'}(\mathbb {G})$ and Chebyshev inequality imply that the distribution function of $V$ satisfies for every $\delta >0$:

(2.17)\begin{equation} \mu(\delta)=\left\vert\{g\in \mathbb{G}\mid |V(g)|>\delta \}\right\vert \leq \frac{1}{\delta^{r'}} \int_{\{|V|>\delta\}} |V|^{r'} {\rm d}g < \infty. \end{equation}

Since $r(1+\alpha )<2r=2^*(s)$, Hölder inequality thus gives

\[ \left({\unicode{x2A0F}}_{\{|V|>\delta\}} u^{r(1+\alpha)}{\rm d}g\right)^{\frac{1}{r(1+\alpha)}}\leq \left({\unicode{x2A0F}}_{\{|V|>\delta\}} u^{2^*(s)}{\rm d}g\right)^{\frac{1}{2^*(s)}}, \]

or equivalently, recalling that $2r=2^*(s)$:

(2.18)\begin{align} \left(\int_{\{|V|>\delta\}} u^{r(1+\alpha)}{\rm d}g\right)^{\frac{1}{r}} & \leq \mu(\delta)^{\frac{1}{r}-\frac{1+\alpha}{2r}} \left(\int_{\{|V|>\delta\}} u^{2^*(s)}{\rm d}g\right)^{\frac{1+\alpha}{2^*(s)}}\\ & = \mu(\delta)^{\frac{1-\alpha}{2r}} \left(\int_{\{|V|>\delta\}} u^{2^*(s)}{\rm d}g\right)^{\frac{1+\alpha}{2^*(s)}}. \nonumber \end{align}

Using (2.17), (2.18), and $r'/r=1/(1-r)$ in (2.16) we obtain:

\[ \lVert G_\varepsilon\circ u\rVert_{L^{2^*(s)}(\mathbb{G})}^{2}\leq \frac{2S^2}{\alpha} \frac{\lVert V\rVert_{L^{r'(\mathbb{G})}}^{1+\frac{1-\alpha}{2(1-r)}}}{\delta^{\frac{1-\alpha}{2(1-r)}}} \lVert u\rVert^{1+\alpha}_{L^{2^*(s)}(\mathbb{G})}. \]

Letting $\varepsilon$ go to 0, noting that $\lim _{\varepsilon \rightarrow 0}G_\varepsilon (u)=u^{(1+\alpha )/2}$ gives:

\[ \left( \int_{\mathbb{G}}u^{(1+\alpha)r}\right)^{1/r}<\infty, \]

which, taking into account also part (a), completes the proof of part (b) of proposition 2.1.

3. Proof of theorem 1.1

The proof consists of several steps detailed in the following sub-sections.

3.1. The localized fractional Sobolev inequality

The proof of theorem 1.1 will use the following version of a localized fractional Sobolev inequality. For an open set $\Omega \subset \mathbb {G}$ we denote by $\mathcal {D}^{s,2}(\Omega )$ the completion of $C_0^\infty (\Omega )$ with respect to the norm:

\[ \lVert v\rVert_{\mathcal{D}^{s,2}(\Omega)}=\left(\int_{\mathbf{G}}\int_{\mathbf{G}} \frac{|\tilde v(g)-\tilde v(h)|^2}{|g^{{-}1}\cdot h|^{Q+2s}}\right)^{1/2}, \]

where $\tilde v$ denotes the extension of $v$ to a function on $\mathbb {G}$, which is equal to zero outside of $\Omega$.

Lemma 3.1 Let $0< s<1$ and $2s< Q$. There exists a constant $C=C(Q,\,s)>0$ such that, for any ball $B_R$ of radius $R$, $r< R$, and $v\in \mathcal {D}^{s,2}(B_R)$ with $\mathrm {supp}\, v\subset B_{r }$ we have

(3.1)\begin{align} & \left[\int_{B_R}|v|^{2^*(s)} \, {\rm d}{h}\right]^{2/2^*(s)}\notag\\ & \quad \leq C\left [ \int_{B_R}\int_{B_R} \frac{| v(g)-v(h)|^2}{|g^{{-}1}\cdot h|^{Q+2s}}{\rm d}g{\rm d}h + \frac{1}{R^{2s}}\left(\frac{R}{R-r}\right)^{Q+2s}\int_{B_R}|v|^2 \, {\rm d}{h}\right]. \end{align}

Proof. The proof is essentially contained in the Euclidean version [4, Proposition 2.3]. We will use the trivial extension and then apply the fractional Sobolev inequality (1.18). Since $v$ has compact support in $B_R$ its extension by zero on the complement of the ball is a function $\tilde v\in \mathcal {D}^{s,2}(\mathbb {G})$. Furthermore, due to the assumption on the support of $v$, we have

(3.2)\begin{align} \lVert \tilde v\rVert^2_{\mathcal{D}^{s,2}(\mathbb{G})}& \leq \int_{B_R}\int_{B_R} \frac{|v(g)-v(h)|^2}{|g^{{-}1}\cdot h|^{Q+2s}}{\rm d}g{\rm d}h + 2 \int_{B_{r }}|v(g)|^2\int_{\mathbb{G}\setminus B_R} \frac{1}{|g^{{-}1}\cdot h|^{Q+2s}} {\rm d}h\, {\rm d}g\\ & \leq \int_{B_R}\int_{B_R} \frac{|v(g)-v(h)|^2}{|g^{{-}1}\cdot h|^{Q+2s}}{\rm d}g{\rm d}h + 2 \int_{\mathbb{G}\setminus B_R} \sup_{g\in B_{r}}\frac{1}{|g^{{-}1}\cdot h|^{Q+2s}} {\rm d}h\, \int_{B_R}|v(g)|^2 {\rm d}g\nonumber\\ & \leq \int_{B_R}\int_{B_R} \frac{|v(g)-v(h)|^2}{|g^{{-}1}\cdot h|^{Q+2s}}{\rm d}g{\rm d}h + C\left(\frac{R}{R-r}\right)^{Q+2s}\frac{1}{R^{2s}}\, \int_{B_R}|v(g)|^2 {\rm d}g. \nonumber \end{align}

In order to see the last of the above inequalities we used polar coordinates as in identity (2.2) to obtain the following inequalities, where $\sigma _Q$ is the area of the unit sphere:

\begin{align*} \int_{\mathbb{G}\setminus B_R} \sup_{g\in B_{r}}\frac{1}{|g^{{-}1}\cdot h|^{Q+2s}} {\rm d}h & \leq \int_{B_{2R}\setminus B_R} \frac{1}{\left(|h|-r\right)^{Q+2s}} {\rm d}h + \int_{\mathbb{G}\setminus B_{2R}} \frac{1}{|g^{{-}1}\cdot h|^{Q+2s}} {\rm d}h\\ & \leq \sigma_Q\left[ \int_R^{2R} \frac{t^{Q-1}}{\left(t-r\right)^{Q+2s}} {\rm d}t + \int_{2R}^\infty \left(\frac{2}{t}\right)^{Q+2s} t^{Q-1}{\rm d}t\right] \end{align*}

since $|g^{-1}\cdot h|\geq |h|-r\geq |h|/2$ when $r< R$, $|g|< r$, and $|h|\geq 2R$. Therefore, we have

\[ \int_{\mathbb{G}\setminus B_R} \sup_{g\in B_{r}}\frac{1}{|g^{{-}1}\cdot h|^{Q+2s}} {\rm d}h\leq C\left[ \frac{R^Q}{Q+2s} \left(\frac{1}{R-r} \right)^{Q+2s}+ \frac{1}{2s}\frac{2^{Q+2s}}{R^{2s}}\right], \]

which gives (3.2). The latter implies trivially (3.1).

3.2. Caccioppoli inequality

We begin by stating the adaptation to our setting of the Caccioppoli inequality for the fractional $p$-Laplacian in Euclidean space [4]. For $\beta \geq 1$ and $\delta >0$ define the following functions for $t\geq 0$:

(3.3)\begin{equation} \phi(t)=(t+\delta)^\beta, \qquad \Phi(t)=\int_0^t|\phi'(\tau)|^{1/2} \, {\rm d}\tau =2\frac{\beta^{1/2}}{\beta+1}(t+\delta)^{(\beta+1)/2}. \end{equation}

For our goals, the precise value of $\delta$ is given in (3.9). Suppose $\Omega '\Subset \mathbb {G}$ and $\psi \in C ^\infty _0(\mathbb {G})$ is a positive function with supp$\, \psi \subset \Omega '$. Let $u$ be a weak nonnegative subsolution to the equation $\mathcal {L}_s u =F$ with $F\in L^{(2^*(s))'}$. Then, we have for some constant $C=C(Q)$, which is independent of $\Omega '$, the inequality:

(3.4)\begin{equation} \begin{aligned} & \int_{\Omega'}\int_{\Omega'} \frac{\Big|\Phi(u(g))\,\psi(g)-\Phi(u(h))\,\psi(h)\Big|^{2}\,}{|g^{{-}1}\cdot h|^{Q+2s}}\leq C\int_\mathbb{G} |F|\,\phi(u(g))\, \psi^2(g)\, {\rm d}g\\ & \quad+ \frac{C}{\beta}\,\left(\frac{\beta+1}{2}\right)^2\,\int_{\Omega'}\int_{\Omega'} \frac{|\psi(g)-\psi(h)|^2}{|g^{{-}1}\cdot h|^{Q+2s}}\,\Big((\Phi(u(g))^2+\Phi(u(h))^2\Big)\, {\rm d}g\,{\rm d}h\\ & \quad+C\,\left(\sup_{h\in \mathrm{supp}\,\psi} \int_{\mathbb{G}\setminus \Omega'} \frac{|u(g)|}{|g^{{-}1}\cdot h|^{Q+2s}}\,{\rm d}g\right)\, \int_{\Omega'} \phi(u)\, \psi^2\, {\rm d}g. \end{aligned} \end{equation}

The proof of the above formula follows from the proof of the localized Caccioppoli inequality [4, Proposition 3.5] after letting $p=2$, replacing the ambient space with the considered homogeneous group and using its homogeneous dimension instead of the Euclidean dimension.

We now fix $g_0\in \mathbb {G}$, and for $0< r< R$, we take a nonnegative smooth bump function $\psi \in C ^\infty _0(\mathbb {G})$ such that

(3.5)\begin{align} \psi\vert_{B_r(g_0)}\equiv 1,\qquad \mathrm{supp}\, \psi\Subset B_{\frac{r+R}{2}}(g_0), \qquad |\psi(g_1)-\psi(g_2)|\leq \frac{C}{R-r} |g_1^{{-}1}\cdot g_2| . \end{align}

In order to achieve (3.5) we take a cut-off function $\psi (g)=\eta (|g_0^{-1}\cdot g|)$, where $\eta$ is a smooth bump function on the real line, such that, $\eta (t)\equiv 1$ on $|t|\leq r$, $\eta \equiv 0$ on $t\geq (R+r)/2$ and for some constant $K>0$ we have $|\eta '(t)|\leq K/(R-r)$ for all $t$. Hence, for any $\rho _1,\, \rho _2\in \mathbb {R}$ we have

\[ |\eta(\rho_1)-\eta(\rho_2)|\leq \frac{K}{R-r}|\rho_1-\rho_2|. \]

Furthermore, if we let $\rho (g)=| g^{-1}\cdot g_o|$, then from the triangle inequality (1.13) it follows that $\rho$ is a Lipschitz continuous function with respect to the gauge distance, with Lipschitz constant equal to 1:

\[ |\rho(g_1)-\rho(g_2)|\leq |g_1^{{-}1}\cdot g_2|, \qquad g_1, g_2\in \mathbb{G} \]

Therefore, for $g_j\in \mathbb {G}$ and $\rho _j=\rho (g_j)$, $j=1,\,2$, we have

\[ |\psi(g_1)-\psi(g_2)|=|\eta(\rho_1)-\eta(\rho_2)|\leq \frac{K}{R-r} |\rho_1-\rho_2|\leq \frac{K}{R-r}|g_1^{{-}1}\cdot g_2|. \]

For the remainder of the proof, for any $r>0$ we will denote by $B_r$ the ball $B_r(g_0)$ with the understanding that the centre is the fixed point $g_0$.

If $u$ is a nonnegative weak subsolution to

\[ \mathcal{L}_s u=Vu, \]

then, with the above choice of $\psi$ and $F=Vu$, (3.4) implies the following inequality:

(3.6)\begin{align} & \int_{B_R}\int_{B_R}\frac{|u_\delta(g)^{(\beta+1)/2}\psi(g)-u_\delta(h)^{(\beta+1)/2}\psi(h)|^2}{|g^{{-}1}\cdot h|^{Q+2s}} \, {\rm d}g\, {\rm d}h \\ & \quad\leq C \beta \left[ \int_{B_R}\psi^2\,V u_\delta^{\beta+1}\, {\rm d}{h} + \ \left(\frac{R}{R-r} \right)^{2} \frac{1}{R^{2s}} \int_{B_R}u_\delta^{\beta+1}\, {\rm d}{h}\right. \notag\\ & \qquad + \left. \left(\frac{R}{R-r}\right)^{Q+2s} \frac{1}{R^{2s}} T(u;g_0,R) \int_{B_R}u_\delta^{\beta} \, {\rm d}{h}\right], \nonumber \end{align}

where $u_\delta =u+\delta$ and $T(u;g_0,\,R)$ is the tail (1.23). The proof of (3.6) is contained in [4, Theorem 3.8 and (3.29)], except that we have to use the Lipschitz bound in (3.5) for the term $|\psi (g)-\psi (h)|^2$ in (3.4).

Next, we apply to inequality (3.6) the localized Sobolev inequality (3.1), with $r$ replaced with $(R+r)/2$ and $v$ with $\psi \,u_\delta ^{(\beta +1)/2}$, taking into account

\[ \left(\frac{R}{R-r} \right)^2 \leq \left(\frac{R}{R-r}\right)^{Q+2s} \]

and also that by the choice of $\psi$ we have $\mathrm {supp}\, (\psi \,u_\delta ^{(\beta +1)/2})\Subset B_{(R+r)/2}\Subset B_R$ . As a result, we obtain:

(3.7)\begin{align} & \left[ \int_{B_{\frac{R+r}{2}}}\psi^{2^*(s)}u_\delta^{(\beta+1)2^*(s)/2 } \, {\rm d}{h}\right]^{2/2^*(s)}\leq C\beta \left[\int_{B_R}\psi^2Vu_\delta^{\beta+1}\, {\rm d}{h}\right.\\ & \quad\left. + \left(\frac{R}{R-r}\right)^{Q+2s}\frac{{1}}{R^{2s}} \int_{B_R}u_\delta^{\beta+1}\, {\rm d}{h} + \left(\frac{R}{R-r}\right)^{Q+2s}\frac{1}{R^{2s}}T(u;g_0,R) \int_{B_R}u_\delta^{\beta}\, {\rm d}{h}\right]. \nonumber \end{align}

3.3. Use the assumptions on $V$

This is the core of the new argument leading to our result. By Hölder's inequality and $\mathrm {supp} \,\psi \Subset B_{(R+r)/2}$, we have

\begin{align*} \int_{B_R}\psi^2Vu_\delta^{\beta+1}\, {\rm d}{h}\ & \leq\ \left( \int_{B_R}V^{t_0}\, {\rm d}{h}\right)^{1/t_0} \left( \int_{B_{\frac{R+r}{2}}} \left(\psi\, u_\delta^{\frac{\beta+1}{2}}\right)^{2^*(s)}\, {\rm d}{h}\right)^{1/t}\\ & \quad \times \left( \int_{B_{\frac{R+r}{2}}} \left(\psi\, u_\delta^{\frac{\beta+1}{2}} \right)^2 \, {\rm d}{h} \right)^{1/\kappa}, \end{align*}

where

\[ t=\frac{2st_0}{Q-2s}\qquad \text{and} \qquad \kappa=\frac{2st_0}{2st_0-Q} \]

so that

\[ \frac{1}{t_0}+\frac 1t+\frac 1{\kappa}=1\qquad \text{and}\qquad \frac{2^*(s)/2}{t}+\frac{1}{\kappa}=1, \]

which is possible due to the assumptions in theorem 1.1. Next, we use Young's inequality $ab\leq \varepsilon \frac {a^{\kappa '}}{\kappa '}+\frac {1}{\varepsilon ^{\kappa -1}}\frac {b^\kappa }\kappa$ in the right-hand side of the above inequality to conclude:

\begin{align*} \int\psi^2Vu_\delta^{\beta+1} \, {\rm d}{h} & \leq \frac{\varepsilon}{\kappa'}\left( \int_{B_{\frac{R+r}{2}}} \left(\psi\, u_\delta^{\frac{\beta+1}{2}}\right)^{2^*(s)}\, {\rm d}{h} \right)^{\kappa'/t}\\ & \quad + \ \frac{1}{\varepsilon^{\kappa-1}\kappa}\left( \int_{B_R}V^{t_0}\, {\rm d}{h}\right)^{\kappa/t_0}\left( \int_{B_{\frac{R+r}{2}}} \psi^2\, u_\delta^{\beta+1}\, {\rm d}{h} \right). \end{align*}

Hence, taking into account $\kappa '/t=2/2^*(s)$, $\kappa /t_0=\frac {2s}{2st_0-Q}$ and the above inequality together with the properties of $\psi$, we obtain from (3.7) the following inequality:

\begin{align*} & \left[ \int_{B_{\frac{R+r}{2}}} \psi^{2^*(s)}u_\delta^{(\beta+1)\frac{2^*(s)}{2} } \, {\rm d}{h}\right]^{\frac{2}{2^*(s)}}\\ & \quad \leq \beta \left \{\frac{C\varepsilon}{\kappa'}\left[ \int_{B_{\frac{R+r}{2}}}\psi^{2^*(s)} u_\delta^{(\beta+1)\frac{2^*(s)}{2} }\, {\rm d}{h} \right]^{\frac{2}{2^*(s)}} \right.\\ & \qquad+ \frac{C}{\varepsilon^{\kappa-1}\kappa}\left( \int_{B_R}V^{t_0}\, {\rm d}{h}\right)^{\frac{2s}{2st_0-Q}}\left( \int_{B_{R}} u_\delta^{\beta+1} \, {\rm d}{h}\right)\\ & \qquad+ \left. C\left(\frac{R}{R-r}\right)^{Q+2s}\left[ \frac{1}{R^{2s}} \int_{B_R}u_\delta^{\beta+1}\, {\rm d}{h} + \frac{1}{R^{2s}}T(u;g_0,R) \int_{B_R}u_\delta^{\beta}\, {\rm d}{h}\right] \right \} . \end{align*}

Choosing $\varepsilon$ such that $\frac {C\varepsilon \beta }{\kappa '}=\frac 12$, we absorb the first term on the right-hand side in the left-hand side, and then reduce the domain of integration, taking into account that $\psi \equiv 1$ on $B_r$, which brings us to the following inequality:

\begin{align*} & \left[ \int_{B_{r}}u_\delta^{(\beta+1)\frac{2^*(s)}{2} }\, {\rm d}{h} \right]^{\frac{2}{2^*(s)}}\\ & \quad \leq C\left[\beta^{\kappa} \left (\int_{B_R}V^{t_0}\, {\rm d}{h}\right)^{\frac{2s}{2st_0-Q}} +\left(\frac{R}{R-r}\right)^{Q+2s}\frac{\beta}{R^{2s}} \right] \int_{B_R}u_\delta^{\beta+1}\, {\rm d}{h} \\ & \qquad+ C\left(\frac{R}{R-r}\right)^{Q+2s}\frac{\beta}{R^{2s}}T(u;g_0,R) \int_{B_R}u_\delta^{\beta}\, {\rm d}{h}. \end{align*}

Since $u_\delta ^\beta \leq u_\delta ^{\beta +1}/\delta$, the above inequality allows us to conclude:

\begin{align*} \left[ \int_{B_{r}}u_\delta^{(\beta+1)\frac{2^*(s)}{2} }\, {\rm d}{h} \right]^{\frac{2}{2^*(s)}}& \leq C\beta^{\kappa} \left[\left(\int_{B_R}V^{t_0}\, {\rm d}{h}\right)^{\frac{2s}{2st_0-Q}} +\left(\frac{R}{R-r}\right)^{Q+2s}\frac{1}{ R^{2s}} \right. \\ & \quad\left. + \left(\frac{R}{R-r}\right)^{Q+2s}\frac{1}{\delta R^{2s}}T(u;g_0,R) \right] \int_{B_R}u_\delta^{\beta+1}\, {\rm d}{h}. \end{align*}

We recall that in the latter inequality we have radii $0< r< R$ and all balls are centred at the fixed point $g_0$. Suppose, in addition, that $2R_0= {|g_0|}$ and $0< R\leq R_0$. Then, we have

\[ B_R=B(g_0,R)\subset B(g_0,R_0)\subset \mathbb{G}\setminus B(0,R_0), \]

taking into account the triangle inequality (1.13). Therefore, for $R_0\geq 2\bar R_0$ the decay assumption of $V$, cf. theorem 1.1(ii), and the above inclusions imply that for some constant $C= C(Q,\,s,\, K_0)$ we have the bound

\begin{align*} \left(\int_{B_R}V^{t_0}\, {\rm d}{h}\right)^{2s/(2st_0-Q)}& \leq \left(\int_{B_{R_0}}V^{t_0}\, {\rm d}{h}\right)^{2s/(2st_0-Q)}\\ & \leq \left(\int_{\{ |g|>R_0\} }V^{t_0}\, {\rm d}{h}\right)^{2s/(2st_0-Q)} \leq \frac{C}{R_0^{2s}}\leq \frac{C}{R^{2s}} \end{align*}

after using $0< R\leq R_0$ for the last inequality. Therefore, also observing that $R/(R-r)>1$, we have proven that there exists a constant $C= C(Q,\,s,\, K_0)$, such that for any $\beta \geq 1$, $g_0$ such that $R_0=\frac {|g_0|}{2}\geq \bar R_0$, and radii $0< r< R\leq R_0$ we have

(3.8)\begin{align} \left[ \int_{B_{r}}u_\delta^{(\beta+1)\frac{2^*(s)}{2} }\, {\rm d}{h} \right]^{\frac{2}{2^*(s)}}\leq \frac{C\beta^{\kappa}}{R^{2s}} \left(\frac{R}{R-r}\right)^{Q+2s}\left[1 +\frac{T(u;g_0,R)}{\delta } \right] \int_{B_R}u_\delta^{\beta+1}\, {\rm d}{h}. \end{align}

3.4. Moser's iteration

By proposition 2.1(c) we have that $u\in L^{q}(\mathbb {G})\cap L^\infty (\mathbb {G})$ for any $q\geq 2^*(s)/2$, hence $u\in L^{q_0}_{loc}(\mathbb {G})$ for $q_0\geq 2$. In fact, for the proof of the theorem we can assume $q_0=2$, but the argument is valid for any $q_0\geq 2$. We also let $\beta =q_0 -1$.

Recalling that the exponent $r=2^*(s)/2=Q/(Q-2s)>1$, see (1.19), we define the sequence

\[ q_{j+1}=r q_j>q_j, \quad j=0,1,2,\ldots. \]

From (3.8) we have with $B_{r_{j}}=B(g,\,r_j)$, $r_j=\frac {R}{2}(1+2^{-j})$, $j=0,\,1,\,2,\,\dots$ the inequality:

\begin{align*} \left(\int_{B_{r_{j+1}}}u_\delta^{q_{j+1}}\, {\rm d}{h}\right)^{1/q_{j+1}}& \leq\ \frac{C^{ 1/{q_{j}}}q_j^{\kappa/{q_j}}} {r_j^{{2s}/{q_{j}}}} \left[\left( \frac{r_j}{r_j-r_{j+1}}\right)^{Q+2s}\right]^{1/q_{j}}\left[1+ \frac{T(u,g_0,r_j)}{\delta}\right]^{1/q_{j}} \\ & \quad\times\ \left( \int_{B_{r_{j}}} u_\delta^{q_{j}}\, {\rm d}{h}\right)^{1/{q_{j}}}. \end{align*}

The definition of the tail (1.23) gives for a fixed $R\ge \bar R_0$ and $R/2\le r_j< R$ the inequality:

\[ T(u;g_0,r_j)={r_j}^{2s}\int_{| g^{{-}1}\cdot h|>r_j}\frac{u(h)}{| g^{{-}1}\cdot h|^{Q+2s}}{\rm d}h \leq 2^{2s}T(u;g_0,R/2) \]

while a simple estimate shows

\[ \left(\frac{r_j}{r_j-r_{j+1}}\right)^{Q+2s} \leq 2^{(j+2)(Q+2s)}. \]

Hence, letting

\[ M_j=\left( \int_{B_{r_{j+1}}}u_\delta^{q_{j+1}}\, {\rm d}{h}\right)^{1/q_{j+1}}\qquad\text{and}\qquad T=1+\frac{T(u;g,R/2)}{\delta} \]

we have with some constants $C_0$ and $C_1$ depending on $Q$ and $s$ the inequality:

\[ M_{j+1}\leq \frac{C_0^{ 1/q_{j}}C_1^{(j+2)/q_{j}}(q_j)^{\kappa/q_j}}{R^{2s/q_{j}}}\, T^{ 1/q_{j}}\, M_j. \]

Therefore, for

(3.9)\begin{equation} \delta=T(u;g,R/2), \end{equation}

we have $T=2$ and we obtain the inequality:

\[ M_{j+1}\ \leq\ \frac{C^{ (j+2)/{q_{j}}}(q_j)^{\kappa/q_j}} {R^{{2s}/{q_{j}}}} M_j. \]

Therefore, recalling that $q_{j+1}=r^{j} q_0$, we obtain

\[ \sup_{B(g,R/2)} (u+\delta)\ \leq\ C \left(\frac{1}{R^{2s}} \right)^{\frac{1}{q_0}\sum_{j=0}^\infty r^{{-}j} }\, C^{\frac{1}{q_0}\sum_{j=0}^\infty (j+2)r^{{-}j}}\prod_{j=0}^\infty (q_j)^{\kappa/q_j} \, M_0. \]

From the definitions of the exponents $r$ and its Hölder conjugate $r'=Q/(2s)$ we have

\[ \frac{1}{q_0}\sum_{j=0}^\infty \frac{1}{r^{j}}=\frac{1}{q_0}\frac{r}{r-1}=\frac{r'}{q_0} =\frac{Q}{2sq_0} \]

and

\[ \prod_{j=0}^\infty (q_j)^{\kappa/q_j}<\infty \quad \mbox{since}~ \sum_{i=1}^\infty \frac{\log(q_j)}{q_j}<\infty. \]

Thus, we find:

\[ \sup_{B(g_0,R/2)} (u+\delta)\ \leq\ C \left ({\unicode{x2A0F}}_{B(g_0,R)} (u+\delta)^{q_0}\, {\rm d}{h} \right)^{1/{q_0}} . \]

If we let $q_0=2$ and take into account the definition of $\delta$ we have shown that there is a constant $C_0$, depending on $Q$ and $s$, such that the inequality

(3.10)\begin{equation} \sup_{B(g_0,R/2)} u \leq C_0 \left ({\unicode{x2A0F}}_{B(g_0,R)} u^{2} \, {\rm d}{h} \right)^{1/{2}} + {C_0}T(u; g_0,R/2). \end{equation}

holds for any $g_0\in \mathbb {G}$ with $|g_0|=2R_0\geq 2\bar R_0$ and $0< R\leq R_0$, where $\bar R_0$ is the radius in the assumptions of theorem 1.1.

3.5. Lowering the exponent

To lower the exponent in the average integral in the above inequality we follow the standard argument, see for example [22, p. 223, Theorem 7.3], except that we need to account for the tail term similarly to [31, Corollary 2.1]. In view of the eventual use of the sought estimate in obtaining the asymptotic behaviour of the solution, it is also important to keep the constant in the inequality independent of $R$ as in (3.10). For any $\rho >0$, let:

\[ M_\rho\overset{def}=\sup_{B(g_0,\rho)}u. \]

First, we will show the following slight modification of (3.10). There is a constant $C_1$, depending on $Q$ and $s$, such that, for all $g_0\in \mathbb {G}$ with $|g_0|=2R_0\geq 4\bar R_0$ and $0< r< R\leq R_0$ we have

(3.11)\begin{equation} M_r\leq C_1 \left(\frac{R}{R-r}\right)^{Q}\left[\left({\unicode{x2A0F}}_{B(g_0,R)} u^{2} \, {\rm d}{h} \right)^{1/2} + T(u; g_0, R)\right]. \end{equation}

Letting $\tau =r/R$, $0<\tau <1$, the above inequality is equivalent to showing, with the same constant $C_1$, that we have

(3.12)\begin{equation} M_{\tau R}\leq \frac{C_1}{(1-\tau)^{Q}}\left[\left({\unicode{x2A0F}}_{B(g_0,R)} u^{2} \, {\rm d}{h}\right)^{1/2} + T(u; g_0, R)\right]. \end{equation}

We turn to the proof of (3.12). Let $g_1\in B(g_0,\,\tau R)$ and $\rho$ be sufficiently small, in fact,

\[ \rho=\frac{(1-\tau)R}{4}, \]

so that,

\[ B(g_1,\rho)\subset B(g_1,2\rho)\subset B(g_0, R)\quad\text{ and }\quad \sup_{B(g_0,\tau R)} u = \sup_{B(g_1,\rho)} u. \]

Notice that by the triangle inequality we have $|g_1|\geq 2\bar R_0$, which follows from $|g_0|=2R_0\geq 4\bar R_0$, cf. the line above (3.11), hence we can apply (3.10) to the ball $B(g_1,\,\rho )$, which gives

(3.13)\begin{align} M_{\tau R} & = \sup_{B(g_1,\rho)} u\leq\ C_0 \left ({\unicode{x2A0F}}_{B(g_1,2\rho)} u^{2} \, {\rm d}{h} \right)^{1/{2}} + {C_0}T(u; g_1,\rho)\\ & \leq C_02^{{-}Q/2}\left(\frac{R}{\rho} \right)^{Q/2}\left( {\unicode{x2A0F}}_{B(g_0,R)} u^{2}\, {\rm d}{h}\right)^{1/2} + {C_0}T(u; g_1,\rho)\nonumber\\ & = \frac{C_02^{Q/2}}{(1-\tau)^{Q/2}} \left( {\unicode{x2A0F}}_{B(g_0,R)} u^{2}\, {\rm d}{h}\right)^{1/2} + {C_0}T(u; g_1,\rho), \nonumber \end{align}

taking into account that by the definition of $\rho$ we have $R/\rho =\frac {4}{1-\tau }$. We will estimate the tail term in the last line by using the tail term centred at $g_0$ and radius $R$, and the average of $u$ over the ball $B(g_0,\,R)$. For this we split the domain of integration of the integral in the formula for the tail,

\[ T(u; g_1,\rho)=\rho^{2s}\int_{\mathbb{G}\setminus B(g_1,\rho)} \frac{u(h)}{|h^{{-}1}\cdot g_1|^{Q+2s}}\, {\rm d}h, \]

in two disjoint sets

\[ {\mathbb{G}\setminus B(g_1,r)}=\left( \mathbb{G}\setminus B(g_0,R)\right)\cup \left( B(g_0,R)\setminus B(g_1,\rho)\right). \]

The integral over the second of the above sets is estimated by using $h\notin B(g_1,\,\rho )$, followed by Hölder's inequality, to obtain

(3.14)\begin{align} & \rho^{2s}\int_{B(g_0,R)\setminus B(g_1,\rho)} \frac{u(h)}{|h^{{-}1}\circ g_1|^{Q+2s}}\, {\rm d}h\notag\\ & \quad \leq \frac{\rho^{2s}}{\rho^{Q+2s}}\int_{B(g_0,R)\setminus B(g_1,\rho)}u\, {\rm d}h\\ & \quad \leq \omega_Q\left(\frac{R}{\rho}\right)^Q{\unicode{x2A0F}}_{B(g_0,R)}u\, {\rm d}h\leq \omega_Q\left(\frac{R}{\rho}\right)^Q\left({\unicode{x2A0F}}_{B(g_0,R)}u^2\, {\rm d}h\right)^{1/2}\nonumber\\ & \quad = \frac{4^Q\omega_Q}{\left(1-\tau\right)^Q}\left({\unicode{x2A0F}}_{B(g_0,R)}u^2\, {\rm d}h\right)^{1/2}, \nonumber \end{align}

where $\omega _Q$ is the volume of the unit gauge ball.

In order to estimate the integral in the tail over $\mathbb {G}\setminus B(g_0,\,R)$, we use the triangle inequality, $h\notin B(g_1,\,\rho )$ and $g_1\in B(g_0,\,\tau R)$, which give

\[ \frac{|h^{{-}1}\cdot g_0|}{|h^{{-}1}\cdot g_1|}\leq \frac{|h^{{-}1}\circ g_1|+|g_1^{{-}1}\cdot g_0|}{|h^{{-}1}\cdot g_1|}\leq 1+\frac{\tau R}{\rho}=1+\frac{4\tau}{1-\tau}=\frac{1+3\tau}{1-\tau}<\frac{4}{1-\tau}=\frac{R}{r}. \]

Hence, we have

(3.15)\begin{align} \rho^{2s}\int_{\mathbb{G}\setminus B(g_0,R)} \frac{u(h)}{|h^{{-}1}\cdot g_1|^{Q+2s}}\, {\rm d}h & \leq \rho^{2s}\left(\frac{R}{\rho} \right)^{Q+2s}\int_{\mathbb{G}\setminus B(g_0,R)} \frac{u(h)}{|h^{{-}1}\cdot g_0|^{Q+2s}}\, {\rm d}h\\ & = \left(\frac{R}{r} \right)^{Q} T(u;g_0,R)=\frac{4^Q}{\left(1-\tau \right)^{Q} } T(u;g_0,R). \nonumber \end{align}

Inequalities (3.13)–(3.15) give

(3.16)\begin{align} M_{\tau R} & \leq \frac{C_02^{Q/2}}{(1-\tau)^{Q/2}} \left( {\unicode{x2A0F}}_{B(g_0,R)} u^{2}\, {\rm d}{h}\right)^{1/2}+ \frac{{C_0}4^Q}{\left(1-\tau \right)^{Q} } T(u;g_0,R)\notag\\ & \quad + \frac{{C_0}4^Q\omega_Q}{\left(1-\tau\right)^Q}\left({\unicode{x2A0F}}_{B(g_0,R)}u^2\, {\rm d}h\right)^{1/2}\\ & \leq \frac{(C_0+\omega_Q)4^Q}{(1-\tau)^{Q}}\left( {\unicode{x2A0F}}_{B(g_0,R)} u^{2}\, {\rm d}{h}\right)^{1/2} + \frac{C_04^Q}{\left(1-\tau \right)^{Q} } T(u;g_0,R) \nonumber \end{align}

since $0<1-\tau <1$. The proof of (3.11) is complete.

Let us note that for $r$ and $R$ as in (3.11) satisfying, in addition, $R_0/2\leq r< R\leq R_0$ we have ${R}/{(R-r)}\leq 2$ and

\[ T(u;g_0,R)\leq 2^{2s}\left(\frac{R}{R_0} \right)^{2s}T(u;g_0,\frac{R_0}{2})\leq 2^{2s}T\left(u;g_0,\frac{R_0}{2}\right). \]

Therefore, inequality (3.11) implies that for all $g_0\in \mathbb {G}$ with $|g_0|=2R_0\geq 4\bar R_0$ and $R_0/2\leq r< R\leq R_0$ we have

(3.17)\begin{align} M_r& \leq 2^{Q+2s}C_1 \left[\left({\unicode{x2A0F}}_{B(g_0,R)} u^{2} \, {\rm d}{h} \right)^{1/2} + T\left(u; g_0,\frac{R_0}{2}\right)\right]\\ & \leq M_R^{1/2}\, 2^{Q+2s}C_1 \left(\frac{1}{\omega_Q R^Q}\int_{B(g_0,R_0)} u \, {\rm d}{h} \right)^{1/2} + 2^{Q+2s}C_1 T\left(u; g_0,\frac{R_0}{2}\right). \nonumber \end{align}

Inequality (3.17) implies, using $ab\leq \frac 12(a^2+b^2)$ and $R-r< R$, the inequality

(3.18)\begin{equation} M_r\leq \frac 12 M_R + \frac{A}{(R-r)^{Q}}+B, \end{equation}

where

\[ A=4^{Q+2s}C_1^2 \frac{1}{\omega_Q }\int_{B(g_0,R_0)} u\, {\rm d}{h} \quad\text{and}\qquad B= 2^{Q+2s}C_1 T\left(u; g_0,\frac{R_0}{2}\right). \]

Therefore, by a standard iteration argument, see for example [22, p. 191, Lemma 6.1], there exists a constant $c_Q$ so that $M_r\leq c_Q[ {A}{(R-r)^{-Q}}+B]$. Hence, for any $R_0\ge 2\bar R_0$, and $g_0\in \mathbb {G}$ with $|g_0|=2R_0$, we have

\[ \sup_{B(g_0,R_0/2)} \leq C \left[ {\unicode{x2A0F}}_{B(g_0,R_0)} u\, {\rm d}{h} + T(u; g_0,R_0/2)\right]. \]

This completes the proof of theorem 1.1.

4. Proof of theorem 1.2

Recall that here we are considering a nonnegative subsolution $u$ to the Yamabe type equation $\mathcal {L}_s u=u^{2^*(s)-1}.$

4.1. The optimal Lorentz space regularity

The first step is to obtain the optimal Lorentz space regularity of $u$. For this we can adapt to the current setting [3, Propositions 3.2 and 3.3], which gives

(4.1)\begin{equation} u\in L^{r,\infty}(\mathbb{G})\cap L^\infty(\mathbb{G}), \end{equation}

recalling that $r= {2^*(s)}/{2}$, cf. (1.19). Notice that in the cited results from [3], valid in the Euclidean setting, the authors do not assume that the solution is radial, but the radial symmetry is used ultimately to obtain the rate of decay of the solution of the fractional Yamabe equation.

For the sake of completeness and self-containment of the proof, in the setting of a homogeneous group, and right-hand side of the equation modelled on the fractional Yamabe equation, we include a proof of the sharp Lebesgue space regularity (4.1), relying on proposition 2.1. First, proposition 2.1 implies that $u\in L^{q}(\mathbb {G})\cap L^\infty (\mathbb {G})$, for any $q>r=2^*(s)/2$. Indeed, if $V=u^{2^*(s)-2}$ then since $u\in L^{2^*(s)}(\mathbb {G})$ it follows that $V\in L^{r'}(\mathbb {G})$. Hence, by proposition 2.1(b) it follows $u\in L^{q}(\mathbb {G})$ for all $q$ such that $\frac {2^*(s)}{2}< q<\infty$. Hence, part (c) gives that we also have $u\in L^{\infty }(\mathbb {G})$. Finally, we can see that $u \in L^{2^*(s)/2,\infty }(\mathbb {G})$ as follows. Take $F_t(u)=\min \{u,\,t\}$. Using the equation and the fractional Sobolev inequality we have

(4.2)\begin{equation} \lVert F_t\circ u\rVert_{L^{2^*(s)}(\mathbb{G})}^2\leq C\int_{\mathbb{G}}VuF_t(u){\rm d}g, \end{equation}

where $V=u^{2^*(s)-2}$. Using first that $F_t(u)\leq t$ and then the definition of $V$ we have

(4.3)\begin{equation} \int_{\mathbb{G}}VuF_t(u){\rm d}g\leq t\int_{\mathbb{G}}Vu {\rm d}g \leq t\int_{\mathbb{G}}u^{2^*(s)-1}{\rm d}g<\infty \end{equation}

since $u^{2^*(s)-1}\in L^1(\mathbb {G})$ noting that $2^*(s)-1>2^*(s)/2$. Let $\mu (t)$ be the distribution function of $u$. From the definition of $F_t$ we have trivially:

(4.4)\begin{equation} \int_{\mathbb{G}}(F_t( u))^{2^*(s)}{\rm d}g= t^{2^*(s)}\mu(t)+ \int_{\{u< t\}} u^{2^*(s)} {\rm d}g \geq t^{2^*(s)}\mu(t). \end{equation}

Therefore, bounding from above the left-hand side of the above inequality using (4.2) and then using (4.3) we have

\[ t^{2^*(s)}\mu(t)\leq \left(C\int_{\mathbb{G}}VuF_t(u){\rm d}g \right)^{2^*(s)/2}\leq C t^{2^*(s)/2}, \]

which shows that $u \in L^{2^*(s)/2,\infty }(\mathbb {G})$.

4.2. Asymptotic behaviour of the tail term

We shall reduce the problem to a question of $L^p$ regularity of certain truncated powers of the homogeneous norm, which we define next. For $R>0$ and $\alpha >0$, let:

\[ \rho(g)=\rho_{\alpha,R}(g)=\left\{ \begin{array}{@{}ll} |g|^{-\alpha}, & |g|\ge R\\ 0, & |g|< R. \end{array} \right. \]

Lemma 4.1 For $Q/p<\alpha$ the Lorentz norms of $\rho _{\alpha,R}$ are given by the following formulas:

(4.5)\begin{equation} \lVert \rho_{\alpha,R}(g)\rVert_{L^{p,\sigma}} = \left[ \int_0^\infty \left( t^{1/p}\rho^*(t)\right)^\sigma \frac{{\rm d}t}{t}\right] ^{1/\sigma} = \ \frac{C_{Q,\sigma}}{R^{\alpha-\frac Q{p}}}. \end{equation}

Proof. Let $\mu (s)=\left \vert \left \{ g\mid \rho _{\alpha,R}(g)>s \right \}\right \vert$ be the distribution function of $\rho _{\alpha,R}$. From the representation of the Haar measure in polar coordinates (2.2), we have

\[ \mu(s) =\left\{ \begin{array}{@{}ll} 0, & s>\rho(R)\\ \displaystyle\dfrac{\sigma_Q}{Q} \left( s^{{-}Q/\alpha} - R^Q \right), & 0< s \le \rho(R). \end{array} \right. \]

The corresponding radially decreasing rearrangement is

\[ \rho^*(t)=\inf \left\{ s\ge 0 \mid \mu(s)\le t\right\} = \left( \frac{Q}{\sigma_Q}t +R^Q\right)^{-\alpha/Q} \]

since $s=\rho ^*(t)$ is determined from $\sigma _Q/Q (s^{-Q/\alpha }-R^Q )=t>0$. A small calculation shows then that for some constant $C_{Q,\sigma }$ we have (4.5).

Next, we use the optimal Lorentz space estimate and lemma 4.1 to bound the tail.

Lemma 4.2 With the standing assumption, i.e. $u\in \mathcal {D}^{s,2}(\mathbb {G})$ is a nonnegative subsolution to the Yamabe type equation (1.24), we have that the tail has the following decay:

\[ T(u;g_0,R)\equiv R^{2s}\int_{\mathbb{G}\setminus B(g_0,R)} \frac{u(g)}{|g^{{-}1}\cdot g_0|^{Q+2s}}{{\rm d}g}\ \leq C R^{-({Q-2s})} \]

with $C$ a constant depending on the homogeneous dimension $Q$.

Proof. By Hölder's inequality we have

(4.6)\begin{equation} T(u;g_0,R)\equiv R^{2s}\int_{\mathbb{G}\setminus B(g_0,R)} \frac{u(g)}{|g^{{-}1}\cdot g_0|^{Q+2s}}{{\rm d}g}\le R^{2s}\lVert u\rVert_{L^{r,\infty}} \lVert \rho_{Q+2s,R}\rVert_{L^{r',1}}, \end{equation}

recalling the definition of $r$ in (1.19) and using the weak $L^{r,\infty }$ regularity of $u$ that we already proved. Hence, the claim of the lemma follows by lemma 4.1 which shows that for some constant $C=C(Q)$ we have

(4.7)\begin{equation} \lVert \rho_{Q+2s,R}\rVert_{L^{r',1}}\leq CR^{{-}Q}. \end{equation}

As a consequence, taking into account that $r'=Q/(2s)$ we obtain (4.7).

4.3. The slow decay

The proof of theorem 1.2 will also use a preliminary ‘slow’ decay of the solution $u$, see [44, Lemma 2.1] for case of the Yamabe equation on a Riemannian manifold with maximal volume growth.

Lemma 4.3 If $u\in \mathcal {D}^{s,2}(\mathbb {G})$ is a nonnegative subsolution to the Yamabe type equation, then $u$ has the slow decay $|g|^{({Q-2s})/2}u\in L^\infty (\mathbb {G})$.

Proof. The key to this decay is the scale invariance of the equation, i.e. the fact that

\[ u_\lambda(g)=\lambda^{({Q-2s})/2}u(\delta_\lambda g) \]

is also a subsolution to the Yamabe type equation and the scale invariance of the $\mathcal {D}^{s,2}(\mathbb {G})$ and the $L^{2^*(s)}(\mathbb {G})$ norms. In order to show the slow decay, it is then enough to show that there exist constants $\lambda _o$ and $C$, depending only on $Q$ and $s$, and the invariant under the scaling norms, such that for all $g_0$ with $\lambda =|g_0|/2>\lambda _0$ we have on the ball $B(h_0,\,1)$ with $h_0= \delta _{\lambda ^{-1}}g_0$, the estimate:

(4.8)\begin{equation} \max_{h\in B(h_o,1)}u_\lambda(h) \leq C. \end{equation}

Indeed, (4.8) implies:

\[ \left(\frac{|g_o|}{2}\right)^{(Q-2s)/2}u(g_o)\leq \left(\frac{|g_o|}{2}\right)^{(Q-2s)/2}\sup_{B(g_0,\lambda) } u( g)=\max_{B(h_0,1)}u_{\lambda}(h)\leq C, \]

which gives the desired decay. Bound (4.8) will be seen from the local version of proposition 2.1(c) in the case $V=u_\lambda ^{2^*(s)-2}$ by showing that the local supremum bound is independent of $\lambda$. To simplify the notation let $v=u_\lambda$. We follow the argument in the proof of theorem 1.1 with $V=v^{2^*(s)-2}$. Furthermore, for $\frac 12< r< R<\frac 32$ we take a bump function $\psi$, so that,

\[ \psi\vert_{B_r}\equiv 1,\qquad \mathrm{supp}\, \psi\Subset B_{\frac{r+R}{2}}, \]

where here and for the remainder of the proof, for any $r>0$ we will denote by $B_r$ the ball $B(h_0,\,r)$ with the understanding that the centre is $h_0$.

In particular, we have (3.7) with $u_\delta$ replaced by $v_\delta$, but now we can absorb the first term on the right-hand side in the left-hand side for all sufficiently large $\lambda$. Indeed, applying Hölder's inequality we have

(4.9)\begin{equation} \int\psi^2Vv_\delta^{\beta+1}\, {\rm d}{h}\leq \left[\int_{B_{\frac{r+R}{2}}}V^{r'}\, {\rm d}{h}\right]^{1/r'} \left[ \int_{B_{\frac{R+r}{2}}}\psi^{2^*(s)}v_\delta^{(\beta+1)2^*(s)/2 }\, {\rm d}{h} \right]^{2/2^*(s)}.\end{equation}

Since $V=v^{2^*(s)-2}$ the first term can be estimated as follows:

\[ \int_{B_{\frac{r+R}{2}}}V^{r'}{\rm d}h=\int_{B_{\frac{r+R}{2}}}v^{2^*(s)}{\rm d}h\leq \int_{B(g_0,\lambda)}u^{2^*(s)}\, {\rm d}{h}\rightarrow 0\quad\text{as}\quad \lambda\rightarrow \infty, \]

using the scaling property of the $L^{2^*(s)}$ norm and $u\in L^{2^*(s)}(\mathbb {G})$. Therefore, we have the analogue of (3.8), i.e. for all $\lambda \ge \lambda _0$ there exists a constant $C= C(Q,\,s,\, K_0)$, such that, the following inequality holds true:

(4.10)\begin{align} \left[ \int_{B_{r}}v_\delta^{(\beta+1)\frac{2^*(s)}{2} } \, {\rm d}{h}\right]^{\frac{2}{2^*(s)}} \le \frac{C\beta^{\kappa}}{R^{2s}}\left[\left(\frac{R}{R-r} \right)^2\right]\left[1 +\frac{T(v;h_0,R)}{\delta } \right] \int_{B_R}v_\delta^{\beta+1}\, {\rm d}{h}. \end{align}

A Moser type iteration argument shows then the existence of a constant $C$ such that for all $\lambda \ge R_0$, $h_0= \delta _{\lambda ^{-1}}g_0$ and $|g|=2\lambda$ we have the inequality

(4.11)\begin{align} \sup_{B(h_0,1)} v & \leq\ C \left[ \left ({\unicode{x2A0F}}_{B(h_0,2)} v^{2^*(s)} \, {\rm d}{h} \right)^{1/{2^*(s)}} + T(v; h_0,1/2)\right]\\ & \leq C \left[ \left (\int_{\mathbb{G}} v^{2^*(s)} \, {\rm d}{h}\right)^{1/{2^*(s)}} + T(v; h_0,1/2)\right]\nonumber\\ & \leq C\left[\lVert u\rVert_{\mathcal{D}^{s,2}(\mathbb{G})}+\lVert u\rVert_{L^{2^*(s)}(\mathbb{G})} \lVert \rho_{Q+2s,1}\rVert_{L^{2^*(s)',1}}\right]\le C, \nonumber \end{align}

after using the fractional Sobolev inequality, Hölder's inequality, (4.5), and the invariance under scalings of the $\mathcal {D}^{s,2}(\mathbb {G})$ and $L^{2^*(s)}(\mathbb {G})$ norms.

4.4. Conclusion of the proof of theorem 1.2

We begin by noting that, from what we have already proved, theorem 1.1 can be applied to the potential $V=u^{2^*(s)-2}$. Indeed, the slow decay of $u$, cf. lemma 4.3, gives that for some constant $C$ we have

\[ u(g)\leq C|g|^{-({Q-2s})/2}, \]

which together with (2.2) implies the needed assumptions on $V$, in particular, for $t_0>r'= {Q}/{(2s)}$, cf. (1.19), we have

\begin{align*} \int_{|g|\geq R}V^{t_0}\, {\rm d}{h} & =\int_{|g|\geq R} u^{t_0(2^*(s)-2)}\, {\rm d}{h}\\ & \leq C^{\frac{4st_0}{Q-2s}}\int_{|g|\geq R} |g|^{{-}2st_0}\, {\rm d}{h} = \frac{\sigma_Q C^{\frac{4st_0}{Q-2s}}}{2st_0-Q} \frac{1}{R^{2st_0-Q }}. \end{align*}

Therefore, theorem 1.1 gives that for all $g\in \mathbb {G}$ and $2R=|g|$ sufficiently large we have (1.22), i.e. there exists a constant $C$ independent of $g$, such that

(4.12)\begin{equation} \sup_{B(g,R/2)} u\ \leq\ C {\unicode{x2A0F}}_{B(g,R)} u + {C}T(u; g,R/2). \end{equation}

Furthermore, the weak $L^{2^*(s)/2}$ regularity (4.1) shows that for $r=2^*(s)/2$ we have the inequality

(4.13)\begin{equation} {\unicode{x2A0F}}_{B(g,R)} u \, {\rm d}{h}\leq \frac{r}{r-1}\frac{1}{|B_R|^{1/{r}}}\lVert u\rVert_{L^{2^*(s)/2,\infty}}=\frac{C}{R^{Q-2s}}\lVert u\rVert_{L^{r,\infty}}, \end{equation}

taking into account that for $1\leq p<\infty$ the $L^{p,1}(\mathbb {G})$ norm of the characteristic function of the gauge ball $B_R$ is $p|B_R|^{1/p}$.

Now we are ready to conclude the proof of theorem 1.2 since by (4.12), (4.13), and lemma 4.2 we can claim the following estimate for all sufficiently large $2R=|g|$,

\[ u(g)\leq \max_{B(g,R/2)} u\ \leq\ \frac{C}{R^{Q-2s}}\lVert u\rVert_{L^{2^*(s)/2,\infty}} + \frac{C}{R^{Q-2s}} \]

with a constant $C$ independent of $g$.