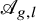Angehrn-Siu-Helmke’s method applied to abelian varieties

Abstract

We apply Angehrn-Siu-Helmke’s method to estimate basepoint freeness thresholds of higher dimensional polarized abelian varieties. We showed that a conjecture of Caucci holds for very general polarized abelian varieties in the moduli spaces $\mathcal {A}_{g, l}$ with only finitely many possible exceptions of primitive polarization types l in each dimension g. We improved the bound of basepoint freeness thresholds of any polarized abelian $4$ -folds and simple abelian $5$ -folds.

1 Introduction

We work over the field of complex numbers throughout this paper.

Syzygies of abelian varieties have attracted lots of attention in recent years. Recall the following question asked by Ito for $p\geq 0$ and by Lozovanu for $p=-1$ as well (see [Ito1] and [Loz]).

Conjecture 1.1. Let $(A, L)$ be a polarized abelian variety of dimension g and $p\geq -1$ an integer. If $(L^g)>(g(p+2)))^g$ and $(L^d\cdot B)>(d(p+2))^d$ for any abelian subvariety B of A of dimension $0<d<g$ , then L satisfies Property $(N_p)$ .

We summarize recent progress towards this conjecture.

1.1 $\mathbb Q$ -twisted sheaves

Given a coherent sheaf $\mathcal {F}$ on A and a rational number $t\in \mathbb Q$ , following [JP], we formally define the $\mathbb Q$ -twisted sheaves $\mathcal {F}\langle tL \rangle $ . We say that $\mathcal {F}\langle tL\rangle $ is IT $^0$ if the i-th cohomological support loci $V^i(\mu _b^*\mathcal {F}\otimes L^{\otimes b^2t})$ is empty for each $i>0$ , where b is an integer such that $b^2t\in \mathbb Z$ . Note that this definition does not depend on the choice of b. Assume that D is an effective $\mathbb Q$ -divisor on A such that D is $\mathbb Q$ -equivalent to $tL$ . We will also write $\mathcal {F}\langle D\rangle =\mathcal {F}\langle tL \rangle $ .

Similarly, we say that $\mathcal {F}\langle tL \rangle $ is M-regular (resp. GV) if

$$ \begin{align*}\operatorname{\mathrm{codim}}_{\operatorname{\mathrm{Pic}}^0(A)}V^i(\mu_b^*\mathcal{F}\otimes L^{\otimes (b^2t)})>i\end{align*} $$

(resp. $\operatorname {\mathrm {codim}}_{\operatorname {\mathrm {Pic}}^0(A)}V^i(\mu _b^*\mathcal {F}\otimes L^{\otimes (b^2t)})\geq i$ ) for all $i>0$ . We can similarly define the cohomology ranks of $\mathcal {F}\langle tL\rangle $ :

$$ \begin{align*} h^i(A, \mathcal{F}\langle tL\rangle):=\frac{1}{b^{2\dim A}}h^i(A, \mu_b^*\mathcal{F}\otimes L^{\otimes b^2t}\otimes Q), \end{align*} $$

where $Q\in \operatorname {\mathrm {Pic}}^0(A)$ is general. The main result of [JP] says that the function $t\rightarrow h^i(A, \mathcal {F}\langle tL\rangle )$ is locally polynomial on a left or right neighborhood of a rational number and can be extended to a continuous function from $\mathbb R$ to $\mathbb R$ . We call this function the i-th cohomological rank function of $\mathcal {F}$ and denote it by $h^i_{\mathcal {F}, L}(t)$ .

Let $\mathcal {I}_o$ be the ideal sheaf of the neutral element o of A. The basepoint freeness threshold $\beta (L)$ is defined to be

$$ \begin{align*} \beta(L):=\inf\{t\in \mathbb Q\mid \mathcal{I}_o\;\langle tL\rangle \;\text{is}\; \mathrm{IT}^0\}. \end{align*} $$

By the main theorem of [Hac], $\beta (L)$ is also equal to

$$ \begin{align*} \inf\{t\in \mathbb Q\mid \mathcal{I}_o\;\langle tL\rangle \;\text{is}\; \mathrm{GV}\}. \end{align*} $$

It was observed in [JP] that $\beta (L)\leq 1$ and equality holds iff $|L|$ has a basepoint. This shows that $\beta (L)$ may vary in families. More generally, if for some rational number $t=\frac {a}{b}$ , $V^i(\mu _b^*\mathcal {I}_o\otimes L^{\otimes (ab)})$ is a nonempty proper subset of $\operatorname {\mathrm {Pic}}^0(A)$ , $\beta (L)=t$ . However, there is, by far, no general way to determine $\beta (L)$ . It is also not clear whether or not $\beta (L)$ is always a rational number.

By [JP, Section 8] and [C, Theorem 1.1], knowing the exact number of the basepoint freeness threshold $\beta (L)$ helps to understand the syzygies of L.

Theorem 1.2. For $p\geq -1$ , if $\beta (L)<\frac {1}{p+2}$ , L satisfies Property $(N_p)$ .

Remark 1.3. Ito refined this criteria in [Ito4, Theorem 1.5] by showing that if $\mathcal {I}_o\langle \frac {1}{p+2}L\rangle $ is M-regular for some integer $p> 0$ , L satisfies Property $(N_p)$ .

Caucci then asked the following question, which would imply Conjecture 1.1.

Conjecture 1.4. Let $(A, L)$ be a polarized abelian variety of dimension g and $p\geq -1$ an integer. If $(L^g)>(g(p+2)))^g$ and $(L^d\cdot B)>(d(p+2))^d$ for any abelian subvariety B of A of dimension $0<d<g$ , then $\beta (L)<\frac {1}{p+2}$ .

1.2 Known results

By Theorem 1.2, in order to solve Conjecture 1.1 and Conjecture 1.4, it suffices to prove that

(1) $$ \begin{align} &\beta(L)\leq n(L):=\\ \nonumber \mathrm{inf}\{\frac{d}{\sqrt[d]{(L^d\cdot B)}}\mid\qquad & B \text{ is an abelian subvariety of dimension}\; 1\leq d \leq g\}. \end{align} $$

There are various ways to estimate $\beta (L)$ . In [Ito2], Ito showed that the Angehrn-Siu method (see [AS, Hel, K]), which was initially applied to attack Fujita’s basepoint-freeness conjecture, can also be used to estimate $\beta (L)$ . To be more precise, let

$$ \begin{align*} &\quad r'(L):=\mathrm{inf}\{t\in \mathbb Q\mid \text{ there exists a } \mathbb{Q}\text{-divisor}\; D\sim_{\mathbb Q}tL \\ &\text{such that }\; o\; \text{is an isolated component of}\; \mathrm{Nklt}(A, D)\}, \end{align*} $$

where the non-klt locus $\mathrm {Nklt}(A, D)$ is the subscheme of A defined by the multiplier ideal $\mathcal {J}(A, D)$ (see, for instance, [Laz2, Section 9 and 10]). Ito proved in [Ito2] that we always have $\beta (L)\leq r'(L)$ , $ r'(L)\leq n(L)$ when $(A, L)$ is a polarized simple abelian 3-fold, and Conjecture 1.1 and Conjecture 1.4 hold for any polarized abelian 3-fold.

In higher dimensions, we have proved in [Jz] via generic vanishing that $\beta (L)\leq 2n(L).$ This implies that given a polarized abelian variety $(A, L)$ , if $(L^d\cdot B)>(2(p+2)d)^d$ for any abelian subvariety B of dimension $1\leq d\leq g$ , L satisfies Property $(N_p)$ .

Based on Bridgeland’s stability condition on surfaces, Lahoz-Rojas [LR] and Rojas [R] almost determined the cohomological rank functions $h^i_{\mathcal {I}_o, L}$ for any polarized abelian surface $(A, L)$ of Picard number $1$ . When L is of polarization type $(1, d)$ , Rojas proved that $\beta (L)=\frac {1}{\sqrt {d}}$ when d is a perfect square or $\beta (L)=\frac {2y}{x-1}$ where $(x, y)$ is the minimal or the second minimal positive solution of the Pell’s equation $X^2-4dY^2=1$ when d is not a perfect square. In either case, we have $\beta (L)< \frac {\sqrt {d}+1}{d}<\frac {\sqrt {2}}{\sqrt {d}}=n(L)$ when $d\geq 6$ .

Via a degeneration method, Ito studied in [Ito3] the syzygies of general polarized abelian varieties of type $(1,\ldots ,1, d)$ . He proved that when $d\geq \frac {(p+2)^{g+1}-1}{p+1}$ , L satisfies Property $(N_p)$ .

Inspired by the results of Ito and Rojas, one may believe that $\beta (L)$ should be quite close to $\displaystyle\frac {1}{\sqrt[g]{h^0(A, L)}}=\frac {\sqrt[g]{g!}}{\sqrt[g]{(L^g)}}$ for general polarized abelian variety $(A, L)$ of dimension g.

1.3 Main results

In this paper, we follow Ito’s approach to apply the Angehrn-Siu method to estimate $\beta (L)$ for polarized abelian varieties. We improve the known upper bounds for $\beta (L)$ for higher dimensional polarized abelian varieties.

We denote, respectively, by g and l the dimension and the polarization type of a polarized abelian variety $(A, L)$ . There is a quasi-projective variety $\mathcal {A}_{g, l}$ parametrizing such polarized abelian varieties. We may assume that l is primitive.

Theorem 1.5. Let $(A, L)$ be a very general polarized abelian variety in $\mathcal {A}_{g, l}$ . When $g=4$ or $5$ or $6$ , we have $\beta (L)\leq n(L)$ . When $g\geq 7$ , we also have $\beta (L)\leq n(L)$ , except for possibly finitely many primitive polarization types l in each dimension g.

Theorem 1.6. Let $(A, L)$ be a polarized abelian $4$ -fold. Assume that $(L^4)>((2+\frac {4}{\sqrt {3}})(p+2))^4$ and $(L^d\cdot B)>((p+2)d)^d$ for any abelian subvariety B of dimension $1\leq d\leq 3$ . Then, $\beta (L)<\frac {1}{p+2}$ .

Remark 1.7. Note that $2+\frac {4}{\sqrt {3}}\approx 4.31$ . Thus, Theorem 1.6 is quite close to Conjecture 1.4 for abelian 4-folds.

Theorem 1.8. Let $(A, L)$ be a polarized abelian $5$ -fold. Assume that A is simple and $(L^5)>(8(p+2))^5$ . Then, $\beta (L)< \frac {1}{p+2}$ .

In higher dimensions, we also have slight improvements of $\beta (L)$ . For $g\geq 6$ , we define $\alpha _{g, g-2}=\sqrt [g-2]{\frac {15(g-3)!}{g-1}}$ , $\alpha _{g, 2}=\sqrt {\frac {5(g-2)+1}{g-1}}$ . For $3\leq d\leq g-3$ ,

$$ \begin{align*}\alpha_{g,d}=\sqrt[d]{\frac{\frac{5}{2}d!(g-d)}{\binom{g-1}{g-d}}},\end{align*} $$

and let

$$ \begin{align*}\alpha_g:=\mathrm{min}\{\alpha_{g,d}\mid 2\leq d\leq g-2\}.\end{align*} $$

Theorem 1.9. Let $(A, L)$ be a polarized abelian variety of dimension $g\geq 6$ . Assume that A is simple and $(L^g)>((2g-\alpha _g)(p+2))^g$ , $\beta (L)< \frac {1}{p+2}$ .

Note that $\alpha _6=\alpha _{6,3}=\sqrt [3]{\frac {9}{2}}$ , $\alpha _7=\alpha _{7, 3}=\sqrt [3]{4}$ , $\alpha _8=\alpha _{8, 3}=\sqrt [3]{\frac {25}{7}}$ .

2 Preliminaries

2.1 Log canonical centers

Let X be a smooth projective variety and $\Delta $ be an effective $\mathbb Q$ -divisor on X. We take $\mu : Y\rightarrow X$ a log resolution of $(X, \Delta )$ , and we write

$$ \begin{align*}K_Y=\mu^*(K_X+\Delta)+\sum_Ea(E, X, \Delta)E, \end{align*} $$

where E runs through prime divisors of Y and $\mu _*(\sum _Ea(E, X, \Delta )E)=-\Delta $ .

We say that $(X, \Delta )$ is log canonical at x if $a(E, X, \Delta )\geq -1$ for all prime divisors E on Y such that $x\in \mu (E)$ . For a prime divisor E on Y, if $(X, \Delta )$ is log canonical at the generic point of $\mu (E)$ and the discrepancy $a(E, X, \Delta )=-1$ , we call $\mu (E)$ a log canonical center of $(X, \Delta )$ .

When $(X, \Delta )$ is log canonical at x, there are finitely many log canonical centers containing x, and the intersection of two such log canonical centers is the union of certain log canonical centers containing x (see, for instance, [K, Proposition 1.5] or [F, Theorem 9.1]). Thus, there exists a unique minimal log canonical center Z of $(X, \Delta )$ through x. Moreover, Z is normal and has rational singularities around x. More precisely, locally around x, there exists a $\mathbb Q$ -effective divisor $\Delta _Z$ such that $(Z, \Delta _Z)$ is klt (see, for instance, [FG, Theorem 7.1]). However, the singularities of Z away from x cannot be controlled in general. The following result due to Xiaodong Jiang from [Jx, Proposition 5.1] will be applied later.

Proposition 2.1. Let X be a smooth projective variety and $\Delta $ an effective $\mathbb Q$ -divisor on X with Z a log canonical center of $(X, \Delta )$ ¹ . Let $\nu : \overline {Z}\rightarrow Z$ be the normalization. Then, there exists an effective $\mathbb Q$ -divisor $\Delta _{\overline {Z}}$ on $\overline {Z}$ such that $\nu ^*(K_X+\Delta )\sim _{\mathbb Q} K_{\overline {Z}}+\Delta _{\overline {Z}}$ .

We then have the immediate corollary.

Corollary 2.2. Under the above assumption, we have

$$ \begin{align*}\operatorname{\mathrm{vol}}(Z, (K_X+\Delta)|_Z)=\operatorname{\mathrm{vol}}(\overline{Z}, K_{\overline{Z}}+\Delta_{\overline{Z}})\geq \operatorname{\mathrm{vol}}(Z', K_{Z'}), \end{align*} $$

where $Z'$ is any $\mathbb Q$ -Gorenstein partial resolution of $\overline {Z}$ .

Proof. Let $\rho : Z'\rightarrow \overline {Z}$ be a $\mathbb Q$ -Gorenstein partial resolution and let $\mu : \tilde {Z}\xrightarrow {\sigma }Z'\xrightarrow {\rho } \overline {Z}$ be a log resolution of $(\overline {Z}, \Delta _{\overline {Z}})$ . Let $\Delta _{\tilde {Z}}=\mu _*^{-1}(\Delta _{\overline {Z}})$ . We then have $K_{\tilde {Z}}+\Delta _{\tilde {Z}}+E_1=\mu ^*(K_{\overline {Z}}+\Delta _{\overline {Z}})+E_2$ and $K_{\tilde {Z}}+E_3=\sigma ^*K_{Z'}+E_4$ , where $E_i$ are $\mathbb Q$ -effective $\mu $ -exceptional divisors for $1\leq i\leq 4$ . Thus, $ \operatorname {\mathrm {vol}}(\overline {Z}, K_{\overline {Z}}+\Delta _{\overline {Z}})=\operatorname {\mathrm {vol}}(\tilde {Z}, \mu ^*(K_{\overline {Z}}+\Delta _{\overline {Z}})+E_2+E_3)=\operatorname {\mathrm {vol}}(\tilde {Z}, K_{\tilde {Z}}+\Delta _{\tilde {Z}}+E_1+E_3)\geq \operatorname {\mathrm {vol}}(Z', K_{Z'})$ .

The reason that we are interested to know the lower bound of the restricted volume is that we like to apply Helmke’s induction to cut down log canonical centers.

We briefly recall Helmke’s work [Hel]. Let D be an ample effective $\mathbb Q$ -divisor on a smooth projective variety X of dimension n. We assume that $x\in D$ and $\mathrm {mult}_xD=m>0$ . Let $c:=\mathrm {lct}(D, x)$ be the log canonical threshold of D at x; namely, c is the maximal rational number such that $(X, cD)$ is log canonical at x. Let Z be the minimal log canonical center of $(X, cD)$ through x and denote $d=\dim Z$ . Helmke and Ein independently introduced the local discrepancy ² $b_x(X, cD)$ of $(X, cD)$ at x, which is the rational number

$$ \begin{align*} \mathrm{max}\{\mathrm{mult}_x E\mid\qquad & E\;\text{ is an effective}\; \mathbb Q\text{-divisor}\; \\ & \text{such that}\; (X, cD+E) \;\text{is log canonical at}\; x\}. \end{align*} $$

It is known that $0\leq b_x(X, cD)\leq n-cm$ , $b_x(X, cD)\leq d$ and $b_x(X, cD)=0$ iff $Z=\{x\}$ (see [Hel] and [E]).

Helmke’s induction can be summarized as follows (see [Hel, Proposition 3.2 and Theorem 4.3]).

Proposition 2.3. Under the above assumption, assume that $c<1$ .

1. (1) If $(D^{\dim Z}\cdot Z)>(\frac {b_x(X, cD)}{1-c})^{\dim Z}\mathrm {mult}_xZ$ , there exists a rational number $0<c'<1-c$ , an effective $\mathbb Q$ -divisor $D'\sim _{\mathbb Q}c'D$ , such that $(X, cD+D')$ is log canonical at x and the minimal log canonical center $Z'$ of $(X, cD+D')$ through x is a proper subset of Z.

2. (2) If $m\geq n$ , let $c_1=c+c'$ and $D_1=cD+D'$ . Then, $\frac {b_x(X, D_1)}{1-c_1}<\frac {b_x(X, cD)}{1-c}\leq n$ .

3. (3) $\mathrm {mult}_xZ\leq \binom {n-\lceil b_x(X, cD) \rceil }{n-d}$ .

Remark 2.4. Let $(A, L)$ be a polarized abelian variety. Assume that an effective $\mathbb Q$ -divisor $D\sim _{\mathbb Q}tL$ such that $(X, D)$ is log canonical around the neutral element o, and Z is the minimal log canonical center through o. Choose an integer $m\geq 1$ such that $\mathcal {I}_Z\otimes L^{\otimes m}$ is globally generated. Then, by a standard argument (see, for instance, [K, Proposition 2.3]), we know that for a small perturbation $D'=(1-\epsilon )D+\eta H$ of D, where $0<\epsilon , \eta \ll 1$ and $H\in |\mathcal {I}_Z\otimes L^{\otimes m}|$ general, Z is an irreducible component of $\mathrm {Nklt}(A, D')$ . In particular, if $Z=\{o\}$ , we have $\beta (L)\leq r'(L)\leq t$ . When Z is an abelian subvariety of A and $\beta (L|_Z)\leq t$ , we also have $\beta (L)\leq t$ by Ito’s work [Ito2, Proposition 6.6].

2.2 A generic vanishing approach

We recall some results from [Jz].

Lemma 2.5. Let $(A, L)$ be a polarized abelian variety. Assume that $D\sim _{\mathbb Q} tL$ is an effective $\mathbb Q$ -divisor such that there exists an effective divisor $H\preceq D$ . Then, $\mathcal {I}_{K}\langle tL\rangle $ is GV, where K is the neutral component of the kernel of the morphism

$$ \begin{align*} &\varphi_H: A\rightarrow \operatorname{\mathrm{Pic}}^0(A)\\ & x\rightarrow t_x^*\mathcal{O}_A(H)\otimes \mathcal{O}_A(-H). \end{align*} $$

In particular, when H is ample, $\mathcal {I}_o\langle tL\rangle $ is GV and thus, $\beta (L)\leq t$ .

Proof. This follows directly from the proof of (1) of [Jz, Proposition 4.1].

The following theorem is essentially the main result of [Jz].

Theorem 2.6. Let $(A, L)$ be a polarized abelian variety. Assume that

• ○ there exists an irreducible normal subvariety Z of A such that $\mathcal {I}_Z\langle t_0L\rangle $ is IT $^0$ for some positive rational number $t_0$ and a smooth model of Z is of general type;

• ○ and there exists an effective $\mathbb Q$ -Weil divisor $D_Z$ on Z and a big $\mathbb Q$ -Cartier divisor $V_Z$ such that $K_Z+D_Z$ is $\mathbb Q$ -Cartier and $t_0L|_Z\sim _{\mathbb Q}2(K_Z+D_Z)+V_Z$ .

We have $\beta (L)\leq t_0$ .

Since [Jz, Theorem 1.4] is not stated in this way, let’s briefly recall the proof for the readers’ convenience.

Proof. It suffices to show that $\mathcal {I}_0\langle t_0L\rangle $ is GV. After a translation, we may assume that $o\in Z$ is a smooth point of Z. We have the short exact sequence

$$ \begin{align*}0\rightarrow \mathcal{I}_Z\rightarrow \mathcal{I}_o\rightarrow \mathcal{I}_{o, Z}\rightarrow 0.\end{align*} $$

By the first condition, it suffices to show that $\mathcal {I}_{o, Z}\langle t_0L\rangle $ is GV.

We may also assume that Z is smooth (one may check [Jz, Subsection 5.1] for the full argument). Then, since Z is of general type, $\omega _Z\otimes \mathcal {I}_{o, Z}$ is GV (see [Jz, Lemma 2.6]). Then, one can take an integer M sufficiently divisible such that $Mt_0\in \mathbb Z$ and consider the multiplication-by-M map $\pi _M: A\rightarrow A$ and denote by $Z^M$ the inverse image $\pi _M^{-1}(Z)$ . By the second condition, $\mathcal {O}_{Z^M}(M^2t_0L-K_{Z^M})$ has a nontrivial section s. Indeed, the second condition implies that $M^2t_0L|_{Z^M}-K_{Z^M}$ is $\mathbb Q$ -equivalent to the sum of $K_{Z^M}$ and a big divisor, and one can conclude by Nadel’s vanishing and generic vanishing that $M^2t_0L|_{Z^M}-K_{Z^M}$ has a global section. ³

Via this section, we have a short exact sequence

$$ \begin{align*} 0\rightarrow K_{Z^M}\xrightarrow{\cdot s} \mathcal{O}_{Z^M}(M^2t_0L)\rightarrow \mathcal{Q}\rightarrow 0. \end{align*} $$

We then check that all terms in this short exact sequence are GV. We may assume that the zero locus of s does not intersect with $o_M:=\pi _M^{-1}(o)$ . Then, we have another short exact sequence

$$ \begin{align*} 0\rightarrow \mathcal{I}_{o_M}\otimes K_{Z^M}\xrightarrow{\cdot s} \mathcal{I}_{o_M}\otimes\mathcal{O}_{Z^M}(M^2t_0L)\rightarrow \mathcal{Q}\rightarrow 0. \end{align*} $$

Note that $\mathcal {I}_{o_M}\otimes K_{Z^M}=\pi _M^{*}(\mathcal {I}_o\otimes K_Z)$ is GV. Hence, $\mathcal {I}_{o_M}\otimes \mathcal {O}_{Z^M}(M^2t_0L)$ is also GV. This implies that $\mathcal {I}_{o,Z}\langle t_0L\rangle $ is GV.

We shall apply Theorem 2.6 in the following way.

Proposition 2.7. Let $(A, L)$ be a polarized simple abelian variety. Assume that an effective $\mathbb Q$ -divisor $D\sim _{\mathbb Q}t_0L$ and $\mathrm {lct}(D, o)<\frac {1}{2}$ . Then, $\beta (L)\leq 2\mathrm {lct}(D, o)t_0<t_0$ .

Proof. We take $c=\mathrm {lct}(D)$ the global log canonical threshold of D (i.e., c is the maximal rational number such that $(A, cD)$ is a log canonical pair). Then, $c\leq \mathrm {lct}(D, o)<\frac {1}{2}$ . After a small perturbation of $cD$ and taking a translation, we may assume that the log canonical pair $(A, cD)$ has only one log canonical center Z, which is thus an irreducible normal subvariety of A and $o\in Z$ . Therefore, the multiplier ideal sheaf $\mathcal {J}(A, cD)=\mathcal {I}_Z$ , and by Nadel’s vanishing, we have $\mathcal {I}_Z\langle 2ct_0L\rangle $ is IT $^0$ . Since A is simple, a smooth model of Z is of general type.

However, by the main theorem of [FG], we know that there exists an effective $\mathbb Q$ -Weil divisor $D_Z$ on Z such that $K_Z+D_Z$ is $\mathbb Q$ -Cartier and $cD|_Z\sim _{\mathbb Q}K_Z+D_Z$ . Thus, for any rational number $0<\epsilon \ll 1$ , $(2ct_0+\epsilon )L|_Z\sim _{\mathbb Q} 2(K_Z+D_Z)+\epsilon L|_Z$ . Therefore, by Theorem 2.6, $ \beta (L)\leq 2ct_0+\epsilon $ for any rational number $0<\epsilon \ll 1$ . We then have $\beta (L)\leq 2\mathrm {lct}(D, o)t_0<t_0.$

2.3 Canonical volumes of subvarieties of abelian varieties

Barja, Pardini and Stoppino studied higher dimensional Severi type inequalities for varieties of maximal Albanese dimension in [BPS]. One of their main results is the following (see [BPS, Corollary 6.12]).

Theorem 2.8. Let $a: X\rightarrow A$ be a morphism from a smooth projective variety of general type of dimension $d\geq 2$ to an abelian variety A. Assume that $a: X\rightarrow a(X)$ is of degree $1$ . Then, $\operatorname {\mathrm {vol}}(K_X)\geq \frac {5}{2}d!\chi (\omega _X) .$

However, Pareschi and Popa generalized the Castelnuovo-De Franchis inequality in [PP]. The following result is a simple corollary of [PP, Theorem 3.3].

Theorem 2.9. Let $a: X\rightarrow A$ be a morphism from a smooth projective variety of general type of dimension $d\geq 2$ to an abelian variety A of dimension g. Assume that A is simple and a is generically finite from X onto its image. Then, $\chi (\omega _X)\geq g-d$ .

Proof. Since a is generically finite onto its image, we have

$$ \begin{align*}\chi(\omega_X)=\chi(a_*\omega_X),\end{align*} $$

and for each $i\geq 0$ ,

$$ \begin{align*}V^i(\omega_X, a):=\{P\in\operatorname{\mathrm{Pic}}^0(A)\mid H^i(X, \omega_X\otimes a^*P)\neq 0\}=V^i(a_*\omega_X)\end{align*} $$

by Grauert-Riemenschneider vanishing (see for instance [Laz1, Theorem 4.3.9]. In particular, each irreducible component of $V^i(a_*\omega _X)$ is a translation of an abelian subvariety of $\operatorname {\mathrm {Pic}}^0(A)$ by the main result of [GL].

However, by the main result of [Hac], $a_*\omega _X$ is GV. Thus, $V^i(a_*\omega _X)$ is a union of translations of proper abelian subvariety of $\operatorname {\mathrm {Pic}}^0(A)$ for each $i\geq 1$ . Since A is simple by assumption, so is $\operatorname {\mathrm {Pic}}^0(A)$ . Thus, $V^i(a_*\omega _X)$ consists of finitely many points for each $i\geq 1$ . In particular, the neutral element $\mathcal {O}_A$ is an isolated component of $V^i(a_*\omega _X)$ for each $i\geq 1$ . According to [PP, Definition 3.1], the generic vanishing index of $a_*\omega _X$ is $g-d$ . By [PP, Theorem 3.3], we have $\chi (\omega _X)=\chi (a_*\omega _X)\geq g-d $ .

Remark 2.10. In [LP], Lazarsfeld and Popa conjectured that under that assumption of Theorem 2.9, $\chi (\omega _X)> g-d$ when g is large compared to $\chi (\omega _X)$ . Coandă verified that $\chi (\omega _X)\geq 3$ when $g\geq 5$ and $g-d=2$ ([LP, Proposition 4.10])

Combining Theorem 2.8 and 2.9, we have an estimation of the canonical volume of irreducible subvarieties of simple abelian varieties.

Corollary 2.11. Let Z be an irreducible subvariety of dimension d of a simple abelian variety A of dimension g. Let $\rho : X\rightarrow Z$ be a desingularization. Then, $\operatorname {\mathrm {vol}}(K_X)\geq \frac {5}{2}d!(g-d)$ .

When X is a surface, we can slightly improve Theorem 2.8.

Proposition 2.12. Let $a: S\rightarrow A$ be a morphism from a smooth projective surface of general type to an abelian variety A of dimension $g\geq 3$ . Assume that $a: S\rightarrow a(S)$ is of degree $1$ . Then, $\operatorname {\mathrm {vol}}(K_S)\geq 5\chi (\omega _S)+1$ .

Proof. We follow the proof of [BPS, Theorem 5.5 and Corollary 5.6], where it was proved that $\operatorname {\mathrm {vol}}(K_S)\geq 5\chi (\omega _S)$ . It suffices to show that the equality can never hold.

We may assume that S is minimal and $a^*: \operatorname {\mathrm {Pic}}^0(A)\rightarrow \operatorname {\mathrm {Pic}}^0(S)$ is injective.

Fix an ample divisor H on A. Following [BPS], we consider the continuous rank functions: $F(t):=h^0_a(A, a_*\omega _S\otimes H^t)$ and $G(t):=h^0_a(A, a_*(\omega _S^{\otimes 2})\otimes H^{2t})$ . By definition, continuous rank functions are the $0$ -th cohomological rank functions. Thus, in our terminology, $F(t)=h^0_{a_*\omega _S, H}(t)$ and $G(t)=h^0_{a_*(\omega _S^{\otimes 2}), H}(2t)$ .

Following the proof in [BPS, Theorem 5.5], we know that $D^{-1}G(t)\geq 6 D^{-1}F(t)$ for $t\leq 0$ , where $D^{-1}G(t)$ and $D^{-1}F(t)$ are, respectively, the left derivative of the functions F and G at t. We also observe that $G(t)=F(t)=0$ when $t\ll 0$ . Hence, if $G(0)=6F(0)$ (i.e., $\operatorname {\mathrm {vol}}(K_S)= 5\chi (\omega _S)$ ), $G(t)=6F(t)$ for all $t\leq 0$ .

Since $a_*(\omega _S^{\otimes 2})$ is an IT $^0$ sheaf on A, for $-1\ll t<0$ , $a_*(\omega _S^{\otimes 2})\langle 2tH\rangle $ remains to be IT $^0$ (see [JP, Theorem 5.2]). Thus,

$$ \begin{align*}G(t)=\chi(S, 2K_S+2tH)=2(H^2)_St^2+3(K_S\cdot H)_St+K_S^2+\chi(\omega_S)\end{align*} $$

is a degree $2 $ polynomial function for $-1\ll t<0.$

However,

$$ \begin{align*} F(t)&=\chi(S, K_S+tH)+h^1_{a_*\omega_S, H}(t)-h^2_{a_*\omega_S, H}(t)\\ &=\frac{1}{2}(H^2)_St^2+\frac{1}{2}(K_S\cdot H)_St+\chi(\omega_S)+h^1_{a_*\omega_S, H}(t)\\ &\quad - h^2_{a_*\omega_S, H}(t). \end{align*} $$

We recall the formula in [JP, Proposition 2.3]:

$$ \begin{align*} h^i_{a_*\omega_S, H}(t)=\frac{(-t)^g}{\chi(H)}\chi({\varphi}_H^*R^i\Phi_{\mathcal{P}}(a_*\omega_S)\otimes H^{-\frac{1}{t}}), \end{align*} $$

for $-1\ll t<0.$

Since $a^*: \operatorname {\mathrm {Pic}}^0(A)\rightarrow \operatorname {\mathrm {Pic}}^0(S)$ is injective, we know that $R^2\Phi _{\mathcal {P}}(a_*\omega _S)$ is the skyscraper sheaf at $o_{\hat {A}}$ . Hence, $h^2_{a_*\omega _S, H}(t)=\chi (H)(-t)^g$ for $-1\ll t<0.$

Similarly, since S is of general type, $a_*\omega _S$ is a M-regular sheaf on A (see Subsection 2.3). Then, $\operatorname {\mathrm {codim}}_{\operatorname {\mathrm {Pic}}^0(A)}V^1(a_*\omega _S)\geq 2$ . We also know that the support of $R^1\Phi _{\mathcal {P}}(a_*\omega _S)$ is contained in $V^1(a_*\omega _S)$ . Consider the Chern characters of $R^1\Phi _{\mathcal {P}}(a_*\omega _S)$ . We have $\mathrm {ch}_i(\varphi _H^*R^1\Phi _{\mathcal {P}}(a_*\omega _S))=0\in H^{2i}(A, \mathbb Q)$ for $i=0, 1$ . If the codimension of the support of $R^1\Phi _{\mathcal {P}}(a_*\omega _S)$ is equal to $2$ , we may assume that $\mathcal {Z}_1,\ldots , \mathcal {Z}_s$ are the codimension- $2$ components of its support and $a_i>0$ is the rank of $R^1\Phi _{\mathcal {P}}(a_*\omega _S)$ at the generic point of $\mathcal {Z}_i$ . Then, $\mathrm {ch}_2(\varphi _H^*R^1\Phi _{\mathcal {P}}(a_*\omega _S))=\sum _ia_i[\varphi _H^{-1}\mathcal {Z}_i]$ . Hence,

$$ \begin{align*}h^1_{a_*\omega_S, H}(t)=\alpha_{2}t^2+\sum_{3\leq i\leq g}\alpha_it^i\end{align*} $$

for $-1\ll t<0,$ where $\alpha _2\geq 0$ .

We compare the coefficient of $t^2$ for $F(t)$ and $G(t)$ , which are, respectively, $\frac {1}{2}(H^2)_S+\alpha _2$ and $2(H^2)_S$ and conclude that $G(t)\neq 6F(t)$ .

The following corollary seems to be new, and it would be interesting to characterize the surfaces where the equality holds.

Corollary 2.13. Let Z be a smooth projective surface of general type. Assume that $q(Z)\geq 4$ and Z is of maximal Albanese dimension, and either Z is birational to a product of two smooth projective curves of genus $2$ or $\operatorname {\mathrm {vol}}(Z, K_Z)\geq 16$ .

Proof. We first assume that Z is a minimal surface of general type. Then, $\chi (\omega _Z)\geq 1$ and $\operatorname {\mathrm {vol}}(Z, K_Z)=K_Z^2>0$ .

When $\chi (\omega _Z)=1$ , by the main result of [HP] (see also [JLT]) and the assumption that $q(Z)\geq 4$ , we know that $q(Z)=4$ and Z is birational to a product of two smooth projective curves of genus $2$ .

We now consider the case $\chi (\omega _{Z})= 2$ and $q(Z)\geq 4$ . When $q(Z)\geq 5$ , then $\chi (\omega _Z)<q(Z)-2$ . By the Castelnuovo-de Francis theorem (see, for instance, [PP, Theorem A]), there exists a fibration $f: Z\rightarrow C$ from Z to a smooth projective curve of genus $\geq 2$ . We denote by F a general fiber of f. Then, $g(C)+g(F)\geq q(Z)\geq 5$ (see, for instance, [D2, the Lemme in the appendix]). We then have

$$ \begin{align*}\operatorname{\mathrm{vol}}(Z, K_Z)=K_Z^2\geq 8(g(C)-1)(g(F)-1)\geq 16\end{align*} $$

(see, for instance, [D2, the Corollaire in the appendix]). When $q(Z)=4$ , then $p_g(Z)=5$ . By the main result of [BNP], we have $16\leq \operatorname {\mathrm {vol}}(Z, K_Z)\leq 18$ .

When $\chi (\omega _Z)\geq 3$ , let $Z'$ be the smooth minimal model of the Albanese image $a_Z(Z)$ . After birational modifications of Z, we have the factorization of the Albanese morphism of Z

$$ \begin{align*}a_Z: Z\xrightarrow{\tau} Z'\xrightarrow{\rho} a_Z(Z)\hookrightarrow A_Z,\end{align*} $$

where $\rho $ is birational.

If $a_Z$ is birational onto its image, we apply Proposition 2.12 to conclude that

$$ \begin{align*}\operatorname{\mathrm{vol}}(Z, K_{Z})\geq 16.\end{align*} $$

We then assume that $\deg \tau>1$ . If $Z'$ is of general type, we have $\operatorname {\mathrm {vol}}(Z, K_Z)\geq (\deg \tau ) K_{Z'}^2\geq 2(K_{Z'}^2)$ by the ramification formula. By the previous discussions, we have already seen that either $Z'$ is birational to a product of two smooth projective curves of genus $2$ or $K_{Z'}^2\geq 16$ . In either case, $\operatorname {\mathrm {vol}}(Z, K_Z)\geq 16$ . Finally, we consider the case that $Z'$ is not of general type. Since $a_Z(Z)$ generates $A_Z$ , we see easily that $\rho $ is an isomorphism and $Z'$ is fibred by an elliptic curve E in $A_Z$ , and the quotient $C':=Z'/E$ is a smooth projective curve of genus $\geq q(Z)-1\geq 3$ . We then have a surjective morphism $Z\rightarrow C'$ from Z to $C'$ . We conclude again by [D2, the Corollaire in the appendix] that $\operatorname {\mathrm {vol}}(Z, K_Z)\geq 16$ .

2.4 Intersections with abelian subvarieties

When we apply Ito’s approach to study basepoint freeness thresholds of polarized abelian varieties $(A, L)$ , it may happen that the log canonical center Z of a pair $(A, D)$ is an abelian subvariety of A or is a subvariety fibred by an abelian subvariety of A.

Ito realized that one can deal with these cases with Poincaré’s reducibility theorem for polarized abelian varieties. When Z is an abelian subvariety, we have the following result [Ito2, Proposition 6.6].

Proposition 2.14. Let $(A, L) $ be a polarized abelian variety. Assume that there exists an effective $\mathbb Q$ -divisor D such that an abelian subvariety B is an irreducible component of $\mathrm {Nklt}(A, D)$ and $tL-D$ is an ample $\mathbb Q$ -divisor. Assume, furthermore, that $\beta (L|_B)<t$ . Then, $\beta (L)<t$ .

When Z is fibred by an abelian subvariety B, it is important to estimate the intersection number $(L^{\dim B}\cdot B)$ . The author learned the following result from a private communication with Atsushi Ito.

Lemma 2.15. Let D be an ample $\mathbb Q$ -divisor on A and let B be a abelian subvariety of A of dimension d. Let $\varphi : A\rightarrow A/B$ be the quotient morphism. Then, there exists an ample $\mathbb Q$ -divisor $H_B$ on $A/B$ such that $D-\varphi ^*H_B$ is a nef $\mathbb Q$ -divisor and $D^g=\binom {g}{d}(D^d\cdot B)(H_B^{g-d})_{A/B}$ .

Proof. Let M be a positive sufficiently divisible integer such that $MD$ is an integral divisor and $L=\mathcal {O}_A(MD)$ is an ample line bundle on A. By Poincaré’s reducibility (see, for instance, [BL, Corollary 5.3.6]), there exists an abelian subvariety K of A such that the natural morphism $K\rightarrow A/B$ is an isogeny and the addition morphism $\pi : K\times B\rightarrow A$ induces an isogeny of polarized abelian varieties $(K, L_K)\times (B, L_B)\rightarrow (A, L)$ , where $L_K$ and $L_B$ are, respectively, the restriction of L on K and B. Then, one can consider the natural isogeny $\mu _K: K\rightarrow A/B$ . Note that $L|_K$ cannot descend to $A/B$ in general, but there exists an effective $\mathbb Q$ -divisor $D_B$ on $A/B$ such that $L|_K$ is algebraically equivalent to $\mu _K^*D_B$ as $\mathbb Q$ -divisors. Let $H_B=\frac {1}{M}D_B$ , and it is easy to check that $H_B$ satisfies the desired properties.

Corollary 2.16. Let $(A, L)$ be a polarized abelian variety of dimension g with $n(L)<\frac {1}{p+2}$ . Assume that Conjecture 1.4 holds in dimension $\leq g-1$ . Let $D=\frac {1}{p+2}L$ . Then, either

(2) $$ \begin{align} (D^d\cdot B)\geq \frac{(D^g)}{\binom{g}{d}(g-d)^{g-d}} \end{align} $$

for all abelian subvariety B of dimension $1\leq d\leq g-1$ or $\beta (L)<\frac {1}{p+2}$ .

Remark 2.17. Let $0<d<g$ be positive integers. Then,

$$ \begin{align*}\frac{g^g}{d^d\binom{g}{d}}>(g-d)^{g-d}.\end{align*} $$

More precisely, by Stirling’s formula, for any positive integer n, we have

$$ \begin{align*}\sqrt{2\pi}n^{n+\frac{1}{2}}e^{-n+\frac{1}{12n+1}}<n!<\sqrt{2\pi}n^{n+\frac{1}{2}}e^{-n+\frac{1}{12n}}.\end{align*} $$

Thus,

$$ \begin{align*}\frac{g^g}{d^d\binom{g}{d}}>(g-d)^{g-d}\sqrt{\frac{2\pi d(g-d)}{g}}.\end{align*} $$

Proof. If the inequalities (2) fail for some abelian subvarieties, we pick B such that $\dim B=d$ is maximal and $(D^d\cdot B)< \frac {(D^g)}{\binom {g}{d}(g-d)^{g-d}}$ . By Lemma 2.15, there exists an ample $\mathbb Q$ -divisor $H_{B}$ such that $D-\varphi ^*H_{B}$ is a nef $\mathbb Q$ -divisor and

$$ \begin{align*}(H_{B}^{g-d})_{A/B}=\frac{(D^g)}{\binom{g}{d}(D^d\cdot B)}>(g-d)^{g-d}.\end{align*} $$

Moreover, by the argument of Lemma 2.15, for each abelian subvariety C of $A/B$ of dimension $r>0$ , $(H_{B}^r\cdot C)_{A/B}=\frac {(D^{d+r}\cdot \varphi ^{-1}(C))}{\binom {d+r}{d}(D^d\cdot B)}$ . By the maximality of B, we conclude that

$$ \begin{align*} (H_{B}^r\cdot C)_{A/B}=\frac{(D^{d+r}\cdot \varphi^{-1}(C))}{\binom{d+r}{d}(D^d\cdot B)}>\qquad &\frac{ \binom{g}{d}(g-d)^{g-d}}{\binom{d+r}{d}\binom{g}{d+r}(g-d-r)^{g-d-r}}\\ &=\frac{ (g-d)^{g-d}}{(g-d-r)^{g-d-r}\binom{g-d}{r}}\\ & >r^r. \end{align*} $$

Since Conjecture 1.4 holds in dimension $g-d$ , $\mathcal {I}_{o_{A/B}}\langle (1-\epsilon )H_B\rangle $ is GV for some $0<\epsilon \ll 1$ . Thus, $\mathcal {I}_B\langle (1-\epsilon )\varphi ^*H_B\rangle $ is also GV. Since $D-\varphi ^*H_B$ is a nef $\mathbb Q$ -divisor, $\mathcal {I}_B\langle (1-\epsilon )D\rangle $ is GV. We then consider the short exact sequence

$$ \begin{align*}0\rightarrow \mathcal{I}_B\rightarrow \mathcal{I}_o\rightarrow \mathcal{I}_{o, B}\rightarrow 0.\end{align*} $$

Since by the assumption that Conjecture 1.4 holds in dimension d, $\mathcal {I}_{o, B}\langle (1-\epsilon )D\rangle $ is also GV and hence, $\mathcal {I}_o\langle (1-\epsilon )D\rangle $ is GV. Thus, $\beta (L)<\frac {1}{p+2}$ .

Lemma 2.18. Let D be an effective ample $\mathbb Q$ -divisor on an abelian variety A. Assume that $(A, D)$ is log canonical at o, and the minimal log canonical center Z through o is a subvariety of dimension d fibred by an abelian subvariety B of dimension $d'$ such that the desingularization of $Z/B$ is of general type. Then,

$$ \begin{align*}(D^d\cdot Z)\geq \binom{d}{d'}(D^{d'}\cdot B)\operatorname{\mathrm{vol}}(K_{(Z/B)'}),\end{align*} $$

where $(Z/B)'$ is a smooth model of the quotient $Z/B$ .

Proof. We consider the quotient morphism $A\rightarrow A/B$ . We may assume that $MD$ is an integral divisor corresponding to a line bundle L for some integer $M>0$ . By Poincaré’s reducibility theorem (see, for instance, [BL, Corollary 5.3.6]), there exists an abelian subvariety K of A such that the natural morphism $K\rightarrow A/B$ is an isogeny, and the addition morphism $\pi : K\times B\rightarrow A$ induces an isogeny of polarized abelian varieties $(K, L_K)\times (B, L_B)\rightarrow (A, L)$ , where $L_K$ and $L_B$ are, respectively, the restriction of L on K and B.

Note that $\tilde {Z}:=Z\times _A(K\times B)$ is isomorphic to the product $\tilde {Z/B}\times B$ , where $\tilde {Z/B}=(Z/B)\times _{A/B}K$ . Thus,

$$ \begin{align*} (D^d\cdot Z)&=\frac{1}{M^d}(L^d\cdot Z)=\frac{1}{M^d\deg\pi}((L_K\boxtimes L_B)^d\cdot\tilde{Z})\\ &=\frac{1}{M^d\deg\pi}\big((L_K\boxtimes L_B)^d\cdot(\tilde{Z/B}\times B)\big)\\ &=\frac{\binom{d}{d'}}{M^{d-d'}\deg\pi}(D^{d'}\cdot B)(L_K^{d-d'}\cdot \tilde{Z/B}). \end{align*} $$

Let $\nu : \overline {Z}\rightarrow Z$ be its normalization. By Proposition 2.1, $\nu ^*D\sim _{\mathbb Q}K_{\overline {Z}}+V_{\overline {Z}}$ for some effective $\mathbb Q$ -divisor $V_{\overline {Z}}$ . Consider the pullback of this $\mathbb Q$ -linear equivalence on the normalization $ \overline {\tilde {Z}}$ of $\tilde {Z}$ and then restricting it to a general fiber of $ \overline {\tilde {Z}}\rightarrow B$ . We see that $\varsigma ^*(\frac {1}{M}L_{K})\sim _{\mathbb Q}K_{\widehat {Z/B}}+V_{\widehat {Z/B}} $ , where $\varsigma :\widehat {Z/B}\rightarrow \tilde {Z/B}$ is the normalization and $V_{\widehat {Z/B}}$ is an effective $\mathbb Q$ -divisor on $\widehat {Z/B}$ . Thus, $(L_K^{d-d'}\cdot \tilde {Z/B})= M^{d-d'}\operatorname {\mathrm {vol}}(\widehat {Z/B}, K_{\widehat {Z/B}}+V_{\widehat {Z/B}})\geq (\deg \pi )M^{d-d'}\operatorname {\mathrm {vol}}(K_{(Z/B)'})$ .

3 Very general polarized abelian varieties

In [Jz], we applied Helmke’s induction to confirm that Conjecture 1.4 holds for Hodge theoretically very general polarized abelian varieties with special polarizations. We now show that the calculation indeed implies that Conjecture 1.4 holds for almost all generic polarized abelian varieties in fixed dimensions.

Recall that we say a polarized abelian variety $(A, L)$ is Hodge theoretically very general if $\dim _{\mathbb Q}H^{k,k}(A, \mathbb Q)=1$ for all $1\leq k\leq g-1$ . We observe that by Hard Lefschetz and Poincaré duality, $(A, L)$ is Hodge theoretically very general iff $\dim _{\mathbb Q}H^{\lfloor \frac {g}{2}\rfloor ,\lfloor \frac {g}{2}\rfloor }(A, \mathbb Q)=1$ . We also observe that when $(A, L)$ is Hodge theoretically very general, A is a simple abelian variety and hence, $n(L)=\displaystyle\frac {g}{\sqrt [g]{(L^g)}}$ . Note that in order to compare $\beta (L)$ and $n(L)$ , we can assume that L is primitive.

Theorem 3.1. Let $(A, L)$ be a Hodge theoretically very general polarized abelian variety of type $l=(1,\delta _2,\ldots ,\delta _g)$ . Assume that

$$ \begin{align*} \delta:=\delta_2\cdots\delta_g\geq \mathrm{ max}\big\{\frac{(k(k+1)\cdots(g-1))^{\frac{g(g-1)}{k}}}{(g!)^{\frac{(g-1)(g-k)}{k}}}\mid 2\leq k\leq g-2\big\}. \end{align*} $$

Then, $\beta (L) \leq n(L).$

Proof. We apply Ito’s strategy that it suffices to show $r'(L)\leq n(L)$ . Let $t\in (n(L), n(L)+\epsilon )$ be a rational number, where $0<\epsilon \ll 1$ and denote by $D\sim _{\mathbb Q} tL$ an effective rational number such that $\mathrm {mult}_0D>g$ (there exists such D since $((tL)^g)>g^g$ ). We then just need to apply Proposition 2.3 to get a divisor $D'\sim _{\mathbb Q}cD$ with $0<c<1$ , and the neutral element o of A is a minimal log canonical center of $(A, D')$ . By Proposition 2.3, it suffices to verify that $(D^{k}\cdot Z)>\binom {g-1}{g-k}g^k$ for any irreducible subvariety Z of dimension k for $1\leq k\leq g-1$ .

Recall that $\beta (L)\leq 1$ and equality holds if and only if $|L|$ has a basepoint. Thus, we may assume that $(L^g)>g^g$ (i.e., $n(L)<1$ ). Then, since the class $[L]$ is the generator of $\mathrm {NS}(A)=\operatorname {\mathrm {Pic}}(A)/\operatorname {\mathrm {Pic}}^0(A)$ , we can assume that Z is of codimension $\geq 2$ . Since A is simple, by the main result of [D1], for any irreducible curve Z of A, $(L\cdot Z)>\sqrt [g]{(L^g)}>g$ . Thus, it suffices to verify that $(D^{k}\cdot Z)>\binom {g-1}{g-k}g^k$ for any irreducible subvariety Z of dimension k, where $2\leq k\leq g-2$ .

For any irreducible subvariety Z of dimension k, we denote by $[Z]\in H^{g-k, g-k}(A, \mathbb Z)\subset H^{2g-2k}(A, \mathbb C)$ its cohomology class. Then, $[Z]$ is a positive integral multiple of $\frac {L^{g-k}}{(g-k)!\delta _2\cdots \delta _{g-k}}$ by the assumption that A is Hodge theoretically very general. Thus,

$$ \begin{align*} (D^k\cdot Z)> \frac{g^k}{(L^g)^{\frac{k}{g}}}(L^k\cdot Z) \geq g^k\frac{(L^g)^{\frac{g-k}{g}}}{(g-k)!\delta_2\cdots\delta_{g-k}}. \end{align*} $$

We just need to verify that

(3) $$ \begin{align} \frac{(L^g)^{\frac{g-k}{g}}}{(g-k)!\delta_2\cdots\delta_{g-k}}\geq \binom{g-1}{g-k}. \end{align} $$

Note that $\delta _2\cdots \delta _{g-k}\leq \delta ^{\frac {g-k-1}{g-1}}$ . Thus,

$$ \begin{align*} \frac{ (L^g)^{\frac{g-k}{g}}}{(g-k)!\delta_2\cdots\delta_{g-k}} \geq &\delta^{\frac{g-k}{g}-\frac{g-k-1}{g-1}}\frac{ (g!)^{\frac{g-k}{g}}}{(g-k)!}\\ &\ \ =\delta^{\frac{k}{g(g-1)}}\frac{ (g!)^{\frac{g-k}{g}}}{(g-k)!}. \end{align*} $$

The assumption on $\delta $ makes sure that $ \frac {(L^g)^{\frac {g-k}{g}}}{(g-k)!\delta _2\cdots \delta _{g-k}}\geq \binom {g-1}{g-k}$ for $2\leq k\leq g-2$ .

By the above theorem, we see that in order to verify Conjecture 1.1 and Conjecture 1.4 for very general polarized abelian varieties, it suffices to check finitely many families in each dimension. By the same computation with some extra efforts, we finish the proof of Theorem 1.5.

Proof. When $g=4$ , the assumption in Theorem 3.1 is simply that $\delta =h^0(A, L)\geq \frac {6^6}{24^3}=\frac {27}{8}$ . However, for $\delta \leq 3$ , $n(L)=\frac {4}{\sqrt [4]{24\delta }}>1$ we know that $|L|$ has basepoints and $\beta (L)=1$ .

When $g=5$ , we repeat the argument in the proof of Theorem 3.1. We first check (3) for $g=5$ and $k=2$ or $3$ , which are $(L^5)^{\frac {3}{5}}\geq 24\delta _2\delta _3$ and $(L^5)^{\frac {2}{5}}\geq 12\delta _2$ . It is easy to verify that both inequalities hold when $\delta _5\geq 5$ or $\delta _2\geq 3$ . When $\delta _2=1$ , $\delta \geq 5$ implies that both inequalities hold and if $\delta <5$ , $\beta (L)=1$ . When $\delta _2=2$ and $\delta _5\leq 4$ , the above inequalities fail only when the polarization type is $(1,2,2,2,2)$ . But in this case, $n(L)=\displaystyle\frac {5}{\sqrt [5]{120\delta (L)}}>1$ . Thus, we still have $\beta (L)\leq 1<n(L)$ .

When $g=6$ , we first remark that we may assume that l is not of the form $(1,\ldots , 1, \delta _6)$ . If l is of the form $(1,\ldots , 1, \delta _6)$ , since $(A, L)$ is very general, by [Ito3, Theorem 1.5], $\beta (L)\leq \displaystyle\frac {1}{\lfloor \sqrt [6]{\delta _6}\rfloor }<\frac {6}{\sqrt [6]{720\delta _6}}$ . Thus, we will assume that $\delta _5\geq 2$ . We then check (3) for $k=2$ , $3$ and $4$ , which are $(L^6)\geq (20\delta _2)^3$ , $(L^6)\geq (60\delta _2\delta _3)^2$ and $(L^6)^{\frac {2}{3}}\geq 120\delta _2\delta _3\delta _4$ . Since $\delta _5\geq 2$ , we see that these inequalities hold when $\delta _6\geq 5$ . When $\delta _6=4$ , one can verify that these inequalities hold, except the polarization types $(1,4,4,4,4,4)$ or $(1,1, 1,1,2,4)$ or $(1, 2, 2, 2, 2, 2, 4)$ . When $\delta _6=3$ , these inequalities hold, except the polarization types $(1,3,3,3,3,3)$ . Note that for the polarization types $(1, 1, 1,1, 2,4)$ , or $(1,2,2,2,2,4)$ or with $\delta _6\leq 2$ , $n(L)>1$ and thus, $\beta (L)<n(L)$ . We finally need to consider the polarization types $(1,4,4,4,4,4)$ and $(1,3,3,3,3,3)$ . These two cases can be dealt by a result of Ito. When $(A, L)$ is a polarized abelian sixfold of polarization type $(1,4,4,4,4,4)$ (resp. $(1,3,3,3,3,3)$ ), let $(S, L')$ be a very general polarized abelian surface of polarization type $(1, 4)$ (resp. $(1, 3)$ ). By [Ito5, Proposition 5.1], we always have $\beta (L)\leq \beta (L')$ . It is known that $\beta (L')=\frac {1}{2}$ (resp. $\frac {2}{3}$ ) (see [Ito3, Proposition 4.3 and Lemma A.4]). We then still have $\beta (L)<n(L)$ for the polarization types $(1,4,4,4,4,4)$ and $(1,3,3,3,3,3)$ .

Remark 3.2. The last paragraph of the above proof is due to the anonymous referee.

4 Abelian fourfolds

4.1 The proof of Theorem 1.6

Proof. It suffices to show that $\beta (L)<\frac {1}{p+2}$ or equivalently $\mathcal {I}_o\langle \frac {1}{p+2}L\rangle $ is IT $^0$ , where o is the neutral element of A.

Since $(L^4)>((2+\frac {4}{\sqrt {3}})(p+2))^4$ , there exists an effective $\mathbb Q$ -divisor $D\sim _{\mathbb Q}\frac {1}{p+2}L$ such that $\mathrm {mult}_o(D)=m>2+\frac {4}{\sqrt {3}}$ . Let $c=\mathrm {lct}(D, o)\leq \frac {4}{m}$ be the log canonical threshold of D at o and let Z be the minimal log canonical center of $(A, cD)$ through o. By Proposition 2.7, we may assume that $c\geq \frac {1}{2}$ .

Step 1. We first deal with the case that Z is a divisor. By Lemma 2.5, $\mathcal {I}_K\langle \frac {c}{p+2}L\rangle $ is GV, where K is the kernel of $\varphi _{Z}$ . If K is a point, we are done. Otherwise, by the assumption that $(L^d\cdot B)>(d(p+2))^d$ for any abelian subvariety B of dimension $1\leq d\leq 3$ and Ito’s results [Ito1, Ito2], $\mathcal {I}_{o,K}\langle \frac {1}{p+2}L\rangle $ is IT $^0$ . Thus, from the short exact sequence

$$ \begin{align*}0\rightarrow \mathcal{I}_K\rightarrow \mathcal{I}_o\rightarrow \mathcal{I}_{o, K}\rightarrow 0,\end{align*} $$

we conclude that $\mathcal {I}_o\langle \frac {1}{p+2}L\rangle $ is IT $^0$ .

Step 2. If Z is a curve, by Proposition 2.3, Z is smooth at o and as soon as $(D\cdot Z)>4\geq \frac {b_o(A, cD)}{1-c}$ , there exists $D_1\sim _{\mathbb Q}c_1D$ with $c<c_1<1$ such that $(A, D_1)$ is log canonical at o and $\{o\}$ is a minimal log canonical center. Then, we have by Remark 2.4 that $\beta (L)\leq r'(L)\leq \frac {c_1}{p+2}<\frac {1}{p+2}$ .

By Corollary 2.2, we know that

$$ \begin{align*}(cD\cdot Z)\geq 2g(\overline{Z})-2,\end{align*} $$

where $\nu : \overline {Z}\rightarrow Z$ is the normalization. Thus, we are done once $g(\overline {Z})\geq 3$ .

When $g(\overline {Z})=1$ , $Z=\overline {Z}$ is an elliptic curve. We conclude again by Proposition 2.14, Remark 2.4 and the assumption that $(L\cdot Z)>p+2$ and thus, $\beta (L|_Z)<\frac {1}{p+2}$ .

If $g(\overline {Z})=2$ , Z generates an abelian surface B of A. By Corollary 2.16, we may assume that $(D^2\cdot B)\geq \frac {4^4}{\binom {4}{2}2^2}= \frac {32}{3}$ . Then, by Hodge index theorem,

$$ \begin{align*}(D\cdot Z)_B\geq \sqrt{(Z^2)_B(D^2\cdot B)} \geq 8/\sqrt{3}>4.\end{align*} $$

Step 3. When Z is a surface, we need to apply Helmke’s induction. We may assume that Z is not an abelian surface. Otherwise, we conclude directly by Proposition 2.14 and Ito’s work in [Ito1]. We know that $\mathrm {mult}_oZ\leq 3$ by Proposition 2.3. Let $\mu : \tilde {Z}\rightarrow \overline {Z}\rightarrow Z$ be the minimal resolution of the normalization $\overline {Z}$ of Z. Since Z is not an abelian variety, $\tilde {Z}$ is a surface of maximal Albanese dimension of Kodaira dimension $\geq 1$ . Since there exist no rational curves on $\overline {Z}$ , $\tilde {Z}$ is minimal.

Claim. $((cD)^2\cdot Z)\geq 16$ when $\mathrm {mult}_oZ=3$ ; $((cD)^2\cdot Z)\geq 16$ or $(D^2\cdot Z)\geq 16\sqrt [3]{6}$ when $\mathrm {mult}_oZ=2$ ; $(D^2\cdot Z)>16$ when Z is smooth at o.

When $\tilde {Z}$ is not of general type, Z is fibred by an elliptic curve E, and since Z has rational singularities around o, Z is indeed smooth at o. We need to show that $(D^2\cdot Z)>16$ . Let $C=Z/E$ be the quotient and $\tilde {C}$ be the normalization of C. By Corollary 2.16, we may assume $(D\cdot E)\geq \frac {64}{27}$ and by Lemma 2.18, we have

$$ \begin{align*}(D^2\cdot Z)\geq 2(2g(\tilde{C})-2)(D\cdot E).\end{align*} $$

Thus, we are done when $g(\tilde {C})\geq 3$ . When $g(\tilde {C})=2$ , Z generates an abelian $3$ -fold B of A and $C\hookrightarrow B/E$ is an ample divisor. Thus, $(C^2)_{B/E}=a\geq 2$ and hence, $Z^2$ is algebraically equivalent to $aE$ as $1$ -cycles of B. By Corollary 2.16, we may assume that $(D^3\cdot B)\geq 4^3$ . Then, by Hodge index,

$$ \begin{align*} (D^2\cdot Z)=((D|_B)^2\cdot Z)_B\quad &\geq \sqrt{(D^3)_B(D|_B\cdot Z^2)_B}\\ & \geq \sqrt{4^3\cdot a\cdot \frac{64}{27}}\geq 16\sqrt{\frac{32}{27}}>16. \end{align*} $$

We now assume that $\tilde {Z}$ is of general type.

If $q(\tilde {Z})\geq 4$ , by Corollary 2.13, $\operatorname {\mathrm {vol}}(K_{\tilde {Z}})\geq 16$ or $\tilde {Z}\simeq C_1\times C_2$ , where $C_i$ is a smooth projective curve of genus $2$ . In the latter case $\mu $ is the normalization of Z, and since Z is normal at o, it is smooth at o. We apply Proposition 2.1 and conclude that

$$ \begin{align*} (D^2\cdot Z)=(\mu^*(D)^2)_{\tilde{Z}}\quad & \geq (K_{\tilde{Z}}\cdot \mu^*D)_{\tilde{Z}} \\ &=2(C_1\cdot \mu^*D)_{\tilde{Z}} +2(C_2\cdot \mu^*D)_{\tilde{Z}}. \end{align*} $$

Note that the image of $C_i$ generates an abelian surface $B_i$ of A. By Corollary 2.16, we may assume that $(D^2\cdot B_i)\geq \frac {32}{3}$ . Thus, by Hodge index, $(C_i\cdot \mu ^*D)_{\tilde {Z}}\geq 8/\sqrt {3}$ . Therefore, we have $(D^2\cdot Z)\geq 32/\sqrt {3}>16$ . In the former case, $ ((cD)^2\cdot Z)=\operatorname {\mathrm {vol}}(Z, cD)\geq \operatorname {\mathrm {vol}}(K_{\tilde {Z}})\geq 16$ by Corollary 2.2.

If $q(\tilde {Z})=3$ , Z generates an abelian 3-fold $B\subset A$ . Then, Z is an ample divisor of B. Moreover, in this case, the embedded dimension of Z at o is at most $3$ . Thus, $\mathrm {mult}_o(Z)\leq 2$ by the well-known facts about isolated rational surface singularities (see [A, Corollary 6]). As before, by Corollary 2.16, we may assume that $(D^3\cdot B)\geq 4^3$ . Since Z is an ample divisor of B, $(Z^3)_B\geq 6$ . Thus,

$$ \begin{align*}(D^2\cdot Z)\geq \sqrt[3]{(D^3\cdot B)^2(Z^3)_B}\geq 16\sqrt[3]{6}.\end{align*} $$

Step 4.

If Z is smooth at o, we have shown that $(D^2\cdot Z)>16$ . Thus, by Proposition 2.3, there exists an effective $\mathbb Q$ -divisor $D_1\sim _{\mathbb Q} c_1D$ with $c_1<1$ such that $(A, D_1)$ is log canonical at o whose minimal log canonical center $Z_1$ through o is a proper subset of Z and $\frac {b_o(A, D_1)}{1-c_1}\leq \frac {b_o(A, cD)}{1-c}\leq 4$ . We then finish the proof by going back to Step 2.

If $\mathrm {mult}_oZ= 3$ , we have already seen that $((cD)^2\cdot Z)\geq 16$ . In order to apply Helmke’s induction, we need to verify that

$$ \begin{align*}(D^2\cdot Z)>3\big(\frac{b_o(A, cD)}{1-c}\big)^2.\end{align*} $$

Note that $b_o(A, cD)\leq 4-cm<4-(2+\frac {4}{\sqrt {3}})c$ . It is elementary to verify that

$$ \begin{align*}\frac{16}{c^2}\geq 3\big(\frac{4-(2+\frac{4}{\sqrt{3}})c}{1-c}\big)^2\end{align*} $$

always holds. We then finish the proof as before.

If $\mathrm {mult}_oZ=2$ and $((cD)^2\cdot Z)\geq 16$ , we conclude as the multiplicity $3$ case. If $\mathrm {mult}_oZ=2$ and $(D^2\cdot Z)\geq 16\sqrt [3]{6}$ , we need to verify that

$$ \begin{align*}(D^2\cdot Z)>2\big(\frac{b_o(A, cD)}{1-c}\big)^2.\end{align*} $$

Since $c\geq \frac {1}{2}$ , we have $\frac {b_o(A, cD)}{1-c}<4-\frac {(\frac {4}{\sqrt {3}}-2)c}{1-c}\leq 6-\frac {4}{\sqrt {3}}$ . We then check that $16\sqrt [3]{6}>2(6-\frac {4}{\sqrt {3}})^2$ .

4.2 The proof of Theorem 1.8

We apply the same strategy as in the proof of Theorem 1.6.

Fix an effective $\mathbb Q$ -divisor such that $D\sim _{\mathbb Q}\frac {1}{p+2}L$ , such that $\mathrm {mult}_oD>8$ . Let $c_1=\mathrm {lct}(D, o)<\frac {5}{8}$ be the log canonical threshold of D at o and let $Z_1$ be the minimal log canonical center of $(A, c_1D)$ at o. By Proposition 2.7, we may assume that $c_1\geq \frac {1}{2}$ . Thus,

$$ \begin{align*}\frac{b_o(A, c_1D)}{1-c_1}\leq \frac{5-c_1\mathrm{mult}_oD}{1-c_1}<5-3\frac{c_1}{1-c_1}\leq 2.\end{align*} $$

If $Z_1$ is a divisor, we conclude by Lemma 2.5.

If $Z_1$ is a threefold, we apply Helmke’s induction. Let $\rho : \tilde {Z_1}\rightarrow Z_1$ be a desingularization. Then, $\operatorname {\mathrm {vol}}(K_{\tilde {Z_1}})\geq \frac {5}{2}\times 3!\times 3=45$ by Theorem 2.8, Theorem 2.9 and Remark 2.10. We also note that $\mathrm {mult}_oZ_1\leq 6$ . Note that

$$ \begin{align*}(D^3\cdot Z_1)\geq \operatorname{\mathrm{vol}}(K_{\tilde{Z_1}})/c_1^3>\frac{45}{(\frac{5}{8})^3}\geq 48>\big(\frac{b_o(A, c_1D)}{1-c_1}\big)^3\mathrm{mult}_{o}(Z_1).\end{align*} $$

Thus, there exists an effective $\mathbb Q$ -divisor $ D_2\sim _{\mathbb Q}c_2D$ such that $c_1<c_2<1$ , such that $(A, D_2)$ is log canonical at o, whose minimal lc center through o is a proper subvariety $Z_2$ contained in $Z_1$ , and $\frac {b_o(A, D_2)}{1-c_2}<\frac {b_o(A, c_1D)}{1-c_1}<2$ .

When $Z_2$ is a surface, we have $\mathrm {mult}_oZ_2\leq 4$ . Let $\tilde {Z}_2$ be its smooth model. Since A is simple, $\tilde {Z}_2$ is of general type and hence, $\operatorname {\mathrm {vol}}(K_{\tilde {Z_2}})\geq 16$ by Corollary 2.13. We then have

$$ \begin{align*}(D^2\cdot Z_2)>\operatorname{\mathrm{vol}}(K_{\tilde{Z_2}})\geq 16>\big(\frac{b_o(A, D_2)}{1-c_2}\big)^2\mathrm{mult}_oZ_2.\end{align*} $$

Thus, by Helmke’s induction, we may assume that $Z_2$ is a curve. In this case, we verify easily that $(D\cdot Z_2)>\frac {b_o(A, D_2)}{1-c_2}$ . Therefore, there exists an effective $\mathbb Q$ -divisor $D_3\sim _{\mathbb Q}c_3L$ with $c_2<c_3<1$ such that $(A, D_3)$ is log canonical at o and o is a minimal log canonical center of $(A, D_3)$ . We then finish the proof of Theorem 1.8.

4.3 The proof of Theorem 1.9

The proof of Theorem 1.9 is identical to that of Theorem 1.8. We first observe that $\alpha _g\leq \alpha _{g,2}=\sqrt {\frac {5(g-2)+1}{g-1}}<\sqrt {5}$ . We then fix an effective $\mathbb Q$ -divisor $D\sim \frac {1}{p+2}L$ such that $\mathrm {mult}_oD>2g-\alpha _g$ and thus let $c<\frac {g}{2g-\sqrt {5}}$ be the log canonical threshold of $(A, D)$ at o. We may assume that $c\geq \frac {1}{2}$ by Proposition 2.7. Then

$$ \begin{align*}\frac{b_o(A, cD)}{1-c}\leq \frac{g-c (\mathrm{mult}_oD)}{1-c}\leq g-(\mathrm{mult}_oD-g)<\alpha_g.\end{align*} $$

Let Z be the minimal log canonical threshold of $(A, cD)$ through o. Let $d=\dim Z$ . Then, $\mathrm {mult}_oD\leq \binom {g-1}{g-d}$ .

If $d=g-1$ , we conclude by Lemma 2.5. Thus, we may assume that $1\leq d\leq g-2$ .

For a smooth model $\tilde {Z}$ of Z, by Theorem 2.8, Theorem 2.9, Remark 2.10 and Proposition 2.12, we have $\operatorname {\mathrm {vol}}(K_{\tilde {Z}})\geq \frac {15}{2}(g-2)!$ when $d=g-2$ , $\operatorname {\mathrm {vol}}(K_{\tilde {Z}})\geq \frac {5}{2}d!(g-d)$ when $3\leq d\leq g-3$ , $\operatorname {\mathrm {vol}}(K_{\tilde {Z}})\geq 5(g-2)+1$ when $d=2$ and $\operatorname {\mathrm {vol}}(K_{\tilde {Z}})\geq 2(g-1)$ when $d=1$ . By Corollary 2.2, $(D^d\cdot Z)\geq \frac {1}{c^d}\operatorname {\mathrm {vol}}(K_{\tilde {Z}})>\operatorname {\mathrm {vol}}(K_{\tilde {Z}})$ . We then have

$$ \begin{align*}(D^d\cdot Z)> (\alpha_g)^d\mathrm{mult}_oD.\end{align*} $$

We then repeatedly apply Helmke’s induction and the above calculation to cut down the log canonical centers and finish the proof.