Published online by Cambridge University Press: 18 September 2023
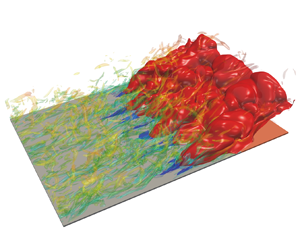
Lean hydrogen/air premixed flame flashback in a turbulent boundary layer over a flat plate is investigated using three-dimensional direct numerical simulation with detailed chemical kinetics. The upstream propagation of the flame takes place in near-wall turbulence and the interaction between the flame and the approaching reactant flow is studied. It is found that backflow regions are always present immediately upstream of flame bulges that are convex towards the reactants, confirming earlier observations. A flashback speed, including the effects of flame displacement speed and flow velocity, is introduced to quantify the flame flashback behaviour. This analysis indicates that the flashback speed is overall positive and it is considerably affected by the presence of the backflow regions. A budget analysis of the pressure transport equation is performed to explain the presence of the backflow regions. It is suggested that the positive dilatation and thermal diffusion terms near the leading edge of flame bulges are the main reasons for the pressure increase, leading to an adverse pressure gradient. The effects of the flame-induced adverse pressure gradient on the structures of the turbulent boundary layer are also investigated. It is revealed that the near-wall mean velocity and skin-friction coefficient are reduced due to the adverse pressure gradient. The coherent vortical structures of the boundary layer turbulence are lifted by the adverse pressure gradient. The analysis of the Reynolds stress component showed that the ejection event is augmented by combustion while the sweep event is attenuated, which facilitates the occurrence of flame flashback.