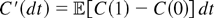We consider a storage model that can be on or off. When on, the content increases at some state-dependent rate and the system can switch to the off state at a state-dependent rate as well. When off, the content decreases at some state-dependent rate (unless it is at zero) and the system can switch to the on position at a state-dependent rate. This process is a special case of a piecewise deterministic Markov process. We identify the stationary distribution and conditions for its existence and uniqueness.

We propose an analytically tractable model of loading-dependent cascading failure that captures some of the salient features of large blackouts of electric power transmission systems. This leads to a new application and derivation of the quasibinomial distribution and its generalization to a saturating form with an extended parameter range. The saturating quasibinomial distribution of the number of failed components has a power-law region at a critical loading and a significant probability of total failure at higher loadings.

In this article, we study comparison theorems for stochastic functionals like V(∞;C) = sup0≤t {M(t) − C(t)} or V(T;C) = sup0≤t≤T {M(t) − C(t)}, where {M(t)} and {C(t)} are two independent nondecreasing processes with stationary increments. We will tacitly assume that the considered stochastic functionals are proper random variables. We prove that V(T;C′) ≤icxV(T;C) ≤icxV(T;C′′), where and C′′(dt) = c(0) dt, provided dC(t) is absolute continuous with density c(t). Similarly, we show that V(∞;C′) ≤icxV(∞;C) ≤icxV(∞;C′′). For proofs, we develop the theory of the ≤idcv ordering defined by increasing directionally concave functions. We apply the theory to M/G/1 priority queues and M/G/1 queues with positive and negative customers.

Consider a single-commodity inventory system in which the demand is modeled by a sequence of independent and identically distributed random variables that can take negative values. Such problems have been studied in the literature under the name cash management and relate to the variations of the on-hand cash balances of financial institutions. The possibility of a negative demand also models product returns in inventory systems. This article studies a model in which, in addition to standard ordering and scrapping decisions seen in the cash management models, the decision-maker can borrow and store some inventory for one period of time. For problems with back orders, zero setup costs, and linear ordering, scrapping, borrowing, and storage costs, we show that an optimal policy has a simple four-threshold structure. These thresholds, in a nondecreasing order, are order-up-to, borrow-up-to, store-down-to, and scrap-down-to levels; that is, if the inventory position is too low, an optimal policy is to order up to a certain level and then borrow up to a higher level. Analogously, if the inventory position is too high, the optimal decision is to reduce the inventory to a certain point, after which one should store some of the inventory down to a lower threshold. This structure holds for the finite and infinite horizon discounted expected cost criteria and for the average cost per unit time criterion. We also provide sufficient conditions when the borrowing and storage options should not be used. In order to prove our results for average costs per unit time, we establish sufficient conditions when the optimality equations hold for a Markov decision process with an uncountable state space, noncompact action sets, and unbounded costs.

The problem of controlling transmission rate over a randomly varying channel is cast as a Markov decision process wherein the channel is modeled as a Markov chain. The objective is to minimize a cost that penalizes both buffer occupancy (equivalently, delay) and power. The nature of the optimal policy is characterized using techniques adapted from classical inventory control.

Consider the random Dirichlet partition of the interval into n fragments at temperature θ > 0. Explicit results on the law of its size-biased permutation are first supplied. Using these, new results on the comparative search cost distributions from Dirichlet partition and from its size-biased permutation are obtained.

In this article, we give several results on (multivariate and univariate) stochastic comparisons of generalized order statistics. We give conditions on the underlying distributions and the parameters on which the generalized order statistics are based, to obtain stochastic comparisons in the stochastic, dispersive, hazard rate, and likelihood ratio orders. Our results generalize some recent results for order statistics, record values, and generalized order statistics and provide some new results for other models such as k-record values and order statistics under multivariate imperfect repair.

In this article, we obtain, in a unified way, a closed-form analytic expression, in terms of roots of the so-called characteristic equation of the stationary waiting-time distribution for the GIX/R/1 queue, where R denotes the class of distributions whose Laplace–Stieltjes transforms are rational functions (ratios of a polynomial of degree at most n to a polynomial of degree n). The analysis is not restricted to generalized distributions with phases such as Coxian-n (Cn) but also covers nonphase-type distributions such as deterministic (D). In the latter case, we get approximate results. Numerical results are presented only for (1) the first two moments of waiting time and (2) the probability that waiting time is zero. It is expected that the results obtained from the present study should prove to be useful not only for practitioners but also for queuing theorists who would like to test the accuracies of inequalities, bounds, or approximations.