Introduction
In order to develop theoretical models for the flow of ice masses, it is necessary to know the constitutive law for the non-elastic deformation of polycrystalline ice. For steady state and multiaxial stresses, it is generally assumed that strain-rates ⋵ij are related to the corresponding deviatoric stresses by

where ɳ is viscosity, B and n are constants, and τ is the effective shear stress defined by τ2 = 1/2 ∑ij(τ'ij)2.
Equation (1) was verified by Reference DuvalDuval (1976) with creep tests on isotropic polycrystalline ice in torsion, compression, and torsion-compression. The exponent n is about 3, at least over the stress range 0.1 MPa < τ < 0.5 MPa. For low stresses and small grain sizes, diffusional creep is the dominant creep mechanism with n = 1 (Reference Goodman, Frost and AshbyGoodman and others 1981).
Equation (1) cannot be used when fabrics (preferred orientation of the c-axis) have been created. Natural ice masses exhibit strong crystallographic anisotropy, as observed by Reference Gow and WilliamsonGow and Williamson (1976) in the Byrd ice core.
The aim of this paper is to determine the deformation mechanisms of Antarctic ice and to explain the formation of the typical single-maximum fabrics. The influence of preferred c-axis orientation of the ice crystals on the creep behaviour and the variations of strain-rate with temperature are also discussed.
1. Deformation Mechanisms of Antarctic Ice
1.1 Crystalline texture of the Dome C ice core
Changes in crystal size with increasing depth for the 905 m long Dome C ice core were described by Reference Duval and LoriusDuval and Lorius (1980). Four typical thin-section photographs showing the crystalline texture of ice are presented in Figure 1. The expected increase of crystal size with age is observed down to 400 m. At greater depths, a significant decrease of crystal size is associated with the transition from the last glacial age to present climatic conditions. However, as shown in Figure 1, the typically equant texture of crystals is observed down to 905 m. The driving force for grain growth is thus probably only provided by the surface free energy of grain boundaries. The larger crystals grow at the expense of the smaller ones. A distribution of grain sizes is necessary for grain growth. From Reference Duval and LoriusDuval and Lorius (1980), the total microparticle content in the glacial ice in the Dome C ice core seems too low for them to inhibit grain growth.

Fig.1. Thin-section photographs of crystalline texture of ice in Dome C ice core between crossed polaroids (X1)
1.2 Deformation mechanisms In the first 905 m near Dome C
For the first 900 m of the Ice sheet near Dome C, shear stress caused by the surface slope Is too small to produce significant shear strain. However, the 1ce mass 1s plastically thinned with depth and the vertical velocity, for a constant thickness of Ice H, 1s given by
where b is the accumulation rate, z the distance from the bottom, and zm a constant (Reference LliboutryLliboutry in press).
The strain rate ⋵zz is
with b = 4 × 10−2 m a−1 and H = 3 400 m (Reference Lorius, Merlivat, Jouzel and PourchetLorius and others 1979). ⋵zz is about 10−13 s−1. From Reference Ritz, Lliboutry and RadoRitz and others (1982), the ice temperature is -53°C at the snow-ice transition and -47°C at 900 m. From the deformation map given by Reference Goodman, Frost and AshbyGoodman and others (1981), creep appears to be dominated by diffusional creep down to 905 m.The normal grain growth structure illustrated in Figure 1 supports this assumption
1.3 Formation mechanisms of single-maximum fabrics
Single-maximum fabrics are requently observed in Antarctic ice (Reference Lorius and VallonLorius and Val lon 1967, Reference KizakiKizaki 1969, Reference AndertonAnderton 1974, Reference Gow and WilliamsonGow and Williamson 1976, Reference Russell-Head and BuddRussell-Head and Budd 1979).In the Byrd ice core, Reference Gow and WilliamsonGow and Willamson (1976) found an increase in the preferred c-axis orientation from the surface down to 1 200 m. Fabrics with vertical c-axes are observed between 1 200 and 1 800 m. A transformation from single to multiple maximum fabrics occurs below 1 800 m. This variation in the crystal orientation fabrics with depth seems to be typical of cold ice sheets (Reference Russell-Head and BuddRussell-Head and Budd 1979).
It is important to determine the mechanisms for the formation of these single-maximum fabrics.Reference Hooke and HudlestonHooke and Hudleston (1980) suggest that marked single maximum fabrics occur at large cumulative strains or high stresses. For the Byrd ice core, assuming that the horizontal velocity is uniform along the core and provided that the ice thickness is constant, the vertical strain-rate is given by Equation (3).With b = 173 mm of ice a−1 and H = 2 164 m (Reference GowGow, 1975), ⋵zz is slightly less than 10−4 a−1. At 1 200 m, the total compressive strain in the vertical direction could be nearly 100%.This strain might be high enough to produce a strong fabric. However, the direction of the c-axes does not correspond to that induced by basal glide.
The vertical c-axes could be produced by horizontal shear. The shear stress, caused by the surface slope α is
where ρ is the density, g the acceleration due to gravity, and h the depth. With α =0.002 2 rad, taken from the contour maps dated 1964 of the American Geographical Society, the shear stress increases with depth and is only 0.2 × 105 Pa at 1 200 m. The corresponding shear strain-rate, deduced from the deformation maps given by Reference Goodman, Frost and AshbyGoodman and others (1981) for isotropic ice, is only 3 × 10−5 a−1. The singlemaximum fabrics found in the Byrd ice core therefore cannot be induced by horizontal shear.
This is supported by the fabrics study made by Reference Korotkevich, Petrov, Barkov, Sukhonosova, Dmitriyev and PortnovKorotkevich and others (1978) on the Vostok ice core. A gradual i ncrease in the preferred c-axis orientation of ice crystals is observed from the surface downwards.Fabrics with vertical c-axes are developed above 900 m. Considering that the surface slope near Vostok is very small (α<0.0013 rad) and the low ice temperature (<-55°C) down to 900 m, the progressive near vertical orientation of the c-axes cannot be produced by the horizontal shear, as for the Byrd ice core.
A possible explanation for the formation of single-maximum fabrics in Antarctic ice is the selective growth of ice crystal s with a vertical c-axis orientation. During the grain growth process, as observed in the Dome C ice (Fig.1), the larger crystals grow at the expense of the smaller ones.The single-maximum fabrics could arise from the selective growth of crystals with a vertical c-axis orientation in the first metres of the firn induced by the important thermal gradient.Another possible explanation is the growth of favourably oriented crystals with regard to the state of stress, as explained by Reference KambKamb (1959).The favoured crystal orientations are those which would have nearly maximum elastic strain energy if ice is only thinned in the vertical direction. Both these fabric formation mechanisms are compatibl e with diffusional creep. Obviously, this fabric promotes horizontal shear and from one depth, the dislocation creep will be the dominant creep mechanism, stopping grain growth and making stronger fabrics.A fabric study of the Dome C ice core is in progress to test these assumptions.
1.4 Ice viscosity in relation to fabrics
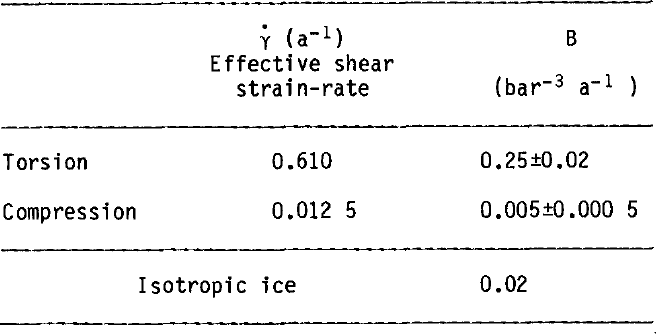
An ice sample with the fabric shown in Figure 2 was tested at first in torsion and then in compression with the apparatus described by Reference DuvalDuval(1976).

Fig.2. C-axis plot for the sample tested in torsion and compression.
The sample was cut so as to have the best orientation for basal glide in torsion (c-axis of crystals parallel to the axis of the cylinder) and, as a result, the least favourable orientation for basal glide in compression. The diameter of the sample was 90 mm and the length was 130 mm. Crystal size was around 6 mm. The effective shear stress was 1.35 × 105Pa for the two stress cases and the temperature was -7°C±0.l°C. Results are given in Table I. The effective shear strain-rates were calculated after 50 h. A very large variation in parameter B of Equation (1) was found. The strain-rate in torsion is 40 x the strain-rate in compression and about 10 x the minimum strain-rate calculated with isotropic ice. These results are in accordance with those of Reference LileLile(1978) for smaller shear stresses.However, this study gives a higher enhancement factor (strain-rate relative to that of the isotropic ice), probably induced by a more pronounced fabric.
Creep recovery tests were also performed to verify the influence of preferred orientation on the strain measured after unloading. Typical creep recovery curves are shown in Figure 3 in torsion and compression with the same sample discussed above. The loading time before unloading was always about 50 h. As for creep tests, recovery strain strongly depends on the orientation of the basal planes with respect to the planes of maximum shearing stress. The strain measured after unloading in torsion is about 20 x the expected elastic strain calculated with a shear modulus equal to 3xl09 Pa. These results bear out that recovery creep is produced by the glide of dislocations induced by internal stresses Reference Duval(Duval 1978).

Fig.3. Creep recovery curves after unloading in torsion and compression. Temperature is -7.2°C. The fabric of the studied sample is given in Figure 2.
TABLE I. EFFECTIVE SHEAR STRAIN-RATE y AND VALUES OF THE CONSTANT B (γ̇ = Bτ3) FOR ANISOTROPIC ICE. τ = 1.35xl05 Pa, T = -7°C±0.1°C. THE VALUE OF B FOR ISOTROPIC ICE IS ALSO GIVEN (FROM LE GAC UNPUBLISHED)
2. Creep Activation Energy
Mechanical creep tests were performed with the apparatus described by Duval(1977) in a cold room with the temperature varying between -30.2°C and -4.9°C. The maximum variation of the measured air temperature during a test was 0.2 deg.
Creep tests were performed in torsion on a sample from the Dome C ice core (depth: 155 m). Crystal size was about 6 mm2. It is important to note that at this depth the crystal size measured in the field was less than 2 mm2.
This result was discussed by Reference Vassoille, Mai, Perez, Tati bouet, Duval and MaccagnanVassoille and others (1980). No significant preferred orientation of c-axes was found. A constant torque was applied for this test. The shear stress calculated for the outer surface of the cylinder was about 2.8xl05 Pa. The first creep test was conducted at -7.2°C. A minimum creep rate was measured for a strain of 1%. No measurable variation of strain-rate was found over a time interval of about 24 h. Next, the cold-room temperature was changed. Creep rate for the new temperature was measured 24 h after the temperature change. The same strain-rate values were also found during the decrease and the increase of temperature.
Results are given in Table II. Below -7.2°C, the calculated apparent activation energy Q is 78±1 kJ mol−1. Above this temperature, the increase in Q always observed in polycrystals was noted. The activation energy derived by Reference Barnes, Tabor and WalkerBarnes and others (1971) below -8°C is similar to the value found in this study. It is higher than the value for self-diffusion found by Reference RamseierRamseier (1967). Taking Into account the temperature dependence of the elastic modulus, Reference Homer and GlenHomer and Glen (1978) calculated the corrected activation energy for polycrystalline ice and suggested that, except at very high temperatures, the rate limiting mechanism is that which controls the glide of dislocations across the basal plane.
From Reference DuvalDuval (1978) and Reference Le Gae, Duval and TrydeLe Gac and Duval (1980), the strain-rate limiting mechanism appears to be recovery processes. Consequently, the steady state strain-rate can be written
where r is the recovery rate and h the strain-hardening coefficient Reference Duval(Duval 1976).
The activation energy for creep depends mainly on the variation of the recovery rate with temperature. However, the temperature dependence of the strain-hardening coefficient cannot be neglected. From Reference Le GaeLe Gac (unpublished), the coefficient h for a natural ice sample from D 10 station, Antarctica, is 1.5xl09 Pa at -10°C and 2.1xl09 Pa at -20°C. Taking into account the variation of the strain-hardening coefficient with temperature, the activation energy for the recovery rate would be about 60 kJ mol-1. This value is near the one given by Reference RamseierRamseier (1967) for self-diffusion.
Work is now in progress to determine the recovery processes which should control strain-rate in polycrystalline ice when dislocation creep is the dominant mechanism.
TABLE II VARIATION WITH TEMPERATURE OF γ̇ AND Bτ = 2.8xl05 Pa

Acknowledgements
We thank A Chaillou for extensive technical assistance and the Centre National de la Recherche Scientifique for financial support. This work is a contribution to the International Antarctic Glaciological Project.







