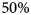Miniaturization of folded circular SIW cavity filters

Abstract

This contribution presents miniaturization techniques for folded circular substrate integrated waveguide (FCSIW) cavity filters. The cavities support dual-mode operation. Two of these cavities are combined to form a fourth-order filter. Two filter designs are implemented. The first one serves as a reference and exhibits good manufacturability. For the second one, the cavity is modified, which leads to $50\%$ more compactness. The designs are experimentally verified with 10 GHz bandpass filters. Even though the achieved level of compactness requires higher technological effort, the measurements validate the miniaturization concept. The out-of-band rejection exceeds 40 dB between 11.2 and 30 GHz for the reference filter and between 11.3 and 33 GHz for the miniaturized one. The implemented components are compared with state-of-the-art filters.

Introduction

Bandpass filters are essential components in many microwave systems. The ever increasing system complexity requires filters that are compact and show good electrical performance.

Substrate integrated waveguides (SIWs) [1, 2] have been the object of extensive research in the last few years. They exhibit excellent shielding properties and can be manufactured in conventional printed circuit board (PCB) technology. As standard SIWs are rather bulky, miniaturized variants have been proposed. Folded SIWs [3], which require a multilayer PCB stack, achieve up to $50\%$ surface reduction [4] and allow for compact filter designs [5]. Miniaturization can be pushed further by altering the cavity shape, as demonstrated with folded circular SIWs (FCSIWs) in [6, 7]. A subsystem based on such components has been successfully implemented in [8], while the authors in [9] address their application to higher-order filters. The multimode operation provides another – intrinsic – means to reduce the circuit size [10, 11]. Besides, loading of SIW cavities is an alternative miniaturization approach [12]. However, in contrast to folded SIWs, it impairs the shielding properties.

This work proposes miniaturization techniques for filters based on the FCSIW cavity introduced in [13]. Here, a compact 10 GHz filter with a large stopband is shown. The design relies on a novel type of FCSIW cavity. Compared to [6] and [7], it possesses a modified septum with a gap along the outer edge instead of the tip. This septum shape leads to a significantly reduced cavity size and a larger stopband. Further miniaturization is achieved by tuning a higher-order mode for dual-mode operation. The present work proposes yet another step to substantially improve compactness by reducing the cavity dimensions while preserving its resonant frequency. To demonstrate this miniaturization concept, a novel X-band filter is presented and compared with the reference design from [13] as well as to the state of the art.

The remainder of this paper is organized as follows. Section “Cavity properties” presents the geometry and analyzes the electrical properties of the FCSIW cavity. Section “Filter designs” describes the design, the optimization, and the realization of both filters. Additionally, it compares the implemented components with the state of the art. Section “Conclusion” concludes the paper.

Cavity properties

Figure 1 illustrates the proposed FCSIW cavity. The vias with diameter d and pitch p form a sector cylinder with angle α, radius R, and height 2 h. The permittivity of the substrate inside the cavity is $\epsilon _r$. A septum with a slot of width s and length x _s is positioned in the middle.

Fig. 1. Model of the FCSIW cavity with a slotted septum.

As R ≫ h, only modes that are transverse magnetic (TM) with respect to z need to be considered in the analysis. They are labeled TM_np0, with p = 1,  2,  3,  … being the radial order and n = 0,  1,  2,  … the azimuthal one. For n ≥ 1, the superscripts O and E distinguish between modes with a perfect electric conductor (PEC) and a perfect magnetic conductor (PMC) in the septum plane, respectively. Unless otherwise stated, it is assumed that $\epsilon _r = 3.62$, s ₀ = 0.35 mm, h = 0.3 mm, d = 0.4 mm, and p = 0.7 mm. The slot length x _s is initially set to 0 mm. The electrical field magnitude of the fundamental TM₀₁₀ mode and of the higher-order TM$_{110}^E$, TM$_{110}^O$, and TM₀₂₀ modes is shown in Fig. 2.

Fig. 2. Magnitude of the electrical field for (a) the TM₀₁₀, (b) the TM$_{110}^E$, (c) the TM$_{110}^O$, and (d) the TM₀₂₀ mode.

Figure 3(a) illustrates the resonant frequency of the fundamental TM₀₁₀ mode versus the angle α for different R. The resonant frequency increases for smaller angles. However, when changing the angle from 90 to 30°, i.e. reducing the surface area by a factor of three, the resonant frequency increases by only about $20\%$. Hence, the cavity can be significantly downsized without severely affecting the resonant frequency. The frequency shift can be compensated by increasing the cavity radius R.

Fig. 3. Resonant frequency versus angle α of (a) the fundamental TM₀₁₀ mode for different radii R (in mm) and (b) higher-order modes with R = 5.5 mm. An FCSIW cavity with b ₀/R = 0.45 is assumed.

Higher-order modes need to be considered prior to the filter design, since they may create parasitic passbands and impair the stopband characteristics. As depicted in Fig. 3(b), the resonances of the fundamental and higher-order modes are quite far apart for smaller angles. However, due to the pronounced angular dependence of the higher-order mode resonances, the mode separation is reduced as α increases.

Cavity miniaturization

As will be shown in this section, the cavity can be miniaturized by controlling the parameter b ₀ (see Fig. 1). As presented in Fig. 4, the resonant frequency of the fundamental TM₀₁₀ mode decreases as b ₀/R is reduced. This property enables to control the miniaturization of the FCSIW cavity.

Fig. 4. Resonant frequency of the fundamental TM₀₁₀ mode of an unloaded FCSIW cavity versus the ratio b ₀/R for different R (in mm) with α = 45°.

As an example, consider a design resonant frequency of f _res = 10 GHz. For a cavity with R = 6 mm, b ₀/R ≈ 0.45 has to be adjusted. Suppose b ₀/R is reduced to 0.35. To maintain the resonant frequency of 10 GHz, the radius needs to be reduced to R′ = 5 mm. Thus, the cavity area is miniaturized according to the scaling factor

(1)$$S = 1-\left({R'\over R} \right)^2,\; $$

where R is the radius of the initial cavity and R′ is the radius of the scaled one. For the above example, $S = 30\%$. Thus, a significant size reduction of the cavity is possible by reducing b ₀/R while preserving its resonant frequency. It is noted that this miniaturization is an inherent property of the FCSIW cavity. Scaling the area of conventional cavity types, e.g.rectangular SIW cavities always increases their resonant frequency.

However, cavity miniaturization is geometrically limited. For very small b ₀ values, the septum corners, marked with blue circles in Fig. 1, overlap, yielding completely different electromagnetic properties. Hence, the miniaturization needs an adjustment of technological parameters, i.e. the reduction of s ₀, d, and p. This, on the other hand, increases the sensitivity to manufacturing tolerances.

Dual-mode operation

Besides the geometrical means already addressed, the compactness of the cavity can be improved even further by shaping its mode spectrum. In the following, the tuning of a higher-order mode is discussed, which enables dual-mode operation.

Figure 5 illustrates the surface current flow on the septum for the four relevant modes. Along the edge around φ = α/2, the currents of the TM₀₁₀, TM$_{110}^O$, and TM₀₂₀ modes flow in radial direction and those of the TM$_{110}^E$ mode in azimuthal direction. Hence, a slot placed there (see Fig. 1) has almost no effect on the former modes, but strongly disturbs the latter one. This is confirmed in Fig. 6 which reports their resonant frequencies versus the slot length x _s. The slot lowers the resonant frequency of only the TM$_{110}^E$ mode, which, for x _s ≈ 3 mm, is about 10 GHz and thus very close to that of the TM₀₁₀ mode. Figure 7 illustrates this effect. The slot forces the current of the TM$_{110}^E$ mode around the slot, which causes the resonant frequency to decrease. This enables dual-mode operation and simultaneously keeps spurious modes almost 30 GHz away.

Fig. 5. Current density on the septum of (a) the TM₀₁₀, (b) the TM$_{110}^E$, (c) the TM$_{110}^O$, and (d) the TM₀₂₀ mode.

Fig. 6. Resonant frequencies of the first four modes and their dependence on the slot length x _s for an FCSIW cavity with R = 5.5 mm, α = 45°, b ₀/R = 0.45, and s = 0.2 mm.

Fig. 7. Magnitude of the electrical field of (a) the TM₀₁₀ and (b) the TM$_{110}^E$ mode of a slotted FCSIW cavity. Current density on the septum of a slotted FCSIW cavity of (c) the TM₀₁₀, and (d) the TM$_{110}^E$ mode.

Intracavity mode coupling

Next, the coupling between the TM₀₁₀ and the TM$_{110}^E$ modes within a cavity is analyzed. For this, the cavity is weakly coupled by a stripline at in- and output, as depicted in Fig. 8. The simulated transmission S ₂₁ (Fig. 9) shows two resonances, with f ₀₁ being the eigen-mode frequency of TM₀₁₀. To clarify, whether they result from mode-splitting, the eigen-frequencies of the cavity are determined. For this, the TM₀₁₀ (TM$_{110}^E$) mode must be excited by an even (odd) stimulus, as it satisfies the PMC (PEC) condition at the symmetry plane φ = α/2. According to [14], this translates into

(2)$$S_{11, e} = S_{11} + S_{21},\; $$

(3)$$S_{11, o} = S_{11}-S_{21},\; $$

where S _11,e and S _11,o are the even- and the odd-mode S-parameters, respectively. The associated group delays are shown in Fig. 9. Their maxima, which mark the eigen-frequencies of the cavity, coincide with the magnitude peaks of S ₂₁. Thus, no mode-splitting occurs and the modes are not coupled [14].

Fig. 8. Slotted FCSIW cavity coupled with a stripline at input (port 1) and output (port 2).

Fig. 9. S ₂₁ (blue line) and group delays of S _11,e (red, solid line) and S _11,o (red, dashed line) of a weakly coupled dual-mode cavity.

Input/output coupling

The in- and output coupling of the slotted dual-mode cavity is discussed in this section. The coupling is controlled by the position of the stripline. Figure 10(a) illustrates a loaded two-port cavity with R = 5.5 mm. The previous subsection demonstrated no coupling between the TM₀₁₀ and TM$_{110}^E$ modes within the cavity. Thus, the stripline at the input has to excite both modes and their external quality factors Q _e must be similar. The latter can be independently extracted by an even and an odd stimulus [14], respectively.

Fig. 10. Loaded slotted dual-mode FCSIW cavity with (a) R = 5.5 mm and (b) R = 4 mm.

Figures 11(a) and 11(b) show the dependencies for both modes as b ₀ varies. To match the resonant frequency of 10 GHz, the parameter b ₀ needs to be 2.25 mm, which corresponds to an external quality factor of Q _e ≈ 11.

Fig. 11. (a) External quality factor and (b) resonant frequency of the TM₀₁₀ and TM$_{110}^E$ modes of the loaded slotted cavity with R = 5.5 mm and x _s = 3.5 mm.

According to (1), the cavity is downsized by $S = 47\%$ as the radius reduces to R = 4 mm. Figure 12 reports the dependence of the cavity characteristics on b ₀. To achieve a resonant frequency of 10 GHz, b ₀ needs to be reduced to 1.7 mm, which also guarantees sufficient coupling. Thus, a filter based on a miniaturized cavity is feasible.

Fig. 12. (a) External quality factor and (b) resonant frequency of the TM₀₁₀ and TM$_{110}^E$ modes of the loaded slotted cavity with R = 4 mm and x _s = 3 mm.

The downside of this scaling is marked in Fig. 10(b) with a blue circle. Here, the gaps around the feeding stripline and the septum slot become very small, which increases the demands on the manufacturing process.

Filter designs

In this section, two FCSIW cavity filters are designed. The first filter from [13] serves as a reference filter. The second filter additionally relies on the miniaturization techniques from Section “Cavity miniaturization.” Both filters possess a fourth-order Chebyshev characteristic in the passband, a center frequency of f ₀ = 10 GHz, and a fractional bandwidth (FBW) of $10\%$. Figure 13(a) shows the corresponding layout. It consists of two symmetrically arranged dual-mode cavities with α = 45°.

Fig. 13. (a) Schematic of the proposed filter and (b) the PCB stack.

Corresponding to the analysis from Section “Input/output coupling,” the parameter b ₀ adjusts the in- and the output coupling, which is realized by a 50 Ω stripline. The interresonator coupling, which, among others, determines the filter bandwidth, is fine-tuned by b and b _v. As explained above, the center frequency is controlled by the radius R, the slot length x _s, and the length b ₀. The coupling topology is depicted in Fig. 14. The operating modes TM₀₁₀ and TM$_{110}^E$ of the same cavity are not coupled. The two dual-mode cavities thus lead to four transmission poles and two transmission zeros, which, due to symmetry, occur at the same frequency [15]. The transmission zeros result from the simultaneous excitation of the TM₀₁₀ and the TM$_{110}^E$ modes. Indeed, the electrical field of the former one points in the same direction at the input and the output, whereas it changes the sign for the latter (see Figs 7(a,b)). This leads to destructive interference [15, 16]. The final dimensions are obtained from optimization with CST Microwave Studio Filter Designer 3D.

Fig. 14. Coupling topology of the proposed filter with S and L representing source and load, respectively.

Both filters are manufactured on a multilayer PCB using Megtron 6 laminates from Panasonic with the stack shown in Fig. 13(b). The permittivity $\epsilon _r$ and the loss tangent tan (δ) are 3.62 and 0.005, respectively. Additionally, a hyper very low profile copper foil with a root mean square surface roughness of R _q = 0.5 μm is used. The minimum realizable feature size is ≈100 μm.

Reference filter

Figure 15 presents the manufactured reference filter [13]. For better manufacturability, the parameter b ₀ is kept ≈2 mm. The operating modes of the manufactured cavities have simulated unloaded quality factors between 130 and 160. Table 1 lists the filter dimensions.

Fig. 15. Manufactured reference filter with the septum contour marked in blue. The reference planes for TRL calibration are indicated with RP.

Table 1. Dimensions of the manufactured reference filter

A Through-Reflect-Line (TRL) calibration is performed at the reference planes indicated in Fig. 15. Figures 16 and 17 show the narrowband and the wideband simulation and measurement results, respectively. Except for a frequency shift of 70 MHz, they agree very well. In the passband, the measured insertion loss remains below 2.4 dB and the reflection coefficient below −18 dB. The out-of-band rejection exceeds 40 dB between 11.2 and 30 GHz.

Fig. 16. Simulated (dashed) and measured (solid) filter responses S ₁₁ (blue line) and S ₂₁ (red line) of the reference filter.

Fig. 17. Simulated (dashed) and measured (solid) filter responses S ₁₁ (blue line) and S ₂₁ (red line) of the reference filter.

The measured stopband response does not show the pronounced transmission zeros which are both located at 13.77 GHz in simulation. This is due to the limited internal isolation of the used network analyzer.

Miniaturized filter

The cavity of the miniaturized filter is designed as mentioned in Section “Cavity miniaturization.” The miniaturization goal is to achieve scaling of $S = 50\%$ with respect to the reference filter [13]. To implement the miniaturized filter, parameters {d,  s,  p,  s ₀} are reduced to {0.2,  0.12,  0.4,  0.215} mm. This reduces the unloaded quality factor of the operating cavity modes to values between 116 and 120.

The manufactured filter is depicted in Fig. 18. Table 2 lists its dimensions. Similarly to the measurements of the reference filter, a TRL calibration is performed at the indicated reference planes. Figures 19 and 20 show the narrowband and the wideband simulation and measurement results of the miniaturized filter, respectively. They confirm its functionality and feasibility. The reflection coefficient is better than −10 dB and the insertion loss remains below 3.4 dB. The loss increase stems from the reduced unloaded quality factor.

Fig. 18. Manufactured miniaturized filter with the septum contour marked in blue. The reference planes for TRL calibration are indicated with RP.

Fig. 19. Simulated (dashed) and measured (solid) filter responses S ₁₁ (blue line) and S ₂₁ (red line) of the miniaturized filter.

Fig. 20. Simulated (dashed) and measured (solid) filter responses S ₁₁ (blue line) and S ₂₁ (red line) of the miniaturized filter.

Table 2. Dimensions of the manufactured miniaturized filter

The degraded reflection coefficient results from the increased tolerance sensitivity. The passband is shifted by 270 MHz. The out-of-band rejection exceeds 40 dB between 11.3 and 33 GHz. Additionally, the parasitic passband around 36 GHz is less pronounced for the miniaturized filter with a minimum isolation of 17 dB, in contrast to 5 dB for the reference filter (see Fig. 17). The reason for the significantly improved out-of-band behavior is the reduced radius R since it shifts the higher-order modes to higher frequencies. In general, a larger deviation between simulation and measurement in comparison with the reference filter results is observed. This is due to the smaller feature sizes which are more prone to tolerance effects. As an example, Fig. 21 analyzes the sensitivity of the reflection coefficient on the slot width s. Changing the slot width by 20 μm yields a reflection coefficient of −15 and −10 dB for the reference and for the miniaturized filter, respectively.

Fig. 21. Simulated S ₁₁ of (a) the reference filter for s = 0.2 mm (blue) and s = 0.18 mm (red) and (b) the miniaturized filter for s = 0.12 mm (blue) and s = 0.1 mm (red).

Comparison with state of the art

Table 3 compares the presented filters with state-of-the-art SIW components, where the reference filter is labeled as F1 and the miniaturized one as F2. Here, λ ₀ is the substrate wavelength at center frequency f ₀. The last column reports the stopband normalized to f ₀. The proposed filters exhibit the best performance in terms of real estate and out-of-band rejection. Compared to F1 [13], F2 is $50\%$ more compact, albeit having the same passband properties and even a better stopband behavior.

Table 3. Comparison with state-of-the-art SIW filters

Conclusion

This work presents two X-band filters with a center frequency of 10 GHz. They are based on a novel FCSIW cavity, where a slot in the septum enables dual-mode operation. Two such dual-mode cavities are connected to form fourth-order filters. Two filters are designed, which both are more compact than state-of-the-art components, the second one being even $50\%$ more compact than the first one. This is accomplished by exploiting the particular properties of the FCSIW cavity, which allow it to significantly downsize its dimensions without increasing its resonant frequency. This greatly improves the compactness as also verified experimentally. Besides, both filters exhibit a large stopband. The filters are especially suited for systems requiring a high integration density in conjunction with multilayer PCBs.