No CrossRef data available.
Published online by Cambridge University Press: 18 April 2023
We give an example of a path-wise connected open set of  $C^{\infty }$ partially hyperbolic endomorphisms on the
$C^{\infty }$ partially hyperbolic endomorphisms on the 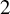 $2$-torus, on which the (unique) Sinai–Ruelle–Bowen (SRB) measure exists for each system and varies smoothly depending on the system, while the sign of its central Lyapunov exponent changes.
$2$-torus, on which the (unique) Sinai–Ruelle–Bowen (SRB) measure exists for each system and varies smoothly depending on the system, while the sign of its central Lyapunov exponent changes.