No CrossRef data available.
Article contents
Scale recurrence lemma and dimension formula for Cantor sets in the complex plane
Part of:
Smooth dynamical systems: general theory
Complex dynamical systems
Dynamical systems with hyperbolic behavior
Published online by Cambridge University Press: 25 March 2024
Abstract
We prove a multidimensional conformal version of the scale recurrence lemma of Moreira and Yoccoz [Stable intersections of regular Cantor sets with large Hausdorff dimensions. Ann. of Math. (2) 154(1) (2001), 45–96] for Cantor sets in the complex plane. We then use this new recurrence lemma, together with Moreira’s ideas in [Geometric properties of images of Cartesian products of regular Cantor sets by differentiable real maps. Math. Z. 303 (2023), 3], to prove that under the right hypothesis for the Cantor sets 
$K_1,\ldots ,K_n$ and the function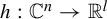 
$h:\mathbb {C}^{n}\to \mathbb {R}^{l}$, the following formula holds: 
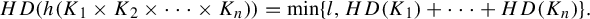$$ \begin{align*}HD(h(K_1\times K_2 \times \cdots\times K_n))=\min \{l,HD(K_1)+\cdots+HD(K_n)\}.\end{align*} $$
MSC classification
- Type
- Original Article
- Information
- Copyright
- © The Author(s), 2024. Published by Cambridge University Press
References
Araújo, H. and Moreira, C. G.. Stable intersections of conformal Cantor sets. Ergod. Th. & Dynam. Sys. 43 (2023), 1–49.10.1017/etds.2021.97CrossRefGoogle Scholar
Buzzard, G. T.. Infinitely many periodic attractors for holomorphic maps of 2 variables. Ann. of Math. (2) 145(2) (1997), 389–417.10.2307/2951819CrossRefGoogle Scholar
Hochman, M. and Shmerkin, P.. Local entropy averages and projections of fractal measure. Ann. of Math. (2) 175 (2012), 1001–1059.10.4007/annals.2012.175.3.1CrossRefGoogle Scholar
Lopez, J. E.. Stable projections of cartesian products of regular Cantor sets. PhD Thesis, Instituto Nacional de Matematica Pura e Aplicada, 2012.Google Scholar
Lowther, G.. Subadditivity of the
$n$
th root of the volume of
$r$
-neighborhoods of a set. Mathematics Stack Exchange. Available at: https://math.stackexchange.com/q/1651441 (version: 2016-02-12).Google Scholar
$n$
th root of the volume of
$r$
-neighborhoods of a set. Mathematics Stack Exchange. Available at: https://math.stackexchange.com/q/1651441 (version: 2016-02-12).Google ScholarMoreira, C.. Geometric properties of the Markov and Lagrange spectra. Ann. of Math. (2) 188 (2000), 145–170.Google Scholar
Moreira, C. and Yoccoz, J.-C.. Stable intersections of regular Cantor sets with large Hausdorff dimensions. Ann. of Math. (2) 154(1) (2001), 45–96.10.2307/3062110CrossRefGoogle Scholar
Moreira, C. G.. Geometric properties of images of Cartesian products of regular Cantor sets by differentiable real maps. Math. Z. 303 (2023), 3.10.1007/s00209-022-03151-zCrossRefGoogle Scholar
Moreira, C. G. and Yoccoz, J.-C.. Tangences homoclines stables pour des ensembles hyperboliques de grande dimension fractale. Ann. Sci. Éc. Norm. Supér. (4) 43(1) (2010), 1–68.10.24033/asens.2115CrossRefGoogle Scholar
Palis, J. and Takens, F.. Hyperbolicity and Sensitive Chaotic Dynamics at Homoclinic Bifurcations: Fractal Dimensions and Infinitely Many Attractors in Dynamics (Cambridge Studies in Advanced Mathematics, 35). Cambridge University Press, Cambridge, 1995.Google Scholar
Peres, Y. and Shmerkin, P.. Resonance between Cantor sets. Ergod. Th. & Dynam. Sys. 29(1) (2009), 201–221.10.1017/S0143385708000369CrossRefGoogle Scholar
Shmerkin, P.. Moreira’s theorem on the arithmetic sum of dynamically defined cantor sets. Preprint, 2008, arXiv:0807.3709.Google Scholar
Zamudio, A.. Complex Cantor sets: scale recurrence lemma and dimension formula. PhD Thesis, Instituto Nacional de Matematica Pura e Aplicada, 2017.Google Scholar