Comparing Facial 3D Analysis With DNA Testing to Determine Zygosities of Twins

Abstract

The aim of this study was to compare facial 3D analysis to DNA testing in twin zygosity determinations. Facial 3D images of 106 pairs of young adult Lithuanian twins were taken with a stereophotogrammetric device (3dMD, Atlanta, Georgia) and zygosity was determined according to similarity of facial form. Statistical pattern recognition methodology was used for classification. The results showed that in 75% to 90% of the cases, zygosity determinations were similar to DNA-based results. There were 81 different classification scenarios, including 3 groups, 3 features, 3 different scaling methods, and 3 threshold levels. It appeared that coincidence with 0.5 mm tolerance is the most suitable feature for classification. Also, leaving out scaling improves results in most cases. Scaling was expected to equalize the magnitude of differences and therefore lead to better recognition performance. Still, better classification features and a more effective scaling method or classification in different facial areas could further improve the results. In most of the cases, male pair zygosity recognition was at a higher level compared with females. Erroneously classified twin pairs appear to be obvious outliers in the sample. In particular, faces of young dizygotic (DZ) twins may be so similar that it is very hard to define a feature that would help classify the pair as DZ. Correspondingly, monozygotic (MZ) twins may have faces with quite different shapes. Such anomalous twin pairs are interesting exceptions, but they form a considerable portion in both zygosity groups.

Twin zygosity analyses are usually started immediately after the birth of twin babies by observing whether their faces are similar or not. Faces carry our main identification role, and there are many genes behind facial developmental processes during pre- and postnatal phases. Not only genes, but also epigenetic and environmental factors –– frequently called ‘functional matrix’ or ‘nurture’ –– have an influence on faces in the course of growth (Corruccini, 1999; Galton, 1875; Kempthorne & Osborne, 1961; Lundström, 1954). Modern DNA analyses are routine today and allow high-quality precision in zygosity determination even before birth (Levy et al., 2002). Postnatally, these tests are easier to do, but they can also be quite expensive if used for heritability determinations in a large group of twins (Becker et al., 1997; Forget-Dubois et al., 2003).

During recognition of zygosities, the result of DNA analysis may show the high-level identity of the target genes, but phenotypic concordance may be missing, that is, intra-pair appearances of the twins may not be similar. This may result from the fact that DNA analysis is based on a limited number of gene sequences, giving, however, high statistical significance to probability calculations. The types of genetic–epigenetic and prenatal environmental post-zygotic mechanisms that induce discordance within such twin pairs may vary from heterokaryotypia to skewed inactivation of X-chromosomes (Machin, 2009). Methylation of genes –– also other genes than X-chromosomal genes –– after conception may interfere with development of chorionity associated with the implantation site distance of two blastocysts, and diverse placental growth may cause vascular anastomoses and unbalanced nutrition of the fetuses through compensation mechanisms (Meulepas et al., 1988; Strong & Corney, 1967).

Because of the misconception that MZ twins are necessarily identical in phenotype, many MZ twin pairs are mistakenly designated as DZ if analysis is done without DNA testing. The DNA method may also indicate that a highly concordant pair of very young same sex twins thought to be MZ are in fact DZ, which is exhibited also phenotypically at a later age. Taken together, these processes can give rise to subgroups of twins who may influence estimates of heritability through inaccurate zygosity determination (Christian et al., 1974; Kempthorne & Osborne, 1961).

Twin zygosity determinations can be performed with anthropologic, serologic, and genetic markers. However, many methods are more than occasionally inefficient, often expensive, and sometimes inaccurate. Zygosity analyses may also be successfully done by using questionnaires and extended statistical proposals (Heath et al., 2003; Sarna & Kaprio, 1980) or by comparing extraordinary skills (Forrai & Gordos, 1983). Clinical benefits of accurate zygosity determination include correct solid organ transplantation matching if one twin requires a donation for a non-genetically mediated disease; the opportunity of preventive management for disorders that do not manifest synchronously; and better counseling for parents regarding their individually unique and often psychologically puzzling twin offspring.

More detailed zygosity testing may be required in twin pairs with complex and confusing biological origins. For example, intermediate trigametic and tetragametic chimeric DZ twins are reviewed, some of whom are, nevertheless, monochorionic (MC). Because of inter-fetal vascular anastomoses in MC twins, genetic results from blood samples may not accurately reflect discordance in solid organs. It has been proposed that familial MZ twinning is more common than suggested by the literature (Machin, 1990, 2009).

In shape observing methods (laser-scanning), three-dimensional (3D) analyses revealed differences in the facial shapes of MZ and DZ twins (Naini & Moss, 2004; Weinberg et al., 2013). The relative contributions of genetic and environmental factors were different for the upper, middle, and lower facial thirds, but zygosity did not seem to influence the amount of facial symmetry (Burke, 1989; Burke & Healy, 1993; Djordjevic et al., 2012). Facial shape analyses showed greater similarity in MZ twins, with the lower third being the least similar. The average faces of MZ and DZ males were coincident in the forehead, the supraorbital and infraorbital ridges, the bridge of the nose, and the lower lip. In MZ and DZ females, the eyes, supraorbital and infraorbital ridges, philtrum and lower part of the cheeks were coincident. The lower facial third was the most asymmetric. In other words, the relative contribution of genetic and environmental factors are different for the upper, middle, and lower facial thirds and in age and gender groups, not only in twins, but in all individuals (Djordjevic et al., 2013; Lundström & Mc William, 1987).

The aim of this study was to compare facial 3D zygosity analysis to DNA testing. Statistical comparisons of MZ and DZ twins based on 3D images has been done in previous studies (Djordjevic et al., 2012; Naini & Moss, 2004), but in this study, we particularly wanted to know how well it is possible to recognize zygosity from facial 3D data. To obtain the best possible result, we went through several different statistical approaches and software tools.

Material and Methods

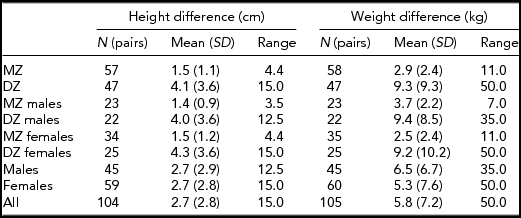
This study included 106 Lithuanian twin pairs of the same sex (46 male pairs and 60 female pairs). The twins were selected on the basis of their willingness to participate in the study from the Lithuanian University of Health Sciences Twin Centre. The exclusion criteria were: previous orthodontic treatment, permanent dental extractions, facial trauma, congenital disorders such as cleft lip and palate or other craniofacial deformity, and pregnancy. The faces of the subjects were recorded by a portable 3D surface-imaging system based on stereogrammetry technology (3dMD, Atlanta, USA) in Kaunas, Lithuania, in 2011–13. One male pair was excluded because of long beards, which caused distortion to the shape of the 3D image. The mean age of the twins was 20.5 years. Their mean height was 170.8 cm and their mean weight was 65.5 kg (Table 1). Table 2 includes means of height and weight differences separately for different groups divided on the basis of gender, zygosity or both. A blinded DNA test was done for each pair to determine their true zygosity. Gender recognition and gender differences among same- and opposite-sex twins were also tested, based on the facial appearance of the twin pairs, but these results will be given in a separate report.

TABLE 1 Characteristics of the Study Sample

TABLE 2 Mean Differences in Height and Weight Within Twin Pairs

Zygosity Determination using a DNA Test

An AmpFℓSTR®Identifiler® (Applied Biosystems, USA) polymerase chain reaction (PCR) method was used for comparison of genetic profiles, which ensures accurate and reliable zygosity determination. Presently, zygosity determination using this molecular genetic technique reaches 99.9% accuracy, facilitating specific DNA markers (D8S1179, D21S11, D7S820, CSF1PO, D3S1358, TH01, D13S317, D16S539, D2S1338, D19S433, vWA, TROX, D18S51, D5S818, FGA) and Amel fragment STRs (short tandem repeats).

Processing

During imaging, the participants were asked to sit on a chair and look at the eyes of their reflection in a mirror ahead to maintain a neutral facial expression. Any hair overlying the face was kept away by a hairband and a black scarf was placed on the shoulders to cover colorful clothes. Each image was processed so that distinct parts like hair were removed. The edges of the facial shell were removed so that the size of the shell was almost equal between twins. Still, the size of the shell varied between pairs. The position of the faces was standardized (Zhurov et al., 2010) by fitting them into the same reference frame, with the point halfway between the inner canthi of the eyes as the origin. A mirror shell of the original shell was created and the two shells were superimposed. The coronal plane (XY plane) was determined by the axis of the cylinder that fit all the data points of the original-mirror face structure. The sagittal plane (YZ plane) was chosen to pass through the middle of the face. It was defined as the symmetry plane of the original-mirror face structure. The transverse plane (XZ plane) was set to pass through the inner canthi of the eyes and was perpendicular to the two previous planes. Twenty-one facial landmarks (Farkas, 1994) were manually identified on each facial shell (Figure 1). Pose was standardized to facilitate landmark identification. The images were processed using 3dMD Patient and Rapidform2006 (INUS Technology, Inc., Seoul, Korea) software.

FIGURE 1 Twenty-one landmarks which were manually identified for each twin: (1) Glabella; (2) Nasion; (3) Endocanthion right; (4) Endocanthion left; (5) Exocanthion right; (6) Exocanthion left; (7) Palpebrale superius right; (8) Palpebrale superius left; (9) Palpebrale inferius right; (10) Palpebrale inferius left; (11) Pronasale; (12) Subnasale; (13) Alare right; (14) Alare left; (15) Labiale superius; (16) Labiale inferius; (17) Crista philtri right; (18) Crista philtri left; (19) Cheilion right; (20) Cheilion left; and (21) Pogonion.

Analysis

The analyses described below were carried out for three groups –– all the twin pairs as well as the male and female pairs separately. Two different scaling methods were used for each group. Landmark coordinates were translated so that their centroid lied at the origin. The Frobenius norm was used as a shape size metric (Dryden & Mardia, 1998):

\begin{equation*} ||A||_F = \sqrt {\mathop \sum \limits_{i = 1}^n \left[ {\left( {x_i - \bar x} \right)^2 + \left( {y_i - \bar y} \right)^2 + \left( {z_i - \bar z} \right)^2 } \right]} \end{equation*}

where the n × 3 matrix A is composed of the translated landmark coordinates, so that its first row contains the coordinates of the first translated landmark. Means $\bar x$ , $\bar y$ , and $\bar z$ are the averages of the coordinates and n is the number of coordinates, which is 21 in this study. The Frobenius norm of the translated coordinates was calculated for every face and then the average of the norms was computed for each group. In the first scaling method, each face was scaled by its own scaling parameter. The scaling parameter was obtained by dividing the group's average Frobenius norm by the individual's Frobenius norm. In the second scaling method each face was also scaled, but for each pair, the same scaling parameter based on the Frobenius norm of the other twin was used. The shape size metric Frobenius norm is often called the centroid size in the literature dealing with scaling (Dryden & Mardia, 1998). Analyses were also done without any scaling.

After scaling, the faces of a twin pair were superimposed using best-fit registration based on an iterative closest point algorithm. Then three feature statistics were computed to characterize the differences between the twins. These were the average distance (mm), the standard deviation of distances (mm), and the percentage coincidence of distances within 0.5 mm tolerance. Rapidform software provides ready-made tools for computation of these statistics (Djordjevic et al., 2012). The software first examines the overlap region between the two shells and then one of the shells is chosen as a source shell and the other is a target shell. Then, for each point in the source shell (vertex in a dense triangulation of the shell surface), the closest point in the target shell is computed. The average standard deviation, and 0.5 mm coincidence statistics are computed from these distances between the closest points in the two shells. Scaling, registration, and computation of the statistics have been automated with a set of in-house VBA (Visual Basic for Applications) subroutines developed for Rapidform, so there is no need to do every part of the analysis separately for every twin pair.

Next, the pairs were classified into MZ and DZ twins in each of the three groups separately. Statistical pattern recognition methodology was used for the classification (Holmström & Koistinen, 2010; Webb, 1999). For good results, the training set for a pattern classifier should preferably be as large as possible. However, one should not use all available data for both training and testing the classifier, because the training and testing data should be independent to avoid overly optimistic estimates of classifier performance. Leave-one-out cross-validation (LOOCV) was used to avoid this problem (Webb, 1999). In LOOCV, a single observation is used as testing data while the rest of the available observations serve as the training data. Thus, if the whole group considered consists of n twin pairs, the training set for recognition of the zygosity of a pair includes all other twin pairs, and its size therefore is n-1.

Two pattern recognition methods were tested. One was a quadratic classifier that assumes Gaussian class distributions (Holmström & Koistinen, 2010; Webb, 1999). It separates the objects into two classes with a quadratic surface determined by the estimated class probability density functions of the object features considered. Multiple features can be used simultaneously for classification. To construct a quadratic classifier, one must estimate class-specific mean vectors and covariance matrices and also define prior probabilities for the two classes. Sample mean vectors and sample covariance matrices of the training data were used as estimates. The value 0.5 was chosen as the prior probability for both classes. It turned out that including more than one feature simultaneously did not give better recognition results than using only one feature at a time. The probable reason for this is that the features correlated strongly with each other.

The other method tested is quite simple. It assigns objects to one of the two classes simply based on the central tendency of the difference feature statistics considered. In the end, such a simple method turned out to perform as well as the quadratic classifier in recognizing zygosity. The simple method is easy to describe and computationally less expensive than the quadratic classifier, and we therefore use it here as an example of a classification technique. Thus, for each statistic, its mean and median were first computed and then used as thresholds in classification. Based on the DNA test, there were 35 MZ and 25 DZ female twins, while for males the number of MZ and DZ twins was 23 and 22, respectively. For each statistic, ordered training data were divided into two groups in the proportions suggested by the DNA test, and the value separating the two groups was considered as the third classification threshold along with the mean and the median. Twins with average distance or standard deviation below a threshold were classified as MZ, while twins with a coincidence feature below the value were classified as DZ. Statistical classification was done using Matlab R2013b (MathWorks, Natick, Massachusetts).

Measurement Errors

The 3dMDface^™ system is verified to consistently record geometric accuracy of < 0.2 mm RMS (root mean square). It is not possible for a subject to have exactly the same neutral facial expression, should measurements be repeated. Most of the twins were imagined twice and 83 of them were chosen for testing reproducibility of the neutral expression. Only images that would have been accepted for 3D zygosity analysis were used in the test. Two images of each test subject were processed, then superimposed and finally the average distance of the faces was calculated exactly in the same way as it was done for the twin pairs in previous sections. The mean of the average distances and a 95% coincidence interval were calculated and the result was 0.17 ± 0.02 mm. For comparison, the means for MZ, DZ, and all the twins were 0.82 ± 0.06 mm, 1.62 ± 0.15 mm and, 1.18 ± 0.11 mm, respectively. Reproducibility of facial soft tissue landmarks has been studied by Toma et al. (2009). Accuracy of landmarks identification was reported to generally range from 0.39 to 1.49 mm.

Results

There were three twin groups, three scaling methods, three features to measure the difference between faces, and three types of thresholds to assign pairs to two classes. Consequently, there were 81 different classification scenarios altogether. The percentage of classification success was computed for every scenario, with the results of the DNA test regarded as giving the correct assignment. The success rates for classification are displayed in Table 3. Clearly, as far as scaling is considered, the first method performs worse than the two other approaches. The only exception is the female group with the standard deviation feature and the mean, or the DNA zygosity ratio, as the classification threshold. In the first scaling method, the level of size adjustment was determined separately for each face. This type of scaling probably changes the relative size of faces within a pair too much, and therefore makes classification more difficult. The results reported in Table 3 in fact suggest that no scaling generally is better than either type of scaling considered. In fact, the coincidence feature of unscaled faces performed best for nearly all the group-threshold combinations. One exception was the male group; using the average distance statistic and the DNA zygosity ratio threshold, it performed best with the second scaling method.

TABLE 3 Success Rates for 3D Zygosity Classification Compared to DNA Testing

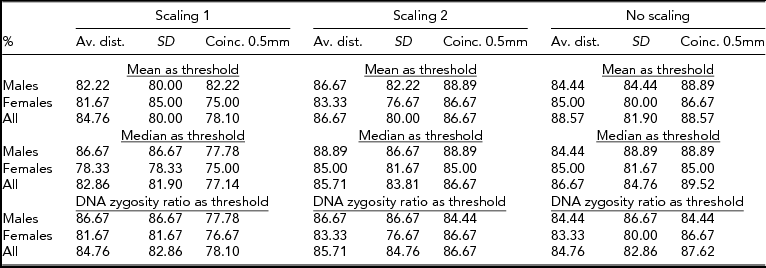
As for the three statistics tested, it appears that coincidence with 0.5 mm tolerance is a more suitable feature for classification than the average distance or the standard deviation. Also, as noted above, leaving out scaling improves the results in most cases. One might assume that, on average, larger faces have larger differences than smaller faces. Scaling was expected to equalize the magnitude of differences and therefore lead to better recognition performance. However, the two scaling techniques impaired twin recognition and it remains open, whether some other scaling method would work better. Another observation was that no particular classification threshold appeared to work better than the others.

There was one situation where the quadratic classifier outperformed all the other methods. This was the case for the female group with no scaling and the coincidence with 0.5 mm tolerance statistic. The success rate was 90%, which is over three percentage units greater than the best rates for the female group with any simple classifier.

Discussion

According to our results, relatively reliable zygosity recognition is possible even with a simple classifier. Almost all unscaled approaches as well as those based on the second scaling method achieved at least 80% success rates. Still, better feature statistics or more effective scaling methods could further improve the results. Also larger samples and more restricted age distributions would produce better outcomes. Examining erroneously classified twin pairs, we concluded that most of them could be regarded as outliers in the sample. Thus, it may be that the faces of DZ twins are so similar that it is very hard to define a feature that would help classify the pair as DZ. In Figure 2A, twins were classified as MZ by 3D analysis but the DNA test indicated that the pair was DZ. Young pairs who were DZ, according to the DNA test were misclassified more often than older twins. Young persons have rounder faces than adults. That is why they have less distinct features on their face and that makes their faces very similar.

FIGURE 2 (A) Female twin pair regarded as DZ by DNA, but MZ by 3D analysis. (B) Female twin pair regarded as MZ by DNA, but DZ by 3D analysis. (C) Male twin pair regarded as MZ by DNA and 3D analysis. (D) Male twin pair regarded as DZ by DNA and 3D analysis.

Correspondingly, MZ twins may have faces that differ substantially in shape. Figure 2B shows a misclassified MZ pair. As it can be seen, the twins look the same and the shapes of their faces are the same, but one's face is clearly smaller. Another misclassified MZ pair is shown in Figure 3. This is an interesting pair because when the mirrored face of the other twin was used in 3D analysis, the pair was classified as MZ, the same as in the DNA test. Such divergent twin pairs, as in the examples above, are interesting exceptions, but they form a considerable portion in both zygosity groups. There are also figures of correctly classified MZ and DZ twins (Figures 2C and D).

FIGURE 3 Female twin pair was MZ according to the DNA test, but DZ according to 3D analysis. When the other twin's face was mirrored in the 3D analysis, zygosity was classified as MZ.

Measurement errors were deemed to be minor. The error in both the 3D device and in repeating neutral expression was below 0.2 mm. Reproducibility in landmarking is weaker, but landmarking was used only for scaling in this study and the best classification result was obtained without scaling. Success rate results themselves indicate the complete error of the method.

Genetically, twinning has been considered a heritable trait and the genes involved may interfere with many facial traits, which is more common in twins than in singletons. Examples are asymmetry of the face (Beckwith–Wiedemann and Russel–Silver syndromes) and some oculo–vertebral spectrum abnormalities (Baynam et al., 2011). This spectrum includes conditions with phenotypic overlaps that predominantly are derivatives of first and second branchial arch developmental aberrations. These phenotypes are highly variable and those with most evident facial asymmetry are individuals with the hemifacial microsomia phenotype. This varied presentation is indicative of a causal heterogeneity, where a number of environmental, genetic, and multifactorial associations are reported (Hall, 2003). The incidence of multiple pregnancies is almost 10 times more common in association with oculo–vertebral syndromes than in controls (Lawson et al., 2002). There is an excess of such malformations in MZ twins and it has led to the conclusion of a common cause for MZ twinning and these aberrations (Schinzel et al., 1979). Facial asymmetry has been demonstrated in MZ twins with earlier 3D technology by Burke & Healy (1993).

Facial asymmetry may be of the fluctuating type, with random deviations in the symmetry of traits, or it may be of the directional type, where an anatomical feature is systematically greater on one side than the other (Peck et al., 1991; Van Valen, 1962). In MZ twins, some special types of asymmetry may also appear, such as a rare mirror-image type of asymmetry and functional laterality disturbance, which are of significant scientific interest. Some clinical reports provide evidence of an interwoven genesis of these traits (Annett, 2003; Thacker et al., 2009; Townsend et al., 1994). In our twins, an example of almost complete face mirroring came up in the MZ (DNA) group, but was recognized as DZ by 3D (Figure 3).

The difference between male and female twin pairs observed in this study is intriguing and likely due to the fact that males have more prominent, rectangular faces, whereas those of females are round with thicker soft tissue. Therefore, males have more distinct features than females and this can be seen in the results. In most of the cases (22 out of 27), male pairs achieved better success rates in classification than female pairs even though the male sample was smaller. This result will be discussed more thoroughly in a forthcoming paper on gender differences and opposite sex twins, who may have reduced dimorphism in various traits, due to suspected prenatal hormonal interference between fetuses (Heikkinen et al., 2013).

General knowledge of facial morphology –– its development and control mechanisms –– is very important. There is no doubt about the interaction of genes and environmental factors in the etiology of dentofacial proportions. We expect that the 3D method will provide new, interesting data on genetic and gestational influences on facial morphology. This research has given birth to new ideas and development of further studies. It is said that humans are the world's best pattern recognition machines, at least for now. So, it would be interesting to test how well people recognize zygosity from 3D images compared with the analysis of this study. It would be sensible also to compare different areas of faces separately (Djordjevic et al., 2012). Some areas might be more similar than others within MZ twins, and this might lead to better zygosity classification results. Also, examining different facial areas of MZ twins might explicate the effect of specific genes in different parts of the face.

Conclusion

Determining zygosity based on facial 3D data of the twins can be done with good results. In the best cases, 90% of twin pairs were classified as in a DNA test, which is a good rate in pattern recognition. 3D classification cannot compete with DNA testing in reliability, but it is a much faster and cheaper way to test zygosity. It cannot be used for exact zygosity analysis but it can be useful, for example, in approximating zygosities in a large set of twin data.