No CrossRef data available.
Article contents
The Formation of Galactic Halos in Universes Dominated by Cold Dark Matter
Published online by Cambridge University Press: 04 August 2017
Extract
If the universe is dominated by cold dark matter (CDM), had an inflationary phase, and has Ω = 1, then the spectrum of primordial density perturbations can be calculated. Using the spectrum calculated by J. Bardeen, we have found the structure of halos which form around peaks in the density. If the initial density is given by 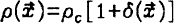 , where
, where 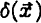 ) is a Gaussian process, then the mean run of density around a peak is proportional to the correlation function of
) is a Gaussian process, then the mean run of density around a peak is proportional to the correlation function of 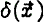 . If the mass about a peak comes to equilibrium in near-circular orbits, the exact equilibrium configuration can be calculated. The resulting rotation curve for a 1.5σ perturbation is shown as the curve HB in the figure below. As the baryon mass cools and condenses, it drags the CDM in with it, conserving the adiabatic invariants. Putting a 5×1010 M⊙ exponential disk and a 2×1010 M⊙ bulge at the center of the system, the rotation curve of the resulting compressed dark halo is given by the curve HA. D and B show the disk and bulge contributions, and T gives the net velocity. There are many arguments for wanting large galaxies to form from perturbations rarer than 1.5σ. Our spectrum was normalized by assuming that mass clusters as galaxies do on large scales; if Ω = 1, however, galaxies cannot trace the mass and the amplitude must decrease. The flatness of the rotation curve from the baryon-dominated to the CDM-dominated region comes about, in this case, from the imposition of the phenomenological baryon distribution, but the following argument indicates that the result is general. The ratio of baryonic to dark matter is ∼1/15; the rotation parameter Λ, the ratio of rotation velocity to halo dispersion, is also ∼1/15. When baryons fall though the CDM to the point at which they are rotationally supported, their density increases by (15)3. The CDM density increases by (15)2. Hence, baryon and CDM densities are comparable at the mean baryon radius.
. If the mass about a peak comes to equilibrium in near-circular orbits, the exact equilibrium configuration can be calculated. The resulting rotation curve for a 1.5σ perturbation is shown as the curve HB in the figure below. As the baryon mass cools and condenses, it drags the CDM in with it, conserving the adiabatic invariants. Putting a 5×1010 M⊙ exponential disk and a 2×1010 M⊙ bulge at the center of the system, the rotation curve of the resulting compressed dark halo is given by the curve HA. D and B show the disk and bulge contributions, and T gives the net velocity. There are many arguments for wanting large galaxies to form from perturbations rarer than 1.5σ. Our spectrum was normalized by assuming that mass clusters as galaxies do on large scales; if Ω = 1, however, galaxies cannot trace the mass and the amplitude must decrease. The flatness of the rotation curve from the baryon-dominated to the CDM-dominated region comes about, in this case, from the imposition of the phenomenological baryon distribution, but the following argument indicates that the result is general. The ratio of baryonic to dark matter is ∼1/15; the rotation parameter Λ, the ratio of rotation velocity to halo dispersion, is also ∼1/15. When baryons fall though the CDM to the point at which they are rotationally supported, their density increases by (15)3. The CDM density increases by (15)2. Hence, baryon and CDM densities are comparable at the mean baryon radius.
- Type
- Research Article
- Information
- Copyright
- Copyright © Reidel 1987


